Preprint
Article
Investigations of self-heating effects in Silicon-Photomultipliers
This is a preprint, it has not been peer-reviewed.
Submitted:
12 March 2024
Posted:
13 March 2024
You are already at the latest version
A peer-reviewed article of this preprint also exists.
Abstract
The main consequence of radiation damage on a Silicon-Photomultiplier (SiPM) is a significant increase in the dark current. If the SiPM is not adequately cooled, the power dissipation causes it to heat up, which in turn affects its performance based on the temperature. In order to investigate this heating effect, experiments were carried out using a KETEK SiPM glued on an Al2O3 substrate, as well as HPK SiPMs that were either glued onto an Al2O3 substrate or a flexible PCB. The assemblies were connected either directly to a temperature-controlled chuck on a probe station or through layers of materials with well-defined thermal resistance. A DC-mode LED was used to illuminate the SiPM, and the resulting SiPM current was measured to determine the steady-state temperature. This temperature depended on the power dissipated in the multiplication region of the SiPM and the thermal resistance. This information can be utilized to adjust the SiPMs working point, taking into account the effects of self-heating. Similarly, this approach can be applied to packaged SiPMs that have an unknown thermal contact to a heat sink.
Keywords:
-
1. Introduction
Silicon Photomultipliers (SiPMs) are the detector of choice for numerous applications in high-energy physics, astroparticle physics and space research, medical imaging, and societal applications. The operating conditions in these applications largely vary, e.g. temperatures can range from cryogenic [1] to 100 °C. Usually, the devices are operated in the dark, and visible photons are the signal to be detected, but in applications such as LiDAR systems (Light Detection and Ranging), SiPMs need to be operated in the presence of ambient light background [2,3]. At collider experiments, SiPMs are operated in a harsh radiation environment. The principal effect of radiation damage already at moderate fluences of cm−2 is an increase of dark current [4,5]. For the Phase 2 Upgrade the CMS collaboration at LHC plans two new detectors equipped with SiPMs [6]. In the High Granularity Calorimeter (HGCAL) plastic scintillator tiles with SiPM readout will equip the rear active layers which, during the detector lifetime will accumulate a 1 MeV neutron equivalent fluence cm−2 [7]. Even worse will be the operation condition in the barrel MIP Timing Detector (MTD) which will reach a fluence of cm−2 [8]. Understanding the SiPM’s response after radiation exposure is crucial for these applications. While the dark count rate (DCR) of non-irradiated SiPMs is typically in the range of 10-500 kHz/mm when operating in a dark environment at room temperature, it has been shown that the DCR increases by five orders of magnitude to above GHz/mm after a fluence cm−2 even with cooling to °C [8,9,10,11]. The same high (photo-)current is registered by SiPMs operated with background ambient light. These elevated currents result in significant power dissipation, potentially causing a rise in the SiPM temperature, an effect known as self-heating.
To maintain stable SiPM temperatures in such high dark count rate conditions, it is crucial to ensure a small thermal resistance between the SiPM and the heat sink [12]. Efficient heat dissipation is necessary to prevent self-heating and subsequent variations in the breakdown voltage of the SiPM, which changes its performance parameters such as gain and photon detection efficiency.
This research seeks to devise a method for gauging the temperature increase in SiPM caused by the dissipation of power in its amplification layer.
The SiPM’s performance depends on the temperature T. The photocurrent measured at a constant bias voltage and photon rate decreases with increasing temperature, expressed as , where is the photodetection efficiency, G is the gain, and is the excess charge factor [13]. The temperature sensitivity of the is introduced via the voltage-dependent Geiger-breakdown probability, , with the device fill factor, and the wavelength-dependent quantum efficiency. An increase of T reduces the carriers’ velocity due to increased scattering on the lattice. The ionization coefficients for holes and electrons decrease with T. Consequently, at a fix decreases, and the breakdown voltage, increases. An increase in with temperature leads to a decrease in gain, described by the relation: .
In the study by Lucchini et al. [14], a method is proposed to evaluate the heating of SiPMs in various package configurations and to evaluate changes in temperature during operation at elevated dark count rates. The SiPM current () is measured under constant illumination, and changes in are inferred from alterations in when the bias voltage is changed or the thermal conditions are changed by the air flow from a fan. This paper uses a similar approach for deducing temperature variations from current measurements. However, in our method, which we have introduced in [15], is measured in a cycle at a constant bias voltage while varying the intensity of light. This prevents abrupt variations in the SiPM working condition and parameters (G, , ). The primary assumption underlying our analysis is that under constant illumination is solely dependent on . As part of this study, we crosscheck that both methods, Lucchini and ours, measure a consistent temperature increase in the SiPM, independently if the power increase is induced by a light intensity or a bias voltage increase.
2. SiPMs investigated and experimental setup
Two types of SiPMs were investigated. First, an R&D SiPM from KETEK [16] type MP15V09, with at 25 °C [17], pixel size of 15 x 15 and number of pixels . The active region is circular with a radius . This SiPM is manufactured on a 700 thick silicon wafer and does not have a specially manufactured entrance window. The bare silicon sensor is glued on an alumina (Al2O3) substrate, with area 20 x 25 and thickness . The second sample is produced by Hamamatsu Photonics K.K. (HPK) [18] MPPC S14160-9769, with an active area of 3.0 x 3.0 pixel size 10 x 10 number of pixels: 89600 and at 25 °C. It is delivered as a surface-mount package with a silicon resin and glass-epoxy entrance window and has an overall thickness of 0.8 mm. This sample is tested both soldered on a flexible PCB, and on the same alumina as the KETEK sample to compare the different substrates. The information is summarised in Table 1.
The current of the samples, mounted on a temperature-controlled chuck ( ), is measured using probe needles connected to a Keithley 6517B voltage source/current meter [19]. The alumina or PCB substrate is fastened to the chuck using a vacuum pump. For good thermal contact, the substrate is placed directly on the surface of the chuck. To emulate a degraded thermal contact of the SiPM, two polyoxymethylene (POM) layers of different thicknesses (1.5 and 3.0 ±0.1 mm) are placed between the substrate and the cold chuck, increasing the thermal resistance. The thermal conductivity of POM is close to that of commercially available PCB materials. The thermal contact between POM and Al2O3/HPK-housing is simply via an air-gap and may not be optimal. Three temperature sensors (PT-100) glued with a thermal foil are used. Two sensors record the temperature on the surface of the substrate, and , and a third that of the chuck, , according to the sketch of Figure 1.
The illumination is provided with light of a wavelength from an LED operated in DC mode, and surrounded by a diffuser. The setup is located in a light-tight and electrically shielded box.
3. Method
Two sets of data are recorded. The calibration data set, with the substrate in direct contact with the chuck, is taken at several LED intensities, , with ranging from below to about 10 overvoltage (), and varied in five steps of °C centred at 25 °C and °C respectively. From the calibration data, the sensitivity function described in Section 4 is extracted. The sensitivity function is applied to the second set of data, the measurement cycle, used to determine the increase in the SiPM temperature from its measured current, in conditions with good and degraded thermal contact.
Figure 2 exemplary presents the measurement cycles performed on the KETEK SiPM in three different thermal contact configurations. is recorded at constant and , in three time intervals of 320 each. This time interval is sufficient for reaching thermal equilibrium. The light intensity is set to zero in the first and third intervals, and the dark current, , is measured. In the second interval, the light intensity is set via the current value , previously tuned to induce 25, 50, 75, and 100 of dissipated power in the SiPMs. These particular values of dissipated powers are chosen for this study to mimic a non-irradiated SiPM the power observed after neutron irradiation of the HPK SiPM to a fluence cm−2 operated at °C and 2 V overvoltage. The I-V curves used for this consideration are shown in Figure 3. Compared to a fresh SiPM, the irradiated HPK SiPMs demonstrate an increase in the dark current after irradiation by a factor at the excess bias voltage = 2 V. The same photo-current is induced on a fresh KETEK SiPM by illumination with LED light adjusted to = 0.47 mA, and operating the SiPM at = 10 V. Other combinations of and would also be possible to induce the same power.
The photo-current is defined as .
4. Analysis
From the calibration data, , the photo-current sensitivity is obtained using the formula:
for different values. The sensitivity gives the relative change of for a temperature change of 1 . In Eq. 1 it is assumed that the sensitivity for a given photon flux only depends on the excess voltage . The sensitivity curves obtained from the calibration data for various LED currents as a function of are shown in Figure 4 for KETEK (left) and for HPK (right). The HPK measurements were taken at temperatures of 25 °C and °C. In the range of interest of the measurements, the sensitivity is between 0.004 and K−1. As expected, increases with for a constant , since the current is proportional to the rate of converted photons producing a Geiger discharge () and the , [13]. But it can be seen that does not depend on . Effects of heating and occupancy can be ignored when extracting this calibration function. The T-dependence of the breakdown voltage is derived from the calibration data recorded at different , yielding to the values presented in Table 1.
Once the sensitivity curve is known, the change in SiPM temperature during a measurement cycle (or during operation in an experiment) is calculated from the relative change in photo-current, divided by the sensitivity:
where , is defined as the (time-dependent) difference of the photo-current to the first measured value after the LED is switched on. If the time is sufficiently close to the time of the LED switching, it is assumed that the temperature before and after the switch is still the same. This can be used to impose continuity constraints on the measured temperature profile during a scan. In Figure 5 the temperature changes induced on the SiPM after switching on the LED in the second interval of the cycles reported in Figure 2 are calculated using Eq. 2. The first points of the blue curve show that the main change is in the first step, therefore the assumption of no T change for the first point after the switching on the LED is not completely fulfilled. This may induce the largest error in the calibration of the temperature increase. The temperature of SiPM mounted directly on the alumina increases only by half a degree, while the SiPMs isolated via POM experience an increase of 3-4 K, depending on the thickness of the substrate.
The cycle presented in Figure 2 assumes that the SiPM parameters at fixed bias voltage are not instantaneously varied by the increase of light intensity in the second step (B). To evaluate the difference between our assumption and the one applied by Lucchini et al. [14], we performed a second cycle scan, depicted in Figure 6, where the LED intensity is kept fixed and the SiPM bias voltage is changed from a value below to above breakdown, to generate in step B exactly the same power as in the previous cycle.
The the temperature changes induced on the SiPM in step B of both cycles shown in Figure 6, are compared in Figure 7. The values obtained and the temperature gradients are the same for both scans, indicating that the change in SiPM parameters from below to above breakdown do not influence significantly the measurements of self-heating.
In the following section values for long enough times are quoted for which the current has reached a steady state after the switch.
5. Determination of Self-Heating
The procedure described in Section 3 and the analysis of Section 4 are applied to investigate the two SiPMs under study, described in Table 1. Four LED light intensities are chosen to study the temperature increase of the SiPMs in the relevant power range in the dark expected after irradiation. The value of 50 is marked as a reference in the plots, which corresponds to the power of a HPK SiPM irradiated to a fluence = 1 × 1013 cm−2 and operated at 2 and °C.
As expected, is proportional to the power, as demonstrated in Figure 8, for measurements performed at °C.
The best thermal contact is obtained for the SiPM mounted on the alumina substrate and directly placed on the chuck. In this configuration, the increase in temperature calculated using Eq. 2 is . The thick POM layer degrades the thermal contact of the SiPM on the alumina substrate to the same level as the flexible PCB. For both configurations . This demonstrates the significant influence of the thermal contact on the self-heating of SiPMs, the better the thermal contact the lower the increase on .
Figure 9 compares the results for the two SiPM types investigated: At best thermal contact the minimum temperature increase by self-heating is measured for the KETEK sample, . This value is considerably lower than that obtained for the HPK sample tested in the same condition. This may be attributed to the thermal property of the HPK package, which has a higher thermal resistance than the bare thermal contact compared to the bare KETEK silicon sensor. The same is observed when comparing the temperature increase of HPK on PCB with that of KETEK on alumina plus thick POM layer, which according to the result shown in Figure 8 should lead to the same thermal increase. The increase of is not scaling linearly with the thickness of POM indicating that maybe the thermal coupling via air gap is not ideal or that a more complex volume effect has to be considered. To confirm this effect, detailed 3D finite element simulations of the experimental setup are ultimately required, including the correct boundary conditions.
Table 2 summarizes the values at 50 with the corresponding breakdown voltage increase, , which is proportional to the increase in temperature and is determined using the reported in Table 1.
Measurements taken at 25 °C and at °C with the HPK sample soldered on PCB are compared in Figure 10, and analysed using Eq. 2. The results are reported in Figure 11.
The values for 50 are summarised in Table 3. The induced temperature increase by self-heating at °C is slightly higher than at 25 °C. While the relative change in current is the same at high and low temperatures, the sensitivity is at °C () is lower than at 25 °C ().
6. Conclusions
A method is developed to determine the self-heating of a SiPM from the measurement of its photo-current. The method is tested as a function of induced power, thermal contact, and two different substrates on two SiPM models with different packages. Self-heating causes an increase in breakdown voltage and a decrease in SiPM photo-current operated at fixed bias voltage. In these measurements, the SiPM is illuminated by a blue LED, and its current transients are measured at constant bias voltage when changing the LED current.
The developed method is used to determine the temperature increase for a SiPM with dissipated power around 50 , which is the measured power of an HPK SiPM (MPPC S14160-976X) irradiated to a fluence = 1 × 1013 cm−2 and operated at 2 above breakdown voltage at °C, taken as reference. For different thermal resistances between the HPK SiPM and the chuck, the following results are obtained: for ideal thermal contact (SiPM on Al2O3 substrate) an increase of between and is observed depending on the SiPM package; for realistic thermal contact (SiPM mounted on a PCB), the temperature is larger and ranges between 2 - 3 , corresponding to an increase in of 50 to 100 .
These results demonstrate the importance of a proper investigation of the thermal properties of SiPM package, substrate, and thermal connection to a cooling system when planning to operate at high (dark or photo-) currents. Also, the method proposed in this paper can be used to apply adjustments on the operation voltage depending on the value of the SiPM current during operation.
Author Contributions
Conceptualization and resources, E. Garutti; methodology, supervision, review and editing, E. Garutti and J. Schwandt; software, setup development, S. Martens.; data curation, formal analysis, original draft preparation, writing, C. Villalba; All authors have read and agreed to the published version of the manuscript.
Funding
We acknowledge the support from BMBF via the High-D consortium. This work is supported by the Deutsche Forschungsgemeinschaft (DFG, German Research Foundation) under Germany’s Excellence Strategy, EXC 2121, Quantum Universe (390833306).
Data Availability Statement
Data will be made available on request.
Acknowledgments
We thank Robert Klanner and Elena Popova for the fruitful discussion on the topic of self-heating of SiPMs. Robert Klanner developed the fundamental approach for conducting the measurements and defining sensitivity.
Conflicts of Interest
The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.
References
- Kochanek, I. Packaging strategies for large SiPM-based cryogenic photo-detectors. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment 2020, 980, 164487. [Google Scholar] [CrossRef]
- Agishev, R.; Comerón, A.; Bach, J.; Rodriguez, A.; Sicard, M.; Riu, J.; Royo, S. Lidar with SiPM: Some capabilities and limitations in real environment. Optics & Laser Technology 2013, 49, 86–90. [Google Scholar] [CrossRef]
- Riu, J.; Sicard, M.; Royo, S.; Comerón, A. Silicon photomultiplier detector for atmospheric lidar applications. Optics Letters 2012, 37, 1229–1231. [Google Scholar] [CrossRef] [PubMed]
- Garutti, E.; Musienko, Y. Radiation damage of SiPMs. Nucl. Instrum. Methods Phys. Res. A 2019, 926, 69–84. [Google Scholar] [CrossRef]
- Heering, A.; Musienko, Y.; Ruchti, R.; Wayne, M.; Karneyeu, A.; Postoev, V. Effects of very high radiation on SiPMs. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment 2016, 824, 111–114, Frontier Detectors for Frontier Physics: Proceedings of the 13th Pisa Meeting on Advanced Detectors. [Google Scholar] [CrossRef]
- Contardo, D.; Klute, M.; Mans, J.; Silvestris, L.; Butler, J. Technical Proposal for the Phase-II Upgrade of the CMS Detector. Technical report, Geneva, 2015. [CrossRef]
- CMS Collaboration. The Phase-2 Upgrade of the CMS Endcap Calorimeter. Technical report, CERN, Geneva, 2017. [CrossRef]
- CMS Collaboration. A MIP Timing Detector for the CMS Phase-2 Upgrade. Technical report, Geneva, 2019.
- Garutti, E.; Klanner, R.; Lomidze, D.; Schwandt, J.; Zvolsky, M. Characterisation of highly radiation-damaged SiPMs using current measurements. arXiv 2017, arXiv:1709.05226. [Google Scholar]
- Cerioli, S.; Garutti, E.; Klanner, R.; Martens, S.; Schwandt, J.; Zvolsky, M. Analysis methods for highly radiation-damaged SiPMs. Nucl. Instrum. Meth. A 2020, 958, 162729. [Google Scholar] [CrossRef]
- Musienko, Y.; Heering, A.; Ruchti, R.; Wayne, M.; Andreev, Y.; Karneyeu, A.; Postoev, V. Radiation damage of prototype SiPMs for the CMS HCAL Barrel phase I upgrade. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment 2018, 912, 359–362, New Developments In Photodetection 2017. [Google Scholar] [CrossRef]
- Bornheim, A.; et al. Integration of thermo-electric coolers into the CMS MTD SiPM arrays for operation under high neutron fluence. JINST 2023, 18, P08020. [Google Scholar] [CrossRef]
- Klanner, R. Characterisation of SiPMs. Nucl. Instrum. Methods Phys. Res. A 2019, 926, 36–56. [Google Scholar] [CrossRef]
- Lucchini, M.; Musienko, Y.; Heering, A. Experimental method to monitor temperature stability of SiPMs operating in conditions of extremely high dark count rate. Nucl. Instrum. Methods Phys. Res. A 2020, 977, 164300. [Google Scholar] [CrossRef]
- Garutti, E.; Klanner, R.; Popova, E.; Martens, S.; Schwandt, J.; Villalba, C. Self-heating effect in Silicon-Photomultipliers. Nucl. Instrum. Methods Phys. Res. A 2022, 1039, 167026. [Google Scholar] [CrossRef]
- KETEK GmbH. Available online: https://www.ketek.net/ (accessed on 22 January 2024).
- Chmill, V.; Garutti, E.; Klanner, R.; Nitschke, M.; Schwandt, J. Study of the breakdown voltage of SiPMs. Nucl. Instrum. Meth. A 2017, 845, 56–59. [Google Scholar] [CrossRef]
- Hamamatsu Photonics K.K. Available online: https://www.hamamatsu.com (accessed on 22 January 2024).
- Keithley 6517B electrometer. Available online: https://www.tek.com/de/manual/specialty-instruments/keithley-high-resistance-low-current-electr-0-keithley-high-resistance-low-current-electrom (accessed on 22 January 2024).
Figure 1.
Photos and schematic of the experimental setup. Layout on the probe station: KETEK SiPM glued on the alumina substrate, with a POM layer to degrade the thermal contact with the chuck. The distances of the on the alumina from the SiPM center are () and (). The alumina substrate is depicted on the right, on the left is the PCB substrate.
Figure 1.
Photos and schematic of the experimental setup. Layout on the probe station: KETEK SiPM glued on the alumina substrate, with a POM layer to degrade the thermal contact with the chuck. The distances of the on the alumina from the SiPM center are () and (). The alumina substrate is depicted on the right, on the left is the PCB substrate.
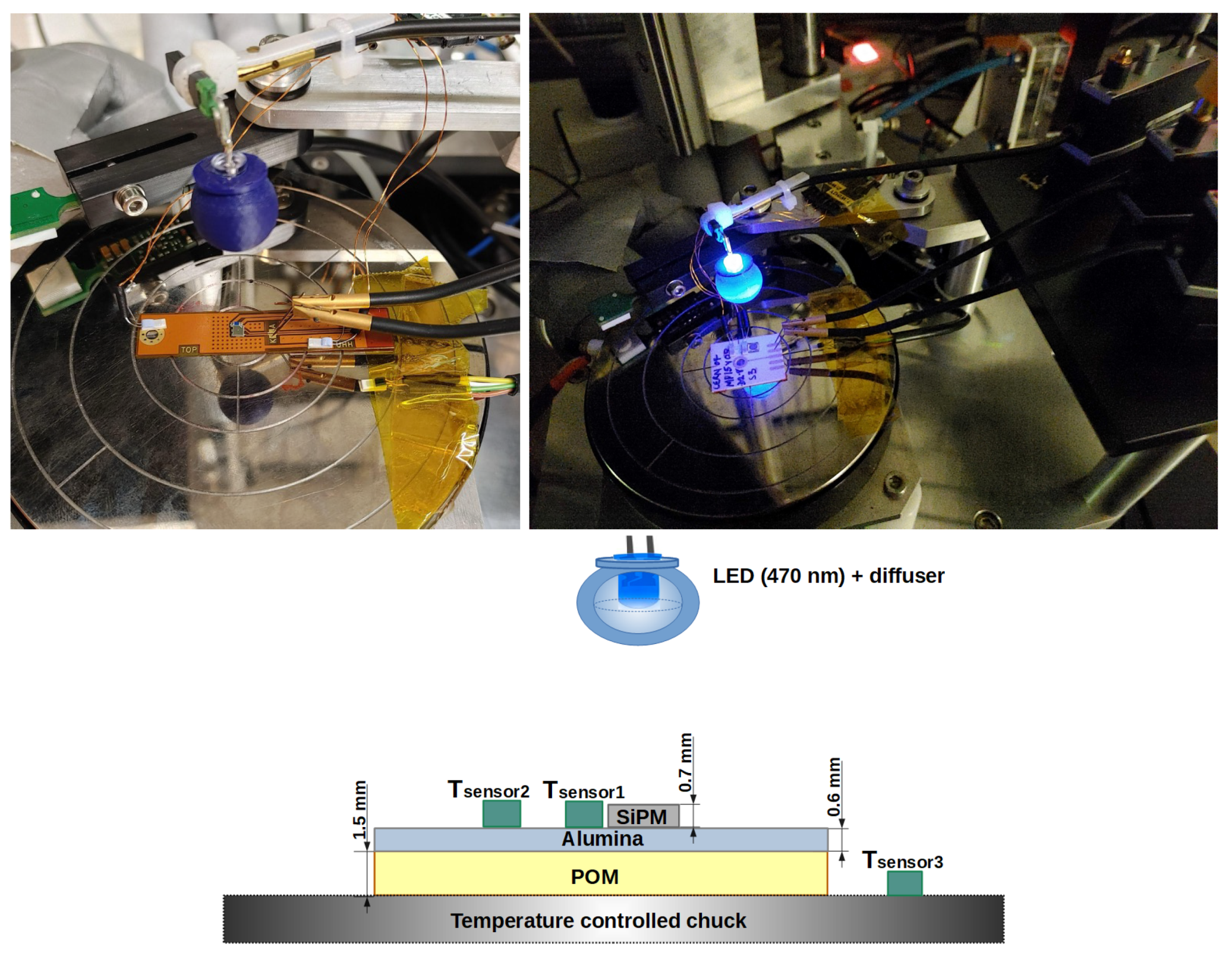
Figure 2.
Example of three measurement scan cycles for the KETEK SiPM mounted on alumina (blue) with two POM spacers thicknesses. The temperature change of sensor 1 is plotted versus time (top) and compared to the SiPM current measurement (bottom). The current of the LED is set to zero in the first and third intervals of the cycle. In the second interval, it is switched to a fixed value kept constant for the three cycles. Measurements are taken at 25 °C.
Figure 2.
Example of three measurement scan cycles for the KETEK SiPM mounted on alumina (blue) with two POM spacers thicknesses. The temperature change of sensor 1 is plotted versus time (top) and compared to the SiPM current measurement (bottom). The current of the LED is set to zero in the first and third intervals of the cycle. In the second interval, it is switched to a fixed value kept constant for the three cycles. Measurements are taken at 25 °C.
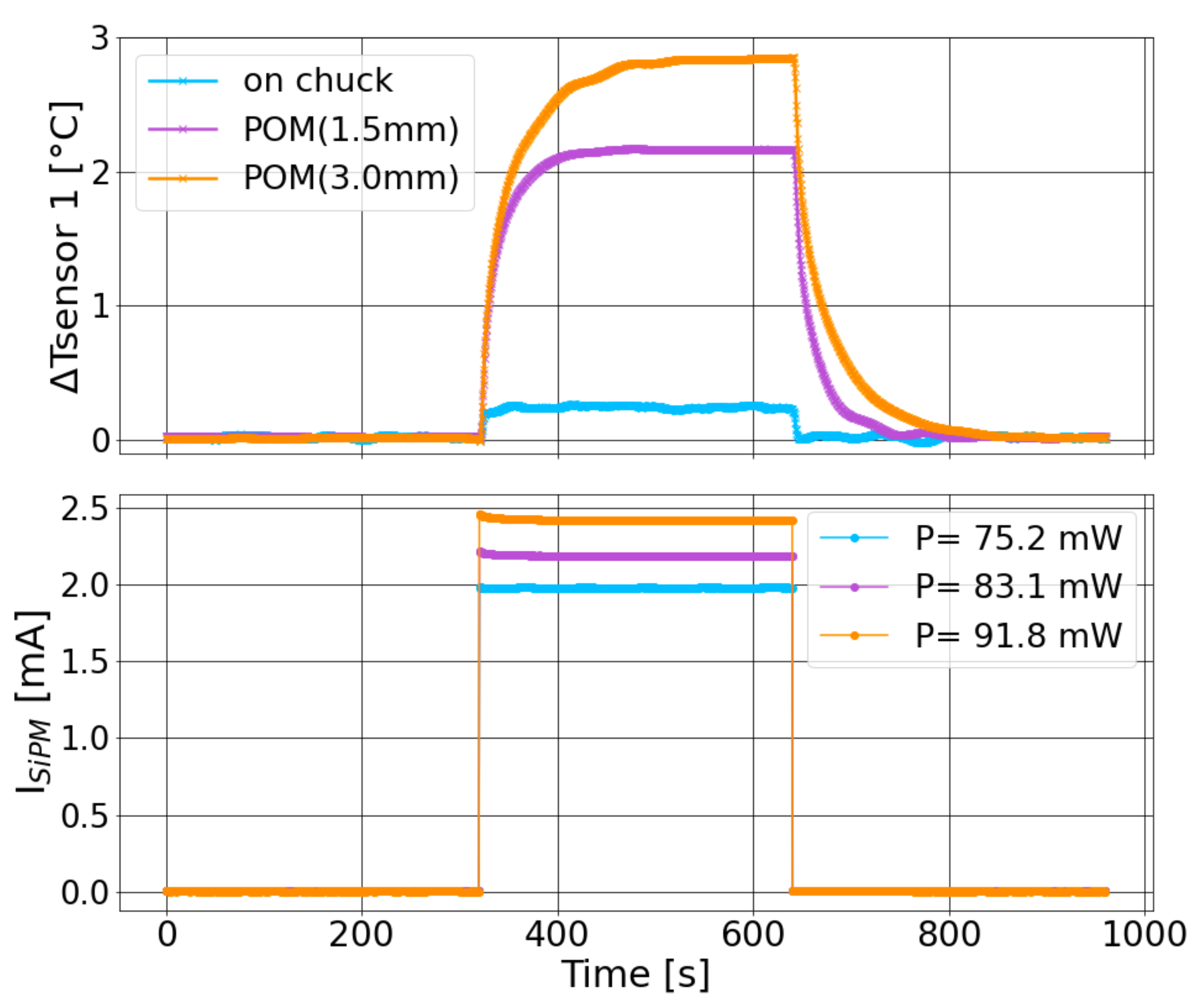
Figure 3.
Dark current for a fresh HPK SiPM (black) and the same SiPM irradiated with neutrons at a fluence cm−2 (green), compared to the photocurrent of a fresh KETEK SiPM exposed to LED light. The light intensity is tuned to reach the same range as the irradiated SiPM at an excess bias voltage = 2 V.
Figure 3.
Dark current for a fresh HPK SiPM (black) and the same SiPM irradiated with neutrons at a fluence cm−2 (green), compared to the photocurrent of a fresh KETEK SiPM exposed to LED light. The light intensity is tuned to reach the same range as the irradiated SiPM at an excess bias voltage = 2 V.
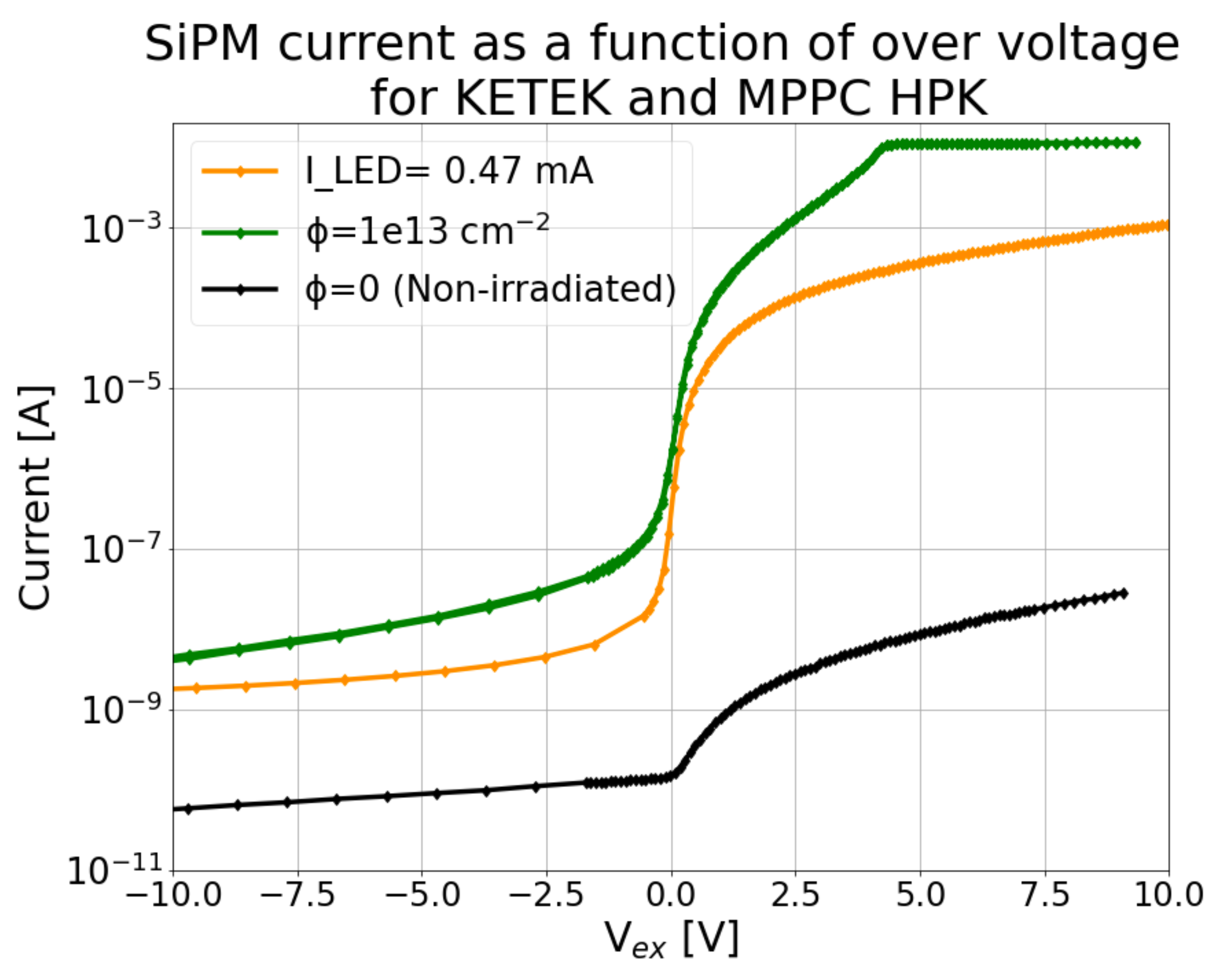
Figure 4.
Sensitivity curve at several LED currents for the KETEK sample at 25 °C (left), and for the HPK sample (right). For HPK data with red (blue) symbols are taken at 25 °C ( °C). The dashed lines indicate the overvoltages at which the measurement cycles presented in Section 5 are taken: (KETEK), (HPK, 25 °C), and (HPK, °C). At the bottom the ratio of sensitivity to the one for the largest are shown.
Figure 4.
Sensitivity curve at several LED currents for the KETEK sample at 25 °C (left), and for the HPK sample (right). For HPK data with red (blue) symbols are taken at 25 °C ( °C). The dashed lines indicate the overvoltages at which the measurement cycles presented in Section 5 are taken: (KETEK), (HPK, 25 °C), and (HPK, °C). At the bottom the ratio of sensitivity to the one for the largest are shown.
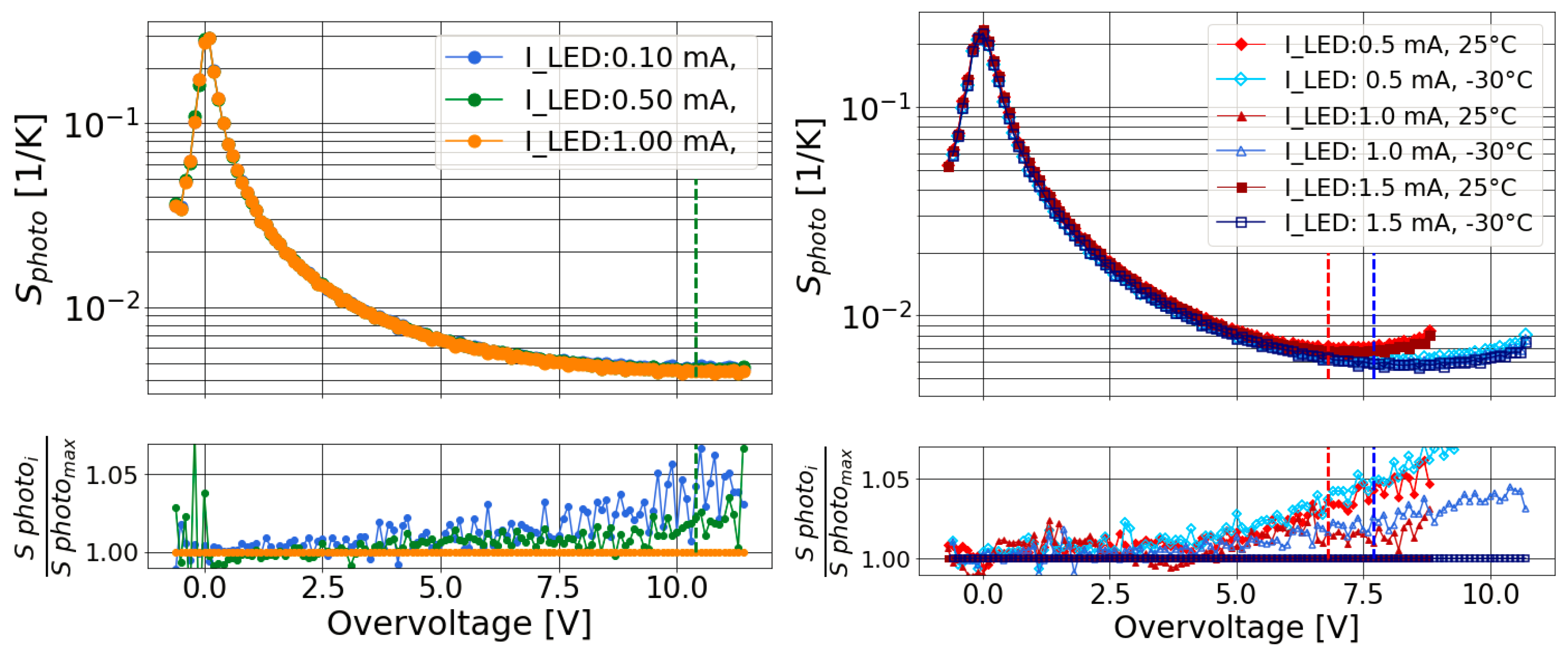
Figure 5.
Top) Current of the KETEK SiPM measured at 25 °C in the central interval of the cycles of Figure 2, normalized to the first measurement after the LED switch. The LED light intensity is fixed for the three cycles. The SiPM mounted on alumina thermalizes with a fast time constant, while the SiPM isolated via POM presents a longer thermalization constant. Bottom) Temperature change induced on the SiPM after switching on the LED, calculated using Eq. 2.
Figure 5.
Top) Current of the KETEK SiPM measured at 25 °C in the central interval of the cycles of Figure 2, normalized to the first measurement after the LED switch. The LED light intensity is fixed for the three cycles. The SiPM mounted on alumina thermalizes with a fast time constant, while the SiPM isolated via POM presents a longer thermalization constant. Bottom) Temperature change induced on the SiPM after switching on the LED, calculated using Eq. 2.
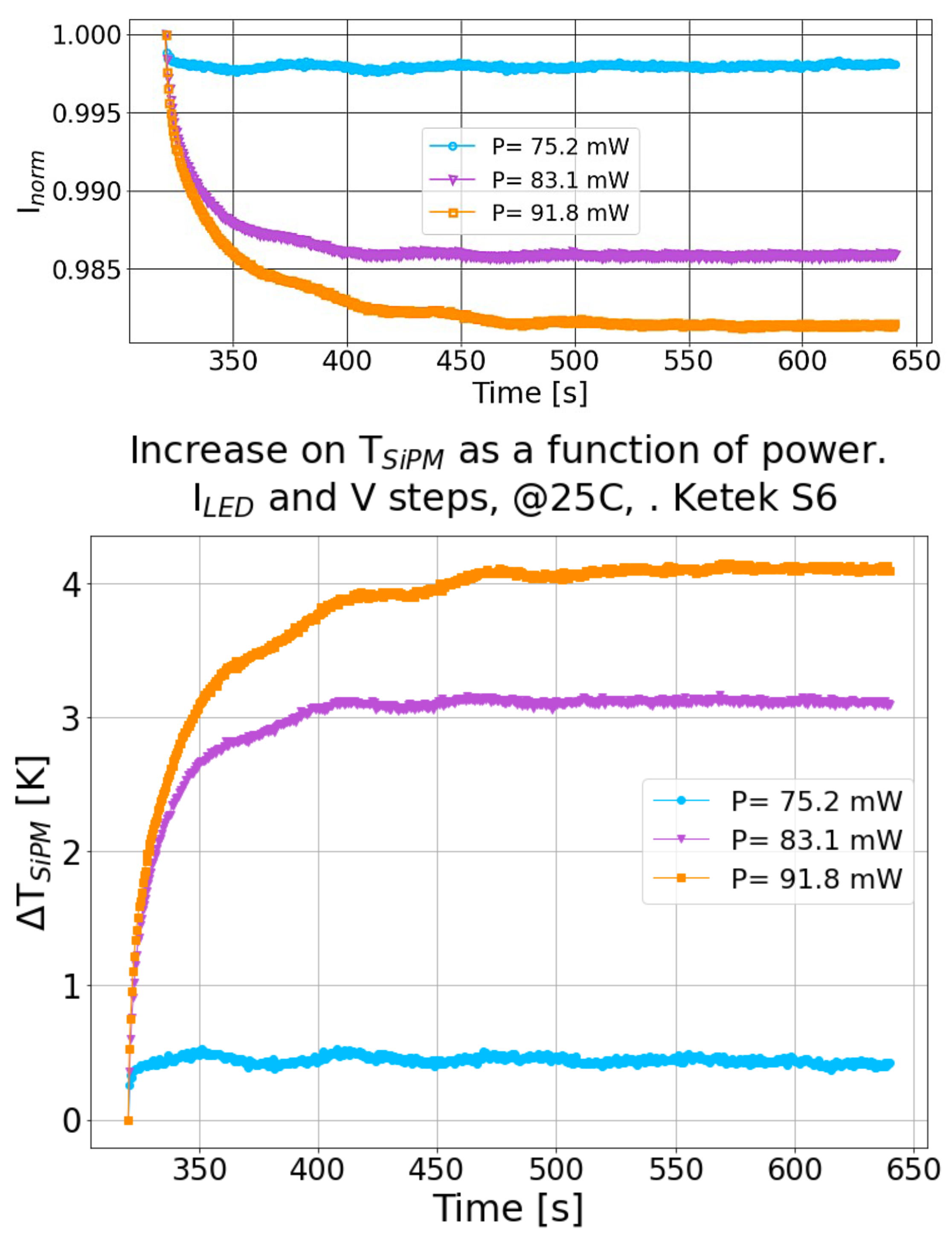
Figure 6.
Schematic of the two type of cyles performed to to obtained the results presented in Figure 7. Left) The LED current is stepped from zero to a tuned value and back, while keeping the SiPM bias voltage fixed. Right) The SiPM bias voltage is stepped from 2 V below breakdown to a tuned value and back, while keeping the LED current fixed. The tuned values are such that in step B the power on the SiPM is equal in both cycles.
Figure 6.
Schematic of the two type of cyles performed to to obtained the results presented in Figure 7. Left) The LED current is stepped from zero to a tuned value and back, while keeping the SiPM bias voltage fixed. Right) The SiPM bias voltage is stepped from 2 V below breakdown to a tuned value and back, while keeping the LED current fixed. The tuned values are such that in step B the power on the SiPM is equal in both cycles.
Figure 7.
Temperature change induced on the SiPM in step B of the two cycles discussed in Figure 6.
Figure 7.
Temperature change induced on the SiPM in step B of the two cycles discussed in Figure 6.
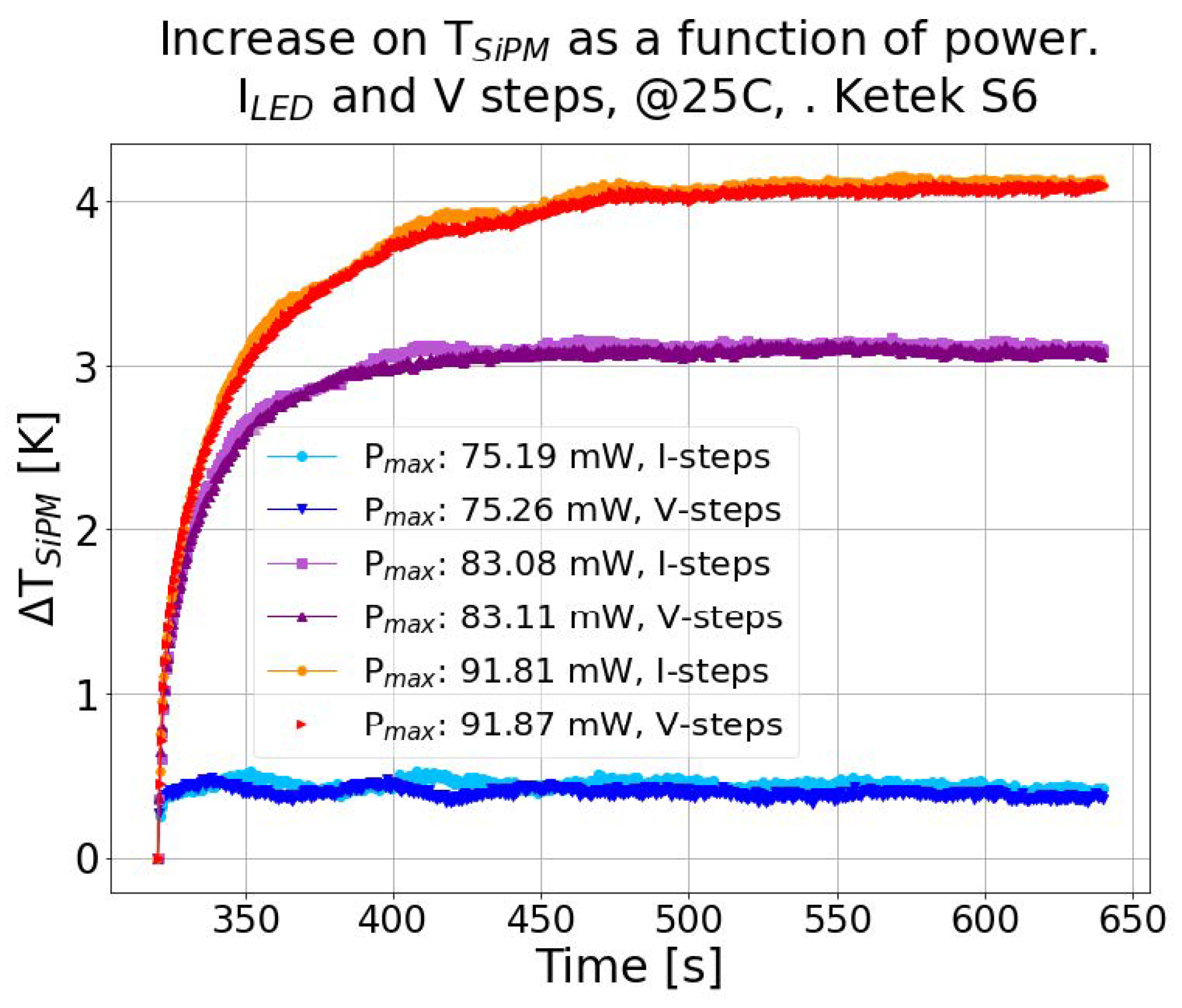
Figure 8.
Temperature increase by self-heating as a function of the power dissipated in the SiPM. Best thermal contact is obtained with alumina substrate directly on the chuck (green squares). The thermal contact of the flexible PCB (red circles) shows the same performance as the alumina substrate with thick POM spacer (green triangles).
Figure 8.
Temperature increase by self-heating as a function of the power dissipated in the SiPM. Best thermal contact is obtained with alumina substrate directly on the chuck (green squares). The thermal contact of the flexible PCB (red circles) shows the same performance as the alumina substrate with thick POM spacer (green triangles).
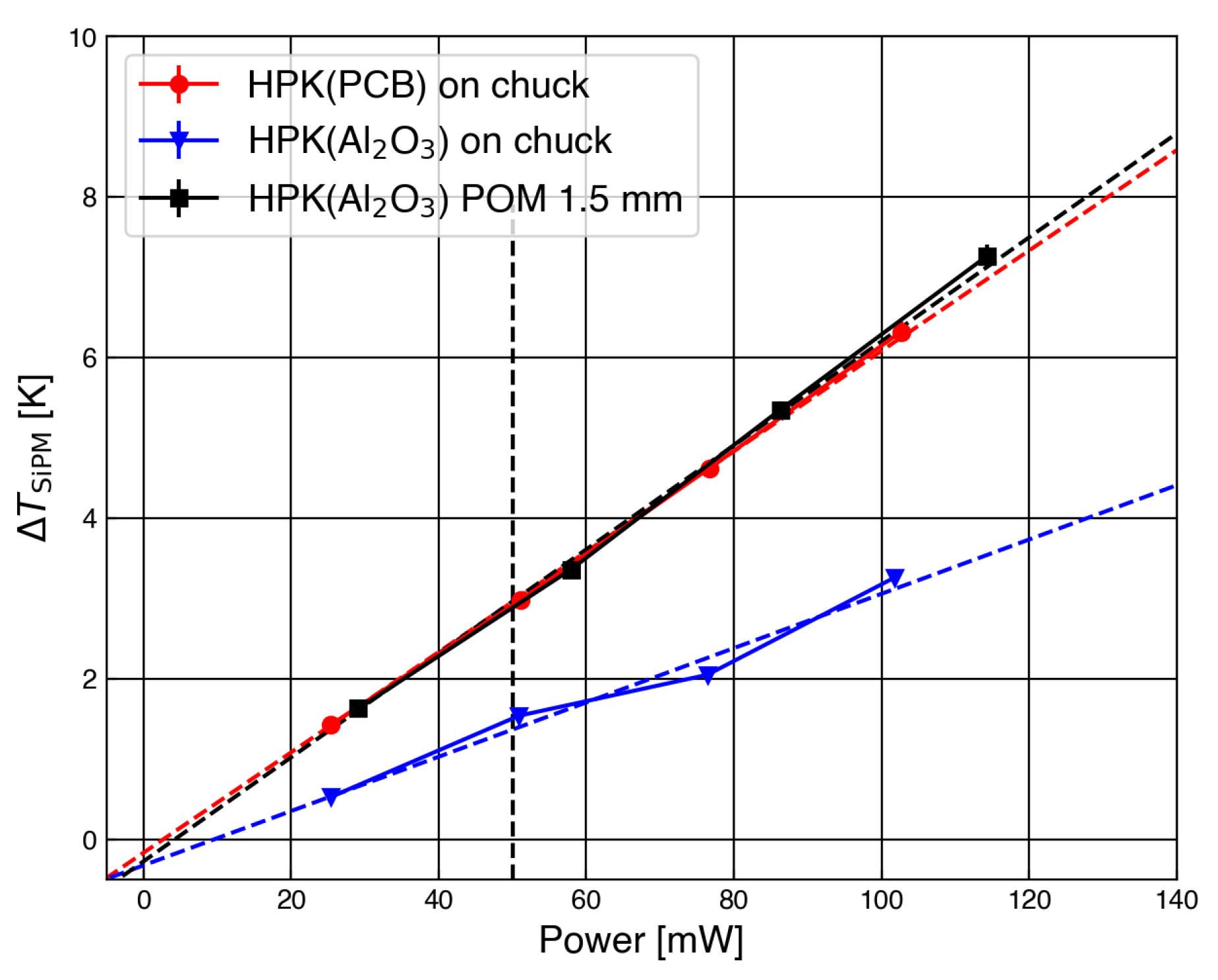
Figure 9.
Comparison of temperature increase by self-heating as a function of the power dissipated for two SiPM models: KETEK (blue), and HPK on PCB (red). Circles represent data taken with the substrate on top of the chuck surface, triangles are for data using the thinner POM layer in between, and squares for the thicker POM layer. The dotted lines are linear fits to the data. The vertical dashed line corresponds to the reference power of 50.
Figure 9.
Comparison of temperature increase by self-heating as a function of the power dissipated for two SiPM models: KETEK (blue), and HPK on PCB (red). Circles represent data taken with the substrate on top of the chuck surface, triangles are for data using the thinner POM layer in between, and squares for the thicker POM layer. The dotted lines are linear fits to the data. The vertical dashed line corresponds to the reference power of 50.
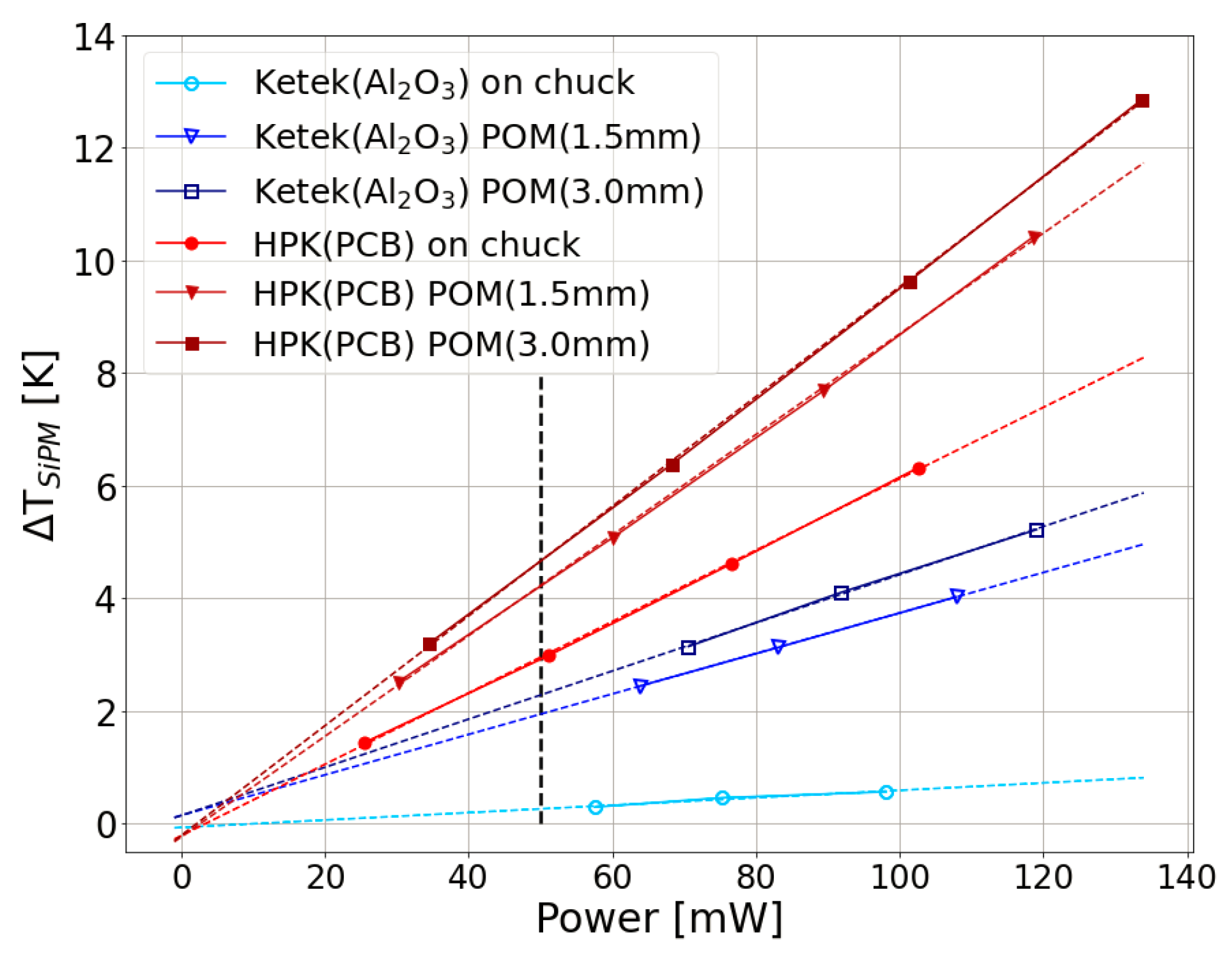
Figure 10.
SiPM current measured in step B of the cycle performed with the HPK SiPM at 25 °C and at °C.
Figure 10.
SiPM current measured in step B of the cycle performed with the HPK SiPM at 25 °C and at °C.
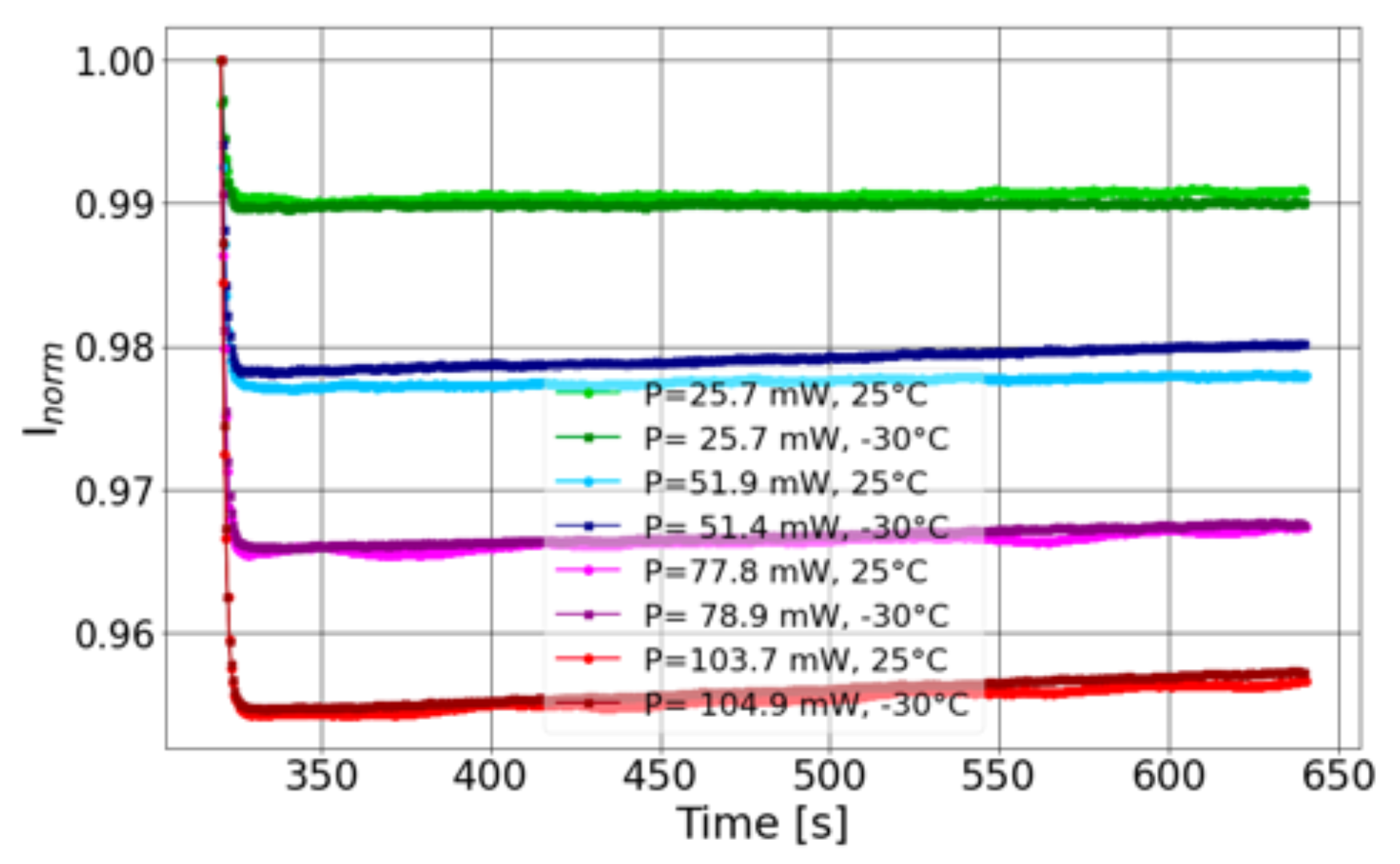
Figure 11.
Comparison of temperature increase by self-heating (left) and breakdown voltage shift (right) as a function of the power dissipated for 25 °C (red) and °C (cyan). Circles indicate data taken with the substrate on top of the chuck surface, and triangles (squares) data using the thinner (thicker) POM layer. The dotted lines are linear fits to the data. The vertical dashed line corresponds to 50.
Figure 11.
Comparison of temperature increase by self-heating (left) and breakdown voltage shift (right) as a function of the power dissipated for 25 °C (red) and °C (cyan). Circles indicate data taken with the substrate on top of the chuck surface, and triangles (squares) data using the thinner (thicker) POM layer. The dotted lines are linear fits to the data. The vertical dashed line corresponds to 50.
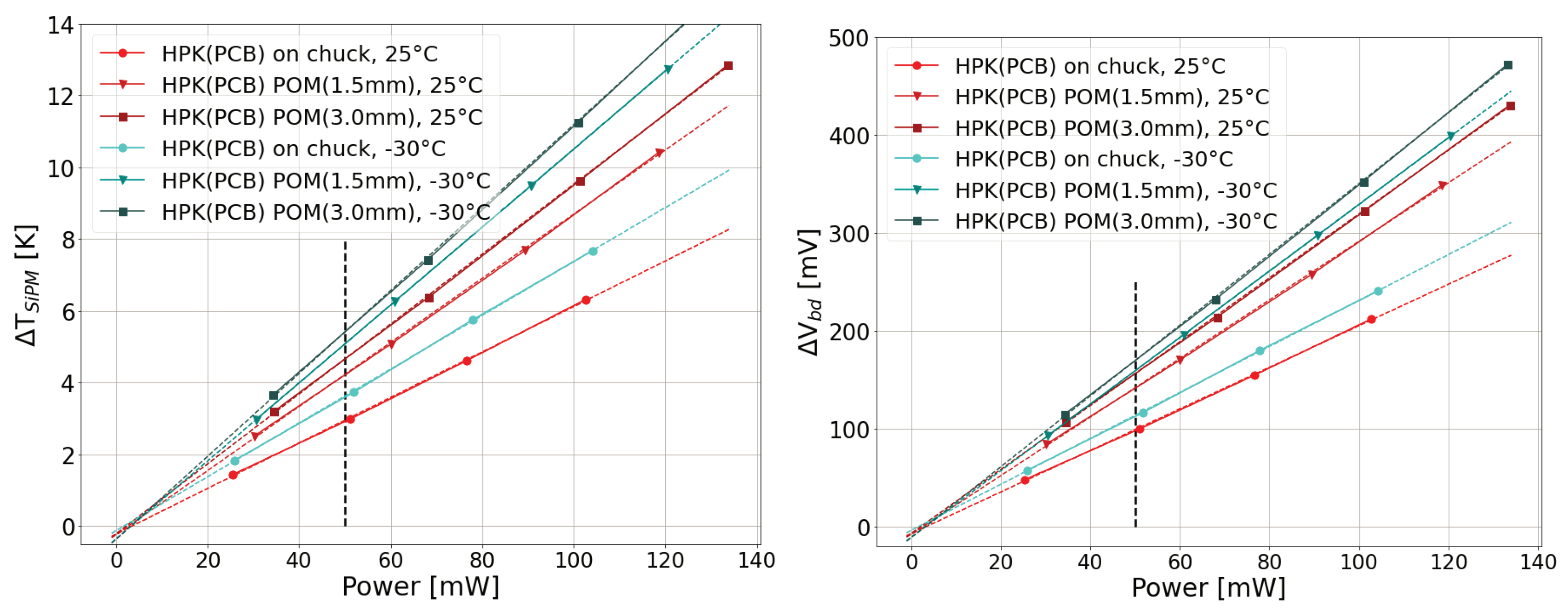
Table 1.
Summary information of the two SiPM models used in this study.
| Sample ID | S6 | S14160-9769 |
|---|---|---|
| Manufacturer | KETEK | HPK (MPPC) |
| # pixels | 27367 | 89600 |
| Pixel size [ | 15 | 10 |
| Active area [ | 6.2 | |
| Sample thickness [ | 700 | 800 |
| Substrate | Alumina | PCB/Alumina |
| (@ 25 °C) [ | ||
| (@ °C) [ | - | |
| [ | 22.4 | 33.5 (RT) |
| [ | - | low |
| [(@ 25 °C) | OV) | |
| [(@ °C) | - |
Table 2.
Breakdown voltage and temperature increase by self-heating for KETEK and HPK SiPMs at 25 °C dissipating 50 power. The overvoltage during the measurement cycles were (KETEK), (HPK).
Table 2.
Breakdown voltage and temperature increase by self-heating for KETEK and HPK SiPMs at 25 °C dissipating 50 power. The overvoltage during the measurement cycles were (KETEK), (HPK).
| Sample | Substrate | POM [mm] | [ | [ |
|---|---|---|---|---|
| KETEK | Al2O3 | 0 | 6 | 0.2 |
| KETEK | Al2O3 | 1.5 | 44 | 2.0 |
| HPK | Al2O3 | 0 | 50 | 1.5 |
| HPK | Al2O3 | 1.5 | 100 | 3.0 |
| HPK | PCB | 0 | 100 | 3.0 |
| HPK | PCB | 1.5 | 140 | 4.2 |
| HPK | PCB | 3.0 | 154 | 4.6 |
Table 3.
Breakdown voltage and temperature increase by self-heating HPK SiPM at 25 °C and °C dissipating 50power. The overvoltage during the measurement cycles were (HPK, 25 °C), and (HPK, °C).
Table 3.
Breakdown voltage and temperature increase by self-heating HPK SiPM at 25 °C and °C dissipating 50power. The overvoltage during the measurement cycles were (HPK, 25 °C), and (HPK, °C).
| Sample | Substrate | POM [mm] | T [ °C] | [ | [ |
|---|---|---|---|---|---|
| HPK | PCB | 0 | 25 | 10 | 3.0 |
| HPK | PCB | 0 | -30 | 113 | 3.6 |
| HPK | PCB | 1.5 | 25 | 140 | 4.2 |
| HPK | PCB | 1.5 | -30 | 160 | 5.1 |
| HPK | PCB | 3.0 | 25 | 158 | 4.7 |
| HPK | PCB | 3.0 | -30 | 170 | 5.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Alerts
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






