Prerpints.org logo
Preprint
Article

Dynamic of Quantum Correlation in Qubit-Qutrit States (Perpendicular Direction) under Multilocal and Global Dephasing: Classical Noises Effect

Altmetrics

Downloads

119

Views

35

Comments

0

Submitted:

12 March 2024

Posted:

13 March 2024

You are already at the latest version

Alerts
Abstract
Time evolution of the quantum correlation of the hybrid qubit-qutrit system in multilocal and global dephasing under the effect of a classical noises environment is presented. We considered the qutrit in z direction and the qubit x direction. In addition, our system presents two configurations: the different and the common environment and our discussion involves three types of noises: A Static Noise (SN), Random Telegraph Noise (RTN) and Ornstein- Uhlenbeck noise (OU). By using the density matrix approach to determine the evolution of the state of the system, the found that the static noise is fatal for the quantum entanglement, the quantum correlation and for RTN and OU noises the quantum entanglement, the quantum correlation decreases monotonically with a sufficiently long time. Therefore, we can say that, entanglement and the quantum correlation are more robust to the noise precisely those Random telegraph noise and Ornstein-Uhlenbeck noise.
Keywords: 
Subject: Physical Sciences  -   Quantum Science and Technology

1. Introduction

Quantum entanglement is a fundamental key resource for quantum information processing communication and exponential speed up of some computational tasks[1,2]. However, it has been shown both theoretically and experimentally, that entanglement cannot capture all the quantumness of correlations, being only a special kind of quantum correlation [3,4]. A characteristic example is the separable mixed state that has vanishing entanglement but non-vanishing quantum correlation. Such states can be employed as a resources for the implementation of deterministic quantum computation with one qubit (DQC) both theoretically and experimentally [4,5]. In this sense, it has been revealed that there exists other quantum correlation such as measurement induced disturbance [6]and quantum discord [7,8] which are useful as well. As quantum systems interact with the environment, these interactions lead to the destruction of quantum properties resulting in system decoherence [9]. One of the most important results of these entanglements is the total loss of entanglement called sudden entanglement death (SED) [10,11]. However, it should also be noted that the survival or preservation of quantum properties can be induced by these interactions [12,13,14,15,16,17,18,19,20,21,22,23,24]. Environments can be considered as noise and can be classified into two categories. namely Markovian and non-Markovian [25,26,27] environments or noise. It is therefore important to study and optimise the dynamics of quantum correlation as these deteriorate under the effect of interaction with the environment. Thus, a detailed analysis of the dynamics of entanglement and quantum correlation for qubit-qutrit states has a parameter under the influence of classical Independent or common noise. However, the study of the dynamics of entanglement interacting with the environment in a hybrid qubit-qutrit system along perpendicular directions has also been studied with an initial state are maximally entangled. hybrid systems with one and two entanglement parameters have been studied [28,29]. thus, further research is being carried out on decoherence for different environments [30,31,32,33,34,35,36,37,38,39,40,41]. The objective of our paper is to analyse the temporal evolution of entanglement and quantum correlation in one-parameter hybrid systems in perpendicular directions interacting with independent or collective classical noise. These are static noise recently used to describe electron transport and photon propagation in disordered structures [42,43]. However, the other quantum correlation can be quantified a using Measurement- Induce- Disturbance [44]. choices of this subject is motivated by the fact, what will be the effect of classical noises of our system? Answering this question well then be the subject of our study. This work is organized as follows: In sect. 1, we present introduction. In Sect. 2, we present two physical confurations of qubit-qutrit acting with a noises in independent, common and bipartite environments and methods. In Sect. 3, we present the different noises use in this paper. 4,we report result numerical , in sect.5 we have analytical results, and we end the work in Sect. 6 with a conclusion.

2. Materials and Methods

2.1. Methods

2.1.1. Measure of Correlation of Qubit-Qutrit System

To measure of entanglement and quantum correlation we can use Measured-Induce Disturbance (MID)[44] and negativity[45]
N = 1 2 ( ‖ ρ T K ‖ − 1 )
Where ρ T K is the partial transpose of the density matrix ρ A B with respect to system and   ‖ .... ‖ Denotes the trace norm. The density matrix is given by
ρ A B ( t ) = U A B ( t 0 , t ) ρ A B ( t 0 ) U † A B ( t 0 , t )
Where U A B ( t 0 , t ) is a operator evolution and ρ A B ( t 0 ) is a density matrix at initial state. We also use MID as a measure of quantum correlation of the system it is defined by
M I D ( ρ A B ( t ) ) = I ( ρ A B ( t ) ) − I ( ∏ ( ρ A B ( t ) ) )
Where I is the mutual quantum information given by
I ( ρ A B ( t ) ) = S ( ρ A ) + S ( ρ B ) − S ( ρ A B )
∏ ( ρ A B ) = ∑ i , j ( ( ∏ i A ⊗ ∏ j B ) ρ A B ( ∏ i A ⊗ ∏ j B ) )
( ∏ i A ) and ( ∏ i B ) are sets of orthogonal one dimensional Eigen projection operators for systems A and B

2.2. The Physical Model

Our Physical model, we have qubit (spin-1/2) and qutrit (spin-1) are hybrid non-interacting system But, interacting locally in Figure 1 or collectively in Figure 2 with classical dephasing noise channels.
The Hamiltonian of our system is given by.
H = H A ⊗ I 2 + H B ⊗ I 3
H A = [ g A T A ( t ) + g V V ( t ) ] σ X   is   Hamiltonian   of   qubit   interact   with   environment
H B = [ g B T B ( t ) + g V V ( t ) ] S Z   is   Hamiltonian   of   qutrit   interact   with   environment
We have { g i ; T i } ; i ∈ { A , B } are continuous connection of qubit-qutrit with various environments and random parameters, connected to the particular noise characteristics { g C ; V ( t ) } qubit-qutrit constant coupling with a shared environment and random parameter, connected to the unique properties of noise.

3. Noises Used

3.1. Static Noise

The fact that the dimensionless parameters are used in this section to represent the static noise
g B ( t ) = g B   g A ( t ) = g A ; V ( t ) = V and g A , g B , g C . based on the probability distribution provided by, and presumed to be time-independent random variables
P ( ς ) = { 1 l m   f o r   | ς − l 0 | ≤ l m 2 0   otherwise
ς = { g A ; g B ; V } represents the stochastic variable l m determines how chaotic the local and collective environments are, whereas l 0 denotes the mean value of the ditributions the autocorrelation function of ς reads 〈 δ ς ( t ) δ ς ( 0 ) 〉 = l m 2 12 and δ -function with a zero-frequency center gives its power spectrum as a result. This implies that the impacts of the static noise on the environment do not ever completely disappear. We can formally refer to such a noise as non-markovian as a result. Static noise drives the qubit-qutrit system, causing disorder in either independent (ie) or collective (ce) environment interactions. To fully capture the dynamics of the system in a chaotic environment on average. All conceivable noise configurations must be considered. The integral of the time-evolved states, each of which corresponds to a particular selection of the noise parameters, provides such an average for static noise.

3.2. Random Telegraph Noise

The RTN, which may characterize a variety of common phenomena impacting solid-state devices on the nanoscale, is the other type of noise that we look at. It explains a discrete stochastic process, the random telegraph noise g i   i = A ; B and V ( t ) , which is assumed to flip randomly between the values -1 and 1 at the rate μ i   and   μ , with t ∈ [ 0 , ∞ [ . The autocorrelation function of the random va- riable
ς ( t ) = { g A ( t ) ; g B ( t ) ; g C ( t ) }   reals 〈 δ ς ( t ) δ ς ( t ' ) 〉 = exp { − 2 μ | t − t ' | }
with lorentzian power spectrum
S ( ω ) = 4 μ ω 2 + μ 2
Accounting for the ratio a = μ υ . The dynamics of quantum correlation are classified into two regimes. the Markovian government a ≫ 1 ; fast   RTN and the non-Markovian govenment a ≪ 1 : slow   RTN . The dephasing RTN channel's impact over a period of time 0 ,   t   . both the qubit and qutrit accumulate either the phase factor
L k = − υ ∫ 0 t g K ( t ' ) d t ' L c = − υ ∫ 0 t V ( t ' ) d t '

3.3. Ornstein-Uhenbeck

It has a lorentzian spectrum and is a stationary Markovian and Gaussian process. S ( ω ) = 4 μ ω 2 + μ 2 . The autocorrelation function of the stochastic parameter ζ ( t ) used to describe the OU process reads:
〈 δ ζ ( t ) δ ζ ( t ' ) 〉 = μ 2 exp { − μ | t − t ' | }
The average is computed using the characteristic function of a Gaussian random process with zero mean, and the outcomes are stated as expressions of the form
Q n = exp { − n 2 2 w ( e − w τ + w τ − 1 ) }
w = μ υ is the scaled spectral width of the process and   τ = υ t .

4. Numerical Results

The overall system's starting condition is
ρ ( t 0 ) = 1 2 ( r 0 0 0 0 r 0 r 0 0 0 0 0 0 p p 0 0 0 0 p p 0 0 0 0 0 0 r 0 r 0 0 0 0 r )
Where p = 1 − 2 r and r is the restricted-to-the-range entanglement parameter 0 ≤ r ≤ 1 2 to guarantee the positivity condition of ρ ( t 0 ; r ) Recall that the initial state
is entangled in the mentioned range, except at r = 1 3

4.1. Static Noise

  • Density matrix
Density matrix describes by for the independent and shared environment.
ρ i e ( c e ) ( t ) = ( ρ 11 0 0 0 0 ρ 16 0 r 2 0 0 0 0 0 0 ρ 33 ρ 34 0 0 0 0 ρ 34 ρ 33 0 0 0 0 0 0 r 2 0 ρ 16 0 0 0 0 ρ 11 )
ρ 11 = ( 3 r − 1 ) 4 sin c ( g i l m t ) − ( r − 1 ) 4 ρ 16 = ρ 34 = r 2 sin c ( g i l m t ) ρ 33 = ( 1 − 3 r ) 4 sin c ( g i l m t ) − ( r − 1 ) 4
The density matrix of the total system a time t is
ρ A B ( t ) = U A B ( t 0 , t ) ρ A B ( t 0 ) U † A B ( t 0 , t )
with i ∈ { A ; B ; C } for a independent environment V = 0
N i e ( c e ) = 1 2 ( 2 | χ 1 i e ( c e ) | + | χ 3 ± i e ( c e ) | + | χ 4 ± i e ( c e ) | − 1 )
  • Different environment/common environment
When the system is coupled to the static noise, we find after performing calculating that the negativity and MID respectively, can be written as follows
χ 3 ± i e ( c e ) ( t ) = 3 r − 1 4 sin c ( g A ( C ) l m t ) − r − 1 4 ± r 2 sin c ( g B ( C ) l m t ) χ 1 i e ( c e ) = χ 2 i e ( c e ) = r 2
χ 4 ± i e ( c e ) ( t ) = − 3 r + 1 4 sin c ( g A ( C ) l m t ) − r − 1 4 ± r 2 sin c ( g B ( C ) l m t ) lim t → 0 N i e ( c e ) = 1 2 ( 2 r + | 1 − r | 2 + | 1 − 3 r | 2 ) ;   lim t → ∞ N i e ( c e ) = 0 M I D = S ( ρ ' A B i e ( c e ) ) − S ( ρ A B i e ( c e ) )
MID = − 2 ρ 11 i e ( c e ) log ( ρ 11 i e ( c e ) ) − 2 ρ 33 i e ( c e ) log ( ρ 33 i e ( c e ) ) + ∑ s = ± Q ' s
where
Q ' S = | ρ 11 i e ( c e ) ± ρ 16 i e ( c e ) | log | ρ 11 i e ( c e ) ± ρ 16 i e ( c e ) | + | ρ 33 i e ( c e ) ± ρ 34 i e ( c e ) | log | ρ 33 i e ( c e ) ± ρ 34 i e ( c e ) | lim t → 0 M I D i e ( c e ) = 1 2 ( r log r + | 1 − 3 r | 2 log | 1 − 3 r | 2 + | 1 − 2 r | 2 log | 1 − 2 r | 2 + | 1 − r | 2 log | 1 − r | 2 ) ρ 11 i e ( c e ) ± ρ 16 i e ( c e ) =   χ 3 ± i e ( c e ) ρ 33 i e ( c e ) ± ρ 34 i e ( c e ) = χ 4 ± i e ( c e ) ρ 11 i e ( c e ) = 1 4 ( − 3 r H 2 q + H 2 q − r + 1 )   ρ 33 i e ( c e ) = 1 4 ( − r + H 2 q ( 3 r − 1 ) + 1 ) lim t → ∞ M I D i e ( c e ) = 0

4.2. Random Telegraph Noise

  • Density matrix
When the system is coupled to the RTN, we find after performing calculating that the negativity and MID respectively, can be written as follows
ρ i e ( t ) = 〈 〈 ρ ( L A ( t ) , L B ( t ) ) 〉 L A 〉 L B ρ c e ( t ) = 〈 ρ ( L c ( t ) ) 〉 L c
ρ ( t ) = ρ A B i e = ρ A B c e = ( ρ 11 0 0 0 0 ρ 16 0 r 2 0 0 0 0 0 0 ρ 33 ρ 34 0 0 0 0 ρ 34 ρ 33 0 0 0 0 0 0 r 2 0 ρ 16 0 0 0 0 ρ 11 )
Where   ρ 11 i e , c e = 1 4 ( − r + H 2 ( 3 r − 1 ) + 1 ) ;   ρ 16 i e , c e = ρ 34 i e , c e = r 2 H 2   ρ 33 i e , c e = 1 4 ( − 3 r H 2 + H 2 − r + 1 ) H n ( τ ) = { e − q τ [ cosh ( κ q n τ ) + τ κ q n sinh ( κ q n τ ) ]   τ > n q e − q τ [ cos ( κ q n τ ) + τ κ q n sin ( κ q n τ ) ]   τ < n q κ q n = | τ 2 − ( q n ) 2 | f o r   τ > n q   we   have   a   Markovian   regime   and   τ < nq   we   have   a   non - Markovian   regime
  • Negativity
Here we present the analytical results of entanglement in terms of negativity when the two subsystems are affected by RTN noise in different and common environments
N i e ( c e ) = 1 2 ( 2 | χ 1 i e ( c e ) | + | χ 3 ± i e ( c e ) | + | χ 4 ± i e ( c e ) | − 1 ) χ 1 i e ( c e ) = χ 2 i e ( c e ) = r 2
With different values
χ 3 ± i e ( c e ) = 1 4 ( − 3 r H 2 q + H 2 q − r + 1 ) ± r 2 H 2 q χ 4 ± i e ( c e ) = 1 4 ( − r + H 2 q ( 3 r − 1 ) + 1 ) ± r 2 H 2 q lim t → ∞ N i e ( c e ) = 0 lim t → 0 N i e ( c e ) = 1 8 ( | 2 − 7 2 r | + | 2 − 9 2 r | + 4 r )

4.3. ORNSTEIN-UHENBECK

  • Density matrix
ρ ( t ) = ρ A B i e = ρ A B c e = ( ρ 11 0 0 0 0 ρ 16 0 r 2 0 0 0 0 0 0 ρ 33 ρ 34 0 0 0 0 ρ 34 ρ 33 0 0 0 0 0 0 r 2 0 ρ 16 0 0 0 0 ρ 11 ) ρ 11 i e , c e = 1 4 ( − r + Q 2 ( 3 r − 1 ) + 1 ) ρ 16 i e , c e = ρ 34 i e , c e = r 2 Q 2 ρ 33 i e , c e = 1 4 ( − 3 r Q 2 + Q 2 − r + 1 )
  • Negativity
Here we present the analytical results of entanglement in terms of negativity when the two subsystems are affected by OU noise in different and common environments.
N i e ( c e ) = 1 2 ( 2 | χ 1 i e ( c e ) | + | χ 3 ± i e ( c e ) | + | χ 4 ± i e ( c e ) | − 1 ) χ 1 i e ( c e ) = χ 2 i e ( c e ) = r 2 χ 3 ± i e ( c e ) = 1 4 ( − 3 r Q 2 w + Q 2 w − r + 1 ) ± r 2 Q 2 w χ 4 ± i e ( c e ) = 1 4 ( − r + Q 2 w ( 3 r − 1 ) + 1 ) ± r 2 Q 2 w

5. Analytical Results

The analytical and numerical findings of entanglement in terms of negativity when the two subsystems are impacted by static noise in various and typical situations are presented here

5.1. Static Noise

  • Negativity
Different environment
Figure 1: Time evolution of negativity as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A = g B = 10
Figure 2: Time evolution of negativity as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A = g B = 1 We see that the entanglement at the initial instant is at its maximum and disappears abruptly when the distance increases under the static noise and the another figure clearly shows that for large coupling factors the entanglement at the initial instant is at its maximum and disappears abruptly then appears again but with a small amplitude, the death and survives with a smaller amplitude and dies definitively when the time increases
Figure 3: Time evolution of negativity as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A > g B
Figure 4: Time evolution of negativity as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A < g B The figure clearly shows that for large coupling factors for qubit the entanglement at the initial instant is at its maximum and disappears abruptly then appears again but with a small amplitude, the death and survives with a smaller amplitude and dies definitively when the time increases it seems that the coupling factor of the qubit on the noise and neglected that of the qutrit and The another figure clearly shows that for large coupling factors for qutrit the entanglement at the initial instant is at its maximum and disappears abruptly it seems that the coupling factor of the qutrit on the noise and neglected that of the qubit. The qutrit is very dephasing compared to the qubit
Common environment
When the system is coupled to the static noise in common environment, we have In Figure 5 we have Time evolution of negativity as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for common environment. This figure clearly shows that for an initial states of system for r =0.5, the system is not affected by the static noise. This is not the case when the system interact with the static noise in common environment, entanglement essentially vanish abruptly (sudden death) except for values of r in ] 0.3 ; 0.5 ] with time [ 0 ; 2.25 ] for r =0.3 where separability occus in the qubit-qutrit it is found that the system exhibits non-zero quantum correlation.
  • Measured-Induced Disturbance (MID)
Different environment/common environment
When the system is coupled to the static noise in independent environment (common environment), we find after performing calculating that the MID can be written as follows
Figure 6 : Time evolution of MID as a function of the scaled time and the parameter r when the system is coupled to a static noise for different environment with g A = g B = 10
Figure 7 : Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A = g B = 10 For weak coupling factors, at the initiel time quantum correlation has a level and when the time increases it decreases asymptotically and we observe a phenomenon of sudden death and survival then the total disappearance of the quantum correlation. And for large coupling factors, at the initial time quantum correlation has a level and when the time increases it decreases asymptotically and we observe a phenomenon of sudden death and survival then the total disappearance of the quantum correlation.
Figure 8: Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A > g B ; Figure 9: Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for different environment with g A < g B . For large coupling factors of qubit, at the initial time quantum correlation has a level and when the time increases it decreases asymptotically and we observe a phenomenon of sudden death and survival and oscillates then the total disappearance of the quantum correlation.
For large coupling factors of qutrit, at the initial time quantum correlation has a level and when the time increases it decreases asymptotically then the total disappearance of the quantum correlation.
When the system is coupled to the static noise in common environment, we find after performing calculations that the MID can be written as follows. Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a static noise for common environment. This figure clearly shows that for an initial state of system we have one level, the correlation decreases asymptotically and we have a phenomenon of sudden death and survival for a time 3 ; 6 with smaller amplitudes unit it is cancelled in Figure 10

5.2. Random Telegraph Noise

  • Negativity and MID
Here, we give the numerical entanglement findings in terms of negativity when the two subsystems are exposed to RTN noise in various settings. The non-Gaussian noise known as random telegraph noise results from a bistable fluctuator that alternates between two states at a certain switching rate. Two regimes Markovian and non-Markovian regimes appear depending on the relationship between the switching rate and coupling constant. Non-Markovian regime. The non-Markovian regime is so called slow regime. This maps describes memory dynamics. The definition of negativity is reads:
Different environment/ common environment
A non-Markovian regime
H n q = e − γ t [ cos ( κ n q t ) + γ κ n q sin ( κ n q t ) ] τ < n q   κ n q = | τ 2 − ( n q ) 2 |
In Figure 11: Time evolution of Negativity as a function of the scaled time τ and the parameter r when the system is coupled to a RTN noise for common environment/different environment, and in Figure 12: Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a RTN noise for common environment/different environment. For a coupling factors, at the initial time entanglement has a level and when the time increases it decreases amplitude and we observe a phenomenon of sudden death and survival then the entanglement oscillates. For a coupling factors, at the initial time quantum correlation has a level and when the time increases it decreases amplitude and we observe a phenomenon of sudden death and survival then the quantum correlation oscillates.
A Markovian regime
Different environment/ common environment
This regime is usually referred to as fast or Markovian regime. so Markovian maps, describes.
This regime is usually referred to as fast or Markovian regime. so Markovian maps, describes memoryless dynamics
H n q = e − q τ [ cosh ( κ n q τ ) + γ κ n q sin ( κ n q τ ) ] γ > n q  
In Figure 13: Time evolution of NEGATIVITY as a function of the scaled time τ and the parameter r when the system is coupled to a RTN noise for common environment/independent environment and the Figure 14: Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a RTN noise for common environment/ independent environment. For a coupling factors, at the initial time entanglement its maximally and when the time increases it decreases amplitude. For a coupling factors, at the initial time quantum correlation has a level and when the time increases it decreases asymptotically.

5.3. Ornstein-Uhlenbeck (OU)

  • Negativity
Here we present the analytical results of entanglement in terms of negativity when the two subsystems are affected by OU noise in different and common environments.
Figure 15 Time evolution of NEGATIVITY as a function of the scaled time τ and the parameter r when the system is coupled to a OU noise for common environment and in Figure 16 Time evolution of NEGATIVITY as a function of the scaled time τ and the parameter r when the system is coupled to a OU noise for different environment w 1 = w 2 These figure clearly shows that for an initial states of system for, the system is not affected by the OU. This is not the case when the system interact with the OU in common environment, entanglement essentially vanish abruptly.
  • Measured- Induced Disturbance (MID)
Figure 17 Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a OU noise for common environment. w 1 = w 2
Figure 18 : Time evolution of MID as a function of the scaled time τ and the parameter r when the system is coupled to a OU noise for common environment

6. Conclusion

Our system initially prepared in a one-parameter qubit-qutrit state entangled and then subjected to classical noise: random telegraph noise, static noise Ornstein-Uhlenbeck, we study the dynamics of quantum correlation. qubit-qutrit system coupled in independent and common environment. Negativity and MID has been computed, using both analytical and numerical technique and we found that, as a result of the interaction of the system with the environment for a non-Markovian we observe a phenomenon of sudden death and survival then the entanglement oscillates and for a static noise in common environment, entanglement essentially vanish abruptly (sudden death) except for values of r in ] 0.3 ; 0.5 ] .

References

  1. C.H. Bennet and S. j. Wiesner, Phys. Rev. Lett. 69 (1992) 2881.
  2. Shor et al: Algorithms exist on a quantum computer science and appears in the SIAM journal of computing26 pp.1484-1509 (1997).
  3. M. Piani, P. Horodecki, R. Horodecki, No-local-broadcasting theorem for multipartite quantum correlations, Phys. Rev. Lett. 100 (9) (2008) 090502. [CrossRef]
  4. B.P. Lanyon, M. Barbieri, M.P. Almeida, A.G. White, Experimental quantum computing without entanglement, Phys. Rev. Lett. 101 (20) (2008) 200501. [CrossRef]
  5. Datta, A. Shaji, C.M. Caves, Quantum discord and the power of one qubit, Phys. Rev. Lett. 100 (5) (2008) 050502. [CrossRef]
  6. S. Luo, Using measurement-induced disturbance to characterize correlations as classical or quantum, Phys. Rev. A 77 (2) (2008) 022301. [CrossRef]
  7. H. Ollivier, W.H. Zurek, Quantum discord: a measure of the quantumness of correlations, Phys. Rev. Lett. 88 (1) (2001) 017901. [CrossRef]
  8. L. Henderson, V. Vedral, Classical, quantum and total correlations, J. Phys. A, Math. Gen. 34 (35) (2001) 6899–6905. [CrossRef]
  9. W.H. Zurek, Decoherence, einselection, and the quantum origins of the classical, Rev. Mod. Phys. 75 (3) (2003) 715–775. [CrossRef]
  10. T. Yu, J. Eberly, Sudden death of entanglement: classical noise effects, Opt. Commun. 264 (2) (2006) 393–397. [CrossRef]
  11. T. Yu, J.H. Eberly, Sudden death of entanglement, Science 323 (5914) (2009) 598–601. [CrossRef]
  12. Franco, G. Compagno, Experimental recovery of quantum correlations in absence of system environment back-action, Nat. Commun. 4 (2013) 2851. [CrossRef]
  13. Orieux, A. D’Arrigo, G. Ferranti, R.L. Franco, G. Benenti, E. Paladino, G. Falci, F. Sciarrino, P. Mataloni, Experimental on-demand recovery of entanglement by local operations within non-Markovian dynamics, Sci. Rep. 5 (1) (2015). [CrossRef]
  14. D’Arrigo, G. Benenti, R.L. Franco, G. Falci, E. Paladino, Hidden entanglement, system-environment information flow and non-Markovianity, Int. J. Quantum Inf. 12 (02) (2014) 1461005. [CrossRef]
  15. Mortezapour, R.L. Franco, Protecting quantum resources via frequency modulation of qubits in leaky cavities, Sci. Rep. 8 (1) (2018). [CrossRef]
  16. Z.-X. Man, Y.-J. Xia, R.L. Franco, Cavity-based architecture to preserve quantum coherence and entanglement, Sci. Rep. 5 (1) (2015). [CrossRef]
  17. D’Arrigo, R.L. Franco, G. Benenti, E. Paladino, G. Falci, Hidden entanglement in the presence of random telegraph dephasing noise, Phys. Scr. T 153 (2013) 014014. [CrossRef]
  18. F. Benatti, R. Floreanini, M. Piani, Environment induced entanglement in Markovian dissipative dynamics, Phys. Rev. Lett. 91 (7) (2003). [CrossRef]
  19. R.L. Franco, G. Compagno, Overview on the phenomenon of two-qubit entanglement revivals in classical environments, in: Quantum Science and Technology, Springer International Publishing, 2017, pp. 367–391.
  20. K. Modi, A. Brodutch, H. Cable, T. Paterek, V. Vedral, The classical-quantum boundary for correlations: discord and related measures, Rev. Mod. Phys. 84 (4) (2012) 1655–1707. [CrossRef]
  21. Leggio, R.L. Franco, D.O. Soares-Pinto, P. Horodecki, G. Compagno, Distributed correlations and information flows within a hybrid multipartite quantum classical system, Phys. Rev. A 92 (3) (2015). [CrossRef]
  22. F. Altintas, A. Kurt, R. Eryigit, Classical memoryless noise-induced maximally discordant mixed separable steady states, Phys. Lett. A 377 (1–2) (2012) 53–59. [CrossRef]
  23. J. Trapani, M.G.A. Paris, Nondivisibility versus backflow of information in understanding revivals of quantum correlations for continuous-variable systems interacting with fluctuating environments, Phys. Rev. A 93 (4) (2016). [CrossRef]
  24. T. Yu, J.H. Eberly, Finite-time disentanglement via spontaneous emission, Phys. Rev. Lett. 93 (14) (2004) 140404. [CrossRef]
  25. Bellomo, R. Lo Franco, G. Compagno, Non-Markovian effects on the dynamics of entanglement, Phys. Rev. Lett. 99 (16) (2007) 160502. [CrossRef]
  26. Tchoffo, M., Tsokeng, A.T., Tiokang, O.M., Nganyo, P.N., Fai, L.C.: Phys. Lett. A 383, 1856 (2019).
  27. Y.Akbari-Kourbolagh1 ·Z.Pazhotan1 : Dynamics of Entanglement for a qubit-qutrit System Coupled to the Independent Classical Noises in Perpendicular Directions International Journal of Theoretical Physics (2022).
  28. Kenfack, L.T., Tchoffo, M., Javed, M., Fai, L.C.: Quantum Inf. Process. 19, 107 (2020).
  29. 30 K. Ann, G. Jaeger, Entanglement sudden death in qubit–qutrit systems, Phys. Lett. A 372 (5) (2008) 579–583. [CrossRef]
  30. G. Karpat, Z. Gedik, Correlation dynamics of qubit–qutrit systems in a classical dephasing environment, Phys. Lett. A 375 (47) (2011) 4166–4171. [CrossRef]
  31. W. Hai-Rui, R. Bao-Cang, L. Tao, H. Ming, D. Fu-Guo, Dynamics of entanglement for a two-parameter class of states in a qubit-qutrit system, Commun. Theor. Phys. 57 (6) (2012) 983. [CrossRef]
  32. M. Ramzan, M.K. Khan, Decoherence and entanglement degradation of a qubit-qutrit system in non-inertial frames, Quantum Inf. Process. 11 (2) (2012) 443–454. [CrossRef]
  33. G. Karpat, Z. Gedik, Invariant quantum discord in qubit–qutrit systems under local dephasing, Phys. Scr. 2013 (T153) (2013) 014036. [CrossRef]
  34. H. Yuan, L.-F. Wei, Correlation dynamics of two-parameter qubit—qutrit states under decoherence, Chin. Phys. B 22 (5) (2013) 050303. [CrossRef]
  35. J.-L. Guo, H. Li, G.-L. Long, Decoherent dynamics of quantum correlations in qubit–qutrit systems, Quantum Inf. Process. 12 (11) (2013) 3421–3435. [CrossRef]
  36. Y. Yang, A.-M. Wang, Correlation dynamics of a qubit—qutrit system in a spin chain environment with Dzyaloshinsky—Mariya interaction, Chin. Phys. B 23 (2) (2014) 020307. [CrossRef]
  37. K.O. Yashodamma, P.J. Geetha Sudha, Effectiveness of depolarizing noise in causing sudden death of entanglement, Quantum Inf. Process. 13 (11) (2014) 2551–2565. [CrossRef]
  38. J.-L. Guo, J.-L. Wei, W. Qin, Enhancement of quantum correlations in qubit–qutrit system under decoherence of finite temperature, Quantum Inf. Process. 14 (4) (2015) 1399–1410. [CrossRef]
  39. M. Jafarpour, F.K. Hasanvand, D. Afshar, Dynamics of entanglement and measurement-induced disturbance for a hybrid qubit-qutrit system interacting with a spin-chain environment: a mean field approach, Commun. Theor. Phys. 67 (1) (2017) 27. [CrossRef]
  40. Basit, H. Ali, F. Badshah, G.-Q. Ge, Enhancement of quantum correlations in qubit-qutrit systems under the non-Markovian environment, Commun. Theor. Phys. 68 (1) (2017) 29. [CrossRef]
  41. Thompson, G. Vemuri, G.S. Agarwal, Anderson localization with second quantized fields in a coupled array of waveguides, Phys. Rev. A 82 (5) (2010) 053805. [CrossRef]
  42. G. De Chiara, G.M. Palma, Berry phase for a spin 1 / 2 particle in a classical fluctuating field, Phys. Rev. Lett. 91 (9) (2003) 090404. [CrossRef]
  43. G. De Chiara, G.M. Palma, Berry phase for a spin 1 / 2 particle in a classical fluctuating field, Phys. Rev. Lett. 91 (9) (2003) 090404. [CrossRef]
  44. Fiasconaro, B. Spagnolo, Stability measures in metastable states with Gaussian colored noise, Phys. Rev. E 80 (4) (2009) 041110. [CrossRef]
  45. M.A.C. Rossi, M.G.A. Paris, Non-Markovian dynamics of single- and two-qubit systems interacting with Gaussian and non-Gaussian fluctuating transverse environments, J. Chem. Phys. 144 (2) (2016) 024113. [CrossRef]
  46. G. Vidal, R.F. Werner, Computable measure of entanglement, Phys. Rev. A 65 (3) (2002) 032314. [CrossRef]
Preprints 101196 g001
Figure 1. A qubit-qutrit system interacting with independent environments g A ( t ) and g B ( t ) classical noise channels is shown schematically. dashes in black. Red wavy lines show the interaction between each subparty and the classical noise channel, whereas lines show the entanglement between the subsystems.
Figure 1. A qubit-qutrit system interacting with independent environments g A ( t ) and g B ( t ) classical noise channels is shown schematically. dashes in black. Red wavy lines show the interaction between each subparty and the classical noise channel, whereas lines show the entanglement between the subsystems.
Preprints 101196 g001
Preprints 101196 g002
Figure 2. shows a schematic of a qubit-qutrit system interacting with regular V(t) classical noise channels in the surroundings. dashes in black. Red wavy lines show the interaction between the classical noise channel and each component, whereas lines show the entanglement between the subsystems.
Figure 2. shows a schematic of a qubit-qutrit system interacting with regular V(t) classical noise channels in the surroundings. dashes in black. Red wavy lines show the interaction between the classical noise channel and each component, whereas lines show the entanglement between the subsystems.
Preprints 101196 g002
Preprints 101196 g003
Figure 3.
Figure 3.
Preprints 101196 g003
Preprints 101196 g004
Figure 4.
Figure 4.
Preprints 101196 g004
Preprints 101196 g005
Figure 5.
Figure 5.
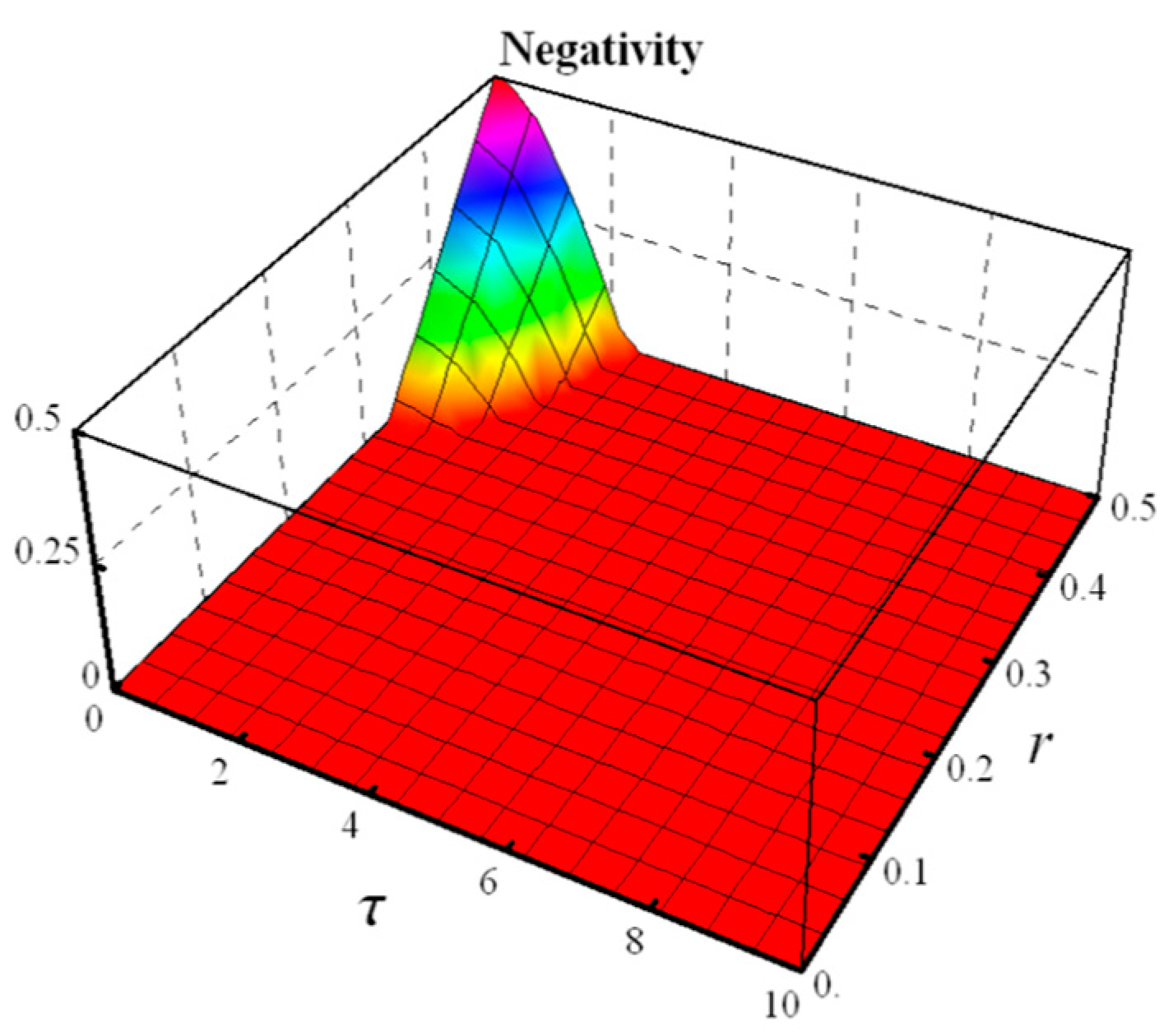
Preprints 101196 g005
Preprints 101196 g006
Figure 6.
Figure 6.
Preprints 101196 g006
Preprints 101196 g007
Figure 7.
Figure 7.
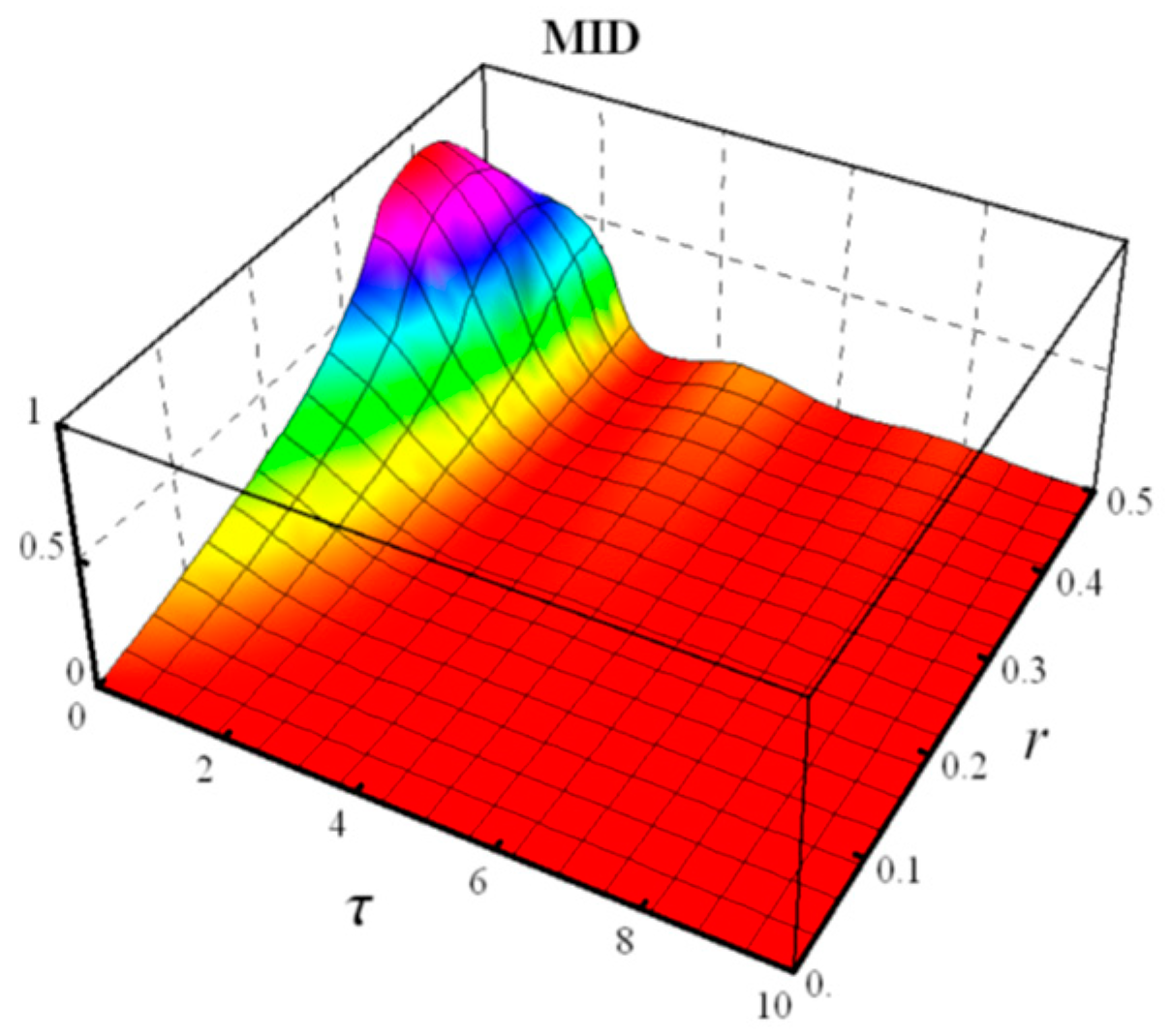
Preprints 101196 g007
Preprints 101196 g008
Figure 8.
Figure 8.
Preprints 101196 g008
Preprints 101196 g009
Figure 9.
Figure 9.
Preprints 101196 g009
Preprints 101196 g010
Figure 10.
Figure 10.
Preprints 101196 g010
Preprints 101196 g011
Figure 11.
Figure 11.
Preprints 101196 g011
Preprints 101196 g012
Figure 12.
Figure 12.
Preprints 101196 g012
Preprints 101196 g013
Figure 13.
Figure 13.
Preprints 101196 g013
Preprints 101196 g014
Figure 14.
Figure 14.
Preprints 101196 g014
Preprints 101196 g015
Figure 15.
Figure 15.
Preprints 101196 g015
Preprints 101196 g016
Figure 16.
Figure 16.
Preprints 101196 g016
Preprints 101196 g017
Figure 17.
Figure 17.
Preprints 101196 g017
Preprints 101196 g018
Figure 18.
Figure 18.
Preprints 101196 g018
Preprints 101196 g019
Figure 1.
Figure 1.
Preprints 101196 g019
Preprints 101196 g020
Figure 2.
Figure 2.
Preprints 101196 g020
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated