TEOS-10 Equations for the Lifted Condensation Level (LCL) and Climatic Feedback of Marine Clouds

1. Introduction

In typical global balance models, the energy exchange at the ocean-atmosphere interface includes quantitative estimates of five major contributions, namely, solar downward irradiation (≈ 165 W m^-2), thermal upward radiation from the sea surface (≈ – 400 W m^-2), thermal downward radiation from clouds and greenhouse gases (≈ 343 W m^-2), latent heat of evaporated water (≈ – 95 W m^-2), and sensible heat exchange (≈ – 12 W m^-2) by molecular conduction across the interface (Budyko 1963; Josey et al. 2013; Macdonald and Baringer 2013; Rapp 2014). The figures given in brackets are respective rough estimates for the enthalpy fluxes per ocean surface area, which result in a net imbalance of about 1 W m^-2 that is warming up the ocean, an amount 25 times as large as the world’s total energy consumption by humans in 2022 (You 2024). Similarly, the long-term global energy balance at the top of the atmosphere also amounts to about 1 W m^-2 (Meehl et al. 2011). This way, to the globally accumulated energy of the warming climate, the ocean contributes about 89 % (von Schuckmann et al. 2023) or 90 % (Abraham et al. 2013; Cheng et al. 2024) while the atmosphere takes only a minor share of just 1 %. With the advent of the Thermodynamic Equation of State of Seawater – 2010 (TEOS-10) as the current comprehensive international geophysical thermodynamic standard, previous methods for estimating the ocean heat content (Abraham et al. 2013) could be replaced by more accurate modern formulas (McDougall 2003; McDougall et al. 2021), see Section 3.

Each of the energy fluxes mentioned above may, to some extent, be responsible for the observed ocean warming. However, identifying the causes and processes of the enhanced ocean heat imbalance is scientifically challenging. The magnitude of the air-sea flux imbalance is well below the typical uncertainty threshold of at least 10 W m^-2 of climate models (Josey et al. 2013). “The drivers of a larger [Earth energy imbalance] EEI in the 2000s than in the long-term period since 1971 are still unclear, and several mechanisms are discussed in literature. For example, Loeb et al. (2021) argue for a decreased reflection of energy back into space by clouds (including aerosol cloud interactions) and sea ice and increases in well-mixed greenhouse gases (GHG) and water vapor to account for this increase in EEI. Kramer et al. (2021) refer to a combination of rising concentrations of well-mixed GHG and recent reductions in aerosol emissions to be accounting for the increase, and Liu et al. (2020) address changes in surface heat flux together with planetary heat redistribution and changes in ocean heat storage. Future studies are needed to further explain the drivers of this change, together with its implications for changes in the Earth system” (von Schuckmann et al. 2023).

Figure 1. Scattered subtropical marine cumulus clouds developing above a uniform lifted condensation level (LCL). Photo taken at Las Brujas, Cuba, in February 2014.

Figure 1. Scattered subtropical marine cumulus clouds developing above a uniform lifted condensation level (LCL). Photo taken at Las Brujas, Cuba, in February 2014.

“Low-altitude clouds with cloud top temperatures close to the ground environment generally lead to a weak greenhouse effect. In addition, the radiative cooling at the cloud top and the warming at the cloud base may enlarge the vertical temperature gradient and consequently intensify the turbulence” (Yang et al. 2021: p. 1). However, in some climate models “the low cloud response to [sea-surface temperature] SST change may be too weak” (Loeb et al. 2022: p. 14). As a contributor to the marine heat balance, low-level clouds affect the upwelling thermal radiation by the surface area fraction they cover, as well as by their altitude and the related cloud-base temperature driving their downward longwave radiation. As a function of the surface temperature and relative humidity (RH), an adiabatically ascending air parcel reaches its dew-point at the so-called lifted condensation level (LCL). In this article, equations for the LCL are derived from the international standard TEOS-10, the Thermodynamic Equation of Seawater – 2010 (IOC et al. 2008). As a longwave radiation feedback effect, deviations of the downward black-body radiation of those clouds can this way be estimated from observed trends related to global warming of the ocean surface.

In Section 2 of this paper, selected details of ocean warming are briefly reviewed and discussed in relation to other climatic trends, such as cloudiness reduction, SST increase, or apparently constant marine RH and wind speeds. Section 3 provides a short introduction into the thermodynamic framework of TEOS-10. In Section 4, TEOS-10 equations are formulated for the LCL pressure and temperature as functions of surface pressure, temperature and relative humidity. Rigorous results from those international standard equations may serve as reference values for other estimates. In addition to selected numerical results obtained from an iteration scheme, analytical approximations are suggested for the almost saturated marine troposphere as well as for the tutorial case of simple crude Gibbs function, as explicitly defined in Appendix C. Section 5 investigates the climatic sensitivity of the LCL temperature on the SST at constant RH, with selected numerical solutions of TEOS-10 equations and approximate formulas for crude Gibbs functions. Section 6 concludes the paper with a discussion of the climatological context and derived solutions. Appendix A introduces the Jacobi method for a convenient manipulation of partial derivatives used in TEOS-10. Appendix B calculates from TEOS-10 an equation for the adiabatic lapse rate of the dew point, as required in Section 4. Appendix D mathematically explains Lambert’s W function that is frequently encountered in atmospheric balance equations. Finally, Appendix E provides a list of symbols used in this paper.

2. Ocean Warming and Cloudiness

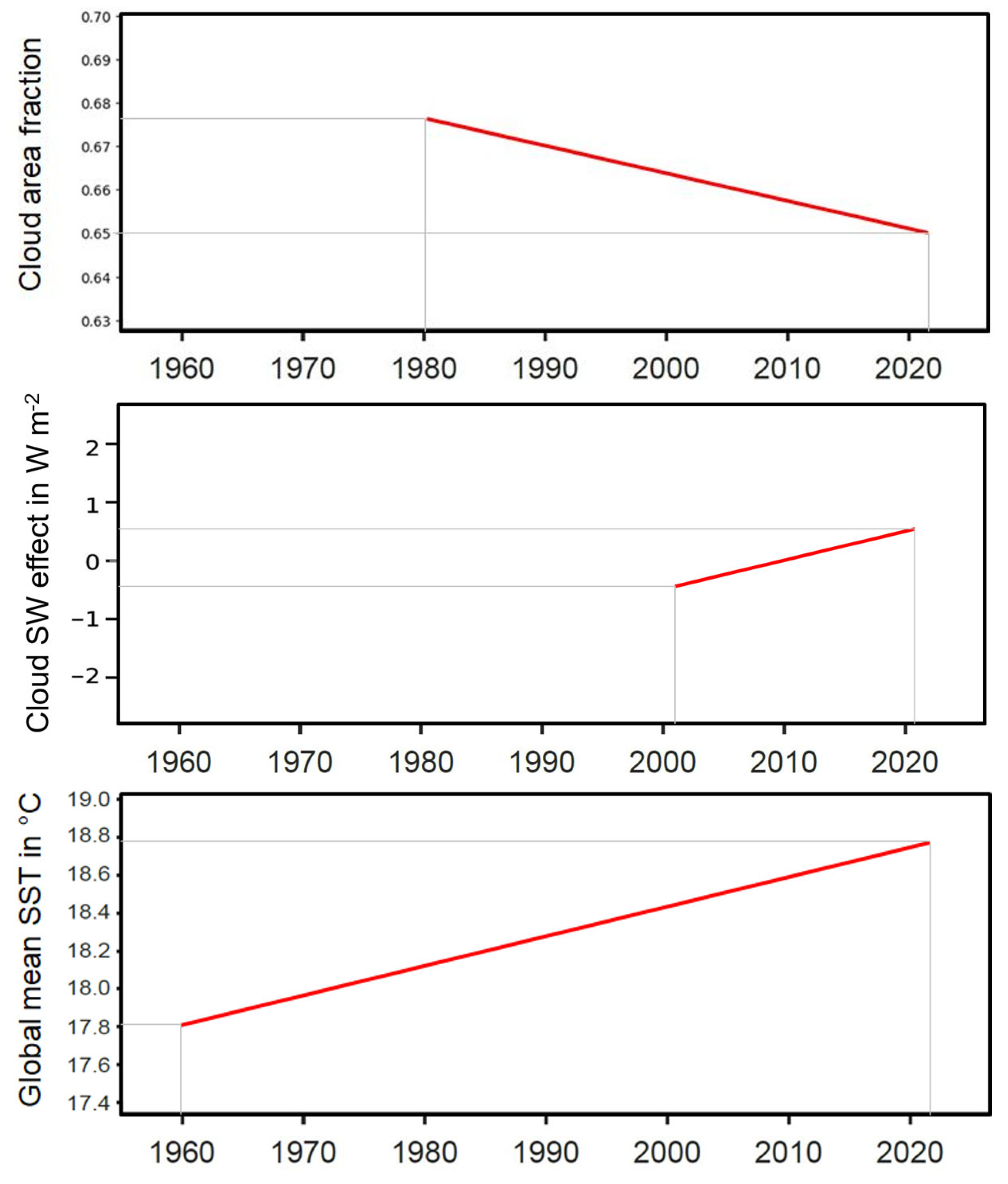
Through the last 70 years, the global mean sea surface temperature (SST) has increased from about 17.8 °C in 1955 to about 18.8 °C in 2023 (Cheng et al. 2024), see Figure 5 below, with an estimated linear trend of

$$t/°\mathrm{C}\approx 18.4+0.015\times \left(yr-2000\right).$$

(1)

Here, $yr$ is the number of the calendar year. Accordingly, depending on the dataset used for the analysis, the heat content of the upper 2000 m of the world ocean is currently increasing at a rate of either about 0.9 W m^-2 or 1.3 W m^-2 per ocean surface area (You 2024). These values are consistent with the total Earth energy imbalance exceeding 1 W m^-2 (Loeb et al. 2022) and the fact that the ocean alone stores 90 % of the globally accumulated heat (Cheng et al. 2024).

Recent ocean warming is strongest along the global west-wind belts, Figure 2, and is spatially correlated with regions of high mean cloud coverage, Figure 3.

On the input side of the ocean’s energy imbalance, the systematic global reduction of cloudiness is estimated by the linear trend (Foster et al. 2023),

$$C\approx 0.664-0.006\times \left(yr-2000\right),$$

(2)

Here, $C$ is defined by the fraction of the surface area covered by clouds. Evidently, this trend is negatively correlated with the tropospheric increase of temperature and specific humidity. Consistent with reduced cloudiness, between 1998 and 2017 Earth’s albedo declined by 0.5 W m^-2 (Goode et al. 2021; Feistel and Hellmuth 2021).

Decreased cloudiness has increased the mean solar irradiation, $∆J$, passing through clear-sky regions,

$$∆J/\left(\mathrm{W}{\mathrm{m}}^{-2}\right)\approx -0.41+0.037\times \left(yr-2000\right),$$

(3)

by about 0.74 W m^-2 in the past 20 years (Phillips and Foster 2023), see Figure 4 below. This phenomenon is known as the warming “shortwave cloud radiative effect” (SW CRE). Possibly, this value may explain the observed ocean warming if the ocean’s heat loss remains sufficiently below the SW CRE heat gain.

Figure 2. Observed upper 2000 m ocean heat content trend (i.e., energy difference divided by observation period) from 1958 to 2022 (WMO 2024). Image reproduction permitted by WMO Copyright. Image modified here by indicating regions 1, 2 and 3 of strongest cloudiness, see Figure 3.

Figure 2. Observed upper 2000 m ocean heat content trend (i.e., energy difference divided by observation period) from 1958 to 2022 (WMO 2024). Image reproduction permitted by WMO Copyright. Image modified here by indicating regions 1, 2 and 3 of strongest cloudiness, see Figure 3.

Figure 3. Global distribution of cloudiness July 2002 - April 2015. NASA Earth Observatory image by Jesse Allen and Kevin Ward, using data provided by the MODIS Atmosphere Science Team, NASA Goddard Space Flight Center. https://earthobservatory.nasa.gov/images/85843/cloudy-earth . Image reproduction permitted by NASA Copyright. Image modified here by adding a legend and indicating regions 1, 2 and 3 of strongest cloudiness for comparison with Figure 2.

Figure 3. Global distribution of cloudiness July 2002 - April 2015. NASA Earth Observatory image by Jesse Allen and Kevin Ward, using data provided by the MODIS Atmosphere Science Team, NASA Goddard Space Flight Center. https://earthobservatory.nasa.gov/images/85843/cloudy-earth . Image reproduction permitted by NASA Copyright. Image modified here by adding a legend and indicating regions 1, 2 and 3 of strongest cloudiness for comparison with Figure 2.

On the output side of the ocean’s energy imbalance, the sensible heat exchange of roughly 10 W m^-2 between ocean and atmosphere is relatively small and does not exceed the uncertainty of flux estimates (Josey et al. 2013). The ocean’s heat capacity exceeds that of the atmosphere by a factor of 1000, so that 1 % of added ocean’s heat content corresponds to an atmospheric temperature increase 10 times as large as that of the ocean. Sensible heat flux may be decreasing due to the faster rise of tropospheric temperatures compared to those of the sea. Estimates for such a trend are not available, though, and the quantitative contribution of sensible heat flow to ocean warming remains a pending problem.

Figure 4. The shortwave cloud radiative effect (SW CRE, blue curve) on solar irradiation has an increasing annual trend of 0.037 W m^-2 (dashed line). However, in the total cloud radiative effect (CRE, grey curve) this warming is almost completely cancelled against the cooling effect of reduced cloudiness on the ocean’s thermal radiation. Figure kindly provided by Coda Phillips (priv. comm. 10 Feb 2024). Note that this figure is slightly corrected in comparison to the original publication (Phillips and Foster 2023).

Figure 4. The shortwave cloud radiative effect (SW CRE, blue curve) on solar irradiation has an increasing annual trend of 0.037 W m^-2 (dashed line). However, in the total cloud radiative effect (CRE, grey curve) this warming is almost completely cancelled against the cooling effect of reduced cloudiness on the ocean’s thermal radiation. Figure kindly provided by Coda Phillips (priv. comm. 10 Feb 2024). Note that this figure is slightly corrected in comparison to the original publication (Phillips and Foster 2023).

On the output side of the ocean’s energy imbalance, the problem of ocean warming is closely related to a suspected change of the strength of the hydrological cycle. Cheng et al. (2024: p. 6) argue that the observed increase in salinity contrast in the upper 2000 m “is generally consistent with many atmosphere-based estimates and strengthens the evidence that the global water cycle has been amplified with global warming”. On the other hand, Held and Soden (2006: p. 5687-5689) had already emphasised that “it is important that the global-mean precipitation or evaporation, commonly referred to as the strength of the hydrological cycle, does not scale with Clausius–Clapeyron. … We can, alternatively, speak of the mean residence time of water vapor in the troposphere as increasing with increasing temperature”. In contrast to some model predictions (Weller et al. 2022), there is no evidence yet that mean global marine evaporation has intensified which in that case would have cooled the ocean in contrast to the observed warming in reality (Feistel and Hellmuth 2021, 2023; Blunden et al. 2023).

Quantitatively, corresponding to about 1200 mm of annual evaporation, the latent heat exchange of roughly 100 W m^-2 between ocean and atmosphere is the dominating way of oceanic heat loss and atmospheric energy supply (Randall 2012; Rapp 2014; Feistel and Hellmuth 2021). According to the Dalton equation, the evaporation flux depends mainly on the RH and the wind speed (Stewart 2008; Feistel and Hellmuth 2023, 2024). Trends in marine surface RH are weak and are, if at all, well below measurement uncertainty (Lovell-Smith et al. 2016; Dunn et al. 2023; Willett et al. 2023). “There is a tendency for the RH of the air to remain approximately constant as the climate changes” (Randall 2012: p. 147). Similarly, there is no significant trend in marine wind speeds (Azorin-Molina et al. 2023), so that relevant trends in global mean marine evaporation rates are neither expected nor observed (Held and Soden 2006). However, the high sensitivity of latent heat flux with respect to RH may be estimated to about 5 W m^-2 per %rh (Feistel and Hellmuth 2021), so that a small increase by 0.2 %rh may already suffice to explain the oceanic warming rate. Such a minor change of RH, however, could be disguised by the much larger observation uncertainty of 1 – 5 %rh (Lovell-Smith et al. 2016). “Relative humidity over oceans remained highly uncertain” (Willett et al. 2023: p. S49). Therefore, the relevance of latent heat with respect to ocean warming remains unclear.

Figure 5. Correlated, approximate linear long-term trends of observed global mean cloudiness (top, Foster et al. 2023), of solar irradiation (from Figure 4) and of global mean oceanic sea surface temperature (bottom, Chen et al. 2024).

Figure 5. Correlated, approximate linear long-term trends of observed global mean cloudiness (top, Foster et al. 2023), of solar irradiation (from Figure 4) and of global mean oceanic sea surface temperature (bottom, Chen et al. 2024).

On the output side of the ocean’s energy imbalance, the thermal radiation of the sea surface may easily be estimated from the Stefan-Boltzmann law of black-body radiation. Only a small fraction of this radiation may pass the troposphere in the so-called atmospheric window at about 8-11 µm wavelength, see Figure 6; most energy is blocked by the infrared opacity of clouds and greenhouse gases which send back down to the ocean long-wave radiation, according to their particular altitudes and temperatures. This “longwave cloud radiative effect” (LW CRE) of reduced cloudiness is cooling the ocean by letting more thermal radiation pass to space and emitting less downward radiation, Figure 7. It is estimated (Phillips and Foster 2023) that this cooling LW CRE almost completely cancels out the warming SW CRE of cloudiness, so that, following that analysis, ocean warming may be attributed to decreasing cloudiness only to a minor extent.

Figure 6. Spectral distribution of radiative opacity between 70 % and 85 % of the troposphere. This figure was prepared by Robert A. Rohde for the Global Warming Art project. Reproduction permitted by Creative-Commons Copyright, https://de.m.wikipedia.org/wiki/Datei:Atmospheric_Transmission.png.

Figure 6. Spectral distribution of radiative opacity between 70 % and 85 % of the troposphere. This figure was prepared by Robert A. Rohde for the Global Warming Art project. Reproduction permitted by Creative-Commons Copyright, https://de.m.wikipedia.org/wiki/Datei:Atmospheric_Transmission.png.

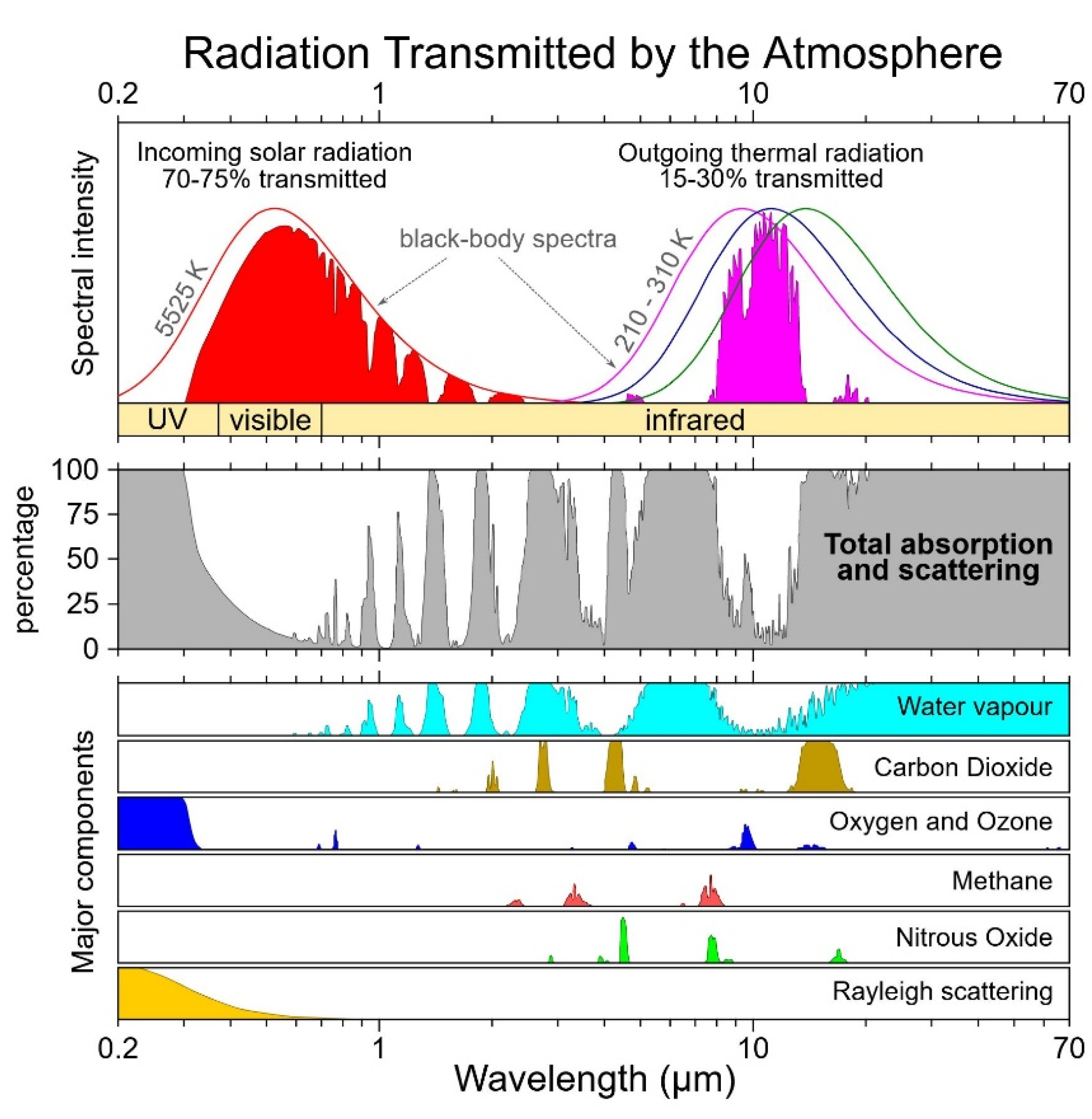
Figure 7. The longwave cloud radiative effect (LW CRE, orange curve) on thermal surface radiation has a decreasing annual trend of –0.038 W m^-2 (dashed line). However, in the total cloud radiative effect (CRE, grey curve) this cooling is almost completely cancelled against the warming effect of reduced cloudiness on solar irradiation. Figure kindly provided by Coda Phillips (priv. comm. 10 Feb 2024). Note that this figure is slightly corrected in comparison to the original publication (Phillips and Foster 2023).

Figure 7. The longwave cloud radiative effect (LW CRE, orange curve) on thermal surface radiation has a decreasing annual trend of –0.038 W m^-2 (dashed line). However, in the total cloud radiative effect (CRE, grey curve) this cooling is almost completely cancelled against the warming effect of reduced cloudiness on solar irradiation. Figure kindly provided by Coda Phillips (priv. comm. 10 Feb 2024). Note that this figure is slightly corrected in comparison to the original publication (Phillips and Foster 2023).

In addition to merely the lateral area covered by clouds, the mutual energy balance between SW and LW CRE is influenced also by other processes.

(i)

By contrast to the SW CRE, the LW CRE depends strongly on the concentration of greenhouse gases present in the cloudless sky. If the clear sky absorbs infrared radiation to a similar extent as the clouds do, the greenhouse effect will not decrease significantly by the substitution of a cloud fraction by a cloudless fraction. The increasing concentration of water vapour in the marine troposphere (Willett et al. 2023) results in a stronger absorption of longwave radiation (Goody and Robinson 1951; Goody 1952; Rothmann et al. 2004; Zhong and Haigh 2013; Feistel and Hellmuth 2021). The vertically distributed opacity of the clear-sky troposphere results in an effective radiating height of roughly 5000 m at 500 hPa, where the temperature is around 255 K (Colman and Soden 2021). According to Figure 5, the infrared opacity of the troposphere is between 70 % and 85 %. A rule-of-thumb estimate (Feistel and Hellmuth 2021) for the tropical marine infrared absorption coefficient of 71 % is consistent with that range. These values are relatively close to the opacity of clouds. “The clear-sky infrared absorption/emission is very important, so ideally the assumed value for the clear sky is calculated using a radiative transfer model … driven by reanalysis fields. Cloud radiative effect [as shown in Figure 7] is the difference between the all-sky observed and this modeled cloudless atmosphere” (Coda Phillips, priv. comm.).

(ii)

The SW CRE is relevant at day time only, while the LW CRE is acting all day and night. It is unclear weather the reported reduction of global cloudiness is different between day and night (Turbet et al. 2021) and may have distinct impacts on SW and LW CRE in that case.

(iii)

Cloudiness is most pronounced in the tropics and the west-wind bands (Figure 3). Moreover, the SW CRE is most relevant at low latitudes, while the LW CRE is acting all over the globe. It is unclear how the reported reduction of global cloudiness is correlated with latitude and may have different impacts on SW and LW CRE.

(iv)

Through the LCL, the increasing ocean SST has an effect on the altitude of cloud formation which is changing the cloud base temperature and, in turn, its downward thermal radiation. This feedback effect is analysed thermodynamically in Section 4 and Section 5 of this paper. In addition, low-level cumulus cloud formation is highly correlated with the diurnal cycle of solar irradiation, with latitude and with land-ocean distribution.

(v)

Through the LCL, a so far unnoticed minor increase of ocean surface RH may have an effect on the altitude of cloud formation which is changing the cloud base temperature and, in turn, its downward thermal radiation in opposite direction compared to the SST trend. This negative feedback effect is briefly quantified thermodynamically in Section 4.2.

3. TEOS-10 Equations of State

The world ocean is a vast and dynamically changing storage of heat, however, the theoretical description of its heat transport and conversion processes is thermodynamically challenging (McDougall 2003; Abraham et al. 2013; McDougall et al. 2013, 2021, 2023; Graham and McDougall 2013; Tailleux and Dubos 2024). For example, the former 1980 Equation of State of Seawater (EOS-80) did not provide any equations for the calculation of seawater entropy or enthalpy (Unesco 1981; Fofonoff and Millard 1983). In order to meet the growing demands of climate research and to support numerical circulation models of the ocean, starting in 2005 an improved comprehensive standard description of seawater thermodynamics had been developed by the SCOR/IAPSO Working Group 127 in cooperation with the International Association for the Properties of Water and Steam (Pawlowicz et al. 2012).

Proper axiomatic systems of mathematical statements possess the internal properties of independence, consistency and completeness. In contrast to any earlier collections of empirical seawater property equations, the International Thermodynamic Equation of Seawater – 2010 (TEOS-10) is designed in such an axiomatic manner and offers a comprehensive description of thermodynamic properties of water in its geophysical mixtures, phases and mutual equilibria. Thermodynamics has a reputation of being mathematically difficult. For beginners, though, an encouraging anecdote quoted by Fink (2009: p.1) is Arnold Sommerfeld’s experience with the use of the thermodynamic formalism: “Thermodynamics is a funny subject. The first time you go through it, you don’t understand it at all. The second time you go through it, you think you understand it, except for one or two points. The third time you go through it, you know you don’t understand it, but by that time you are so used to that subject, it doesn’t bother you anymore.” Appendix A provides a brief tutorial introduction into the mathematics used throughout TEOS-10. However, extensive software libraries are available from the web (www.teos-10.org), as outlined in the digital supplement of Wright et al. (2010), which conveniently provide direct access to numerical properties without detailed expertise to be required regarding sometimes demanding algorithms behind (Feistel et al. 2010a; Wright et al. 2010).

More than 150 years ago, in 1873, Willard Gibbs had discovered that a single mathematical function is sufficient to describe all thermodynamic properties of a given substance in its equilibrium state. Such functions are known as “thermodynamic potentials”. At its very core, TEOS-10 is mathematically defined (IOC et al. 2010) in terms of empirical correlation equations for just three thermodynamic potentials, namely,

(i)

the specific Gibbs energy of seawater, $g^{\mathrm{S}\mathrm{W}}\left(S,T,p\right)$, as a function of absolute salinity, $S$, absolute temperature, $T$, and absolute pressure, $p$, (Wagner and Pruß 2002; Millero et al. 2008; Feistel 2008),

(ii)

the specific Gibbs energy of ambient hexagonal ice Ih, $g^{\mathrm{I}\mathrm{h}}\left(T,p\right)$, as a function of temperature and pressure (Feistel and Wagner 2005, 2006), and

(iii)

the specific Helmholtz energy of humid air, $f^{\mathrm{A}\mathrm{V}}\left(A,T,\rho \right)$, as a function of the dry-air mass fraction, $A$, and the mass density of humid air, $\rho$ (Lemmon et al. 2000; Feistel et al. 2010b). From these potential functions, all thermodynamic properties of seawater, ice and humid air, as well as their mutual equilibria, can be derived mathematically in a perfectly consistent way by analytical or numerical means (IAPWS AN6-16 2016; Feistel 2018).

“Helmholtz energy” is sometimes also known as “free energy”, and “Gibbs energy “ as “free enthalpy” in the literature. In TEOS-10, temperature is expressed on the 1990 International Temperature Scale (ITS-90, Preston-Thomas 1990). In the vicinity of the triple point of water, ITS-90 deviates only insignificantly from the current Thermodynamic Temperature Scale (BIPM 2019) and will remain in practical use for the foreseeable future (Harvey et al. 2023). The isotopic composition of water is assumed to be that of Vienna Standard Mean Ocean Water (VSMOW, IAPWS G5-01 2016). Salinity $S$ of IAPSO Standard Seawater is defined by the Reference-Composition Salinity Scale (Millero et al. 2008) as the mass fraction of dissolved salt in seawater. The dry-air mass fraction $A$ of humid air is related to the specific humidity $q=1-A$, the mixing ratio $r$ and the water-vapour mole fraction $x$ by

$$\frac{1}{r}=\frac{1}{q}-1={\left(\frac{1}{A}-1\right)}^{-1}=\frac{M_{\mathrm{A}}}{M_{\mathrm{W}}}\left(\frac{1}{x}-1\right).$$

(4)

Here, $M_{\mathrm{W}}=\mathrm{18.015\; 268}\mathrm{g}{\mathrm{m}\mathrm{o}\mathrm{l}}^{-1}$ and $M_{\mathrm{A}}=\mathrm{28.965\; 46}\mathrm{g}{\mathrm{m}\mathrm{o}\mathrm{l}}^{-1}$ , respectively, are the molar masses of water and of dry air (IAPWS G08-10 2010). The freely adjustable constants for the absolute energies and entropies of water, sea salt and dry air are consistently defined at reference states chosen to be the triple point of water and the standard ocean state (Wagner and Pruß 2002; Feistel et al. 2008, 2010a,b; Feistel 2018).

The Gibbs function of freshwater, $g^{\mathrm{S}\mathrm{W}}\left(0,T,p\right)$, equals the Gibbs function of liquid water derived from the specific Helmholtz energy,$f^{\mathrm{F}}\left(T,\rho \right)$, of fluid water (Wagner and Pruß 2002) evaluated at high densities of liquid water,

$$g^{\mathrm{S}\mathrm{W}}\left(0,T,p\right)\equiv g^{\mathrm{W}}\left(T,p\right)=f^{\mathrm{F}}\left(T,\rho \right)+\frac{p}{\rho },$$

(5)

where the pressure is given by

$${p={\rho }^2\left(\frac{{\partial f}^{\mathrm{F}}}{\partial \rho }\right)}_T.$$

(6)

The specific entropy of seawater, ${\eta }^{\mathrm{S}\mathrm{W}}$, is available from the Gibbs function by

$${\eta }^{\mathrm{S}\mathrm{W}}=-{\left(\frac{{\partial g}^{\mathrm{S}\mathrm{W}}\left(S,T,p\right)}{\partial T}\right)}_{S,p},$$

(7)

and the specific enthalpy of seawater, $h^{\mathrm{S}\mathrm{W}}$, by

$$h^{\mathrm{S}\mathrm{W}}\left(S,{\eta }^{\mathrm{S}\mathrm{W}},p\right)=g^{\mathrm{S}\mathrm{W}}\left(S,T,p\right)+{\eta }^{\mathrm{S}\mathrm{W}}T.$$

(8)

This way, the ocean heat content, $OHC$, is estimated from the integral (McDougall et al. 2021),

$$OHC=\int \left[h^{\mathrm{S}\mathrm{W}}\left(S,{\eta }^{\mathrm{S}\mathrm{W}},p_0\right)-h_{\mathrm{S}\mathrm{O}}^{\mathrm{S}\mathrm{W}}\right]{\rho }^{\mathrm{S}\mathrm{W}}\left(S,{\eta }^{\mathrm{S}\mathrm{W}},p\right)\mathrm{d}V,$$

(9)

carried out over the ocean’s volume with its local in-situ properties $\left[S,{\eta }^{\mathrm{S}\mathrm{W}}\left(S,T,p\right),p\right]$. By definition (Feistel et al. 2008; IOC et al. 2010; Feistel 2018), the TEOS-10 enthalpy of the standard-ocean reference state is $h_{\mathrm{S}\mathrm{O}}^{\mathrm{S}\mathrm{W}}\equiv 0$. The local in-situ density ${\rho }^{\mathrm{S}\mathrm{W}}\left(S,{\eta }^{\mathrm{S}\mathrm{W}},p\right)$ is available from the relation

$$\frac{1}{{\rho }^{\mathrm{S}\mathrm{W}}}={\left(\frac{\partial h^{\mathrm{S}\mathrm{W}}}{\partial p}\right)}_{S,{\eta }^{\mathrm{S}\mathrm{W}}}.$$

(10)

Formula (9) describes a fictitious process by which each mass element, $\mathrm{d}m={\rho }^{\mathrm{S}\mathrm{W}}\mathrm{d}V$, in the volume $V$ is isentropically lifted to the surface pressure,$p_0$, where it transfers all its excess enthalpy, $h^{\mathrm{S}\mathrm{W}}\left(S,{\eta }^{\mathrm{S}\mathrm{W}},p_0\right)-h_{\mathrm{S}\mathrm{O}}^{\mathrm{S}\mathrm{W}}$, via sensible heat flux to an external measurement device. Subsequently, the same amount of heat is reversibly conducted back, after which the parcel returns to its original spatial position. Then, $OHC$ is the total amount of heat reversibly removed from the ocean across its surface by this process.

The approach of eq. (9) is therefore consistent with the fact that thermodynamically, heat is an exchange quantity rather than a state quantity, so that, rigorously spoken, any kind of stored “heat content” does not unambiguously exist but only an amount of heat which is exchanged between a given system and its surrounding (Landau and Lifschitz 1966: §13). According to Clausius (1876) and Gibbs (1873),

$$\mathrm{d}{h}^{\mathrm{S}\mathrm{W}}=T^{*}\mathrm{d}{\eta }^{\mathrm{S}\mathrm{W}}=c_p^{\mathrm{S}\mathrm{W}}\mathrm{d}{T}^{*}$$

(11)

is the proper thermodynamic expression for heat exchange at constant pressure and constant amounts of water and salt. Here, $T^{*}$ is the parcel’s changing surface temperature during the fictitious heat transfer process, and $c_p^{\mathrm{S}\mathrm{W}}$ is its isobaric heat capacity.

The Helmholtz function of dry air, $f^{\mathrm{A}\mathrm{V}}\left(1,T,\rho \right)$, equals (up to modified reference-state conditions) the equation of state of Lemmon et al. (2000),

$$f^{\mathrm{A}\mathrm{V}}\left(1,T,\rho \right)\equiv f^{\mathrm{A}}\left(T,\rho \right).$$

(12)

The Helmholtz function of pure water vapour, $f^{\mathrm{A}\mathrm{V}}\left(0,T,\rho \right)$, equals the specific Helmholtz energy,$f^{\mathrm{F}}\left(T,\rho \right)$, of fluid water (Wagner and Pruß 2002) evaluated at low densities of water vapour

$$f^{\mathrm{A}\mathrm{V}}\left(0,T,\rho \right)\equiv f^{\mathrm{F}}\left(T,\rho \right).$$

(13)

For the following calculations of condensation levels, the specific entropy of humid air, $\eta$, is available from the related Gibbs function

$$g^{\mathrm{A}\mathrm{V}}\left(A,T,p\right)=f^{\mathrm{A}\mathrm{V}}\left(A,T,\rho \right)+{\rho \left(\frac{{\partial f}^{\mathrm{A}\mathrm{V}}}{\partial \rho }\right)}_{A,T}$$

(14)

by

$$\eta =-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T,p\right)}{\partial T}\right)}_{A,p}.$$

(15)

Note that derived from (14) for atmospheric applications, a simplified analytical low-pressure formulation for the Gibbs function $g^{\mathrm{A}\mathrm{V}}$ is available from Appendix B of Feistel and Hellmuth (2023).

The specific enthalpy of humid air is computed from the Gibbs function as

$$h^{\mathrm{A}\mathrm{V}}\left(A,\eta ,p\right)=g^{\mathrm{A}\mathrm{V}}\left(A,T,p\right)+\eta T.$$

(16)

For the computation of phase equilibria such as dew- or frost-points, TEOS-10 provides the chemical potentials of water in the different phases or mixtures.

The chemical potential of water vapour, ${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}$, in humid air is available from the Gibbs function by

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}=g^{\mathrm{A}\mathrm{V}}-A{\left(\frac{g^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p},$$

(17)

or equivalently from the enthalpy by, see eq. (A26),

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}=h^{\mathrm{A}\mathrm{V}}-\eta {\left(\frac{{\partial h}^{\mathrm{A}\mathrm{V}}}{\partial \eta }\right)}_{A,p}-A{\left(\frac{{\partial h}^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{\eta ,p}.$$

(18)

The chemical potential of liquid water equals its specific Gibbs energy,

$${\mu }_{\mathrm{W}}^{\mathrm{W}}=g^{\mathrm{W}}\left(T,p\right),$$

(19)

and, similarly, the chemical potential of ice is,

$${\mu }_{\mathrm{W}}^{\mathrm{I}\mathrm{h}}=g^{\mathrm{I}\mathrm{h}}\left(T,p\right).$$

(20)

The dry-air mass fraction, $A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right)$, of humid air saturated with respect to liquid water is defined by the equilibrium condition

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}},T,p\right)={\mu }_{\mathrm{W}}^{\mathrm{W}}\left(T,p\right),$$

(21)

and with respect to ice by

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}},T,p\right)={\mu }_{\mathrm{W}}^{\mathrm{I}\mathrm{h}}\left(T,p\right).$$

(22)

To make the use of TEOS-10 equations more convenient, the numerical solutions of eqs. (21) and (22) for either $A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right)$, $T\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}},p\right)$, or $p\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}},T\right)$, together with numerous other properties (Feistel et al. 2010a; Wright et al. 2010), are implemented in the freely available from the open source code, sea-ice-air (SIA) library of TEOS-10, accessible at www.teos-10.org.

After the official adoption of TEOS-10, the IAPSO/SCOR/IAPWS Joint Committee on the Properties of Seawater (JCS) continued to address pending climate-related problems (Feistel et al. 2016) that could have not yet or only insufficiently been addressed by TEOS-10. Among those is the ambiguity and mutual inconsistency of different definitions of RH in practical use, such as between meteorology and climatology (Lovell-Smith et al. 2016). With respect to deviations from water phase equilibria such as saturation properties, the real-gas equivalent of conventional RH is the relative fugacity (RF) (Feistel et al. 2010b: eq. (10.10) therein, Feistel and Lovell-Smith 2017),

$${\psi }_f\left(A,T,p\right)\equiv \exp \left\{\frac{{\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A,T,p\right)-{\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right),T,p\right)}{R_{\mathrm{W}}T}\right\}.$$

(23)

Here, $R_{\mathrm{W}}=R/M_{\mathrm{W}}$ is the specific gas constant of water, and $R$ is the universal molar gas constant. As a function of $\left(T,p\right)$, the chemical potential at saturation, ${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}},T,p\right)$, may be expressed by means of either eq. (21) or (22). Note that eq. (23) is valid only for temperatures below the boiling point of water at the given pressure (Feistel and Lovell-Smith 2017), a condition which is naturally fulfilled under ambient geophysical conditions.

In ideal-gas approximation, RF is identical with conventional RH in terms of water-vapour partial pressures. For air close to saturation, such as the marine surface layer with about 80 %rh, the Clausius-Clapeyron formula is an excellent approximation of TEOS-10 values for RF, even if refraining from the perfect-gas assumption (Feistel et al. 2022). RF appears naturally in expressions for the Onsager driving force of non-equilibrium fluxes, such as evaporation from the ocean surface (Feistel and Hellmuth 2023, 2024). For the numerical computation of RF, a source code extension to the SIA library is available from Feistel et al. (2022).

Mathematical transformation between different thermodynamic quantities and partial derivatives with respect to alternative independent variables, such as frequently exploited in TEOS-10 to derive the desired functional dependencies, may conveniently and error-free be executed by means of the formal Jacobi method developed by Norman Shaw (1935), as briefly described in Appendix A.

4. Isentropically Lifted Condensation Level (LCL)

“Synoptic weather observations from ships throughout the World Ocean have been analyzed to produce a climatology of total cloud cover and the amounts of nine cloud types. … Among the cloud types, the most widespread and consistent relationship is found for the extensive marine stratus and stratocumulus clouds” (Eastman et al. 2011: p. 5914). Subtropical marine clouds show a pronounced “transition between unbroken sheets of stratocumulus and fields of scattered cumulus“ (Sandu et al. 2010). The cumulus cloud base is typically observed below 500 m height, as shown in Figure 1. For the climatic feedback effect of marine clouds, the lifted condensation level (LCL) is a key parameter that describes the pressure (or altitude) at which an isentropically ascending parcel reaches its dew- or frost-point, starting from given values of temperature and RH, such as at the sea surface. A typical empirical equation for the LCL height, $z_{\mathrm{L}\mathrm{C}\mathrm{L}}$, is

$$z_{\mathrm{L}\mathrm{C}\mathrm{L}}-z={\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}\left(T-T_{\mathrm{d}\mathrm{p}}\right),$$

(24)

where $z$, $T$ and $T_{\mathrm{d}\mathrm{p}}$, respectively, are the initial values of the parcel’s height, in-situ and dew-point temperature. Commonly, the latter is related to the saturation vapour pressure and the RH by the Clausius-Clapeyron formula. Previous estimates for the coefficient ${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}$ range widely between $123\mathrm{m}{\mathrm{K}}^{-1}$ and $165\mathrm{m}{\mathrm{K}}^{-1}$ (Romps 2017), with an optimum of $125\mathrm{m}{\mathrm{K}}^{-1}$ suggested by Lawrence (2005). Also, nonlinear mathematical expressions, more complicated than eq. (24), had been suggested in the literature, derived from varying approximate thermodynamic relations for humid air, liquid water and ice. Often, such relations are chosen according to the authors’ personal preferences and are scarcely supported by internationally agreed standard formulations. In Section 4.2 below, for typical values of the marine troposphere, selected highly accurate TEOS-10 results for ${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}$ range between 125 and 129 $\mathrm{m}{\mathrm{K}}^{-1}$.

Humid air at the sea surface has a typical climatological RH of about 80 %rh, a value that may vary seasonally or regionally between 70 %rh and 90 %rh, but appears likely to be only very weakly affected by global warming, if at all (Thomas and Stamnes 1999; Liou 2002; Dai 2006; Randall 2012; Rapp 2014; Blunden 2023). Assuming that an air parcel starts ascending at constant specific humidity, $A=A_0=\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{s}\mathrm{t}$, from the sea surface at the air pressure, $p_0$, and the sea surface temperature, $T_0$, the value of the initial air fraction $A_0$ can be computed in implicit form from the sea surface relative fugacity, ${\psi }_f={\psi }_f\left(A_0,T_0,p_0\right)$, using eq. (23), by

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A_0,T_0,p_0\right)=g^{\mathrm{W}}\left(T_0,p_0\right)+R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(25)

Here, surface values of independent variables are indicated by the subscript 0 which may be dropped at constant $A$ for simplicity. Similarly, the subscript LCL is used for those properties at the lifted condensation state. Associated function values such as ${\psi }_f$ or $g^{\mathrm{W}}$ are without state-dependent subscript as long as there is no risk of confusion.

The tropospheric entropy, assumed to remain constant during the uplift, can be expressed by eq. (15),

$$\eta ={\eta }_0=-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}.$$

(26)

At the LCL, the entropy value,

$$\eta ={\eta }_{\mathrm{L}\mathrm{C}\mathrm{L}}=-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p},$$

(27)

needs to be the same as at the surface, eq. (26), and may be eliminated from the mathematical problem by equating eq. (26) with (27),

$${\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p}.$$

(28)

The LCL pressure, $p_{\mathrm{L}\mathrm{C}\mathrm{L}}$, is approached when at fixed values of $\left(A,\eta \right)=\left(A_0,{\eta }_0\right)$ the temperature has dropped to the dew-point, $T_{\mathrm{L}\mathrm{C}\mathrm{L}}$, eq. (21), that means,

$${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)=g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right).$$

(29)

The chemical potential of water vapour in humid air, ${\mu }_{\mathrm{V}}^{\mathrm{A}\mathrm{V}}$, may be expressed by the related Gibbs function, eq. (17),

$$g^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-A{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A}\right)}_{T,p}=g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right).$$

(30)

If the initial value of RH is given, rather than the initial specific humidity, $q=1-A$, then eq. (25) may be added to the system in the form of

$$g^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)-A{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A}\right)}_{T,p}=g^{\mathrm{W}}\left(T_0,p_0\right)+R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(31)

Eqs. (28), (30) and (31) constitute a closed system of three nonlinear implicit equations for the three unknowns $\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)$ as functions of the given input values $\left({\psi }_f,T_0,p_0\right)$. These thermodynamic relations are the exact conditions for the LCL properties with the only minor approximation that the dissolution of air in liquid water, influencing the saturation state, is neglected. This system may be solved iteratively using the TEOS-10 functions implemented in the SIA library, see Appendix B, or it may be exploited analytically after introducing quantitatively reasonable approximations.

4.1. Numerical Iterative Solution

The system of equations, (28) and (30), for the calculation of $T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}$ from $A$, and of eq. (31) for the determination of $A$ from the given surface values of $T_0,p_0$ and the relative fugacity, can be solved iteratively, with highest accuracy currently available. Expanding eq. (31) into a power series up to linear terms in the increment, $∆A$, of a starting estimate, $A$,

$$g^{\mathrm{A}\mathrm{V}}\left(A+∆A,T_0,p_0\right)-\left(A+∆A\right){\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A+∆A,T_0,p_0\right)}{\partial A}\right)}_{T,p}=g^{\mathrm{W}}\left(T_0,p_0\right)+R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(32)

leads to the solution for the improvement, $A_{i+1}=A_i+∆A_i$, of iteration step $i$,

$$∆A_i=\frac{g^{\mathrm{A}\mathrm{V}}\left(A_i,T_0,p_0\right)-A_i{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A_i,T_0,p_0\right)}{\partial A}\right)}_{T,p}-g^{\mathrm{W}}\left(T_0,p_0\right)-R_{\mathrm{W}}{T}_0\ln {\psi }_f}{A_i{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A_i,T_0,p_0\right)}{\partial A^2}\right)}_{T,p}}.$$

(33)

Practically, this Newton-Cotes iteration may start from almost dry air, such as $A_0=0.999999$.

In a similar manner, the other increments $∆T_{\mathrm{L}\mathrm{C}\mathrm{L}},∆p_{\mathrm{L}\mathrm{C}\mathrm{L}}$ follow from eqs. (28) and (30), in matrix notation,

$$\left(\begin{array}{cc}{a}_{11}& a_{12}\\ a_{21}& a_{22}\end{array}\right)\left(\begin{array}{c}∆T_{\mathrm{L}\mathrm{C}\mathrm{L}}\\ ∆p_{\mathrm{L}\mathrm{C}\mathrm{L}}\end{array}\right)=\left(\begin{array}{c}{b}_1\\ b_2\end{array}\right).$$

(34)

By Cramer’s rule, the solution is

$$∆T_{\mathrm{L}\mathrm{C}\mathrm{L}}=\frac{\left|\begin{array}{cc}{b}_1& a_{12}\\ b_2& a_{22}\end{array}\right|}{\left|\begin{array}{cc}{a}_{11}& a_{12}\\ a_{21}& a_{22}\end{array}\right|}=\frac{b_1{a}_{22}-b_2{a}_{12}}{a_{11}{a}_{22}-a_{21}{a}_{12}}$$

(35)

and

$$∆p_{\mathrm{L}\mathrm{C}\mathrm{L}}=\frac{\left|\begin{array}{cc}{a}_{11}& b_1\\ a_{21}& b_2\end{array}\right|}{\left|\begin{array}{cc}{a}_{11}& a_{12}\\ a_{21}& a_{22}\end{array}\right|}=\frac{a_{11}{b}_2-a_{21}{b}_1}{a_{11}{a}_{22}-a_{21}{a}_{12}}.$$

(36)

The coefficients of this system are

$$a_{11}={\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T^2}\right)}_{A,p},$$

(37)

$$a_{12}={\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T\partial p}\right)}_{A,p},$$

(38)

$$a_{21}={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p}-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A\partial T}\right)}_p-{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_p,$$

(39)

$$a_{22}={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial p}\right)}_{A,T}-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A\partial p}\right)}_T-{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial p}\right)}_T,$$

(40)

$$b_1={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p}$$

(41)

$$b_2=g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-g^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)+A{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A}\right)}_{T,p}.$$

(42)

All derivatives required here are numerically available from the TEOS-10 SIA library (Wright et al. 2010).

Table 1 reports selected LCL states computed from TEOS-10, iteratively solving eqs. (34), (35), (36) at $p_0=1013.25\mathrm{h}\mathrm{P}\mathrm{a}$ and ${\psi }_f=80\%\mathrm{r}\mathrm{h}$. Results rounded to 6 digits. The climatic warming trend moves LCL to higher altitudes ($p_{\mathrm{L}\mathrm{C}\mathrm{L}}$) where the temperature difference between ocean ($T_0$) and cloud base ($T_{\mathrm{L}\mathrm{C}\mathrm{L}}$) gets larger and consequently, the downward radiative heat flux becomes reduced as compared to (fictitious) lower clouds. This cooling effect is a negative, stabilising feedback of the cloud cover, slowing down ocean warming. The observed shrinking cloudiness, in turn, likely renders this cooling influence of clouds globally smaller.

4.2. Linear Analytical Approximation

If the initial temperature, $T_0$, is only slightly higher than the dew point, $T_{\mathrm{d}\mathrm{p}}$, such as typically at the ocean surface, the LCL pressure may be estimated from TEOS-10 equations linearised in the small differences $\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}}-T_0\right)$ and $\left(p_{\mathrm{L}\mathrm{C}\mathrm{L}}-p_0\right)$. Doing this, the relative fugacity may be very accurately expressed by the Clausius-Clapeyron equation for the dew point (Feistel and Hellmuth 2022: eq. 14 therein),

$$R_{\mathrm{W}}{T}_0\ln {\psi }_f\approx L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)\left(1-\frac{T_0}{T_{\mathrm{d}\mathrm{p}}}\right).$$

(43)

Here, $L_{\mathrm{L}}$ is the specific evaporation enthalpy of liquid water at equilibrium with (saturated) humid air. Its value is available from the thermodynamically rigorous relations (Feistel et al. 2010b; Feistel and Hellmuth 2022: eqs. C.5 and C.6 therein),

$$\frac{L_{\mathrm{L}}\left(T,p\right)}{T}={\eta }^{\mathrm{A}\mathrm{V}}\left(A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right),T,p\right)-A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right){\left(\frac{{\partial \eta }^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-{\eta }^{\mathrm{W}}\left(T,p\right),$$

(44)

which is equivalent to

$$\frac{L_{\mathrm{L}}\left(T,p\right)}{T}=-A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T,p\right){\left(\frac{{\partial A}^{\mathrm{s}\mathrm{a}\mathrm{t}}}{\partial T}\right)}_p{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}}{\partial A^2}\right)}_{T,p}.$$

(45)

Here, ${\eta }^{\mathrm{A}\mathrm{V}}$ and ${\eta }^{\mathrm{W}}$, respectively, are the specific entropies of humid air and liquid water.

Eq. (43) represents the initial linear term of a truncated Taylor series with respect to powers of $\left(T_0-T_{\mathrm{d}\mathrm{p}}\right)$, regardless of whether ideal-gas assumptions have been applied or not.

A similar series expansion of the Gibbs function of liquid water in eq. (30) with respect to $∆T\equiv \left(T_{\mathrm{L}\mathrm{C}\mathrm{L}}-T_0\right)$ and $∆p\equiv \left(p_{\mathrm{L}\mathrm{C}\mathrm{L}}-p_0\right)$ gives

$$g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)\approx g^{\mathrm{W}}\left(T_0,p_0\right)+{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_0,p_0\right)}{\partial T}\right)}_p∆T+{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_0,p_0\right)}{\partial p}\right)}_T∆p.$$

(46)

The expansion coefficients are the specific entropy, ${\eta }^{\mathrm{W}}$, and the specific volume, $v^{\mathrm{W}}$, of liquid water at $\left(T_0,p_0\right)$,

$${∆g}^{\mathrm{W}}\equiv g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-g^{\mathrm{W}}\left(T_0,p_0\right)\approx -{\eta }^{\mathrm{W}}∆T+v^{\mathrm{W}}∆p.$$

(47)

A similar expansion about $\left(T_0,p_0\right)$ can be carried out for the Gibbs function of humid air,

$${∆g}^{\mathrm{A}\mathrm{V}}\equiv g^{\mathrm{A}\mathrm{V}}\left({A,T}_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-g^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)\approx -{\eta }^{\mathrm{A}\mathrm{V}}∆T+v^{\mathrm{A}\mathrm{V}}∆p.$$

(48)

Subtracting eq. (31) from (30) results in

$${∆g}^{\mathrm{A}\mathrm{V}}-A{\left(\frac{{\partial ∆g}^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-{∆g}^{\mathrm{W}}=-R_{\mathrm{W}}{T}_0\ln {\psi }_f,$$

(49)

which may by expressed in terms of the linear offsets (47), (48), as

$$\left[{\eta }^{\mathrm{A}\mathrm{V}}-A{\left(\frac{{\partial \eta }^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-{\eta }^{\mathrm{W}}\right]∆T-\left[{v^{\mathrm{A}\mathrm{V}}-A{\left(\frac{{\partial v}^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-v}^{\mathrm{W}}\right]∆p=R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(50)

Up to small terms linear in the subsaturation, $A-A^{\mathrm{s}\mathrm{a}\mathrm{t}}\left(T_0,p_0\right)$, at the surface, the coefficient of $∆T$ equals the specific evaporation entropy, eq. (44),

$$\frac{L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)}{T_{\mathrm{d}\mathrm{p}}}=∆{\eta }_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)\approx {\eta }^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)-A{\left(\frac{{\partial \eta }^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-{\eta }^{\mathrm{W}}\left(T_0,p_0\right)$$

(51)

and the term in front of $∆p$ equals approximately the specific evaporation volume (Feistel et al. 2010b),

$$∆v_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)\approx v^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)-A{\left(\frac{{\partial v}^{\mathrm{A}\mathrm{V}}}{\partial A}\right)}_{T,p}-v^{\mathrm{W}}\left(T_0,p_0\right).$$

(52)

Still in linear approximation with respect to the LCL pressure and temperature offset, eq. (50) then reads

$$\frac{L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)}{T_{\mathrm{d}\mathrm{p}}}∆T-∆v_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)∆p=R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(53)

During adiabatic uplift, temperature and pressure change are mutually related by the moist lapse rate, eq. (A18),

$$∆T=\mathsf{\Gamma }∆p.$$

(54)

Similar to eq. (24), the final formula (53) for the LCL pressure is therefore, making also use of eq. (43),

$$p_{\mathrm{L}\mathrm{C}\mathrm{L}}-p_0=\frac{L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)\left(1-\frac{T_0}{T_{\mathrm{d}\mathrm{p}}}\right)}{\frac{L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)}{T_{\mathrm{d}\mathrm{p}}}\mathsf{\Gamma }-∆v_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)}=-\frac{{T_0-T}_{\mathrm{d}\mathrm{p}}}{\mathsf{\Gamma }\left(T_{\mathrm{d}\mathrm{p}},p_0\right)+{\mathsf{\Gamma }}_{\mathrm{d}\mathrm{p}}\left(T_{\mathrm{d}\mathrm{p}},p_0\right)}.$$

(55)

Here, the lapse rate of the dew-point is negative, see Appendix B, eq. (B5),

$${\mathsf{\Gamma }}_{\mathrm{d}\mathrm{p}}={\left(\frac{\partial T_{\mathrm{d}\mathrm{p}}}{\partial p}\right)}_A=-\frac{T_{\mathrm{d}\mathrm{p}}∆v_{\mathrm{L}}}{L_{\mathrm{L}}\left(T_{\mathrm{d}\mathrm{p}},p\right)}.$$

(56)

The hydrostatic balance equation (height $z$, gravity constant $g_{\mathrm{E}}=9.81\mathrm{m}{\mathrm{s}}^{-2}$),

$$v^{\mathrm{A}\mathrm{V}}\mathrm{d}p=-g_{\mathrm{E}}\mathrm{d}z,$$

(57)

can be integrated along an isentropic vertical profile, due to the thermodynamic relation,

$$v^{\mathrm{A}\mathrm{V}}={\left(\frac{\partial h^{\mathrm{A}\mathrm{V}}}{\partial p}\right)}_{A,\eta },$$

(58)

to give

$$z_{\mathrm{L}\mathrm{C}\mathrm{L}}-z_0=-\frac{1}{g_{\mathrm{E}}}\left[h^{\mathrm{A}\mathrm{V}}\left(A,\eta ,p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-h^{\mathrm{A}\mathrm{V}}\left(A,\eta ,p_0\right)\right].$$

(59)

This enthalpy difference may be used to estimate the coefficient of the LCL height, eq. (24), from the LCL pressure, through

$${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}\equiv \frac{z_{\mathrm{L}\mathrm{C}\mathrm{L}}-z_0}{T_0-T_{\mathrm{d}\mathrm{p}}}.$$

(60)

Rigorous numerical TEOS-10 results for $\left(z_{\mathrm{L}\mathrm{C}\mathrm{L}}-z_0\right)$ and ${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}$ from eqs. (59) and (60) at selected typical marine conditions, obtained through iteratively solving eqs. (34), (35), (36) are reported in Table 2 and Table 3.

A linear approximation based on the LCL pressure, eq. (55), is

$${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}\approx -\frac{v^{\mathrm{A}\mathrm{V}}}{g_{\mathrm{E}}}\frac{\left(p_{\mathrm{L}\mathrm{C}\mathrm{L}}-p\right)}{\left(T-T_{\mathrm{d}\mathrm{p}}\right)}\approx \frac{v^{\mathrm{A}\mathrm{V}}}{g_{\mathrm{E}}\left(\mathsf{\Gamma }+{\mathsf{\Gamma }}_{\mathrm{d}\mathrm{p}}\right)}.$$

(61)

In Table 3, in addition to the calculated LCL coefficients, also the climatic feedback to possible RH trends is reported as the thermal radiation flux, ${\sigma }_{\mathrm{S}\mathrm{B}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}}^4$, downward from the cloud base, which tends to warm the ocean if RH is rising.

If in eq. (52) the specific volume of humid air is much larger that the remaining terms of the evaporation volume, $∆v_{\mathrm{L}}\approx v^{\mathrm{A}\mathrm{V}}$, the simplified LCL coefficient given by eq. (61) is, expressing the lapse rate by eq. (A18), $\mathsf{\Gamma }={\alpha }^{\mathrm{A}\mathrm{V}}{v}^{\mathrm{A}\mathrm{V}}T/c_p^{\mathrm{A}\mathrm{V}}$, in terms of the thermal expansion coefficient, ${\alpha }^{\mathrm{A}\mathrm{V}}$, and the specific isobaric heat capacity, $c_p^{\mathrm{A}\mathrm{V}}$, of humid air, by virtue of eq. (56) and $T\approx T_{\mathrm{d}\mathrm{p}}$,

$${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}\approx {\left[g_{\mathrm{E}}{T}_{\mathrm{d}\mathrm{p}}\left(\frac{{\alpha }^{\mathrm{A}\mathrm{V}}}{c_p^{\mathrm{A}\mathrm{V}}}-\frac{1}{L_{\mathrm{L}}}\right)\right]}^{-1}.$$

(62)

In ideal-gas approximation, ${\alpha }^{\mathrm{A}\mathrm{V}}\approx 1/T_{\mathrm{d}\mathrm{p}}$, this becomes

$${\gamma }_{\mathrm{L}\mathrm{C}\mathrm{L}}\approx {\left[g_{\mathrm{E}}\left(\frac{1}{c_p^{\mathrm{A}\mathrm{V}}}-\frac{1}{∆{\eta }_{\mathrm{L}}}\right)\right]}^{-1}.$$

(63)

Here, $∆{\eta }_{\mathrm{L}}=L_{\mathrm{L}}/T_{\mathrm{d}\mathrm{p}}$ is the evaporation entropy of liquid water, eq. (51).

Analytical low-pressure approximations for the TEOS-10 Gibbs functions of liquid water and humid air are provided by Feistel and Hellmuth (2023: Appendix A therein) and in extremely simplified, crude form in Appendix C of this paper, as successfully used in the following section.

4.3. Clausius-Clapeyron Expansion

In the context of global warming, the Clausius-Clapeyron equation for the saturated vapour pressure of water has become a widely exploited and sufficiently accurate approximation for estimating the increasing humidity of the troposphere. Here, an expression for the LCL is derived with a similar accuracy from the TEOS-10 equations.

Eqs. (28), (30) and (31) constitute a closed system of three nonlinear implicit equations for the three unknowns $\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)$ as functions of the given input values $\left({\psi }_f,T_0,p_0\right)$. These relations may be formulated in terms of “crude” approximations, taking all heat capacities as constant, liquids as incompressible, and gases as perfect gases. Related crude Gibbs functions, ${\tilde{g}}^{\mathrm{W}}\left(T,p\right)$ and ${\tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T,p\right)$, are indicated here by tildes and are provided in Appendix C.

Using those Gibbs functions, the conservation of entropy during adiabatic ascent, eq. (28),

$${\left(\frac{{\partial \tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}={\left(\frac{{\partial \tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p},$$

(64)

takes the form of the conventional adiabatic equation of state,

$${\tilde{c}}_p^{\mathrm{A}\mathrm{V}}\ln \frac{T_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}=R_{\mathrm{A}\mathrm{V}}\ln \frac{p_0}{p_{\mathrm{L}\mathrm{C}\mathrm{L}}}.$$

(65)

Here, ${\tilde{c}}_p^{\mathrm{A}\mathrm{V}}\left(A\right)\equiv \left(1-A\right){\tilde{c}}_p^{\mathrm{V}}+A{\tilde{c}}_p^{\mathrm{A}}$ and $R_{\mathrm{A}\mathrm{V}}\left(A\right)\equiv \left(1-A\right)R_{\mathrm{W}}+A{R}_{\mathrm{A}}$, respectively, are the heat capacity and the specific gas constant of humid air.

The saturation of air at the LCL, eq. (30), expresses equation chemical potentials of water in the gas and the liquid phase,

$${\tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-A{\left(\frac{{\partial \tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A}\right)}_{T,p}={\tilde{g}}^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right).$$

(66)

In crude approximation, this condition reads

$$\left({\tilde{c}}_p^{\mathrm{W}}-{\tilde{c}}_p^{\mathrm{V}}\right)T_{\mathrm{L}\mathrm{C}\mathrm{L}}\ln \frac{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}{T_{\mathrm{t}}}+R_{\mathrm{W}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}}\ln \frac{x{p}_{\mathrm{L}\mathrm{C}\mathrm{L}}}{p_{\mathrm{t}}}={\tilde{g}}_0^{\mathrm{W}}+{\tilde{g}}_1^{\mathrm{W}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}}+\frac{p_{\mathrm{L}\mathrm{C}\mathrm{L}}}{{\tilde{\rho }}^{\mathrm{W}}}.$$

(67)

Here, $T_{\mathrm{t}}$ and $p_{\mathrm{t}}$, respectively, are temperature and pressure at the triple point of water, and ${\tilde{\rho }}^{\mathrm{W}}$ is the density of liquid water. They appear in this context because that triple point is used as the reference state at which the arbitrary constants ${\tilde{g}}_0^{\mathrm{W}}$ and ${\tilde{g}}_1^{\mathrm{W}}$ have been adjusted to the phase equilibrium properties of fluid water, computed from TEOS-10, see Appendix C.

Finally, the relative fugacity (or RH) at the surface is specified by eq. (31) in terms of crude Gibbs functions

$${\tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)-A{\left(\frac{{\partial \tilde{g}}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A}\right)}_{T,p}={\tilde{g}}^{\mathrm{W}}\left(T_0,p_0\right)+R_{\mathrm{W}}{T}_0\ln {\psi }_f.$$

(68)

Inserting the crude equations given in Appendix C, results in

$$\left({\tilde{c}}_p^{\mathrm{W}}-{\tilde{c}}_p^{\mathrm{V}}\right)T_0\ln \frac{T_0}{T_{\mathrm{t}}}+R_{\mathrm{W}}{T}_0\ln \frac{{xp}_0}{{{\psi }_{f}p}_{\mathrm{t}}}={\tilde{g}}_0^{\mathrm{W}}+{\tilde{g}}_1^{\mathrm{W}}{T}_0+\frac{p_0}{{\tilde{\rho }}^{\mathrm{W}}}.$$

(69)

From the latter equation, the vapour mole fraction at the surface, $x\left({\psi }_f,T_0,p_0\right)$, is obtained from the relative fugacity, ${\psi }_f$,

$$x\left({\psi }_f,T_0,p_0\right)={\psi }_f\frac{p_{\mathrm{t}}}{p_0}\exp \left\{\frac{{\tilde{g}}_0^{\mathrm{W}}+{\tilde{g}}_1^{\mathrm{W}}{T}_0}{R_{\mathrm{W}}{T}_0}+\frac{p_0}{R_{\mathrm{W}}{T}_0{\tilde{\rho }}^{\mathrm{W}}}-\frac{\left({\tilde{c}}_p^{\mathrm{W}}-{\tilde{c}}_p^{\mathrm{V}}\right)}{R_{\mathrm{W}}}\ln \frac{T_0}{T_{\mathrm{t}}}\right\}.$$

(70)

From $x$, the dry-air mass fraction $A$ is available through eq. (4).

Subtracting eq. (67) from (69) and inserting entropy conservation (65) as

$$\ln \frac{p_{\mathrm{L}\mathrm{C}\mathrm{L}}}{p_{\mathrm{t}}}=\ln \frac{p_0}{p_{\mathrm{t}}}+\frac{{\tilde{c}}_p^{\mathrm{A}\mathrm{V}}}{R_{\mathrm{A}\mathrm{V}}}\ln \frac{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}{T_0},$$

(71)

the saturation equation (68) can be written in the form

$$Q\ln \frac{T_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}-\ln {\psi }_f=\frac{{\tilde{g}}_0^{\mathrm{W}}}{R_{\mathrm{W}}}\left(\frac{1}{T_0}-\frac{1}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}\right)+\frac{1}{{\tilde{\rho }}^{\mathrm{W}}{R}_{\mathrm{W}}}\left(\frac{p_0}{T_0}-\frac{p_{\mathrm{L}\mathrm{C}\mathrm{L}}}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}\right).$$

(72)

Here, the abbreviation

$$Q\equiv \frac{{\tilde{c}}_p^{\mathrm{W}}-{\tilde{c}}_p^{\mathrm{V}}}{R_{\mathrm{W}}}+\frac{{\tilde{c}}_p^{\mathrm{A}\mathrm{V}}}{R_{\mathrm{A}\mathrm{V}}}$$

(73)

is introduced. The commonly used Clausius-Clapeyron equation for the saturation vapour pressure makes use of the fact that far from the critical point of water, the liquid density is much higher than the vapour density. This may be quantified by saying that the dimensionless parameter

$$\epsilon =\frac{p_0}{R_{\mathrm{W}}{T}_0{\tilde{\rho }}^{\mathrm{W}}}\ll 1$$

(74)

is a small number. Of the perturbation expansion

$$T_{\mathrm{L}\mathrm{C}\mathrm{L}}=T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}+\epsilon T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(1\right)}+\dots ,$$

(75)

applied to eq. (72), the leading term may be regarded as Clausius-Clapeyron approximation,

$$Q\ln \frac{T_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}}-\ln {\psi }_f=\frac{{\tilde{g}}_0^{\mathrm{W}}}{R_{\mathrm{W}}}\left(\frac{1}{T_0}-\frac{1}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}}\right).$$

(76)

Eq. (76) may be rearranged into the form

$$\frac{B{T}_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}}\exp \left(-\frac{{BT}_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}}\right)=E$$

(77)

with the abbreviations,

$$B\equiv -\frac{{\tilde{g}}_0^{\mathrm{W}}}{Q{R}_{\mathrm{W}}{T}_0}$$

(78)

$$E\equiv B\exp \left\{-B+\frac{\ln {\psi }_f}{Q}\right\}.$$

(79)

The solution of the nonlinear equation (77) is

$$T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}=-\frac{B}{w\left(-E\right)}{T}_0.$$

(80)

Here, the function $w\left(y\right)$ is the well-defined Lambert W function, see Appendix D, which is one of the two solutions of the equation

$$w\exp \left(w\right)=y.$$

(81)

Because $y=-E$ is negative, such as $E=\mathrm{0.174\; 718}$ at $T_0=292\mathrm{K}$, solutions for the lower branch, $w\left(y\right)<-1$, must be chosen here,

$$T_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}=287.658\mathrm{K}.$$

(82)

Eventually, by means of eq. (71), the Clausius-Clapeyron approximation for the LCL pressure is

$$p_{\mathrm{L}\mathrm{C}\mathrm{L}}^{\left(0\right)}=p_0\exp \left\{\frac{{\tilde{c}}_p^{\mathrm{A}\mathrm{V}}}{R_{\mathrm{A}\mathrm{V}}}\ln \left[-\frac{B}{w\left(-E\right)}\right]\right\}.$$

(83)

In fact, these “crude” Clausius-Clapeyron approximations (80) and (83) agree already quite well with the rigorous iterative TEOS-10 results of eq. (34), see Table 4.

5. Marine Climatic LCL Feedback

Refraining from the complexity of spectrally resolved greenhouse-gas attenuation as shown in Figure 6 and from proper 3D radiation propagation models (Pelkowski 2012, 2014; Zhong and Haigh 2013), low-level marine clouds as well as the ocean surface may approximately be described as planar black bodies, mutually interacting according to the Stefan-Boltzmann law of thermal radiation, Figure 8.

Denoting the Stefan-Boltzmann constant by ${\sigma }_{\mathrm{S}\mathrm{B}}=5.670374419\times {10}^{-8}\mathrm{W}{\mathrm{m}}^{-2}{\mathrm{K}}^{-4}$, the net radiative heat loss, $J_{\mathrm{E}}$, of the cloud-covered ocean is in a 1D model,

$$J_{\mathrm{E}}={\sigma }_{\mathrm{S}\mathrm{B}}\left(T_0^4-T_{\mathrm{L}\mathrm{C}\mathrm{L}}^4\right).$$

(84)

Its sensitivity with respect to ocean warming is

$${\left(\frac{\partial J_{\mathrm{E}}}{\partial T_0}\right)}_{p_0,{\psi }_f}=4{\sigma }_{\mathrm{S}\mathrm{B}}\left[T_0^3-T_{\mathrm{L}\mathrm{C}\mathrm{L}}^3{\left(\frac{\partial T_{\mathrm{L}\mathrm{C}\mathrm{L}}}{\partial T_0}\right)}_{p_0,{\psi }_f}\right],$$

(85)

assuming that the marine surface RH remains unaffected by the SST change. For the LCL values listed in Table 1, the upward and downward radiative fluxes, $J_{\uparrow }={\sigma }_{\mathrm{S}\mathrm{B}}{T}_0^4$ and $J_{\downarrow }={\sigma }_{\mathrm{S}\mathrm{B}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}}^4$, respectively, as well as estimated sensitivities, eq. (85), are calculated in Table 5.

The mathematical dependence of $T_{\mathrm{L}\mathrm{C}\mathrm{L}}$ on $T_0$ is complicated, even in the case of crude approximations, eq. (80). In this section, a rigorous theoretical expression for this dependence is derived systematically from the TEOS-10 approach. As an aside, note that this longwave radiative interaction is an irreversible process with an entropy production rate of (Planck 1906; Kabelac 1994; Feistel 2011)

$$P=J_{\mathrm{E}}\left(\frac{1}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}-\frac{1}{T_0}\right).$$

(86)

Latest assessments (Blunden et al. 2023) have shown that at the ocean surface, current climate change is characterised by an increase of SST along with constant surface RH, or similarly, in the TEOS-10 formalism, with constant relative fugacity, ${\psi }_f$. As a dimensionless quantity, a suitable thermodynamic expression describing a related climatic trend of the LCL greenhouse effect is the sensitivity of the cloud base temperature, $T_{\mathrm{L}\mathrm{C}\mathrm{L}}\left(T_0,p_0,{\psi }_f\right)$, with respect to an increase of the surface temperature, $T_0$,

$$\beta \equiv {\left(\frac{\partial T_{\mathrm{L}\mathrm{C}\mathrm{L}}}{\partial T_0}\right)}_{p_0,{\psi }_f}.$$

(87)

This LCL temperature sensitivity controls the sensitivity of the ocean-cloud heat flux balance, eq. (85), with respect to rising SST. A rigorous formula for this sensitivity may be derived from the set of LCL equations, which describe entropy conservation during uplift, eq. (28),

$$f_1\equiv {\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p}=0,$$

(88)

air saturation at the cloud base, eq. (30),

$$f_2\equiv g^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)-A{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A}\right)}_{T,p}-g^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)=0,$$

(89)

and the given relative humidity at the sea surface, eq. (31),

$$f_3\equiv g^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)-A{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A}\right)}_{T,p}-g^{\mathrm{W}}\left(T_0,p_0\right)-R_{\mathrm{W}}{T}_0\ln {\psi }_f=0.$$

(90)

During the climatic increase of the SST, $T_0$, at constant RH,${\psi }_f$, and surface pressure,$p_0$, these equations remain always fulfilled, for $i=\mathrm{1,2},3$,

$$f_i\equiv 0,\frac{\mathrm{d}{f}_i}{\mathrm{d}{T}_0}\equiv 0,$$

(91)

so that other properties, namely, $A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}$, may vary along with $T_0$. Taking the partial derivatives of the functions $f_1$, $f_2$ and $f_3$ with respect to $T_0$, while considering the dependence of $A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}$ on $T_0$, a set of three equations is obtained for their three unknown sensitivities with respect to ocean warming,

$$\alpha \equiv {\left(\frac{\partial A}{\partial T_0}\right)}_{p_0,{\psi }_f},\beta ,\mathrm{and}\gamma \equiv {\left(\frac{\partial p_{\mathrm{L}\mathrm{C}\mathrm{L}}}{\partial T_0}\right)}_{p_0,{\psi }_f}.$$

(92)

These derivatives are mutually related by eqs. (91),

$${\left(\frac{\partial f_i}{\partial A}\right)}_{T_0,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}}\alpha +{\left(\frac{\partial f_i}{\partial T_{\mathrm{L}\mathrm{C}\mathrm{L}}}\right)}_{A,T_0,p_{\mathrm{L}\mathrm{C}\mathrm{L}}}\beta +{\left(\frac{\partial f_i}{\partial p_{\mathrm{L}\mathrm{C}\mathrm{L}}}\right)}_{{A,T}_0,T_{\mathrm{L}\mathrm{C}\mathrm{L}}}\gamma +{\left(\frac{\partial f_i}{\partial T_0}\right)}_{A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}}=0.$$

(93)

In matrix notation, this is

$$\left(\begin{array}{ccc}{a}_{11}& a_{12}& a_{13}\\ a_{21}& a_{22}& a_{23}\\ a_{31}& 0& 0\end{array}\right)\left(\begin{array}{c}\alpha \\ \beta \\ \gamma \end{array}\right)=\left(\begin{array}{c}{b}_1\\ 0\\ b_3\end{array}\right).$$

(94)

By Cramer’s rule, the solution of this system for the LCL sensitivity $\beta$ is straight forward,

$$\beta =\frac{\left|\begin{array}{ccc}{a}_{11}& b_1& a_{13}\\ a_{21}& 0& a_{23}\\ a_{31}& b_3& 0\end{array}\right|}{\left|\begin{array}{ccc}{a}_{11}& a_{12}& a_{13}\\ a_{21}& a_{22}& a_{23}\\ a_{31}& 0& 0\end{array}\right|}=\frac{b_1{a}_{31}{a}_{23}-b_3\left(a_{11}{a}_{23}-a_{21}{a}_{13}\right)}{a_{31}\left(a_{12}{a}_{23}-a_{22}{a}_{13}\right)}.$$

(95)

The coefficients of this system of linear equations are:

$$a_{11}={\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A\partial T}\right)}_p-{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A\partial T}\right)}_p,$$

(96)

$$a_{12}=-{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T^2}\right)}_{A,p}=\frac{c_p^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(97)

$$a_{13}=-{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T\partial p}\right)}_A,$$

(98)

$$a_{21}=-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A^2}\right)}_{T,p},$$

(99)

$$a_{22}={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_{A,p}-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A\partial T}\right)}_p-{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial T}\right)}_p=\frac{L_{\mathrm{L}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(100)

$$a_{23}={\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial p}\right)}_{A,T}-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial A\partial p}\right)}_T-{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_{\mathrm{L}\mathrm{C}\mathrm{L}},p_{\mathrm{L}\mathrm{C}\mathrm{L}}\right)}{\partial p}\right)}_T=∆v_{\mathrm{L}},$$

(101)

$$a_{31}=-A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A^2}\right)}_{T,p},$$

(102)

$$b_1=-{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T^2}\right)}_{A,p}=\frac{c_p^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{T_0},$$

(103)

$$b_3=-{\left(\frac{{\partial g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial T}\right)}_{A,p}+A{\left(\frac{{{\partial }^{2}g}^{\mathrm{A}\mathrm{V}}\left(A,T_0,p_0\right)}{\partial A\partial T}\right)}_p+{\left(\frac{{\partial g}^{\mathrm{W}}\left(T_0,p_0\right)}{\partial T}\right)}_p+R_{\mathrm{W}}\ln {\psi }_f.$$

(104)

All partial derivatives required here, eq. (96) through eq. (104), are explicitly numerically available from the TEOS-10 SIA library. Reported in Table 1, the LCL sensitivities computed from eq. (94) are the rigorous figures close to the previous estimates of Section 4. A value of $\beta <1$ means that $T_{\mathrm{L}\mathrm{C}\mathrm{L}}$ is rising slower than $T_0$, so that the net radiation balance between sea surface and cloud base is distorted in favour of the upward heat flux, reducing the ocean’s warming rate.

The cloud base is cooling along with global SST warming, see Figure 5, and the net upward radiative heat flux of about 24 W m^–2 is intensifying by 0.45 W m^–2 per each °C of SST, as a negative feedback to the warming ocean by currently about 1 W m^–2 (You 2024). This feedback does not provide a possible explanation for the warming, on the opposite, it tightens the problem. While the LCL feedback is not dominating the sea-air energy transfer, it is anyway large enough to not remain ignored. The effect is about 100 times as large as the rate of atmospheric warming.

For tutorial reasons or first estimates, the coefficients of eq. (94) may be expressed in crude approximation of the Gibbs functions, Appendix C. These are:

$${\tilde{a}}_{11}=\left({\tilde{c}}_p^{\mathrm{V}}-{\tilde{c}}_p^{\mathrm{A}}\right)\ln \frac{T_0}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}}+\left(R_{\mathrm{A}}-R_{\mathrm{W}}\right)\ln \frac{p_0}{p_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(105)

$${\tilde{a}}_{12}=\frac{{\tilde{c}}_p^{\mathrm{A}\mathrm{V}}}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(106)

$${\tilde{a}}_{13}=-\frac{R_{\mathrm{A}\mathrm{V}}}{p_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(107)

$${\tilde{a}}_{21}=-\frac{R_{\mathrm{A}}{R}_{\mathrm{W}}}{\left(1-A\right)R_{\mathrm{A}\mathrm{V}}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}},$$

(108)

$${\tilde{a}}_{22}=\frac{L_{\mathrm{L}}}{T_{\mathrm{L}\mathrm{C}\mathrm{L}}},$$

(109)

$${\tilde{a}}_{23}=\frac{R_{\mathrm{W}}{T}_{\mathrm{L}\mathrm{C}\mathrm{L}}}{p_{\mathrm{L}\mathrm{C}\mathrm{L}}}-\frac{1}{{\tilde{\rho }}^{\mathrm{W}}},$$

(110)

$${\tilde{a}}_{31}=-\frac{R_{\mathrm{A}}{R}_{\mathrm{W}}}{\left(1-A\right)R_{\mathrm{A}\mathrm{V}}}{T}_0,$$

(111)

$${\tilde{b}}_1=\frac{{\tilde{c}}_p^{\mathrm{A}\mathrm{V}}}{T_0},$$

(112)

$${\tilde{b}}_3={\tilde{g}}_1^{\mathrm{W}}+\left({\tilde{c}}_p^{\mathrm{V}}-{\tilde{c}}_p^{\mathrm{W}}\right)\ln \frac{T_0}{{eT}_{\mathrm{t}}}-R_{\mathrm{W}}\ln \frac{{xp}_0}{{\psi }_f{p}_{\mathrm{t}}}.$$

(113)

With either the TEOS-10 SIA library functions, or even with the simplified equations of Appendix C, the calculation of the LCL sensitivity, eq. (95) is straight forward but does likely require to be carried out numerically.

6. Discussion

Climate research relies on observation and causal prediction models. Causality itself cannot be observed but is the most reliable prediction tool (Hume 1967; Russell 1919; Feistel 2023a, b). The comparison of predicted phenomena with future observations serves as a validity criterion for causal models (Hertz 1894; Stips et al. 2016). In 2023, the global ocean heat content (OHC) has increased by $1.5\times {10}^{22}\mathrm{J}$, a value 25 times as large as the world’s total human energy consumption (You 2024), and even at least 100 times exceeding the atmospheric heat gain (von Schuckmann et al. 2023). This currently observed excessive OHC increment (Cheng et al. 2023) at a rate of 1.3 W m^–2 remains largely unexplained so far (Loeb et al. 2021; Blunden et al. 2023), and raises questions regarding correctness, completeness and internal consistency of climate models.

The dominating input-output processes of the ocean heat balance include solar shortwave and tropospheric longwave irradiation, thermal radiation from the ocean surface, latent heat exchange by phase transitions including water vapour and ice, and sensible heat exchange with the atmosphere and lithosphere. The input and output processes each amount to a total of about 500 W m^–2, and the observed imbalance arises from a small mutual mismatch by just 0.2 % between those. Evidently, to reasonably explain a small difference of 1 W m^–2 between two big numbers of about 500 W m^–2, each of those must be known to within an uncertainty below 1 W m^–2. A key question is how this small amount may selectively be attributed to the various individual physical contributions involved. Unfortunately, at the ocean-atmosphere interface, current climate models exhibit uncertainties larger than 10 W m^–2 and cannot reliably resolve the requisite heat flux differences.

TEOS-10, the Thermodynamic Equation of Seawater – 2010, provides the most accurate and mutually consistent equations currently available for the thermodynamic properties of seawater, humid air and ice. It was adopted as an international geophysical standard by IOC/UNESCO in 2009 and by IUGG in 2011 to support climate research.

Current numerical climate models tend to underestimate ocean warming (). They typically implement Dalton equations to estimate evaporation rates (Stewart 2008) in a historic form which forces increasing evaporation as a consequence of rising temperature and, in turn, of the vapour pressure of seawater. Quantitatively underpinned by TEOS-10, irreversible thermodynamics, however, suggests constant marine relative humidity (RH) as the driving force of evaporation, rather than increasing vapour pressure (Feistel and Hellmuth 2023, 2024). A putative tiny increase of global mean RH, even below instrumental resolution, may suffice to explain the rate of ocean warming; this renders latent heat a preferred candidate to be responsible for the observed OHC imbalance (Feistel and Hellmuth 2021).

A second candidate possibly contributing to the OHC increase is the radiation balance, strongly affected by clouds and greenhouse gases. Global cloudiness has systematically been decreasing in the past decades, this way enhancing oceanic shortwave irradiation by decreasing shadowed areas. At the same time, the downward longwave irradiation from those clouds is also decreasing with an estimated result that these two effects almost completely cancel one another (Phillips and Foster 2023). However, global warming does not only reduce cloudiness, the trend of higher sea-surface temperature (SST) also elevates marine clouds by an increasing lifted condensation level (LCL), in turn cooling the cloud base and reducing their downward thermal radiation. In order to include such a cooling effect in the OHC balance investigations, in this paper the sensitivity of LCL and of the related ocean-cloud radiation balance is analysed with the TEOS-10 thermodynamic formalism. Rigorous equations are derived for estimating LCL effects in numerical climate models.

The 2023 global mean SST is about 292 K. At this temperature, the upward thermal radiation flux from the sea surface is 412 W m^–2 (Table 5) while the downward flux from the LCL cloud base is 388 W m^–2. Under LCL cloud cover, the remaining net upward radiation of 24 W m^–2 will grow by 0.45 W m^–2 per one degree of further ocean warming. This LCL feedback effect is relevant in comparison to the 2023 OHC gain of 1.3 W m^–2 and may not be ignored in balance investigations. As a negative, cooling feedback this LCL effect does not help to explain observed ocean warming, rather, it enlarges the problematic heating rate to be explained by other contributing effects of the OHC balance, such as a putative uncertain minor climatic change of evaporation.

However, atmospheric RH can be observed to within an uncertainty in the range 1 %rh - 5 %rh (Lovell-Smith et al. 2016). An increase of ocean surface RH by 1 %rh is estimated to reduce the latent heat flux of evaporation by about 5 W m^–2 (Feistel 2015; Feistel and Hellmuth 2021, 2023), thus warming up the ocean by this rate. In addition, a 1 %rh increase may lower the LCL by about 25 m, in turn intensifying the thermal downward radiation flux from the cloud bottom by more than 1 W m^–2 (see Table 3). LCL height may serve as a remotely measured, sensitive estimate for sea surface relative fugacity or conventional RH. It appears a plausible working hypothesis that the excessive ocean warming of 2023 by 1.3 W m^–2 may be caused to a large extent by slightly rising ocean surface RH not exceeding the uncertainty of observation.