Preprint
Article
The Impact of Induced Acceleration Perturbations in Selected Phases of the Gait Cycle on Kinematic and Kinetic Parameters
Altmetrics
Downloads
98
Views
36
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
15 April 2024
Posted:
16 April 2024
You are already at the latest version
Alerts
Abstract
Background: The prevalence of falls among the older population underscores the imperative of comprehending human adaptations to gait perturbations. Dual-belt treadmills offer a controlled setting for such investigations. The purpose of this study was to examine the effect of acceleration of one belt of the treadmill during three different phases of the gait cycle on kinematic and kinetic parameters and relate these changes to unperturbed gait. Methods: Twenty-one healthy young females walked on a treadmill in a virtual environment, in which five unexpected perturbations were applied to the left belt at the Initial Contact (IC), Mid Stance (MS), and Pre-Swing (PS) phase of the gait cycle. Data from the undisturbed gait and the first disturbance of each trial were extracted for analysis. Results: All perturbations significantly affected the gait pattern, mainly by decreasing the knee extension angle. The perturbation in the IC phase had the most significant effect, resulting in a 248.48% increase in knee flexion torque. The perturbation in the MS phase mainly affected plantar flexion torque, increasing it by 118.18%, while perturbation in the PS phase primarily increased the hip extension torque by 73.02%. Conclusions: The presence of perturbations in the IC and PC phases caused the most aggressive and significant changes in gait parameters.
Keywords:
Subject: Biology and Life Sciences - Other
1. Introduction
Over the years, researchers have been fascinated by the gait and postural control dynamics and its complicated responses to potential perturbations. In fields such as physics, mathematics, and biology, perturbation refers to a small change or disturbance applied to a system or process, which can lead to alterations in its behavior, properties, or outcomes [1,2]. These disturbances can arise from external forces [3], such as environmental conditions or physical interactions with objects in the surroundings [4]. Alternatively, they can originate from internal factors, including physiological changes or neural signals within the organism [5]. These disturbances might manifest intentionally, such as during experimental manipulations [6], or they could occur accidentally due to unforeseen events or natural variability in the system. It is worth mentioning that external perturbations are not limited to mechanical disturbances. They can also manifest as sensory disturbances, including hearing and vision [7]. Roeles, et al. [7] demonstrated that mechanical perturbations significantly altered the gait patterns of both young and older adults.
In contrast, sensory perturbations did not exhibit a notable effect on the gait pattern. Similar conclusions were in the papers [8,9]. These findings suggest that giving priority to the analysis of responses to mechanical perturbations is more justified. To date, many perturbation methods have been proposed in the literature. These include placing obstacles underfoot [10], using slippery or unstable surfaces [11], moving plates [12], and unexpected treadmill accelerations and decelerations [4,10,13]. The field of gait perturbation research, particularly involving both one and dual-belt treadmill setups, is getting increasing attention and is presently the most commonly used equipment in such studies [14]. Semaan, et al. [15] demonstrated that treadmill gait is a reliable approximation of overground walking. Furthermore, treadmills offer the advantage of high repeatability in perturbations and walking conditions [16]. Modern dual belt treadmills, such as GRAIL, facilitate the generation of many perturbation types across various gait phases and planes. This comprehensive capability enables a detailed analysis of individual gait characteristics in response to perturbations.
Research focused on perturbations is strictly related to the adaptive functions of the human neuromuscular system that allow the maintenance of balance and straight position [4,6,17,18,19]. As individuals age, they typically exhibit decreased capacity to adapt to external disturbances, potentially heightening their vulnerability to falls [20,21]. It is concerning that nearly a quarter of the elderly population is susceptible to falling [22]. As the global population is constantly aging, understanding reactions to perturbations is going to become crucial. To this day, numerous studies have explored kinematics, kinetics, and spatiotemporal parameters, as well as muscle activity, during gait perturbations [14]. However, to the best of our knowledge, no one has so far studied the extreme values of the joint torques in perturbated non-dominant limbs at Initial Contact, Mid Stance, and Pre-Swing altogether. To address this issue, the purpose of this study was to examine the effect of acceleration of one belt of a treadmill during three different phases of the gait cycle on kinematic and kinetic parameters and relate these changes to unperturbed gait.
2. Materials and Methods
2.1. Participants
The study group consisted of 21 healthy young females with a mean age of 21.38 ± 1.32 years, body weight of 61.38 ± 6.48 kg, and body height of 165.9 ± 4.53 cm. Participants needed to meet specific eligibility criteria, including having no muscular or neurological disorders, no lower limb injuries within the last six months, engaging in physical activity at least twice weekly, right leg dominance [23], and prior experience with treadmill walking. Exclusion criteria encompassed inexperience with treadmill walking, balance issues, or medications affecting the nervous system. All participants provided written informed consent to participate in the study. The study received approval from the University Review Committee (no. SKE01-15/2023) and followed the ethical guidelines and principles of the Declaration of Helsinki.
2.2. Measurement Protocol
The kinematic and kinetic parameters of the perturbed gait were measured in the Interactive Gait Real-Time Analysis Laboratory (GRAIL, Motek Medical B.V., Amsterdam, Netherlands). The GRAIL comprised a dual split-belt treadmill (1000 Hz), a motion capture system (Vicon Metrics Ltd., Oxford, UK) operating at 100 Hz, three video cameras, synchronized virtual reality environments, and a safety harness suspended from the ceiling. The accompanying D-Flow software (Motek Medical B.V., Amsterdam, Netherlands) was used for model adjustment, initiating perturbations, and data collection.
As the participants arrived, anthropometric measurements were collected, and 26 reflective markers were placed on their bodies according to Human Body Model 2 (HBM2) guidelines. Subsequently, participants stepped on the treadmill and aligned in a T-pose to achieve an accurate D-Flow body model fit. The test protocol included three walks at a constant speed of 1.2 m/s containing perturbations involving the acceleration of the left lane of the treadmill (non-dominant limb) during the Initial Contact (IC), Mid Stance (MS), Pre-Swing (PS) phases, respectively (Figure 1).
Perturbations were systematically introduced at the 30th, 40th, 50th, 60th, and 70th seconds of the gait, and participants walked in flat-sole athletic shoes. The perturbation magnitude was set to 5 on a scale of 1 – 5, involving a shift in treadmill belt speed of 0.5 meters per second [24]. Using a high perturbation magnitude facilitated a clearer estimation of peak loads, as the body's response to significant unexpected forces was more pronounced, making it easier to observe and measure resulting joint loads.
2.3. Data Processing
Data obtained from D-Flow software were saved as *.mox files and then imported into Matlab 2021a (MathWorks, Natick, MA, USA) using a toolbox developed by Feldhege, et al. [25]. Subsequently, they were transformed into OpenSim 4.2 input files: *.trc, which contained positions of markers placed on a subject at different times during a motion capture trial, and *.mot, which included ground reaction force data [26]. The gait2354_model, a three-dimensional, 23-degree-of-freedom computer model of the human musculoskeletal system with 54 musculotendon actuators representing 27 muscles in the lower extremities and torso, was adjusted to participants' anthropometry using the Scale Tool. Joint angles were determined using the Inverse Kinematics Tool (IK) based on marker positions during motion. Joint torques were obtained using the Inverse Dynamics Tool (ID) and ground reaction forces (GRF). The perturbed gait cycle for the left leg was determined in the OpenSim software, considering the visualization of inverse kinematics and GRF. Data from the undisturbed gait sample and the first disturbance of each trial were extracted for further analysis. The gait cycle length for each perturbed trial and the gait without perturbations was estimated using the Euclidean distance equation based on the position of the left heel marker at the heel strike, as follows:
where: x1, y1, z1 denote the position of the heel marker at the beginning of the gait cycle, and x2, y2, z2 denote the position of the heel marker at the end of the gait cycle, which was taken into consideration. For subsequent statistical analysis, the stride length, extreme values (minimal and maximal) of kinematic and kinetic parameters were extracted for gait cycles both with and without perturbations.
2.4. Statistical Analysis
Statistical analysis was conducted using PQStat 2021 software v.1.8.2.238 (PQStat Software, Poznań, Poland). The normality of the distribution of the analysed data was assessed using the Shapiro-Wilk test. This test indicated normal distributions for extreme values of joint angles and stride length in gait without perturbation as well as for gait with perturbation in IC, MS, and PS. Different from the normal distribution was found for extreme values of joint torques and stride length during MS perturbation.
ANOVA with Tukey's HSD post-hoc test was used to identify statistically significant differences in the extreme values of joint angles achieved in gait cycles with perturbations in IC, MS, and PS and gait cycles without perturbation (N). Friedman's ANOVA test with Bonferroni's post-hoc test was employed to detect statistically significant differences in the extreme values of joint torques and stride length achieved in the gait cycle with perturbations in IC, MS, and PS, and the gait cycle without perturbation (N).
Paired t-tests using statistical parametric mapping (SPM) [27] were employed on continuous curves, specifically focusing on angles and muscle torques at the lower limb joints. The SPM1D package (https://www.spm1d.org) facilitated these analyses. Comparisons were made for curves that incorporated perturbations in the IC, MS, and PS phases, referencing curves recorded during free gait. All analyses were performed in Matlab R2021a.
3. Results
3.1. Analysis of the Magnitude in Terms of Area Changes
Participants exhibited various stepping strategies in response to the studied perturbations, which were triggered during the IC, MS, and PS phases of the gait cycle by accelerating the left treadmill lane (Figure 1), leading to changes in gait cycle length and both kinematic and kinetics parameter values (Table 1, Figure 2).
When studying the comparison of the curves for ankle angles with perturbation in the IC phase (Figure 2A) and the curve without perturbation, it is noteworthy that statistically significant differences occur not only at the moment of perturbation but also extend to a significantly larger area, as follows: [0 – 34.6]%, [39.18 – 64.38]%, and [70.42 – 88]% of the gait cycle. For the knee angle (Figure 2B), differences manifest in two clusters: [10.62 – 72.96]% and [90 – 100]% of the gait cycle. However, for the hip joint (Figure 2C), the differences cover the entire swing phase and part of the support phase, starting from the beginning of the Terminal Stance phase [39 – 100]% of the gait cycle. Similarly, when examining the behavior of ankle torque (Figure 2D), it is worth noting that statistically significant differences occur in shifted areas compared to those observed for kinematic parameters. These differences manifest as follows: [5 – 36]%, [46.74 – 69.29]%, and [92.11 – 96.85]% of the gait cycle. For the knee joint (Figure 2E), differences are recorded across almost the entire gait cycle, presenting in six clusters: [0 – 4]%, [7 – 35.4]%, [45.6 – 56.3]%, [60 – 67]%, [72 – 74.36]%, and [91.6 – 95]% of the gait cycle. However, in the case of the hip joint (Figure 2F), differences include the following areas: [0 – 4]%, [52.6 – 67]%, and [97 – 100]% of the gait cycle. In particular, an additional area of [0 – 4]% appeared for the knee and hip torque, which was not observed for the angles.
When comparing the curves for the ankle joint angle with perturbation in the MS phase (Figure 2A) to the curve for gait without perturbation, three areas exhibit statistically significant differences: [0 – 9]%, [43 – 67]%, and [81 – 92]% of the gait cycle. Similar to previous findings, differences are observed for additional joints but are confined to two clusters: [5 – 60]% and [93 – 100]% of the gait cycle for the knee joint (Figure 2B) and [34 – 46]% and [51 – 60]% of the gait cycle for the hip joint (Figure 2C). Similar to the previous perturbation, the analysis of muscle torques provides different information. When comparing the curves for ankle torque with perturbation in the Mid Stance phase (Figure 2D) to the curves for gait without perturbation, three areas, similar to those observed for angles, exhibit statistically significant differences: [23.47 – 51.17]%, [57.81 – 59.22]%, and [92.36 – 98]% of the gait cycle. In the knee joint (Figure 2E), differences are evident throughout the entire gait cycle, with as many as six clusters: [2 – 4.22]%, [6.63 – 20]%, [31.31 – 37.02]%, [52 – 59]%, [62 – 69]%, and [85.5 – 93]% of the gait cycle. However, for the hip joint (Figure 2F), differences manifest in two clusters: [6 – 22.1]% and [62.77 – 85.80]% of the gait cycle.
Most differences were observed for curves containing perturbations during the Pre-Swing phase. Regarding the ankle joint, four clusters with differences were identified, spanning the entire gait cycle with small 10% intervals: [9 – 17]%, [43 – 48]%, and [66 – 77]%. For the knee joint, similar to previous perturbations, two clusters with noticeable differences are apparent: [17 – 55]% and [88 – 100]% of the gait cycle. In the case of the hip joint, differences were identified in the following regions: [16 – 45]%, and [81 – 100]% of the gait cycle. When comparing the curves for ankle torque with perturbation in the Pre-Swing phase (Figure 2D) to the curve for gait without perturbation, it is noteworthy that statistically significant differences extend beyond the moment of perturbation, covering a significantly larger area: [5 – 36]%, [46.74 – 69.29]%, and [92.11 – 96.85]% of the gait cycle. In the knee joint (Figure 2E), differences are observed in three segments: [13 – 21.18]%, [36.31 – 52.14]%, and [72.69 – 98.44]% of the gait cycle. However, for the hip joint (Figure 2F), differences manifest in two areas: [55 – 60]% and [72.41 – 95]% of the gait cycle. The above description illustrates how individual perturbations radiate and impact the affected areas, which do not overlap for kinetic and kinetic parameters.
3.2. Analysis of the Volume of Changes
To understand the extent of changes induced by perturbations, it is valuable to not only compare the affected areas, but also to assess their magnitude by comparing the peak values (Table 2) observed in each curve with those documented during unperturbed gait.
In analysing extreme values for gait with perturbations compared to gait without them, it becomes evident that most substitutions occurred sequentially during the perturbation in the Initial Contact phase (6 significant for kinematic parameters and 4 for kinetic parameters), Pre-Swing (5 significant for kinematic parameters and 2 for kinetic parameters), and Mid Stance phase (2 for kinematic parameters and 3 for kinetic parameters). Furthermore, in most cases, there were significant increases in extreme values in the presence of perturbations.
It is worth highlighting that the most substantial increases in kinematic parameters are for ankle plantar flexors, which exceed those achieved during treadmill gait by 382.06%, 321.98%, and 241.13% for perturbations in the IC, PS, and MS phases, respectively. A less marked but consistent trend is evident for ankle dorsiflexors, with values for IC, PS, and MS perturbations exceeding those recorded for treadmill gait by 36.61%, 23.32%, and 12.84%, respectively. The hip joint tends to reduce its maximal extension compared to treadmill gait by 36.62%, 1.97%, and 22.96% for perturbations in the IC, PS, and MS phases, respectively. Simultaneously, flexion values increased by 8.8% for gait with perturbation in the MS phase and by 25.43% and 29.71% during perturbation in the PS and IC phases, respectively. The knee joint remains in flexion, with maximum values increasing by 12.26% during perturbations in the IC phase and by 10.13% during PS phase perturbations. Notably, knee extension was observed during treadmill gait, but this phenomenon was not repeated during any perturbation (Table 2).
The response to changes in kinematic parameters is also evident for kinetic parameters. Notably, the alterations in ankle joint torques are relatively minor compared to the previously discussed kinematic values. This phenomenon can probably be attributed to the involvement of the bi-articular muscles, in this case directing their influence mainly to the knee joint. Within the knee joint, a significant increase of 248.48% is observed in the flexion torque when perturbation is introduced during the IC phase, in contrast to gait without perturbation. Meanwhile, the hip joint experiences a substantial increase in the activation of hip flexor muscles, particularly during perturbations in the IC and PS phases, with increases of 78.69% and 14.75%, respectively (Table 2).
4. Discussion
The aim of the study was to identify at which phase of the gait cycle (Initial Contact, Mid Stance, or Pre-Swing) induced one-belt acceleration perturbations caused the most substantial alterations in gait parameters and potentially led to the highest overloads of the perturbed lower limb.
This study employed a perturbation protocol using the Grail system (Motek Medical BV, Amsterdam, the Netherlands), which allowed for precise timing and intensity control of perturbations. The findings indicate that all perturbations significantly influenced the examined parameters, probably due to the application of high perturbation force [4]. A consistent significant alteration observed across all perturbations was the reduction in full knee extension, as evidenced by the knee joint angle curves (Figure 2B) and extreme values for the knee extension angle (Table 2). All this suggests a critical role of the knee joint in adapting to perturbations, a trend similarly noted by Shokouhi, et al. [28], who examined adjustments in lower limb joint power and work in response to trip-induced perturbations. In contrast to Shokouhi, et al.'s [28] trip-like perturbation, which involved rapidly decelerating the belt under the dominant foot, this study utilized accelerations. Additionally, Błażkiewicz and Hadamus [4] assessed gait regularity during external perturbations caused by treadmill belt acceleration and deceleration across different phases of the gait cycle. They found that perturbations caused by treadmill belt deceleration contribute to higher irregularities, with the most irregular behavior observed during the Pre-Swing phase (vertical direction) among those caused by acceleration. In this paper, an analysis of the kinetic and kinematic values of the perturbed gait indicated that perturbations induced during the Initial Contact (IC) phase were the most intense. Perturbations in the Pre-Swing (PS) phase also had a significant impact on joint angular values, but they did not result in substantial changes in torque values, thus making them less likely to cause overloads. Perturbations in the Mid Stance (MS) phase were determined to have the least significance. These findings will be discussed sequentially, starting with the most impactful perturbations.
4.1. Perturbations in the Initial Contact Phase
The initial contact (IC) phase, marking the beginning of the gait cycle, plays a fundamental role in locomotion [29]. It requires good coordination, balance, and effective shock absorption capabilities. The perturbation induced in the IC phase affects the entire gait cycle, which is probably the reason why it accounts for the most significant changes observed in the examined parameters.
The most noteworthy discovery revolved around the alteration in knee joint torques, as the areas showing significant changes covered 55.86% of the gait cycle (Figure 2E). Specifically, the peak knee flexion torque reached 1.15 ± 0.29 Nm/kg, marking a staggering 248.48% increase compared to unperturbed gait. It was the most substantial kinetic shift across all perturbations and joints. Additionally, there was a noteworthy rise in peak knee extension torque -0.66 ± 0.21 Nm/kg in the presence of perturbation, compared to the -0.31 ± 0.03 Nm/kg recorded during unperturbed gait. Moreover, the perturbation during the initial contact (IC) phase induced substantial alterations in knee kinematics, affecting up to 72.34% of the gait cycle. However, there was only a minor increase of 12.26% in extreme flexion values.
Perturbations significantly altered the ankle joint angle curve values in 77.38% of the gait cycle area compared to a typical gait pattern. These alterations were most notable in the extremes of plantar flexion, exhibiting a substantial increase from -23.91 ± 9.42 deg to -4.96 ± 2.25 deg. Despite this pronounced shift, the torque exerted by the plantar flexors experienced a notable decrease of 27.72% compared to the torque observed during unperturbed gait.
Perturbations also significantly affected the kinematic parameters associated with the hip joint. These alterations impacted 61% of the gait cycle, particularly visible during the late stance and all swing phases. Notably, perturbation during the initial contact phase induced the most substantial changes in the extreme angular values of the hip joint, with hip flexion increasing by 29.71% and hip extension by 36.62%. In terms of torque, there was a noteworthy 78.69% increase in hip flexion torque.
It is worth noting that reviews by Taylor, et al. [14], as well as Ferreira, et al. [10], have underscored that heel strike perturbations are prevalent. This study corroborates this finding, demonstrating that perturbations during the IC phase significantly impacted all examined parameters except torques related to dorsiflexion and hip extension.
Notably, knee flexion torque exhibited a nearly threefold increase, emphasizing the pivotal role of the knee joint and quadriceps muscle in responding to such perturbations. This observation is particularly relevant given that even short periods of immobilization can lead to rapid muscle weakness [30], potentially heightening the risk of falls triggered by perturbations.
4.2. Perturbations in the Pre-Swing Phase
The Pre-Swing phase occurs at 50-60% of the gait cycle, immediately preceding the swing phase. Perturbation induced in this phase had the most significant impact on increasing step length, artificially accelerating the swing phase, and forcing participants to actively decelerate the limb. However, concerns arise as the gait cycle for perturbations in the PS phase was disrupted even before the perturbation occurred.
This disruption does not seem attributable to mistimed perturbation induction, as Golyski, et al. [31] reported an average delay of 56 ms in perturbation induction by GRAIL and D-Flow systems. Nor does it appear to stem from changes in percentage intervals due to gait cycle shortening or lengthening. A more plausible explanation seems to be that the perturbation in the PS phase was induced last, prompting participants to adopt a different gait pattern in anticipation, as observed in studies by Swart, et al. [32]. The hypothesis that participants adopted a different gait strategy is also supported by the kinematic curves of the knee joint, showing a lack of full extension compared to unperturbed gait. This relationship is particularly visible in the area of [7 – 35.4]% of the gait cycle (Figure 2E). Significant changes also occur in the hip joint [16 – 45]% and in the ankle joint [9 – 17]%; [43 – 48]%, in areas before the disturbance. The perturbation in the PS phase significantly affected all extreme kinematic values, except for the hip extension angle. The greatest changes compared to unperturbed gait were observed for plantar flexion -20.93 ± 8.22 vs. -4.96 ± 2.25 deg and knee extension 3.4 ± 3.81 vs. -3.28 ± 1.95 deg.
As for the analysis of kinetic parameters, changes in gait pattern appeared in the [5 – 36]% of the gait cycle for the ankle torque and within [13 – 21.18]%, [36.31 – 52.14]% of the gait cycle for the knee joint torque curves. However, changes in the kinetic parameters of the hip joint are directly related to the moment of perturbation, appearing, respectively, in the areas of [55 – 60]% and [72.41 – 95]% of the gait cycle. The most significant alteration in extreme values occurred at the hip joint, with the hip extension torque increasing by 73.02%. This represented the most substantial change in this parameter among all examined perturbations. These results are consistent with Vlutters, et al. [33], who also identified the hip joint as a primary control for this type of disturbance.
Regarding the perturbation during the Pre-Swing (PS) phase, the most notable changes were noted for the hip extension torque. However, the existence of significant alterations preceding the induced perturbation is intriguing and challenging to explain. These pre-perturbation changes are possibly attributable to anticipatory muscle activation in preparation for the impending perturbation. But this issue requires further research.
4.3. Perturbations in the Mid Stance Phase
The perturbations induced during the Mid Stance phase had minimal impact on the parameters studied compared to the previously mentioned perturbations. Notably, only this perturbation did not alter stride length compared to unperturbed gait. However, this finding contrasts with the Madehkhaksar, et al. [34] report, which observed a significantly shorter step length under similar conditions.
The most common strategy adopted in response to this perturbation involved rising onto the toes (Figure 1). This is evident in the notable deviations observed in ankle plantar flexion occurring within the intervals [23.47 – 51.17; 57.81 – 59.22]% of the gait cycle. Moreover, extreme torque values for plantar flexion increased by 118.18% compared to those recorded during undisturbed gait, marking the most significant change in this parameter of all the perturbations. Furthermore, there was a significant increase in extreme torque values for knee flexion – 93.94% – compared to unaffected gait. Aligned with the findings presented in this study are the results of Taborri, et al. [35]. Taborri, et al. [35] demonstrated a noticeable decrease in ankle dorsiflexion and plantar flexion when locomotion was perturbed. However, it is worth noting that the authors analysed ranges of motion rather than angles.
Before perturbation, as for perturbations in the Pre-Swing phase, some areas showed significant differences from the unperturbed gait cycle. These included changes in [0 – 9]% and [5 – 60]% of the gait cycle for ankle and knee kinematics, respectively. For kinetic parameters, the changes regions were [2 – 4.22; 6.63 – 20]% and [6 – 22.1]% of the gait cycle for the knee and hip joint, respectively (Figure 2). It seems that the explanation for this phenomenon may be similar to that for perturbation in the Pre-Swing phase, and it relates to the premature activation of muscles in subconscious anticipation of a perturbation, as this type of perturbation was studied as the second one in the experiment. Santuz, et al. [36], by applying the muscle synergy theory, demonstrated that humans are able to modify their motor control strategies, especially in terms of timing, when walking in unsteady conditions.
This study has some limitations. First, due to the technical limitations of the treadmill, the perturbations did not occur at the same time of the gait cycle, only in the same interval, which could lead to different muscle activations and thus different behaviors. However, as presented, the results were pretty consistent within the perturbations. Another limitation concerns the analysis of the perturbed limb only. In subsequent studies, it would also be worthwhile to consider recovery limb. Given that there was a delay in the triggering of the perturbation due to limitations in the configuration of the treadmill device, the frame in which the perturbation was presented could not be detected, making point analysis impossible and forcing an interval analysis (SPM). It is not known whether the extreme values were after the perturbation or at the time of its occurrence. Thus, some cautions in interpreting the results should be taken into account, considering that depending on the moment of the perturbation within the gait cycle the response is different.
5. Conclusions
The research findings suggest that each type of examined one-belt-acceleration perturbation presents a challenge to balance capabilities. However, the perturbation in the IC phase was associated with the highest loads, particularly in terms of the extreme values of the knee flexion torque, which were three times higher compared to normal gait. Perturbations in the PS phase primarily influenced the hip joint, with the most significant change observed in the extension torque. The perturbations that occurred in the MS phase mainly affected the ankle joint, with a notable influence on the plantar flexion torque.
Research, carried out in healthy young people, employing high treadmill speed and perturbation force, may serve as a valuable reference point for future studies and the development of rehabilitation protocols.
Author Contributions
Conceptualization, M.B.; methodology, K.C. and M.B.; software, K.C. and M.B.; validation, R.B.; formal analysis, K.C. and M.B.; investigation, M.B. and K.C.; resources, M.B.; data curation, K.C.; writing—original draft preparation, K.C., R.B., M.B.; writing—review and editing, K.C., R.B., M.B.; visualization, M.B.; supervision, M.B.; project administration, M.B.; funding acquisition, M.B. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the Józef Piłsudski University of Physical Education in Warsaw, grant number UPB no. 2 (114/12/PRO/2023).
Institutional Review Board Statement
This study was conducted in accordance with the Declaration of Helsinki, and approved by the Institutional Review Board of Józef Piłsudski University of Physical Education in Warsaw, Poland (protocol code SKE01-15/2023 and date of approval 24.03.2023).
Informed Consent Statement
Written informed consent has been obtained from the patient(s) to publish this paper.
Data Availability Statement
The measurement data used to support the findings of this study are available from the corresponding author upon request.
Acknowledgments
We would like to thank Karol Kowieski, Martyna Jarocka, Katarzyna Kaczmarczyk, and Agnieszka Zdrodowska for their help in data collection.
Conflicts of Interest
The authors declare no conflicts of interest.
References
- Bender, C.; Orszag, S. Advanced Mathematical Methods for Scientists and Engineers: Asymptotic Methods and Perturbation Theory; 1999; Volume 1.
- Holmes, M.H. Introduction to perturbation methods. 2013. [CrossRef]
- Rogers, M.W.; Mille, M.-L. Chapter 5 - Balance perturbations. In Handbook of Clinical Neurology, Day, B.L., Lord, S.R., Eds.; Elsevier: 2018; Volume 159, pp. 85–105.
- Błażkiewicz, M.; Hadamus, A. Influence of Perturbation’s Type and Location on Treadmill Gait Regularity. Applied Sciences 2024, 14, 493. [Google Scholar] [CrossRef]
- Blazkiewicz, M.; Wiszomirska, I.; Kaczmarczyk, K.; Brzuszkiewicz-Kuzmicka, G.; Wit, A. Mechanisms of compensation in the gait of patients with drop foot. Clin Biomech (Bristol, Avon) 2017, 42, 14–19. [Google Scholar] [CrossRef] [PubMed]
- Vonesch, A.; Duhot, C.; Lelard, T.; Léonard, G.; Błażkiewicz, M.; Mouras, H. Non-Linear Measures of Postural Control in Response to Painful and Non-Painful Visual Stimuli. Entropy (Basel) 2023, 25. [Google Scholar] [CrossRef] [PubMed]
- Roeles, S.; Rowe, P.J.; Bruijn, S.M.; Childs, C.R.; Tarfali, G.D.; Steenbrink, F.; Pijnappels, M. Gait stability in response to platform, belt, and sensory perturbations in young and older adults. Medical & biological engineering & computing 2018, 56, 2325–2335. [Google Scholar] [CrossRef]
- Thies, S.B.; Richardson, J.K.; Ashton-Miller, J.A. Effects of surface irregularity and lighting on step variability during gait: a study in healthy young and older women. Gait Posture 2005, 22, 26–31. [Google Scholar] [CrossRef] [PubMed]
- Rogers, H.L.; Cromwell, R.L.; Grady, J.L. Adaptive changes in gait of older and younger adults as responses to challenges to dynamic balance. Journal of aging and physical activity 2008, 16, 85–96. [Google Scholar] [CrossRef] [PubMed]
- Ferreira, R.N.; Ribeiro, N.F.; Figueiredo, J.; Santos, C.P. Provoking Artificial Slips and Trips towards Perturbation-Based Balance Training: A Narrative Review. Sensors 2022, 22, 9254. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Joo, K.S.; Liu, J.; Sohn, J.H. Lower extremity kinematics during forward heel-slip. Technology and health care : official journal of the European Society for Engineering and Medicine 2019, 27, 345–356. [Google Scholar] [CrossRef] [PubMed]
- Sawers, A.; Pai, Y.C.; Bhatt, T.; Ting, L.H. Neuromuscular responses differ between slip-induced falls and recoveries in older adults. J Neurophysiol 2017, 117, 509–522. [Google Scholar] [CrossRef]
- Liu, X.; Bhatt, T.; Wang, S.; Yang, F.; Pai, Y.C. Retention of the "first-trial effect" in gait-slip among community-living older adults. GeroScience 2017, 39, 93–102. [Google Scholar] [CrossRef]
- Taylor, Z.; Walsh, G.S.; Hawkins, H.; Inacio, M.; Esser, P. Perturbations during Gait: A Systematic Review of Methodologies and Outcomes. Sensors (Basel, Switzerland) 2022, 22. [Google Scholar] [CrossRef]
- Semaan, M.B.; Wallard, L.; Ruiz, V.; Gillet, C.; Leteneur, S.; Simoneau-Buessinger, E. Is treadmill walking biomechanically comparable to overground walking? A systematic review. Gait & Posture 2022, 92, 249–257. [Google Scholar] [CrossRef]
- Tesio, L.; Rota, V. Gait Analysis on Split-Belt Force Treadmills: Validation of an Instrument. American Journal of Physical Medicine & Rehabilitation 2008, 87. [Google Scholar]
- Visser, J.E.; Carpenter, M.G.; van der Kooij, H.; Bloem, B.R. The clinical utility of posturography. Clinical neurophysiology : official journal of the International Federation of Clinical Neurophysiology 2008, 119, 2424–2436. [Google Scholar] [CrossRef]
- Borkowski, R.; Błażkiewicz, M. Postural Reactions to External Mediolateral Perturbations: A Review. Applied Sciences 2023, 13, 1696. [Google Scholar] [CrossRef]
- Bartsch-Jimenez, A.; Błażkiewicz, M.; Azadjou, H.; Novotny, R.; Valero-Cuevas, F.J. "Fine synergies" describe motor adaptation in people with drop foot in a way that supplements traditional "coarse synergies". Frontiers in sports and active living 2023, 5, 1080170. [Google Scholar] [CrossRef]
- Thibaud, M.; Bloch, F.; Tournoux-Facon, C.; Brèque, C.; Rigaud, A.S.; Dugué, B.; Kemoun, G. Impact of physical activity and sedentary behaviour on fall risks in older people: a systematic review and meta-analysis of observational studies. European Review of Aging and Physical Activity 2012, 9, 5–15. [Google Scholar] [CrossRef]
- Jiang, Y.; Wang, M.; Liu, S.; Ya, X.; Duan, G.; Wang, Z. The association between sedentary behavior and falls in older adults: A systematic review and meta-analysis. Frontiers in public health 2022, 10, 1019551. [Google Scholar] [CrossRef]
- Salari, N.; Darvishi, N.; Ahmadipanah, M.; Shohaimi, S.; Mohammadi, M. Global prevalence of falls in the older adults: a comprehensive systematic review and meta-analysis. Journal of Orthopaedic Surgery and Research 2022, 17, 334. [Google Scholar] [CrossRef]
- Promsri, A.; Haid, T.; Federolf, P. How does lower limb dominance influence postural control movements during single leg stance? Human movement science 2018, 58, 165–174. [Google Scholar] [CrossRef]
- Sloot, L.H.; van den Noort, J.C.; van der Krogt, M.M.; Bruijn, S.M.; Harlaar, J. Can Treadmill Perturbations Evoke Stretch Reflexes in the Calf Muscles? PLOS ONE 2015, 10, e0144815. [Google Scholar] [CrossRef]
- Feldhege, F.; Richter, K.; Bruhn, S.; Fischer, D.C.; Mittlmeier, T. MATLAB-based tools for automated processing of motion tracking data provided by the GRAIL. Gait Posture 2021, 90, 422–426. [Google Scholar] [CrossRef] [PubMed]
- Delp, S.L.; Anderson, F.C.; Arnold, A.S.; Loan, P.; Habib, A.; John, C.T.; Guendelman, E.; Thelen, D.G. OpenSim: open-source software to create and analyze dynamic simulations of movement. IEEE Trans Biomed Eng 2007, 54, 1940–1950. [Google Scholar] [CrossRef] [PubMed]
- Pataky, T.C. Generalized n-dimensional biomechanical field analysis using statistical parametric mapping. J Biomech 2010, 43, 1976–1982. [Google Scholar] [CrossRef]
- Shokouhi, S.; Mokhtarzadeh, H.; Lee, P.V.-S. Lower extremity joint power and work during recovery following trip-induced perturbations. Gait & Posture 2024, 107, 1–7. [Google Scholar] [CrossRef]
- Perry, J.; Burnfield, J.M. Gait analysis. Normal and pathological function 2nd ed. California: Slack, 2010. [Google Scholar]
- Marusic, U.; Narici, M.; Simunic, B.; Pisot, R.; Ritzmann, R. Nonuniform loss of muscle strength and atrophy during bed rest: a systematic review. Journal of applied physiology (Bethesda, Md. : 1985) 2021, 131, 194–206. [Google Scholar] [CrossRef] [PubMed]
- Golyski, P.R.; Vazquez, E.; Leestma, J.K.; Sawicki, G.S. Onset timing of treadmill belt perturbations influences stability during walking. J Biomech 2022, 130, 110800. [Google Scholar] [CrossRef]
- Swart, S.B.; den Otter, R.; Lamoth, C.J.C. Anticipatory control of human gait following simulated slip exposure. Scientific Reports 2020, 10, 9599. [Google Scholar] [CrossRef]
- Vlutters, M.; van Asseldonk, E.H.F.; van der Kooij, H. Lower extremity joint-level responses to pelvis perturbation during human walking. Sci Rep 2018, 8, 14621. [Google Scholar] [CrossRef]
- Madehkhaksar, F.; Klenk, J.; Sczuka, K.; Gordt, K.; Melzer, I.; Schwenk, M. The effects of unexpected mechanical perturbations during treadmill walking on spatiotemporal gait parameters, and the dynamic stability measures by which to quantify postural response. PLoS One 2018, 13, e0195902. [Google Scholar] [CrossRef]
- Taborri, J.; Santuz, A.; Brüll, L.; Arampatzis, A.; Rossi, S. Measuring Kinematic Response to Perturbed Locomotion in Young Adults. Sensors (Basel, Switzerland) 2022, 22. [Google Scholar] [CrossRef] [PubMed]
- Santuz, A.; Ekizos, A.; Eckardt, N.; Kibele, A.; Arampatzis, A. Challenging human locomotion. 2018.
Figure 1.
Example of a participant’s typical response. The left (red) lower extremity was perturbed, while the right (green) lower limb was recovered. The moments when the perturbation occurred are marked in red at the top of the figures, representing the phase of the gait cycle.
Figure 1.
Example of a participant’s typical response. The left (red) lower extremity was perturbed, while the right (green) lower limb was recovered. The moments when the perturbation occurred are marked in red at the top of the figures, representing the phase of the gait cycle.
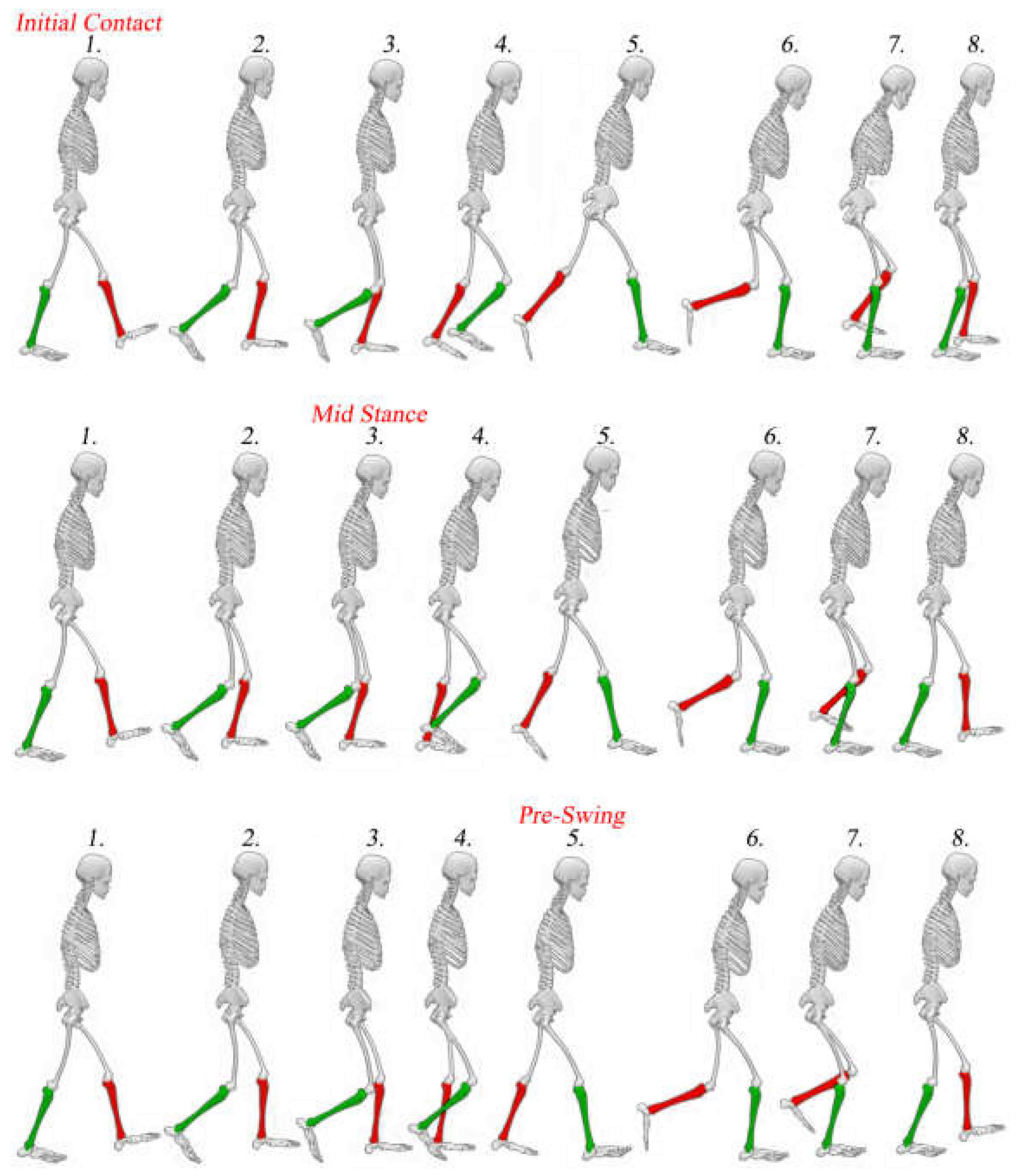
Figure 2.
The means and standard deviations of curves for the A. ankle angle joint, B. knee angle joint, C. hip angle joint, D. ankle torque, E. knee torque and F. hip torque throughout the gait cycle. The first column shows the curves for the affected limb without perturbation (black), with perturbation in the Initial Contact (red), Mid Stance (green) and Pre-Swing (blue) phase of gait cycle. The second, third, and fourth columns display the SPM (t) statistic as a function of the gait cycle, with critical threshold (t) values located on the right axis. Highlighted areas indicate ranges where statistically significant differences exist compared to gait without perturbation, where: df - dorsiflexion, pf – plantarflexion, flex – flexion, ext – extension.
Figure 2.
The means and standard deviations of curves for the A. ankle angle joint, B. knee angle joint, C. hip angle joint, D. ankle torque, E. knee torque and F. hip torque throughout the gait cycle. The first column shows the curves for the affected limb without perturbation (black), with perturbation in the Initial Contact (red), Mid Stance (green) and Pre-Swing (blue) phase of gait cycle. The second, third, and fourth columns display the SPM (t) statistic as a function of the gait cycle, with critical threshold (t) values located on the right axis. Highlighted areas indicate ranges where statistically significant differences exist compared to gait without perturbation, where: df - dorsiflexion, pf – plantarflexion, flex – flexion, ext – extension.
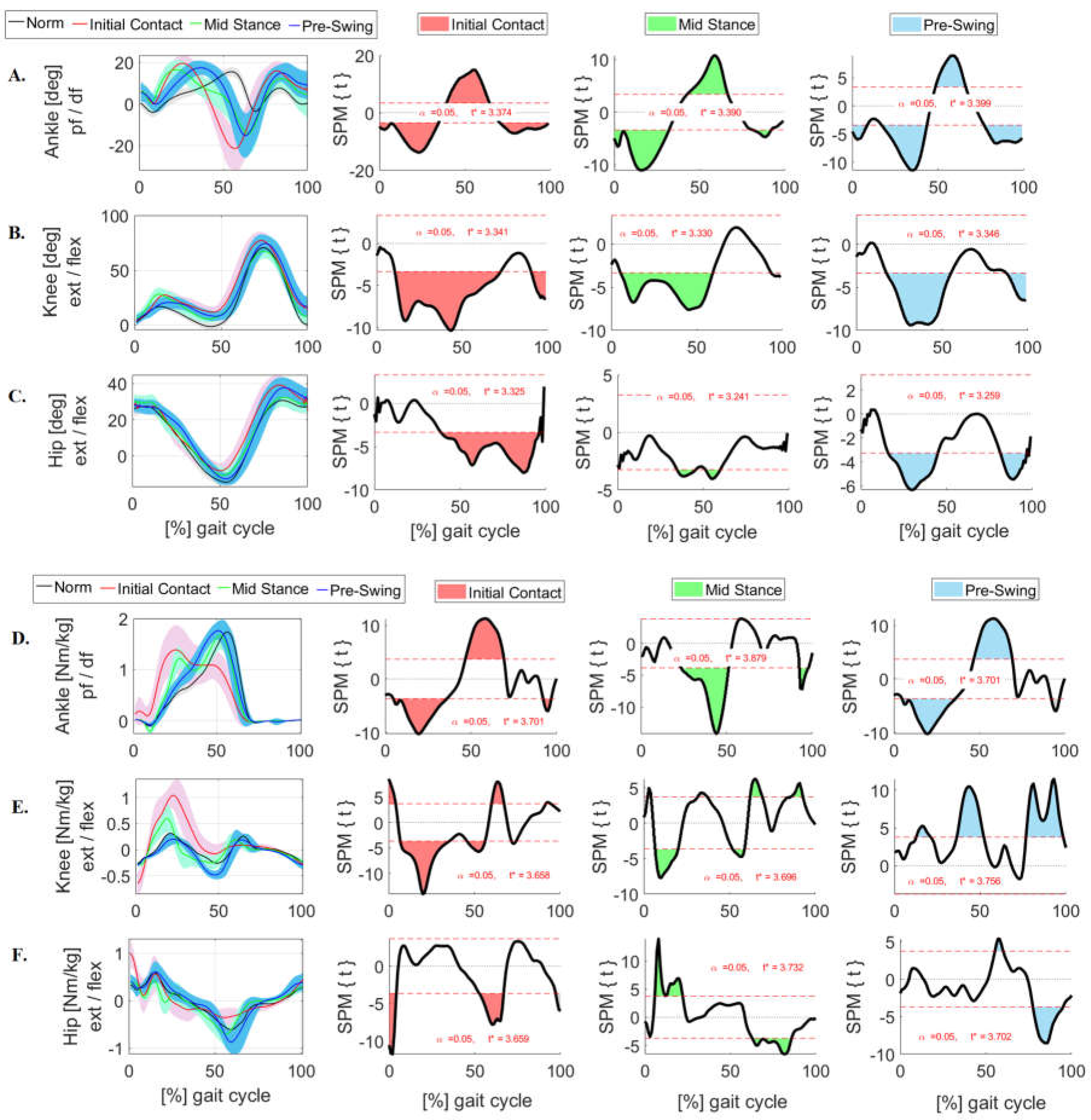
Table 1.
The impact of induced acceleration perturbation at the selected gait cycle phases: Initial Contact (IC), Mid Stance (MS), and Pre-Swing (PS) on the step length, compared to a standard treadmill gait (N).
Table 1.
The impact of induced acceleration perturbation at the selected gait cycle phases: Initial Contact (IC), Mid Stance (MS), and Pre-Swing (PS) on the step length, compared to a standard treadmill gait (N).
| Parameter | Initial Contact (IC) | Mid Stance (MS) | Pre-Swing (PS) | Treadmill Gait (N) | IC vs N | MS vs N | PS vs N |
|---|---|---|---|---|---|---|---|
| Stride length [m] | 0.13 ± 0.073 | 0.051 ± 0.029 | 0.142 ± 0.087 | 0.024 ± 0.019 | p = 0.0001 | - | p = 0.0001 |
Table 2.
Mean, standard deviation and percentages of extreme values for lower limb joint kinematics and kinetics curves containing the impact of induced acceleration perturbation at the: Initial Contact (IC), Mid Stance (MS), and Pre-Swing (PS) gait phases, compared to a standard treadmill gait (N), where: * – significance level p < 0.05. Negative values alongside percentages indicate the extent of decrease compared to treadmill gait or whether the sign has been reversed.
Table 2.
Mean, standard deviation and percentages of extreme values for lower limb joint kinematics and kinetics curves containing the impact of induced acceleration perturbation at the: Initial Contact (IC), Mid Stance (MS), and Pre-Swing (PS) gait phases, compared to a standard treadmill gait (N), where: * – significance level p < 0.05. Negative values alongside percentages indicate the extent of decrease compared to treadmill gait or whether the sign has been reversed.
| Parameter | Initial Contact (IC) | Mid Stance (MS) | Pre-Swing (PS) | Treadmill Gait (N) | IC vs N | MS vs N | PS vs N |
|---|---|---|---|---|---|---|---|
| Kinematic parameters [deg] | |||||||
| Ankle max (df) | 21.38 ± 3.96 | 17.66 ± 4.75 | 19.3 ± 3.54 | 15.65 ± 2.18 | 36.61% * | 12.84% | 23.32% * |
| Ankle min (pf) | -23.91 ± 9.42 | -16.92 ± 9.26 | -20.93 ± 8.22 | -4.96 ± 2.25 | 382.06% * | 241.13% * | 321.98% * |
| Knee max (flex) | 79.21 ± 8.34 | 68.08 ± 6.62 | 77.71 ± 6.69 | 70.56 ± 3.19 | 12.26% * | -3.51% | 10.13% * |
| Knee min (ext) | 3.66 ± 2.91 | 2.57 ± 3.85 | 3.4 ± 3.81 | -3.28 ± 1.95 | -211.59% * | -178.35% * | -203.66% * |
| Hip max (flex) | 40.25 ± 5.06 | 33.76 ± 4.41 | 38.92 ± 5.21 | 31.03 ± 3.19 | 29.71% * | 8.8% | 25.43% * |
| Hip min (ext) | -9.33 ± 4.15 | -11.34 ± 4.22 | -14.43 ± 3.57 | -14.72 ± 2.64 | -36.62% * | -22.96% | -1.97% |
| Kinetic parameters [Nm/kg] | |||||||
| Ankle max (df) | 1.57 ± 0.37 | 1.75 ± 0.23 | 1.88 ± 0.15 | 1.75 ± 0.09 | -10.29% | 0% | 7.43% |
| Ankle min (pf) | -0.08 ± 0.09 | -0.24 ± 0.04 | -0.11 ± 0.05 | -0.11 ± 0.04 | -27.72% * | 118.18% * | 0% |
| Knee max (flex) | 1.15 ± 0.29 | 0.64 ± 0.26 | 0.36 ± 0.05 | 0.33 ± 0.04 | 248.48% * | 93.94% * | 9.09% |
| Knee min (ext) | -0.66 ± 0.21 | -0.38 ± 0.08 | -0.51 ± 0.08 | -0.31 ± 0.03 | 112.9% * | 22.58% | 64.52% * |
| Hip max (flex) | 1.09 ± 0.23 | 0.55 ± 0.1 | 0.7 ± 0.16 | 0.61 ± 0.11 | 78.69% * | -9.84% | 14.75% |
| Hip min (ext) | -0.54 ± 0.15 | -0.78 ± 0.16 | -1.09 ± 0.23 | -0.63 ± 0.05 | -14.29% | 23.81% * | 73.02% * |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated







