Prerpints.org logo
Preprint
Article

How to Cristallize a Glass with a Femtosecond Laser

Altmetrics

Downloads

106

Views

77

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

23 April 2024

Posted:

24 April 2024

You are already at the latest version

Alerts
Abstract
Crystallization of glass through conventional thermal annealing in a furnace is a well-understood process. However, crystallization by femtosecond (fs) laser brings another dimension to this process. The pulsed nature of the irradiation necessitates a reevaluation of parameters for optimal crystallization and to understand the particularities in the case of using fs laser. This includes adjusting the laser pulse energy, the repetition rate, and the writing speed to either initiate nucleation or achieve substantial crystal growth. Therefore, a key challenge to this work is to establish reliable calculations for understanding the relation of the size of the crystallized region with respect to an ongoing transition (e.g., solid-to-solid, liquid-to-solid), and accounting for the aforementioned laser parameters. In this context and based on previous work, we simulate a temperature distribution (in space and time) to model the thermal treatment at any point in the glass. By setting the condition that the temperatures are between the glass transition and melting temperature, we can deduce the crystallized region size to be compared with experimental observations. For that purpose, the knowledge of the beam width at the focal point and of the absorbed beam energy fraction are critical inputs that were extracted from experiments found in the literature. After that, management of the crystallization process and the width of the crystallization line can be achieved according to pulse energy e.g. crystallite size, and also the effect of the scanning speed can be understood. Among the main conclusions to highlight, we disclose the laser conditions that determine the extent of the crystallized area and deduce that it is never interesting to increase the pulse energy too much but rather the repetition rate for uniform crystallized line.
Keywords: 
Subject: Engineering  -   Other

1. Introduction

3D localized crystallization in glasses brings interest as it introduces the possibility to precipitate crystalline phases that present optical nonlinear, ferroelectric, or pyroelectric, properties. For example, it leads to formation of non-center-symmetric crystals in optically suitable glasses [1]. This approach underscores that crystallization, a thermo-kinetics process [2,3], is influenced by temperature (and time), occurring between the glass transition temperature (Tg) and the melting temperature (Tm) of the given precipitated phase(s), and dictating nucleation and growth rates. While spatially selective crystallization using a femtosecond laser has been extensively demonstrated in various glasses [1], this process appears more complex than using a continuous-wave (CW) laser, arising from the pulsed nature of the laser (up to several MHz), and its high peak intensity triggering nonlinear processes. For example, in lithium niobium silicate glasses (LNS) [4], crystallization patterns of the LiNbO3 phase strongly depend on pulse energy or laser polarization.
While some physical aspects of the crystallization mechanisms have been understood, we aim to further explain and predict semi-quantitative aspects, particularly the correlation between laser parameters and specific optical or material properties. A goal is to minimize the number of experiments by performing calculations that facilitate a selection of relevant parameters to induce crystallization. For this purpose, we build upon a simple analytical solution developed in a previous work ([5], chapter 2), to physically describe the thermal dynamics induced by pulsed laser absorption and subsequent transformations.
In this work, we first simulate the evolution of the temperature distribution induced by a pulsed fs laser. Once the temperature modulation is known, it can be combined with time – temperature - transformation (TTT) diagram) for crystallization. The temperature calculation relies on modelling the energy source as spherical, and requires knowledge of several parameters:
1) the ratio (labelled Rτ) between the pulse period (τRR = 1/RR, with RR being the pulse repetition rate) and the heat diffusion time (τD = w2/4Dth, w being the light beam waist radius at 1/e at the focus, and Dth the heat diffusion coefficient or diffusivity). While Rτ depends thus on w, the latter usually in not reliably known due to the complexity of non-linear effects in the light-matter interaction. For this purpose, we performed a compilation of values extracted from literature permitted to clarify its dependency on the incident pulsed laser energy (Please refer to Appendix 1).
2) the fraction of the incident energy effectively absorbed by the material (labeled A). Absorption primarily occurs through nonlinear absorption (multiphoton ionization). Plasma reflection or scattering is also expected, reducing the coefficient A. After absorption of a small part of the pulse energy, the absorption becomes linear due to limitations of the electron plasma density [6] by a balance of multistep absorption, trapping, electron-electron or electron-phonon collisions. Given the lack of precise information about all these processes, extracted values from the literature (reported in appendix 2) enables one to set A values. As one is not aware about that, A is a function of the incident pulse energy, the pulse duration, and the material composition.
Another problem more specific to crystallization is the location of the crystallization domain in the TTT diagram in the confined condition of the tightly focused fs pulsed laser. Indeed, the specificities of the process may change the limit of the crystallization domain compared to the classical one [7,8,9]. Due to these uncertainties, we tested our approach using LNS glasses for laser conditions employed in [4,10,11] for which these aspects are relatively well known.
Paper is organized in such a way that following a brief recall on classical crystallization process (section 2), and temperature calculation from specific hypothesis (section 3), we are describing how the crystallization process behaves, firstly, within heat accumulation regime (later defined, see Section 4) and, secondly, without heat accumulation, see section 5, large temperature oscillations). Finally, we compare the results from the approach with respect to several experimental values according to pulse energy and scanning speed (section 6) and draw conclusion -1- about optimization of laser parameters, -2- that the width of crystallization area can be understood -3- that incident light energy fraction may change with the number of pulses.

2. Basic: Brief Recall on the Crystallization Process

The appearance of crystals in glass is a two-step process: nucleation and growth [2,9]. Crystallization is generally growth-limited at low temperatures and nucleation-limited at higher ones. Moreover, the domain of crystallization is defined from a TTT diagram [9]. It collects the time (called incubation time) requested at a given temperature to observe a first seed. This diagram, generally established for isothermal treatment, takes the time origin (t = 0) when the sample is in the condition to crystallize for a given temperature. This temperature is comprised between Tg and Tm. A boundary can be plotted in this TTT diagram delimitating the appearance of typ. 10-6 part crystallized in the glass volume (thick black line in Figure 1). The position of the crystallization domain in the TTT diagram is dependent on the treatment pathway. For example, its position will be shifted if the temperature is continuously decreasing (or not) from Tm [12]. In that case, the growth is delayed because the nucleation is slowed down. But for the purpose of this paper, we will ignore this and simply consider the thermal treatment curve experienced by the material at a given spatial position with coordinates α0,β0,γ0. The time-temperature applied to this position (T(α0,β0,γ0,t)). This function of time is simply obtained by the displacement of the temperature distribution function (the variation of the temperature in space) in the static case. This approximation is valid for speed smaller than the diffusion speed (2Dth/w, typ. a few cm/s). As we recalled above, the thermal treatment should exhibit temperatures in the Tg to Tm range. However, heating a material using a pulsed laser, resulting in oscillatory temperature in volume, and impacted by non-linear optical processes, is a complex problem that prevents to easily draw conclusions for managing the process. The important aspect of solving this issue is to take into account the effect of these parameters while preserving the main physics associated to the induced transformations. Consequently, we have developed in [13] a simplified approach for understanding how T(α,β,γ,t) is changing according to the laser parameters (pulse energy, pulse repetition rate, beam scanning speed, beam width and material properties).

3. Temperature Distribution and Its Evolution during Laser Writing

The 3D Fourier equation with a spherical energy source at the focal volume assuming a tightly focused laser beam and temperature independent physico-chemical properties is employed. Usually, for 3D direct laser laser writing, the focusing objective NA is above 0.5. This section is mostly didactic from what has been already published in [13]. The approximation taken into account are that we consider a pulse duration such that the thermal diffusion is not yet active, i.e., << 0.1 µs and a scanning speed (v) much lower than the diffusion speed (typ. few cm/s). Consequently, the shape of the spatial temperature distribution is not time dependent, the only time dependence is introduced by the beam scanning taken in the α direction and appears as α+v.t.. β and γ are fixed at the position α0 and γ0, with γ direction corresponding to the beam propagation axis. The scanning speed (v) defines the pulsed number deposited punctually as Np = 2w.RR/v. The laser pulse energy Ep defines the maximum temperature increment T00, introduced by a single pulse above an initial temperature Tinit: T 00 = A · E p π 3 2 ρ C p w 3 with A the fraction of absorbed energy (previously defined), ρ and Cp are the glass density and heat capacity, respectively. Additionally, a normalized radius rw=r/w is defined, with r being a radial distance from the center of the beam focus.
All calculated temperatures are normalized with respect to T00, as follow:Tnorm = (T-Tinit)/T00. Tnorm is oscillating at 1/RR period at any distance from the focus center. This temperature oscillation is comprised between a maximum (Tmax) and a minimum (Tmin), which varies according to the laser irradiation conditions. After a sufficient number of pulses, both Tmax and Tmin reach a steady state regime. Achieving this steady state regime is dictated by the ratio Rτ as previously defined and taking the form Rτ = 4Dth/RR w2. For instance, for large Rτ values (i.e. >16 for Tmin<0.03Tmax) the pulse contributions are independent of each other whereas for smaller Rτ values (<16), heat accumulation from pulse to pulse contribution begins to appear (and thus Tmin overcomes 0.03Tmax).
NSS corresponds to the minimum number of pulses necessary to reach the steady state regime. Its expression is given in eq.A4 in Appendix 3. This calculation can be performed either for Tmin and Tmax.
The temperature spatial distributions of Tmax and Tmin (normalized in the form of Tnorm) for various Rτ values are provided in Figure 2. When R τ is small (large RR or small diffusivity), Tmin and Tmax have no large relative difference compared to their average values because the oscillation amplitude is always limited to exp ⁡ − r w 2 whereas Tmax and Tmin amplitude is converging to 2/ R τ at the beam center but converges to π R τ .   r w erf ⁡ r w elsewhere on heat accumulation. When this situation can be reached, the pulse energy can be adjusted to compensate for a RR increase. It is also worth to note that, when R τ decreases, the shape of the curve converges to erf(rw)/rw curve (see Eq. A6 Appendix 4). It results that the temperature distribution decreases much slower for the radius increasing than a gaussian one. This last shape is the shape of the energy source and of the temperature distribution for the case where R τ is large. Note that in the case of heat accumulation, the overhal maximum temperature (at the center of the beam actually) is defined by A.Ep.RR i.e. roughly the average incident power.
For large R τ values, corresponding to small RR and / or large Dth, the oscillations are relatively large as pulse contributions are separated and thus Tmin appears to have small values (See Figure 2c). If we consider negligible being Tmin smaller than 3%.Tmax then R τ > 16 (for smaller than 6%.Tmax then R τ > 7 . For R τ > 10 , Tmin is smaller than 4.4%Tmax). This defines the limit of the domain of heat accumulation. The increase of R τ (Figure 2a–c) clearly show that the shape of Tmax is also converging with increasing R τ to the shape of the beam energy distribution (a gaussian beam, with an envelope having a spatial distribution following exp ⁡ − r w 2 ), therefore and in the limited case becoming independent on R τ . The maximum absolute temperature at the center is T00 in this case. It is defined by Ep only (independent of R τ and so especially on RR)
For intermediate R τ values, exemplified in Figure 2b-c, the temperature oscillations are limited between Tmax and Tmin. This is shown with particular cases with energy source volume as a shoe box in [14] for R τ = 2   a n d   20 or in [15] for R τ = 20 .
But, in this paper, we specify that when rw>2, the difference between Tmin and Tmax is vanishing as seen in [16].Therefore we can deduce in what conditions of rw and R τ , the temperature oscillations can be neglected and the use of an average temperature Tmean might be applicable (Appendix 3 Eq.6-8).
Another important point for this current work is the dependence of the full width at half maximum (FWHM) of the temperature distribution with respect to R τ . Its variation is less than a factor 2 in the relative radius scale (rw). In fact, the largest change is at the pedestal of the distribution.

4. Case of Low Rτ Values (High RR or Low Dth) i.e. within Heat Accumulation Regime

Here, Rτ is such that it is much smaller than 10 e.g. Rτ=0.1, due to the use of large repetition rate or a weakly thermally diffusing material. Rτ=10 is the limit for Tmin=4.4%Tmax., corresponds to Tmin very close to Tmax. In that case, a pulse contribution has no time to vanish significantly before a next pulse absorption induced another temperature increase. It is the case of heat accumulation and the behavior is like the one for Continuous Wave (CW) lasers.
In this section, we deal with conditions where the pulse number received punctually (Np as defined earlier) is above the number of pulses required to reach the steady state (Nss). This situation yields to two possibilities for which the temperature is higher than Tg : i) the temperature remains lower than Tm, and ii) the temperature overcomes Tm for some distance from the center of the beam focus during the thermal treatment. Each situation is described below.
i)
Moderate Ep leading to a temperature lower than Tm
To describe the temperature evolution and for illustration purposes, we set the laser parameters so that the temperature values fall within acceptable temperature interval. In this section, we took for the moment as example Tg = 579°C coming from the physico-chemical properties of LNS glass and Tm = 1257°C (Tm is the melting temperature of the formed LiNbO3 crystals in LNS). Additional data requested for the calculation, are provided in the table in Appendix 3. Therefore we used low absorption fraction (A) and pulse energy (Ep) to low values, provided in the caption of Figure 3. The beam width at 1/e has been fixed to a “reasonable value” for such small pulse energy, i.e., w = 1µm. In this condition, similar to Figure 2a, the oscillations (eq. A2 in Appendix 4) are small enough to be neglected. This can be observed in Figure 3, rendering Tmean a valid approximation if requested.
Then, the temperature distribution is transformed into time domain to obtain thermal treatment curves (Figure 4b-c, T-t dependency) replacing the static normalized radius r w by the coordinate of the place we are looking at in the moving material r w d , taking the beam center as a reference(see Figure 4a). The relative position in the beam of this point is related to relative time from the following relation: r w d ( t , v , d , β ) = ( v . t / w + d ) 2 + β 2 . Then, the time origin of the treatment for crystallization is defined when T starts to overcome Tg. This occurs at a distance d from the center of the temperature distribution i.e. at an abscissa d dependent on β (distance from the line axis) i.e. d 2 = r w d g 0 2 − β 2 . r w d g 0 , the radius of the isotherm Tg, is then obtained by solving Tmean( r w d g 0 ,Rτ)=Tg/T00 where Tmean is an average temperature defined in appendix 4 eq. A6. r w d g 0 is the also the maximum width of the crystallized region.
Figure 4 display a time-temperature thermal treatment. Therefore, provided that the crystallization domain is known (TTT diagram, see above) the scanning speed must be adjusted to ensure the thermal curve intersect with the crystallization domain as in Figure 5 at speed v1 or v2. For the speed higher than vlim, the curve does not touch the domain anymore and the crystallization is not possible. We see also that for speed v1, crystallization starts from bottom side of the domain and then crossed it and is thus not limited in growth. However, if Tmax is not so large (Tmax < Tm), the growth can be controlled. For v2, crystallization starts from the growth side and is sensitive to the pre-existence of nucleation seeds.
It is also important to note that r w d g 0 is in principle larger than the half-width of the crystallized region. Indeed, r w d g 0 is a circle where t=0, but the crystallization occurs eventually after some incubation time that could be longer than at the center, due to the Tmax decrease when going to the line edge. This contributes to a narrowing of the line but this effect is small as shown in [17] Figure 4, we see that at the speed limit, the width is not vanishing. The disappearance of the crystallization is thus arising from the process described in Figure 5. The width of the crystallization area is weakly sensitive to the scanning speed for a solid to solid transformation. This valid the criterion on Tg and Tm used in our approach.
ii)
At the periphery of the line (e.g. for β =1.2 in Figure 4), the temperature is smaller than the one at the center, but the crystallization condition remains somewhat similar : the treatment curves cross the crystallization domain almost at the same incubation time. From this view, the results are rather intuitive. First, a lower scanning speed would lead to a larger crystal growth. Second, there is a minimum laser power (P = Ep.RR) necessary to overcome Tg, with a maximum scanning speed necessary for the thermal treatment curve to cross the crystallization boundary. As the power increases, the speed limit increases until the maximum in space of the average temperature reaches Tm. Beyond this point, the crystallization process is different, and is the topic of the next section. Large Ep yielding temperatures overcoming Tm. In this case, if crystallization occurs, it starts from the liquid phase, with the system potentially crystallizing as the temperature decreases below Tm. Similar to previous situation, d(β) is defined by d 2 = r w d m 0 2 − β 2 . r w d m 0 , which is the radius of the isotherm Tm, (i.e. a circle with a radius smaller than the one for Tg), is then obtained by solving the following equation that includes Tm:.Tmean( r w d m 0 ,Rτ)=Tm/T00. r w d m 0 is also the maximum size of the melted region. We have β< r w d m 0 , dashed blue curve in Figure 6. When r w d m 0 < β < r w d g 0 (green curve), the results of section 4i apply. Then, the scanning speed should be adjusted to ensure the treatment curve to reach the crystallization domain. We see that in this case, entry into the crystallization domain is necessarily from the top side (nucleation limited). It is sensitive to the side stimulation because the side has crystallized before.

5. Case of High Rτ Values (Low RR and Large Dth) i.e. Out of Heat Accumulation Regime

Here, Rτ is such that it is larger than 10, due to the use of small repetition rate or a strong thermally diffusing material. In that case, a pulse contribution has enough time to vanish significantly before a next pulse absorption arrival. There is no heat accumulation but as we can see in Figure 2c, this is true only for r w smaller than approximately 3. For the periphery of the heat affected region, there is a smoothening of the temperature oscillations due to heat diffusion.
On the contrary, for smaller reduced radius, Tmin is close to zero, and the temperature oscillates between Tmin and Tmax during each period. Assuming that the pulse number received punctually (Np) is above the pulse number to reach the stationary state (Nss formula eq.A4 Appendix 4), we can simulate the temperature oscillations at the steady state simply. Note that the oscillation at this stage is due to a series of pulse contributions collected in the function T o s c ’ r w , x , R τ where x (between 0 and 1), is the temporal position in the period and is thus the floating value of t.RR. The simulation function of the temperature called SimpulseT is S i m p u l s e T r w d t , v , d , , R τ , E p / T 00 E p = T m i n ( r w d t , v , d , , R τ ) + T o s c ’ ( r w d t , v , d , , x ( t , R R ) , R τ ) (see eq A9 appendix 4) with r w d defined as in the previous section and related to the time origin for crystallization according to one of the two cases for which the temperature is above or below the melting temperature Tm.
i)
Moderate Ep such that the temperature is not overcoming Tm
As we can see in Figure 7, the punctual thermal treatment is beginning when Tmax.T00 is overcoming the melting temperature for the first time during the scanning. The relative position in the beam is translated in relative time from the following relation r w d ( t , v , d , β ) = ( v . t / w + d ) 2 + β 2 : d(β) defined by d 2 = r w d g 0 2 − β 2 with r w d g 0 solution of Tmax( r w d g 0 ,Rτ)=Tg/T00.
However, the existence of oscillations between Tmin and Tmax are such that there are dead times, defined as the time for which the temperature decreases below Tg. Consequently, the efficiency, taken as the ratio between the active time (during which Tg<T<Tm) and the thermal treatment time, is much smaller than one. This is different from small R τ values; again for which Tmax is close to Tmin, and a Tmean can be employed.
The time for which crystallization is possible (as per the definition of efficiency before) is computed by solving the following equation: SimpulseT(rw,x,Rτ)=Tg. This gives the temporal interval x1, x2 graphically defined in Figure 8a during the pulse period and for which the temperature is above Tg according to rw i.e., the normalized distance to the beam center.
As we can see in Figure 8b, the efficiency of the thermal treatment (defined as x1-x2) is a function on the distance of the position away from the beam center (rw). This reduction of the efficiency means that to reach crystallization around the periphery of the beam, the scanning speed must significantly be decreased. In fact, in those conditions, the thermal treatment time is much smaller than the irradiation time. We also pinpoint that beyond some departure from the center (e.g. here β > 0.7, i.e., in the direction perpendicular to the scanning direction, with the parameters used in Figure 7), the crystallization is no more possible from kinetics reason (T<Tg). This r w d g 0 defines the maximum width of the crystallization region.
ii)
Large Ep such that the temperature is overcoming Tm
In that case, a new treatment begins when a pulse oscillation is decreasing below Tm assuming that material melted before.
The use of SimpulseT function is valid. Using this expression, we detect the position in the period (x3 in Figure 9a) for which the temperature has overcome Tm and returns below it. This position is the starting time for thermal treatment that ends at position x1. But if a next temperature pulse induces temperature overcoming Tm, as the material is expected to melt and thus lost its memory, there will be a new time origin to compute from this next pulse. So, the last pulse defines the beginning of the treatment that goes on afterwards, i.e. when Tmax is decreasing below Tm in the course of the scanning. This is obtained by solving SimpulseT(rw,x,Rτ)=Tm. This yields positions x3 and x4 in the period as shown in Figure 9b.
As we see in Figure 9, just a part of the pulse is “active” to induce crystallization (x2‐x1<1) and just a part of the temperature distribution is also possible. Following the picture in Figure 9b, only the tail of the temperature distribution is used. When T is no more overcoming Tm, and as shown in Figure 10 with d=1.92 for β=0, x3 and x4 do not exist anymore and until x1 and x2 still do, the temperature is overcoming Tg.
From the Figure 9a above, it can be noticed that the efficiency for crystallization is again smaller than 100% and that the writing speed must be decreased to trigger the crystallization. Regarding the sides of the line (β≠0), the maximum of Tmax is decreasing with y and the process described here will stop when Tmax will not overcome Tm anymore ( r w d g 0 <β< r w d m 0 ). For these β, the situation is the same as the one described at the beginning of this section. The transition is solid-solid and starts from the front of the T distribution i.e. before the line center (in reference to the laboratory clock).

6. Application to the Case of LNS Glass

The next section is dedicated to applying the above findings to an experimental case using LNS glasses. The objective of this section is to show how the width of the thermally transformed region is linked to the laser parameters especially to the pulse energy. It is based on real case for which the beam waist and fraction of absorbed pulse energy (A in T00 expression, equation A10 Appendix 4) are crucial information, believed much smaller than we show in this paper. After data analysis from different authors, we have pointed out that the dependence on pulse energy of the beam waist w is not very sensitive to the material when comparing silica and LNS family (Appendix 1). On the contrary, the pulse energy dependence of A (appendix 2) is sensitive to the pulse duration and to the material. Therefore, the methodology in this section is to start with the pulse energy, the numerical aperture, and the pulse duration, to show that 1- the beam waist and the fraction of absorbed energy can be defined with enough accuracy for the purpose of this paper, and 2-. then with these quantities and their dependence with the laser parameters established reliably enough, to draw conclusions on the laser induced crystallization process.
We start from low pulse energy (0.5-1 μJ). The experimental half width of crystallized area is measured to be 1.65 μm (using 0.7 μJ in [4]) with the following laser parameters: wavelength 1030 nm, pulse duration 300 fs, RR = 300 kHz, NA = 0.6, v = 5 μm/s [4]. With these values, we know that solid-solid transformation (crystallization, ref [4]) occurs. On the other hand, the presence of nanogratings [18] indicates the size of the laser beam as these structures are only created by light. We deduce that w = 1.65 μm. However, we have to adjust A. There are different processes that contributes to this fraction. The first one is the reflection of light (from Fresnel, or from the electron plasma mirror that is formed by the electron excitation in conduction band or also some light scattering). The second one is that if a part is absorbed, a part is also transmitted as the absorption is never total in transparent materials with non-linear absorption. Since the modification is a solid-solid transformation, the temperature does not overcome Tm (as shown in the previous sections), and the value of A has to be smaller than 0.09 for 0.7 μJ. This value falls within the values found in the literature and presented in Appendix 4 Figure A2. Once all of these parameters are known, the simulation can be carried out similarly as presented in the previous sections.
The results are summarized in Figure 11 and below:
-
The possible crystallization would fall within a fraction of second for v = 5 µm/s provided that the efficiency was 100%, but this is obviously not the case. On the contrary, the maximum efficiency is 22% here (see Figure 11b). The real treatment time is thus (x1-x2) multiplied by the time scale of the Figure 11c. On the other hand, as crystallization is actually observed in LNS for v up to 125µm/s [17] in a 0.5-1 µJ interval, 1030 nm, NA = 0.6, τRR = 250 fs, RR = 200 kHz, w = 1.6 µm (for which the steady state is reached). This leads to position the crystallization nose in the time range of 10 ms, i.e. an order of magnitude lower than the isothermal treatments in conventional furnace, in large volume without stress field [11].
-
The calculated crystallized half width is close to the beam waist one, with the criterion that the temperature should be above Tg. We conclude that there is almost no line broadening originating from the thermal effect for this pulse energy as it is experimentally observed.
Now, and based on the above discussion, one can wonder how does width evolve when Ep is increased. To answer this aspect, we will deal with two distinct examples, at low and higher pulse energies.
First example: We know that solid-solid transformation occurs until 1.0 µJ and that for higher pulse energy, a part of the crystallized region originates from a liquid to solid transformation. For instance, let us consider the pulse energy 1.3 µJ. For this value, the half width of the crystallized region is 3.73 µm. We can measure the laser beam radius from [4] reported in Figure A1 at 2.6 µm. Rτ is thus decreases from 4.4 to 1.8 with the pulse energy increase. The indirect variation of Rτ with the pulse energy was not expected. It pushes the system to be in heat accumulation regime even if RR is constant. Additionnaly, A increases itself to 0.15. Using these data, the computation is summarized in Figure 12.
The provided results indicate that
-
The efficiency is close to 100% due to the effect of heat accumulation that increases Tmin above Tg.
-
The calculated crystallized radius is found to be at 1.25w. There is thus a small broadening of 25% compared to the beam waist w. There is thus a crystallized part that is not submitted to the beam where there is no submicrostructuring induced by the laser light. There is also a melted region smaller than the beam waist (half width = 0.48w). We see also that there is no obvious correlation between the beam width and the melted region.
For completeness, another experimental result available for 1.8 µJ has been computed and reported in the Table 1. It shows reasonable agreement with the experimental results.
Second example: considering much higher pulse energy, let’s say 4.2 µJ as used by Veenhuizen et al. [10], with laser parameters as follows 175fs, 200 kHz and varied scanning speed v. The authors of the aforementioned reference observed a crystallization full width of 31.3 µm for 15 µm/s and 9.82 µm for 75µm/s i.e. a significant variation. From Figure A1 in Appendix 3, w seems to be around 4.5 µm and thus Rτ=0.9, A can be evaluated to 0.32 from Figure A2 in Appendix 4. Results are shown in Figure 13.
The Figure 13 allows a comparison of the treatment originated from the solid region and from the melted regions. We can see that the two curves penetrate the crystallization domain after a similar incubation time. However, in real time, the first one starts in front of the beam at a distance 3.4w from its center, that is before the temperature maximum whereas the second starts 1.7w after the temperature maximum. Consequently, the thermal treatment curve for the periphery is penetrating the crystallization domain before the one for the center. The periphery is thus in situation to stimulate the nucleation of the melted region. In addition, as both of treatment curves have almost no oscillations, the efficiency is obviously 100%.
Let us examine now, the effect of the scanning speed as reported in [10]. A large variation of the width of the crystallization region was found (by a factor 3), whereas the speed varied by a factor 5. The origin of this variation is not arising mainly from the time shift of the treatment curves with the speed as we can see in Figure 5. The effect is quite small. Another possibility could be from the pulse number received punctually and that could be smaller than the quantity for reaching the steady state. The steady state is reached after Nss = 7.6 104 pulses (from Eq. A4 Appendix 1), whereas the number of pulses received punctually Np is 1.2 104 pulses for v=15 µm/s. However, for v=75 µm/s, Np=2400, which is significantly lower than Nss (>30 times less). Consequently, the steady-state situation is not reached at those speeds. On the other hand, we can see from Figure 2 that the width at the beginning of the irradiation is like for (Rτ large, separated pulse contribution) and increases in the course of irradiation due to heat accumulation. To compute the width in the transitory stage, we assume here, that T( r w d g 0 ) crosses Tg for r w d g 0 >2. The use of Tmean is thus a good approximation. We have therefore to solve the equation below, for deducing r w d g 0 according to the number of pulses. It reads (from Eq. A8 Appendix 4):
T ¯ r w d g 0 , R τ , N = π R τ . r w d g 0 . erf ⁡ r w d g 0 − e r f r w d 0 1 + N . R τ = T g T 00   w i t h   T 00 = A . E p π 3 / 2 ρ C p w 3
The increase of the width, as a function of the pulse number is plotted in Figure 14. We see the broadening with the number of pulses that arises from heat accumulation as Rτ is around 1. But we find r w d 0 =3.5 for the speed in consideration, i.e. a decrease from 3.8, the value at the steady state. This does not account for variation by a factor 3. Even, if we consider the beginning of the irradiation, the possible variation would only be by a factor 2. On the contrary, results reported in [17] and used in Figure 11 are in agreement with this explanation (Laser parameters are: λ = 1030 nm; RR = 200 kHz; NA = 0.6; pulse duration = 250 fs). In that case, Nss is around 50 whereas Np>5280 for the speed lower than 125 µm/s. The steady state is always reached and the width is weakly varying as experimentally observed (the explanation is given about Figure 5).
The question is thus: is there other reasons to change the crystallization width? The difference between the beginning and the steady state could be the change of absorption due to the creation of defects in addition to the non-linear one, that play role of trapping centers of excited electrons produced by previous pulses. It is worth noticing that just a variation of A from 0.135 to 0.32 may explain that r w d g 0 change from 1.6 to 3.8.
On the other hand, it is observed in [10] that for a given pulse energy above some speed, the crystallization is only solid to solid transformation. This means according to the calculation that Tmax is no more overcoming Tm. This is in agreement with lower absorption at larger speed. Furthermore, the observation of the shift of this turning point (called here vt) with the pulse energy leads to consider that a particular dose D0 is necessary for the absorption increase and for A to reach the value at the steady state. We would have therefore, the following relation: Ep.2w.RR/vt=D0. So, it would be possible to have an increase of temperature disconnected from the heat accumulation but arising from an increase of absorption related to defect creation.
Finally, from the Table 1, we can see that the steady state width of the crystallized region varies with the pulse energy for two reasons: because the beam waist increases with Ep and because the temperature distribution expends relatively with the beam waist because Rτ decreases and the interaction is thus more and more sensitive to heat accumulation and also because A increases on pulse energy.
Table 1. sum up of experimental and computed width according to the pulse energy.
Table 1. sum up of experimental and computed width according to the pulse energy.
Ep(µJ) Pulse duration τp (fs) w(µm) A Rτ rw(crystal)
computed		 rw(crystal)
experimental		 rw(melt)
computed		 rw(melt)
experimental		 ref
0.5 300 1.23 0.035 7.9 1 uk na na This work
0.7 300 1.65 0.09 4.4 1.05 1.00 na na [4]
1 300 2.23 0.12 2.4 1.18 uk na na This work
1.3 300 2.6 0.15 1.8 1.47 1.6 0.48 1.1 This work
1.8 300 3.0 0.2 1.3 2.29 1.86 1.02 uk [4]
4.2 175 4.5 0.32 0.9 3.79 3.50 1.71 uk [19]
N.B.: na means not applicable and uk means unknown.

7. Discussion

Considering laser-induced crystallization, analytical formula can be used to compute the temperature oscillations in time when the steady is reached. These oscillations are limited between a maximum and minimum temperature, whatever the laser parameters and material properties, and established in the frame of a spherically symmetric energy source [13]. The broadening effect when increasing the pulse energy or the pulse density (inversely proportional to the beam scanning speed) was simulated.
We have shown previously how the spatial distribution and specifically its FWHM is changing during the transitory to a steady state depending on the ratio between the pulse period and the heat diffusion time (defined as Rτ). On the other hand, the temperature magnitude is defined by the beam waist and the fraction of absorbed incident energy (A). We show that when applied to real case and reliable simulation is requested, it is necessary to consider 1) an increase of the beam waist (w) with the pulse energy, and 2) the increase of the A factor with the pulse energy and the pulse duration. Such constraints that originate from non-linear effect in the solid, can only be extracted at the moment mainly from the experimental results available in literature (see Appendix 1 and 2) as mechanisms in the case of fs laser absorption are not clear enough for a robust simulation.
In such a way, within the approximation we used, we can deduce the following:
1 - The crystallization management is facilitated in a heat accumulation regime (Rτ<<10). This allows the temperature oscillations to be quite small compared to average temperature values, thus, the laser parameters (i.e. Ep, R) can be easily adjusted to be completely above the Tg and below Tm during the thermal treatment time that is starting when the temperature is overcoming Tg. We were thus able to deduce thermal treatment curves. The scanning speed is also straightforward for the thermal curve to efficiently penetrate the crystallization domain. The crystallization process will originate from a solid-solid transition, and the width of the crystallized area is close to the beam waist. For the inorganic oxides, the thermal diffusivity is usually around 10-6 m2/s (within a factor 2) so the diffusion time from a source with a beam waist of 1 µm is around < 0.5 µs. We are in the above conditions when the repetition rate frequency is much larger than 0.4-0.2 Mhz. However, if the focusing is not so tight, let say w = 2 µm, the heat diffusion time is four times larger and reaches 1-2 µs. So, the repetition rate has to be much larger than 5-10 MHz. However, in organic materials for which the diffusion time is 10 – 20 times longer, the heat accumulation regime is more easily reached and the thermal width more easily predictable.
If, the pulse energy is increased such that the temperature is overcoming the melting temperature around the center of the written line, the process changes, the starting time of the incubation is no more when the temperature overcomes Tg but when T decreasing below Tm as the process is a liquid to solid transformation. The crystallization domain is penetrated from the growth side renders the process sensitive to external stimulation, in particular to crystallization induction from the periphery where the temperature does not overcome Tm. As departing from the center and the temperature maximum of the treatment curve decreasing below Tm, a solid to solid transformation is occuring again. There are two regions in the crystallized line. It is shown that the solid to solid transformation region crystallizes sooner than the melted one and thus are source of nucleation for the melted one. The size of the crystallized region overcomes largely the beam waist in that case. The texture orientation of the line (if any) is thus defined by the peripheral region out of the beam and so defined mainly by the temperature gradient orientation. The large broadening with the pulse energy is defined in this case mainly by the pedestal of the temperature distribution (which follows a hyperbolic shape (T~1/rw) although beam waist and absorption fraction gives rise also to a broadening contribution. We pointed out also a unexpected result: the stimulation of heat accumulation regime on pulse energy increase and not only on RR.
2 - On the opposite, when heat accumulation is small or negligible (Rτ>10, small RR, large diffusion time), the above processes are the same according to the pulse energy but the temperature oscillates largely in proportion. It results that just a small part of the oscillation is efficient (a few % or tens). This contributes to decrease proportionally the scanning speed in addition to the repetition rate that could be here smaller.
However, the weak efficiency for growth rate and a moderate pulse energy allows to penetrate the crystallization on the growth limited side of the crystallization domain and may allow to produce independent nanocrystals that have been revealed to be orientable with the laser polarization in the case of LNS glasses [20].
3 - We pointed out that the absorption may increase in the course of the irradiation and produce a large increase of temperature changing the crystallization process (from solid/solid to liquid/solid). This effect could be dose dependent (D0=Ep.2w.RR/vt) and leads to a transition speed vt varying with the pulse energy. We suggest that this phenomenon arises from defects formation more easily to ionize.

8. Conclusions

Using analytical formula computed from very simplified approximations (spherical approximation), we show that it is possible to use them for simulating crystallization processes by irradiation with a fs laser. However, for obtaining compatible results, the beam waist and the light absorption fraction have to be approximately known beside the physico-chemical data of the glass. After that, management of the crystallization process and the width of the crystallization line can be achieved according to pulse energy e.g. crystallite size, and also the effect of the scanning speed can be understood. For example, using the example of LNS glass, we show, in particular, that the crystallization process may changes with the scanning speed.
Lastly, the application of the temperature calculation can be extended to other processes depending on the temperature – distribution of the fictive temperature, viscoplastic effect (stress distribution), any chemical reaction that is thermally activated, chemical migration or the maximum energy not erasing type X or type II modification or luminescent defects creation or decomposition temperature of organic material. This management shall be even more accurate when fs laser is applied to organic matter in order to avoid complete material decomposition.

Author Contributions

Conceptualization, B.P.; funding acquisition, M.L.; investigation, R.Q. and B.P.; methodology, B.P.; project administration, B.P. and M.L.; resources, M.L.; supervision, B.P.; validation, R.Q.; visualization, M.C.; writing—original draft, B.P.; writing—review and editing, R.Q., B.P., M.C. and M.L. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by Agence Nationale de la Recherche (ANR), FLAG-IR Project, award number ANR-18-CE08-0004-01, and REFRACTEMP project, award number ANR-22-CE08-0001-01. R.Q. acknowledges the China Scholarship Council (CSC) for the funding No. 201808440317 of her PhD fellowship.

Data Availability Statement

Data is contained within the article.

Conflicts of Interest

The authors declare no conflicts of interest.

Appendix 1 The Beam Width from the Literature and from Our Experiments

For that, we used the width of modification lines achieved by non thermal effect. The most obvious one is the line width of type II nanogratings as their structure is achieved only by the laser light whatever the material chemical composition.
We can first consider the beam focused with a length with NA and the effect of self-focusing. The formula for effective width at the focus in the material is given by Marburger et al. [21], Ashcom et al. [22] and Schaffer et al. [23] but their formula below does not take into account the defocusing effect due to the electron excitation. The defocusing effect is dependent of the excited electron density during the pulse and thus a too short lifetime or a weak pulse energy in the excited state of the electron may lead to the absence of this effect. The effective beam waist radius is given below:
w e f f = λ 2 / 0.9 π 2 π N A 2 1 − N A 2 + I . λ 2 P c r with P c r = 3.77 λ 2 8 π n 0 n 2 n2 being the non linear refractive index (3.2 10-16 cm2/W [24,25]), n0 the relative one, I the light intensity.
We can see that increasing the pulse energy, the intensity increases and thus the width decreases due to non-linear effects. It is the contrary of what it is observed in the case of laser leading to electron excitations [26].
In Lei et al. ([27], ), the width increases with the energy above 0.2 μJ although the electron density is of the order of 0.01 excited electrons.nm-3. Even for 0.1 excited electrons.nm-3, it is said that there is a weak defocusing effect and the half width is 0.4 μm. However, without defocusing effect, the width should decrease on pulse energy increase.
These results and others are collected in table below
Table A1. some half width according to pulse energy, numerical aperture, and materials.
Table A1. some half width according to pulse energy, numerical aperture, and materials.
Wavelength (nm) Pulse energy (μJ) NA Half width (μm) Material ref
1030 0.1 0.6 0.31 silica Ashcom et al. [28]
1030 0.5 0.6 0.24 silica idem
1030 1 0.6 0.1 silica idem
1030 0.19 0.3 0.4 silica Lei et al. To be published in LPR Figure S2
1030 0.55 0.3 1.25 silica idem
1025 0.7 Not known 1. Beresna et al. [29] (in air) Figure 4
1030 1 0.6 2 silica Qiong et al. [30]
1030 0.5 0.6 1.3 G1? idem
1030 0.6 0.7 B33 idem
1030 0.6 1.65 ULE idem
1030 3 0.6 2.1 ULE idem
1030 1 0.6 1.25 BGG-ZnO idem
1030 2 0.6 2.25 BGG-ZnO idem
1030 0.7 0.6 1.65 LNS Cao et al. [4]
1030 1.3 0.6 2.6 LNS Cao et al. [4]
1030 0.5 0.6 1.6 LNSB Muzi et al. [17]
1030 0.2 0.6 0.5 Suprasil Our work
1030 5 0.6 5 Suprasil Our work
1030 0.15 0.6 0.45 Suprasil Our work
1030 2 0.6 1.75 Suprasil Our work
800 0.18 1.35 0.4 glasses Gamaly et al.[31] Figure 3
1030 0.8 0.6 2 Zn BGG Yao et al. [32] Figure 2
1030 0.19 0.3 0.4 silica [33] Figure S2
1030 0.34 0.23 0.65 silica [33] Figure S2
1030 0.55 0.16 2.2 silica [33] Figure S2
Preprints 104662 g0a1
Figure A1. the half beam width according to the pulse energy from Table A1 for various compounds, λ=NIR, various NA, often at the steady state.
Figure A1. the half beam width according to the pulse energy from Table A1 for various compounds, λ=NIR, various NA, often at the steady state.
Preprints 104662 g0a1

Appendix 2 Fraction of Absorbed Beam Energy

The incident beam intensity from the laser is a parameter that is dependent of the material properties as well as the beam propagation especially on the focusing strength. Indeed, for working in volume, materials do not absorb linearly but non-linearly with an exponent of the intensity light that depends on the materials and on the pulse duration because it depends on the excited electron density that occurs in the few 10s fs [6]. The fraction of incident light absorbed is thus dependent on the intensity at the focus and around (before and after). In addition, the excited electron density may behave like a mirror and reflect a part of the incident intensity [34]. Moreover, for largest incident power, the excited electron density may have a defocusing effect that decrease the intensity and thus the non-linear absorption [26]. All of these processes are intricated and complex and leads to difficult prediction without heavy computations. As we need an estimate of this quantity for our computation, we have collected various results in the table below.
Table A2. fraction of absorbed energy according to pulse energy, pulse duration, numerical aperture of the focusing lens, and materials.
Table A2. fraction of absorbed energy according to pulse energy, pulse duration, numerical aperture of the focusing lens, and materials.
Wavelength (nm) Pulse energy (μJ) Pulse duration (fs) NA fraction material ref
800 0.1-0.7 50 0.5 0.2 silica Couairon et al. [35]
800 1 90 Not mentioned 0.35 silica Wu et al. [36]
800 2 90 Not mentioned 0.45 silica Wu et al. [36]
800 3 90 Not mentioned 0.54 silica Wu et al. [36]
800 0.06-0.12 100 Not mentioned <0.12 Metallic surface Meng et al. 2023 Materials Surface métallique
800 0.25 100 Not mentioned 0.25 silica Zhang et al. [37]
800 1/7.4 100 Not mentioned <0.1 silica Zhang et al. [37]
1026 4.2 175 0.58 silica Computed from Veenhuisen et al. [10] see text
1030 0.6 190 0.16 0.25 silica Wang et al. [38]
1030 1 190 0.16 0.36 silica Wang et al. [38]
1030 0.2 300 0.3 0.04 to 0.06 Silica from 1 to 30 pulses Lei et al. [39]
1030 0.6 300 0.16 0.05 silica Wang et al. [38]
1030 0.69 300 0.16 <0.15 silica Wang et al. [38]
1030 1 300 0.16 0.25 silica Wang et al. [38]
1030 0.5 300 0.6 0.035 LNS This work
1030 0.7 300 0.6 0.09 LNS This work
1030 1.3 300 0.6 0.16 LNS This work
1030 1.8 300 0.6 0.2 LNS This work
1026 4.2 175 0.6 0.32 LNS This work
1030 0.7 500 0.16 0.15 silica Wang et al. [38]
1030 1 500 0.16 0.19 silica Wang et al. [38]
1030 1 700 0.16 0.1 silica Wang et al. [38]
1030 0.1 800 0.6 0.15 LNSB-Eu Ari et al.[40]Figure 5a
1030 0.5 800 0.6 0.22 LNSB-Eu Ari et al.[40]Figure 5a
1030 1.2 800 0.6 0.24 LNSB-Eu Ari et al.[40]Figure 5a
Preprints 104662 g0a2
Figure A2. Plot of the absorbed energy fraction versus the pulse energy according to pulse duration (fs). The colors of the points make reference to the Table A2: the blue curves is for silica and the red one for LNS glasses. However, lines are just guides for the eyes.
Figure A2. Plot of the absorbed energy fraction versus the pulse energy according to pulse duration (fs). The colors of the points make reference to the Table A2: the blue curves is for silica and the red one for LNS glasses. However, lines are just guides for the eyes.
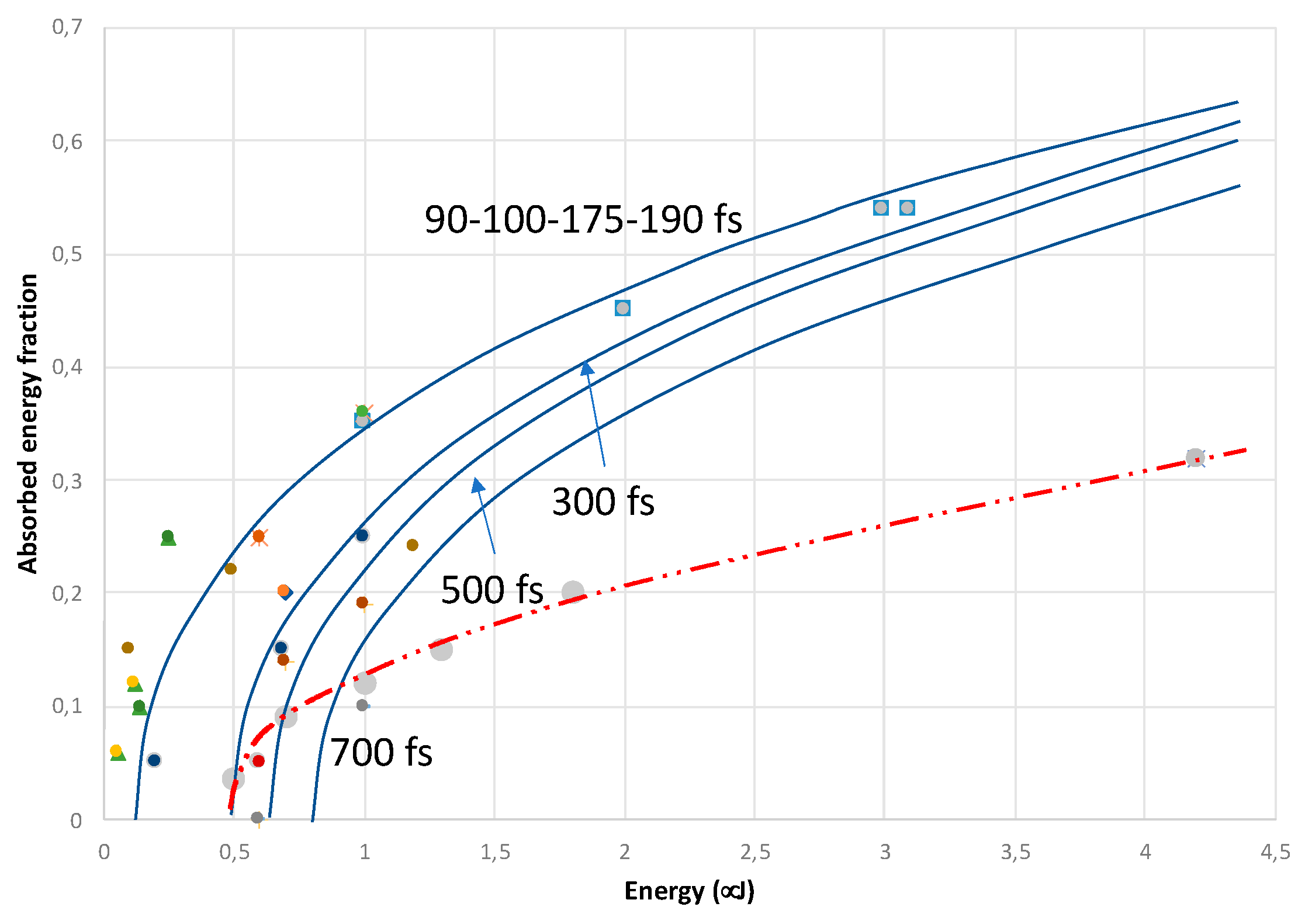
Preprints 104662 g0a2
The absorbed energy fraction is very sensitive to the pulse duration for < 3,7microJ but less above (Zhang et al. [37])

Appendix 3

Table A3. physico-chemical and laser parameters.
Table A3. physico-chemical and laser parameters.
LNS glass : 33Li2O-33Nb2O5- 34SiO2 (mol %) ref
specific density (ρ, kg/m3) 3830 Interpolated between SiO2 and NbLiO3.
Heat capacity (J/kg) 650 [41]
Κ=Dth.ρ.Cp (J/K.m.s) 2.65 Computed from diffusivity
Dth (m2/s) 9.1 10-7 [42,43]
Tg (°C) 579 [44,45]
Tm (°C) 1257 [44,45]

Appendix 4

After reaching the steady state (the number of pulses for that is given in Table 3 of [13]), the expressions of the temperature maximum, minimum and oscillations are:
T m a x r w , R τ = exp ⁡ − r w 2 1 + x m · R τ 1 + x m · R τ 3 2 + exp ⁡ − r w 2 1 + ( 1 + x m ) · R τ 2 1 + 1 + x m · R τ 3 2 + π R τ . r w e r f r w 1 + ( 1 + x m ) · R τ
With x m = R τ 9 R τ + 32 r w 2 − 3 R τ − 8 8 R τ with rw > 3 2 + 2 R τ ( x m l i m i t e d   t o   1 ) or x m = 0 , when, rw < 3 2 + 2 R τ whatever R τ or R τ less than 2 r w 2 − 1.5 when r w 2 > 1.5 ).
T o s c r w , R τ ≈ exp ⁡ − r w 2 1 + x m . R τ 1 + x m . R τ 3 2 + π R τ . r w e r f r w 1 + ( 1 + x m ) . R τ − π R τ . r w e r f r w 1 + R τ
T m i n r w , R τ = exp ⁡ − r w 2 1 + ( 1 + x m ) · R τ 2 1 + 1 + x m · R τ 3 2 + π R τ . r w e r f r w 1 + R τ
N s s m a x r > 1 R τ 2 R τ . ε . T m a x r w , ∞ 2 − 1 N s s m i n r > 1 R τ 2 R τ . ε . T m i n r w , ∞ 2 − 1
T m a x r w , R τ , N ≈ exp ⁡ − r w 2 1 + x m · R τ 1 + x m · R τ 3 2 + exp ⁡ − r w 2 1 + ( 1 + x m ) · R τ 2 1 + 1 + x m · R τ 3 2 + exp ⁡ − r w 2 1 + N − 1 + x m · R τ 2 1 + N − 1 + x m · R τ 3 2 + π R τ · r w e r f r w 1 + ( 1 + x m ) · R τ − e r f r w 1 + N − 1 + x m · R τ
When the mean T can be used T ¯ r , N = 1 τ R R ∫ p u l s e   p e r i o d a t   N T r , t d t , the formula is even simpler to be used after reaching the steady state. τRR is the period = 1/RR
T ¯ r w , R τ , ∞ = π R τ .   r w erf ⁡ r w               N s s m r r w = 1 R τ 2 . r w π . ε . e r f r w 2 − 1
T ¯ r w , R τ , N = π R τ .   r w . erf ⁡ r w − e r f r w 1 + N . R τ
Formula built for the simulation of the temperature oscillations at the steady state:
S i m p u l s e T r w , x , R τ , E p = T m i n r w , R τ + T o s c ' r w , x , R τ . T 00 E p
where T o s c ' r w , x , R τ is just the function T o s c r w , R τ where xm is replaced by x.
The maximum temperature induced by one pulse is T 00 = A · E p π 3 2 ρ C p w 3 with A the fraction of absorbed energy, ρ and Cp are the glass density and heat capacity, w the beam waist at 1/e and Ep the pulse energy. Eq. A 10
Note that the time is introduced in the formula by r w d which is a “moving” rw (as the beam is scanning) and in x by rendering it periodic i.e. in taking only the floating value of t.RR.

References

  1. Komatsu, T.; Honma, T. Laser patterning and characterization of optical active crystals in glasses. Journal of Asian Ceramic Societies 2013, 1, 9–16. [Google Scholar] [CrossRef]
  2. Neuville, D.; Cormier, L.; Caurant, D.; Montagne, L. From glass to crystal. Nucleation, growth and phase separation: from research to applications: EDP Sciences). 2017.
  3. Teng, Y.; Zhou, J.; Sharafudeen, K.; Zhou, S.; Miura, K.; Qiu, J. Space-selective crystallization of glass induced by femtosecond laser irradiation. Journal of Non-Crystalline Solids 2014, 383, 91–96. [Google Scholar] [CrossRef]
  4. Cao, J.; Lancry, M.; Brisset, F.; Mazerolles, L.; Saint-Martin, R.; Poumellec, B. Femtosecond Laser-Induced Crystallization in Glasses: Growth Dynamics for Orientable Nanostructure and Nanocrystallization. Crystal Growth & Design 2019, 19, 2189–2205. [Google Scholar]
  5. Que R 2022 Demonstration of anisotropy control of optical properties induced by IR fs laser irradiation in organic materials : birefringence, di-attenuation, SHG, photoluminescence.
  6. Lancry, M.; Groothoff, N.; Poumellec, B.; Guizard, S.; Fedorov, N.; Canning, J. Time-resolved plasma measurements in Ge-doped silica exposed to infrared femtosecond laser. Physical Review B 2011, 84. [Google Scholar] [CrossRef]
  7. Uhlmann, D.R. A kinetic treatment of glass formation. Journal of Non-Crystalline Solids 1972, 7, 337–348. [Google Scholar] [CrossRef]
  8. Weinberg, M.C.; Birnie, D.P.; Shneidman, V.A. Crystallization kinetics and the JMAK equation. Journal of Non-Crystalline Solids 1997, 219, 89–99. [Google Scholar] [CrossRef]
  9. Deubener, J.; Allix, M.; Davis, M.J.; Duran, A.; Höche, T.; Honma, T.; Komatsu, T.; Krüger, S.; Mitra, I.; Müller, R.; Nakane, S.; Pascual, M.J.; Schmelzer JW, P.; Zanotto, E.D.; Zhou, S. Updated definition of glass-ceramics. Journal of Non-Crystalline Solids 2018, 501, 3–10. [Google Scholar] [CrossRef]
  10. Keith Veenhuizen, Sean McAnany D N, Bruce Aitken, Volkmar Dierolf1 & and Jain H 2017 Fabrication of graded index single crystal in glass. Sci. Rep. 7,44327.
  11. Vigouroux H 2012 Etude de vitrocéramiques optiques pour le doublement de fréquence In: Sciences Chimiques, (University Bordeaux: University of Bordeaux, France) p 278.
  12. Yinnon H and D.R. Uhlmann D R 1983 ed D.R. Uhlmann and N.J. Kreidl: Academic Press).
  13. Que, R.; Lancry, M.; Poumellec, B. Usable Analytical Expressions for Temperature Distribution Induced by Ultrafast Laser Pulses in Dielectric Solids. Micromachines 2024, 15, 196. [Google Scholar] [CrossRef]
  14. Miyamoto, I.; Horn, A.; Gottmann, J.; Wortmann, D.; Yoshino, F. Fusion Welding of Glass Using Femtosecond Laser Pulses with High-repetition Rates. Journal of Laser Micro Nanoengineering 2007, 2, 57–63. [Google Scholar] [CrossRef]
  15. Beresna, M.; Gertus, T.; Tomašiūnas, R.; Misawa, H.; Juodkazis, S. Three-Dimensional Modeling of the Heat-Affected Zone in Laser Machining Applications. Laser Chemistry 2008, 2008, 976205. [Google Scholar] [CrossRef]
  16. Miyamoto, I.; Horn, A.; Gottmann, J. Local Melting of Glass Material and Its Application to Direct Fusion Welding by Ps-laser Pulses. Journal of Laser Micro Nanoengineering 2007, 2, 7–14. [Google Scholar] [CrossRef]
  17. Muzi, E.; Cavillon, M.; Lancry, M.; Brisset, F.; Que, R.; Pugliese, D.; Janner, D.; Poumellec, B. Towards a Rationalization of Ultrafast Laser-Induced Crystallization in Lithium Niobium Borosilicate Glasses: The Key Role of The Scanning Speed. Crystals 2021, 11. [Google Scholar] [CrossRef]
  18. Bricchi, E.; Klappauf, B.G.; Kazansky, P.G. Form birefringence and negative index change created by femtosecond direct writing in transparent materials. Optics Letters 2004, 29, 119–121. [Google Scholar] [CrossRef] [PubMed]
  19. Cao, J. 2017 Creation and orientation of nano-crystals by femtosecond laser light for controlling optical non-linear response in silica-based glasses Création et orientation de nano-cristaux par irradiation laser femtoseconde pour le contrôle de l’orientation des propriétés optiques non-linéaires dans des verres à base de silice. Université Paris Saclay (COmUE)).
  20. Cao, J.; Matthieu, L.; François, B.; Bertrand, P. Orientable Nonlinear Optical Crystals and Periodic Nanostructure by Femtosecond Laser Irradiation 激光与光电子学进展 Laser & Optoelectronics Progress 2022, 59, 1516001.
  21. Marburger, J.H. Self-focusing: Theory. Progress in Quantum Electronics 1975, 4 Pt 1, 35–110. [Google Scholar] [CrossRef]
  22. Ashcom, J.B.; Gattass, R.R.; Schaffer, C.B.; Mazur, E. Numerical aperture dependence of damage and supercontinuum generation from femtosecond laser pulses in bulk fused silica. J. Opt. Soc. Am. B 2006, 23, 2317–2322. [Google Scholar] [CrossRef]
  23. Schaffer, C.B.; Brodeur, A.; Mazur, E. Laser-induced breakdown and damage in bulk transparent materials induced by tightly focused femtosecond laser pulses. Measurement Science and Technology 2001, 12, 1784. [Google Scholar] [CrossRef]
  24. Kato, T.; Suetsugu, Y.; Nishimura, M. Estimation of non-linear refractive index in various silica-based glasses for optical fibers. Optics Letters 1995, 20, 2279–2281. [Google Scholar] [CrossRef] [PubMed]
  25. Le Boiteux, S.; Segonds, P.; Canioni, L.; Sarger, L.; Cardinal, T.; Duchesne, C.; Fargin, E.; Le Flem, G. Nonlinear optical properties for TiO2 containing phosphate, borophosphate, and silicate glasses. Journal of Applied Physics 1997, 81, 1481–1487. [Google Scholar] [CrossRef]
  26. Couairon, A.; Mysyrowicz, A. Femtosecond filamentation in transparent media. Physics Reports 2007, 441, 47–189. [Google Scholar] [CrossRef]
  27. Lei, Y. 2023 Laser Modification of Materials at Micro- and Nanoscale for Photonics and Information Technology. In: ORC: Southampton).
  28. Ashcom, J.B.; Gatlass, R.R.; CSchaffer, C.B.; Mazur, E. Numerical aperture dependence of damage and supercontinuum generation from femtosecond laser pulses in bulk fused silica. Journal of Optical Society of America B 2006, 23, 2317–2322. [Google Scholar] [CrossRef]
  29. Beresna, M.; Kazansky, P.G.; Svirko, Y.; Barkauskas, M.; Danielius, R. High average power second harmonic generation in air. Applied Physics Letters 2009, 95, 121502. [Google Scholar] [CrossRef]
  30. Xie, Q.; Cavillon, M.; Pugliese, D.; Janner, D.; Poumellec, B.; Lancry, M. On the Formation of Nanogratings in Commercial Oxide Glasses by Femtosecond Laser Direct Writing. Nanomaterials 2022, 12. [Google Scholar] [CrossRef] [PubMed]
  31. Gamaly, E.G.; Juodkazis, S.; Nishimura, K.; Misawa, H.; Luther-Davies, B.; Hallo, L.; Nicolai, P.; Tikhonchuk, V.T. Laser-matter interaction in the bulk of a transparent solid: Confined microexplosion and void formation. Physical Review B 2006, 73, 214101. [Google Scholar] [CrossRef]
  32. Zaiter, R.; Lancry, M.; Fargues, A.; Adamietz, F.; Dussauze, M.; Rodriguez, V.; Poumellec, B.; Cardinal, T. Optical and structural characterization of femtosecond laser written micro-structures in germanate glass. Scientific Reports 2023, 13, 11050. [Google Scholar] [CrossRef] [PubMed]
  33. Wang, H.; Lei, Y.; Shayeganrad, G.; Svirko, Y.; Kazansky, P.G. Increasing Efficiency of Ultrafast Laser Writing Via Nonlocality of Light-Matter Interaction. Laser & Photonics Reviews 2024, 2301143. [Google Scholar]
  34. Garcia-Lechuga, M.; Haahr-Lillevang, L.; Siegel, J.; Balling, P.; Guizard, S.; Solis, J. Simultaneous time-space resolved reflectivity and interferometric measurements of dielectrics excited with femtosecond laser pulses. Physical Review B 2017, 95, 214114. [Google Scholar] [CrossRef]
  35. Couairon, A.; Sudrie, L.; Franco, M.; Prade, B.; Mysyrowicz, A. Filamentation and damage in fused silica induced by tightly focused femtosecond laser pulses. Physical Review B 2005, 71, 125435. [Google Scholar] [CrossRef]
  36. Wu, A.Q.; Chowdhury, I.H.; Xu, X. Femtosecond laser absorption in fused silica: Numerical and experimental investigation. Physical Review B 2005, 72, 085128. [Google Scholar] [CrossRef]
  37. Zhang, S.; Menoni, C.; Gruzdev, V.; Chowdhury, E. 2022 Ultrafast Laser Material Damage Simulation&mdash;A New Look at an Old Problem. In: Nanomaterials,.
  38. Wang, H.; Lei, Y.; Wang, L.; Sakakura, M.; Yu, Y.; Shayeganrad, G.; Kazansky, P.G. 100-Layer Error-Free 5D Optical Data Storage by Ultrafast Laser Nanostructuring in Glass. Laser & Photonics Reviews, 2022; 16, 2100563. [Google Scholar]
  39. Lei, Y.; Shayeganrad, G.; Wang, H.; Sakakura, M.; Yu, Y.; Wang, L.; Kliukin, D.; Skuja, L.; Svirko, Y.; Kazansky, P.G. Efficient ultrafast laser writing with elliptical polarization. Light: Science & Applications 2023, 12, 74. [Google Scholar]
  40. Ari, J.; Cavillon, M.; Lancry, M.; Poumellec, B. Polarization-dependent orientation of LiNbO3:Eu3+ nanocrystals using ultrashort laser pulses in borosilicate glasses. Advanced Optical Technologies 2023, 12. [Google Scholar] [CrossRef]
  41. Company I C C 1927 Chemical Engineering Catalog vol Twelfth Annual Edition (New York, USA: The Chemical Catalog Company, Inc.
  42. Kingery, W.D. Thermal Conductivity: XII, Temperature Dependence of Conductivity for Single-Phase Ceramics. Journal of the American Ceramic Society 1955, 38, 251–255. [Google Scholar] [CrossRef]
  43. Morgan, R.A.; Kang, K.I.; Hsu, C.C.; Koliopoulos, C.L.; Peyghambarian, N. Measurement of the thermal diffusivity of nonlinear anisotropic crystals using optical interferometry. Appl. Opt. 1987, 26, 5266–5271. [Google Scholar] [CrossRef] [PubMed]
  44. Sigaev, V.N.; Golubev, N.V.; Stefanovich, S.Y.; Komatsu, T.; Benino, Y.; Pernice, P.; Aronne, A.; Fanelli, E.; Champagnon, B.; Califano, V.; Vouagner, D.; Konstantinova, T.E.; Glazunova, V.A. Second-order optical non-linearity initiated in Li2O–Nb2O5–SiO2 and Li2O–ZnO–Nb2O5–SiO2 glasses by formation of polar and centrosymmetric nanostructures. Journal of Non-Crystalline Solids 2008, 354, 873–881. [Google Scholar] [CrossRef]
  45. Shimada, M.; Honma, T.; Komatsu, T. Laser patterning of oriented LiNbO3 crystal particle arrays in NiO-doped lithium niobium silicate glasses. International Journal of Applied Glass Science 2018, 9, 518–529. [Google Scholar] [CrossRef]
Preprints 104662 g001
Figure 1. Crystallization domain in a Time – Temperature – Transformation (TTT) diagram (in Yellow). Its boundary is black. The glass and melting T is deep blue. T1 is an example of isothermal treatment that leads to the incubation time t1 for beginning to crystallize.
Figure 1. Crystallization domain in a Time – Temperature – Transformation (TTT) diagram (in Yellow). Its boundary is black. The glass and melting T is deep blue. T1 is an example of isothermal treatment that leads to the incubation time t1 for beginning to crystallize.
Preprints 104662 g001
Preprints 104662 g002
Figure 2. Spatial distribution of Tmin (blue dash, by Eq.A3) and Tmax (red, by Eq.A1) in the normalized form (see text) and as a function of the reduced radius rw when (a) R τ = 0.1 , (b)   R τ = 1 and (c)   R τ = 10
Figure 2. Spatial distribution of Tmin (blue dash, by Eq.A3) and Tmax (red, by Eq.A1) in the normalized form (see text) and as a function of the reduced radius rw when (a) R τ = 0.1 , (b)   R τ = 1 and (c)   R τ = 10
Preprints 104662 g002
Preprints 104662 g003
Figure 3. Temperature distribution for Tmax and Tmin with respect to the normalized radial distance rw for R τ = 4 D t h R R w 2 ( c a l l e d   R t a u 1   i n   t h e   f i g u r e s   o f   t h i s   p a p e r ) = 0.13 ,  A = 0.1, w = 1.65μm, RR = 300 kHz, Ep (called Ep1 in the figures of this paper) = 54 nJ for (a) and 37 nJ for (b), Tg and Tm, shifted by room temperature, are also shown. Tmax and Tmin is calculated from eq. A1 and A3 in Appendix 4.
Figure 3. Temperature distribution for Tmax and Tmin with respect to the normalized radial distance rw for R τ = 4 D t h R R w 2 ( c a l l e d   R t a u 1   i n   t h e   f i g u r e s   o f   t h i s   p a p e r ) = 0.13 ,  A = 0.1, w = 1.65μm, RR = 300 kHz, Ep (called Ep1 in the figures of this paper) = 54 nJ for (a) and 37 nJ for (b), Tg and Tm, shifted by room temperature, are also shown. Tmax and Tmin is calculated from eq. A1 and A3 in Appendix 4.
Preprints 104662 g003
Preprints 104662 g004aPreprints 104662 g004b
Figure 4. Plot of the treatment curves in the time scale from the time when the temperature overcomes Tg a) scheme for illustrating the various normalized distances used in the calculation of the thermal treatment curve, b) treatment curve at the center β = 0 or at r = 1.2w (β = 1.2). c) same as (b) but showing the time in log scale. A = 0.1, w = 1.65 μm, Rτ = 0.13, Ep = 54 nJ. v = 100μm/s. The thermal treatment of the point at coordinates 0,0,0 begins when the temperature overcomes Tg, here when the beam center is distant by 1.9w (third parameter in rwd), whereas, it begins later, at 1.5w, for a point in the periphery of the line at coordinates 0,1.2,0.
Figure 4. Plot of the treatment curves in the time scale from the time when the temperature overcomes Tg a) scheme for illustrating the various normalized distances used in the calculation of the thermal treatment curve, b) treatment curve at the center β = 0 or at r = 1.2w (β = 1.2). c) same as (b) but showing the time in log scale. A = 0.1, w = 1.65 μm, Rτ = 0.13, Ep = 54 nJ. v = 100μm/s. The thermal treatment of the point at coordinates 0,0,0 begins when the temperature overcomes Tg, here when the beam center is distant by 1.9w (third parameter in rwd), whereas, it begins later, at 1.5w, for a point in the periphery of the line at coordinates 0,1.2,0.
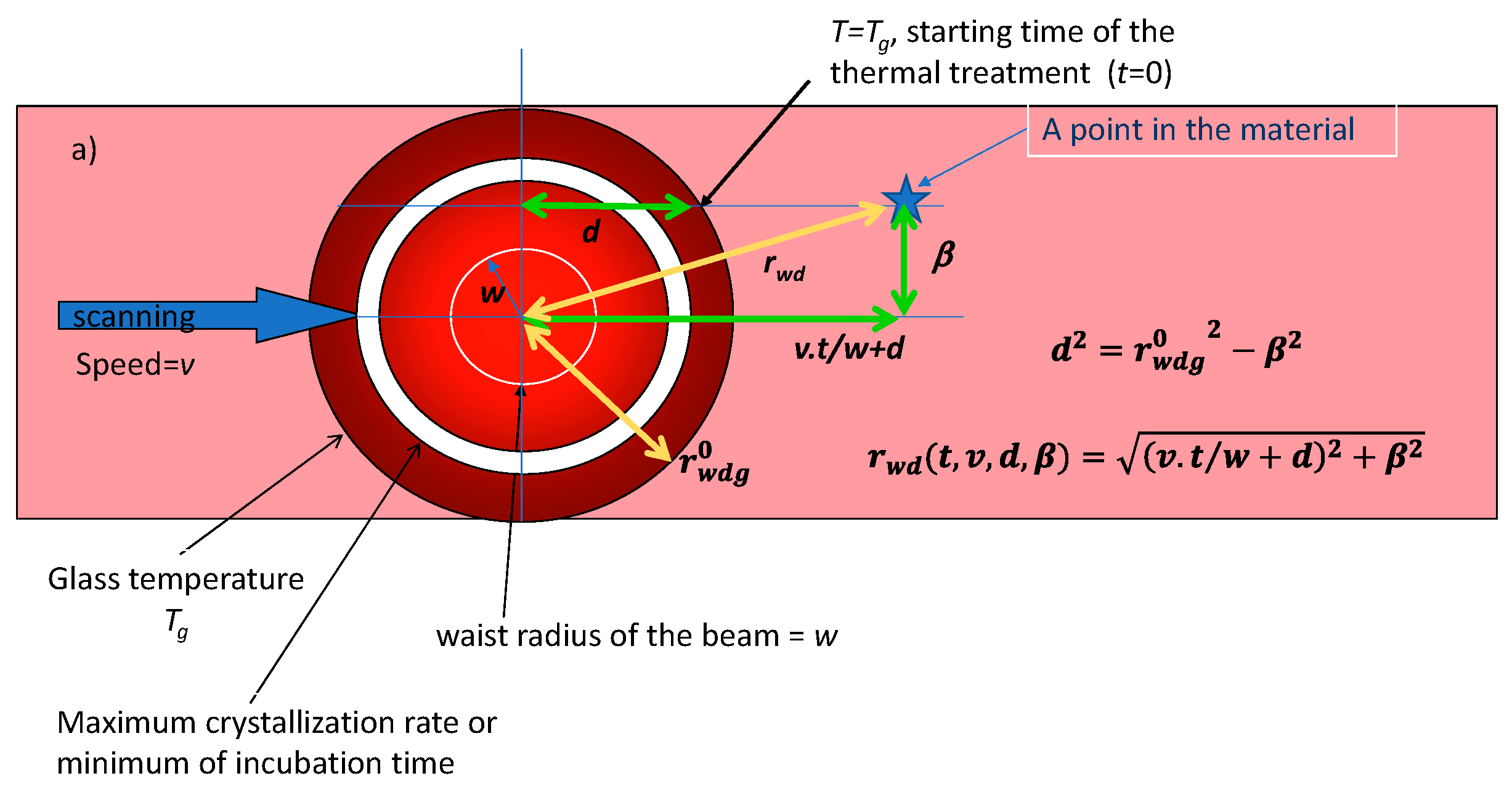
Preprints 104662 g004aPreprints 104662 g004b
Preprints 104662 g005
Figure 5. Show of position of the treatment curve versus the crystallization domain in the case of solid glass to crystal transformation (Tg<T<Tm).
Figure 5. Show of position of the treatment curve versus the crystallization domain in the case of solid glass to crystal transformation (Tg<T<Tm).
Preprints 104662 g005
Preprints 104662 g006
Figure 6. the position of the treatment curve versus the crystallization domain when a part of the trace is melting i.e. a liquid to crystal transformation. The region delimited by red one is for growth only.
Figure 6. the position of the treatment curve versus the crystallization domain when a part of the trace is melting i.e. a liquid to crystal transformation. The region delimited by red one is for growth only.
Preprints 104662 g006
Preprints 104662 g007
Figure 7. Thermal treatment curve (Tmax, Tmin, SimpulseT) for a point at the center (0,0,0) of the written line. R τ = 13 ,   A = 0.15, w = 1.65 μm, Ep = 0.5 μJ, 100 kHz, v = 10μm/s, (RR is fixed to 400 Hz just for illustrative purpose). time is in a) linear and b) log scale.
Figure 7. Thermal treatment curve (Tmax, Tmin, SimpulseT) for a point at the center (0,0,0) of the written line. R τ = 13 ,   A = 0.15, w = 1.65 μm, Ep = 0.5 μJ, 100 kHz, v = 10μm/s, (RR is fixed to 400 Hz just for illustrative purpose). time is in a) linear and b) log scale.
Preprints 104662 g007
Preprints 104662 g008
Figure 8. a) positions x1, x2 in the pulse period, between which the temperature is above Tg, b) the efficiency (definition in the text) as a function of rW, x1 red curve, x2 dashed blue curve. Same laser parameters as in Figure 7 are used.
Figure 8. a) positions x1, x2 in the pulse period, between which the temperature is above Tg, b) the efficiency (definition in the text) as a function of rW, x1 red curve, x2 dashed blue curve. Same laser parameters as in Figure 7 are used.
Preprints 104662 g008
Preprints 104662 g009
Figure 9. Values of x for the crossing points according to the distance from the beam center rw. a) : definition of the remarkable temporal locations in the period. x1 and x2 where the temperature equals the glass one, x3, x4 where it equals the melting one, b) : variation of the remarkable temporal locations according to the distance from the beam center. The part of the heat affected region where crystallization is possible is also indicated. This computation has been achieved with Ep = 5μJ, w = 1.65 μm, A=0.2, RR = 60kHz, R τ = 22 .
Figure 9. Values of x for the crossing points according to the distance from the beam center rw. a) : definition of the remarkable temporal locations in the period. x1 and x2 where the temperature equals the glass one, x3, x4 where it equals the melting one, b) : variation of the remarkable temporal locations according to the distance from the beam center. The part of the heat affected region where crystallization is possible is also indicated. This computation has been achieved with Ep = 5μJ, w = 1.65 μm, A=0.2, RR = 60kHz, R τ = 22 .
Preprints 104662 g009
Preprints 104662 g010
Figure 10. Temperature evolution over time when pulses has overcome Tm corresponding to a liquid to solid transition. The last pulse contribution that does not overcome Tm defines the time origin. Computation is made with parameters of Figure 9 with a scanning speed of 15μm/s.
Figure 10. Temperature evolution over time when pulses has overcome Tm corresponding to a liquid to solid transition. The last pulse contribution that does not overcome Tm defines the time origin. Computation is made with parameters of Figure 9 with a scanning speed of 15μm/s.
Preprints 104662 g010
Preprints 104662 g011
Figure 11. temperature simulation in LNS glass for Ep = 0.7μJ, A = 0.09, w = 1.65 μm, Rτ= 4.4, scanning speed=5 μm/s for a, b and c., a) : Tmax and Tmin distribution, b) : useful part of the T oscillations c) : corresponding treatment curve for v = 5 μm/s, d) the same with v = 125 μm/s.
Figure 11. temperature simulation in LNS glass for Ep = 0.7μJ, A = 0.09, w = 1.65 μm, Rτ= 4.4, scanning speed=5 μm/s for a, b and c., a) : Tmax and Tmin distribution, b) : useful part of the T oscillations c) : corresponding treatment curve for v = 5 μm/s, d) the same with v = 125 μm/s.
Preprints 104662 g011
Preprints 104662 g012
Figure 12. Temperature computation in LNS glass for Ep=1.3 µJ, w=2.6 µm, A=0.15, Rτ=1.8. Laser parameters : λ=1030nm, τp=300fs, RR=300kHz, NA=0.6,v=5 µm/s. a) T distribution, b) efficiency c) simulation on time.
Figure 12. Temperature computation in LNS glass for Ep=1.3 µJ, w=2.6 µm, A=0.15, Rτ=1.8. Laser parameters : λ=1030nm, τp=300fs, RR=300kHz, NA=0.6,v=5 µm/s. a) T distribution, b) efficiency c) simulation on time.
Preprints 104662 g012
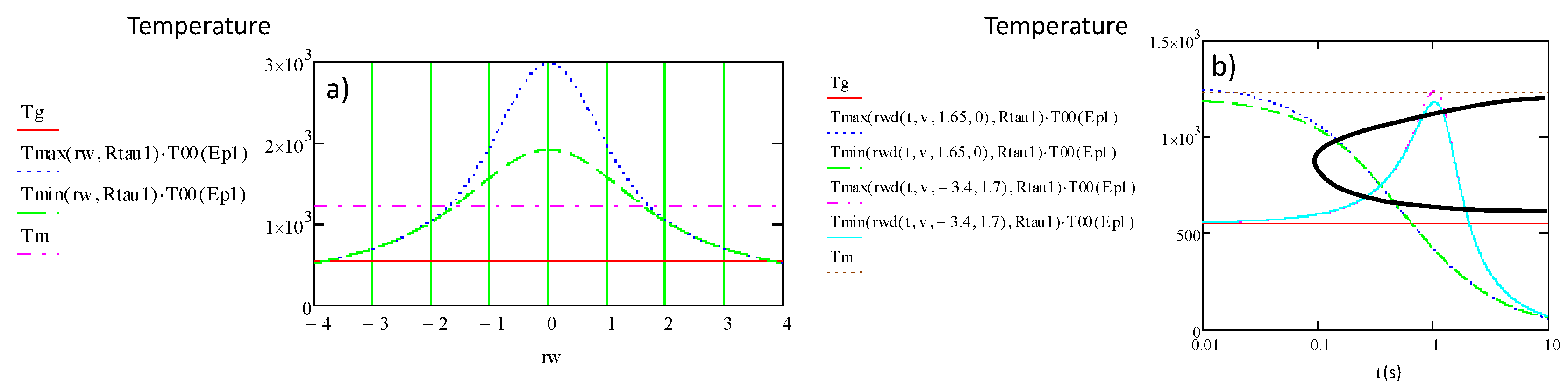
Preprints 104662 g013
Figure 13. Temperature computation in LNS glass for Ep=4.2 µJ, w=4.5 µm, A=0.32, Rτ=0.9 Laser parameters : λ=1030nm, τp=300fs, RR=200kHz, NA=0.6, v=5 µm/s. a) temperature distribution, b) simulation on time for two positions y=0 (center of the line) and β=1.7 (periphery).
Figure 13. Temperature computation in LNS glass for Ep=4.2 µJ, w=4.5 µm, A=0.32, Rτ=0.9 Laser parameters : λ=1030nm, τp=300fs, RR=200kHz, NA=0.6, v=5 µm/s. a) temperature distribution, b) simulation on time for two positions y=0 (center of the line) and β=1.7 (periphery).
Preprints 104662 g013
Preprints 104662 g014
Figure 14. The half width variation during the transitory stage (Np<Nss). 3.8w is the maximum width (w=4.5 µm) for Np>Ns (at steady state), for Ep=4.2 µJ, R τ =0.9.
Figure 14. The half width variation during the transitory stage (Np<Nss). 3.8w is the maximum width (w=4.5 µm) for Np>Ns (at steady state), for Ep=4.2 µJ, R τ =0.9.
Preprints 104662 g014
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated