Prerpints.org logo
Preprint
Review

Features of adaptive phase correction of optical wave distortions under conditions of intensity fluctuations

Altmetrics

Downloads

67

Views

16

Comments

0

A peer-reviewed article of this preprint also exists.

supplementary.docx (12.95KB )

This version is not peer-reviewed

Submitted:

09 April 2024

Posted:

25 April 2024

You are already at the latest version

Alerts
Abstract
An analysis of the features of measurements and correction of phase distortions in optical waves propagating in the atmosphere at various levels of turbulence was performed. It is shown that with increasing intensity fluctuations, the limiting capabilities of phase correction decrease, and the phase of an optical wave that has passed through a turbulence layer consists of two components: potential and vortex. It was found that even in the region of weak fluctuations there is an overlap of spectral filtering functions for intensity and phase fluctuations. Areas of turbulence inhomogeneities have been identified that will have mutual influence and negatively affect the operation of the phase meter. It is noted that correlation functions, both phase and intensity, are less susceptible to this compared to structural functions. The results of experimental studies of the reconstruction of the wavefront of laser radiation distorted by atmospheric turbulence using a Shack-Hartmann wavefront sensor during vignetting and central screening of the entrance pupil of the optical system are presented. Studies have been carried out on the propagation of laser radiation along a horizontal atmospheric path for various levels of turbulence. The results are analyzed in terms of Zernike polynomials.
Keywords: 
Subject: Physical Sciences  -   Optics and Photonics

1. Introduction

The phase problem [1] in optics has always been and remains central. At the same time, the tasks of measuring the phase and its correction pose the problem of separating the amplitude and phase fluctuations of optical waves passing through a randomly inhomogeneous medium, such as atmospheric turbulence. Such a separation can be quite effective only in the region of weak intensity fluctuations [2,3], and then problems begin. This is due to the fact that the phase of the wave is not a measured, but a calculated characteristic.
Due to the widespread use of optical systems, quite a lot of publications have appeared [4,5,6,7,8,9,10,11] devoted to the operation of systems on long atmospheric paths. In particular, statements have appeared [12] about the impossibility of obtaining good correction when using adaptive optics (AO) systems. However, when analyzing the operation of AO systems under strong intensity fluctuations, the reason for this, as a rule, was not given. Most often, it was suggested that the reason for poor correction was the inability of the wavefront sensor (WFS) of the AO system to operate effectively. It was found that as the level of amplitude fluctuations increases, the amplitudes of the lower modes of decomposition of phase fluctuations—tilts, defocusing, and astigmatism—are first subject to distortion. And the behavior of these modes begins to differ greatly from the classical one, corresponding to the regime of weak fluctuations.
To analyze the influence of the manifestations of amplitude fluctuations on the operation of the WFS, studies were carried out [8,12,13,14,15] on the behavior of the vortex components of phase fluctuations, reconstructed from measurement data under various operating modes. In this regard, it should be considered quite important to analyze the limiting capabilities of the AO phase system, based on the parameters of the components included in it. As a rule, on long horizontal atmospheric paths (for the visible wavelength range), already with a length of the order of 1 km, the Fried parameter [16,17] becomes less than the radius of the first Fresnel zone. And it is known that when an optical wave propagates, its diffraction properties are determined precisely by the minimum size of the physical parameter that determines the properties of the radiation beam. And therefore, this size of diffraction in the region of strong fluctuations [18,19,20] becomes the Fried parameter. As a result, amplitude fluctuations begin to appear in the wave [16]. Under such conditions, AO phase systems lose their efficiency and it is no longer possible to achieve any improvement using phase correction. The dependence of phase systems on the level of intensity fluctuations is manifested primarily in changes in the brightness (flickering) of images in each of the subapertures of the Hartmann matrix. In this case, phase measurements become incorrect [21,22,23,24].
To understand when this begins from the point of view of the manifestation of diffraction, the work will perform calculations of spectral filter functions for the intensity and phase fluctuations of an optical wave propagating in a turbulent medium. Analysis of families of graphs of these functions shows that the region of spatial frequencies of inhomogeneities of the refractive index of the atmosphere, affecting both phase and intensities fluctuations amplitude fluctuations, depends on the distance, wavelength and on the initial parameters of the optical radiation beam or the size of the receiving aperture.

2. The Influence of Amplitude Fluctuations on Phase Measurements during the Propagation of Optical Waves in Random Media

The question of the influence of amplitude (or intensity) fluctuations on phase measurements under conditions of propagation of optical waves in random media is extremely important and is associated with several features of the use of phase systems. It is known that phase distortions, primarily acquired when passing through an optically inhomogeneous medium, as the wave propagates, are transformed into modulation of the spatial distribution of intensity [19,22,23]. With sufficiently deep amplitude modulation of the field, points with zero intensity appear in it [19]. If we describe any optical wave in terms of the complex amplitude U, then such points are formed at the intersection (or touching) of the lines, where its real and imaginary parts are equal to zero. A similar analysis of the influence of amplitude on the phase in such a formulation is possible in principle, but this is too theoretical a solution and it is unlikely to help in analyzing such an influence on an optical experiment.
At the same time, it showed that during phase measurements and adaptive phase correction, the appearance of numerous wavefront dislocations completely disrupts the continuity of the two-dimensional phase distribution. As a result, the error of any wavefront approximation (for example, when describing phase distortions by polynomials or representing them by an adaptive mirror) will increase. Currently existing algorithms for constructing an aberration map for a wave passing through an inhomogeneous medium, used for most wavefront sensors, produce a continuous function of transverse coordinates at the output. This means that such algorithms filter the “vortex” part of the phase distribution. Another aspect of this is that in some cases, such a phase obtained from measurements can [19,25] even degrade the quality of the generated image or radiation beam.

3. Numerical Experiments on Adaptive Correction of Turbulent Distortions

As a rule, when analyzing the effectiveness of using measuring and correcting phase systems, it is assumed that there are no intensity fluctuations. It is interesting to consider another limiting case - the case of strong intensity fluctuations, assuming that the measuring or adaptive optics (AO) system has unlimited spatiotemporal resolution with respect to phase distortions.
To do this, let us analyze the results of numerical experiments performed in [20,23,26], which analyze the influence of intensity fluctuations and the wavefront dislocations generated by them on the efficiency of correction of turbulent distortions. At the same time, the following two aspects are of both practical and scientific interest. The first is how significantly the loss of amplitude information affects the effectiveness of phase correction. The second is how much the loss of information contained in the vortex part of the phase measurements reduces the efficiency of adaptation.
The numerical experiments we analyzed were carried out in [20] for two adaptive phase correction schemes. The first is a distortion compensation circuit, in which measurements and correction of phase distortions are carried out in the observation plane. Such a phase correction scheme for the received wave can be called “on-receive” correction. The second is phase correction for the optical signal transmitted through a random medium, which works using the phase conjugation (PC) method, using distortions measured using a reference source. For simplicity of analysis, we will limit ourselves to considering the propagation of a plane optical wave in a turbulent atmosphere.
In [20], for these two schemes, the use of two options for measuring the corrected phase was considered. The first option used an ideal adaptive system that instantly and accurately reproduces the phase of the reference wave over the entire cross-sectional plane, including special points of the wave front. In the second option, only the component corresponding to the potential part of the wavefront field distribution is corrected [19,22]. We will further call such a correction the correction of the “potential” (or “irrotational”) phase.
As a result, in numerical experiments [20], four schemes for applying phase correction were implemented:
1) an ideal correction system “on reception” of the signal,
2) a correction system “on reception” of only the “potential” part of phase aberrations,
3) an ideal PC system operating “for transmission” using a reference source,
4) an adaptive phase correction system that implements the PC algorithm and works “for transmission”, which corrects only the “potential” part of the phase aberrations.
It was assumed that turbulence corresponds to the Kolmogorov-Obukhov model. Then, according to the theory of similarity [16], the problem of describing the propagation of a plane wave in a turbulent atmosphere is characterized by only two scales. Moreover, the coherence radius r0 is chosen as the transverse scale of the problem [16], and the wave diffraction length at the coherence radius LT = kr02 is chosen as the longitudinal scale, here r0 is the Fried radius (or parameter) [16,17], and k is the wave number of radiation.
As a result, it can be shown that when describing the problem of propagation of a plane optical wave, one can, for example, use two dimensionless parameters: the atmospheric path length L/LT, normalized to the wave diffraction length at the coherence radius, and, normalized to the coherence scale, the aperture diameter D/r0. According to Rytov’s theory [16], the level of intensity fluctuations in an optical wave can be characterized through the so-called scintillation index for a plane wave βint2. For a power-law turbulence spectrum [16], this parameter turns out to be uniquely related to the normalized optical path length:
β int 2 = 2 , 9 ( L / L T ) 5 / 6 .
Therefore, this scintillation index βint2 can be used as a problem parameter along with the ratio of the path length to the longitudinal scale L/LT.
The main results of numerical experiments carried out in [20,22] are presented in Figure 1 and Figure 2. In this case, the normalized aperture diameter had several values: D/r0 = 10, 20, 30. As a result, each of the system operation schemes corresponds to a family of three curves.
Figure 1 shows the dependence of the Strehl parameter SR for a circuit operating “to receive” radiation on the flicker index in the received optical wave. From Figure 1 it is clear that with phase correction of only the “irrotational” part of the phase (scheme 2), the correction efficiency decreases quite significantly with increasing scintillation index (this can be seen from three curves corresponding to values D/r0 = 10, 20, 30). A twofold reduction in the value of the SR ratio is achieved already at values of the order of 1,5. A further increase in intensity fluctuations leads to the fact that the Strehl parameter SR tends to the uncorrected value. A decrease in the correction efficiency by an order of magnitude occurs at βint2 = 3, which approximately corresponds to the fact that the path length L turns out to be approximately equal to LT.
Similar results with phase correction are obtained for phase conjugation (according to scheme 4). This is shown in Figure 2. As can be seen from Figure 2, the use of “irrotational” phase correction significantly loses its effectiveness when the path length reaches the diffraction length at the coherence radius LT. It should be emphasized that, as shown in [20], the efficiency of correction even with ideal phase conjugation depends on the magnitude of intensity fluctuations. However, we note that, as shown in [2], this dependence is not as strong as might be expected. This is due to the fact that in the phase conjugation system the correction is performed at the entrance to the turbulent atmosphere; in fact, there is a preliminary distortion of the original optical wave. Moreover, this preliminary distortion is calculated based on phase measurements in a reference source, which propagates towards the original wave.
For cases where D/r0 = 10, 20, 30, already at a parameter value of βint2 = 1, the value of the Strehl parameter SR decreases to a value of 0.8 and practically weakly depends on the normalized aperture diameter.

4. Comparison of Calculations with the American Experiment

Unfortunately, it turned out that in order to compare the data of these calculations (Figure 1 and Figure 2), there is a very limited number of experimental data with which the presented results of the numerical analysis can be compared. However, one similar experiment (Figure 3) was carried out at the Lincoln Laboratory in the USA on a 5.5 km distance path [9,25]. The adaptive optical system used Hartmann wavefront sensor and a deformable mirror. For correction of distortions a phase conjugation algorithm was used. Phase correction was carried out in a focused laser beam using measurements in the reference beam. The corrected (initial) and reference laser beams had different wavelengths: 633 and 514 nm, respectively. In fact, the focused laser beam received “predistortions” in the original plane, which ensured its focusing even with strong phase distortions. Figure 3 shows the results of a comparison of these measurements (black triangles) and the results of calculations according to scheme 4 [20,22] (solid curve). In this case, the value of the Strehl parameter for the laser beam in the focusing plane is plotted along the vertical axis of the graph, and the calculated dispersion of fluctuations of the logarithm of the amplitude for the reference spherical wave is plotted along the horizontal axis. The vertical bars on the measurement data show the scatter of experimental data in each experiment.
It should be noted that according to data from the Lincoln Laboratory (Figure 3), there is a fairly good agreement between experiments and calculation results [20,22]. This suggests that the use of an algorithm for reconstructing the wavefront of the reference laser beam, which actually filters the “vortex” phase, is a decisive factor causing a decrease in the correction efficiency. This, in turn, indicates the importance of taking into account amplitude fluctuations in phase measurements carried out along long atmospheric paths. Let us recall that the American experiment took place on a 5.5 km long highway.

5. Operation of the Phase Correction System under Conditions of Weak Fluctuations

It should be noted that it has been intuitively shown many times that the phase correction system is capable of giving a positive result only when certain conditions are met in the atmosphere. One of them is the condition that on the measured path, which includes a random environment, the isoplanatism angle must be greater than the resolution of the system [22], i.e.,
r 0 / L > λ / r 0 .
As it turns out, this condition corresponds to the manifestation of “weak fluctuations” of intensity on the path
β int 2 = ( k 7 / 6 С n 2 L 11 / 6 ) < 1.
In this case, the wavefront sensor provides correct phase data and the influence of amplitude fluctuations in the system can be neglected. At the same time, AO phase systems are highly efficient.
On vertical paths this condition is easily realized, but on horizontal paths the situation is reversed, i.e., the Fried coherence radius becomes smaller than the first Fresnel zone [16]:
r < 0 λ L .
This means that amplitude fluctuations begin to appear, and at the same time the magnitude of the dispersion of intensity fluctuations, calculated using Rytov’s formula,
β int 2 = ( k 7 / 6 С n 2 L 11 / 6 ) ,
become more than 1.
Under these conditions, AO phase systems lose their efficiency and it is no longer possible to achieve any improvement using phase correction [24,26]. To confirm this, we note that in experiments with the Shack-Hartmann wavefront sensor it was discovered [24] that there is a dependence of phase systems on the level of intensity fluctuations, which manifests itself, first of all, in a change in the brightness (scintillation) of focal spots in the Hartmann matrix. In this case, phase measurements become incorrect, and errors appear in the assessment of certain mode components. And at the same time, the dependence of the phase measurement data (or phase correction) on the radius of the first Fresnel zone, calculated for the path on which these phase measurements will be performed, necessarily appears.

6. Comparison of Phase Adaptive Correction in Areas of Weak and Strong Intensity Fluctuations

Based on what is presented in sections 3 and 4, the following conclusions can be drawn:
1. The efficiency of phase correction of turbulent distortions decreases approximately by half as the normalized dispersion of intensity fluctuations (5) increases from zero to unity. In this range of intensity fluctuation dispersion values, the correction efficiency is practically independent of the relationship between the aperture diameter and the coherence radius. With a further increase in intensity fluctuations, the dependence of the correction efficiency on the aperture diameter begins to appear. An increase in the dispersion of intensity fluctuations β int 2 to 3 leads to a drop in the efficiency of correction by an order of magnitude or more, and the Strehl parameter SR tends to the value obtained in the system without correction.
2. Since level β int 2 = 1 approximately corresponds to the limit of applicability of the smooth perturbation method (SPM) [16], we can assume that the applicability of calculations in the SPM approximation is possible only in the region where there are no phase dislocations.
3. The decrease in the efficiency of adaptive correction of the “irrotational” phase with increasing dispersion of intensity fluctuations occurs approximately equally both in the “receive” phase compensation scheme and in the “transmit” phase conjugation scheme. The differences between a plane wave and a beam are also insignificant, as follows from a comparison with the Lincoln Laboratory experiment [25].
It is interesting to note that, as shown in [22,24], even with large intensity fluctuations, the use of adaptive phase correction provides a certain and quite significant gain compared to the case without correction. Table 1 shows a comparison of the ratio of the corrected Strehl parameter - SRс to the uncorrected value of this parameter – SR0 for a compensation scheme for only the “potential” phase for the case when the dispersion of intensity fluctuations for a plane wave β int 2 = 3. Authors should discuss the results and how they can be interpreted from the perspective of previous studies and of the working hypotheses. The findings and their implications should be discussed in the broadest context possible. Future research directions may also be highlighted.
From Table 1 it can be seen that for D/r0 values from 10 to 30, the corrected value of the Strehl parameter SRc is approximately four times greater than the uncorrected SR0.

7. Study of the Influence of Amplitude Fluctuations on Phase Measurements

Another approach to analyzing the relationship between phase and amplitude (intensities) fluctuations is to consider this issue based on an analysis of the formulas of the smooth perturbation method [16], which describes the propagation of optical waves in a turbulent atmosphere. In [27], calculations were made of the behavior of spectral filtering functions for the correlation and structure functions of phase and amplitude [16] for various parameters of optical radiation beams and generated images. Calculations of spectral filtering functions were performed for the intensities and phase of an optical wave propagating in a turbulent medium [16,27]. In these numerical calculations, the Kolmogorov model of the turbulence spectrum was used [16].
In this case, spectral filtering functions were calculated [27] corresponding to the correlation functions of intensity and phase fluctuations, which are given by the following formulas:
f χ , S ( ρ , κ ) 1 =   J 0 ( κ ρ ) [ 1 ± cos ( κ 2 L / k ) ]
Spectral filter functions were also analyzed for the intensity and phase structure functions, which are expressed as:
f χ , S ( ρ , κ ) 2 =   [ 1 − J 0 ( κ ρ ) ] [ 1 ± cos ( κ 2 L / k ) ]
Here J 0 (   κ ρ ) is the zero-order Bessel functions of the first kind, L is the propagation distance of the optical wave, κ is the wave number characterizing the size of turbulent inhomogeneities, and ρ is the modulus of the two-dimensional vector of the observation point.
Further, when carrying out calculations in [27], a change of variables was made, then we obtain
κ ρ = t 1 / 2 Ω 1 / 2
where Ω = k ρ 2 / L is the so-called wave number of the aperture, which now becomes a parameter of the problem.
As a result, the formulas for calculating spectral filter functions are as follows:
f χ ,   S ( Ω , ​   t ) 1 =   J 0 ( t 1 / 2 Ω 1 / 2 ) [ 1 ± cos ( t ) ] ,
f χ ,   S ( Ω , ​   t ) 2 =   [ 1 − J 0 ( t 1 / 2 Ω 1 / 2 ) ] [ 1 ± cos ( t ) ] .
Formula (9) is given for calculating spectral filter functions for the correlation of intensity fluctuations (plus sign in square brackets) and phase S (minus sign in square brackets). Formula (10) is for calculating spectral filter functions for the structure function of intensity (plus sign in square brackets) and phase (minus sign in square brackets).
Calculations were performed using formulas (9) and (10) in [27], and here below they are presented in the form of graphs of the dependence on the argument for a set of values of the aperture wave number. In this case, the variable changes from 0 to in increments. The parameter Ω was set equal to 100, 50, 10, 5, 2, 1. Thus, narrow, diffraction and wide wave optical beams were considered in the calculations.
First of all, let’s consider a family of graphs (Figure 4) for the correlation functions of amplitude and phase, calculated using formula (9). In Figure 4, the curves drawn with thin lines correspond to the curves describing the behavior of functions corresponding to intensity fluctuations, and thicker ones correspond to phase ones. In the six fragments of Figure 4 in the first column the values of the parameter Ω are given, respectively equal to 100, 50, 10, 5, 2, 1.
Six fragments of Figure 5 show, calculated in a similar way, graphs of spectral filtering functions for the structure functions of amplitude and phase (10). The structure of Figure 5 corresponds to the structure of Figure 4.
Analysis of families of graphs (Figure 4 and Figure 5) of spectral filtering functions of intensity and phase shows that the region of spatial frequencies of inhomogeneities of the refractive index of the atmosphere, affecting both phase fluctuations and intensity fluctuations, depends on the distance, radiation wavelength and on the initial parameters optical radiation beam or the size of the receiving aperture.
The areas of overlap of the spectral filter functions for intensity and phase fluctuations indicate areas of inhomogeneities that will mutually influence and negatively affect the operation of phase meters. It should be noted that correlation functions, both phase and intensity, are less susceptible to this compared to structural functions. This is due to the fact that the structure functions already represent differences in fluctuations at displaced points. This to some extent makes the differences in phase fluctuations at spaced points similar to intensity fluctuations.

8. Phase Measurements in the Region of Strong Fluctuations

In addition to numerical calculations, in recent years, full-scale experiments [24,26] have been carried out with mock-ups of AO systems on long vertical and horizontal atmospheric paths. Measurements made using WFS in the surface layer of the atmosphere showed that, in addition to seasonal and diurnal variations, there is a rapid [24] variability of turbulence intensity, which leads to variability in its integral value even on long horizontal paths. In particular, measurements of the mode components of phase distortions [24] showed that intensity fluctuations lead to distortion, including the appearance of parasitic modulation of lower modes of phase distortions. In turn, this leads to loss of efficiency of phase correction.
It is known that the Shack-Hartmann wavefront sensor determines phase fluctuations by changing the positions of the centers of gravity of the system of focal spots. Under conditions of weak intensity fluctuations, these displacements of focal spots in the sensor are measured with high accuracy [28,29,30]. However, when intensity fluctuations appear in the optical field, the pattern of focal spots changes significantly: compare the left and right fragments in Figure 6. Intensity fluctuations lead to fluctuations in the illumination of individual spots, up to their complete disappearance. This leads to a loss of information content of such measurements [24,31]. In this way, intensity fluctuations directly affect the phase fluctuation measurements.
To further analyze the situation, an analysis [24] was performed on the accuracy of estimating the positions of the centers of gravity of focal spots in the wavefront sensor patterns using different illuminance thresholds. It was believed that the sensor could correctly measure the position of the focal spot, as long as the illumination level of the spots exceeded their background value, due to the presence of interference in the signals. Figure 7 and Figure 8 show the illumination distributions for two different frames of focal spots along the aperture of the wavefront sensor at different illumination values. Of course, frame number 306 cannot be processed from the point of view of obtaining correct information about the phase distribution, since a significant number of focal spot maxima are practically absent in it.
The presence in the recording of frames with significant attenuation of illumination during WFS operation require special processing. For example, from the point of view of the development of AO systems, it seems important to us to search for possibilities for the operation of an AO system with “strong” amplitude manifestations. One of the methods to combat [24] the influence of flicker can be the automatic rejection of individual subapertures in the sensor image that will not be used to restore the phase. In this case, in the process of calculating phase fluctuations, only “good” subapertures will be used, where the illumination exceeds the threshold.
Figure 9 shows a sequence of frames in the implementation of an optical experiment where a Shack-Hartmann sensor was used. Figure 9 gives the dependence of the number of subapertures at which the maximum illumination is below the threshold. The threshold illumination is taken to be 1,5 times higher than the background illumination. Apparently this level can be considered a threshold level from the point of view of the possibility of operation of the phase sensor. In Figure 9, the following notations are used: N1 – the number of subapertures in which the illumination at the maximum of the diffraction pattern is below the threshold, N0 – the total number of fully illuminated subapertures in the exit pupil of the telescope. Thus, it turns out that in individual frames the light level “fades” to almost zero. Such subapertures should be excluded from analysis when operating the sensor. This in turn leads to a loss of accuracy of its operation.
In addition to culling individual subapertures, it is also possible to use multi-stage phase correction to combat the influence of amplitude fluctuations, for example, using non-phase sensors to measure fluctuations in the overall tilt and wavefront defocusing.

9. Experiments with WFS

Of course, a real limitation on the possibility of conducting correct phase measurements can be considered the loss of illumination at some subapertures of the Shack-Hartmann WFS. In this case, practical vignetting of the sensor’s entrance pupil occurs, which may be due, for example, to a low signal-to-noise ratio [32]. As a result, the number of subapertures whose data is used for wavefront reconstruction will be reduced. In this regard, we consider it important to assess the influence of vignetting of part of the sensor aperture on the accuracy of wavefront reconstruction.
To test this position, an experiment was set up. Its goal [33] was to experimentally evaluate the effect of the loss of part of the measurement data in the sensor during vignetting or central shielding of the entrance pupil of the optical system on the reconstruction of the phase front by the Shack-Hartmann wavefront sensor. The diagram of the optical experiment is shown in Figure 10. The laser radiation beam passed along a horizontal atmospheric path. At reception, optical radiation passing through a layer of a turbulent medium fell on two Shack-Hartmann WFSs. The measurements were carried out on a horizontal atmospheric path with a length of about 105 meters. The measurements were carried out using two identical Shack-Harman wavefront sensors (WFS Sh-H) manufactured by Visionika [28,29].
The collimated laser beam (wavelength 535 nm), having passed through the atmospheric path, was divided into two beams 48 mm in size using a beam splitting cube and received by the receiving aperture of each WFS. During the experiments, the Fried parameter for optical radiation, according to the method proposed in [29], was equal to 1-2 cm. WFS1 worked as a reference sensor in which there are no missing data caused by the effect of shielding or vignetting of the entrance pupil of the optical system. The operation of the second WFS2 was studied in the following configurations: variable shielding coefficients of 13%, 22% and vignetting coefficients of 0.65 were used; 0.45. For this purpose, the corresponding WFS subapertures were programmatically excluded from the calculation when reconstructing the wavefront of laser radiation distorted by atmospheric turbulence. All results were analyzed in terms of Zernike polynomials [11]. The wave front can be represented as a series of Zernike polynomials, where each member of the series characterizes a certain aberration.
Several series of measurements of 1000 frames were performed. The frame rate of the WFS camera is 200 frames per second. To compare the results, the root mean square (RMS) value of the set of measured values of the coefficients for the Zernike polynomials was calculated, since the instantaneous values of the coefficients had different signs. RMS is the square root of the arithmetic mean of the squares of the values. First of all, measurements were carried out with two WFS simultaneously without shielding when laser radiation passed along a horizontal atmospheric path, including three series of measurements (Figure 11).
From the results obtained, it can be concluded that the measurements of the two Sh-H WFS are identical, with the exception of the aberration astigmatism along the X-axis, which is probably due to the mismatch of the planes in which the measurements were performed. To assess the influence of central shielding of the pupil on the reconstruction of the wavefront distorted by atmospheric turbulence, the subapertures in the center of WFS1 with a shielding coefficient of 13% were programmatically excluded (Figure 12), while WFS2 reconstructed the wavefront based on data from all 245 subapertures.
Experimental data on wavefront reconstruction using Shack-Hartmann sensors (when optical waves propagate along a horizontal atmospheric path with turbulence), obtained with vignetting and central screening of the entrance pupil, excluding the corresponding WFS subapertures from phase front reconstruction, showed that:
1. The influence of the central shielding of the input aperture of the WFS does not significantly affect the quality of wavefront reconstruction; only the spherical aberration is underestimated, which may require modification of the adaptive correction algorithm when operating AO systems.
2. When the pupil of the entrance aperture of the WFS is vignetted, differences arise in the magnitude of aberrations of the reconstructed wavefront, which can be considered quantitatively significant for the aberrations coma and astigmatism. Moreover, it was found that the magnitude of these aberrations turns out to be higher than without vignetting.
3. As a result, it can be expected that the distortion of WFS data associated with the non-use of part of the subapertures when reconstructing the wavefront caused by the action of turbulence will affect the quality of operation of the AO system when correcting laser radiation on the atmospheric path.
4. In our experiments with the Shack-Hartmann sensor, it was discovered that there is a dependence of phase systems on the level of intensity fluctuations, which manifests itself, first of all, in a change in the brightness (flickering) of focal spots in the Hartmann matrix. In this case, the phase measurements become incorrect, or rather, errors appear in the assessment of certain mode components.
5. Summarizing the results of these experiments, it should be noted that such a simulation of operation associated with data loss in some subapertures of the Shack-Hartmann wavefront sensor practically simulates cases of complete loss of information from some part of the receiving aperture of the sensor. At the same time, the influence of intensity fluctuations apparently occurs somewhat differently.

10. Conclusion

So, what conclusions can be drawn based on the analysis performed? We investigated the processes occurring in the operation of the Shack-Hartmann type WFS during deep amplitude modulation of the illumination of individual spots, up to their complete fading. Is there a reasonable question? Does this lead to a loss of signal information? It turned out that a possible solution to the problem is to design the operation of the WFS using an analysis of the behavior of the focal patterns of the Hartmann sensor at various threshold illumination values, up to background values. At the same time, a comparison of the behavior of the measured mode components of phase distortions for weak and strong illumination modulations shows that the appearance of intensity fluctuations leads to parasitic modulation of the lower modes of the phase distribution, which causes a loss of efficiency of phase correction. Based on the study of the behavior of the mode components of phase fluctuations, reconstructed from measurement data under various operating modes, it was found that, first of all, the lowest modes of decomposition of phase fluctuations - tilts, defocusing and astigmatism - are subject to distortions and, as analysis shows, these modes can greatly differ from the classical ones corresponding to the regime of weak fluctuations.
One of the methods to combat the influence of flicker can be selection to use only data from “good” subapertures to restore the phase. Also, to combat the influence of intensity fluctuations, it is possible to use multistage phase correction using non-phase sensors to measure fluctuations in the overall slope and defocusing of the wavefront. As an implementation of this, an analysis of the illumination of the focal patterns of the Hartmann sensor was performed using various threshold illumination values, up to a value of 1.5 greater than the background. Also, to combat the influence of amplitude fluctuations, we can recommend the use of multistage phase correction using non-phase sensors to measure fluctuations in the overall slope and defocusing of the wavefront. This increases the coherence of the optical field that the WFS deals with, which reduces the effect of intensity fluctuations on the data received from the WFS.
As for the random signal fading that occurs when the WFS of AO system operates in the region of “strong” fluctuations, when large-scale and long-term continuous signal fading is possible, further in-depth studies are required to explain this.

Supplementary Materials

The following supporting information can be downloaded at the website of this paper posted on Preprints.org.

Funding

This research received no external funding.

References

  1. Born, M.; Wolf, E. Fundamentals of optics; Publisher:, M. Fizmatlit. Russia, 1970, 720 p.
  2. Hiroaki, T.; Takahashi, T. Least-squares phase estimation from the phase difference. J.Opt.Soc.Am. A. 1988, 5, 416–425. [Google Scholar]
  3. Hiroaki, T.; Takahashi, T. Noniterative mothod for obtaining the exact solution for the normal equation in least-squares phase estimation from the phase difference. J. Opt. Soc. Am. A. 1988, 5, 1818–1827. [Google Scholar]
  4. Hunt, B.R. Matrix formulation of the reconstruction of phase value from phase differences. J. Opt. Soc. Am. 1979, 69, 393–399. [Google Scholar] [CrossRef]
  5. Herrmann, J. Least-squares wave front errors of minimum norm. J. Opt. Soc. Am. 1980, 70, 28–35. [Google Scholar] [CrossRef]
  6. Baranova, N.B.; Zeldovich, B.Ya. Dislocations of the wave-front surface and zeros of the amplitude. Zh. Eksp. Teor. Fiz. 1981, 8, 1789–1797. [Google Scholar]
  7. Fried, D.L.; Vaught, J.L. Branch cuts in the phase function. Appl. Opt. 1992, 31, pp.2865–2882 •. [Google Scholar] [CrossRef] [PubMed]
  8. Fortes, B.V.; Kanev, F.Yu.; Konyaev, P.A.; Lukin, V.P. Potential capabilities of аdaptive optical systems in the atmosphere. J. Opt. Soc. Am. A. 1994, 11, 903–907. [Google Scholar] [CrossRef]
  9. Primmerman, C.A.; Price, T.R.; Humphreys, R.A.; Zollars, B.G.; Barclay, H.T.; Herrmann, J. Appl. Opt. 1995, 34, 2081–2089. [CrossRef] [PubMed]
  10. Baranova, N.B.; Mamaev, A.V.; Pilipetski, N.F.; Shkunov, V.V.; Zel’dovich, B.Ya. Wave-front dislocations: topological limitations for adaptive systems with phase conjugation. J. Opt. Soc. Am. 1983, 7, 525–528. [Google Scholar] [CrossRef]
  11. Baranova, N.B.; Zel’dovich, B.Ya. Dislocations of the wave-front surface and zeros of amplitude. Zh. Eksp. Teor. Fiz. 1981, 8, l789–l797. [Google Scholar]
  12. Lukin, V.P.; Fortes, B.V. Estimation of turbulent degradation and required spatial resolution of adaptive systems. Proc. SPIE. Remote Sensing 1998, 3494, 191–202. [Google Scholar]
  13. Arsenyan, T.I.; Kaul, S.I.; Korolenko, P.V.; Ubogov, S.A.; Fedotov, N.N. Wavefront dislocations in the turbulence medium. Radiotekhn. Elektron. 1992, 37, l773–l777. [Google Scholar]
  14. Arsenyan, T.I.; Korolenko, P.V.; Kul’yagina, E.A.; Fedotov, N.N. Amplitude and phase distortions and wavefront dislocations of optical beams on sloping path neart he earth. Radiotekhn. Elektron. 1994, 39, 1471–1476. [Google Scholar]
  15. Aksenov, V.P.; Kolosov, V.V.; Tartakowskii, V.A.; Fortes, B.V. Optical vortices in inhomogeneous media. Atmosperic and Oceanic Optics 1999, 12, pp.912–919. [Google Scholar]
  16. Gurvich, A.S.; Kon, A.I.; Mironov, V.L.; Khmelevtsov, S.S. Laser radiation in a turbulent atmosphere. Publisher: Moscow: Science, Russia, 1976; 277 p.
  17. Noll, R.J. Zernike polynomials and atmospheric turbulence. J. Opt. Soc. Am. 1976. 66, 207–211. [CrossRef]
  18. Lukin, V.P.; Fortes, B.V. Adaptive correction of a focused beam under conditions of strong intensity fluctuations. Atmospheric and Oceanic Optics 2000. 13, 515–520.
  19. Lukin, V.P.; Sennikov, V.A.; Tartakovsky, V.A. Optical vortices: creation, annihilation, and modeling. SPIE Press. Selected Papers. 2000, 4338, 57–67. [Google Scholar]
  20. Lukin, V.P.; Fortes, B.V. Adaptive correction of the focused beam in conditions with strong fluctuations of intensity. Proc. SPIE 2000, 4034, 176–183. [Google Scholar]
  21. Barchers, J.D. Optimal control of laser beams for propagation through a turbulent medium J. Opt. Soc. Am. A. 2001, 18, 3098–3109. [Google Scholar] [CrossRef] [PubMed]
  22. Lukin, V.P.; Fortes, B.V. Phase-correction of turbulent distortions of an optical wave propagating under strong intensity fluctuations. Applied Optics 2002, 41, 5616–5624. [Google Scholar] [CrossRef]
  23. Lukin, V.P.; Kanev, F.Yu.; Sennikov, V.A.; Makenova, N.A.; Tartakovsky, V.A.; Konyaev, P.A. Quantum Electronics 2004, 33, 825–832. [CrossRef]
  24. Lukin, V.P.; Botygina, N.N.; Emaleev, O.N.; Lavrinov, V.V. Peculiarities of adaptive phase correction of optical wave distortions under conditions of ‘strong’ intensity fluctuations. Quantum Electronics 2020, 50, 866–875. [Google Scholar] [CrossRef]
  25. Special Issue on Adaptive Optics. Lincoln Laboratory Journal 1992, 5, 1–25.
  26. Lukin, V.P.; Botygina, N.N.; Konyaev, P.A.; Kulagin, O.V.; Gorbunov, I.A. The combined use of adaptive optics and nonlinear optical wavefront reversal techniques to compensate for turbulent distortions when focusing laser radiation on distant objects. Computer Optics 2020, 44, 519–532. [Google Scholar] [CrossRef]
  27. Kazakov, D.V.; Lukin, V.P. Study of the influence of amplitude fluctuations on phase measurements. Collection of proceedings of the conference “Actual problems of radiophysics”: X International scientific and practical conference. Publisher: TSU Publishing House, Russia. 2023, A43, 229-233.
  28. Larichev, A.V.; Iroshnikov, N.G. Certificate of state registration of the computer program “Specialized software for image processing”. Version 13 (Shah). № 2021619024, 2021.
  29. Andreeva, M.S.; Iroshnikov, N.G.; Koryabin, A.V.; Larichev, A.V.; Shmalgauzen, V.I. Using a wavefront sensor to estimate the parameters of atmospheric turbulence. Optoelectronics, Instrumentation and Data Processing 2012, 48, 103–111. [Google Scholar] [CrossRef]
  30. GOST RISO 15367-2-2012 Lasers and laser installations (systems). Methods for measuring the wavefront shape of a laser radiation beam. Part 2. Publisher: M.: Standartinform, Russia, 2013.
  31. Huang, J.; Yao, L.; Wu, S.; Wang, G. Wavefront Reconstruction of Shack-Hartmann with Under-Sampling of Sub-Apertures. Photonics 2023, 10, 65. [Google Scholar] [CrossRef]
  32. Mikhelson, N.N. Optical telescopes. Theory and design. Publisher: M.: Nauka, Russia. 1976. 512 p.
  33. Bolbasova, L.A.; Lukin, V.P.; Soin, E.L. Experimental studies of the influence of vignetting and central shielding of the entrance pupil on the reconstruction of a wavefront distorted by atmospheric turbulence using a Shack-Hartmann sensor. Journal of Optical Technology 2024.
Preprints 103491 g001
Figure 1. Dependence of the Strehl ratio SR on the scintillation index during phase correction of fluctuations in the potential phase of the “receiving” signal (according to scheme 2).
Figure 1. Dependence of the Strehl ratio SR on the scintillation index during phase correction of fluctuations in the potential phase of the “receiving” signal (according to scheme 2).
Preprints 103491 g001
Preprints 103491 g002
Figure 2. The same as in Figure 1 for a phase conjugation system operating “for transmission” according to scheme 4.
Figure 2. The same as in Figure 1 for a phase conjugation system operating “for transmission” according to scheme 4.
Preprints 103491 g002
Preprints 103491 g003
Figure 3. Comparison of experimental data from the Livermore Laboratory (USA) and calculations [20,22].
Figure 3. Comparison of experimental data from the Livermore Laboratory (USA) and calculations [20,22].
Preprints 103491 g003
Preprints 103491 g004aPreprints 103491 g004b
Figure 4. Spectral filtering functions for correlation functions of phase and amplitude fluctuations, calculated using formula (9).
Figure 4. Spectral filtering functions for correlation functions of phase and amplitude fluctuations, calculated using formula (9).
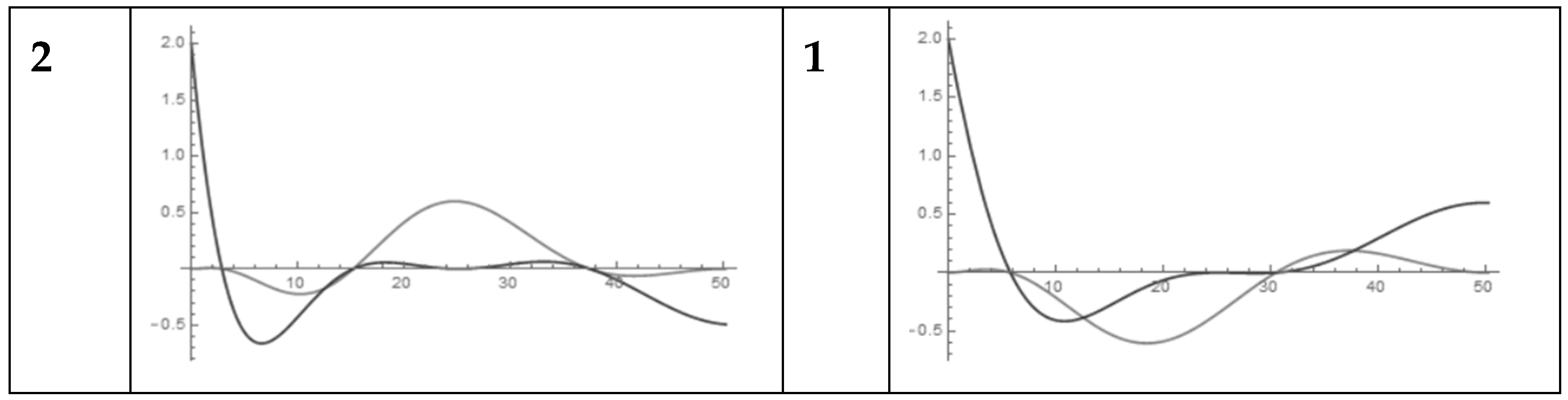
Preprints 103491 g004aPreprints 103491 g004b
Preprints 103491 g005
Figure 5. Spectral filtering functions for the structure functions of phase and intensity, calculated using formula (10).
Figure 5. Spectral filtering functions for the structure functions of phase and intensity, calculated using formula (10).
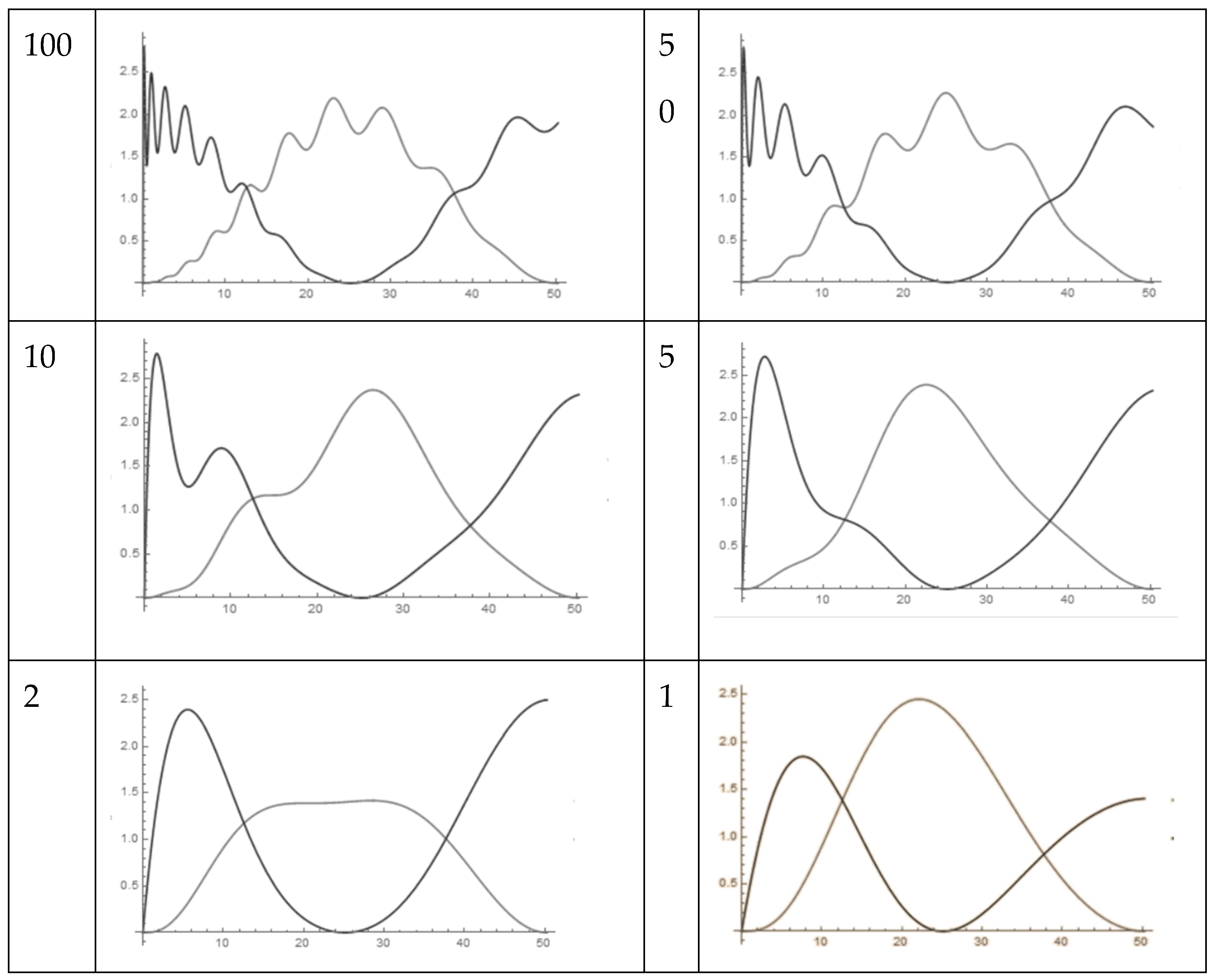
Preprints 103491 g005
Preprints 103491 g006
Figure 6. Appearance of the pattern of focal spots in the Shack-Hartmann sensor with weak (left) and strong (right) intensity fluctuations in the optical wave.
Figure 6. Appearance of the pattern of focal spots in the Shack-Hartmann sensor with weak (left) and strong (right) intensity fluctuations in the optical wave.
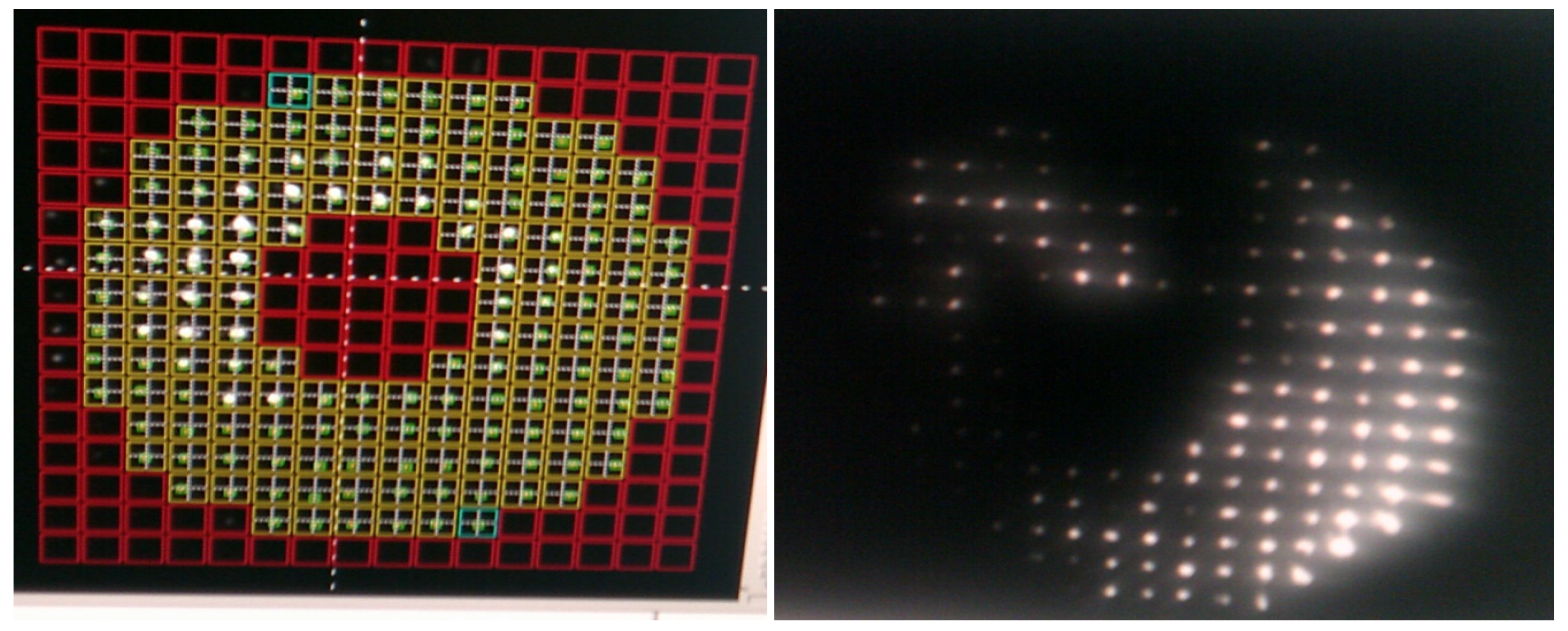
Preprints 103491 g006
Preprints 103491 g007
Figure 7. Example of illumination distribution (for two different frames) of focal spots across the aperture of the wavefront sensor at different illumination values.
Figure 7. Example of illumination distribution (for two different frames) of focal spots across the aperture of the wavefront sensor at different illumination values.
Preprints 103491 g007
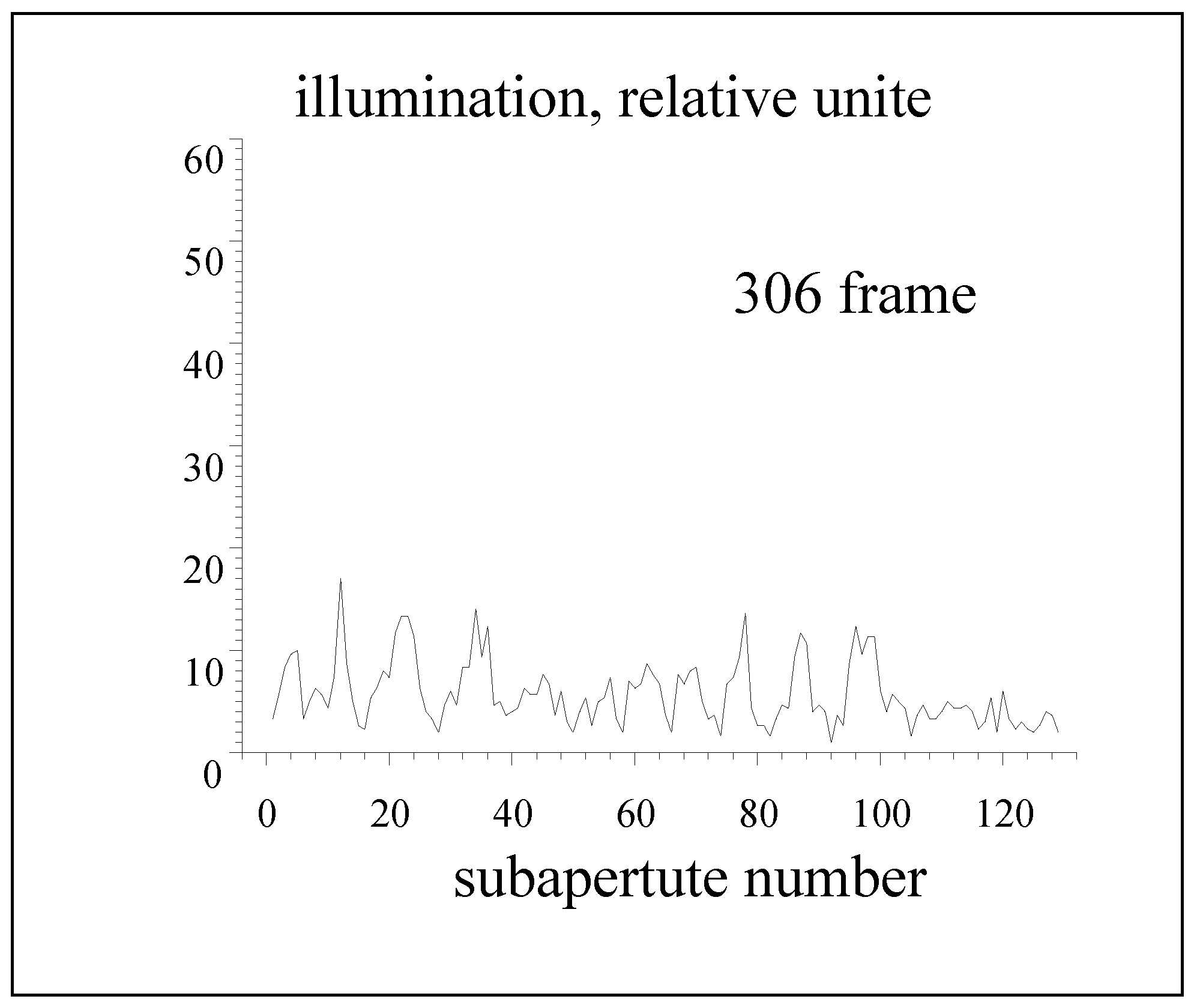
Preprints 103491 g008
Figure 8. Example of illumination distribution (for two different frames) of focal spots across the aperture of the wavefront sensor at different illumination values.
Figure 8. Example of illumination distribution (for two different frames) of focal spots across the aperture of the wavefront sensor at different illumination values.
Preprints 103491 g008
Preprints 103491 g009
Figure 9. Time dependence of the normalized number of subapertures that form images with maximum illumination below the threshold (this threshold of illumination value is 1,5 times greater than background).
Figure 9. Time dependence of the normalized number of subapertures that form images with maximum illumination below the threshold (this threshold of illumination value is 1,5 times greater than background).
Preprints 103491 g009
Preprints 103491 g010
Figure 10. Experimental scheme.
Figure 10. Experimental scheme.
Preprints 103491 g010
Preprints 103491 g011
Figure 11. Simultaneous measurements with two sensors WFS1 and WFS2 at full aperture.
Figure 11. Simultaneous measurements with two sensors WFS1 and WFS2 at full aperture.
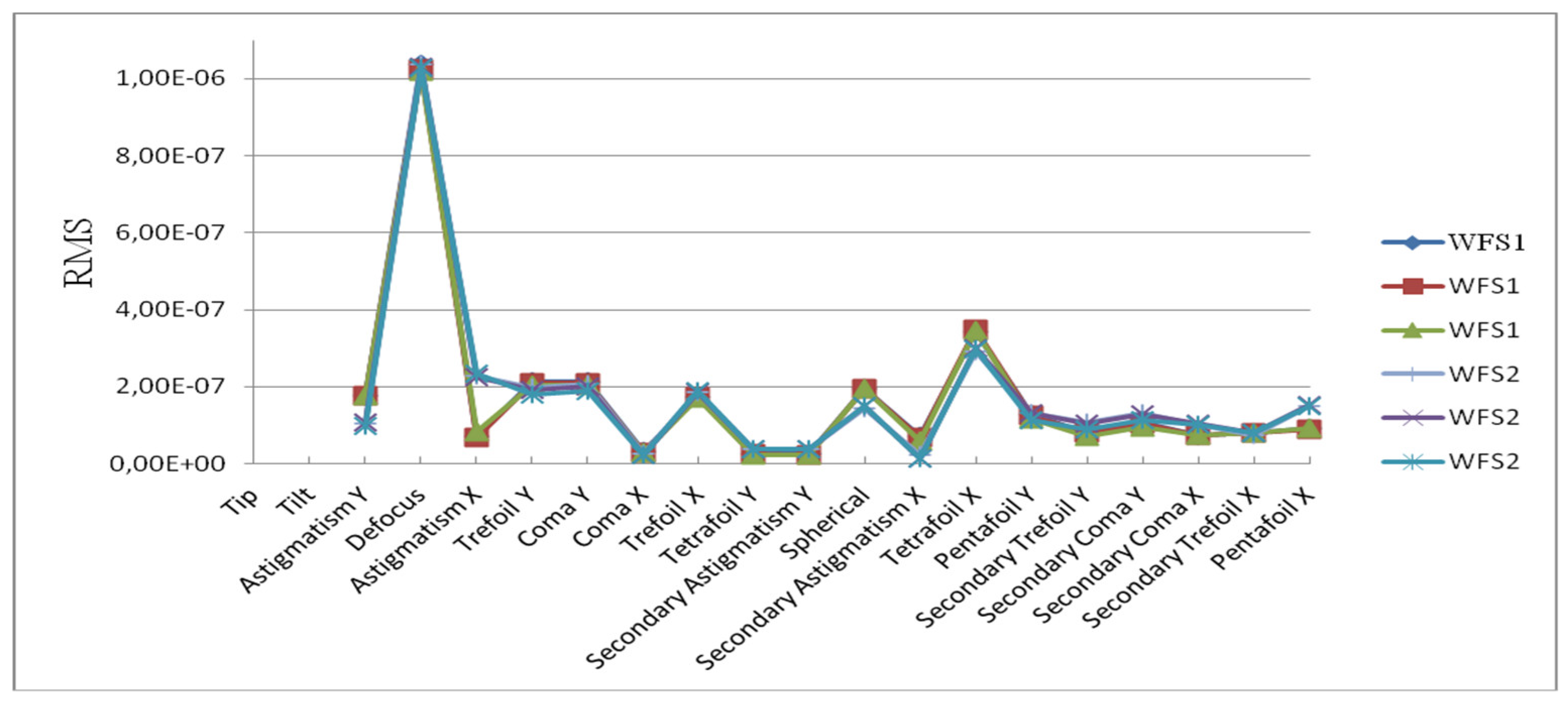
Preprints 103491 g011
Preprints 103491 g012
Figure 12. Simultaneous measurements of the mode components of the phase front: WFS1 with a central shielding coefficient of 13% and WFS2 - without shielding.
Figure 12. Simultaneous measurements of the mode components of the phase front: WFS1 with a central shielding coefficient of 13% and WFS2 - without shielding.
Preprints 103491 g012
Table 1. Strehl parameters for the corrected field in the region of “strong” intensity fluctuations.
Table 1. Strehl parameters for the corrected field in the region of “strong” intensity fluctuations.
D/r0 SR0 SRс SRс/SR0
10 0,0324 0,129 3,98
20 0,0106 0,038 3,58
30 0,0051 0,025 4,90
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated