Approaching the Conformal Limit of Quark Matter with Different Chemical Potentials

Preprint

Article

Approaching the Conformal Limit of Quark Matter with Different Chemical Potentials

Altmetrics

Downloads

Views

Comments

A peer-reviewed article of this preprint also exists.

Connor Brown,

Veronica Dexheimer^*

Rafael Bán Jacobsen

,Ricardo Luciano Sonego Farias

Connor Brown,

Veronica Dexheimer^*

Rafael Bán Jacobsen

,Ricardo Luciano Sonego Farias

This version is not peer-reviewed

Submitted:

02 May 2024

Posted:

03 May 2024

You are already at the latest version

Alerts

Abstract

We study in detail the influence of different chemical potentials (baryon, charged, strange, and neutrino) on how and how fast a free gas of quarks in the zero-temperature limit reaches the conformal limit. We discuss the influence of non-zero masses, the inclusion of leptons, and different constraints, such as charge neutrality, zero-net strangeness, and fixed lepton fraction. We also investigate for the first time how the symmetry energy of the system under some of these conditions approaches the conformal limit. Finally, we briefly discuss what kind of corrections are expected from perturbative QCD as one goes away from the conformal limit.

Keywords:

Subject: Physical Sciences - Nuclear and High Energy Physics

1. Introduction and Formalism

In the zero temperature limit, baryons start to overlap at a few times saturation density and, through some mechanism that is not yet understood, quarks become effectively deconfined [1]. In this work we discuss dense matter in terms of baryon chemical potential

μ_{B}

, instead of baryon (number) density

n_{B}

, as the former (together with other chemical potentials, such as charged

μ_{Q}

or strange

μ_{S}

) is the fixed or independent quantity in the grand canonical ensemble. The correspondence between

n_{B}

and

μ_{B}

is model dependent, but, at finite temperature, the

μ_{B}

at which deconfinement takes place is expected to be even lower (see e.g., [2]), which highlights the importance of studying quark matter. We are particularly interested in understanding the conformal limit, the asymptotically high

μ_{B}

at which matter can be described by a free (non-interacting) gas of massless quarks. For this reason, in the present work, we focus on modelling quark matter only and for the time being restrict ourselves to the zero-temperature limit.

To describe the quarks, we make use of a free Fermi gas under different assumptions. To start, we describe them simply by a massless gas, then introduce different non-zero quark masses, and vary independently the baryon, charged, and strange chemical potentials. We further link the chemical potentials by imposing charge neutrality and/or zero net strangeness. We also discuss the role played by leptons, discussing

β

equilibrium and the role played by neutrinos (with chemical potential

μ_{ν}

). We investigate large

μ_{B}

and different

μ_{Q}

and

μ_{ν}

, as these are important for astrophysical scenarios, such as neutron stars and neutron-star mergers. On the other hand, we investigate the effects of

μ_{S}

, which is important for discussions related to relativistic heavy-ion collisions and the early universe [3].

We also discuss the symmetry energy of quark matter for some of the constraints we study and investigate how it changes as we approach the conformal limit. Several works have addressed the symmetry energy of quark matter [4,5,6,7]. This physical quantity is defined as the difference of energy per baryon

E / N_{B}

(or energy density per baryon density

ε / n_{B}

) of fully isospin asymmetric matter

δ = 1

and isospin-symmetric matter

δ = 0

E_{sym} = \frac{E_{δ = 1}}{N_{B}} - \frac{E_{δ = 0}}{N_{B}} = \frac{ε_{δ = 1}}{n_{B}} - \frac{ε_{δ = 0}}{n_{B}},

(1)

where

δ

was originally defined for matter with neutrons and protons in terms of densities

n_{i}

δ = \frac{n_{n} - n_{p}}{n_{n} + n_{p}} .

(2)

In this case and also when one is considering up and down quarks,

δ

can also be written as

δ = - 2 Y_{I} = 1 - 2 Y_{Q} (non - strange matter),

(3)

with fractions

Y_{i}

summing over

i =

baryons and/or quarks and defined in terms of particle isospin

Q_{I_{i}}

and electric charge

Q_{i}

Y_{I} = \frac{\sum_{i} Q_{I_{i}} n_{i}}{\sum_{i} n_{i}}, Y_{Q} = \frac{\sum_{i} Q_{i} n_{i}}{\sum_{i} n_{i}},

(4)

with baryon (number) density

n_{B} = \sum n_{i}

, where the quark densities are divided by 3.

However, it is important to note that, as discussed in Ref. [8] and Appendix A of Ref. [9], in the presence of hyperons (or in our case strange quarks), Equation (3) does not apply. For this reason, we restrain to the discussion of symmetry energy for the 2-flavor case (with up and down quarks).

When leptons are included, we assume

β

equilibrium, in which case electrons and muons have chemical potential

μ_{e} = μ_{μ} = - μ_{Q}

. In the special case that (electron and muon) neutrinos are trapped,

μ_{ν}

is determined by fixing the lepton fraction

Y_{l} = \frac{\sum_{l e p} n_{l e p}}{\sum_{i} n_{i}},

(5)

usually hold equal to the canonical value

0.4

, to simulate conditions created in supernova explosions [10].

Finally, we briefly discuss the effects of interactions in the case that they are week enough to be discussed perturbatively, i.e., using perturbative Quantum Chromodynamics, pQCD). At large temperatures and/or quark chemical potentials, the strong coupling becomes small enough to allow an infinite number of terms to be approximated by a finite number of terms to describe interactions [11]. At zero temperature, pQCD corrections have been calculated up to next-to-next-to-next-to-leading order (N³LO) [12,13] with non-zero quark masses included until next-to-next-to-leading order (N²LO) [14,15,16] .

2. Results

We describe in detail the free Fermi gas formalism we use in this work (for quarks and leptons) in Appendix A. We begin our discussion by ignoring the contribution of leptons to the thermodynamical quantities (later we include different possibilities and discuss them). In the figures that follow, the pressure P and baryon density

n_{B}

are normalized by respective values of a free gas with the same number of quark flavors included, but with quark masses

m_{i} = 0

and

μ_{Q} = μ_{S} = 0

. Simple analytical equations for the pressure of all the massless cases discussed in this work are derived in Appendix B. We start our discussion considering only one chemical potential, and then expand our discussion to two and three chemical potentials.

2.1. One Chemical Potential $μ_{B}$

We start by comparing the quark mass effect on

n_{B}

versus

μ_{B}

in the left upper panel of Figure 1. Because in this case

μ_{Q}

and

μ_{S}

are zero, all quarks present the same chemical potential

μ_{i} = μ_{u} = μ_{d} = μ_{s} = \frac{1}{3} μ_{B}

. Due to our normalization (thermodynamical quantities divided by the massless case with the respective number of flavors), all massless cases have constant value 1. Nevertheless, this does not mean that they are the same (if not normalized). To discuss the effect of quark masses, we start with 1 flavor with mass corresponding to the Particle Data Group (PDG [17]) mass of the up (

m = 2.3

MeV) or down (

m = 4.8

MeV) quarks, then we look at the 2-flavor case with PDG masses for both light quarks. After that, we look at 3-flavors and use first only non-zero mass for the strange quark (with PDG value of

m = 95

MeV) and then the PDG (from hereon “realistic”) masses for the 3 quarks.

We find that the introduction of realistic quark masses decreases the density for low

μ_{B}

, with the s-quark mass affecting the density until larger

μ_{B}

(up to 621 MeV) than the two light quarks (up to 55 MeV). To calculate these thresholds, we use throughout this paper the criteria of a deviation of

10 %

from the black line with value 1. For P versus

μ_{B}

, shown in the lower panel of Figure 1, the lines are very similar in shape (to the ones in the upper panel of the figure). The introduction of realistic quark masses decreases again P for low

μ_{B}

, with the s-quark mass affecting the pressure until larger

μ_{B}

(up to 834 MeV) than the two light quarks (up to 77 MeV).

2.2. Two Chemical Potentials $μ_{B}$ and $μ_{Q}$

Now, we abandon the unphysical 1-flavor case, and continue with 2- and 3-flavor cases. The 2-flavor case has recently become more relevant for dense matter because it has been shown that the core of neutron stars can harbor 3-, as well as 2-flavor quark matter [18]. For this case we add another (charged) chemical potential, breaking some of the degeneracy in the quark chemical potentials:

μ_{up} = \frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q}

μ_{down} = μ_{strange} = \frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q}

. Once more, we normalize thermodynamical quantities dividing by the respective values of the same quantity for a free gas with the same number of quark flavors included, but with

m_{i} = 0

, in addition to

μ_{Q} = 0

. Following this procedure, we aim at determining how the conformal limit and its deviation depend on

μ_{Q}

When

μ_{Q}

is determined by charge neutrality, the results even for the massless case depend on the number of flavors. In this case, only the 3-flavor case is coincidentally equal to the

μ_{Q} = 0

case (see the explanation following Equations (A29)–(A32) in Appendix B). For 2-flavor, this is not the case, and the pressure is lower than in the

μ_{Q} = 0

case, establishing a new lower conformal limit (see upper panel of Figure 2). Expressions for the pressure for each particular chemical potential case (always keeping

m_{i} = 0

for simplicity) can be found in Appendix B. Compare e.g., Equations (A17) and (A26).

When adding quark masses,

μ_{Q}

determined by charge neutrality lowers the pressure (in comparison to the respective massless case and to the massless case with

μ_{Q} = 0

) such that it goes to the respective conformal limit at larger

μ_{B}

. Using again the criteria of

10 %

deviations from the respective conformal limit, the s-quark mass affects pressure until

μ_{B} = 839

MeV and the two light quark masses until

μ_{B} = 118

MeV.

Nevertheless, one issue about this approach should be noted: we are comparing very small values of

μ_{Q}

with very large values of

μ_{B}

. See the middle panel of Figure 2 for a comparison. This is particularly the case for 3-flavors of quarks, and (except for extremely low

μ_{B}

) this behavior is independent of the quark masses. For small values of

μ_{B}

, both for 2 and 3-flavors, the dependence of

μ_{Q}

and

μ_{B}

can be predicted in fair agreement with Equation (A25). For this reason, next, we add a fixed charged chemical potential to study how it affects the conformal limit, which translates into an increase in pressure (see e.g., the different lines for 3-flavor quark matter with realistic masses in the lower panel of Figure 2), specially at low values of

μ_{B}

. For massless quarks and

μ_{Q} = - 20

MeV, the pressure is always above the conformal limit for

μ_{Q} = 0

, independently of the number of flavors. Once the quark masses are finite, the pressure decreases, specially in the 3-flavor case. For larger absolute values of

μ_{Q}

, the pressure becomes larger, even going above the conformal case (with and without

μ_{Q}

). For example, for the 3-flavor case with realistic quark masses and

μ_{Q} = - 50

MeV, the pressure deviates

10 %

(of the

μ_{Q} = 0

conformal limit) at

μ_{B} = 698

MeV and for

μ_{Q} = - 100

MeV at

μ_{B} = 415

MeV (the latter one from above). Finally, there is one important remark regarding the behavior of the normalized pressure: in the lower panel of Figure 2, it is shown that this physical quantity decreases for small values of

μ_{B}

; however, this behavior doesn’t mean that the pressure itself (not normalized) is not a monotonically increasing function of

μ_{B}

. Here, we must remember that our normalization is carried out by dividing the thermodynamical quantities (such as pressure) by the massless case with the respective number of flavors, and the free Fermi pressure of this system of massless quarks used for normalization scales as

μ_{B}^{4}

; therefore, in those ranges of

μ_{B}

where P for massive quarks increases at a lower rate than

μ_{B}^{4}

, the normalized pressure decreases without implying any thermodynamical inconsistency.

2.3. Three Chemical Potentials $μ_{B}$ , $μ_{Q}$ , and $μ_{S}$ or $μ_{ν}$

Going further, we can add another (strange) chemical potential and constrain it, e.g., to strangeness neutrality. The issue is that at zero temperature strangeness neutrality means that there are no strange quarks, and the 3-flavor reduces to the 2-flavor case. For this reason, we fixed

μ_{S}

instead to specific values.

μ_{S}

breaks the degeneracy in the remaining quark chemical potentials:

μ_{up} = \frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q}

μ_{down} = \frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q}

μ_{strange} = \frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q} + μ_{S}

. Once more, we normalize thermodynamical quantities dividing by the respective values of the same quantity for a free gas with the same number of quark flavors included, but with

m_{i} = 0

, in addition to

μ_{Q} = 0

Fixing

μ_{S}

increases the pressure, similar to fixing

μ_{Q}

. Compare, for example, the massless 3-flavor case in the upper panel in Figure 3 and lower panel in Figure 2 and note that the pressure for a given

μ_{B}

is now much higher. When quark masses are added, the similarity disappears, because

μ_{S}

only affects the strange quarks, which do not appear for low values of

μ_{B}

, unless the

μ_{S}

value is larger than the strange quark mass, which corresponds to our case of

μ_{S} = 100

MeV. For

μ_{S} = 50

and

μ_{S} = 100

MeV, the

10 %

deviation from the conformal limit takes place at

μ_{B} = 1743

and

μ_{B} = 4227

MeV, respectively (both from above).

Now we consider the case in which additionally

μ_{Q} \neq 0

, determined to reproduce charge neutrality (middle panel of Figure 3). For massless 3-flavor quarks, the cases with charge neutrality and without

μ_{Q}

coincide. When masses are introduced, the curves are still very similar (to the upper panel for the

μ_{Q} = 0

case), except at very small

μ_{B}

, where the quark masses are comparable to both

μ_{B}

and

μ_{Q}

. For

μ_{S} = 50

and

μ_{S} = 100

MeV, the

10 %

deviation from the conformal limit takes place at

μ_{B} = 1743

and

μ_{B} = 4227

MeV, respectively (both from above).

When a fixed value of

μ_{Q}

is used, it increases the pressure further, specifically at low

μ_{B}

(see lower panel of Figure 3). For

μ_{Q} = μ_{S} = 50

and

μ_{Q} = μ_{S} = 100

MeV, the

10 %

deviation from the conformal limit takes place at

μ_{B} = 2070

and

μ_{B} = 4723

MeV, respectively (both from above).

Next, we investigate the effects of having much larger values of

μ_{Q}

and

μ_{S}

, comparable to

μ_{B}

, for 3 flavors of quarks in the upper panel of Figure 4. As expected, the changes due to the additional chemical potentials take place at much lower

μ_{B}

(notice the different scale in the y-axis of the figure) and practically all the curves are above the one chemical potential (

μ_{B}

) conformal limit. An exception is the case with large (negative)

μ_{Q}

(and

μ_{S} = 0

) because, according to Equations (A1) and (A5), quarks can only exist after a given

μ_{B} = 381

MeV, at which the momentum

k_{i}

and P become finite (see Equation (A28) for the massless case). In this case, the pressure differs from the one chemical potential conformal limit by more than

10 %

until

μ_{B} = 10 583

MeV. In the case of large

μ_{S}

, quarks can exist at any

μ_{B}

and the pressure differs from the one chemical potential conformal limit by more than

10 %

until

μ_{B} = 44 237

MeV. When we combine large

μ_{S}

and (absolute value of)

μ_{Q}

, the pressure differs from the one chemical potential conformal limit by more than

10 %

until

μ_{B} = 48 897

MeV. In this case, the curve in the upper panel of Figure 4 begins only at

μ_{B} = 1000

MeV. This can be understood once more from Equations (A1) and (A5). The same effect can also be seen (although more subtle) in the bottom panel of Figure 2, where the fixed

μ_{Q}

cases start at

μ_{B} = - μ_{Q}

Finally, we investigate changes due to the inclusion of a free gas of leptons (electrons and muons) in

β

equilibrium (and participating in the fulfillment of charge neutrality). As it can be seen in the lower panel of Figure 4, the inclusion of leptons doesn’t change the pressure. The picture changes though when lepton number is fixed. In this case, which also includes neutrinos, the pressure is considerably higher because the large amount of negative leptons forces the appearance of a large amount of up quarks, changing considerably the quark composition of the system. The grey full line shows a kink for

μ_{B} \sim 400

MeV, when the muons appear. Note that the difference in massless versus massive quarks is still very pronounced when

Y_{l}

is fixed.

2.4. Symmetry Energy

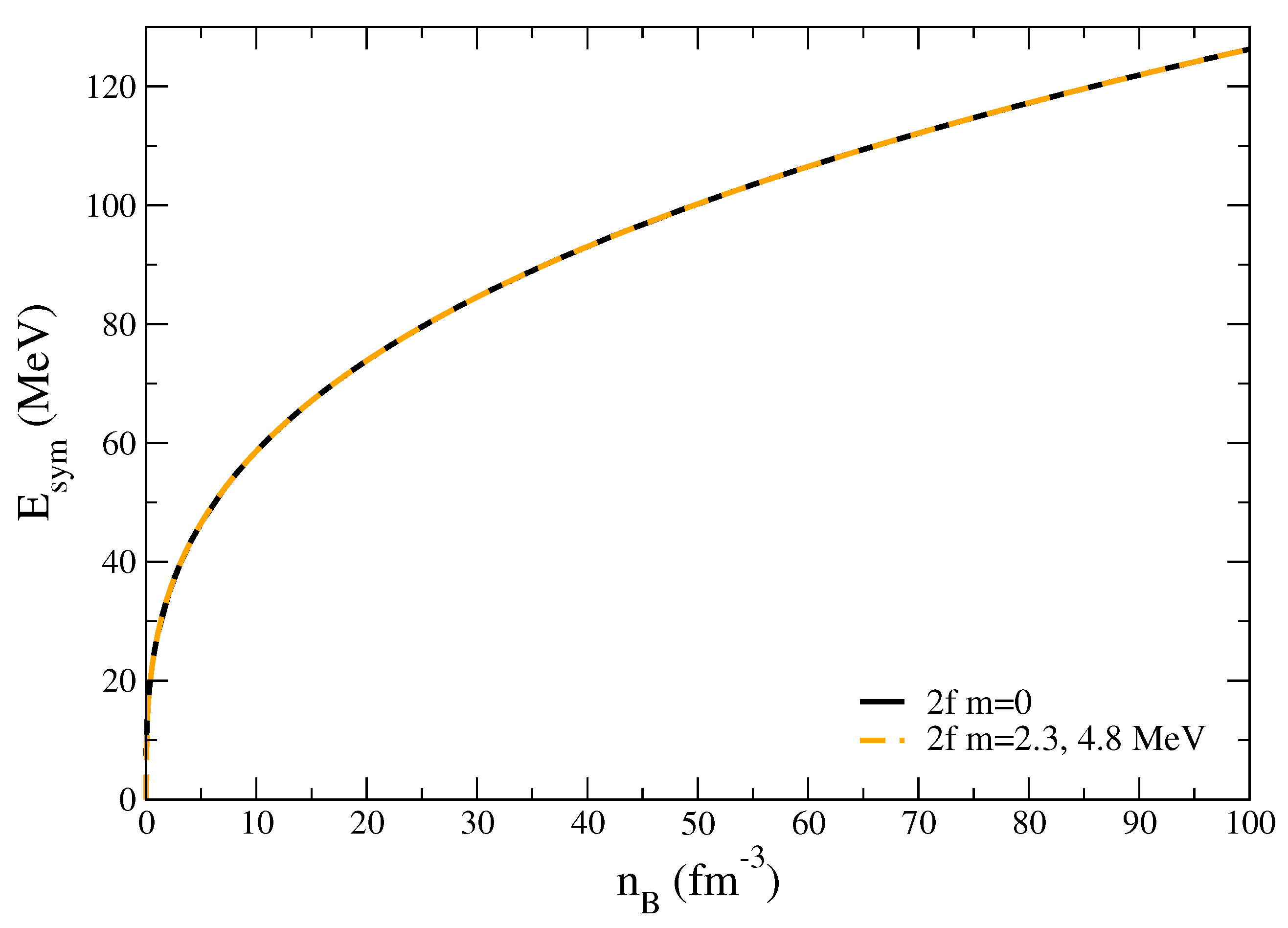
As already discussed, we calculate the symmetry energy only for the 2-flavor case, for which it was originally defined. We fix

n_{B}

in this case (instead of

μ_{B}

as we have been doing) because the symmetry energy is defined for a given

n_{B}

, but limit the x-axis to approximately the corresponding range from the previous figures. Figure 5 shows that the curves are a monotonically increasing function of density. The light quark masses don’t affect the results. Notice that the latter statement applies to every thermodynamical quantity that is not normalized by the respective conformal limit (and does not include derivatives). Numerically, we define

δ = 0

as the 2-flavor

μ_{Q} = 0

case (corresponding to the 2-flavor lines in Figure 1) and

δ = 1

as the 2-flavor

Y_{Q} = 0

case (with

μ_{Q} \neq 0

corresponding to the 2-flavor lines in the top and middle panels of Figure 2).

3. Discussion and Conclusions

Perturbative corrections to a free gas of quarks due to interactions always bring down the pressure to lower values. Although these corrections have been calculated to higher orders for massless and massive (strange) quarks, they cannot accurately be carried out to low baryon chemical potentials

μ_{B}

(or, interchangeably, low baryon densities

n_{B}

in the zero-temperature limit). For example, for the relevant regime of densities inside neutron stars,

μ_{B} \leq 1500

MeV, pQCD predicts that the pressure is lower than

80 %

of the free gas value (see for example Figure 1 of Ref. [15]) but with a very large band going all the way to

P = 0

In this work, we have investigated the equation of state of a free gas of quarks focusing on how the conformal limit is reached when different chemical potentials are varied and different constraints (e.g., , for laboratory vs. astrophysics) are considered. This is done by using combinations of 1, 2, or 3 chemical potentials out of the 4 we consider, each related to a possible conserved quantity: baryon number B (

μ_{B}

), electric charge (

μ_{Q}

), strangeness (

μ_{S}

), and lepton number (

μ_{ν}

). We have also derived expressions for massless quarks under different conditions to illustrate our discussion.

We have studied the effects of using different quark masses (including PDG values), number of flavors, and different ways to fix the various chemical potentials considered. The latter procedure implies enforcing charge neutrality and, when leptons were included,

β

equilibrium. When leptons (electrons, muons, and their respective neutrinos) are present, the pressure in not altered. An exception is the case in which the lepton fraction is fixed. For different cases, we have quantified the deviation from the one-chemical potential (massless) conformal limit by verifying at which

μ_{B}

the pressure deviates by more than

10 %

. This value varied from

μ_{B} = 77

48 897

MeV. This shows that one must be careful about making statements concerning comparisons with "the" conformal limit. Finally, we have shown that the conformal limit of the symmetry energy is monotonically increasing and does not depend on quark masses.

Acknowledgments

We acknowledge support from the National Science Foundation under grants PHY1748621, MUSES OAC2103680, and NP3M PHY-2116686. C. B. acknowledges support from the Kent State University SURE program. This work was partially supported by Conselho Nacional de Desenvolvimento Científico e Tecnológico (CNPq), Grant No. 312032/2023-4 (R.L.S.F.) and is also part of the project Instituto Nacional de Ciência e Tecnologia - Física Nuclear e Aplicações (INCT - FNA), Grant No. 464898/2014-5 (R.L.S.F.)

Abbreviations

The following abbreviations are used in this manuscript:

perturbative Quantum Chromodynamics(pQCD),Particle Data Group(PDG).

Appendix A. General Expressions

For each quark flavor i, we can write

μ_{i} = \frac{1}{3} μ_{B} + Q_{i} μ_{Q} + Q_{S_{i}} μ_{S},

(A1)

where

1 / 3

has been used as the baryon number and

Q_{i}

and

Q_{S_{i}}

are the electric charge and strangeness of each quark.

μ_{B}

μ_{Q}

, and

μ_{S}

are the baryon, charged, and strange independent chemical potentials of the system. In our formalism, the isospin chemical potential

μ_{I} = μ_{Q}

[8].

The general expressions for (number) density, energy density and pressure of a relativistic free Fermi gas of particles i using thee natural system of units are

n_{i} = \frac{g_{i}}{2 π^{2}} \int_{0}^{\infty} d k_{i} k_{i}^{2} (f_{i_{+}} - f_{i_{-}}),

(A2)

ε_{i} = \frac{g_{i}}{2 π^{2}} \int_{0}^{\infty} d k_{i} E_{i} k_{i}^{2} (f_{i_{+}} + f_{i_{-}}),

(A3)

P_{i} = \frac{1}{3} \frac{g_{i}}{2 π^{2}} \int_{0}^{\infty} d k_{i} \frac{k_{i}^{4}}{E_{i}} (f_{i_{+}} + f_{i_{-}}),

(A4)

where

g_{i} = 6

is the spin and color degeneracy factor,

k_{i}

is the momentum,

E_{i} = \sqrt{k_{i}^{2} + m_{i}^{2}} \geq 0,

(A5)

is the energy of the state,

m_{i}

the mass,

f_{\pm}

the distribution function of particles and antiparticles

f_{i_{\pm}} = {(e^{(E_{i} \mp μ_{i}) / T} + 1)}^{- 1}

, with

μ_{i}

being the particle chemical potential, and T the temperature.

In the

T = 0

limit, antiparticles provide no contribution,

f_{-} = 0

, and

f_{+} = 1

up to the Fermi momentum,

k_{i} = k_{F_{i}}

E_{i} = μ_{i}

and the integrals for the above thermodynamic quantities are evaluable analytically

n_{i} = \frac{g_{i}}{6 π^{2}} k_{F_{i}}^{3},

(A6)

ε_{i} = \frac{g_{i}}{2 π^{2}} [(\frac{1}{8} m_{i}^{2} k_{F_{i}} + \frac{1}{4} k_{F_{i}}^{3}) \sqrt{m_{i}^{2} + k_{F_{i}}^{2}} - \frac{1}{8} m_{i}^{4} ln \frac{k_{F_{i}} + \sqrt{m_{i}^{2} + k_{F_{i}}^{2}}}{m_{i}}],

(A7)

P_{i} = \frac{1}{3} \frac{g_{i}}{2 π^{2}} [(\frac{1}{4} k_{F_{i}}^{3} - \frac{3}{8} m_{i}^{2} k_{F_{i}}) \sqrt{m_{i}^{2} + k_{F_{i}}^{2}} + \frac{3}{8} m_{i}^{4} ln \frac{k_{F_{i}} + \sqrt{m_{i}^{2} + k_{F_{i}}^{2}}}{m_{i}}] .

(A8)

Appendix B. Massless Quarks

For the massless particle case, the expressions above further reduce to

n_{i} = \frac{g_{i}}{6 π^{2}} k_{F_{i}}^{3} = \frac{g_{i}}{6 π^{2}} μ_{i}^{3},

(A9)

ε_{i} = \frac{g_{i}}{8 π^{2}} k_{F_{i}}^{4} = \frac{g_{i}}{8 π^{2}} μ_{i}^{4},

(A10)

P_{i} = \frac{1}{3} \frac{g_{i}}{8 π^{2}} k_{F_{i}}^{4} = \frac{1}{3} \frac{g_{i}}{8 π^{2}} μ_{i}^{4},

(A11)

reproducing

ε_{i} = 3 P_{i}

Note that, in the case of massless free quarks, we can also write

μ_{i} = k_{i}

. Therefore, we can write the chemical potential for each quark flavor using Equation (A1)

μ_{u} = \frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q} = k_{u},

(A12)

μ_{d} = \frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q} = k_{d},

(A13)

μ_{s} = \frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q} + μ_{S} = k_{s} .

(A14)

We use the convention that both the strangeness and

μ_{S}

are positive. Alternatively, one could use both as negative without changing the results. Equations (A12) and (A13) are equal if

μ_{Q} = 0

. Equations (A12)–(A14) are equal if

μ_{Q} = 0

and

μ_{S} = 0

. The density and pressure of each quark flavor can be written further as

n_{i} = \frac{μ_{i}^{3}}{π^{2}} = \frac{k_{i}^{3}}{π^{2}},

(A15)

P_{i} = \frac{μ_{i}^{4}}{4 π^{2}} = \frac{k_{i}^{4}}{4 π^{2}} .

(A16)

Next, we discuss the pressure for specific conditions concerning number of flavors and chemical potential constraints (not including leptons):

2-flavor, $μ_{Q} = 0$

P = P_{u} + P_{d} = 2 P_{u} = \frac{2 μ_{u}^{4}}{4 π^{2}} = \frac{μ_{B}^{4}}{162 π^{2}} = \frac{μ_{B}^{4}}{1598.88} .

(A17)

3-flavor, $μ_{Q} = 0$ , $μ_{S} = 0$

P = P_{u} + P_{d} + P_{s} = 3 P_{u} = \frac{3 μ_{u}^{4}}{4 π^{2}} = \frac{μ_{B}^{4}}{108 π^{2}} = \frac{μ_{B}^{4}}{1065.92} .

(A18)

2-flavor, $μ_{Q}$ fixed

\begin{matrix} P = P_{u} + P_{d} & = & \frac{1}{4 π^{2}} (μ_{u}^{4} + μ_{d}^{4}) = \frac{1}{4 π^{2}} [{(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{4} + {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q})}^{4}] \\ = & \frac{1}{4 π^{2}} (\frac{μ_{B}^{4}}{81} + \frac{4 μ_{B}^{3}}{27} \frac{2 μ_{Q}}{3} + \frac{6 μ_{B}^{2}}{9} \frac{4 μ_{Q}^{2}}{9} + \frac{4 μ_{B}}{3} \frac{8 μ_{Q}^{3}}{27} + \frac{16 μ_{Q}^{4}}{81} \\ + & \frac{μ_{B}^{4}}{81} - \frac{4 μ_{B}^{3}}{27} \frac{μ_{Q}}{3} + \frac{6 μ_{B}^{2}}{9} \frac{μ_{Q}^{2}}{9} - \frac{4 μ_{B}}{3} \frac{μ_{Q}^{3}}{27} + \frac{μ_{Q}^{4}}{81}) \\ = & \frac{1}{324 π^{2}} [2 μ_{B}^{4} + 4 μ_{B}^{3} μ_{Q} + 30 μ_{B}^{2} μ_{Q}^{2} + 28 μ_{B} μ_{Q}^{3} + 17 μ_{Q}^{4}] . \end{matrix}

(A19)

2-flavor, $μ_{Q}$ from charge neutrality

Starting from

\sum_{i} Q_{i} n_{i} = 0

\frac{2}{3} n_{u} - \frac{1}{3} n_{d} = 0,

(A20)

\frac{2}{3} \frac{μ_{u}^{3}}{π^{2}} - \frac{1}{3} \frac{μ_{d}^{3}}{π^{2}} = 0,

(A21)

2 μ_{u}^{3} = μ_{d}^{3},

(A22)

2 {(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{3} = {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q})}^{3},

(A23)

2^{\frac{1}{3}} \frac{1}{3} μ_{B} - \frac{1}{3} μ_{B} = - 2^{\frac{1}{3}} \frac{2}{3} μ_{Q} - \frac{1}{3} μ_{Q},

(A24)

μ_{Q} = \frac{- (2^{\frac{1}{3}} - 1) μ_{B}}{2^{\frac{4}{3}} + 1} = - 0.07 μ_{B} .

(A25)

We can then use Equations (A22) and (A25) to calculate the pressure

\begin{matrix} P & = & P_{u} + P_{d} = \frac{1}{4 π^{2}} (μ_{u}^{4} + μ_{d}^{4}) = \frac{1}{4 π^{2}} (μ_{u}^{4} + 2^{\frac{4}{3}} μ_{u}^{4}) \\ = & \frac{1}{4 π^{2}} (1 + 2^{\frac{4}{3}}) μ_{u}^{4} = \frac{1}{4 π^{2}} (1 + 2^{\frac{4}{3}}) {(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{4} \\ = & \frac{1}{4 π^{2}} (1 + 2^{\frac{4}{3}}) {[\frac{1}{3} μ_{B} - \frac{2}{3} (\frac{2^{\frac{1}{3}} - 1}{2^{\frac{4}{3}} + 1} μ_{B})]}^{4} \\ = & \frac{1}{4 π^{2}} (1 + 2^{\frac{4}{3}}) {[\frac{2^{\frac{4}{3}} + 1 - 2^{\frac{4}{3}} + 2}{3 (2^{\frac{4}{3}} + 1)}]}^{4} μ_{B}^{4} \\ = & \frac{1}{4 π^{2} {(2^{\frac{4}{3}} + 1)}^{3}} μ_{B}^{4} = \frac{μ_{B}^{4}}{1721.59} . \end{matrix}

(A26)

3-flavor, $μ_{Q}$ fixed, $μ_{S} = 0$

P = P_{u} + P_{d} + P_{s} = \frac{1}{4 π^{2}} (μ_{u}^{4} + μ_{d}^{4} + μ_{s}^{4}) = \frac{1}{4 π^{2}} (μ_{u}^{4} + 2 μ_{d}^{4}),

(A27)

because

μ_{d} = μ_{s}

are equal, resulting in

\begin{matrix} P & = & \frac{1}{4 π^{2}} [{(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{4} + 2 {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q})}^{4}] \\ = & \frac{1}{4 π^{2}} (\frac{μ_{B}^{4}}{81} + \frac{4 μ_{B}^{3}}{27} \frac{2 μ_{Q}}{3} + \frac{6 μ_{B}^{2}}{9} \frac{4 μ_{Q}^{2}}{9} + \frac{4 μ_{B}}{3} \frac{8 μ_{Q}^{3}}{27} + \frac{16 μ_{Q}^{4}}{81} \\ + & 2 \frac{μ_{B}^{4}}{81} - 2 \frac{4 μ_{B}^{3}}{27} \frac{μ_{Q}}{3} + 2 \frac{6 μ_{B}^{2}}{9} \frac{μ_{Q}^{2}}{9} - 2 \frac{4 μ_{B}}{3} \frac{μ_{Q}^{3}}{27} + 2 \frac{μ_{Q}^{4}}{81}) \\ = & \frac{1}{324 π^{2}} (3 μ_{B}^{4} + 36 μ_{B}^{2} μ_{Q}^{2} + 24 μ_{B} μ_{Q}^{3} + 18 μ_{Q}^{4}) . \end{matrix}

(A28)

3-flavor, $μ_{Q}$ from charge neutrality, $μ_{S} = 0$

Starting again from

\sum_{i} Q_{i} n_{i} = 0

\frac{2}{3} n_{u} - \frac{1}{3} n_{d} - \frac{1}{3} n_{s} = 0,

(A29)

\frac{2}{3} (\frac{μ_{u}^{3}}{π^{2}}) - \frac{1}{3} (\frac{μ_{d}^{3}}{π^{2}}) \frac{1}{3} - (\frac{μ_{s}^{3}}{π^{2}}) = 0,

(A30)

2 μ_{u}^{3} - μ_{d}^{3} - μ_{s}^{3} = 0,

(A31)

but, since in this case

μ_{d} = μ_{s}

, we have:

μ_{u}^{3} = μ_{d}^{3},

(A32)

which implies (from Equations (A12) and (A13))

μ_{Q} = 0

and reproduces the 3-flavor case with

μ_{Q} = 0

μ_{S} = 0

3-flavor, zero net strangeness

Starting from

\sum Q_{S_{i}} n_{i} = 0

, at

T = 0

this implies

n_{s} = 0

, no matter if

μ_{Q} = 0

μ_{Q} \neq 0

. As a consequence, this case reproduces the respective 2-flavor case.

3-flavor, $μ_{Q}$ fixed, $μ_{S}$ fixed

\begin{matrix} P & = & P_{u} + P_{d} + P_{s} = \frac{1}{4 π^{2}} (μ_{u}^{4} + μ_{d}^{4} + μ_{s}^{4}) \\ = & \frac{1}{4 π^{2}} [{(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{4} + {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q})}^{4} + {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q} + μ_{S})}^{4}] . \end{matrix}

(A33)

Using the result from Equation (A28)

\begin{matrix} P & = & \frac{1}{324 π^{2}} (3 μ_{B}^{4} + 36 μ_{B}^{2} μ_{Q}^{2} + 24 μ_{B} μ_{Q}^{3} + 18 μ_{Q}^{4}) \\ + & \frac{1}{4 π^{2}} (μ_{S}^{4} - \frac{4}{27} μ_{Q}^{3} μ_{S} - \frac{4}{3} μ_{Q} μ_{S}^{3} + \frac{4}{3} μ_{B} μ_{S}^{3} + \frac{4}{27} μ_{B}^{3} μ_{S} \\ + & \frac{6}{9} μ_{Q}^{2} μ_{S}^{2} + \frac{6}{9} μ_{B}^{2} μ_{S}^{2} - \frac{12}{27} μ_{B}^{2} μ_{Q} μ_{S} + \frac{12}{27} μ_{B} μ_{Q}^{2} μ_{S} - \frac{12}{9} μ_{B} μ_{Q} μ_{S}^{2}) . \end{matrix}

(A34)

3-flavor $μ_{Q} = 0, μ_{S}$ fixed

Using Equation (A34) with

μ_{Q} = 0

P = \frac{1}{π^{2}} (\frac{1}{108} μ_{B}^{4} + \frac{μ_{S}^{4}}{4} + \frac{1}{3} μ_{B} μ_{s}^{3} + \frac{1}{27} μ_{B}^{3} μ_{S} + \frac{1}{6} μ_{B}^{2} μ_{S}^{2}) .

(A35)

3-flavor, $μ_{Q}$ from charge neutrality, $μ_{S}$ fixed

Starting from

\sum Q_{i} n_{i} = 0

\frac{2}{3} n_{u} - \frac{1}{3} n_{d} - \frac{1}{3} n_{s} = 0,

(A36)

2 μ_{u}^{3} - μ_{d}^{3} - μ_{s}^{3} = 0,

(A37)

2 {(\frac{1}{3} μ_{B} + \frac{2}{3} μ_{Q})}^{3} - {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q})}^{3} - {(\frac{1}{3} μ_{B} - \frac{1}{3} μ_{Q} + μ_{S})}^{3} = 0,

(A38)

\begin{matrix} \frac{2 μ_{B}^{3}}{27} + \frac{12 μ_{B}^{2} μ_{Q}}{27} + \frac{24 μ_{B} μ_{Q}^{2}}{27} + \frac{16 μ_{Q}^{3}}{27} - \frac{2 μ_{B}^{3}}{27} + \frac{6 μ_{B}^{2} μ_{Q}}{27} - \frac{6 μ_{B} μ_{Q}^{2}}{27} \\ + & \frac{2 μ_{Q}^{3}}{27} - μ_{S}^{3} - \frac{3 μ_{B}^{2} μ_{S}}{9} + \frac{6 μ_{B} μ_{Q} μ_{S}}{9} - \frac{3 μ_{Q}^{2} μ_{S}}{9} - \frac{3 μ_{B} μ_{S}^{2}}{3} + \frac{3}{3} μ_{Q} μ_{S}^{2} = 0, \end{matrix}

(A39)

\frac{2 μ_{B}^{2} μ_{Q}}{3} + \frac{2 μ_{B} μ_{Q}^{2}}{3} + \frac{2 μ_{Q}^{3}}{3} - μ_{S}^{3} - \frac{μ_{B}^{2} μ_{S}}{3} + \frac{2 μ_{B} μ_{Q} μ_{S}}{3} - \frac{μ_{Q}^{2} μ_{S}}{3} - μ_{B} μ_{S}^{2} + μ_{Q} μ_{S}^{2} = 0 .

(A40)

In the above expression, we still need to isolate

μ_{Q}

and replace in Equation (A34).

References

Baym, G.; Hatsuda, T.; Kojo, T.; Powell, P.D.; Song, Y.; Takatsuka, T. From hadrons to quarks in neutron stars: A review. Rept. Prog. Phys. 2018, 81, 056902. [Google Scholar] [CrossRef]
Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455–1515. [Google Scholar] [CrossRef]
Letessier, J.; Tounsi, A.; Heinz, U.W.; Sollfrank, J.; Rafelski, J. Strangeness conservation in hot nuclear fireballs. Phys. Rev. D 1995, 51, 3408–3435. [Google Scholar] [CrossRef]
Chu, P.C.; Chen, L.W. Quark matter symmetry energy and quark stars. Astrophys. J. 2014, 780, 135. [Google Scholar] [CrossRef]
Chen, L.W. Symmetry Energy in Nucleon and Quark Matter. Nucl. Phys. Rev. 2017, 34, 20–28. [Google Scholar] [CrossRef]
Wu, X.; Ohnishi, A.; Shen, H. Effects of quark-matter symmetry energy on hadron-quark coexistence in neutron-star matter. Phys. Rev. C 2018, 98, 065801. [Google Scholar] [CrossRef]
Thakur, S.; Dhiman, S.K. Temperature Effects in Quark Matter Symmetry Free Energy. DAE Symp. Nucl. Phys. 2017, 62, 696–697. [Google Scholar]
Aryal, K.; Constantinou, C.; Farias, R.L.S.; Dexheimer, V. High-Energy Phase Diagrams with Charge and Isospin Axes under Heavy-Ion Collision and Stellar Conditions. Phys. Rev. D 2020, 102, 076016. [Google Scholar] [CrossRef]
Yao, N.; Sorensen, A.; Dexheimer, V.; Noronha-Hostler, J. Structure in the speed of sound: From neutron stars to heavy-ion collisions. 2023; arXiv:nucl-th/2311.18819]. [Google Scholar]
Burrows, A.; Lattimer, J.M. The birth of neutron stars. Astrophys. J. 1986, 307, 178–196. [Google Scholar] [CrossRef]
Politzer, H.D. Reliable Perturbative Results for Strong Interactions? Phys. Rev. Lett. 1973, 30, 1346–1349. [Google Scholar] [CrossRef]
Gorda, T.; Kurkela, A.; Paatelainen, R.; Säppi, S.; Vuorinen, A. Cold quark matter at N3LO: Soft contributions. Phys. Rev. D 2021, 104, 074015. [Google Scholar] [CrossRef]
Gorda, T.; Kurkela, A.; Paatelainen, R.; Säppi, S.; Vuorinen, A. Soft Interactions in Cold Quark Matter. Phys. Rev. Lett. 2021, 127, 162003. [Google Scholar] [CrossRef] [PubMed]
Kurkela, A.; Romatschke, P.; Vuorinen, A. Cold Quark Matter. Phys. Rev. D 2010, 81, 105021. [Google Scholar] [CrossRef]
Graf, T.; Schaffner-Bielich, J.; Fraga, E.S. The impact of quark masses on pQCD thermodynamics. Eur. Phys. J. A 2016, 52, 208. [Google Scholar] [CrossRef]
Gorda, T.; Säppi, S. Cool quark matter with perturbative quark masses. Phys. Rev. D 2022, 105, 114005. [Google Scholar] [CrossRef]
Workman, R.L.; Others. Review of Particle Physics. PTEP 2022, 2022, 083C01. [Google Scholar] [CrossRef]
Holdom, B.; Ren, J.; Zhang, C. Quark matter may not be strange. Phys. Rev. Lett. 2018, 120, 222001. [Google Scholar] [CrossRef]

Figure 1. Baryon density (upper panel) and pressure (lower panel) of quarks with different number of flavors and different masses normalized by the respective massless cases.

Figure 2. Pressure (upper panel) and charged chemical potential (middle panel) of quarks with 2 chemical potentials normalized by the respective massless case with one chemical potential,

μ_{B}

. The charged chemical potential is determined by charge neutrality. For massless 3-flavor quarks, the cases with and without

μ_{Q}

coincide. Lower panel: Pressure of quarks with 2 chemical potentials, being

μ_{Q}

fixed to different values, normalized by the respective massless case with one chemical potential,

μ_{B}

Figure 2. Pressure (upper panel) and charged chemical potential (middle panel) of quarks with 2 chemical potentials normalized by the respective massless case with one chemical potential,

μ_{B}

. The charged chemical potential is determined by charge neutrality. For massless 3-flavor quarks, the cases with and without

μ_{Q}

coincide. Lower panel: Pressure of quarks with 2 chemical potentials, being

μ_{Q}

fixed to different values, normalized by the respective massless case with one chemical potential,

μ_{B}

Figure 3. Pressure and charged chemical potential of quarks with 2 or 3 chemical potentials, including the strange chemical potential, normalized by the respective massless case (with one chemical potential,

μ_{B}

). The charged chemical potential is either zero (upper panel), determined by charge neutrality (middle panel), or fixed (lower panel). For massless 3-flavor quarks, the cases with charge neutrality and without

μ_{Q}

coincide.

μ_{B}

μ_{Q}

coincide.

Figure 4. Upper panel: Pressure of quarks with 2 or 3 large chemical potentials, normalized by the respective massless case with one chemical potential,

μ_{B}

. Lower panel: Pressure of quarks and leptons with 2 or 3 chemical potentials, normalized by the respective massless case with one chemical potential,

μ_{B}

. For

β

-equilibrium with leptons,

μ_{Q}

is determined by charge neutrality. When neutrinos are present, their chemical potential

μ_{ν}

is determined by fixing the lepton fraction,

Y_{l}

Figure 4. Upper panel: Pressure of quarks with 2 or 3 large chemical potentials, normalized by the respective massless case with one chemical potential,

μ_{B}

. Lower panel: Pressure of quarks and leptons with 2 or 3 chemical potentials, normalized by the respective massless case with one chemical potential,

μ_{B}

. For

β

-equilibrium with leptons,

μ_{Q}

is determined by charge neutrality. When neutrinos are present, their chemical potential

μ_{ν}

is determined by fixing the lepton fraction,

Y_{l}

Figure 5. Symmetry energy of quarks with 1 or 2 chemical potentials as a function of baryon density for different masses. The two curves overlap.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Approaching the Conformal Limit of Quark Matter with Different Chemical Potentials

Abstract

1. Introduction and Formalism

2. Results

2.1. One Chemical Potential μ B

2.2. Two Chemical Potentials μ B and μ Q

2.3. Three Chemical Potentials μ B , μ Q , and μ S or μ ν

2.4. Symmetry Energy

3. Discussion and Conclusions

Acknowledgments

Abbreviations

Appendix A. General Expressions

Appendix B. Massless Quarks

References

MDPI Initiatives

Important Links

Subscribe

2.1. One Chemical Potential $μ_{B}$

2.2. Two Chemical Potentials $μ_{B}$ and $μ_{Q}$

2.3. Three Chemical Potentials $μ_{B}$ , $μ_{Q}$ , and $μ_{S}$ or $μ_{ν}$