Prerpints.org logo
Preprint
Article

The Effect of Sample Configuration on the Measurement of the Bending Properties of Extruded Polystyrene Using Three-Point, Four-Point, and Compression Bending Tests

Altmetrics

Downloads

82

Views

42

Comments

0

A peer-reviewed article of this preprint also exists.

Submitted:

16 May 2024

Posted:

17 May 2024

You are already at the latest version

Alerts
Abstract
Extruded polystyrene (XPS) is frequently used in the construction of many different structures. It is therefore necessary to appropriately characterize its mechanical properties to ensure the safety of said structures. Among the available characterization tests, static bending tests are simple and easy to perform; owing to these characteristics, they should be performed more frequently than other tests. However, in static bending tests on XPS, there are several challenges owing to the high flexibility of XPS, and the chosen testing method and sample configuration affect the accuracy of characterization. In this study, three bending properties (Young’s modulus, proportional limit stress, and bending strength) of samples cut from an XPS panel were determined using three-point bending (TPB), four-point bending (FPB), and compression bending (CB) tests with varying sample span/depth ratios, and statistical analyses were performed to determine the relevance of the tests. The experimental results suggest that the TPB and CB tests were more feasible than the FPB test when the span/depth ratio was appropriately determined. However, clear differences were observed in the sample bending properties determined in these tests. In light of these findings, further studies should be conducted to elucidate these differences.
Keywords: 
Subject: Chemistry and Materials Science  -   Polymers and Plastics

1. Introduction

Extruded polystyrene (XPS) possesses excellent thermal insulation properties; owing to these properties, it is frequently used as an insulating heat source in many different structures [1,2,3,4,5]. Of late, XPS panels have been used for floorings [6,7,8] and as sandwich panels [9,10,11,12] because their lightweight nature attenuates seismic forces. Additionally, the exceptional processability of XPS enhances its structural utilization. Therefore, adequate characterization of the mechanical properties of XPS is necessary to ensure the safety of structure in which XPS is used as the structural element.
A number of mechanical tests have been utilized to characterize the mechanical properties of foam materials, including XPS, such as static compression [4,13,14,15,16], static tension [15], flexural vibration [16,17], static shear [13,17,18], torsional vibration [18], asymmetric four-point bending [18], and static bending tests [4,14,15,16]. Among these tests, the static bending test is simple and easy to perform; therefore, to characterize the mechanical properties of XPS, this particular test should be used more frequently. In addition, three-point bending (TPB) tests of cellular plastics are standardized in ISO 1209-2:2004 [19] and JIS K 7221-2:2006 [20]; therefore, these standards have resulted in the increased utilization of TPB test. In addition, the four-point bending (FPB) test is used as frequently as TPB tests. However, at present, there are a number of concerns regarding the measurement of the mechanical properties of XPS, such as the Young’s modulus, proportional limit stress, and bending strength. When a sample is not sufficiently slender, the deflection induced by the shearing force affects the measurement of the Young’s modulus and the proportional limit stress. In contrast, when a sample is extremely slender, the load–deflection behavior in the large deflection range deviates from that derived using elementary beam theory (EBT) because of the significant flexibility of XPS, and the bending strength cannot be predicted appropriately using equations based on EBT. Therefore, to appropriately characterize the bending properties of XPS using the aforementioned tests, the effect of the sample configuration must be considered in more detail. Independent of the TPB and FPB tests, compression bending (CB) tests have been performed using numerous carbon fiber-reinforced plastics (CFRP) [21,22,23]. In the CB test, bending deformation is induced by applying an axial load to the sample, and the load–deflection relationship can be determined based on elastica theory. Because the CB test can be performed without applying a lateral load to the sample body, there is no stress concentration due to the lateral load, and bending failure due to the stress concentration can be avoided. CB tests involving solid wood [24], plywood and medium-density fiberboard (MDF) [25,26], and cardboard samples [27] at various span/depth ratios have been performed in previous studies, and the bending properties of these samples could be determined appropriately while reducing the effect of the sample configuration in the relevant span/depth ratio range. If the CB test, as well as the TPB and FPB tests, is applicable to measure the bending properties of XPS, then this shows great promise in the field.
In this study, TPB, FPB, and CB tests were performed using XPS samples at various span/depth ratios. The effects of the chosen testing methods and sample configurations were examined through the use of analysis of variance (ANOVA), and the most feasible method for characterizing the bending properties of these samples was investigated using statistical analyses.

2. Theoretical Background

Figure 1a-c illustrate the diagrams of the TPB, FPB, and CB tests, respectively.
The TPB test method is standardized by the International Organization for Standardization and Japanese Industrial Standards as ISO 1209-02:2004 [19] and JIS K 7221-2:2006 [20], respectively, for characterizing the bending properties of rigid cellular plastics. The load applied to the midspan P and deflection at midspan δM can be obtained, as shown in Figure 1a. From EBT, the Young’s modulus in the length direction of the sample Ex is obtained as follows:
E x = 3 L 3 4 B H 3 · Δ P Δ δ M
where L is the distance between the spans, B and H are the width and depth of the sample, respectively, and ΔP/ΔδM represents the slope of the straight line drawn along the initial linear segment in the P-δM relationship. The bending stress at the outer surface of the midspan σx is also derived from EBT as follows:
σ x = 3 P L 2 B H 2
Therefore, the proportional limit stress Yx is determined as
Y x = 3 P N L L 2 B H 2
where PNL is the load where the aforementioned straight line deviates from the P-δM curve. The method used to determine PNL values is described below. The bending strength Fx can be obtained by substituting the maximum load Pmax into P in Eq. (2). However, when the deflection is extremely large, the direction of the reaction force from the supporting point is inclined rather than positioned upward; therefore, it is often difficult to determine the load–deflection relationship and bending strength using EBT. For carbon fiber reinforced plastics (CFRP), the methods used to calculate the bending strength are standardized with consideration of the effect of large deflection as ASTM D 790-17 [28] and JIS K 7074-1988 [29]. The equations determined in these standards were compared with those based on EBT as follows:
F x = 3 P m a x L 2 B H 2   E B T 3 P m a x L 2 B H 2 1 + 6 δ M b L 2 − 4 δ M b L H L   ( A S T M D 790 − 17 ) 3 P m a x L 2 B H 2 1 + 4 δ M b L 2   J I S K 7074 − 1988          
where δMb = δM at P = Pmax.
Although the FPB test used for rigid cellular plastics is not determined in major standards, it can be performed as easily as the TPB test; therefore, the FPB test methods for various materials have been determined by several major standards. As shown in Figure 1b, in the FPB test, two loads of P/2 are symmetrically applied to the trisectional points, and the deflection at the loading point is defined as δL. In the FPB test methods for CFRP standardized in ASTM D6272-17 [30] and JIS K 7074-1988 [31], the deflection is measured at the midspan using equipment such as a linear variable differential transformer (LVDT). However, for lightweight materials such as XPS, deflection cannot be measured accurately because the reaction force from the LVDT is often large, affecting the bending deformation of the sample. Instead of using an LVDT, δM is calculated from δL, based on EBT, as follows:
δ M = 23 20 δ L
Therefore, Ex and σx are obtained from EBT as follows:
E x = 23 L 3 108 B H 3 · Δ P Δ δ M
and
σ x = P L B H 2
In the FPB test, Yx is determined using Eq. (7) as:
Y x = P N L L B H 2
In ASTM D 6272-17 [30] and JIS K 7074-1988 [31], the equations for FPB are also standardized, and they are demonstrated with the equation derived from EBT as:
F x = P m a x L B H 2   E B T P m a x L B H 2 1 + 4.70 δ M b L 2 − 7.04 δ M b L H L   ( A S T M D 6272 − 17 ) P m a x L B H 2 1 + 4644 529 δ M b L 2 − 162 23 δ M b L H L   J I S K 7074 − 1988
In the CB test shown in Figure 1c, the angle between the vertical axis and length direction at the end of the sample is defined as α. In addition, the displacements at the loading point are defined as x, whereas the curvature at the midspan is defined as κΜ. According to elastica theory, x/L = 0-0.0676, 0.0676-0.259, and 0.259-0.543 in the ranges of α = 0-30°, 30-60°, and 60-90°, respectively, and the δM/L-x/L and κML-x/L relationships under the hinged ends are approximated as follows [27]:
δ M L = 0.62390 x L 0.49745   0 ≤ x / L ≤ 0.0676 0.54880 x L 0.45036   0.0676 < x / L ≤ 0.259 0.47334 x L 0.33983   0.259 < x / L ≤ 0.543
and
κ M L = 6.3625 x L 0.50153   0 ≤ x / L ≤ 0.0676 6.8713 x L 0.52978   0.0676 < x / L ≤ 0.259 7.5113 x L 0.59634   0.259 < x / L ≤ 0.543
From the relationships derived using Eq. (10), σx can be derived as
σ x = 6 P δ M B H 2
When the longitudinal strain at the midspan surface is defined as the bending strain εx, it is derived using κM, as follows:
ε x = κ M H 2
Ex, Yx, and Fx can be obtained using the σx-εx relationship derived from Eqs. (12) and (13).

2. Materials and Methods

2.1. Materials

The test samples used in this study were cut from an XPS panel (STYROFOAM B2; Dupont Styro Corporation, Tokyo, Japan) with initial dimensions of 1820, 910, and 25 mm in length, width, and thickness, respectively. The length, width, and thickness directions of the panel are defined as the L-, T-, and Z-axes, respectively. The panel was initially cut using a circular saw and finished to the final dimensions of the sample using a heat wire. The L-, T-, and Z-axes of the panel coincided with the length, depth, and width directions of the sample, respectively; therefore, sample width B was 25 mm. In contrast, the sample depths, H, were cut to 20 mm and 10 mm. For the sample with H = 20 mm, the length varied from 300 mm to 700 mm at intervals of 100 mm, incontrast, it varied from 550 mm to 700 mm at intervals of 50 mm for the sample with H = 10 mm. The distance between supports L varied from 100 to 500 mm at intervals of 100 mm and from 350 to 500 mm at intervals of 50 mm in the samples with H = 20 and 10 mm, respectively. Therefore, the L/H value was varied from 5 to 50 at intervals of 5. The sample was cut such that the length of the overhung portion was 100 mm. Five samples were used for each test. The density of the sample was 25.5 ± 0.3 kg/m3.

2.2. TPB, FPB, and CB Tests

TPB, FPB, and CB tests were performed, and Ex, Yx, and Fx values were obtained by varying the L/H value.
Figure 2 shows photographs of the TPB, FPB, and CB tests conducted in this study. In the TPB and FPB tests, as shown in Figure 2a and b, respectively, the sample was set on a pair of supports with a radius of 10 mm. A lateral load, P, was applied using a loading nose with a radius of 10 mm. In the CB test, when using a sample with H = 20 mm, cylindrical attachments were set on both ends of the sample to enhance bending deformation, and a load was applied via the cylindrical attachment using a steel plate, as shown in Figure 2c, to enhance the rotation at the sample ends. However, when using the sample with H = 10 mm, bending deformation was induced by the weight of the attachment set on the top of the sample prior to the application of the axial load, and Ex, Yx, and Fx could not be appropriately determined using the σx-εx relationship. To solve this issue, the CB test was performed without the use of the top attachment, as shown in Figure 2d. Even under this condition, rotation was easily induced at the sample ends, and the bending properties were appropriately determined.
The crosshead speed x ˙ was derived from the following relationship [27,31]:
x ˙ = ε x ˙ L 2 6 H   T P B 23 ε x ˙ L 2 108 H   ( F P B ) L 0.31434 ε x ˙ L H 1.9939   C B
Similar to the results of a previous study, the strain rate at the midspan ε x ˙ was determined to be 0.06 /sec [27]. Table 1 lists the crosshead speeds adopted under each test condition.
As described above, the P-δM curve was obtained in the TPB and FPB tests, and ΔP/ΔδM and PNL were determined from a straight line drawn along the initial linear segment of the P-δM curve. The PNL value was determined using the load for deviation from linearity at half the thickness of the plotted curve [32]. Ex was determined by substituting ΔP/ΔδM into Eqs. (1) and (6) in the TPB and FPB tests, respectively. Additionally, Yx was determined by substituting PNL into Eqs. (3) and (8) for the TPB and FPB tests, respectively. In contrast, Pmax was obtained from the maximum load, and Fx was determined by substituting Pmax into Eqs. (4) and (9) for the TPB and FPB tests, respectively. The effects of the equations standardized in the ASTM and JIS standards were examined by comparing the results. In the CB test, Ex, Yx, and Fx were directly determined from the σx-εx relationship obtained from Eqs. (12) and (13).

3. Results

In the TPB and FPB tests, the σx-εx relationship can be determined based on the results of EBT; therefore, the P-δM relationships were transformed into σx-εx relationships, and the effects of the test methods and sample configurations were compared using the σx-εx relationships. In the TPB, test, εx is derived as follows:
ε x = 6 B δ M H 2
In the FPB test, εx is derived as follows:
ε x = 108 B δ M 23 H 2
Figure 3 illustrates comparisons of the representative σx-εx relationships calculated using Eqs. (2) and (15) (TPB tests), Eqs. (7) and (16) (FPB tests), and Eqs. (12) and (13) (CB tests). In the TPB and FPB tests, when the L/H value is sufficiently low, bending failure is often induced in an unstable manner when σx reaches σmax. In contrast, when the L/H value increases, this tendency is not enhanced; however, σx gradually decreases after it reaches σmax. Additionally, the σmax value obtained from EBT decreases as L/H increases, particularly in the FPB test results. In contrast, in the CB tests, the σx-εx relationships were found to be similar to one another, except for those with L/H values of 5 and 10, and bending failure was induced immediately after σx reaches σmax.
In the standards for bending tests of CFRP (ASTM D790-17 [28] and ASTM D6272-17 [30]), the methods determined in these standards are not applicable when εx exceeds 0.05, and other mechanical properties, such as tensile strength, may be more relevant for characterizing different materials. The results shown in Figure 3 indicate that εx at the maximum σx often exceeds 0.05 in TPB and FPB tests on samples with L/H values lower than 25. In the standards for the bending tests of cellular solid plastics (ISO 1209-2:2004 [19] and JIS K 7221-2:2006 [20]), the L/H value is determined to be 12-16. However, to determine the bending strength of XPS under εx < 0.05, the L/H value should be greater than 16. In contrast, in the CB test, a plateau portion in the σx-εx relationship was found within the 0.05 εx limit when using the samples with L/H values higher than 15.
The results shown in Figure 4 demonstrate the dependence of Ex on L/H in the TPB, FPB, and CB tests. As described above, in the TPB and FPB tests, Ex usually decreases the deflection induced by the shearing force, which is enhanced as the L/H value decreases. The low values of Ex at L/H = 5 in the TPB and FPB tests were due to the effect of deflection by the shearing force. However, although the results of previous studies on TPB tests performed using solid wood [33,34] indicate that the dependence of Ex on L/H decreases as the L/H value increases, the Ex values continuously increase in the L/H ranges of 5-50 and 5-40 in the TPB and FPB tests, respectively. In these tests, a slight slippage was induced between the sample and support even when the load was not sufficiently large. Therefore, owing to this slippage, the sample deformed as if it were supported by spans with a distance larger than the actual one. The degree of slippage increased as the L/H value increased; therefore, it can be concluded that the Ex value increases as the L/H value increases. In the CB test, the Ex values at L/H = 5 and 10 were lower than those at L/H ≥ 15. When the L/H value is low, material nonlinearity is induced, and the stiffness of the sample is usually reduced due to the occurrence of material nonlinearity. In contrast, when the CB test is performed using a sample with an L/H value higher than 15, the dependence of Ex on L/H is effectively reduced. Therefore, when a sample with an L/H value lower than 10 was not used, it was more advantageous to use the CB test than the TPB and FPB tests because Ex can be obtained stably without slippage between the sample and span. However, the Ex values obtained from the CB tests are often lower than those obtained from the TPB and FPB tests. Further research is therefore required in order to elucidate the reasons for these differences.
Figure 5 shows the dependence of Yx on L/H in the TPB, FPB, and CB tests. Our statistical analyses revealed no significant differences in the results obtained from the TPB tests. In contrast, significant differences were frequently observed in the results obtained from the FPB and CB tests. The details of the results obtained from the statistical analyses are demonstrated below.
Figure 6 shows the dependence of Fx on L/H in the TPB, FPB, and CB tests. In the TPB test, when Fx is calculated based on EBT, it decreases as the L/H value increases. However, when Fx is calculated using the equations standardized in ASTM D790-17 [28] and JIS K 7074-1988 [29], the dependence of Fx on L/H is effectively reduced. No dependence was observed in the results obtained from the CB tests. In contrast, in the FPB test, the dependence was found to be significant, and the equations determined in ASTM D6272-17 [30] and JIS K 7074-1988 [29] were not effective in reducing the dependence.
In the following section, we discuss the results of our statistical analyses of the samples’ bending properties, and the validity of the TPB, FPB, and CB tests.

4. Discussion

The aforementioned results were analyzed via ANOVA (Tukey’s tests), and the effects of L/H and the test methods used are discussed below from a statistical perspective.
Table 2 lists the results of the ANOVA for Ex corresponding to L/H. In the TPB and FPB tests, the differences among the averages of Ex were not found in the L/H value range of 25 to 50. In contrast, when 5 ≤ L/H ≤ 20, the Ex values were lower than those in the range of L/H values higher than 25 because the deflection due to the shearing force cannot be ignored. Although the L/H was determined to be 15 for the TPB test in ISO 1209-2:2004 [19] and JIS K 7221-2:2006 [20], the results of the statistical analyses suggest that it is preferable to perform TPB tests under L/H values higher than the standardized value. In contrast, in the CB test, differences among the averages of Ex were not found in the L/H value range of 15 to 50, which is wider than the range found in the TPB and FPB tests. Therefore, when considering the range of L/H alone, the CB test is superior to the TPB and FPB tests.
Table 3 lists the results of the ANOVA for Yx corresponding to L/H. As described above, there were no significant differences between the Yx values obtained from the TPB tests. In contrast, in the FPB and CB tests, the L/H range where the differences are not significant is restricted to 20 ≤ L/H ≤ 50 and 15 ≤ L/H ≤ 50, respectively.
Table 4 lists the results of the ANOVA for Fx corresponding to L/H. In the TPB tests, the range of L/H values where the differences among the averages are not significant is restricted to 15 ≤ L/H ≤ 50 when using the equations based on EBT, but the significant differences are reduced when Fx is calculated using the equations determined in ASTM D 790-17 [28] and JIS K 7074-1988 [29]. In contrast, the differences cannot be reduced sufficiently in the FPB tests, even when ASTM- and JIS-standardized equations are used. In the CB test, no significant differences were observed between the average Fx. The results of the statistical analyses show that the TPB and CB tests are more advantageous than the FPB test.
Table 5 lists the Ex, Yx, and Fx values obtained by averaging those in the L/H range, where the significance level is higher than 0.05. The Ex, Yx, and Fx values obtained from the TPB and FPB tests were similar. In contrast, the Ex and Fx values obtained from the CB tests were lower than those obtained from the TPB and FPB tests, whereas the Yx values obtained from the CB tests were close to those obtained from the TPB and FPB tests. These tendencies are often discrepant from those obtained using CFRP [21,22,23], solid wood [24], plywood and MDF [25,26], and cardboard [27]. Further research is thus required to elucidate the discrepancies between the results obtained using different materials.

5. Conclusions

In this study, three-point bending (TPB), four-point bending (FPB), and compression bending (CB) tests were performed on extruded polystyrene (XPS) samples to determine their bending properties. The dependence of the properties on the span/depth ratio was statistically analyzed (Tukey’s tests), and the applicability of the three test methods was examined. Through our analyses, the following results were obtained:
(1) In the TPB and FPB tests, Young’s modulus increased continuously as the span/depth ratio increased because of the slippage between the sample and supporting point, as well as the effect of deflection caused by the shearing force. In the CB test, the Young’s modulus was lower in the small span/depth ratio range where material nonlinearity was induced by axial loading prior to bending deformation. Except for this span/depth ratio range, Young’s modulus could be obtained while reducing the effect of the sample configuration.
(2) The effect of the span/depth ratio on the proportional limit stress was not observed in the TPB test results; in contrast, the effect was found in the results obtained from the FPB and CB tests.
(3) To determine the samples’ bending strength by using the TPB test, the methods for correcting the large defection determined in ASTM D 790-17 and JIS K 7074-1988 were effective; the correction methods for the FPB test determined in ASTM 6272-17 and JIS K 7074-1988 were not sufficient, however. In contrast, the effect of the span/depth ratio was not significant in the CB test results.
(4) From the experimental results, the use of TPB and CB tests is recommended over the FPB test to determine the bending properties of XPS samples. However, the properties determined via the TPB and CB tests were different. Further research should be therefore conducted to explore the source of these differences in more detail.

6. Nomenclature

B = sample width
H = sample depth
Ex = Young’s modulus in the length direction of the sample
Fx = bending strength in the length direction of the sample
L = length between the spans
P = load applied to the sample
Pmax= maximum load
x = displacement at the loading point in the compression bending test
x ˙ = crosshead speed
Yx = proportional limit stress in the length direction of the sample
δL = deflection at the loading point in the four-point bending test
δM = deflection at the midspan
δMb = deflection at the midspan at P = Pmax
εx = bending strain at the midspan
ε x ˙ = strain rate at the midspan
κΜ = curvature at the midspan
σx = bending stress at the midspan
ANOVA = analysis of variance
CB = compression bending
EBT = elementary beam theory
FPB = four-point bending
TPB = three-point bending
L, T, and Z = length, width, and thickness directions of the panel, respectively
XPS = extruded polystyrene

Author Contributions

Conceptualization, H.Y.; methodology, H.Y.; software, H.Y.; validation, H.Y., M.Y. and M.M.; formal analysis, H.Y.; investigation, H.Y.; resources, H.Y.; data curation, H.Y. and M.W.; writing—original draft preparation, H.Y.; writing—review and editing, H.Y.; visualization, H.Y.; supervision, M.Y. and M.M.; project administration, H.Y. All the authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Acknowledgments

This work was supported by JSPS KAKENHI Grant Number 23K26970.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Kim, J.; You, Y.-C. Composite behavior of a novel insulated concrete sandwich wall panel reinforced with GFRP shear grids: effect of insulation types. Materials 2015, 8, 899–913. [Google Scholar] [CrossRef]
  2. Cai, S.; Zhang, B.; Cremaschi, L. Review of moisture behavior and thermal performance of polystyrene insulation in building applications. Build. Environ. 2017, 123, 50–65. [Google Scholar] [CrossRef]
  3. Li, Q.; Wei, H.; Han, L.; Wang, F.; Zhang, Y.; Han, S. Feasibility of using modified silty clay and extruded polystyrene (XPS) board as the subgrade thermal insulation layer in a seasonally frozen region, Northeast China. Sustainability 2019, 11, 804. [Google Scholar] [CrossRef]
  4. Aksit, M.; Zhao, C.; Klose, B.; Kreger, K.; Schmidt, H.-W.; Altstädt, V. Extruded polystyrene foams with enhanced insulation and mechanical properties by a benzene-trisamide-based additive. Polymers 2019, 11, 268. [Google Scholar] [CrossRef]
  5. Li, Q.; Wei, H.; Zhou, P.; Zhang, Y.; Han, L.; Han, S. Experimental and numerical research on utilizing modified silty clay and extruded polystyrene (XPS) board as the subgrade thermal insulation layer in a seasonally frozen region, Northeast China. Sustainability 2019, 11, 3495. [Google Scholar] [CrossRef]
  6. Seto, H.; Saito, I.; Onuki, A.; Takeuchi, M.; Tsuchiya, T. Presumption of the source of indoor air pollution. Amounts of styrene and butanol generation from construction materials. Ann. Rep. Tokyo Metr. Res. Lab. P. H. 2000, 51, 219–222. [Google Scholar]
  7. Aoyagi, R.; Matsunobu, K.; Matsumura, T. Development of continuous vapor generation for calibration styrene with permeation tube method. Indoor Environ. 2009, 52, 97–102. [Google Scholar] [CrossRef]
  8. Matsumoto, T.; Iwamae, A.; Wakana, S.; Mihara, N. Effect of the temperature-humidity condition in the room and under the floor by heat insulation tatami and flooring. Summaries of Technical Papers of Annual Meeting, Kobe, Japan, 13 September; Architectural Institute of Japan (Environmental Engineering II): Tokyo, Japan, 2014.
  9. Vinson, J.R. Sandwich structures. Appl. Mech. Rev. 2001, 54, 201–214. [Google Scholar] [CrossRef]
  10. Hu, Y.; Nakao, T.; Nakai, T.; Gu, J.; Wang, F. Dynamic properties of three types of wood-based composites. J. Wood Sci. 2005, 51, 7–12. [Google Scholar] [CrossRef]
  11. Hu, Y.; Nakao, T.; Nakai, T.; Gu, J.; Wang, F. Vibrational properties of wood plastic plywood. J. Wood Sci. 2005, 51, 13–17. [Google Scholar] [CrossRef]
  12. Kawasaki, T.; Kawai, S. Thermal insulation properties of wood-based sandwich panel for use as structural insulated walls and floors. J. Wood Sci. 2005, 52, 75–83. [Google Scholar] [CrossRef]
  13. Kilar, V.; Koren, D.; Bokan-Bosiljkov, V. Evaluation of the performance of extruded polystyrene boards – implications for their application in earthquake engineering. Polym. Test. 2014, 40, 234–244. [Google Scholar] [CrossRef]
  14. Vitau, C.; Dudescu, M.C. Investigation of mechanical behaviour of expanded polystyrene under compressive and bending loadings. Mater. Plast. 2020, 57, 199–207. [Google Scholar]
  15. Tang, N.; Lei, D.; Huang, D.; Xiao, R. Mechanical performance of polystyrene foam (EPS): experimental and numerical analysis. Polym. Test. 2019, 73, 359–365. [Google Scholar] [CrossRef]
  16. Yoshihara, H.; Ataka, N.; Maruta, M. Measurement of the Young’s modulus and shear modulus of extruded polystyrene foam by the longitudinal and flexural vibration methods. J. Cell. Plast. 2018, 54, 199–216. [Google Scholar] [CrossRef]
  17. Yoshihara, H.; Wakahara, M.; Yoshinobu, M.; Maruta, M. Torsional vibration tests of extruded polystyrene with improved accuracy in determining the shear modulus. Polymers 2022, 14, 1148. [Google Scholar] [CrossRef] [PubMed]
  18. Yoshihara, H.; Maruta, M. Measurement of the shear properties of extruded polystyrene by in-plane shear and asymmetric four-point bending tsts. Polymers 2020, 12, 4. [Google Scholar]
  19. ISO 1209-02-2004, Rigid cellular plastics－Determination of flexural properties－Part 2 : Determination of flexural strength and apparent flexural modulus of elasticity, International Organization for Standardization, Geneva, Switzerland, 2004.
  20. JIS K 7221-2:2006, Rigid cellular plastics-Determination of flexural properties-Part 2 : Determination of flexural strength and apparent flexural modulus of elasticity, Japan Standards Association, Tokyo, Japan, 2006.
  21. Fukuda, H. A new bending test method of advanced composites. Exp. Mech. 1989, 29, 330–335. [Google Scholar] [CrossRef]
  22. Fukuda, H.; Katoh, H.; Uesugi, H. A modified procedure to measure bending strength and modulus of advanced composites by means of compression bending. J. Compos. Mater. 1995, 29, 195–207. [Google Scholar] [CrossRef]
  23. Fukuda, H.; Itabashi, M. Simplified compression bending method for advanced composites. Compos. A 1999, 30, 249–256. [Google Scholar] [CrossRef]
  24. Yoshihara, H.; Oka, S. Measurement of bending properties of wood by compression bending tests. J. Wood Sci. 2001, 47, 262–268. [Google Scholar] [CrossRef]
  25. Yoshihara, H. Bending properties of medium-density fiberboard and plywood obtained by compression bending test. Forest Prod. J. 2010, 61, 56–63. [Google Scholar] [CrossRef]
  26. Santabárbara, A.G., Carrió, J.M., Sastre, R.S., Sakata, H. Determination of bending properties of lauan plywood by compression bending tests. In Proceedings, 6th Int. Confer. Technol. Innov. Build. (CITE 2021), Madrid, Spain, (25 3 2021).
  27. Yoshihara, H.; Yoshinobu, M.; Maruta, M. Bending stiffness and moment capacity of cardboard obtained from three-point and elastica bending tests. Nord. Pulp Paper Res. J. 2023, 38, 73–85. [Google Scholar] [CrossRef]
  28. ASTM D790-17, Standard test methods for flexural properties of unreinforced and reinforced plastics and electrical insulating materials, ASTM International, West Conshohocken, PA, 2017.
  29. JIS K 7074-2:1988, Testing methods for flexural properties of carbon fiber reinforced plastics, Japan Standards Association, Tokyo, Japan, 1988.
  30. ASTM D6272-17, Standard test methods for flexural properties of unreinforced and reinforced plastics and electrical insulating materials by four-point bending, ASTM International, West Conshohocken, PA, 2017.
  31. Adams, D.F.; Carlsson, L.A.; Pipes, R.B. Lamina flexural response. In Experimental characterization of advanced composite materials. 3rd Ed.; CRC Press: Boca Raton: USA; 2003, pp. 121-130.
  32. Davies, P.; Blackman, B.R.K.; Brunner, A.J. Mode II delamination. In Fracture mechanics testing methods for polymers adhesives and composites. Moore, D.R.; Pavan, A.; Williams, J.G., Eds.; Elsevier: Amsterdam: Netherland; 2001; ESIS Publication 28, pp. 307-333.
  33. Yoshihara, H.; Kubojima, Y.; Ishimoto, T. Several examinations on the static bending test methods of wood by using todomatsu (Japanese fir). Forest Prod. J. 2003, 53, 39–44. [Google Scholar]
  34. Yoshihara, H.; Itoh, A. Influence of large deflection on the measurement of bending properties of veneer by three-point static bending tests. Wood Fiber Sci. 2003, 35, 293–300. [Google Scholar]
Preprints 106661 g001
Figure 1. Diagrams of the TPB (a), FPB (b), and CB (c) tests.
Figure 1. Diagrams of the TPB (a), FPB (b), and CB (c) tests.
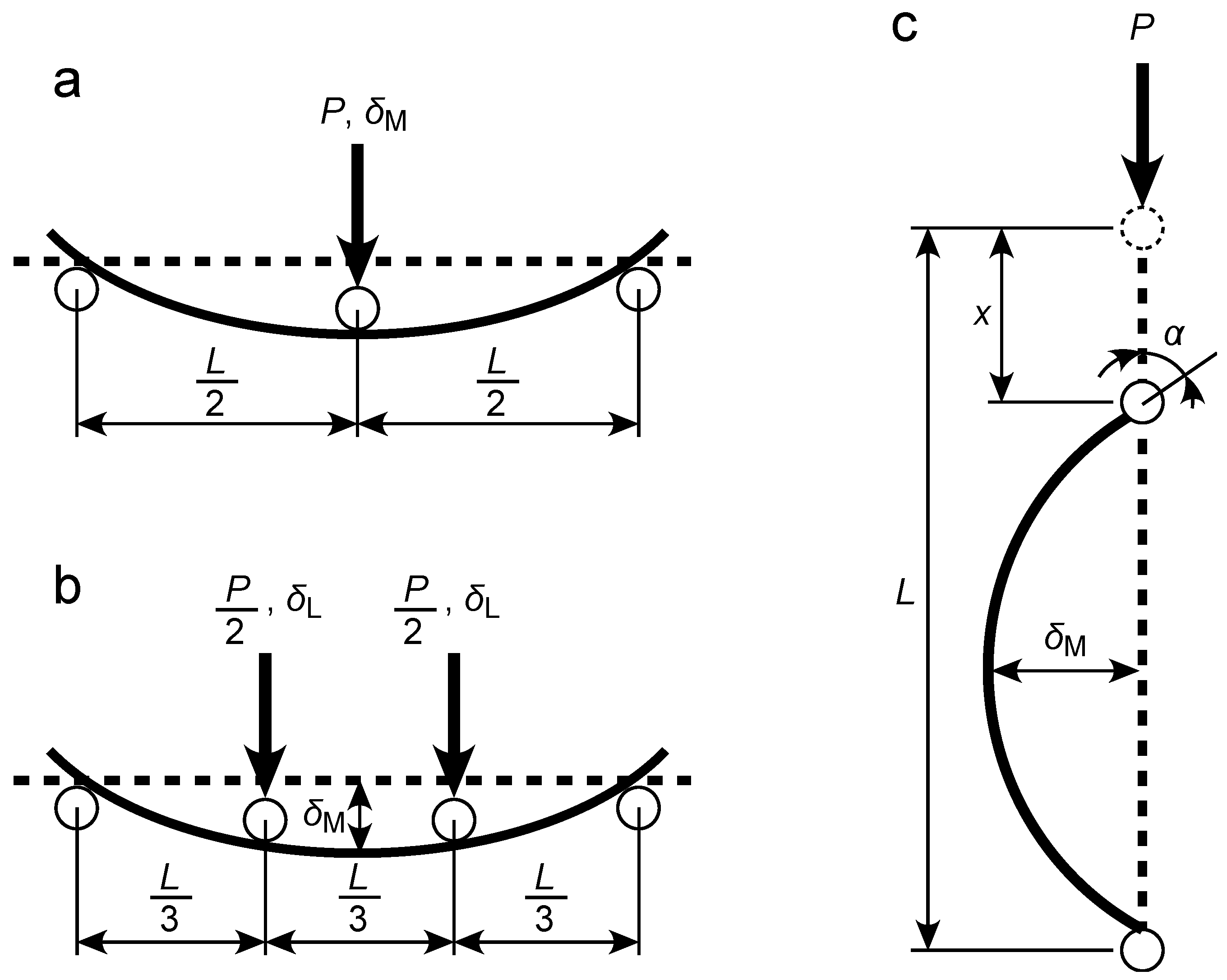
Preprints 106661 g001
Preprints 106661 g002
Figure 2. Photographs of the TPB test (a), FPB test (b), CB test using a sample with H = 20 mm (c), and CB test using a sample with H = 10 mm (d).
Figure 2. Photographs of the TPB test (a), FPB test (b), CB test using a sample with H = 20 mm (c), and CB test using a sample with H = 10 mm (d).
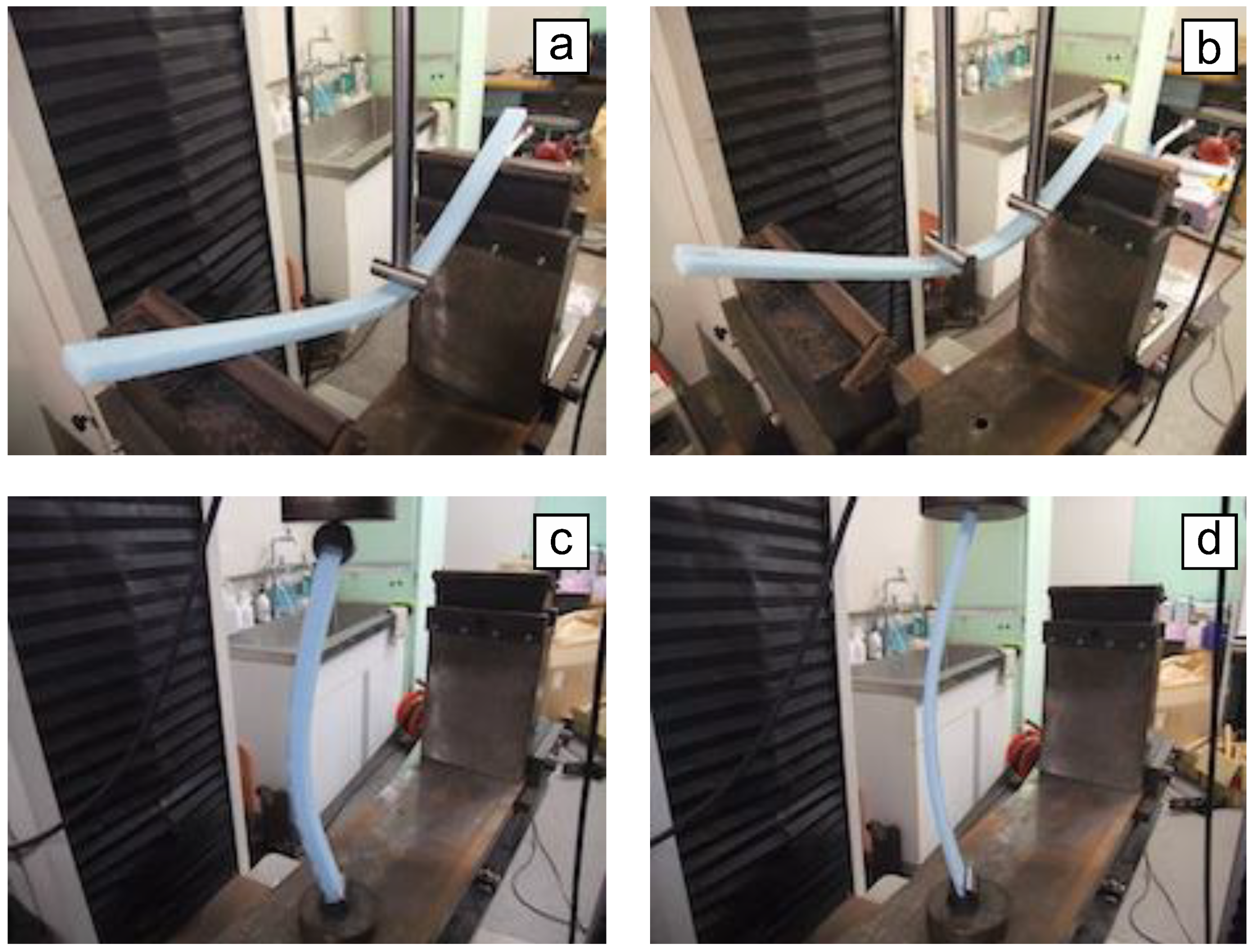
Preprints 106661 g002
Preprints 106661 g003
Figure 3. σx-εx relationships obtained by varying the L/H values in the TPB, FPB, and CB tests.
Figure 3. σx-εx relationships obtained by varying the L/H values in the TPB, FPB, and CB tests.
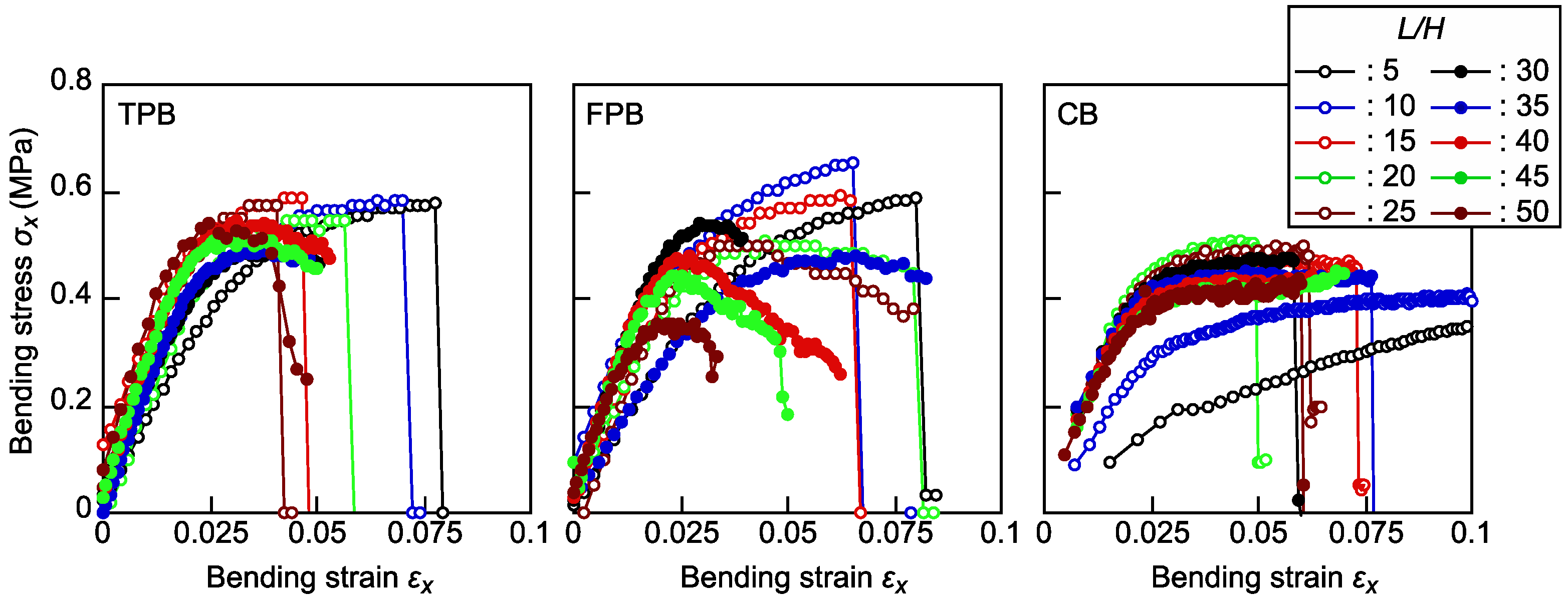
Preprints 106661 g003
Preprints 106661 g004
Figure 4. Dependence of Ex on L/H in the TPB, FPB, and CB tests. Data = average ± standard deviations.
Figure 4. Dependence of Ex on L/H in the TPB, FPB, and CB tests. Data = average ± standard deviations.
Preprints 106661 g004
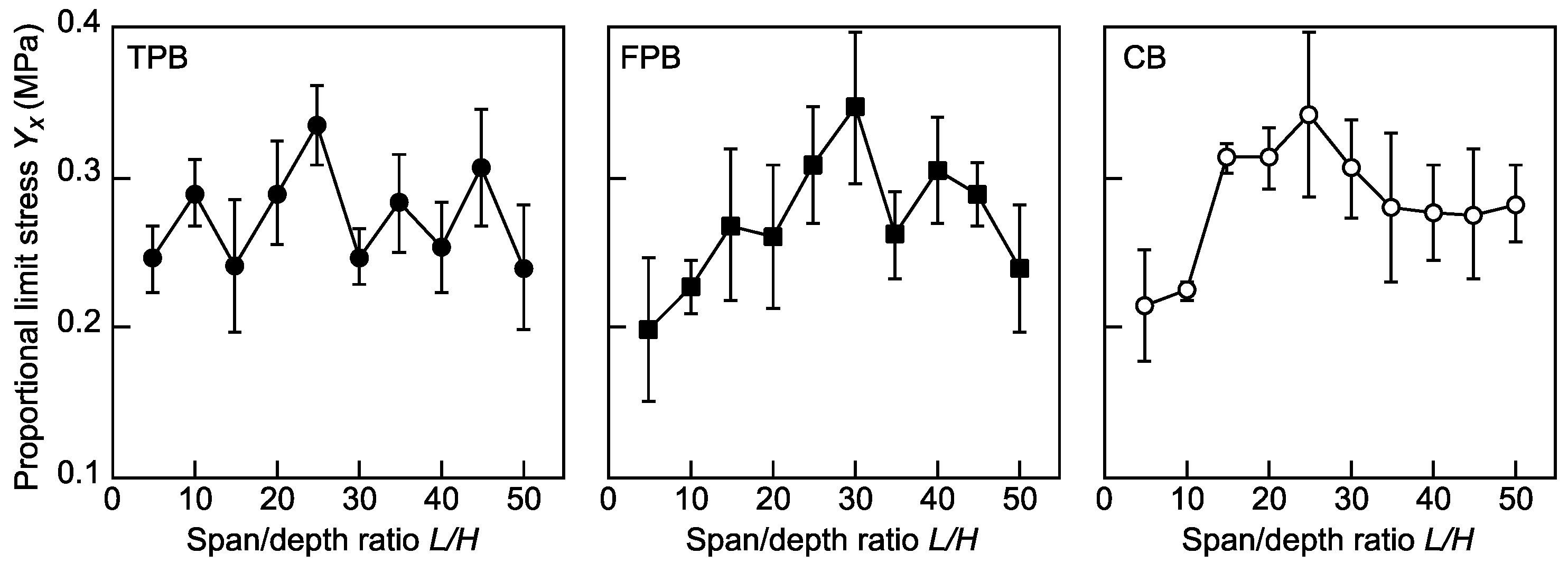
Preprints 106661 g005
Figure 5. Dependence of Yx on L/H in the TPB, FPB, and CB tests. Data = average ± standard deviations.
Figure 5. Dependence of Yx on L/H in the TPB, FPB, and CB tests. Data = average ± standard deviations.
Preprints 106661 g005
Preprints 106661 g006
Figure 6. Dependence of Fx on L/H ratio in the TPB, FPB, and CB tests. Data = average ± standard deviations. EBT = elementary beam theory, ASTM = ASTM D790-17 and ASTM D6272-17 in the TPB and FPB tests, respectively, and JIS = JIS K 7074-1988.
Figure 6. Dependence of Fx on L/H ratio in the TPB, FPB, and CB tests. Data = average ± standard deviations. EBT = elementary beam theory, ASTM = ASTM D790-17 and ASTM D6272-17 in the TPB and FPB tests, respectively, and JIS = JIS K 7074-1988.
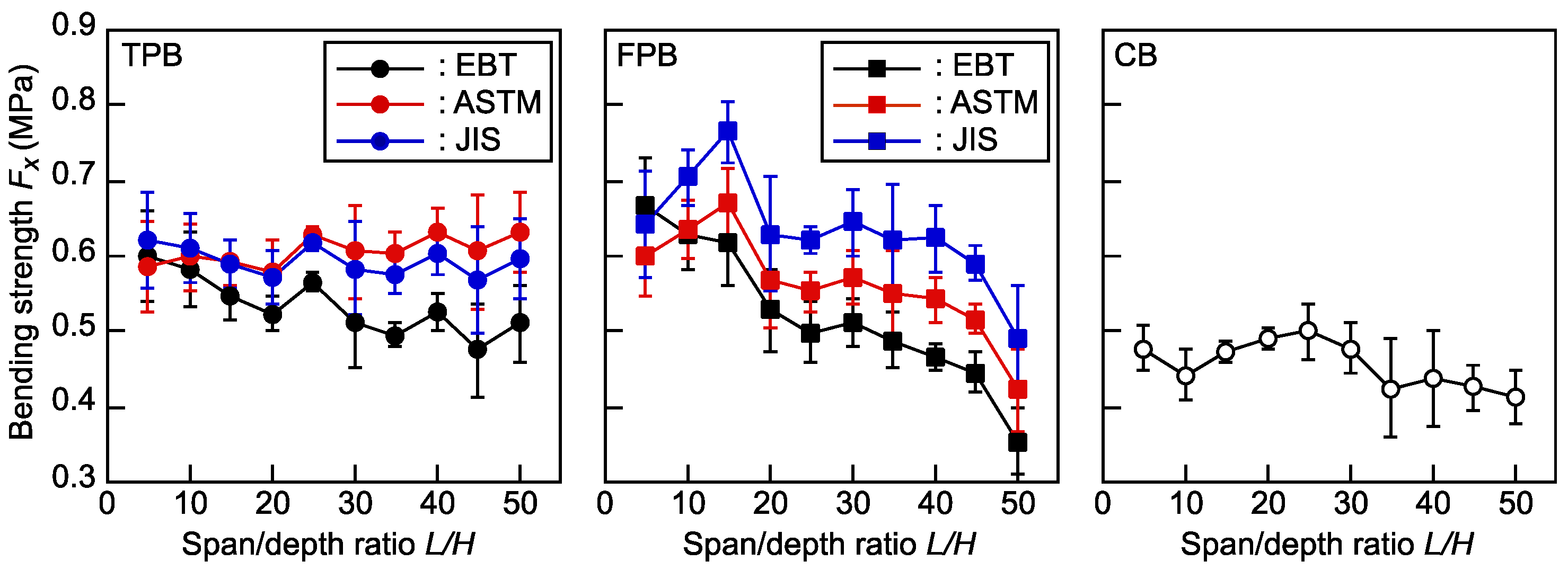
Preprints 106661 g006
Table 1. Crosshead speed in the TPB, FPB, and CB tests.
Table 1. Crosshead speed in the TPB, FPB, and CB tests.
H (mm) 20 10
L (mm) 100 200 300 400 500 300 350 400 450 500
L/H 5 10 15 20 25 30 35 40 45 50
TPB 5.0 20.0 45.0 80.0 125 90.0 123 160 203 250
FPB 6.39 25.6 57.5 102 160 115 156 205 259 319
CB 0.902 7.19 24.2 57.3 112 96.4 153 228 324 445
Unit of crosshead speed = mm/min.
Table 2. ANOVA (Tukey’s tests) results for Ex corresponding to L/H.
Table 2. ANOVA (Tukey’s tests) results for Ex corresponding to L/H.
L/H 5 10 15 20 25 30 35 40 45 50
TPB A AB AC ACD BCDE BCDE BCDE E BDE E
FPB A AB ABC B BD BD CD D CD CD
CB A B B B B AB AB B B
The significance level is higher than 0.05 among the samples with the same letters in the horizontal column. The same bold letters in the horizontal column represent the widest range of L/H where the significance level exceeds 0.05.
Table 3. ANOVA (Tukey’s tests) results for Yx corresponding to the different L/H.
Table 3. ANOVA (Tukey’s tests) results for Yx corresponding to the different L/H.
L/H 5 10 15 20 25 30 35 40 45 50
TPB A A A A A A A A A A
FPB A AB AC AD BCD CD ACD BCD ACD AD
CB A AB C C C BC AC ABC ABC ABC
The significance level is higher than 0.05 among the samples with the same letters in the horizontal column. The same bold letters in the horizontal column represent the widest range of L/H where the significance level exceeds 0.05.
Table 4. ANOVA (Tukey’s tests) results for Fx corresponding to the different L/H.
Table 4. ANOVA (Tukey’s tests) results for Fx corresponding to the different L/H.
L/H 5 10 15 20 25 30 35 40 45 50
TPB, EBT A AB ABC ABC ABC BC BC ABC C ABC
TPB, ASTM A A A A A A A A A A
TPB, JIS A A A A A A A A A A
FPB EBT A A AB BC C C C C CD D
FPB, ASTM A AB A AC AC AC BC BC CD D
FPB, JIS A AB A AC AC AC BC BC CD C
CB A A A A A A A A A A
The significance level is higher than 0.05 among the samples with the same letters in the horizontal column. The same bold letters in the horizontal column represent the widest range of L/H where the significance level exceeds 0.05. EBT = elementary beam theory, ASTM = ASTM D790-17 and ASTM D6272-17 in the TPB and FPB tests, respectively, and JIS = JIS K 7074-1988.
Table 5. Ex, Yx, and Fx in the L/H range, where the significance level exceeded 0.05.
Table 5. Ex, Yx, and Fx in the L/H range, where the significance level exceeded 0.05.
Method Ex
(MPa) 		Yx
(MPa) 		Fx EBT
(MPa) 		Fx ASTM
(MPa) 		Fx JIS
(MPa)
TPB 24.8 ± 3.5 0.273 ± 0.049 0.520 ± 0.045 0.606 ± 0.047 0.593 ± 0.046
FPB 23.9 ± 3.0 0.287 ± 0.049 0.490 ± 0.043 0.551 ± 0.042 0.602 ± 0.070
CB 18.1 ± 2.5 0.298 ± 0.040 0.457 ± 0.046 - -
Data = average ± standard deviations. EBT = elementary beam theory, ASTM = ASTM D790-17 and ASTM D6272-17 in the TPB and FPB tests, respectively, and JIS = JIS K 7074-1988.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated