Preprint
Article
Thermal Expansion of Alkaline-Earth Borates
Altmetrics
Downloads
68
Views
31
Comments
0
A peer-reviewed article of this preprint also exists.
supplementary.docx (56.14KB )
This version is not peer-reviewed
Submitted:
27 May 2024
Posted:
28 May 2024
You are already at the latest version
Alerts
Abstract
Thermal expansion of four alkaline-earth borates, namely Ca3B2O6 (0D), CaB2O4 (1D), Sr3B14O24 (2D) and CaB4O7 (3D), have been studied by in situ high-temperature powder X-ray diffraction (HTXRD). Strong anisotropy of thermal expansion is observed for the structures of Ca3B2O6 (0D) and CaB2O4 (1D) built up from BO3 triangles only: these borates exhibit the maximal expansion perpendicular to the BO3 plane, i.e. along the direction of weaker bonding in the crystal structure. Layered Sr3B14O24 (2D) and framework CaB4O7 (3D) built up from various B-O groups expand less anisotropically. Thermal properties of the studied compounds compared to the other alka-line-earth borates are summarized depending on the selected structural characteristics like anion dimensionality, residual charge per one polyhedron (BO3 BO4), cationic size, and structural com-plexity. For the first time these dependencies were established as an average for both types of polyhedra (triangle and tetrahedron) occurring in the same structure at the same time. The most common trends were identified for : (i) Melting temperature decreases as the dimensionality of the borate polyanion, and more precisely, as the residual charge per one polyhedron (triangle or tetrahedron) decreases; (ii) volumetric expansion decreases while the degree of anisotropy in-creases weakly when the residual charge decreases; (iii)both trends (i) and (ii) are most steady within borates built by the triangles only, while the borates built by both triangles and tetrahedra show a larger scatter in values.
Keywords:
Subject: Chemistry and Materials Science - Physical Chemistry
1. Introduction
Over the past few decades, newly discovered borates have been the focus of research due to their diversity and well known applications [1,2,3,4,5,6]. The crystal chemistry of borates is diverse because boron atom orbitals can hybridized to either sp2 or sp3 configuration forming BO3 or BO4-polyhedra in the same crystal structure. Recently it has been shown [6] that in the case of sp-hybridization, boron can occur also in double coordination by oxygen although this configuration is quite rare. Typically, BO3 and BO4 polyhedra are connected through vertices to form more complicated borate anion. Additionally, the structural diversity of borate crystal chemistry is supplemented by the edge-sharing BO4 tetrahedral units formed in high pressure/high temperature borates [7,8]. Recently edge-sharing borate groups were obtained in borates at normal pressure as well [9,10]. At present, borate systems have been considered to be the most promising optical materials in the UV range nonlinear optical (NLO) materials or host phases for luminophores. The wide practical application is due to the unique properties of borates, in particular, a wide transparency range, outstanding NLO, luminescent, piezoelectric, relatively high resistance against laser-induced damage, etc [5,11,12]. In recent years, one more modern application of the materials with near zero or negative thermal expansion was suggested for using them as a basis of phosphors without thermal quenching or with anti-thermal quenching of luminescence [13,14,15,16,17].
Due to the increasing application of borates in optics, the thermal behavior of these materials is currently being intensively investigated. About 20 years ago high-temperature crystal chemistry of borates had been mainly developed by our team [18,19,20,21,22]. It was shown that borates expand sharply anisotropically up to negative areas [18,23]. For the last decade, an overall interest in thermal expansion studies increased sharply [24,25,26,27,28].
Herein we summarize data on thermal expansion of alkaline earth borates. Some phases of the same stoichiometry like 3MO:1B2O3, 2:1, 1:1, 1:2, 1:3, 1:4 are common to the most systems MO–B2O3 (M = Mg, Ca, Sr, Ba). Since the atomic radii of Ca and Sr are close to each other (Rcryst = 1.26 (Ca) and 1.4 Å (Sr) for CN = 8 [29]), isotypical phases are encountered. Meanwhile the radii of magnesium (Rcryst = 0.86 Å for CN 6) and barium (Rcryst = 1.61 Å, CN 9), differ substantially.
Among Ca borates te following compounds have been structurally characterized: Ca3B2O6 (CaO : B2O3 = 3:1) [30,31,32,33], γ-Ca2B2O5 (2:1) [34,35], α-Ca2B2O5 (2:1), CaB2O4 (1:1) [36,37,38,39,40,41,42], Ca2B6O11 (2:3) [43], CaB4O7 (1:2) [44,45,46], and CaB6O10 (1:3) [49]. Interesting sequence of reversible phase transitions was first described for Sr2B2O5 from thetrmal analysis data [48]. Crystal structures of Sr2B2O5 polymorphs resulting from the γ`↔β↔α’↔α reversible first-order phase transitions were studied by high-temperature single-crystal X-ray diffraction, high-temperature X-ray powder diffraction, differential scanning calorimetry (DSC) and impedance spectroscopy [49]. Similar sequence of reversible first-order phase transitions (γ ↔ β ↔ α) was found for Ca2B2O5 [50]: the γ-Ca2B2O5 low-temperature polymorph exists up to 520 ° С, the β-Ca2B2O5 intermediate modification – in the range 520-580 ° С and the high-temperature modification α-Ca2B2O5exists above 580 °С as. All Ca2B2O5 polymorphs are structurally similar to those of Sr2B2O5.
By doping calcium borates with various lanthanides (Ln), prospective luminescent properties were obtained. The thermoluminescent properties CaB4O7 doped with a number of lanthanide ions (Ce3+, Sm3+, Eu3+, Dy3+ and Yb3+) were studied in [51,52,53]. Ca3(BO3)2:Eu3+ [54] and Ca2B2O5:REE (REE = Eu3+, Tb3+, Dy3+) [55] exhibited high photoluminescence intensity.
Thermal expansion of Mg- [56], Sr- [49,57], Ba- [58,59] and (Sr,Ba)- [59] borates has been investigated, while that of Ca-borates has not been practically studied up to now. The exception was the thermal expansion of γ- and α-Ca2B2O5 modifications: polymorphic transitions of Ca2B2O5, and the crystal structure of the high-temperature polymorph was determined from powder HTXRD data at 600 °С [50]. Therefore, one of the goals of this work was to study the thermal expansion of Ca-borates and the unique triple-layered Sr-borate solved recently [60].
The present paper is intended to clarify how borate structure affects its thermal expansion using an example of alkaline earth borates. For this purpose, our data on thermal expansion of four alkaline earth borates namely Ca3B2O6 (0D), CaB2O4 (1D), Sr3B14O24 (2D) and CaB4O7 (3D), are supplemented by the literature data and summarized. The main values of the thermal expansion tensor and its orientation relatively crystallographic axes are reported. The parameters of thermal expansion of Ca-borates are compared with those of other alkaline earth metals (Mg, Sr, Ba) borates to reveal the common trends for thermal expansion anisotropy of alkaline earth borates. The volume thermal expansion depending on the B2O3 content, as well as on the cation radius and structural complexity is considered.
2. Materials and Methods
2.1. Synthesis
Polycrystalline samples of borates Ca3B2O6, CaB2O4, CaB4O7 were obtained by solid-state reactions in air from a mixture of high purity Н3ВО3 and pre-dried СaCO3 (600 °C, 3 h). The reagent mixtures were grounded in an agate mortar and pressed into tablets. Synthesis was done out in air in platinum crucibles at 500–1400 °C. Thermal treatment time varied from 3 to 22 h. Synthesis of Ca3B2O6, CaB2O4 and CaB4O7 was done under repeated thermal treatment with a sequential increase in temperature to 1450, 1200, 950 °C, respectively.
Sr3B14O24 powder samples was synthesized by glass crystallization. Preliminary calcinated at 600 °C stoichiometric mixture of SrCO3 and high purity H3BO3 was kept at 1250˚C for 40 minutes. The resulting melt was then poured onto a metal substrate. Cooled glassy sample without grinding was placed into a furnace at 780˚С for 30 minutes for crystallization.
2.2. Methods
2.2.1. X-ray Diffraction
Phase composition of polycrystalline samples was studied at room temperature in air in the range of 5–60 °2θ using a Rigaku MiniFlex II (CuKα1+2 radiation, 30 kV / 10 mA, Bragg-Brentano geometry) X-ray diffractometer.
2.2.2. Powder High-Temperature X-ray Diffraction
The thermal expansion of Ca3B2O6, CaB2O4 and CaB4O7 was studied in air by high-temperature X-ray powder diffraction (HTXRD) data collected using a Rigaku Ultima IV powder X-ray diffractometer (CuKα / CoKα radiation, 40 kV/30 mA, Bragg–Brentano geometry, PSD D-Tex Ultra) equipped with a high-temperature camera. The samples were prepared from a heptane suspension on a Pt–Rh plate [61]. Commonly, temperature steps of 20–40 °C were adopted.
The procedure for tensor calculations using the ThetaToTensor [62,63] and RietveldToTensor software [64,65] is as follows: (i) unit-cell parameters at every temperature step were refined by least-squares or Rietveld method; (ii) the temperature dependencies of the unit-cell parameters were usually approximated by linear and quadratic polynomial functions; (iii) using the approximation coefficients, the eigenvalues of a thermal expansion tensor and the orientation of its principal axes with respect to the crystallographic axdirectiones were determined (iv) the three-dimensional surface and the main sections of the figure of thermal expansion tensor were drawn. Each radius vector of this figure represents the value of thermal expansion coefficient (TEC)αin this direction.
2.2.3. Structural Complexity Calculation
The structural complexity calculations provide theoretical estimation of the configurational contributions to the total entropies of crystalline substances [66]. The structural complexity parameters have been calculated using the TOPOS program package [67]. The formalism of calculations is presented in [68,69].
3. Results
3.1. Thermal Expansion of Ca-and Sr-Borates vs Dimensionality of Borate Anion
Here we present data on thermal expansion of four Ca-and Sr-borates, in which the dimensionality of borate anion increases from isolated (0D) groups via chain (1D) and layered (2D) structures up to the framework (3D), Studied borates crystallize with various symmetry Ca3B2O6 (0D) borate with isolated BO3 triangles is trigonal, CaB2O4 (1D) borate with chains of triangles is orthorhombic, layered Sr3B14O24 (2D) and framework CaB4O7 (3D) borates are monoclinic. Temperature dependencies of the unit-cell parameters and volume of these borates (Figure 1) were approximated by linear or quadratic polynomial functions (Table S1). Calculated TECs, particularly thermal expansion tensor eigenvalues (α11, α22, α33) and their orientation in respect to the crystallographic axes as well as the TECs along a, b, c (αa, αb, αc) are given in Table S2. Due to the symmetry in the case of trigonal crystals, the expansion in the ab plane is isotropic α11 = αa = α22 = αb (Table S2) whereas α33 = αc differs from α11. When the symmetry is reduced to monoclinic, the orientation of the α11 and α33 tensor axes is determined by the μc,3 and μa,1 angles in the monoclinic ac plane (Table S2): where μc,3 = (c∧α33) is the angle between c axis and α33 tensor axis and μa,1 = (a∧α11) is the angle between a and α11 (Table S2). Principal axes of thermal expansion tensor are oriented relative to the crystallographic axes using software TTT and RTT [63,65]. With temperature increase the values of αV rise for each of four borates and the degree of anisotropy decreases for all borates except Ca3B2O6. Both tendencies are usual due to an increase in the thermal motion of atoms. Estimation of the degree of anisotropy of thermal expansion, its interpretation and possible structural reasons for Ca- and Sr-borates as well as other alkaline-earth borates are summarized below (pp. 3.2–3.3).
3.2. Thermal Expansion of Alkaline Earth Borates
Selected characteristics of thermal expansion like eigenvalues of tensor, a11, a22, a33, volume TECs (aV = α11 + α22 + α33), the anisotropy parameters for 20 alkaline-earth borates are summarized in Table 1. Thermal expansion of these borates has been examined by high-temperature X-ray powder diffraction before [4,49,50,56,57,58,59,67,70] and supplemented by the data from present work. Here, except for the formula of the compound, its symmetry (system and space group) and the reference of the source, the volume expansion coefficient equal to sum, degree of anisotropy thermal expansion are given. The figure of the thermal expansion coefficients is represented as 3D and its 2D sections images (see Figure 5).
Due to the anisometry and symmetry of the crystals, it is obvious that the thermal expansion is different in various directions in the crystal with the exception of cubic crystals, which expand isotropically. To estimate the maximum degree of anisotropy in a plane in a structure, we should choose the plane of maximal anisotropy. Apparently, for the first time, the degree of anisotropy in a plane was descibed quantitatively as ∆plane = ∑ (αmax – αmin) / (αmax + αmin) = ∑ (αmax – αmin) / 2αav in [71,72]. Here we introduce a new volume criteria for anisotropy of thermal expansion:
∆V = ∑ |αii – αjj| / αav. = (|α11 – α22| + |α11 – α33| + |α22 – α33|) / 3αV, where αV = α11 + α22 + α33.
In the case of isotropic thermal expansion in a plane and in a volume, both ∆max and ∆V are equal to zero. As the difference in the eigenvalues of tensor, αii – αjj, increases, the degree of anisotropy ∆plane and ∆V rises as well.
Table 1.
Main characteristics of the thermal expansion of alkaline-earth borates.
| Chemical Formulae | System, space group | nΔ:m□ ratio* | T, °C | α × 106 °С−1 | ∆plane | ∆V | Refs | |||||||
| α11 | α22 | α33 | αV | |||||||||||
| Isolated BO-groups (0D) | ||||||||||||||
| Isolated BO3 (−3)** | ||||||||||||||
| Mg3B2O6 | Orth., Pnmn | 2Δ | 25 | 13 | 9 | 8 | 30 | 0.24 | 0.33 | [56] | ||||
| 1100 | 20 | 13 | 13 | 46 | 0.21 | 0.30 | ||||||||
| Ca3B2O6 | Trigon., R-3c | 2Δ |
25 | 8 | 8 | 27 | 43 | 0.54 | 0.88 | *** | ||||
| 900 | 9 | 9 | 38 | 57 | 0.62 | 1.04 | ||||||||
| Sr3B2O6 | Trigon., R-3c | 2Δ | 25 | 5 | 5 | 34 | 45 | 0.74 | 1.32 | [67] | ||||
| 900 | 5 | 5 | 39 | 48 | 0.77 | 1.39 | ||||||||
| Ba3Sr3B4O12 | Tetragon., I4/mcm | 4Δ | 100 | 12 | =α11 | 18 | 43 | 0.20 | 0.29 | [59] | ||||
| 800 | 17 | 17 | 29 | 63 | 0.26 | 0.38 | ||||||||
| Average | 〈47〉4 | 0.45 | 0.74 | |||||||||||
| Isolated mixed BO3 and B2O5 pyrogroups (−2.5) | ||||||||||||||
| Ba5B4O11 | Orth., | 12Δ | 100 | 10 | 15 | 13 | 38 | 0.20 | 0.26 | [59] | ||||
| P212121 | 800 | 15 | 13 | 27 | 55 | 0.35 | 0.51 | |||||||
| Ba2Sr3B4O11 | Monocl., | 5Δ | 100 | 3 | 6 | 34 | 43 | 0.84 | 1.44 | [59] | ||||
| C2/c | 800 | 5 | 6 | 51 | 62 | 0.82 | 1.48 | |||||||
| Average | 〈49.5〉2 | 0.55 | 0.92 | |||||||||||
| Isolated B2O5 pyrogroups (−2) | ||||||||||||||
| γ-Ca2B2O5 | Monocl., P21/c | 2Δ | 25 | 19 | 7 | -1 | 25 | 1.11 | 1.6 | [50] | ||||
| 500 | 27 | 7 | 3 | 37 | 0.80 | 1.3 | ||||||||
| α-Ca2B2O5 | Monocl., | 2Δ | 600 | 31 | 7 | -5 | 33 | 1.38 | 2.18 | [50] | ||||
| P21/c | 900 | 33 | 7 | 2 | 42 | 0.89 | 1.48 | |||||||
| γ-Sr2B2O5 | Monocl., P21/c | 2Δ | 25 | 20 | 7 | 1 | 28 | 0.90 | 1.36 | [49] [49] |
||||
| α-Sr2B2O5 | Monocl., P21/c | 2Δ | 828 | 32 | 3 | 4 | 39 | 0.83 | 1.49 | |||||
| Average | 〈34〉4 | 0.96 | 1.5 | |||||||||||
| Isolated cyclic (triborate) groups from BO3 (−1) | ||||||||||||||
| α-BaB2O4 | Hex., R-3c | 3Δ | 20-700 | 6 | 6 | 28 | 40 | 0.65 | 1.1 | [4] | ||||
| β-BaB2O4 | Trigon., R3c | 6Δ | 20-700 | 3 | 3 | 45 | 51 | 0.88 | 1.65 | [58] | ||||
| Average | 〈46〉2 | 0.77 | 1.37 | |||||||||||
| 1D Borates (−1) | ||||||||||||||
| CaB2O4 | Orth., Pnca | 1Δ | 25 | 22 | 6 | 1 | 28 | 0.91 | 1.45 | ** | ||||
| 900 | 33 | 6 | 6 | 45 | 0.69 | 1.2 | ||||||||
| SrB2O4 | Orth., Pbcn | 1Δ | 25 | 4 | 4 | 32 | 39 | 0.78 | 1.4 | [57] | ||||
| 900 | 4 | 4 | 35 | 43 | 0.79 | 1.44 | ||||||||
| Average | 〈39〉2 | 0.79 | 1.37 | |||||||||||
| Layered 2D-Borates (−0.43) | ||||||||||||||
| Sr3B14O24 | Monocl., | 8Δ:6□ | 30 | 2 | 11 | 14 | 27 | 0.75 | 0.8 | ** | ||||
| P21/c | 800 | 11 | 10 | 13 | 33 | 0.12 | 0.2 | |||||||
| Average | 〈30〉 | 0.44 | 0.57 | |||||||||||
| 3D-Borates | ||||||||||||||
| −0.5 | ||||||||||||||
| SrB4O7 | Orth., Pmn21 | 4□ | 25-900 | 7 | 9 | 8 | 24 | 0.13 | 0.17 | [57] | ||||
| α-CaB4O7 | Monocl., P21/n | 4Δ:4□ | 25 | 8 | 8 | 3 | 19 | 0.45 | 0.53 | ** | ||||
| 900 | 13 | 9 | 6 | 28 | 0.37 | 0.5 | ||||||||
| BaB4O7 | Monocl., P21/c | 4Δ:4□ | 20-700 | 23 | -12 | 5 | 16 | 3.18 | 4.38 | [58] | ||||
| Average | 〈21〉3 | 1.24 | 1.69 | |||||||||||
| −0.25 | ||||||||||||||
| SrB8O13 | Monocl., P21/c | 12Δ:4□ | 25 | 21 | 9.6 | 3.9 | 34 | 0.69 | 1.00 | [57] | ||||
| 740 | 20 | 9.6 | 7.3 | 37 | 0.47 | 0.69 | ||||||||
| LT-BaB8O13 | Orth., P22121 | 12Δ:4□ | 100-400 | 6.9 | 11 | -0.5 | 17 | 1.1 | 1.32 | [70] | ||||
| 500 | 9.4 | 11.5 | -1.7 | 19.1 | 1.35 | 1.38 | ||||||||
| Average | 〈27〉2 | 0.9 | 1.09 | |||||||||||
* – Amount triangles and tetrahedra in nΔ:m□ ratio is taken from FBB; ** – Residual charge per a polyhedron (BO3 / BO4); *** – Present work.
3.2.1. Anisotropy of Thermal Expansion vs Dimensionality of Borate Anion
Anion dimensionality (0D, 1D, 2D, 3D). The borates in the MO―B2O3 systems where M is an alkaline-earth metal were assigned to the groups according to the dimensionality of B―O–polyanion (Table 1): zero dimensional (0D), chain or one-dimensional (1D), layered or two-dimensional (2D) and framework or three-dimensional (3D). OD-borates, additionally can be divided into subgroups as the polymerisation degree of BO3 and BO4 polyhedra increases in B-O polyanion (Table 1). The dimensionality of BO-polyanion or degree of polymerization depends highly on chemical composition [18]: it increases as the boron oxide content increases. The reason of this trend is evident: the higher the degree of polymerization, the lower the residual charge per a boron-oxygen coordination polyhedron Z [72].
Degree of polymerization / residual charge per an averaged boron-oxygen polyhedron Z. In the 0D borates of MO―B2O3 systems (0–50 mol. % B2O3) the degree of polymerization increases gradually from isolated single triangle (BO3) – simple group with the greatest residual charge is −3, through isolated mixed anion (BO3 + B2O5)– a triangle and a group of two triangles condensed by an oxygen atom in ratio 2:1 (−2.5), group (B2O5) of two condensed triangles (−2), to B3O6 cyclic (triborate) group of three triangles (−1). It is noteworthy that these isolated anion groups are built up from boron atoms in the triangular coordination only. Chain 1-D borates (1:1 stoichiometry, 50 mol. % B2O3) are composed by boron triangles only as well. With further anion polymerization increasing (or Z residual charge per a polyhedron decreasing) BO4 tetrahedra appear in borate anions forming 3D-borates with 1:2 stoichiometry (66.7 mol. % B2O3) such as MgB4O7 (# 34397-ICSD), CaB4O7 [44,45,46], SrB4O7 [57] and BaB4O7 [58] those crystal structures are built up by both triangles and tetrahedra except SrB4O7 structure composed by tetrahedra only. Among anhydrous borates of alkaline earth metals there exists only Sr3B14O24 layered borate with 3:7 stoichiometry (70 mol. % B2O3) [60] which is located within compositional range of 3D-borates that is not normal but not unusual. Similar situation was observed in the alkali borate systems, where 2D-borates are located within “3D-borates range” of compositions: for instance, α-Na2B4O7 (# 2040-ICSD), Rb3B7O12 (# 76344-ICSD), Cs3B7O12 (# 98582-ICSD), Cs3B13O21 (# 95728-ICSD) etc. It should be noted that the listed 2D-borates can be considered as pseudo-framework; their layer thickness is usually large, the anion consists of several B-O groups, all oxygen atoms are bridging. This somewhat contradicts the general principle of dimensional reduction [73], according to which, the increasing content of the ’ionic’ MO component in the systems is associated with gradual decreasing dimensionality of anions. The fenomena may be due to so-called “boron anomaly” found for predominantly anhydrous alkali and alkaline earth borate crystals and glasses by J. Krogh-Moe in 1960s as described by Wright [74,75]. It was stated that the number of tetrahedrally coordinated boron atoms increases as non-boron metal content increases up to MO : 2B2O3, the greatest amount of tetrahedra (2□ : 2∆ = 1) being observed at MO : 2B2O3 oxide ratio. Then the number of tetrahedra decreases as MO content increases further. As seen from the Table 1 within compositional ranges of 0D-1D-borates (B2O3 content ≤ 50 mol. %), borate anions are built up from triangles only, tetrahedra appear in 2D- and 3D-borates (B2O3 content > 50 mol. %).
Anisotropy of thermal expansion. Borates often exhibit sharply anisotropic thermal expansion [19,23]. This anisotropy is primarily ruled by the distribution of the sharply anisotropic thermal oscillations of atoms. In the BO3 triangles, dimers or cyclic triborate groups, comprising triangles only, the maximal exes of ellipsoids of thermal displacements of atoms and the maximal thermal expansion of structure occur perpendicular to the plane of a triangle or a ring, whereas the minimal expansion is parallel to the BO3 plane [23].
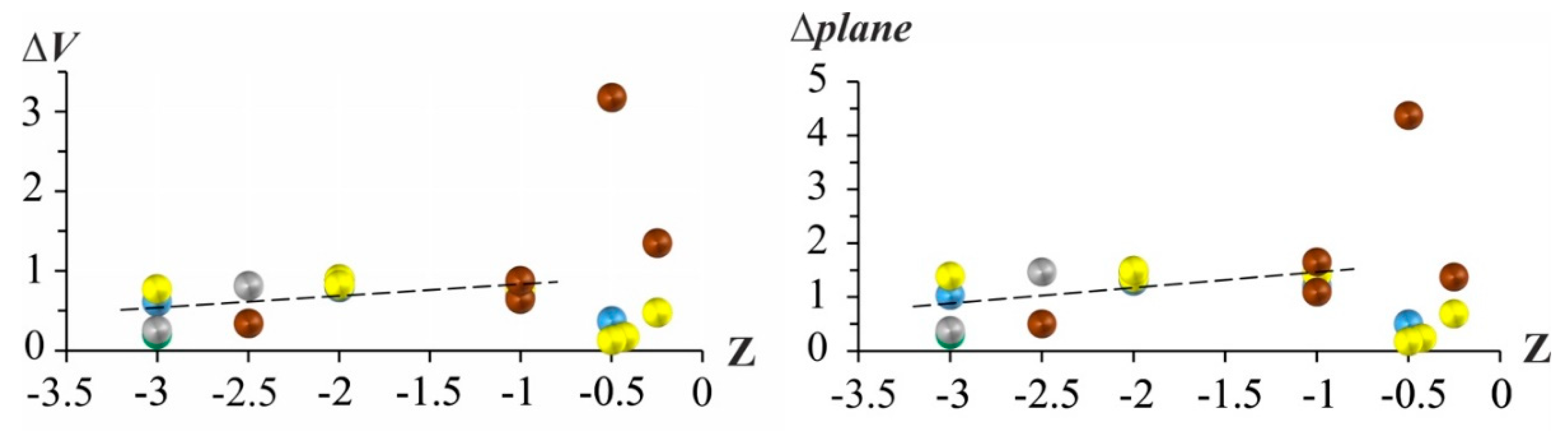
Strong bonds inside boron-oxygen groups and the ability of these groups to rotate with respect to each other around the common oxygen atoms ensure the plastic thermal behavior of borate crystals. As a result, most borates demonstrate greatly anisotropic thermal expansion up to negative values along certain directions. Here the steady high expansion anisotropy estimated as ∆plane and ∆V was observed for M2B2O5 (M = Ca, Sr, Ba) (0D), and MB2O4 (M = Ca, Sr, Ba) (OD and 1D) based BO3 triangles only. Their structures exhibit maximal expansion strongly perpendicular to the BO3 planes, i.e., along the direction of weak bonds in the crystal structure. Figure 2 showed that for the alkaline-earth borates built by BO3 triangles, anisotropy increases slightly but steady as residual charge per the anionic polyhedron [BO3]3− decreases down to Z = −1. Further decreasing the residual charge per the anionic polyhedron ([BO3]3− and [BO4]5−) resulted in a larger scatter of ∆plane and ∆V values (Figure 2).
3.2.2. Thermal Expansion as Function of Cationic and Anionic Properties
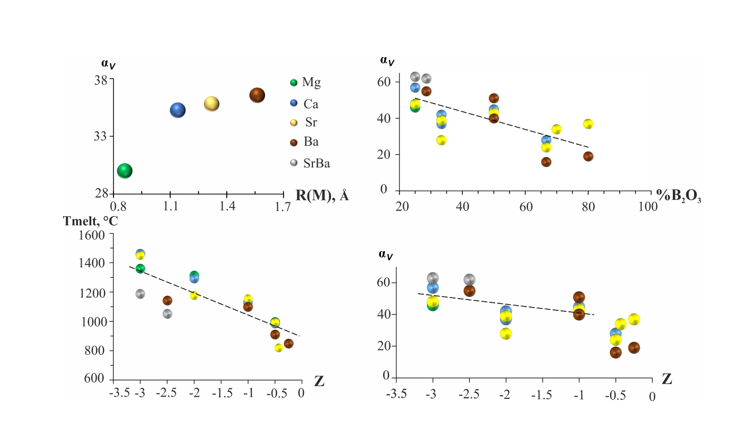
Thermal vibrations of M atoms play an important role in thermal expansion. The dependence of the average volume thermal expansion (αV) on the radius [29] of the alkaline earth metal cation is shown in Figure 3. The borates with relatively small cations exhibit the minimum thermal expansion: the mean values are from αV = 30 for Mg to 36×10−6 C−1 for Ba. An increase of αVis observed with an increase of cationic radius (Figure 3). This trend is caused by the increasing both the average M-O bonds and the coordination number (CN) in MOx polyhedron from M = Mg (x=6) to Ba (x=8-9). Earlier the similar trend was manifested for alkali metals borates [23].
The dependence of αV on the B2O3 content is shown in Figure 3b. With an increase of B2O3 content in the MO–B2O3 systems (M = Mg, Ca, Sr, Ba), the number of triangles and / or tetrahedra in the structure increases, which leads to their condensation and a decrease in the number of oxygen bonds with metals, i.e., further weakening the weakest bonds and lowering the strength properties of the compounds. There is a tendency for volumetric expansion to decrease as a result of an increase of the polymerization degree. The average volume expansion gradually decreases with a decrease in the residual charge by one polyhedron (Table 1, Figure 3).
With a decrease in the strength of the joint, its melting point and hardness, compressibility, solubility, etc., decrease. The correlations between the melting point, the volume thermal expansion, and the residual charge of borates of alkaline earth metals are shown in Figure 3c and d. Analysis of the data for borates of alkaline earth metals with triangular and tetrahedral radicals showed that there is a clear tendency for the melting temperature to decrease as the residual charge of the radical decreases. At the equal values of Z, the melting point is practically independent of the type of the cation, structure and degree of polymerization of radical (isolated cyclic groups of three triangles and chains of triangles, frameworks containing various borate groups). The volumetric thermal expansion decreases slightly also with a decrease in the residual charge per a polyhedron. If we examine the effect of the residual charge on the volumetric expansion for borates built only from triangles only, we can see that the volumetric expansion decreases very slightly, it is almost constant (Figure 3).
Figure 3.
Dependence of average volume TEC (αV) of alkaline-earth borates on cation radius R, αV vs B2O3 content, the melting point and αV vs the residual charge of the polyanion calculated per polyhedron.
Figure 3.
Dependence of average volume TEC (αV) of alkaline-earth borates on cation radius R, αV vs B2O3 content, the melting point and αV vs the residual charge of the polyanion calculated per polyhedron.
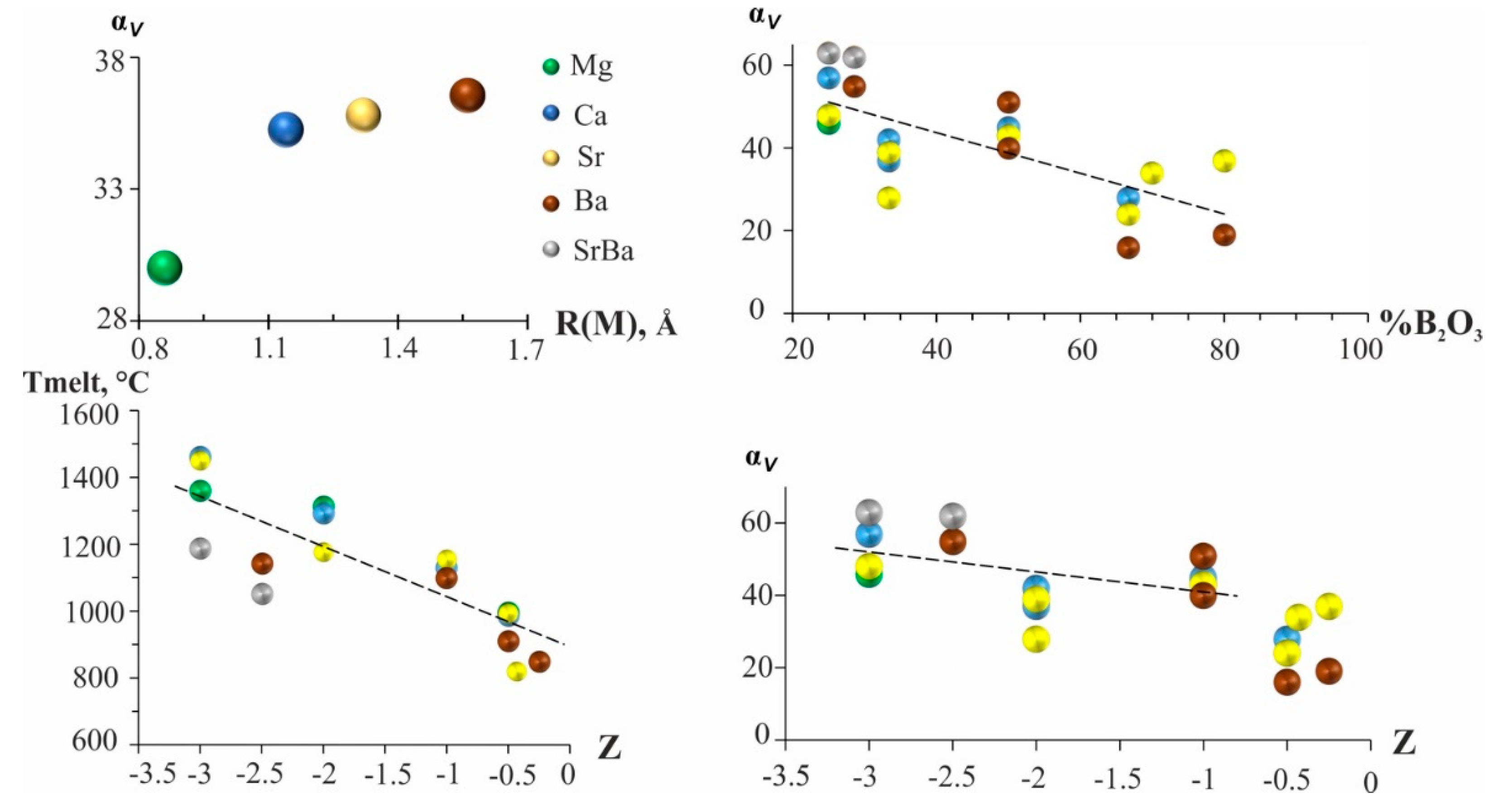
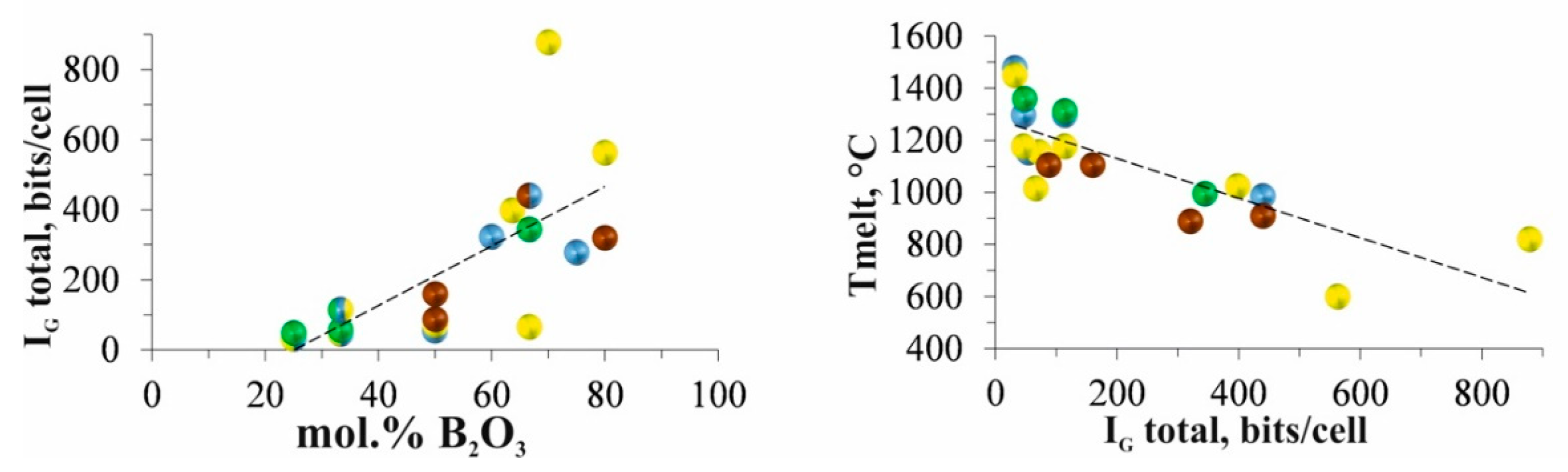
An effective concept for assessing complexity is Shannon's information theory [76] modified by S.V. Krivovichev for crystal structures [66,68,69]. According to the complexity classification of crystal structures, most compounds of the MO – B2O3 systems are either simple (20–100 bits/cell) or intermediate (100–500 bits/cell). The structure of Ba5(BO3)2(B2O5) is very complex (1417.65 bits / cell) (This point is not represented on the graphs due to the large difference in values). Such a sharp increase in structural complexity of this barium borate is associated with content of two types of anionic groups (isolated BO3 triangles and B2O5 diborate groups). In general, with an increase in the B2O3 content in the MO – B2O3 systems (M = Mg, Ca, Sr, Ba), the complexity of the borates also increases (Figure 4).
The correlation between melting point and structural complexity of borates is shown in Figure 4. The observed decrease in the melting temperature of alkaline earth metal borates with increasing structural complexity shows that both properties are a function of the polymerization of boron-oxygen radicals or residual charge per a polyhedron. The reason is evident – chemical compounds with greater complexity melt or decompose at lower temperatures.
Figure 4.
Dependences of the of the structural complexity parameter on the borate composition and melting point of borates on the structural complexity parameter IG total (bits/cell).
Figure 4.
Dependences of the of the structural complexity parameter on the borate composition and melting point of borates on the structural complexity parameter IG total (bits/cell).
3.3. Structural Interpretation of Thermal Expansion of Borates Earth-Alkaline Metals
As was shown before, when a group of atoms have bonds as strong as in BO3, the entire group may produce oscillations called as rigid-body motion. Apparently, thermal vibrations of a group of atoms were first described by Cruickshank [77] and much later these studies were generalized by Downs [78]. Thermal vibrations of any atom in a group are dictated by the common motion of the group. In the case of a BO3 triangle, oxygen and B atoms oscillate mainly perpendicular to the strong B–O bonds [23,79]. There are three B–O bonds in the triangle plane, thus both B and O atoms vibrate maximally approximately perpendicular to the plane of BO3 triangles. Consequently, a triangle as a whole has to vibrate in the same direction, i.e., nearly perpendicular to the triangle plane. If a structure is based on isolated triangles in a preferred nearly parallel orientation, the highly anisotropic thermal expansion is usually observed. In the case of a BO4 tetrahedron, B and O atoms also vibrate practically perpendicular to the strong B–O bonds. However, B-O bonds of BO4 tetrahedron are isometrically distributed in 3D-space. Thus, BO4 tetrahedron itself oscillates randomly and its thermal motion does not affect significantly to anisotropy of thermal expansion of the structure.
Borate rigid group in addition to the simple polyhedra include diborate (2B) and cyclic triborate (3B), tetraborate (4B), pentaborate (5B) groups, etc. [19,79]. These more complex units retain their configuration unchanged and can oscillate or rotate as a whole. Among alkaline-earth borates there are 0D-borates based on isolated triangles (M3B2O6), 0D-borates based on pyroborate groups of two or three cornersharing triangles (М2B2O5 and MB2O4), 1D-borates built up from the chains of triangles (MB2O4) as well 2D- and 3D-borates based on various rigid groups. The approach based on thermal vibrations of rigid groups [19,20,79] was applied below to explain the dramatic anisotropy of many borates.
Borates Based on Isolated Groups of BO3 Triangles
M3B2O6 (M = Mg, Ca, Sr, (Sr,Ba)). (0D). Four phases occur in borates of this stoichiometry which contain isolated BO3 triangles. As size of cation and its CN increase from Mg (C.N. 6, R = 0.86 Å) via Ca (C.N. 8, Rcr = 1.26 Å) and Sr (C.N. 8, Rcr = 1.4 Å) to (Sr, Ba) (C.N. 6–11, Rcr = 1.49–1.54 Å): crystal structures of Mg-, Ca- and Sr-borates of this stoichiometry are similar moreover Ca- and Sr-borates are isotypical while Ba3Sr3[BO3]4 is related to “anti-zeolite” family where isolated BO3 triangles orientationally disordered over the 4 and 8 orientations as a result of splitting of O sites.
Mg3B2O6 (ICSD–31385), 1B : 1Δ : Δ [80]. In the orthorhombic Mg3B2O6 the planes of the isolated BO3 triangles are not parallel to each other. As result it expands weaker in comparison to the other members of the group and its anisotropy is insignificant (Table 1). It is caused obviously by short and strong Mg–O bonds in MgO6 polyhedra [56].
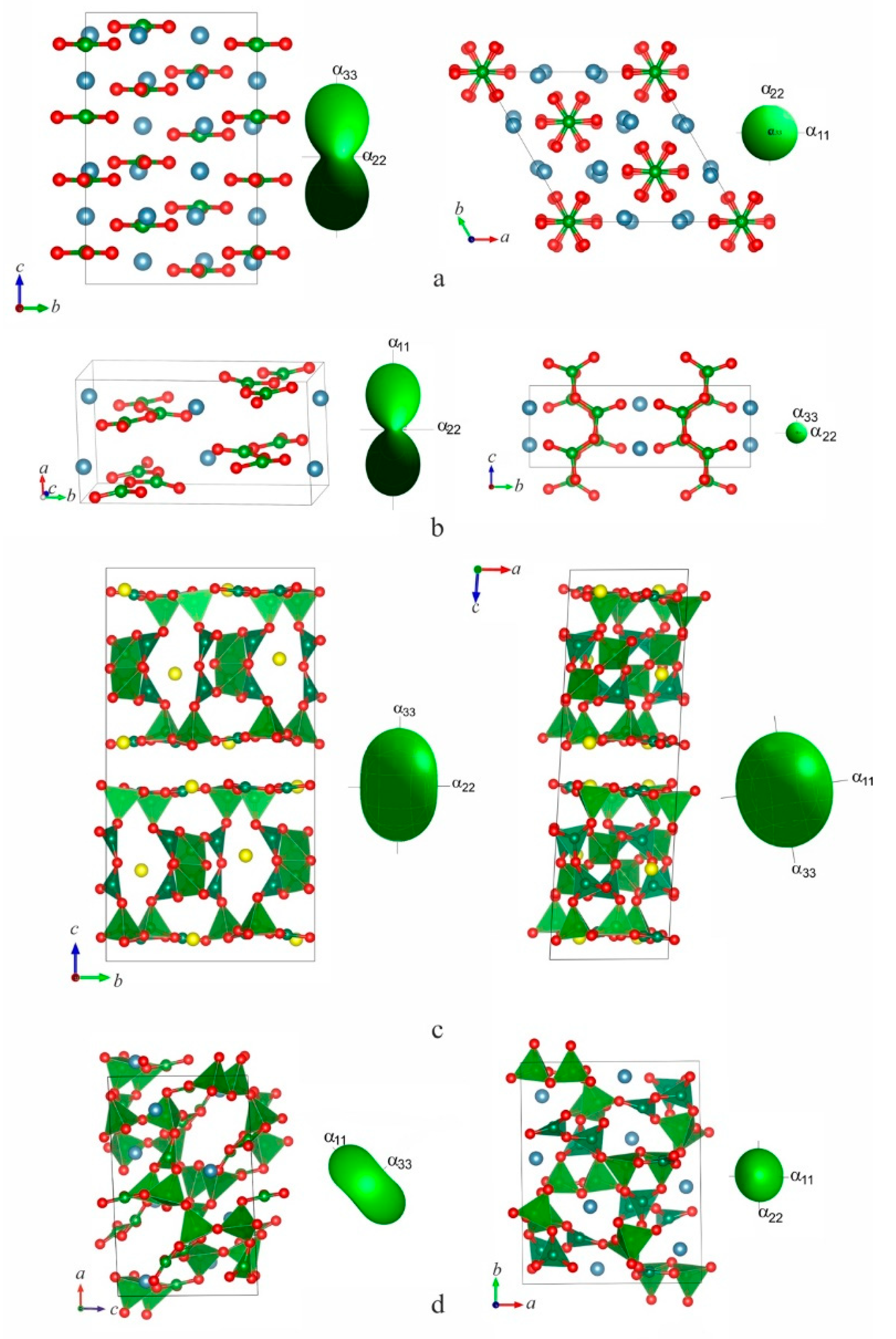
Ca3B2O6 (ICSD–1894) and Sr3B2O6 (ICSD–93395), 1B : 1Δ : Δ,. In these isotypical trigonal structures, Ca3B2O6, and Sr3B2O6, isolated triangles BO3 are arranged perpendicular to the c axis (Figure 5). For this reason, a high expansion anisotropy is observed: both structures expand strongly perpendicular to the planes of the BO3 triangles (Table 1). The values of TECs are quite close (αV = 53 and 57 × 10-6 С-1 for Ca and Sr, respectively) due to the structural similarity.
Figure 5.
Crystal structures of Ca3B2O6 (a), CaB2O4 (b) Sr3B14O24 (c) and α-CaB4O7 (d) in comparison to pole figures.
Figure 5.
Crystal structures of Ca3B2O6 (a), CaB2O4 (b) Sr3B14O24 (c) and α-CaB4O7 (d) in comparison to pole figures.
Ba3Sr3(BO3)4, 4B : 4Δ : Δ,Δ,Δ,Δ, [59]. It is a member of the “anti-zeolite” borate family, and structurally close to the Ba3B2O6 borate. Oxygen atoms bonded to B atoms are split therefore the BO3 triangles orientationally disordered over the 4 and 8 orientations and triangles are oriented in a non-parallel manner. In this case it is expected that anisotropy will be weak. As expected, thermal expansion of Ba3Sr3B4O12 is less anisotropic
0D-Borates with Pyroborate Groups
M5B4O11 (M = (Sr,Ba), Ba), 3B : 3Δ : Δ,ΔΔ, are based on isolated BO3 triangles and B2O5 pyroborate groups in relation of 1:2. Ba5B4O11 (ICSD–250321) is orthorhombic CN Ba 8-10, R = 1.56-1.66 Å. The monoclinic Ba2Sr3B4O11 structure contains B2O5 pyroborate groups orientationally disordered over the 2 sites. Thermal expansion in these group is strongly anisotropic for monoclinic Ba2Sr3B4O11 (Table 1), and less anisotropic for orthorhombic Ba5B4O11 [59].
М2B2O5 (M = Ca, Sr), 2B : 2Δ : ΔΔ.
γ-Ca2B2O5 (ICSD–66516) and α-Ca2B2O5 [50], As it was mentioned above, the structures of both modifications contain isolated B2O5 pyroborate groups composed of two corner-sharing BO3 triangles (Figure 3). Both monoclinic structures expand highly anisotropically up to compression for γ-phase (Table 1). Thermal displacements of atoms occur in a direction almost perpendicular to the plane of both triangles. Strong anisotropy of expansion is also caused by shear deformations in the monoclinic plane which are sharply anisotropic in nature [23]. Some decrease of monoclinic angle is accompanied by the most intensive expansion along [101] direction and minimal thermal expansion including contraction along [101].
The values of volume expansion of γ-, β- and α-Sr2B2O5 are close to each other and Ca2B2O5 except of that of α′-Sr2B2O5. The αV value of α′- Sr2B2O5 (181 ×10−6 C-1) is unusually large. Thermal expansion of the Sr2B2O5 modifications is strongly anisotropic, even with a negative thermal expansion in some directions (Table 1). The anisotropy has a similar character for all of them: the maximal (α11) tensor axis is directed practically perpendicular to planes of BO3 pyroborate groups [49].
0D-Borates with Cyclic (Triborate) Groups B3O6
BaB2O4 (0D), 3B : 3Δ : <3Δ> exists in two polymorphic modifications (α and β), the transition temperature is 925 °С [81,82]. Both modifications are based on the same isolated groups B3O6. Both modifications crystallize in the trigonal system, the space groups are R3c for β-BaB2O4 (a = 12.53, c = 12.72 Å; 69319-ICSD) and R-3c for α-BaB2O4 (a = 7.235, c = 39.192 Å; 14376-ICSD). The anisotropy of the expansion is dictated by the orientation of rigid 3B-groups in the structure, where the expansion in the plane is minimal and perpendicular to the plane is maximal. The comparison between both modifications shows that the nonlinear-optical β-polymorph expands more anisotropically [58] than the centrosymmetric phase, α-BaB2O4 (Table 1) [23], although their volumetric expansion is comparable: αV= 40 and 51×10−6 C-1 for α- and β-BaB2O4, respectively.
1D-Borates Based on Chains of BO3 Triangles
МB2O4 (M = Ca, Sr, Ba), 1B : Δ : Δ (1D). CaB2O4: Са CN 8 (ICSD–62430). The structure contains the chains of BO3 triangles along the с axis. Planes of the triangles are approximately parallel to the (001) plane (Figure 5b). The structure expands intensively along the a axis, in a direction perpendicular to the plane of borate triangles. (Table 1). Minimal expansion occurs along the boron-oxygen chains parallel to the c axis since the strongest bonds are realized in this direction.
2D-and 3D-Borates
Sr3B14O24 (2D) (ICSD–136651)
14B : 8Δ6□ : [B14O30] : <2Δ□><2Δ□>=<2Δ2□><2Δ□>□ΔΔ□. The thick complex layer of the structure (Figure 5c) is composed of two [B4O10] units and [B4O9] double ring connected via two triangles [BO3] forming polyanion [B14O30] [60]. The anisotropic expansion is typical for the layered structures with the maximal expansion perpendicular to the layer plane ab.
МB4O7 (M = Ca, Sr, Ba) (3D)
α-CaB4O7 (3D). C.N. Са 7, 8 (ICSD–200081). The crystal structure (Figure 5d) is characterized by a boron-oxygen polyanion consisting of four crystallographically independent BO3 triangles and four BO4 tetrahedra [45]. The eight triangles and tetrahedra form a repeated [B8O14]4−-unit, which consists of three B–O-groups linked via common vertices – a single tetrahedron, single triborate ring composed of two triangles and a tetrahedron and a tetraborate group – double ring in which two rings from two tetrahedra and a triangle condensed via two tetrahedra. The Ca atoms are in seven- and eight-vertex polyhedra of oxygens atoms. The maximal direction of thermal expansion in the structure (α11 = 8×10–6 °C–) coincides with an acute angle bisector of the ac parallelogram and the minimum expansion occurs along the other diagonal. The structure expands along the b axis less intensely: α22 = αb = 8×10−6 °С−1. Such sharply anisotropic behavior could be explained using shear deformation of the monoclinic plane [23].
SrB4O7, 4B:4□: [φ] <3□>|□| (3D) (ICSD–27404), has almost isotropic thermal expansion. This structure is composed from BO4 tetrahedra only. Moreover, it contains the units built by three tetrahedra shared one common oxygen atom presumably responsible for the isotropic thermal expansion [57].
BaB4O7, 8B: 4Δ4□:<Δ2□><2Δ□>–<Δ2□>, is monoclinic [83]. The framework consists of <Δ2□> triborate (3B) and <2Δ□>–<Δ2□> pentaborate (5B) groups composed from two single threefold rings; in the cavity of the framework, there are two nonequivalent barium atoms. The thermal expansion of the BaB4O7 borate is the most among alkaline-earth borates anisotropic: the expansion is maximum in the ac monoclinic plane, whereas the contraction occurs along the b axis [60]. The anisotropy of deformations of the ac monoclinic plane is caused by the considerable change in the angle β and the related shear deformations as well as arranging 5B-groups. As it discussed before [4,19,79] in the case of structure with 5B-groups the internal oxygen and boron atoms of both single rings vibrate perpendicular to the planes of these rings, while the group as a whole oscillates relative to the axis of the 5B-group – the line drawn parallel to the plane of both rings. The maximum expansion occurs perpendicular to the axis of the group and the minimal expansion is along the axis of group. Similar character of thermal expansion is typical for hydrous and anhydrous alkali pentaborates of 0D-, 1D-, 2D- and 3D-dimensionality [19,23].
It is reasonable to conclude that the 5B-groups are arranged in parallel and in this α33 direction structure expands weakly in comparison to the α11 direction in the ac plane [19]. The thermal contraction of the structure along the b axis can be associated with the displacement of the Ba atoms so that the B–O framework is adapted to the displacement of cations due to the rotation of the B–O groupings according to the hinge mechanism.
МB8O13 (M = Sr, Ba) (3D)
Sr2B16O26, 16B: 12Δ4□ : 2(<2Δ□><2Δ□>–<2Δ□>) [84]. There are two independent Sr atoms, 16 B atoms, and 26 O atoms in asymmetrical unit. Each of two penetrating frameworks consists of two triborate [B3O5]5− rings (two triangles and tetrahedron) and two pentaborate [B5O8]5− groups (two triborate rings shared common tetrahedron). The direction of the maximal thermal expansion is close to c axis and close to axis of one of 5B-grops. There are a few reasons for such character. First of all planes of both 3B-rings are practically perpendicular to c axis; also an explanation could be a shear character of deformations due to the change in monoclinic β angle not fixed by symmetry as it is noted in [57].
Low-temperature BaB8O13 polymorph, 16:∞3: [(5:4Δ + □) + 3(3:2Δ + □) + (2:2Δ)]. The crystal structure is based on the heteropolyhedral framework comprised of vertex-sharing [B5O10]-pentaborate group; three [B3O7]-triborate groups; and one [B2O5]-diborate group. In the fundamental building block of the heteropolyhedral borate framework, the [B2O5]-diborate and [B5O10]-pentaborate groups are connected exclusively by triborate groups via common oxygens. The thermal expansion of the BaB8O13 borate is strongly anisotropic with anisotropy increasing with temperature (Table 1). The crystal structure shrinks along [001] over the studied temperature range (30–680 °C).
4. Conclusions
The thermal expansion of four alkaline-earth borates, Ca3B2O6, CaB2O4, Sr3B14O24 and CaB4O7, has been investigated by in situ powder HTXRD. A comparative analysis of their thermal expansion with the data on the other alkaline-earth borates showed that most of them expand sharply anisotropically.
As it can be expected, strongest anisotropy of thermal expansion was observed for the 0D- and 1D-structures based on BO3 triangles only from the range of compositions 0-50 mol. % B2O3. These borates expand most intensively perpendicular to the BO3 plane, i.e., along the direction of weaker bonding in the crystal structure. Such character of expansion is caused by the minimal thermal atomic vibration within the plane of triangle and the maximal in perpendicular direction. The borates built by complex rigid groups expand dramatically anisotropically when the complex units contain more triangles than tetrahedra or their quantity comparable. In contrast, SrB4O7, composed of tetrahedra only, demonstrates almost isotropic thermal expansion due to the random distribution of the rigid B–O bonds in the crystal structure [4,23,57]; also, there is weak anisotropy in layered Sr3B14O24 and in a few borates where BO3 planes are depicted not in parallel and the amount of BO3 is not significant.
By the way, our analysis showed that ∆plane can serve as an optimal quantitative estimation of the degree of anisotropy of thermal expansion. Both, ∆plane and ∆V give similar results. Thus, in order to estimate the degree of anisotropy, it is generally enough to estimate the anisotropy of the most anisotropic plane, ∆plane.
The most common trends of alkaline earth borates thermal expansion were emerged from the correlations of thermal properties like volume thermal expansion, degree of anisotropy to the dimensionality of B-O anion, residual charge per one polyhedron (BO3 / BO4), cationic size, and structural complexity. First, we can conclude that sharp anisotropy is caused by the strong bonding within the triangle plane: melting temperatures and volume expansion decrease as the dimensionality of the borate polyanion, and more precisely, the residual charge decreases. The structural complexity increases and the melting point values decrease with increase in B2O3 content, i.e., with increase in polymerization degree. Most steady trends have been found for borates based upon BO3 triangles only.
Supplementary Materials
The following supporting information can be downloaded at the website of this paper posted on Preprints.org, Table S1: Temperature dependencies of the unit-cell parameters for Ca-borates and Sr3B14O24 approximated by linear and quadratic polynomial functions a0 + a1 ×10 –3 t + a2 ×10 –6 t2; Table S2 Thermal expansion coefficients for Ca-borates and Sr3B14O24
Author Contributions
Writing—original draft, R.S.B., V.A.Y.; M.G.K.; Writing—review and editing, R.S.B., V.A.Y.; M.G.K..; Investigation, V.A.Y.; M.G.K.; and G.S.S.; Project administration, R.S.B., V.A.Y.; M.G.K.; Data curation, R.S.B., V.A.Y.; M.G.K. and S.K.F.; Visualization, V.A.Y.; and G.S.S.; Supervision, S.K.F. All authors have read and agreed to the published version of the manuscript.
Funding
This work was supported by the Ministry of Science and Higher Education of the Russian Federation within the scientific tasks of the Institute of Silicate chemistry (Russian Academy of Sciences) [project number 0081-2022-0002] (synthesis), and the Russian Science Foundation [grant number 22-13-00317] (data evaluation and generalization, XRD).
Acknowledgments
The X-ray diffraction experiments were performed at The Centre for X-rayDiffraction Studies (Saint Petersburg State University).
Conflicts of Interest
Declare conflicts of interest or state “The authors declare no conflicts of interest.” Authors must identify and declare any personal circumstances or interest that may be perceived as inappropriately influencing the representation or interpretation of reported research results. Any role of the funders in the design of the study; in the collection, analyses or interpretation of data; in the writing of the manuscript; or in the decision to publish the results must be declared in this section. If there is no role, please state “The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results”.
References
- Hawthorne F.C., Burns P. C., Grice J. D. The crystal chemistry of boron. Rev. Miner. 1996, 33, 41–116.
- Touboul, M.; Penin, N.; Nowogrocki, G. Borates: A Survey of Main Trends Concerning Crystal-Chemistry, Polymorphism and Dehydration Process of Alkaline and Pseudo-Alkaline Borates. Sol. Stat. Sci. 2003, 5(10), 1327–1342. [CrossRef]
- Yuan, G.; Xue, D. Crystal chemistry of borates: the classification and algebraic description by topological type of fundamental building blocks. Acta Crystallogr. 2007, B63, 353–362.. [CrossRef]
- Bubnova, R.S.; Filatov, S.K. High-temperature crystal chemistry borates and borosilicates; Nauka: St-Petersburg, Russian , 2008.
- Mutailipu, M.; Poeppelmeier, K.R.; Pan, S.L. Borates: A rich source for optical materials, Chem. Rev. 2021, 121, 1130–1202. [CrossRef]
- Huang, C.; Mutailipu, M.; Zhang, F.; Griffith, K.J.; Hu, C.; Yang, Z.; Griffin, J.M.; Poeppelmeier, K.R.; Pan S. Expanding the chemistry of borates with functional [BO2]− anions. Nat. Commun. 2021, 12, 2597. [CrossRef]
- Huppertz, H.; Eltz, B. Multianvil High-pressure synthesis of Dy4B6O15: the first oxoborate with edge-sharing BO4 tetrahedra. J. Am. Chem. Soc. 2002, 124, 9376–9377. [CrossRef]
- Huppertz, H.; Keszler, D.A. Borates: Solid-State Chemistry. Encyclop. Inorg. Bioinorg. Chem. 2014, 1–12.
- Shifeng, J.; Gemei, C.; Wanyan, W.; Meng, H.; Shunchong, W.; Xiaolong, C. Stable oxoborate with edge-sharing BO4 tetrahedra synthesized under ambient pressure. Angew. Chem. Int. 2010, 49, 4967–4970.. [CrossRef]
- Wu, Y.; Yao, J.Y.; Zhang, J.X.; Fu, P.Z.; Wu, Y.C. Potassium zinc borate, KZnB3O6. Acta Crystallogr. Sect. E 2010, 66, i45. [CrossRef]
- Becker P. A contribution to borate crystal chemistry: rules for the occurrence of polyborate anion types. Z. Kristallogr. 2001, 216, 523–533.
- Chen, C.; Sasaki, T.; Li, R.; Wu, Z.; Lin, Z.; Mori, Y.; Hu, Z.; Wang, J.; Uda, S.; Yoshimura, M.; Kaneda, Y. Nonlinear Optical Borate Crystals, Principles and Applications; Wiley-VCH: Weinheim, Germany, 2012.
- Ge, X.; Mao, Y.; Liu, X.; Cheng, Y.; Yuan, B.; Chao, M.; Liang, E. Negative thermal expansion and broad band photoluminescence in a novel material of ZrScMo2VO12. Sci. Rep. 2016, 6, 24832. [CrossRef]
- Kim, Y.H.; Arunkumar, P.; Kim, B.Y.; Unithrattil, S.; Kim, E.; Moon, S.; Hyun, J.Y.; Kim, K.H.; Lee, D.; Lee, J.; Im, W.B. A zero-thermalquenching phosphor. Nat. Mater. 2017, 16, 543–550.
- Dang, P.; Li, G.; Yun, X.; Zhang, Q.; Liu, D.; Lian, H.; Shang, M.; Lin, J. Thermally stable and highly efficient red-emitting Eu3+-doped Cs3GdGe3O9 phosphors for WLEDs: non-concentration quenching and negative thermal expansion. Light: Sci. Appl. 2021, 10, 29. [CrossRef]
- Zhou, L.; Wang, W.; Xu, D.; Wang, Z.; Yi, Z.; Wang, M.; Lu, Z. Anti-thermal quenching of Eu3+ luminescence in negative thermal expansion Zr(WO4)2. Ceram. Internat. 2021, 47, 34820–34827.
- Takenaka, K. Progress of research in negative thermal expansion materials: paradigm shift in the control of thermal expansion. Front. Chem. 2018, 6, 267. [CrossRef]
- Filatov, S.K.; Bubnova, R.S. Borate Crystal Chemistry. Phis. Chem. Glasses. 2000, 41(5), 216–224.
- Bubnova, R.S.; Filatov, S.K. High-Temperature borate crystal chemistry. Z. Kristallogr. Cryst. Mater. 2013, 228(9), 395–413.
- Filatov, S. K.; Bubnova, R. S.; Atomic nature of the high anisotropy of borate thermal expansion. Phys. Chem. Glasses: Eur. J. Glass Sci. Technol. Sect. B 2015, 56, 24–35.
- Bubnova, R.; Volkov, S.; Albert, B.; Filatov, S. Borates—Crystal Structures of Prospective Nonlinear Optical Materials: High Anisotropy of the Thermal Expansion Caused by Anharmonic Atomic Vibrations. Crystals 2017, 7, 93–112. [CrossRef]
- Volkov, S.N.; Charkin, D.O.; Firsova, V.A.; Aksenov, S.M.; Bubnova, R.S. Gram–Charlier approach for anharmonic atomic displacements in inorganic solids: A review. Crystallogr. Rev. 2023, 29, 2023. [CrossRef]
- Bubnova, R.S.; Filatov, S.K. Strong anisotropic thermal expansion in borates. Phys. Stat. Sol. 2008, 245, 2469–2476. [CrossRef]
- Yao, W.; Jianf, X.; Huang, R.; Li, W.; Huang, C.; Lin, Z.; Li, L.; Chen, C. Area negative thermal expansion in a beryllium borate LiBeBO3 with edge sharing tetrahedra. Chem. Commun., 2014, 50, 13499–13501. [CrossRef]
- Lou, Y.; Li, D.; Li, Z.; Jin, S.; Chen, X. Unidirectional thermal expansion in edge-sharing BO4 tetrahedra contained KZnB3O6. Sci. Rep. 2015, 5, 10996. [CrossRef]
- Jiang, X.; Molokeev, M.S.; Li, W.; Wu, S.; Lin, Z.; Wu, Y.; Chen, C. The mechanism of the area negative thermal expansion in KBe2BO3F2 family crystals: A first-principles study. J. Appl. Phys. 2016, 119, 055901. [CrossRef]
- Mutailipu, M.; Zhang, M.; Li, H.; Fan, X.; Yang, Z.; Jin, S.; Wang, G.; Pan, S. Li4Na2CsB7O14: a new edge-sharing [BO4]5− tetrahedra containing borate with high anisotropic thermal expansion. Chem. Commun. 2019, 55, 1295–1298. [CrossRef]
- Sagatov N.E.; Gavryushkin, P.N.; Bekker, T.B.; Litasovde, K.D. Ba3(BO3)2: the first example of dynamic disorder in a borate crystal. Phys. Chem. Chem. Phys. 2022, 24, 16437-16441. [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. Sect. A 1976, 32, 751–767. [CrossRef]
- Schuckmann, W. Zur kristallstruktur des calcium-borates Ca3(BO3)2 Neues Jahrb. Mineral., Monatsh. 1969, 142–144.
- Majling, J.; Figusch,V.; Hanic, F.; Wiglasz, V.; Corba, J. Crystal data and thermal expansion of tricalciumborate. Mater. Res. Bull. 1974, 9, 1379. [CrossRef]
- Vegas, A.; Cano, F.H.; Garcia-Blanco, S. The crystal structure of calcium orthoborate: a redetermination. Acta Crystallogr. Sect. B 1975, 31, 1416–1419. [CrossRef]
- Kusachi, I.; Henmi, C.; Kobayashi, S. Takedaite, a new mineral from Fuka, Okayama Prefecture, Japan. Mineral. Mag. 1995, 59, 549–552. [CrossRef]
- Hart, P.B.; Brown, C.S. The synthesis of new calcium borate compounds by hydrothermal methods. J. Inorg. Nucl. Chem. 1962, 24, 1057–1065. [CrossRef]
- Ji, Y.; Liang, J.; Xie, S.; Zhu, N.; Li, Y. Structure of 2CaO-B2O3. Acta Crystallogr. C 1993, 49, 78–79. [CrossRef]
- Shashkin, D.N.; Simonov, M.A.; Belov, N.V. Crystal structure of calciborite CaB2O4 = Ca2[BO3BO]2. Dokl. Akad. Nauk SSSR 1970, 195, 345–348.
- Shashkin, D.P.; Simonov, M.A.; Belov, N.V. X-ray diraction study of natural calcium metaborates. Sov. Phys. Crystallogr. 1971, 16, 186.
- Zachariasen, W.H. The Crystal Lattice of Calcium Metaborate, CaB2O4. Proc. Natl. Acad. Sci. U.S.A. 1931, 17, 617. [CrossRef]
- Marezio, M.; Plettinger, H.A.; Zachariasen, W.H. Refinement of the calcium metaborate structure. Acta Crystallogr. 1963, 16, 390–392. [CrossRef]
- Marezio, M.; Remeika, J.P.; Dernier, P.D. The crystal structure of the high-pressure phase CaB2O4(III). Acta Crystallogr. B 1969, 25, 955–964. [CrossRef]
- Marezio, M.; Remeika, J.P.; Dernier, P.D. The crystal structure of the high-pressure phase CaB2O4(IV), and polymorphism in CaB2O4. Acta Crystallogr. B 1969, 25, 965–970.
- Kirfel, A. The electron density distribution in calcium metaborate, Ca(BO2)2. Acta Crystallogr. B 1987, 43, 333–343. [CrossRef]
- Zayakina, N.V.; Brovkin, A.A. Crystal structure of Ca2B6O11. Kristallogr. 1976, 21, 502–506.
- Kindermann, B. Svnthese und kristallographische Daten von Calciumdiborat, CaB4O7. Z. Kristallogr. 1977, 146, 61–66.
- Zayakina, N.V.; Brovkin, A.A. Crystal Structure of CaB4O7. Sov. Phys. Crystallogr. 1977, 22, 156.
- Huppertz, H. β-CaB4O7: a new polymorph synthesized under high-pressure/high-temperature conditions. Zeitschrift für Naturforschung B, 2003, 58(4), 257–265. [CrossRef]
- Chen, X.; Li, M.; Chang, X.; Zang, H.; Xiao, W. Synthesis and crystal structure of a new calcium borate, CaB6O10. J. Alloys Compd. 2008, 464(1-2), 332–336. [CrossRef]
- Witzmann, H.; Beulich, W. Zur Bildung wasserfrier Strontiumborate. Ein Beitrag zum Zustandsdiagramm des Systems SrO-B2O3. Z. Phys. Chem. (Leipzig) 1964, 225, 336–341. [CrossRef]
- Volkov, S.; Dušek, M.; Bubnova R.; Krzhizhanovskaya, M.G.; Ugolkov, V.; Obozova, V.; Filatov, S. Orientational order-disorder γ ↔ β ↔ α′ ↔ α phase transitions in Sr2B2O5 pyroborate and crystal structures of β and α phases. Acta Cryst. B 2017, 73, 1056-1067. [CrossRef]
- Yuhno, V.; Volkov, S.; Bubnova, R.; Povolotskiy, A.; Ugolkov, V. High-temperature γ ↔ β′ ↔ α phase transitions in Ca2B2O5: Thermal expansion and crystal structure of α-phase. Sol. Stat. Sci. 2020, 121, 106726. [CrossRef]
- Fukuda, Y.; Tomita, A.; Takeuchi, N. Thermoluminescence and thermally stimulated exoelectron emission of sintered CaB4O7 doped with Pb, Eu, or Dy. Phys. Status Solidi A 1987, 99, K135–K138. [CrossRef]
- Paun, J.; Iozsa, A.; Jipa, S. Dosimetric characteristics of alkaline-earth tetraborates radiothermoluminescent detectors. Radiochem. Radioanal. Lett. 1977, 28, 411.
- Berezovskaya, I. V.; Efryushina, N. P.; Rodnyi, P. A.; Potapov, A. S.; Pirozhenko, P. V.; Dotsenko, V. P. Luminescence of Yb3+ ions in calcium tetraborate with charge transfer. Optics and Spectroscopy, 2003, 95(5), 741-745. [CrossRef]
- Huang, H.; Feng, X.; Chen, T.; Xiong, Y.; Zhang, F. Synthesis and luminescence properties of Ca3(BO3)2: Eu3+ via two-step method. Lumin. 2018, 33(4), 1–6. [CrossRef]
- Yang, L.; Wan, Y.; Li, Y.; Pu, Y.; Haung, Y.; Chen, C.; Seo, H.J. Hydrothermal synthesis, characterization, and luminescence of Ca2B2O5:RE (RE = Eu3+, Tb3+, Dy3+) nanofibers. J. Nanopart. Res. 2016, 18, 94.
- Firsova, V.A.; Shablinskii, A.P.; Ershov, D.S.; Bubnova, R.S. Thermal Expansion of Mg3B2O6 Borate. Glass Phis. Chem. 2019, 45, 302–304.
- Filatov, S.K., Krzhizhanovskaya, M.G.; Bubnova, R.S.; Shablinskii, A.P.; Belousova, O.L.; Firsova, V.A. Thermal expansion and structural complexity of strontium borates. Struct. Chem. 2016, 27, 1663–1671.
- Filatov, S.K.; Nikolaeva, N.V.; Bubnova, R.S.; Polyakova, I.G. Thermal expansion of β-BaB2O4 and BaB4O7 borates. Glass Phys. and Chem. 2006, 32(4), 471–478. [CrossRef]
- Volkov, S.; Bubnova, R.; Povolotskiy, V.; Ugolkov, V.; Arsent'ev, M. Two novel centrosymmetric barium strontium borates with a deep-UV cut-off edge: Ba2Sr3B4O11 and Ba3Sr3B4O12. J. of Sol. Stat. Chem. 2020, 281, 121023. [CrossRef]
- Wu, С.; Chen, Z.; Chen, J.; Yang, Z.; Zhang, F.; Shi, H.; Pan, S. Sr3B14O24: A New Borate with [B14O30] Fundamental Building Block and Unwonted 2D Double Layer. Dalton Trans., 2022, 51, 618-623. [CrossRef]
- Filatov, S.K. Anomale Warmeausdehnung von V2O5. Kristall. Technik. 1971, 6, 777–785. [CrossRef]
- Firsova, V.A.; Bubnova, R.S.; Filatov, S.K. Program for the Thermal Expansion Tensor Determination for Crystalline Materials. Institute of Silicate Chemistry of Rus. Acad. Sci., St. Petersburg, Russia, 2011.
- Bubnova, R.S.; Firsova, V.A.; Filatov, S.K. Software for determining the thermal expansion tensor and the graphic representation of its characteristic surface (Theta to Tensor—TTT). Glass Phys. Chem. 2013, 39, 347–350. [CrossRef]
- Firsova, V.A.; Bubnova, R.S.; Volkov, S.N.; Filatov, S.K. RietveldToTensor (RTT). Institute of Silicate Chemistry of Rus. Acad. Sci., St Petersburg, Russia, 2015.
- Bubnova, R.S.; Firsova, V.A.; Volkov, S.N.; Filatov, S.K. RietveldToTensor: Program for Processing Powder X-Ray Diffraction Data under Variable Conditions. Glass Phys. Chem. 2018, 39, 347–350.
- Krivovichev, S.V. Structural complexity and configurational entropy of crystals. Acta Crystallogr. Sect. B Struct. Sci. 2016, 72, 274–276. [CrossRef]
- Blatov, V.A.; Shevchenko, A.P.; Serezhkin, V.N. TOPOS3.2: a new version of the program package for multipurpose crystal-chemical analysis. J Appl Crystallogr. 2000, 33, 1193. [CrossRef]
- Krivovichev, S.V. Information-based measures of structural complexity: application to fluorite-related structures. Struct. Chem. 2012, 23, 1045–1052. [CrossRef]
- Krivovichev, S.V. Structural complexity of minerals: information storage and processing in the mineral world. Miner. Mag. 2013, 77, 275–326. [CrossRef]
- Volkov, S.N.; Yukhno, V.A.; Bubnova, R.S.; Aksenov, S.M.; Povolotskiy, A.V.; Charkin, D.O.; Arsent’ev, M.Y.; Ugolkov, V.L.; Krzhizhanovskaya, M.G. Resolving the Problems of the Past: Reinvestigation of the Structure of Acentric Deep UV BaB8O13 Borate. Cryst. Growth Des. 2022, 22, 6267−6274.
- Filatov, S.K. Vysokotemperaturnaya Kristallokhimiya (High temperature crystal chemistry). Nedra: Leningrad, Russian, 1990.
- Filatov, S.K. Negative linear thermal expansion of oblique-angle (monoclinic and triclinic) crystals as a common case. Phys. Status Solidi B, 2008, 245, 2490–2496. [CrossRef]
- Tulsky, E.G.; Long, J.R. Dimensional reduction: a practical formalism for manipulating solid structures. Chem. Mater. 2001, 13, 1149–1166. [CrossRef]
- Wright, A.C. Borate structures: crystalline and vitreous. Glass. Phys. Chem. 2010, 51, 1-39.
- Krogh-Moe, J. Interpretation of the infra-red spectra of boron oxide and alkali borate glassеs. Phys. Chem. Glasses 1965, 6, 46–54.
- Shannon, C.E.; Weaver, W. The Mathematical Theory of Communication, by C.E. Shannon (and Recent Contributions to the Mathematical Theory of Communication). University of illinois Press: Urbana, U.S.A., 1949.
- Cruickshank, D.W.J. Errors in bond lengths due to rotational oscillations of molecules. Acta Crystallogr. 1956, 9(7), 757–758. [CrossRef]
- Downs, R.T. Analysis of harmonic displacement factors. Rev. Miner. 2000, 41, 61–87.
- Bubnova, R.S.; Filatov, S.K. Self-assembly and high anisotropy thermal expansion of compounds consisting of TO3 triangular radicals. Struc. Chem. 2016, 27(6), 1647–1662. [CrossRef]
- Berger, S. V.: The crystal structure of the isomorphous orthoborates of cobalt and magnesium. Acta Chem. Scand. 1949, 3, 660–675. [CrossRef]
- Block, S.; Perloff, A.; Mighell, A.D. The crystal structure of the high temperature form of barium borate, BaO(B2O3). Acta Crystallogr. 1966, 20, 819–823.
- Itoh, K.; Marumo, F.; Ohgaki, M.; Tanaka, K. Structure refinement of β -BaB2O4. Rep. Research Lab. Engineer. Mater., Tokyo Inst. of Technol. 1990, 15, 1–11.
- Blok, S.; Perloff, A. The Crystal Structure of Barium Tetraborate, BaO · 2B2O3. Acta Crystallogr., Sect. B: Struct. Crystallogr. Cryst. Chem. 1964, 19, 297–298. [CrossRef]
- Tang, Z.; Chen, X.A.; Li, M. Synthesis and crystal structure of a new strontium borate, Sr2B16O26. Sol. Stat. Sci. 2008, 10(7), 894–900. [CrossRef]
Figure 1.
The unit-cell parameters of Ca3B2O6, CaB2O4, Ca2B2O5, Sr3B14O24 and CaB4O7 at different temperatures. The errors are smaller than symbols.
Figure 1.
The unit-cell parameters of Ca3B2O6, CaB2O4, Ca2B2O5, Sr3B14O24 and CaB4O7 at different temperatures. The errors are smaller than symbols.
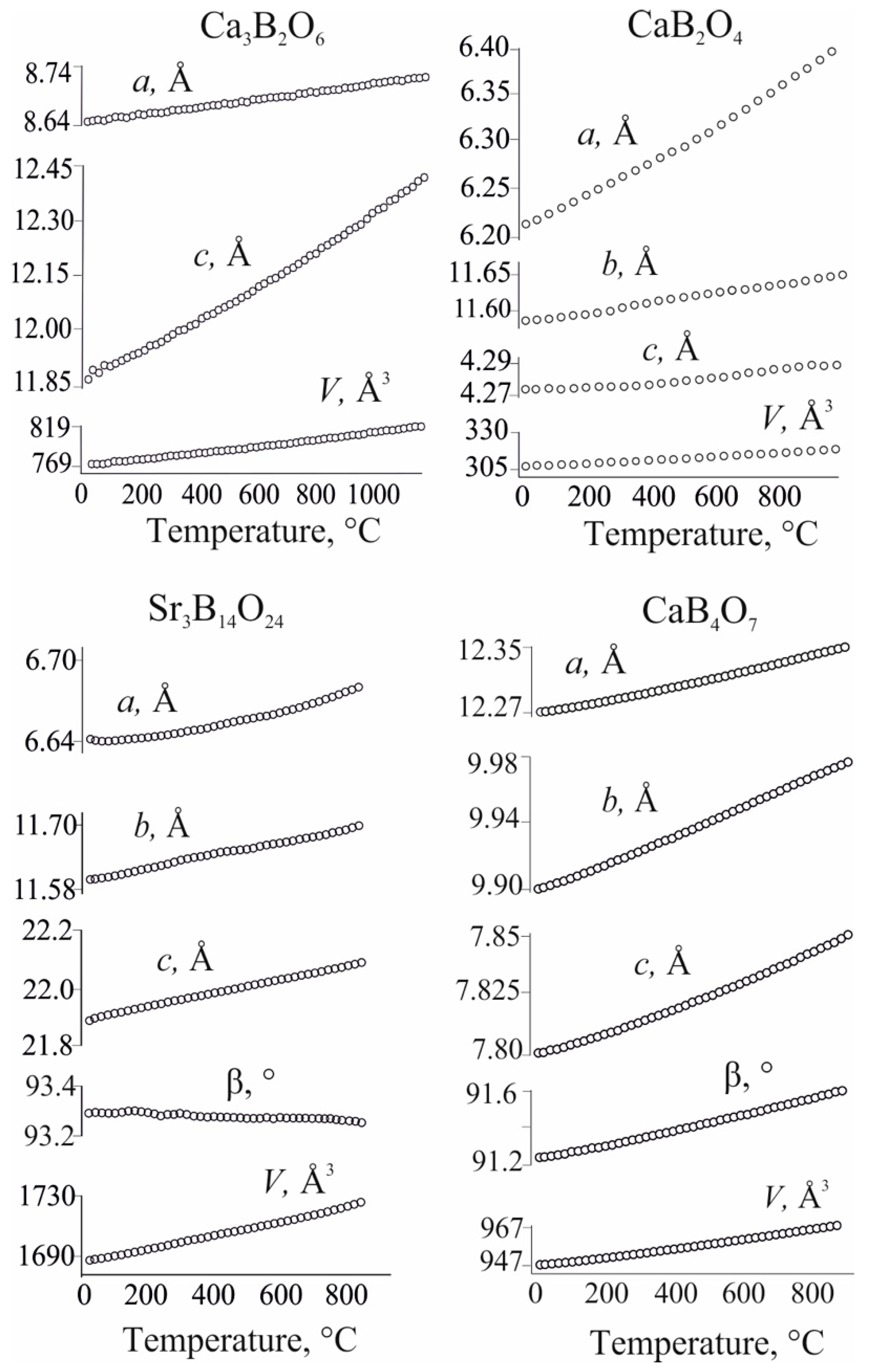
Figure 2.
Dependence of the degree of anisotropy ∆V and as ∆plane on the residual charge of the polyanion calculated per a triangle / tetrahedron.
Figure 2.
Dependence of the degree of anisotropy ∆V and as ∆plane on the residual charge of the polyanion calculated per a triangle / tetrahedron.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






