Prerpints.org logo
Preprint
Article

Rheology of Suspensions Thickened by Cellulose Nanocrystals

Altmetrics

Downloads

99

Views

425

Comments

0

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

03 June 2024

Posted:

04 June 2024

You are already at the latest version

Alerts
Abstract
The steady rheological behavior of suspensions of solid particles thickened by cellulose nanocrystals is investigated. Two different types and sizes of particles are used in the preparation of suspensions, namely TG hollow spheres of Sauter mean diameter of 69 µm and Solospheres S-32 of Sauter mean diameter of 14 µm. The nanocrystal concentration varies from 0 to 3.5 wt% and the particle concentration varies from 0 to 57.2 vol%. The influence of salt (NaCl) concentration and pH on the rheology of suspensions is also investigated. The suspensions generally exhibit shear-thinning behavior. The degree of shear-thinning is stronger in suspensions of smaller size particles. The experimental viscosity data are adequately described by a power-law model. The variations of power-law parameters (consistency index and flow behavior index) under different conditions are determined and discussed in detail.
Keywords: 
Subject: Engineering  -   Chemical Engineering

1. Introduction

Suspensions are dispersions of solid particles in liquids. They are very important industrially as many products of commercial importance are sold or handled in the form of suspensions. Industries where suspensions are encountered include foods, pharmaceuticals, petroleum, ceramics, mineral processing, construction, cosmetics and toiletries, paints, agriculture, biotechnology, polymers, and many more. [1,2,3,4,5,6,7,8,9,10,11]. A good understanding of the rheology of suspensions is essential in the formulation, mixing, processing, transport, and storage of suspensions. One problem with suspensions is that they are prone to phase separation under the influence of gravity. For example, the particles of the suspension would settle at the bottom of the container if the particles were heavier than the suspending medium liquid. As particles of suspension are almost always of different density than the suspending medium, the only option to minimize phase separation in suspensions is to thicken the matrix phase. To that end, thickeners or rheology modifiers are incorporated into the matrix phase of suspensions. According to the Stokes law, the settling velocity of a particle is given as [12]:
U t = ρ p − ρ g d p 2 18 μ
where U t is the terminal settling velocity of a particle, ρ p is density of particle, g is acceleration due to gravity, d p is particle diameter, ρ and μ are matrix fluid density and viscosity, respectively. Thus, sedimentation of particles can be minimized by increasing the matrix viscosity and reducing the particle diameter assuming that the density difference between the particle and the matrix fluid is fixed.
The thickeners used in the formulation of suspensions include polymers, clays, surfactants, and nanoparticles [13]. However, more recently, nanocrystalline cellulose (NCC) is receiving a lot of attention as a rheology modifier or thickener of matrix phase due to the high aspect ratio and surface charge of nanocrystals. Nanocrystalline cellulose, also referred to as cellulose nanocrystals (CNCs), is a promising low-cost nanomaterial with many potential applications [14,15,16,17,18,19,20,21,22,23,24,25,26]. It is non-toxic, biodegradable, and renewable. It is produced from the most abundantly available biopolymer on our planet, namely cellulose by sulfuric acid hydrolysis of amorphous portions of cellulose fibers. The nanocrystals of NCC are rod-shaped and possess a negative charge when dispersed in water.
In this study, we report new results on the rheology of suspensions thickened by nanocrystalline cellulose covering broad ranges of NCC and particle concentrations. Two different size particles are used. The NCC concentration varied from 0 to 3.5 wt% based on the matrix liquid. The particle volume fraction varied from 0 to 0.57. The effects of pH and salt concentration on the rheology of suspensions were also investigated.

2. Materials and Methods

2.1. Materials

Two different types of particles with different particle sizes were used: Low-density hollow spherical particles (trade name Extendospheres TG hollow spheres) with Sauter mean diameter of 69 μ m and solid spherical particles (trade name Solospheres S-32) with Sauter mean diameter of 14 μ m . The particles were supplied by Sphere One, Inc., Chattanooga, Tennessee. Extendospheres TG hollow particles are low density, high strength ceramic particles used extensively in sealants, roofing compounds, caulks, adhesives, and latex flooring. Solospheres S-32 are solid ceramic particles used in the manufacturing of anti-skid floor coatings, gaskets, cementitious coatings, insulative roof coatings, chemical resistance coatings and naval cements.
Cellulose nanocrystals, that is, NCC, was supplied by CelluForce Inc., Windsor, On, Canada, under the trade name of NCC NCV100-NASD90. The nanocrystals were manufactured using sulfuric acid hydrolysis of wood pulp. Figure 1 shows the atomic force microscopy (AFM) image of cellulose nanocrystals. Clearly, nanocrystals are rod shaped particles.

2.2. Preparation of Dispersions of Cellulose Nanocrystals

The dispersions of cellulose nanocrystals (NCC) were prepared at room temperature
( ≅ 23 o C ) by slowly dispersing a known amount of NCC into a known amount of de-ionized water. The mixing of the dispersion was maintained using a turbine homogenizer (Gifford-Wood, model 1L) at a fixed speed. The dispersion was homogenized for at least 60 min for the nanocrystals to disperse and homogenize fully. Six differently concentrated NCC dispersions (0.25, 0.50, 1.0, 1.5, 2.5, and 3.5 wt%) were prepared.

2.3. Preparation of Suspensions of Solid Particles in CNC Dispersion

Suspensions were prepared at room temperature ( ≅ 23 o C ) by adding a known amount of particles (Extendospheres TG hollow spheres or Solospheres S-32) to a known amount of CNC dispersion. Gentle mixing of the fluids was maintained during the addition of particles to the CNC dispersion using the homogenizer. The suspension was finally homogenized in the homogenizer at a high speed for at least 30 min after the addition of the particles to the CNC dispersion. To increase the particle concentration of the suspension, a known amount of more particles was added slowly to an existing lower particle concentration suspension and the mixture was homogenized at a high speed for at least 30 min.

2.4. Measurements

The size distribution of NCC was measured using Dynamic Light Scattering (DLS) carried out in a Zetasizer Nano ZS90 manufactured by Malvern Instruments Ltd, Worcester, UK. The samples consisting of a very dilute dispersion of NCC in water were tested in ZEN0112, low volume disposable sizing cuvette, at 25°C. A 120-second equilibration period was observed prior to analysis.
The rheological measurements were carried using two Fann co-axial cylinder viscometers with different torsion spring constants and a Haake co-axial cylinder viscometer with two different bobs (inner cylinders). A broad range of viscosities could be covered using these devices. The relevant dimensions of the viscometers are given in Table 1. Note that in the Fann viscometer, the outer cylinder is rotated while the inner cylinder is kept stationary whereas in the Haake viscometer, it is the inner cylinder that rotates, and outer cylinder is stationary. In the Fann viscometer, the rotational speed is varied from 0.9 to 600 rpm whereas in the Haake viscometer, the rotational speed is varied from 0.01 to 512 rpm. The viscosity standards of known viscosities were used to calibrate the viscometers. All the viscosity measurements were carried out at room temperature ( ≈ 23 o C ) .
The photomicrographs of particles (TG hollow spheres and solospheres S-32) were taken using a Zeiss optical microscope with transmitted light. From the photomicrographs, the size distribution and mean diameter of the particles were determined. A dilute suspension of particles in deionized water was prepared for observation under the microscope.
The pH measurements were carried out using Fisher Scientific accumet AE150 pH meter. The pH meter was equipped with pH electrode and temperature electrode to measure pH and temperature readings, respectively.

3. Results and Discussion

3.1. Rheology of CNC Dispersions

Figure 2 shows the rheological data for CNC dispersions. At low concentrations of CNC ( ≤ 0.50   w t % ) , the dispersions are Newtonian with constant viscosity. At higher concentrations, the dispersions are non-Newtonian shear-thinning. The viscosity data follows the power-law model:
τ = K γ ˙ n
μ = τ γ ˙ = K γ ˙ n − 1
where τ is shear stress, γ ˙ is shear rate, K is consistency index, and n is flow behavior index. According to the power-law model (Equation (3)), the viscosity versus shear rate relationship is linear on a log-log plot as observed in Figure 2(a) for concentrated CNC dispersions. Figure 2(b) shows the variations of power-law constants ( K   a n d   n ) with CNC concentration. The consistency index K rises sharply with the increase in CNC concentration especially at high concentrations. The flow behavior index n is less than one indicating shear-thinning behavior. The flow behavior index n decreases with the increase in CNC concentration indicating an enhancement of shear-thinning with the increase in CNC concentration.
The shear-thinning behavior in CNC dispersions is due to disaggregation of CNC aggregates and orientation of rod-shaped nanocrystals in the direction of flow [28,29,30,31,32]. As the nanocrystals are very small in size, they undergo aggregation due to Brownian motion. Figure 3 shows the typical size distribution of nanocrystals as determined by DLS measurement. The number average hydrodynamic diameter of the CNC fluctuated with the CNC concentration. Figure 4 shows the plot of number average hydrodynamic diameter of CNC as a function of CNC concentration. The overall average hydrodynamic diameter of the nanocrystals is 6.6 nm.
It should be noted that accurate measurement of the particle size of CNC is difficult due to the following reasons: (a) the particles are rod-shaped (not spherical). The non-spherical shape of particles affects how they scatter light and interact with imaging technique; (b) CNCs tend to aggregate which can distort the observed size distribution; and (c) CNCs consist of a wide range of sizes with varying lengths and widths.

3.2. Rheology of Suspensions of Large Particles (TG Hollow Spheres)

The compositions of suspensions of TG hollow spheres investigated in this study are given in Table 2. The particle concentration ranged from 5 to 50 wt% (6.6 to 57.2 vol%).
The typical photomicrographs of particles are shown in Figure 5. The size distribution is shown in Figure 6. particle size ranged from 10 to 140 μ m with a Sauter mean diameter of 69 μ m.
Figure 7, Figure 8, Figure 9, Figure 10, Figure 11 and Figure 12 show the rheological behavior of suspensions of TG hollow spheres at different volume fractions of particles at a given NCC concentration. The NCC concentration varied from 0.25 to 3.5 wt%. From the figures it is clear that:
  • At low NCC concentrations ( ≤ 1 wt%), the suspensions are Newtonian at low volume fractions of particles. At high volume fractions of particles, shear-thinning is observed.
  • At high NCC concentrations ( ≥ 1.5 wt%), the suspensions are non-Newtonian shear thinning at all volume fractions of particles.
  • The degree of shear thinning increases with the increase in particle volume fraction at any given NCC concentration.
  • All suspensions at different particle volume fractions and different NCC concentrations follow the power-law behavior (see Equations (2) and (3)). The plots of viscosity versus shear rate are linear on a log-log scale.
The power-law constants K and n of suspensions were obtained by fitting the power-law model to the viscosity data. Figure 13 compares the power-law constants of suspensions. Figure 13(a) shows consistency index K as a function of particle volume fraction at different concentrations of NCC whereas Figure 13(b) shows flow behavior index n as a function of particle volume fraction at different concentrations of NCC. Figure 13 reveals the following characteristic of suspensions:
  • At a given NCC concentration, the consistency of suspensions as measured by consistency index K increases with the increase in particle volume fraction.
  • At a fixed particle volume fraction, the consistency of suspension generally increases with the increase in NCC concentration.
  • The suspensions containing NCC are shear-thinning ( n < 1 ) at high volume fraction of particles. The flow behavior index n depends on both NCC and particle concentrations. It generally decreases with the increase in NCC and particle concentrations.

3.3. Rheology of Suspensions of Small Particles (Solospheres S-32)

The compositions of suspensions of Solospheres S-32 investigated in this study are given in Table 3. The particle concentration ranged from 4.7 to 65 wt% (2.3 to 46.7 vol%).
The typical photomicrograph of Solospheres S-32 particles is shown in Figure 14. The size distribution is shown in Figure 15. The particle diameter ranged from approximately 2 to 20 μ m with a Sauter mean diameter of 14 μ m. More than 1000 particles were counted to determine the particle size distribution and the mean diameter. Note that the Solospheres S-32 particles are much smaller in size as compared with TG hollow sphere particles. The Sauter mean diameter of TG hollow spheres was 5 times that of the Solospheres S-32.
Like suspensions of TG hollow sphere particles, the suspensions of Solospheres S-32 particles also followed the power-law behavior, that is, the plots of viscosity versus shear rate were linear on log-log scale and the data could be fitted by the power-law model (see Equations (2) and (3)). Figure 16 compares the power-law parameters ( K and n ) for suspensions of solospheres containing different concentrations of NCC. The consistency index increases with the increase in particle volume fraction at a given NCC concentration. The consistency index also increases with the increase in NCC concentration at a fixed particle volume fraction. At high volume fraction of particles, the suspensions are shear-thinning ( n < 1 ). The degree of shear-thinning increases with the increases in particle volume fraction and NCC concentration. It should be noted that most of the changes occur initially at low volume fractions of particles and then the power-law parameters tend to level off at high particle volume fractions.
Figure 17 compares the consistency index K and flow behavior index n of the two types of suspensions investigated, that is, TG hollow sphere suspensions and Solosphere S-32 suspensions in the absence of any NCC addition, the suspensions of small particles (Solospheres S-32) are much more viscous than the large sized TG hollow sphere suspensions (see Figure 17). Interestingly, the suspensions of small sized particles are also non-Newtonian shear-thinning ( n < 1 ) whereas suspensions of large sized particles are Newtonian over the full range of particle volume fraction investigated. This clearly indicates the suspensions of small particles form aggregates of particles whereas the suspensions of large particles are uniformly dispersed with negligible aggregation.
Figure 18, Figure 19 and Figure 20 compares the consistency index K and flow behavior index n of suspensions of, TG hollow spheres and Solospheres S-32 in the presence of NCC at different concentrations. Interestingly, at low volume fractions of particles, the suspensions of small particles (Solospheres S-32) are still more viscous and shear-thinning than the suspensions of large particles (TG hollow spheres). However, at high volume fraction of particles, both the consistency index K and flow behavior index n of the two types of suspensions (small and large particles) overlap. This clearly indicates that NCC is playing a role in causing aggregation of particles. At high volume fraction of particles, both small sized and large sized suspensions are aggregated due to the presence of NCC and hence the rheological properties of the two suspension systems overlap.

3.4. Effect of Salt on the Rheology of Suspensions

The effect of salt (NaCl) addition on the rheology of NCC solutions (1.5 wt% NCC, without particles) is shown in Figure 21. The NCC solutions become very viscous and highly shear-thinning upon the addition of salt. Note that a sharp jump in the viscosity occurs upon addition of 0.25 wt% salt. With further increase in salt concentration, the rheological properties tend to level off. The influence of salt addition on the power-law parameters is shown in Figure 21(b). After an initial jump in K and a sharp fall in n with the addition of salt, the power-law parameters level off with further increase in salt concentration.
Upon the addition of salt to NCC dispersion, the NCC dispersion tends to gel. The photographs of samples of 1.5 wt% NCC at different salt concentrations are shown in Figure 22. The solution is very clear at 0% salt but becomes milky with the increase in salt concentration due to aggregation of nanocrystals.
Figure 23 shows the effect of salt addition on the rheology of suspensions of TG hollow spheres at two particle concentrations (12 and 25 wt%). The power law parameters consistency index K and flow behavior index n are plotted as a function of salt concentration. For comparison purposes the power law parameters for NCC dispersion (1.5 wt% NCC) without any particles are also plotted. Interestingly the rheology of suspensions is dominated by the rheology of NCC dispersion alone at salt concentrations higher than about 0.3 wt%. The power law parameters of suspensions are almost the same as that of the NCC dispersion without any particles. A similar behavior is exhibited by suspensions of Solospheres S-32 as shown in Figure 24.

3.5. Effect of pH on the Rheology of Suspensions

Figure 25 shows the effect of pH on the rheological behavior of suspensions. Both types of suspensions (TG hollow spheres and Solospheres S-32) show a minimum in the consistency index K under neutral conditions (pH ≅ 7). The consistency of the suspension increases under acidic or alkaline conditions. This observation is consistent with the work of Qi et al. [32] who investigated NCC dispersions without any solid particles. They found that the nanocrystals of NCC undergo severe aggregation under acidic or alkaline conditions causing a large increase in the viscosity of NCC dispersions.

4. Conclusions

The viscous behavior of suspensions thickened by cellulose nanocrystals (referred to as NCC) was investigated experimentally. The effects of cellulose nanocrystal concentration, particle concentration, particle size, salt concentration, and pH on the viscous behavior of suspensions were determined. Based on the experimental work, the following conclusions can be made:
  • The dispersions of cellulose nanocrystals at NCC ≥ 1 wt% are shear-thinning due to disaggregation and orientation of nanocrystals with shear.
  • The suspensions of large size particles (TG hollow spheres, Sauter mean diameter 69 µm) are Newtonian in the absence of cellulose nanocrystals. However, the addition of nanocrystals makes them shear-thinning and more viscous. The degree of shear-thinning increases with the increases in NCC and particle concentrations.
  • The suspensions of small size particles (Solospheres S-32, Sauter mean diameter 14 µm) are shear-thinning at particle volume fractions > 0.1 even in the absence of any nanocrystals. The addition of nanocrystals makes them more shear-thinning and viscous.
  • The addition of salt has a strong influence on the rheology of nanocrystal dispersions and nanocrystal-thickened suspensions. A sharp rise in the consistency index and a large drop in the flow behavior index are observed with the addition of salt.
  • The nanocrystal-thickened suspensions show a minimum in consistency index under neutral condition (pH ≅ 7). The consistency rises substantially with the decrease in pH below 7 and with the increase in pH above 7.

Author Contributions

Conceptualization, R.P.; methodology, K.P. and R.P..; validation, K.P. and R.P.; formal analysis, R.P.; investigation, R.P. and K.P.; resources, R.P.; data curation, R.P.; writing—original draft preparation, R.P.; writing—review and editing, R.P.; visualization, R.P.; supervision, R.P.; project administration, R.P.; funding acquisition, R.P. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by Discovery Grant awarded to R.P. by the Natural Sciences and Engineering Research Council of Canada.

Data Availability Statement

The raw data supporting the conclusions of this article will be made available by the authors on request.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Tadros, T.F. Suspension Concentrates: Preparation, Stability and Industrial Applications; De Gruyter, Berlin, Boston, 2017.
  2. Schramm, L.L. Emulsions, Foams, Suspensions, and Aerosols: Microscience and Applications; Wiley-VCH Verlag, Weinheim, 2014.
  3. Kulshreshtha, A.; Wall, M. Pharmaceutical Suspensions: From formulation development to manufacturing; Springer, London, 2010.
  4. Pal, R. Rheology of Particulate Dispersions and Composites; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
  5. Pal, R. Electromagnetic, Mechanical, and Transport Properties of Composite Materials; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
  6. Stickel, J.J.; Powell, R.L. Fluid mechanics and rheology of dense suspensions. Annu. Rev. Fluid Mech. 2005, 37, 127–149. [Google Scholar] [CrossRef]
  7. Halloran, J.W.; Tomeckova, V. Flow behavior of polymerizable ceramic suspensions as function of ceramic volume fraction and temperature. J. European Ceramic Soc. 2011, 31, 2535–2542. [Google Scholar]
  8. Camargo, I.L.; Morais, M.M.; Fortulan, C.A.; Branciforti, M.C. A review on the rheological behavior and formulations of ceramic suspensions for vat polymerization. Ceramics International 2021, 47, 11906–11921. [Google Scholar] [CrossRef]
  9. Zhou, W.; Li, D.; Wang, H. A novel aqueous ceramic suspension for ceramic stereolithography. Rapid Prototyping Journal 2010, 16, 29–35. [Google Scholar] [CrossRef]
  10. Denn, M.M.; Morris, J.F. Rheology of non-Brownian suspensions. Annu. Rev. Chem. Biomol. Eng. 2014, 5, 203–228. [Google Scholar] [CrossRef] [PubMed]
  11. Guazzelli, E.; Pouliquen, O. Rheology of dense granular suspensions. J. Fluid. Mech. 2018, 852, 1–73. [Google Scholar] [CrossRef]
  12. Pal, R. Modeling of Sedimentation and creaming in suspensions and Pickering emulsions. Fluids 2019, 4, 186. [Google Scholar] [CrossRef]
  13. Pal, R. Rheology of emulsions containing polymeric liquids. In Encyclopedia of Emulsion Technology, Becher, P., Ed.; Marcel Dekker: New York, USA, 1996; Volume 4, pp. 93–263. [Google Scholar]
  14. Prathapan, R.; Thapa, R.; Garnier, G.; Tabor, R.F. Modulating the zeta potential of cellulose nanocrystals using salts and surfactants. Colloids and Surfaces A: Physicochemical and Engineering Aspects 2016, 509, 11–18. [Google Scholar] [CrossRef]
  15. Lu, P.; Hsieh, Y. Preparation and properties of cellulose nanocrystals: rods, spheres, and network. Carbohydrate Polymers 2010, 82, 329–336. [Google Scholar] [CrossRef]
  16. Girard, M.; Vidal, D.; Bertrand, F.; Tavares, J.R.; Heuzey, M. Evidence-based guidelines for the ultrasonic dispersion of cellulose nanocrystals. Ultrasonics Sonochemistry 2021, 71, 105378. [Google Scholar] [CrossRef]
  17. Shojaeiarani, J.; Bajwa, D.S.; Chanda, S. Cellulose nanocrystal-based composites: A review. Composites Part C: Open Access 2021, 5, 100164. [Google Scholar] [CrossRef]
  18. Yang, X.; Biswas, S.K.; Han, J.; Tanpichai, S.; Li, M.; Chen, C.; Zhu, S.; Das, A.K.; Yano, H. Surface and interface engineering for nanocellulosic advanced materials. Advanced Materials 2021, 33, 2002264. [Google Scholar] [CrossRef] [PubMed]
  19. Trache, D.; Hussin, M.H.; Haafiz, M.K.M.; Thakur, V.K. Recent progress in cellulose nanocrystals: sources and production. Nanoscale 2017, 9, 1763–1786. [Google Scholar] [CrossRef] [PubMed]
  20. Aziz, T.; Fan, H.; Zhang, X.; Haq, A.; Ullah, R.; Khan, F.U.; Iqbal, M. Advance study of cellulose nanocrystals properties and applications. J. Polymers and Environment 2020, 28, 1117–1128. [Google Scholar] [CrossRef]
  21. Aziz, T.; Ullah, A.; Fan, H.; Ullah, R.; Haq, F.; Khan, F.U.; Iqbal, M.; Wei, J. Cellulose nanocrystals applications in health, medicine, and catalysis. J. Polymers and Environment 2021, 29, 2062–2071. [Google Scholar] [CrossRef]
  22. Dufresne, A. Nanocellulose processing properties and potential applications. Current Forestry Reports 2019, 5, 76–89. [Google Scholar] [CrossRef]
  23. Vanderfleet, O.M.; Cranston, E.D. Production routes to tailor the performance of cellulose nanocrystals. Nature Reviews/Materials 2021, 6, 124–144. [Google Scholar] [CrossRef]
  24. Panchal, P.; Ogunsona, E.; Mekonnen, T. Trends in advanced functional material applications of nanocellulose. Processes 2019, 7, 1–27. [Google Scholar] [CrossRef]
  25. Zhang, H.; Dou, C.; Pal, L.; Hubbe, M.A. Review of electrically conductive composites and films containing cellulosic fibers or nanocellulose. BioResources 2019, 14, 7494–7542. [Google Scholar] [CrossRef]
  26. Gupta, A.; Mekonnen, T.H. Cellulose nanocrystals enabled sustainable polycaprolactone based shape memory polyurethane bionanocomposites. J.Colloid Interface Sci. 2022, 611, 726–738. [Google Scholar] [CrossRef]
  27. Kinra, S.; Pal, R. Rheology of Pickering emulsions stabilized and thickened by cellulose nanocrystals over broad ranges of oil and nanocrystal concentrations. Colloids Interfaces 2023, 7, 36. [Google Scholar] [CrossRef]
  28. Shafiei-Sabet, S.; Hamad, W.Y.; Hatzikiriakos, S.G. Rheology of nanocrystalline cellulose aqueous suspensions. Langmuir 2012, 28, 17124–17133. [Google Scholar] [CrossRef] [PubMed]
  29. Kadar, R.; Fazilati, M.; Nypelo, T. Unexpected microphase transitions in flow towards nematic order of cellulose nanocrystals. Cellulose 2020, 27, 2003–2014. [Google Scholar] [CrossRef]
  30. Pignon, F.; Challamel, M.; De Geyer, A.; Elchamaa, M.; Semeraro, E.F.; Hengl, N.; Jean, B.; Putaux, J.; Gicquel, E.; Bras, J.; Prevost, S.; Sztucki, M.; Narayanan, T.; Djeridi, H. Breakdown and buildup mechanisms of cellulose nanocrystal suspensions under shear and upon relaxation probed by SAXS and SALS. Carbohydrate Polymers 2021, 260, 117751. [Google Scholar] [CrossRef] [PubMed]
  31. Wu, Q.; Meng, Y.; Wang, S.; Li, Y.; Fu, S.; Ma, L.; Harper, D. Rheological behavior of cellulose nanocrystal suspension: influence of concentration and aspect ratio. J. Appl. Poly. Sci. 2014, 131, 40525. [Google Scholar] [CrossRef]
  32. Qi, W.; Yu, J.; Zhang, Z.; Xu, H.N. Effect of pH on the aggregation behavior of cellulose nanocrystals in aqueous medium. Mater. Res. Express 2019, 6, 125078. [Google Scholar] [CrossRef]
Preprints 108272 g001
Figure 1. AFM image of NCC [27].
Figure 1. AFM image of NCC [27].
Preprints 108272 g001
Preprints 108272 g002
Figure 2. Rheological behavior of CNC dispersions.
Figure 2. Rheological behavior of CNC dispersions.
Preprints 108272 g002
Preprints 108272 g003
Figure 3. Typical size distribution of CNC.
Figure 3. Typical size distribution of CNC.
Preprints 108272 g003
Preprints 108272 g004
Figure 4. Fluctuation of average hydrodynamic diameter of CNC with concentration.
Figure 4. Fluctuation of average hydrodynamic diameter of CNC with concentration.
Preprints 108272 g004
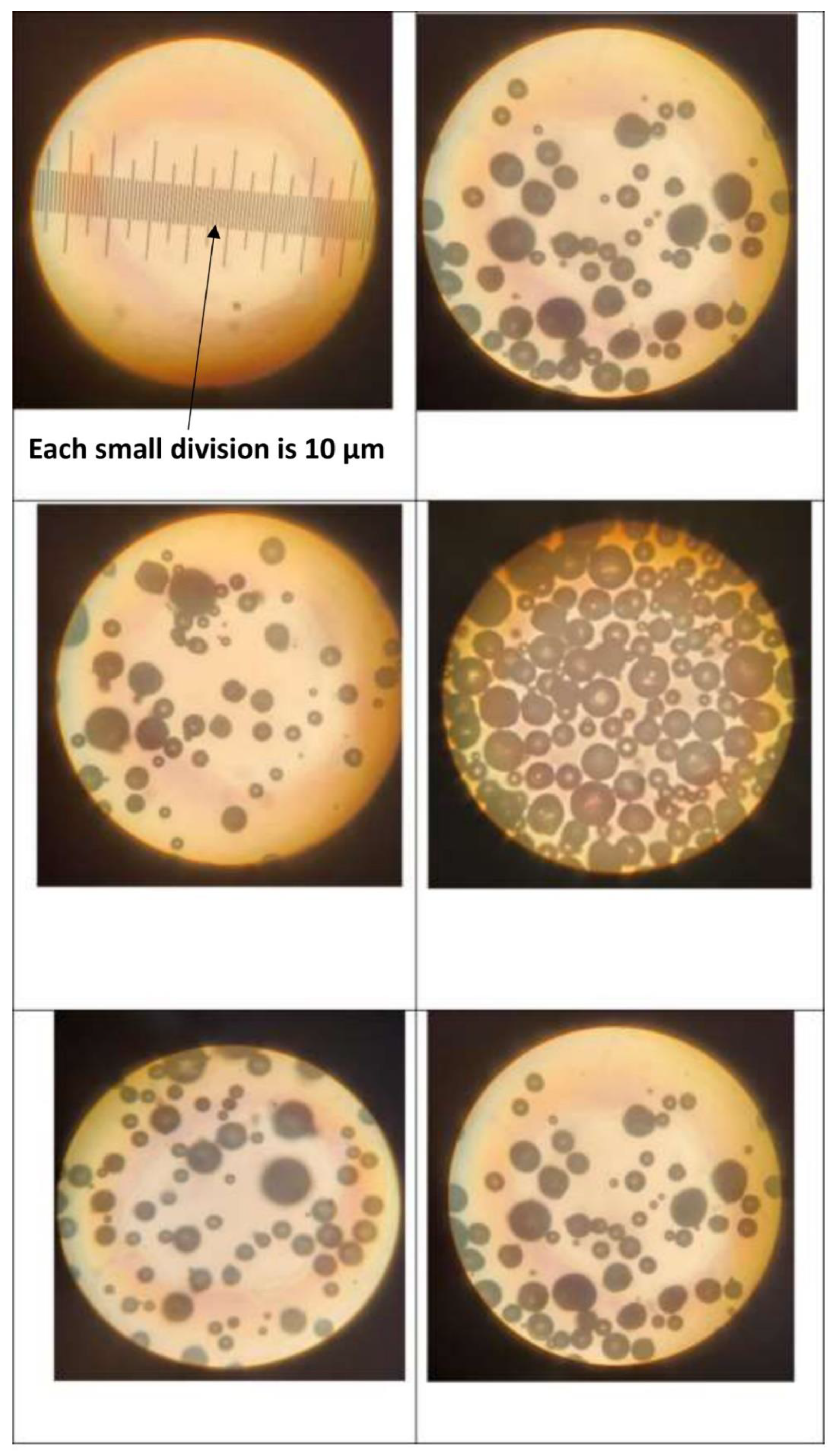
Preprints 108272 g005
Figure 5. Typical photomicrographs of particles (TG hollow spheres).
Figure 5. Typical photomicrographs of particles (TG hollow spheres).
Preprints 108272 g005
Preprints 108272 g006
Figure 6. Size distribution of particles (TG hollow spheres).
Figure 6. Size distribution of particles (TG hollow spheres).
Preprints 108272 g006
Preprints 108272 g007
Figure 7. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 0.25 wt%.
Figure 7. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 0.25 wt%.
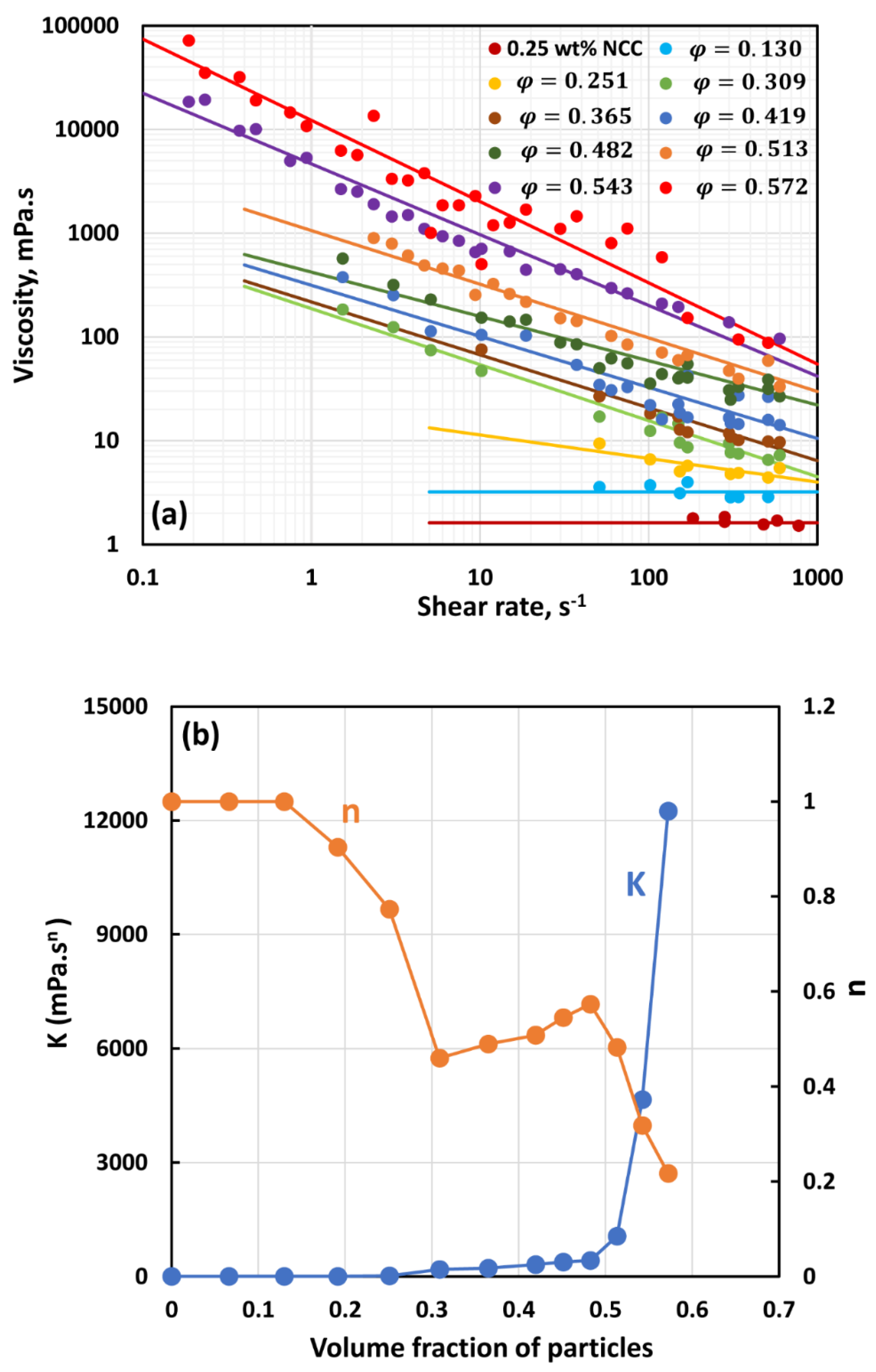
Preprints 108272 g007
Preprints 108272 g008
Figure 8. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 0.50 wt%.
Figure 8. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 0.50 wt%.
Preprints 108272 g008
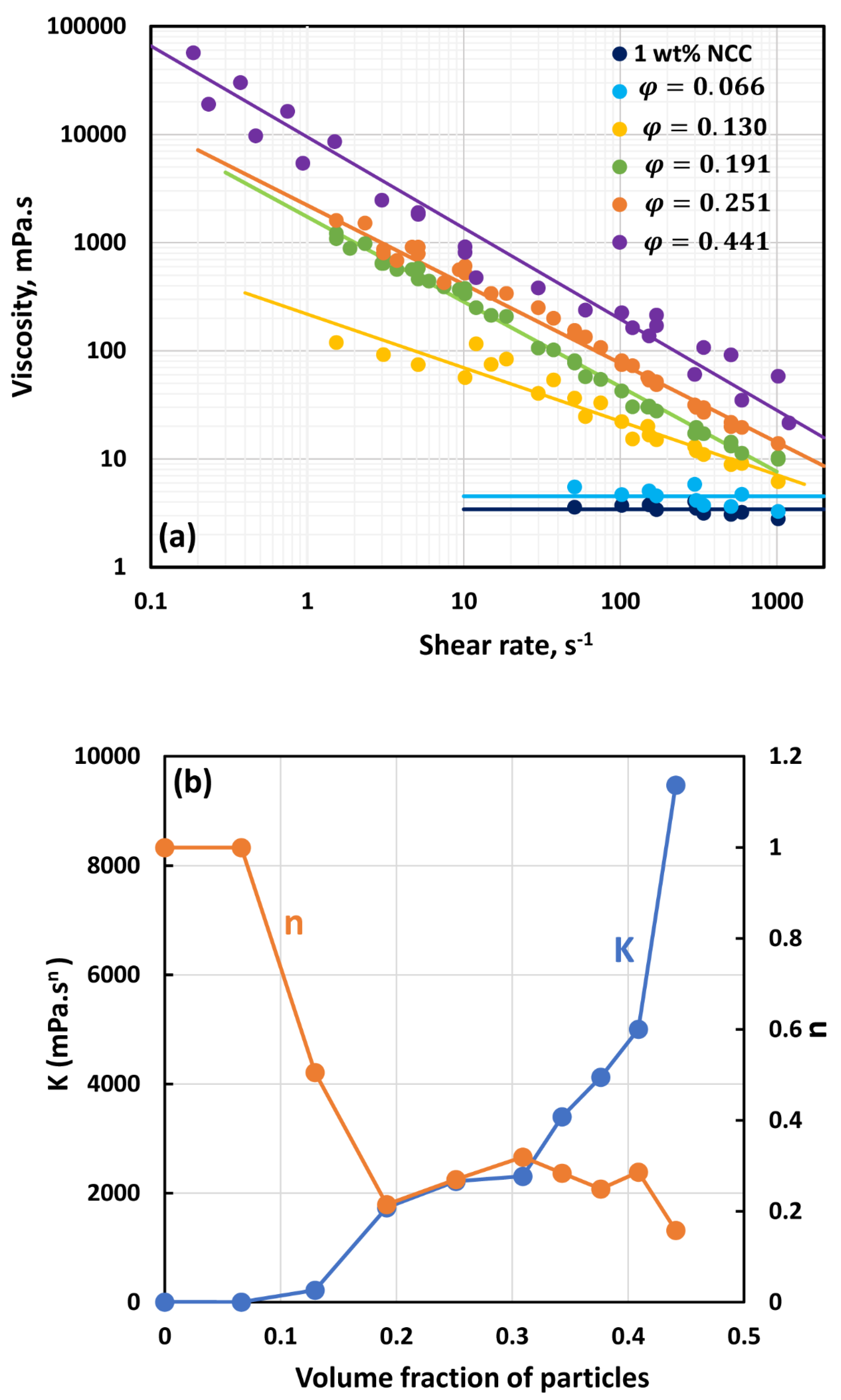
Preprints 108272 g009
Figure 9. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 1.0 wt%.
Figure 9. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 1.0 wt%.
Preprints 108272 g009
Preprints 108272 g010
Figure 10. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 1.5 wt%.
Figure 10. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 1.5 wt%.
Preprints 108272 g010
Preprints 108272 g011
Figure 11. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 2.5 wt%.
Figure 11. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 2.5 wt%.
Preprints 108272 g011
Preprints 108272 g012
Figure 12. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 3.5 wt%.
Figure 12. Flow behavior of suspensions at different volume fractions of particles ( φ ) at a fixed NCC concentration of 3.5 wt%.
Preprints 108272 g012
Preprints 108272 g013
Figure 13. Comparison of power-law constants of suspensions of TG hollow sphere particles at different NCC concentrations: (a) Consistency index K , and (b) Flow behavior index n .
Figure 13. Comparison of power-law constants of suspensions of TG hollow sphere particles at different NCC concentrations: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g013
Preprints 108272 g014
Figure 14. Typical photomicrograph of Solospheres S-32 particles.
Figure 14. Typical photomicrograph of Solospheres S-32 particles.
Preprints 108272 g014
Preprints 108272 g015
Figure 15. Particle size distribution of Solospheres S-32 particles.
Figure 15. Particle size distribution of Solospheres S-32 particles.
Preprints 108272 g015
Preprints 108272 g016
Figure 16. Comparison of power-law constants of suspensions of solospheres S-32 particles at different NCC concentrations: (a) Consistency index K , and (b) Flow behavior index n .
Figure 16. Comparison of power-law constants of suspensions of solospheres S-32 particles at different NCC concentrations: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g016
Preprints 108272 g017
Figure 17. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres with no NCC: (a) Consistency index K , and (b) Flow behavior index n .
Figure 17. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres with no NCC: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g017
Preprints 108272 g018
Figure 18. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 0.5 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Figure 18. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 0.5 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g018
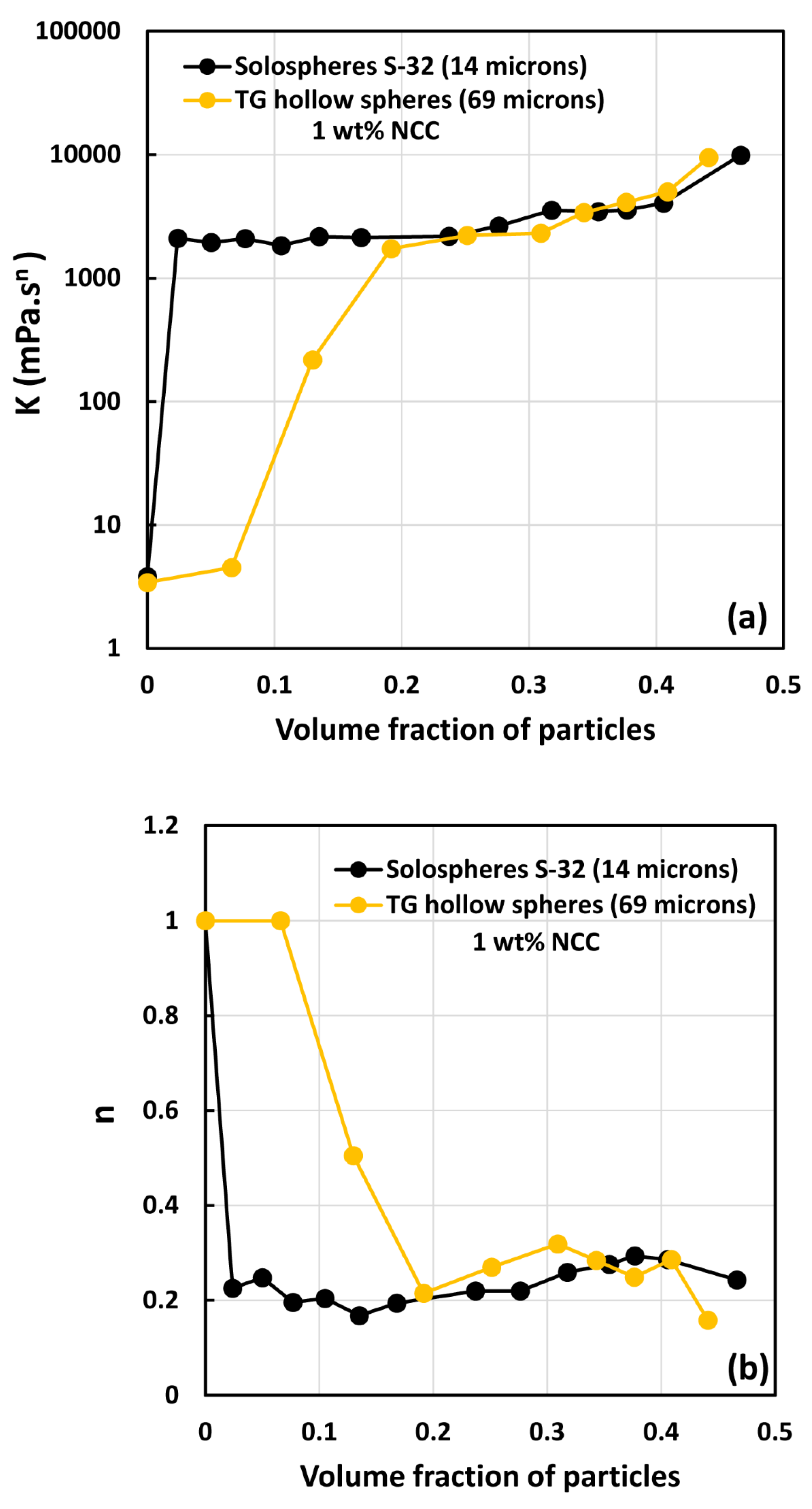
Preprints 108272 g019
Figure 19. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 1 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Figure 19. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 1 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g019
Preprints 108272 g020
Figure 20. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 2.5 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Figure 20. Comparison of power-law constants of suspensions of solospheres S-32 particles with suspensions of TG hollow spheres at NCC concentration of 2.5 wt%: (a) Consistency index K , and (b) Flow behavior index n .
Preprints 108272 g020
Preprints 108272 g021
Figure 21. Effect of salt (NaCl) on the rheology of 1.5 wt% NCC dispersion without any particles (a) Viscosity versus shear rate behavior, and (b) Variation of consistency index K and flow behavior index n with salt concentration.
Figure 21. Effect of salt (NaCl) on the rheology of 1.5 wt% NCC dispersion without any particles (a) Viscosity versus shear rate behavior, and (b) Variation of consistency index K and flow behavior index n with salt concentration.
Preprints 108272 g021
Preprints 108272 g022
Figure 22. Appearance of 1.5 wt% NCC dispersion samples with the addition of salt (NaCl).
Figure 22. Appearance of 1.5 wt% NCC dispersion samples with the addition of salt (NaCl).
Preprints 108272 g022
Preprints 108272 g023
Figure 23. Effect of salt on the power-law constants of suspensions of TG hollow spheres.
Figure 23. Effect of salt on the power-law constants of suspensions of TG hollow spheres.
Preprints 108272 g023
Preprints 108272 g024
Figure 24. Effect of salt on the power-law parameters of suspensions of Solospheres S-32.
Figure 24. Effect of salt on the power-law parameters of suspensions of Solospheres S-32.
Preprints 108272 g024
Preprints 108272 g025
Figure 25. Effect of pH on the power-law parameters of suspensions (a) Suspensions of TG hollow spheres, and (b) Suspensions of Solospheres S-32.
Figure 25. Effect of pH on the power-law parameters of suspensions (a) Suspensions of TG hollow spheres, and (b) Suspensions of Solospheres S-32.
Preprints 108272 g025
Table 1. Relevant dimensions of viscometers used in this study. .
Table 1. Relevant dimensions of viscometers used in this study. .
Viscometer Inner   cylinder   radius ,   R i Outer   cylinder   radius ,   R o Length of inner cylinder Gap-width
Fann 35A/SR-12 (low torsion spring constant) 1.72 cm 1.84 cm 3.8 cm 0.12 cm
Fann 35A (high torsion spring constant) 1.72 cm 1.84 cm 3.8 cm 0.12 cm
Haake Roto- visco RV 12 with MV I 2.00 cm 2.1 cm 6.0 cm 0.10 cm
Haake Roto- visco RV 12 with MV II 1.84 cm 2.1 cm 6.0 cm 0.26 cm
Table 2. Compositions of suspensions of TG hollow spheres investigated in this study.
Table 2. Compositions of suspensions of TG hollow spheres investigated in this study.
CNC Concentration of Matrix Phase (wt%) Solids Concentration of Suspension (wt%) Solids Concentration of Suspension (vol%)
0

0.25		 Ten concentrations: 5, 9.8, 14.4, 19.4, 24.4, 29.8, 34.8, 39.8, 44.8, 49.8
Twelve concentrations: 5, 10, 15, 20, 25, 30, 35, 38, 41, 44, 47, 50
Ten concentrations: 6.6, 12.7, 18.3, 24.4, 30.2, 36.2, 41.7, 47.0, 52.1, 57.1
Twelve concentrations: 6.6, 13.0, 19.1, 25.1, 30.9, 36.5, 41.9, 45.1, 48.2, 51.3, 54.3, 57.2
0.50 Fifteen concentrations: 5, 8, 11, 14, 17, 20, 23, 26, 29, 31, 34, 37, 40, 43,46
Fifteen concentrations: 6.6, 10.4, 14.2, 17.9, 21.5, 25.1, 28.6, 32, 35.4, 37.6, 40.8, 44, 47.2, 50.3, 53.3
1.0 Nine concentrations: 5, 10, 15, 20, 25, 28, 31, 34, 37
Nine concentrations: 6.6, 13.0, 19.1, 25.1, 30.9, 34.3, 37.6, 40.9, 44.1
1.5 Twelve concentrations: 5, 8, 11, 14, 17, 20, 23, 26, 29, 31, 34, 37
Twelve concentrations: 6.6, 10.5, 14.3, 18.0, 21.6, 25.2, 28.7, 32.1, 35.5, 37.7, 40.9, 44.1
2.5 Eleven concentrations: 5, 8, 11, 14, 17, 20, 23, 26, 29.1, 32.1, 35
Eleven concentrations: 6.6, 10.5, 14.3, 18.0, 21.7, 25.2, 28.7, 32.2, 35.6, 38.9, 42.1
3.5 Ten concentrations: 5, 11, 14, 17, 20, 23, 26, 29, 32, 35
Ten concentrations: 6.7, 14.3, 18.1, 21.7, 25.3, 28.8, 32.3, 35.6, 38.9, 42.2
Table 3. Compositions of suspensions of Solospheres S-32 investigated in this study.
Table 3. Compositions of suspensions of Solospheres S-32 investigated in this study.
CNC Concentration of Matrix Phase (wt%) Solids Concentration of Suspension (wt%) Solids Concentration of Suspension (vol%)
0

0.25		 Thirteen concentrations: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60, 65
Thirteen concentrations: 5.9, 10.9, 15.8, 20.8, 25.7, 30.7, 35.6, 40.6, 45.5, 50.5, 56.2, 61.6, 65.3		 Thirteen concentrations: 2.4, 5.0, 7.7, 10.5, 13.6, 16.8, 20.2, 23.9, 27.8, 32, 36.5, 41.4, 46.6
Thirteen concentrations: 2.9, 5.4, 8.1, 11.0, 14.0, 17.3, 20.7, 24.4, 28.3, 32.5, 37.7, 43.1, 47
0.50 Thirteen concentrations: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55, 60, 65
Thirteen concentrations: 2.4, 5.0, 7.7, 10.5, 13.6, 16.8, 20.3, 23.9, 27.8, 32.0, 36.6, 41.4, 46.7
1.0 Thirteen concentrations: 4.9, 10, 15, 19.9, 24.8, 29.9, 39.7, 44.7, 49.6, 53.7, 56.1, 59.1, 64.9
Thirteen concentrations: 2.4, 5, 7.7, 10.5, 13.5, 16.8, 23.7, 27.6, 31.8, 35.4, 37.7, 40.6, 46.6
1.5 Ten concentrations: 4.7, 9.7, 14.7, 20.1, 25.4, 30.7, 36, 41.4, 45.9, 50.1 Ten concentrations: 2.3, 4.8, 7.6, 10.6, 13.9, 17.3, 21.1, 25, 28.6, 32.1
2.5
Nine concentrations: 5.6, 10.6, 15.6, 21.1, 26, 31, 35.4, 40.4, 45.4
Nine concentrations: 2.8, 5.3, 8.1, 11.3, 14.3, 17.6, 20.7, 24.4, 28.3
3.5
Five concentrations: 5.1, 10.1, 15, 20.1, 25.1
Five concentrations: 2.5, 5.1, 7.8, 10.7, 13.8
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated