Mathematical Modeling of the Heat Transfer Process in Spherical Objects With Flat, Cylindrical and Spherical Defects

This article discusses the application of thermal quality control methods for plant tissues. The purpose of the work is to determine the optimal parameters of thermal impact on a spherical-shaped plant control object, ensuring the detection of surface and subsurface defects. The authors of the work proposed mathematical models of the temperature field for a spherical body with defects and a flat sample when exposed to the thermal influence of a pulsed source. As a result of the use of mathematical models, the thermophysical characteristics of plant tissues of varying degrees of disease damage were obtained, which made it possible to simulate the temperature field of the control object and obtain an image of classified tissues. The developed recommendations made it possible to select the optimal parameters of the thermal effect on the test object.

Keywords:

Subject: Computer Science and Mathematics - Applied Mathematics

1. Introduction

Computer vision systems can effectively detect defects in plant tissue through the analysis of images of fruits and vegetables obtained in the range of 8-13 microns [1]. In this case, the main conditions are significant differences in the thermophysical characteristics (TPCs) of defective and defect-free zones of the test object, as well as correctly selected operating parameters of the non-contact thermal non-destructive testing process, providing the required temperature contrast between zones of plant tissue of the object of different quality [2]. To determine the operating parameters of thermal testing, it is necessary to solve the problem of mathematical modeling of heat transfer in heterogeneous spherical bodies containing inclusions (defects) with thermal characteristics that differ from the characteristics of the base material.

The analysis of literary sources makes it possible to conclude that in most cases, the development of mathematical models of heat transfer in bodies made of heterogeneous materials is carried out for objects in the form of a plate, cylinder and sphere. The formulation of the problem of heat transfer in a heterogeneous material in the form of an unlimited plate described in [3], [4], where the problem of freezing of wet soil in a thawed state and having a certain specified distribution of temperature and moisture can be considered classical. At the same time, it should be borne in mind that the process of heat transfer in plant tissues of fruits and vegetables differs significantly from similar processes in the soil due to the different structure of the object. In [3] the coupled problems of stationary thermal conductivity in a non-uniform body consisting of several homogeneous parts, in each of which the thermal conductivity coefficient of the material depends on the temperature distribution in the body, are considered. However, this approach is not applicable for modeling the process of unsteady heat transfer. From this perspective, the study [6] is of particular interest, as it considers a mathematical model of the temperature field in a multilayer inhomogeneous ball consisting of several layers. Each layer at each moment of time is characterized by internal and external radii, temperature, specific heat capacity, density, thermal conductivity, humidity, and heat release density. In this study, dependence was proposed for calculating the layer temperature as a function of the Fourier number. The proposed model can be used to calculate the temperature field in spherical plant objects, for example, apples. However, it does not take into account the influence of defects localized in a limited volume of the object. In [7] a mathematical model of a multilayer cylinder of finite dimensions with a defect in the inner layer is considered. The solution can be carried out using a numerical method. At the same time, the proposed mathematical model makes it possible to determine the temperature fields in a multilayer cylinder during its cooling and in the presence of defects in the inner layer. The presented model can be used to describe the temperature field in cylindrical objects of plant origin (for example, carrots, and onions). In [8] a model of the temperature field in a flat object with cylindrical defects was proposed. In [9] heat transfer in layered rod structures is considered [9]. In [10], based on a statistical model, an artificial neural network model, and a finite element model, the temperature fields of flat objects with various inclusions are studied. In [11], [12] mathematical models of temperature fields on a defect in the form of a crack are considered. It is noted that the spread of heat in an object leads to the appearance of a complex temperature field distribution on its surface, which, by formally fixing and solving the inverse problem of non-stationary thermal conductivity, can determine the position and size of the defect. In [13], [14] models have been developed that make it possible to determine the operating parameters of laser radiation (power, heating spot area, etc.) acting on the surface of a sample according to a non-stationary temperature distribution, providing the necessary temperature contrast between the defective and defect-free zones of the test object, and also determination of their geometric parameters calculated using regression models.

The paper [15] on the development of mathematical models in problems of thermal diagnostics and forecasting the durability of composite structural elements (CSEs) with defects can also be useful in developing algorithms for the shelf life of plant products. The task of predicting durability is considered as calculating the damage parameters of CSEs with diagnosed defects under the influence of external mechanical factors for a given time, as well as calculating the limiting time to failure. The study also contains a mathematical formulation of the geometric inverse problem of thermal conductivity for recognizing three-dimensional shapes and types of defects in CSEs and a numerical method for solving it.

In [16-19], the process of thermal conductivity in a heterogeneous medium is modeled, which is a homogeneous matrix with cylindrical inclusions separated from it by a contact layer. Also in these papers, a study was carried out of the influence of the thickness and material of the layer on the effective properties of the matrix-layer-inclusion system and temperature profiles were obtained for configurations with different concentrations of inclusions and the surface layer, as well as for different thermal conductivity coefficients of the components.

Thus, a review of scientific and technical literature showed, on the one hand, that existing mathematical models of heat transfer in heterogeneous media do not generally take into account the finite dimensions of the test object and are designed for the presence of inclusions of a certain geometric shape. On the other hand, their numerical implementation requires significant computing resources due to their complexity and, as a rule, nonlinearity.

This study aims to determine the optimal parameters of thermal impact on a spherical-shaped plant test object, ensuring the detection of surface and subsurface defects. To achieve this goal, the following problems were solved: mathematical modeling of temperature fields in a spherical object containing defects of flat, cylindrical and spherical shapes; mathematical modeling of temperature fields in a flat sample when exposed to a pulsed heat source; numerical modeling of temperature fields in spherical objects with defects located on their surface and inside when the test object is exposed to a heat flow of constant power.

2. Materials and Methods

2.1. A Mathematical Model of the Temperature Field of a Spherical Object with Defects

When writing mathematical models in the form of boundary value problems of thermal conductivity, which make it possible to calculate the temperature fields of test objects (TO) (or their local areas), the following assumptions were made. Test objects of plant origin, such as apples, potatoes, oranges, lemons have a spherical shape (Figure 1). The defects they contain, including, rot, bruises, hail holes, scab, are close in shape to the shape of the surface area of the object near which they are located. Let us assume that V₁, V₂, V₃, V₄ are a set of points x, y, z, belonging to healthy 1 (Figure 1) tissues of the test object, and tissues containing defects of flat shape 2 (thickness H), cylindrical shape 3 or spherical shape 4 (radius R), respectively. Defects are located at a distance h from the surface of the object. Similarly, S₁, S₂, S₃, S₄ are a set of points x, y, z belonging to the outer surface of healthy tissues and tissues damaged by defects of the corresponding shape. The initial temperature T₀ of plant tissues will be considered constant and uniformly distributed throughout the volume of the test object. When the test object is exposed to a heat flux from a heat source, the change in temperature T on the surface and inside the object does not exceed 3…5°С. With such a change in the surface temperature of the test object, the thermophysical properties of plant tissues can be considered independent of temperature and constant over time.

The mathematical model of the temperature field of a spherical body containing all three types of defects under consideration, in the general case, can be written in the form of a boundary value problem of thermal conductivity [20]:

c_{i} ρ_{i} \frac{\partial T_{i} (x, y, z, τ)}{\partial τ} = d i v [λ_{i} \cdot g r a d T_{i} (x, y, z, τ)],

(1)

i = \bar{1, 4}, τ > 0, x, y, z \in V_{i} T_{i} (x, y, z, 0) = T_{0} = c o n s t,

(2)

{λ_{1} \frac{\partial T_{1} (x, y, z, τ)}{\partial n}|}_{x, y, z \in S_{1}} = q (τ) = \{\begin{matrix} q = c o n s t, 0 < τ < τ_{1}, \\ 0, τ > τ_{1} \end{matrix}

(3)

\{\begin{matrix} {T_{1} (x, y, z, τ)|}_{x, y, z \in S_{i + 1}} = {T_{i + 1} (x, y, z, τ)|}_{x, y, z \in S_{i + 1}} \\ {λ_{1} \frac{\partial T_{1} (x, y, z, τ)}{\partial n_{i + 1}}|}_{x, y, z \in S_{i + 1}} = {λ_{i + 1} \frac{\partial T_{i + 1} (x, y, z, τ)}{\partial n_{i + 1}}|}_{x, y, z \in S_{i + 1}} \end{matrix}, i = \bar{1, 3},

(4)

{\frac{\partial T_{1} (x, y, z, τ)}{\partial x}|}_{x = 0} = {\frac{\partial T_{1} (x, y, z, τ)}{\partial y}|}_{y = 0} = {\frac{\partial T_{1} (x, y, z, τ)}{\partial z}|}_{z = 0},

(5)

where λ, c, ρ, a are temperature, thermal conductivity, specific heat capacity, density and thermal diffusivity of plant tissues, respectively; τ is time; n is a single vector normal to the surface S of the corresponding region of the test object; q is specific heat flux density from an external heat source.

Note that taking into account the insignificant, relative to the initial values, temperature difference of plant tissues during thermal control, instead of the boundary condition specified by the Stefan-Boltzmann law, we use the boundary condition of the second kind (3), where

τ_{1}

is duration of heat exposure on the test object when diagnosing defects.

When mathematically describing the temperature field of a spherical body with defects 1', 2', 3', located on its surface, we supplement the boundary condition (3) with the equations

{λ_{i} \frac{\partial T_{i} (x, y, z, τ)}{\partial n_{i}}|}_{x, y, z \in S_{i}} = q (τ), i = \bar{2, 4} .

(3')

When modeling the temperature field of objects of plant origin that have a spherical shape and contain internal and external defects, it is necessary to know the thermophysical characteristics of the corresponding plant tissues. To study TPCs, the method described below is proposed.

2.2. A Mathematical Model of the Temperature Field in a Flat Sample when Exposed to a Pulsed Heat Source

To determine the TPCs of plant tissues, three samples 1-3 were cut out from the spherical body under study (Figure 2), having the shape of plates with a thickness l. A low-inertia flat heater 4 was placed between samples 2 and 3. The main junction of a differential thermocouple 5 was installed between samples 1 and 2, and an additional junction of the same thermocouple was installed on the lower surface of sample 3.

When an electric voltage pulse is applied to the heater, the temperature field in sample 2 can be written in the form of a boundary value problem

\frac{\partial T (x, τ)}{\partial τ} = a \frac{\partial^{2} T (x, τ)}{\partial x^{2}}, τ > 0, 0 < x < \infty,

(6)

T (x, 0) = T_{0} = 0,

(7)

- λ \frac{\partial T (0, τ)}{\partial x} = q [h (τ) - h (τ - τ_{2})],

(8)

T (\infty, τ) = T_{0} = 0,

(9)

where T(x, τ) is temperature of the sample under study in the plane with coordinate x at time τ, counted from the moment the thermal pulse begins to be supplied; T₀ is the initial temperature; q is the heat flux supplied to the sample over a period of time

0 < τ \leq τ_{2}

; h(τ), h(τ-τ₂) are unit asymmetric step functions specified by the relations

h (τ) = \{\begin{matrix} 0, τ < 0, \\ 1, τ \geq 0; \end{matrix} h (τ - τ_{u}) = \{\begin{matrix} 0 τ < τ_{2}, \\ 1 τ \geq τ_{2}; \end{matrix}

(10)

τ₂ is duration of the thermal pulse when determining TPCs.

The solution to the boundary value problem (6)-(9) has the form

T (x, τ) - T_{0} = \frac{2 q}{λ} \cdot f (x, τ, τ_{2}, a),

where

f (x, τ, τ_{2}, α) = \{\begin{matrix} \sqrt{α τ} \cdot i e r f c (\frac{x}{2 \sqrt{α τ}}) i f 0 < τ \leq τ_{2}, \\ \sqrt{α τ} \cdot i e r f c (\frac{x}{2 \sqrt{α τ}}) - \sqrt{α (τ - τ_{2})} \cdot i e r f c (\frac{x}{2 \sqrt{α (τ - τ_{2}}}) i f τ > τ_{2}, \end{matrix}

i e r f c (u) = \int_{u}^{\infty} e r f c (W) d W = \frac{1}{\sqrt{π}} e^{- u^{2}} - u e r f c (u)

is a special function that is an integral of the function

e r f c (W) = 1 - \erf (W);

\erf (W) = \frac{2}{\sqrt{π}} \int_{0}^{W} e^{- W^{2}} d W

is Gaussian error function.

For time

τ \geq τ_{2}

the above solution will take the form

T (x, τ) - T_{0} = \frac{q x}{λ} [\frac{i e r f c [U (τ)]}{U (τ)} - \frac{i e r f c [U (τ - τ_{2})]}{U (τ - τ_{2})}],

(11)

where

U (τ) = \frac{x}{2 \sqrt{α τ}}

U (τ - τ_{2}) = \frac{x}{2 \sqrt{a (τ - τ_{2})}}

are dimensionless functions depending on

x, τ, τ_{2}, a,

and

U (τ - τ_{2}) = \frac{x}{2 \sqrt{a (τ - τ_{2})}} = \frac{x}{2 \sqrt{a τ \frac{τ - τ_{2}}{τ}}} = U (τ) \sqrt{\frac{τ}{τ - τ_{2}}}

From the analysis of expression (11) it is clear that at some point in time

τ = τ_{m a x}

the change in temperature difference

[T (x, τ) - T_{0}]

reaches its maximum value

[T_{m a x} - T_{0}] = [T (x, τ_{m a x}) - T_{0}]

. And at the specified point in time

τ = τ_{m a x}

corresponds to a certain value of the dimensionless function

U^{m} = U (τ_{m a x}) = \frac{x}{2 \sqrt{α τ_{m a x}}}

3. Results

The algorithm for conducting the experiment and determining the desired TPCs provides that during time τ₂a constant power P is supplied to a flat electric heater of area S and the time change in temperature difference T(l,τ) –T₀ is recorded using a signal from a differential thermocouple. Based on the experimental data obtained, the maximum value of the temperature difference T_max–T₀ is found and the experiment is completed when the condition (T(l,τ)–T₀)/(T_max–T₀)>0.8..0.9 is met. The experimental data obtained are used to determine thermal conductivity and thermal diffusivity. To do this, for a set of values λ_х, а_х, selected from the ranges а_min<а_х<а_max, λ_min<λ_х<λ_max in which the required TPCs may lie, determine the values of the functional

F (a_{x}, λ_{x}) = \sum_{i = 1}^{N} {[T_{t} (l, τ_{i}) - T (l, τ_{i})]}^{2}

, where T_t (l, τ_i) is theoretical temperature calculated at times τ_i using model (11) for given TPCs. The pair

a_{x}, λ_{x}

for which F

\to

min is taken as the desired TPCs.

Using the described algorithm, TPCs of plant tissues of fruits and vegetables were obtained. As an example (Table 1) shows the mathematical expectation M and standard deviation D of the results of five measurements of the TPCs of healthy and disease-affected potato plant tissues. Using the found TPC values and the mathematical ones described above, modeling of temperature fields in spherical objects with defects of various shapes was performed.

The example shown in Figure 3 shows an ellipsoid-shaped object with dimensions of the major and minor semi-axes of 64..70 mm and 23..65 mm, respectively. The defect has a spherical shape and is located at a depth h of up to 3 mm below the surface. The ratio of the volume of defect V₃ to the total volume V₀ of the simulated object was in the range of 1..3%. Calculations were carried out at the initial temperature of the object T₀=293 К. On the external surface of the test object, a heat flux q with a specific power of 50...3000 W/m² was set, acting for a time τ_m from the range of 1..5 s.

Figure 4 shows, as an example, the calculated dependences of the maximum temperature gradient ∆T_max in the region of a spherical defect of the “dry rot” type when exposed to heat flux q for 1..5 seconds.

The obtained calculation data make it possible, depending on the sensitivity of the thermal imaging equipment used for thermal inspection, to select the minimum power of thermal exposure and its duration, ensuring defect recognition. For example, as it is shown in Figure 4, it is clear that when a defect occurs at a depth of up to 3 mm at q = 300 W/m² and τ₁ = 1 s, a temperature gradient of about 0.5 ⁰С will be observed in the region where the defect occurs, which is sufficient for detection by a thermal imager with a sensitivity of 0.05 ⁰С.

The developed recommendations made it possible to select the optimal parameters of thermal impact on the test object. As an example, Figure 5 shows photographs of defective potatoes obtained in the visible and infrared regions of the radiation spectrum when objects were exposed to a heat flux with a density of 300 W/m² for a time of 2..4 s.

The analysis of the data obtained showed that when choosing the parameters of thermal exposure according to the recommendations outlined above, in 95% of cases it is possible to identify potato defects such as mechanical damage (Figure 5 a, b), cracks (Figure 5 c), mold (Figure 5 d), late blight (Figure 5 e).

4. Conclusions

When thermally inspecting defects in plant-based test objects, there are risks of thermal damage to plant tissue due to prolonged or high-intensity heat exposure. On the other hand, insufficient power of thermal impact may lead to the fact that some defects, especially those located under the surface of test objects, will not be detected. Therefore, it is necessary to determine the optimal parameters of thermal exposure, in particular, exposure time and power of radiation sources. To solve these problems, this study carried out mathematical modeling of the temperature fields of test objects of spherical shape, which many fruits and vegetables have. During the modeling process, we considered cases where defects of flat, cylindrical and spherical shapes are located on the surface and under the surface of the test object. When modeling the temperature field in a test object, information about the thermophysical characteristics of plant tissues is required, for which a mathematical model of the method and an algorithm for their experimental determination are proposed. The analysis of the obtained results of mathematical modeling made it possible to give recommendations on the selection of optimal values for the duration of thermal exposure on the test object and the heat flux density from an external source, providing the ability to recognize defects by thermal imagers with different sensitivities.

Author Contributions

P.B., A.G., A.E., S.P. and H.A.Z.: conceptualization, software, data curation, writing—original draft preparation, writing—review and editing, supervision, funding acquisition; P.B., V.Y. and S.B.: validation, formal analysis, investigation, visualization, writing—original draft preparation. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by financial support from the Ministry of Science and Higher Education of the Russian Federation within the framework of the project “Development of a robotic complex of ground and air unmanned platforms for use in agricultural technologies” (FEMU-2024-0008).

Data Availability Statement

Not applicable.

Conflicts of Interest

The authors declare no conflicts of interest.

References

Samanian, N.; Mohebbi, M. Thermography A New Approach in Food Science Studies: A Review. MOJ Food Process. Technol., vol. 2, no. 3, 2016, pp. 110-119. [CrossRef]
Ballester, C.; Jiménez-Bello, M. A.; Castel, J. R.; Intrigliolo, D. S. Usefulness of thermography for plant water stress detection in citrus and persimmon trees. Agric. For. Meteorol., vol. 168, 2013. [CrossRef]
Zhao, L.; Gray D., M.; Male D., H. Numerical analysis of simultaneous heat and mass transfer during infiltration into frozen ground, J. Hydrol., vol. 200, no. 1–4, 1997, pp 345-363. [CrossRef]
Lykov, A.V. Teoriya teploprovodnosti [Theory of thermal conductivity]. Moscow: Vysshaya shkola., 1967, pp 600 (in Russ.).
Zarubin, V.S.; Rodikov, A.V. Mathematical simulation of the temperature state of an inhomogeneous body. High Temp 45, 2007, pp 243–254. doi.:10.1134/S0018151X07020150.
Kurmachev, YU. F. Matematicheskoye modelirovaniye teplovogo polya mnogosloynogo neodnorodnogo shara pri nestatsionarnom rezhime [Mathematical modeling of the thermal field of a multilayer inhomogeneous ball in an unsteady mode], Science & education scientific edition of Bauman MSTU, no. 3, 2007. Available online: https://cyberleninka.ru/article/n/matematicheskoe-modelirovanie-teplovogo-polya-mnogosloynogo-neodnorodnogo-shara-pri-nestatsionarnom-rezhime/viewer.
Sinitsyn, N. N.; Shestakov, I. V. Matematicheskoye modelirovaniye temperaturnogo polya broni miksera s uchetom defekta na vnutrenney poverkhnosti [Mathematical modeling of the temperature field of mixer armor taking into account a defect on the inner surface], Cherepovets State University Bulletin, vol. 4, pp. 118–120, 2008.
Sinitsyn, N.N.; Makonkov, A. V.; Belozor, M. YU. Modelirovaniye nestatsionarnogo temperaturnogo polya asfal’tovogo pokrytiya s napolnitelem iz rezin [Modeling of a non-stationary temperature field of asphalt pavement with rubber filler], Modern materials, equipment and technology Proceedings of the 4th International Scientific and Practical Conference, pp. 400–403, 2014.
Stankevich, I. V. Matematicheskoye modelirovaniye temperaturnogo sostoyaniya prostranstvennykh sterzhnevykh konstruktsiy iz neodnorodnykh materialov [Mathematical modeling of the temperature state of spatial rod structures made of inhomogeneous materials], Symbol of Science, vol. 13, no. 1, pp. 53–57, 2016.
Li, J.; Yang, Y.; Liu, M. Research progress in the prediction of heat transfer properties of fabrics based on structural characteristics, Adv. Text. Technol., vol. 30, no. 1, 2022. [CrossRef]
Kotel’nikov, V. V. Matematicheskoye modelirovaniye protsessa obrazovaniya temperaturnogo polya na defekte v vide treshchiny v oblasti kontsentratora napryazheniya [Mathematical modeling of the process of formation of a temperature field on a defect in the form of a crack in the region of a stress raiser], Occupational Safety in Industry, no. 5, pp. 51–58, 2008. (in Russ.).
Rittel, D. Thermomechanical aspects of dynamic crack initiation, Int. J. Fract., vol. 99, no. 3, 1999, pp. 201–212. [CrossRef]
Divin, A. G.; Karpov, S.V. Using Laser Point Scanning Thermography for Quality Monito- ring of Products Made of Composite Materials. Engineering Technologies and Systems, Eng. Technol. Syst., vol. 34, no. 1, 2024, pp. 145–163. [CrossRef]
Divin, A. G.; Ponomarev, S.V. Application of Laser Scannung Thermography and Regression Analysis to Determine Characteristics of Defects in Polymer Composite Materials, Russ. J. Nondestruct. Test., vol. 60, no. 1, 2024. [CrossRef]
Nikolaev, A. A. Mathematical modeling in problems of thermal diagnostics and forecasting the durability of composite structural elements with defects. Tver: dis...cand. phys. and math.: 05.13.18 Mathematical modeling, numerical methods and software packages, 2010, pp.
Savatorova, V. L.; Talonov, A. V.; Vlasov, A. N. Homogenization of thermoelasticity processes in composite materials with periodic structure of heterogeneities, ZAMM Zeitschrift fur Angew. Math. und Mech., vol. 93, no. 8, 2013, pp. 575-596. [CrossRef]
Vlasov, A. N.; Savatorova, V. L.; Talonov, A. V. Asymptotic averaging technique for heat conduction problems with phase transitions in layered media, J. Appl. Mech. Tech. Phys., vol. 36, no. 5, 1995, pp. 773–780. [CrossRef]
Savatorova, V. L.; Talonov, A. V. Heat transfer in a composite material with structural hierarchy, Compos. Mech. Comput. Appl., vol. 12, no. 2, 2021, pp. 45-59. [CrossRef]
Dmitriev, O. S.; Zhyvenkova, A. A.; Dmitriev, A. O. Data-measuring device to determine the thermophysical properties of polymer composites: information support part, E3S Web of Conferences 402, 11016,2023. [CrossRef]
Ponomarev, S.V.; Divin, A.G.; Ponomareva, E.S. Mathematical Models of Temperature Fields of Potato Tubers with Surface and Internal Defects. Advanced Materials & Technologies, no 4, 2017, pp. 065-075. [CrossRef]

Figure 1. Physical model of spherical objects with defects.

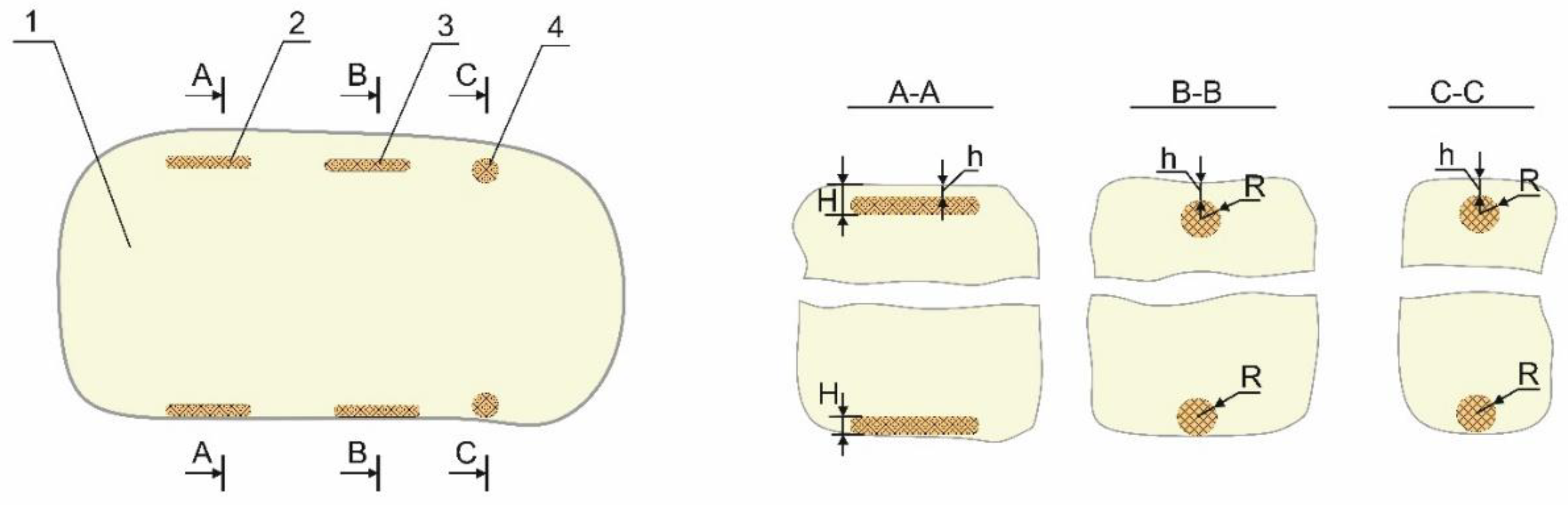
Figure 2. A physical model of the measuring device.

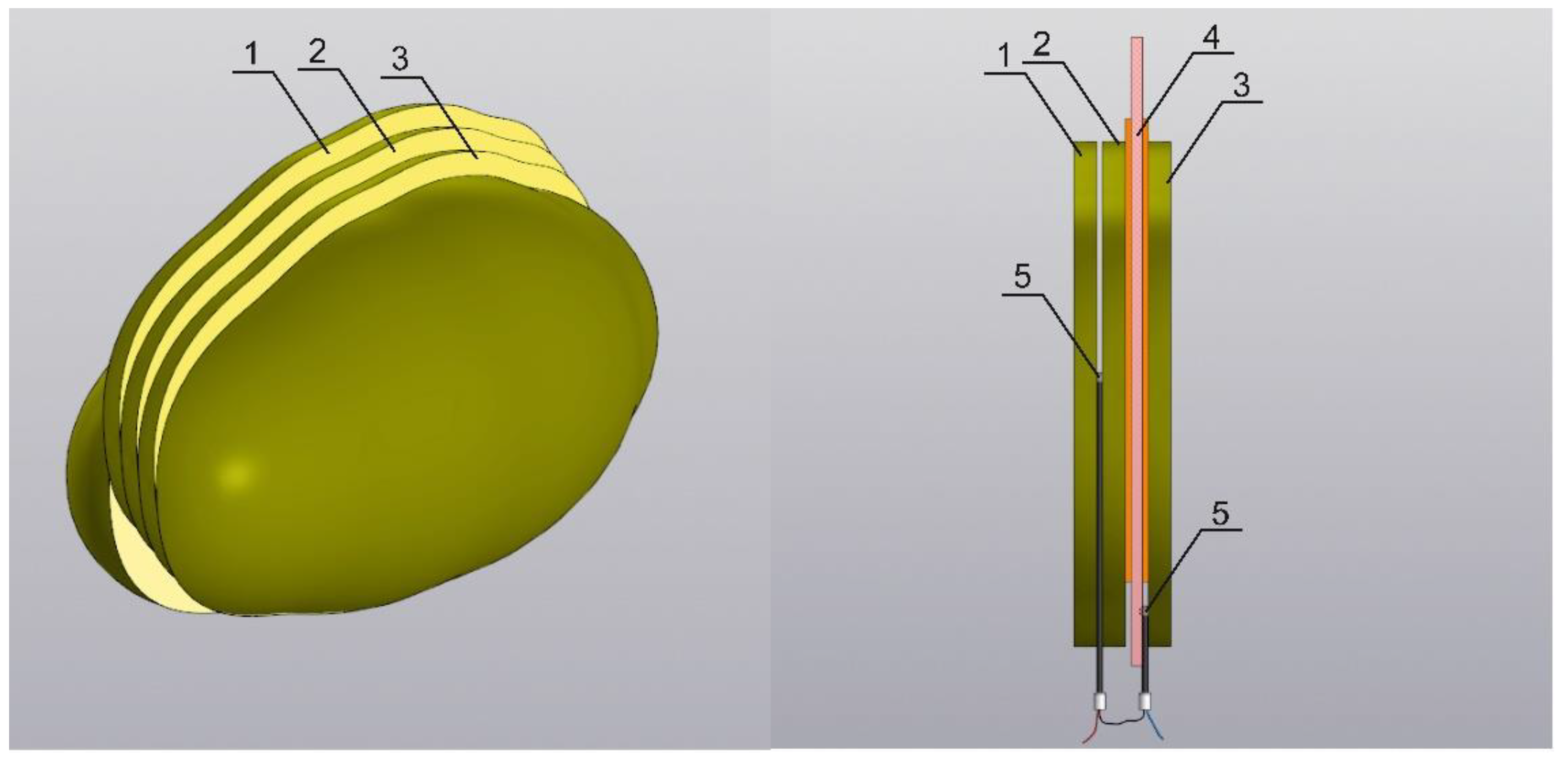
Figure 3. A spherical object with a subsurface spherical defect (a) and the temperature field on its surface in the defect area (b).

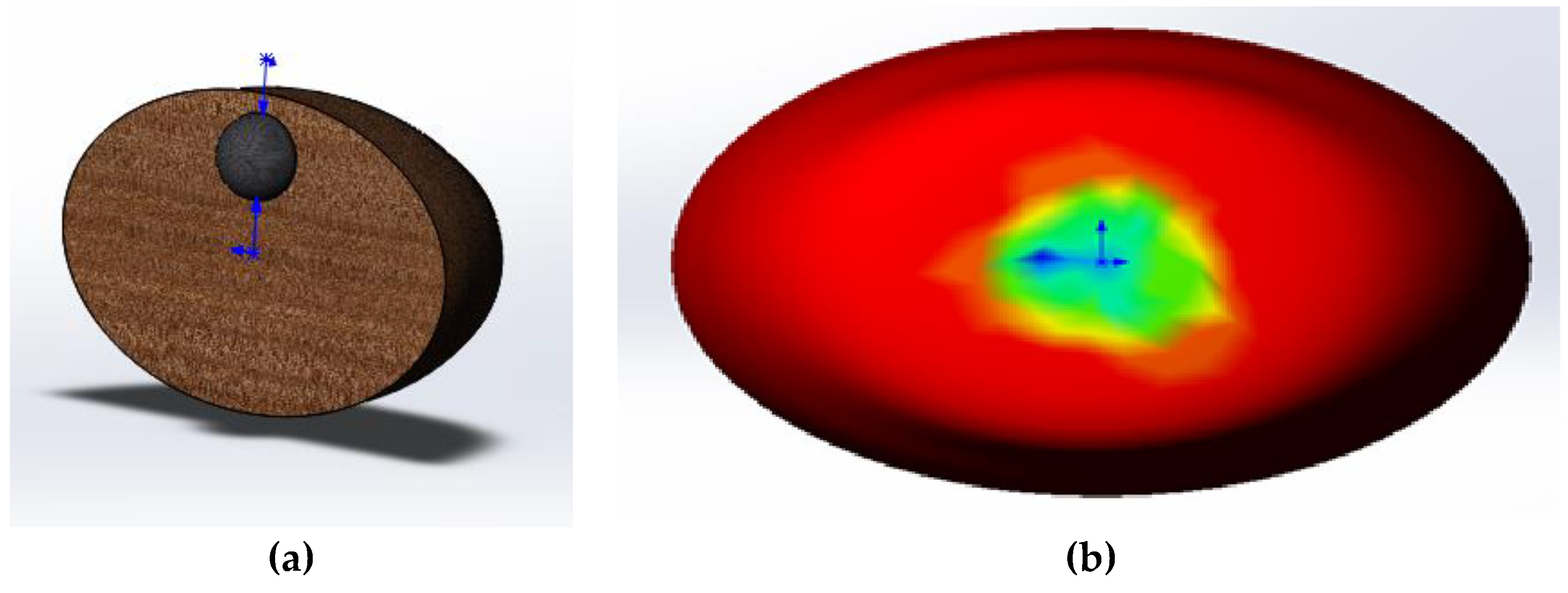
Figure 4. Dependency graphs |∆T_max|=f(q) for spherical subsurface “dry rot” defect.

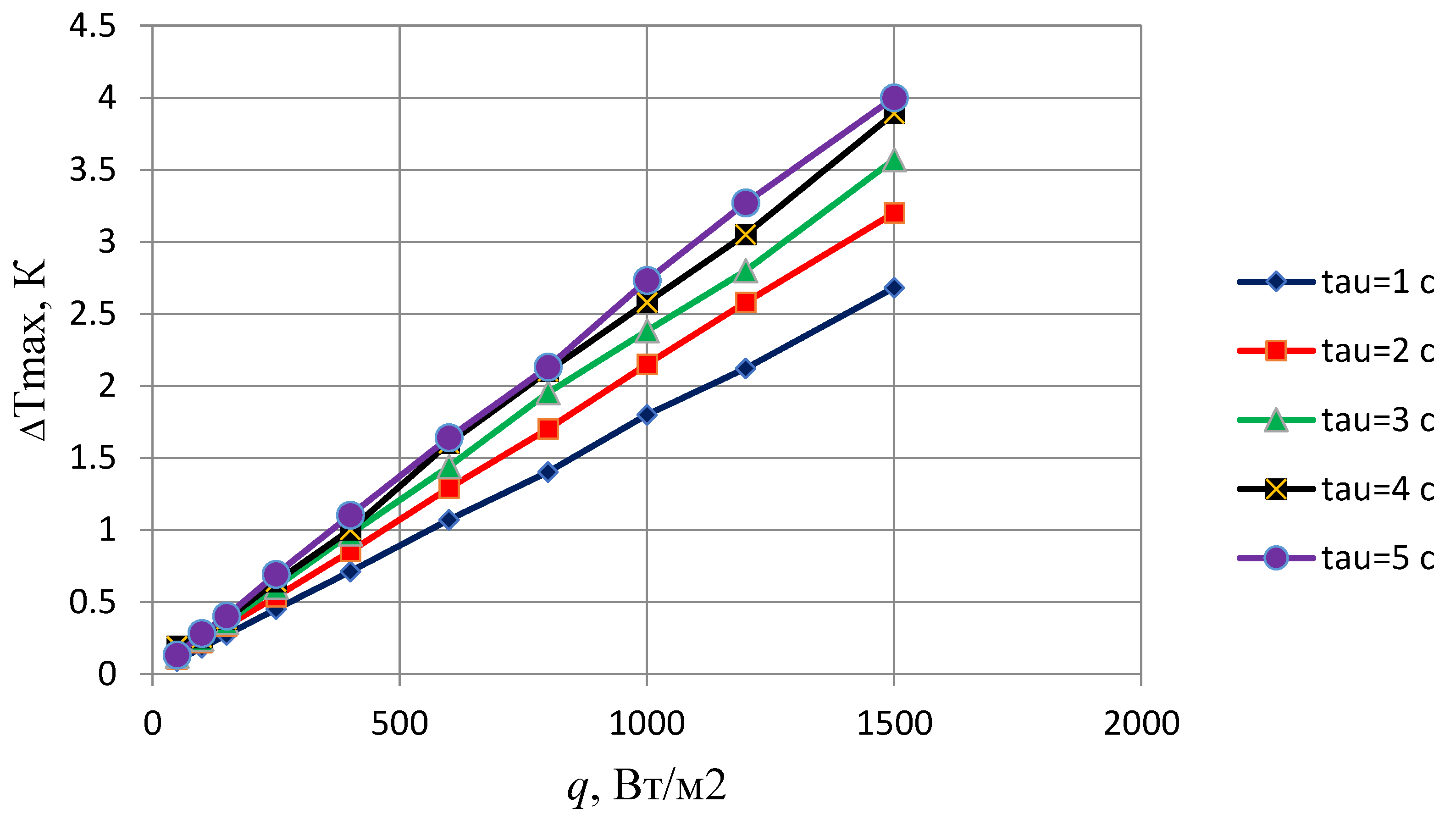
Figure 5. Photographs of defective potato tubers in the infrared and visible regions of the spectrum: ( a ) mechanical damage, ( b ) mechanical damage, ( c ) cracks, ( d ) mold, ( e ) late blight.

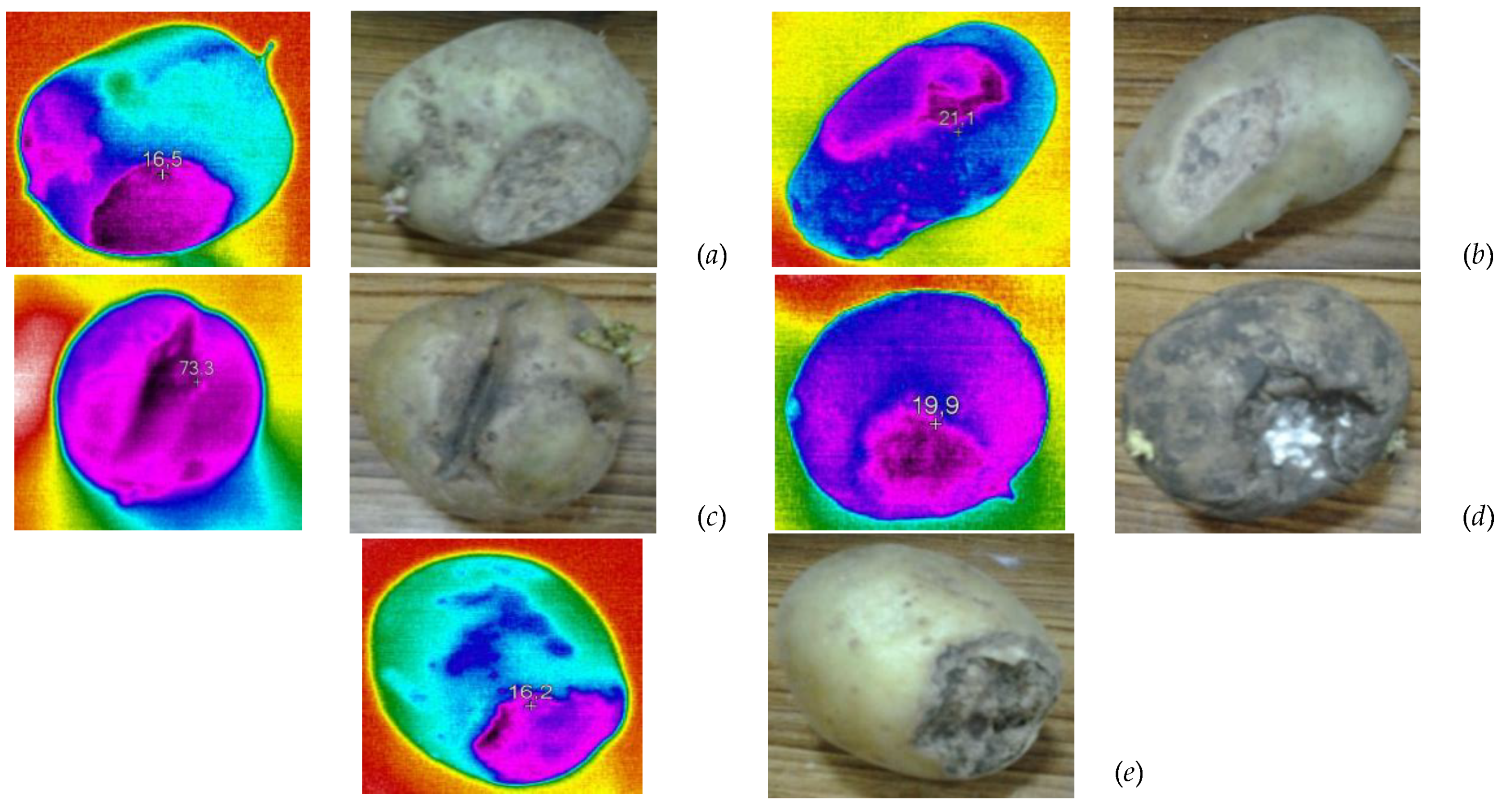
Table 1. TPCs of plant tissues of fruits and vegetables.

Test sample	λ, W/(m⋅K)	a, ( ×10⁷) m²/s	сρ, ( ×10³) kJ /(m³⋅K)
М (healthy tissue)	0.507	1.417	3.56
D (healthy tissue)	0.010	0.022	0.011
М (late blight tissue)	0.550	1.420	3.800
D (late blight tissue)	0.009	0.046	0.017
М (dry rot tissue)	0.384	1.272	3.015
D (dry rot tissue)	0.007	0.053	0.178

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer