Preprint
Article
A Choreographic Approach to Golf Swing Dynamics: Bridging Biomechanics and Laban Movement Analysis
Altmetrics
Downloads
143
Views
111
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
13 June 2024
Posted:
13 June 2024
You are already at the latest version
Alerts
Abstract
This study explores the application of the Laban/Bartenieff Movement System (® LBMS), particularly its Effort component, to analyze the biomechanical dynamics of the golf swing. Focusing on the initiation of the downswing, this paper investigates how the instantaneous screw axis (ISA) with rotational and linear motions contributes to subsequent movements, such as the hip turn. We propose that the physical forces generated by the golf club, characterized by mass moment inertia, extend beyond the body's boundaries, such as through the hand grip. A skilled golfer’s ability to 'allow' these forces to influence body dynamics without opposing them is analyzed through the lens of LBMS's Free Flow Effort, suggesting an indulgent and expansive use of movement energy.
Keywords:
Subject: Engineering - Bioengineering
1. Introduction
Golf is a sport that requires a complex interplay of physical, technical, and strategic skills. Among these, the biomechanical analysis of the golf swing stands out due to its critical role in performance enhancement and injury prevention. The golf swing is a dynamic movement that involves the entire body in a coordinated sequence, with the downswing phase being particularly crucial for the generation of speed and power. Traditionally, research in this area has focused on kinematic and kinetic aspects, such as body alignments, joint angles, and the forces exerted during the swing [1,2]. Researchers have reported on the kinematics and kinetics of the golf swing in terms of the relative contribution of each of the anatomical structures that are involved [3,4,5,6,7,8]. However, these mechanical descriptions often do not fully capture the qualitative aspects of movement fluidity and efficiency that distinguish skilled golfers.
Laban Movement Analysis offers a rich framework for the qualitative analysis of human movement. Developed by Rudolf Laban and colleagues, LMA describes body movements in terms of Body, Effort, Shape, and Space, collectively known as the Laban/Bartenieff Movement System (LBMS) [9]. Effort, or what Laban referred to as the dynamics of movement, involves understanding the dynamic intention and energy behind movements. The Effort category addresses the energetic expression of movement through four factors: Weight, Time, Space, and Flow, each with two polarities. For example, Flow can be either 'Free' or 'Bound', depicting how movement energy is either indulgently released or carefully controlled [10,11]. This effective aspect of movement analysis can provide novel insights into the qualitative aspects that make an efficient or powerful golf swing.
Despite the comprehensive nature of biomechanical models, there remains a research gap in how these models integrate qualitative movement descriptors that can differentiate between skill levels and identify movement efficiency. The application of LBMS, particularly its Effort component, offers a promising avenue to fill this gap. By analyzing golf swings through the lens of Effort qualities such as the Free or Bound nature of Flow, researchers can explore how these dynamics correlate with traditional biomechanical measurements, such as the instantaneous screw axis (ISA), which describes the rotation and translation of body segments [12]. One form of representing the club’s motion is the Instantaneous Screw Axes (ISA) [13,14].
Despite the potential applicability of LBMS in sports sciences, very few studies, if any, have utilized this rich analytical framework to understand and enhance the biomechanics of golf swings or other sports activities. This represents a significant research gap, as LBMS can provide novel insights into the qualitative aspects that make an efficient or powerful golf swing. Notably, the incorporation of LBMS into sports biomechanics remains largely unexplored, marking this study as a pioneering effort to integrate these qualitative descriptors with quantitative biomechanical models.
The current research aims to bridge this gap by applying LBMS’s Effort theory specifically to the biomechanical context of golf swings. By focusing on how the physical forces generated by the golf club (e.g., mass moment of inertia) are managed and utilized by the golfer, this study seeks to uncover the underlying dynamics that contribute to more efficient and powerful swings, particularly during the critical phase of the downswing [7]. This novel approach not only promises to enhance our understanding of the biomechanical aspects of golf swings but also opens the door for broader applications in sports science and rehabilitation.
2. Materials and Methods
2.1. Laban's Effort Actions Theory to Refine and Communicate the Quality of Movement of the Golf Swing
Laban's Eight Effort Actions, also known as Laban Effort Drives, are a key part of Rudolf Laban's system for understanding and notating dance and movement, known as Laban Movement Analysis (LMA) [15,16]. These Effort Actions are used to describe the quality of movement and are organized according to Laban's Effort-Shape theory, which considers both the inner intention and the outer shape of the movement. The eight Effort Actions are categorized into four pairs of opposite qualities, with each pair representing one of the factors of motion: Weight, Time, Space, and Flow (Figure 1(a)). The graph shows how these polarities interact to create various movement qualities, such as Gliding (Light, Sustained, Direct), Flicking (Light, Sudden, Indirect), Pressing (Strong, Sustained, Direct), and Wringing (Strong, Sustained, Indirect). Each combination represents a unique effort and intention in movement. (Adapted from Laban's original work on movement analysis.)
This Effort graph was created by Laban to delineate his organization of inner intent or motivation behind a movement. Light and Strong are the two polarities of Weight Effort, Sustained and Sudden are the two polarities of Time Effort, Direct and Indirect are the two polarities of Space Effort, Free and Bound are the two polarities of Flow Effort. In refining the understanding of the golf swing, this study applies Rudolf Laban’s Eight Effort Actions, part of the Laban Movement Analysis (LMA), which characterizes movement through dynamic qualities. Each of these actions corresponds to a combination of Effort elements—Weight, Time, Space, and Flow—depicting how movement can be experienced and executed with varying intensities and intentions [16].
The golf swing, a sophisticated biomechanical action, encapsulates these Effort elements in its execution. The swing begins with a preparatory phase where the golfer aligns with the target, transitioning to the backswing, culminating in the powerful downswing. Each phase can be mapped to Laban's Effort Actions, offering a qualitative lens through which to analyze and refine movement (Figure 1(b)).
Figure 2. illustrates the application of Laban's Effort Cube and dimensional analysis to the phases of a golf swing. The Effort Cube, along with the three primary planes of motion (horizontal, vertical, and sagittal), provides a comprehensive framework for analyzing the qualitative aspects of movement. The figure includes various components that highlight the spatial and dynamic aspects of movement, aligning with Laban's Effort Actions. By expanding the explanation of Figure 2 with these detailed Table 1, we provide a comprehensive context that clarifies the data and enhances the reader's understanding of the application of Laban's Effort Actions to the golf swing. This integrated approach allows for a deeper analysis of both the qualitative and biomechanical aspects of the movement, ultimately improving performance and understanding.
The initial phase of the swing mirrors the 'Float' Effort Action—light, sustained, and indirect—where the golfer prepares, aligning body and mind with the intended direction and outcome. As the backswing progresses, the 'Glide' Effort Action—light, direct, and sustained—comes into play, signifying a smooth and controlled buildup of potential energy (Figure 3). As the golfer transitions into the downswing, the Effort Actions shift towards more dynamic qualities. The 'Slash' Effort Action—strong, indirect, and quick—aptly describes the initiation of the downswing. Here, the stored potential energy is released into kinetic energy, demonstrating the efficient and powerful execution of the swing. Throughout the swing, minimizing unnecessary muscular tension is vital. A 'Free Flow' Effort quality ensures that movements are executed with minimal restraint, allowing for better coordination and fluidity. This quality is particularly critical during the downswing, where excessive tension can lead to a disrupted kinetic chain and inefficient energy transfer.
Effort quality in the context of the swing also directly impacts efficiency and timing. For instance, a 'Free Flow' in the Effort Action 'Press'—strong, direct, and sustained—can be seen in the even application of force through the impact with the ball, maximizing transfer of energy without unnecessary resistance. The implementation of LBMS in this context serves as an innovative method to convey the complex biomechanical and qualitative nuances of the golf swing. The inclusion of these Effort Actions within training regimens can enhance a golfer’s understanding of the physical and psychological elements at play, potentially leading to improved performance and a deeper appreciation for the subtleties of the sport.
As such, in this study, we seek to further explore these qualitative descriptors provided by Laban’s Effort Actions theory and apply them methodologically to analyze the intricate movements of the golf swing. Through this analysis, we aim to provide actionable insights that can be used to refine golf techniques and inform teaching and coaching practices.
This study employs screw theory [17,18] as a foundational mathematical framework to describe the spatial vector quantities relevant to the golf swing. Screw theory facilitates the representation of the six degrees of freedom of a rigid body (three translational and three rotational) in a unified manner. By defining the instantaneous screw axis (ISA) of the golf club, we capture both the rotational and translational dynamics essential for analyzing the complex movements in golf.
We use Plücker coordinates [19] to represent these ISAs, allowing us to model the line of action and the moment around which the club rotates and translates during the swing. This approach provides a comprehensive understanding of the mechanical interactions between the golfer and the club, crucial for evaluating performance and technique.
The spatial inertia tensor [20], a key concept from dynamics, is utilized to map the spatial acceleration space to the force space. Analogous to the error covariance tensor in estimation theory, this tensor encapsulates the inertia and mass properties of the golf club relative to the wrist joint, which is critical for understanding how golfers perceive and control the club during a swing. By decomposing the spatial inertia tensor into its geometric and constitutive components, we address complex eigenvalue problems that describe how different elements of the club's inertia influence the swing dynamics. This decomposition allows us to explore the interaction between the golfer's biomechanical inputs and the physical responses of the club. In this section of our paper, therefore, we present a method of free-vector decomposition for the spatial rigid-body inertia tensor. The linear map from the spatial acceleration space to the spatial force space was defined as the spatial inertia tensor. This spatial inertia (of a rigid body) has been successfully represented by means of a set of principal screws of inertia [20]. Consider the situation in Figure 4: A club has a mass, m; its center of mass, CM, is given by the position vector, C; and the inertia tensor about its center of mass is J. The club is at rest, and experiences a force, f, acting along a line passing through the center of mass, and a couple, τC. The resulting acceleration is determined by an angular acceleration, α, along an axis passing through the center of mass (CM), the linear acceleration, aC, on CM.
We present results that apply our modeling approach to the inertia tensor that was obtained by geometric scaling [21], from a previously published dataset [22]. Specifically, we did not directly measure the inertia tensor; rather, we used data obtained from a club made by the same manufacturer. Therefore, the inertia matrix included in Figure 4 is a result of our own calculations based on these established methods and datasets. As such, the matrix is our original work, derived from the referenced data. The mass moment of inertia of the club was geometrically scaled by assuming that the moments of inertia of each segment are proportional to the mass times a linear dimension squared, as detailed by Zatsiorsky (1998). We then tuned segment parameters for a sample (including mass, location of center of mass, inertia) as described by MacKenzie & Sprigings (2009), to match the specific club used in our experiment.
The equation of motion is considered as a mapping from the twist-like screw acceleration to a wrench space [23].
 where
where 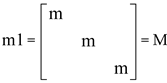 , and 1 is the 3×3
identity matrix.
, and 1 is the 3×3
identity matrix.

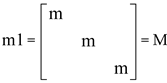 , and 1 is the 3×3
identity matrix.
, and 1 is the 3×3
identity matrix.Since all of the spatial quantities are referred to with respect to the center of mass, the linear and angular components of motion are decoupled – the linear acceleration being entirely due to the force, and the angular acceleration being a result of the couple. To transform Equation 1 into the origin of the joint axis (Figure 4), we obtain
 where H = C × M, I =J+C×MC×T, and C× is
the anti-symmetric skew matrix corresponding to C.
where H = C × M, I =J+C×MC×T, and C× is
the anti-symmetric skew matrix corresponding to C.


Due to its special 6×6 form, the spatial inertial tensor
 is expected to have special eigen structures.
is expected to have special eigen structures.

The spatial inertia tensor M0 represented at the origin is a symmetric, positive definite tensor and transforms to any point ‘A’ by the spatial Jacobian, Φ, according to
 where
where 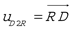 and
and 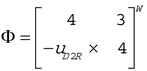 .
.

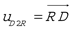 and
and 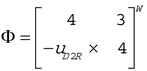 .
.The eigenvalue problem provides a unique decomposition of M0 as
 where mf and mγ are
representing the eigen values of mass and mass moment of inertia, respectively
(following the common notational tactics for the principal axes of inertia, we
use
where mf and mγ are
representing the eigen values of mass and mass moment of inertia, respectively
(following the common notational tactics for the principal axes of inertia, we
use 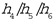 for mγ
and, to the corresponding principal moment of inertia,
for mγ
and, to the corresponding principal moment of inertia,  for
γ).
for
γ).

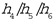 for mγ
and, to the corresponding principal moment of inertia,
for mγ
and, to the corresponding principal moment of inertia, 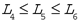 for
γ).
for
γ).One might wonder whether the decomposition based on the solution for the free-vector eigenvalue problem would be different at another point A. We apply the transformation rule (Equation 5) to the above decomposition (Equation 6):


 which shows that MA is decomposed by the same eigenscrews in the same manner, just represented at B. That is, no matter where the problem is posed, the same eigenscrews form the basis of the decomposition.
which shows that MA is decomposed by the same eigenscrews in the same manner, just represented at B. That is, no matter where the problem is posed, the same eigenscrews form the basis of the decomposition.



2.3. Instrumentation and Data Analysis
In this study, rather than collecting new empirical data, we utilized an existing dataset from previous research that detailed the golf swings of two female golfers with different skill levels [23] (Table 2). This dataset was chosen for its comprehensive capture of biomechanical movements using high-precision motion capture technology and ground reaction force measurements, making it highly suitable for our analysis.
The original data were collected using a sophisticated array of twelve high-speed Qualisys cameras, which accurately recorded the three-dimensional positions of reflective markers attached to the golfers and their clubs. This setup was designed according to the standards of the International Society for the Advancement of Kinanthropometry, ensuring that the biomechanical analysis adhered to high measurement accuracy and reliability (Figure 5). Ground reaction forces were measured using a Kistler force plate, providing essential data on the biomechanical forces exerted during the swings.
By reusing this existing dataset, we were able to directly apply our analytical models without the variability and resource constraints associated with new data collection. This approach also allowed us to focus on in-depth analysis using established data, ensuring that our study was both resource-efficient and grounded in reliable biomechanical metrics. The dataset included detailed annotations of each golfer's movements and the corresponding biomechanical outputs, which facilitated a nuanced analysis of the swing mechanics. In total, twenty-four reflective markers and four marker clusters were used for the reconstruction of eight body segments (i.e., the trunk, pelvis, thighs, shanks, and feet). Additionally, specific markers were placed on the club and wrist joint to define their respective reference frames. Marker coordinates for the period between the beginning of the downswing of a golf swing and up to the instant before impact were then acquired.
Motion capture was undertaken using an optoelectronic system of twelve Qualisys cameras (type: Oqus-300, Qualisys AB, Sweden) operating at 300Hz. The collected data were processed using Qualisys Track Manager (QTM) software to ensure accurate tracking and reconstruction of the marker trajectories. For the club, markers were placed at specific points along the shaft and clubhead to capture its motion accurately. The wrist joint was defined using markers placed on anatomical landmarks around the wrist to establish a local reference frame.
Using the predefined anatomical coordinate system from the original study, which was based on key landmarks identified on the golfer and the club, we analyzed the motion data to extract the instantaneous screw axes (ISA) and their evolution during the downswing phase. This previously recorded data provided a robust basis for exploring how biomechanical properties such as the ISA correlate with golfer skill level and technique efficacy.
Reusing data from a well-designed previous study can enhance the efficiency of the research process and contribute to the sustainability of research practices by utilizing existing resources. This method helps to ensure that findings are based on previously validated data, which can support the reliability of the conclusions drawn from the analysis.
3. Results
3.1. A 'Free' Flow Versus a 'Bound' Flow Based on Laban’s Effort Theory
Our analysis of the previously collected data revealed distinct differences in the golf swing mechanics between skilled and novice players, particularly in how they manage the physical forces during the downswing. One skilled golfer exhibited a profound synchronization between the instantaneous screw axis (ISA) and the dynamic forces exerted by the golf club's inertia. This synchronization suggests a voluntary and strategic compliance with the natural forces, allowing these forces to guide the swing without opposition.
Figure 6 and Figure 7 in this manuscript are adapted from Figure 5 and Figure 6 in our previous publication in the International Journal of Golf Science [23]. The original study, titled "Haptic Perception-Action Coupling Manifold of Effective Golf Swing," provided foundational results that are further analyzed and expanded upon in this current research.
Figure 6 provides a dynamic visualization of the ISA paths during a novice golfer’s swing. The trajectories depicted by the solid lines and contrasted against the principal axes of inertia (dashed lines) illustrate a lack of alignment between perceived inertia and actual movement paths. This indicates a struggle with effective force management and synchronization, which is particularly evident from the disjointed ISA paths that exhibit abrupt changes in movement direction and speed. These perturbations suggest a resistance or a lack of coordination in allowing the inertia of the club to influence their body dynamics.
Conversely, Figure 7 illustrates the proficient golfer's ability to synchronize her swing dynamics effectively. The alignment between the ISA (solid lines) and the e3 (dashed lines) demonstrates adept perception-action coupling, allowing her to voluntarily harness the club’s inertia to optimize swing mechanics and energy flow. This contrasted with the novice’s disjointed motion paths reveals significant insights into the qualitative differences in swing mechanics between different skill levels.
The movement patterns of skilled golfers demonstrated that their swings were not only synchronized in terms of timing but also optimized for energy utilization. The ISA paths in skilled golfers were smoother and showed a consistent alignment with the club's inertia forces throughout the swing. This alignment allowed one skilled player to maintain an efficient kinetic chain that enhanced both the power and fluidity of the swing, indicative of her ability to harness and modulate the club's inertia effectively.
In contrast, one novice golfer displayed multiple deviations from the ideal ISA path, with these deviations manifesting as abrupt changes in movement direction and speed. These perturbations indicate a resistance or a lack of coordination in allowing the inertia of the club to influence her body dynamics. The novice swings were characterized by a 'Bound' Flow, where the energy seemed restrained and movements were less fluid, likely resulting from a conscious or unconscious opposition to the inertia forces.
The spatiotemporal representation of the swings, especially the ISA paths, provided a vivid illustration of how the swing dynamics unfolded over time. For the skilled golfer, the ISA and the perceived inertia (e3 pathways) were closely aligned, suggesting a high degree of perceptual-motor integration. This integration enables proficient golfers to anticipate and adapt to the physical demands of the swing, allowing the natural progression of movement influenced by the club's inertia. Conversely, the novice golfer's e3 and ISA paths showed misalignment, indicating less effective perception-action coupling and a disjointed adaptation to the dynamic forces.
These results underscore the critical role of allowing physical forces to act naturally across the golfer's biomechanical system, highlighting a fundamental difference in skill levels. The ability to voluntarily allow and adapt to these forces is a hallmark of proficiency in golf, reflecting a deeper kinesthetic awareness and a more refined control over the complex dynamics of the golf swing.
Figure 8 encapsulates the concept of gathering/scattering as a shaping movement in golf, akin to Dell’s description of accommodating the plastic character of objects and molding space like a sculptor with clay [18]. The sequence from phases 1 to 4 demonstrates the golf club's dynamic downswing, where the club's motion is adapted and refined in response to the forces and trajectory required for an effective strike. Each line traces the club's path, illustrating the adaptation of form and the creative interaction with space, capturing the expressive and sculptural qualities inherent in skilled sports movements.
Sequential visualization of a golf club's downswing, highlighting the dynamic envelope of club inertia. Phases 1 to 4 illustrate the progressive motion of the club: starting from the initial downswing position (1), moving through the increasing acceleration (2, 3), and culminating in the final swing phase (4) where maximum velocity is achieved. The contour lines represent the club's path, emphasizing how the inertia influences the trajectory and speed across the swing.
3.2. Transition from Biomechanical Measures to LBMS Qualitative Types
In our biomechanical analysis, as revealed in Figure 8, we demonstrate how the perception of the disposition of touch surfaces, such as the contour of the club's inertia envelope, is influenced by the golfer's body positioning. The sensitivity of a trained golfer to the geometric pattern of their body, its members, and extremities illustrates the precision of their perception of pose or disposition. This sensitivity is particularly precise in disciplines like ballet, as captured by LBMS. Choreographers have analyzed the movements and pauses of the human body in efforts to establish a dance notation (see Figure 2 and Figure 3). This rich notation system is fundamental to understanding the detailed perception of skeletal movement.
In our study, we propose a simplified coordinate disposition of the golfer's limbs, depending on the distribution of matter in the club. This approach allows us to bridge the gap between quantitative biomechanical data and the qualitative analysis provided by LBMS. Figure 8 visually illustrates this transition by showing the sequential phases of the golf swing, each annotated with the corresponding biomechanical data and the mapped LBMS Effort elements. This figure demonstrates how the golfer’s actions (quantified through biomechanical measures) shape their perception of movement quality (described through LBMS).
In conclusion, this detailed mapping from biomechanical measures to LBMS qualitative types provides a comprehensive framework for understanding the dynamic and expressive qualities of the golf swing. By integrating quantitative and qualitative analyses, we offer a richer, more nuanced perspective on movement, enhancing both theoretical understanding and practical applications in biomechanics and movement studies.
The enveloping shape of the inertia surface in Figure 8, depicted by the red lines, illustrates how the club's inertia changes dynamically throughout the swing. This aligns with the Labanotation's depiction of movement qualities, emphasizing the interplay between physical forces and movement efficiency. Trained athletes perceive these dynamic changes and adjust their posture and muscle engagement accordingly. The gray discrete lines showing the specific locations of the club in Figure 8 can be mapped to the phases illustrated in the Labanotation of Figure 9, providing a visual alignment between the qualitative and quantitative analyses. This alignment helps trained golfers or dancers to understand and visualize the precise positioning needed for optimal performance.
3.3. Motif Writing using Laban Movement Analysis and its Notation are Tied Closely to the Depiction of the Golf Swing
Figure 9 presents the golf swing represented through Labanotation, a graphical system for recording and analyzing movement. This figure serves to bridge the biomechanical analysis with the qualitative descriptions provided by the Laban/Bartenieff Movement System (LBMS). Here, we explain the transition from the previous figures to Figure 9 and address its applicability to different skill levels of golfers.
Building upon this biomechanical foundation (Figure 8), Figure 9 translates these parameters into the qualitative movement language of Labanotation. This system captures the essence of the movement by notating the spatial, temporal, and dynamic aspects of the golf swing. The Effort sign (Figure (a)) “Strong” for the Effort Factor Weight, meaning “resolute with power” when returning from the backswing to impact (touching the ball). Vertical bows (Figure 9(b)) show that actions are done simultaneously, show phrasing, include body parts or add specific aspects to the movement. "Phrasing in movement" refers to how a sequence of movements is structured and connected, much like phrasing in music or language. It involves the flow and continuity of movements, highlighting the transitions and the relationship between different parts of the movement sequence. In the context of Laban Movement Analysis (LMA) and Labanotation, phrasing helps to understand the dynamics, timing, and expressiveness of movement. Basic form of the bow for "touch" (Figure 9(c)) - something is touching something
Figure 9 is designed to be valid for analyzing both skilled and novice golfers, though the notations will differ based on the individual's movement patterns. In skilled golfers, the notation will show more refined and controlled movements, with smooth transitions and efficient use of force. The dynamic qualities will likely reflect a balanced and coordinated effort, with clear directional intent and fluid motion.
For novice golfers, the notation may reveal more erratic and less controlled movements, with abrupt transitions and less efficient use of force. The dynamic qualities might show variability in effort, with more frequent changes in direction and flow.
The differences between skilled and novice golfers can be identified through the patterns and symbols used in the Labanotation , if further developed: Skilled golfers will exhibit consistent and smooth notations, indicating well-coordinated movements. In contrast, novice golfers may show inconsistent notations with frequent disruptions in flow.
The depicted Labanotation score is not merely a static representation but a dynamic blueprint that outlines the essential phases of a golf swing. It visually encodes the golfer’s posture, the sequential flow of the swing, the directionality of the club, and the shifting weight and balance of the golfer's body through space and time.
By applying both the Labanotation and Bartenieff Fundamentals to the golf swing, the analysis becomes a living, evolving process. The systems work both separately and in unison, providing tools to capture and enhance the expressivity and functionality of the golfer’s movements. They enable a deeper investigation into how the golfer’s body engages with and navigates through the biomechanical demands of the swing, aiming for efficiency, power, and harmony. Thus, Figure 9 does not merely depict the mechanical sequence of the golf swing but embodies a deeper dialogue between the golfer and the swing—a synthesis of kinetic elegance and biomechanical precision, continually evolving as our understanding of movement deepens. In conclusion, by incorporating Labanotation and Motif Writing into the analysis, Figure 9 provides a comprehensive visual representation of the golf swing's dynamics and Effort qualities. This detailed explanation bridges the gap between previous figures and Figure 9, clarifying its application for different skill levels.
4. Discussion
The present study aimed to integrate Laban Movement Analysis (LMA) with biomechanical analysis to provide a comprehensive understanding of the golf swing. By combining qualitative and quantitative methods, we sought to enhance the analysis and refinement of movement in golf.
Our approach utilized Labanotation to represent the phases of the golf swing, capturing the dynamic and spatial aspects of movement. Labanotation is an effective tool for visualizing and analyzing complex movements, as it provides a detailed depiction of movement qualities such as direction, effort, and flow. The biomechanical analysis, on the other hand, offered quantitative data on the path, velocity, and inertia of the club head throughout the swing.
Figure 9 illustrated the golf swing using Labanotation, depicting key phases such as the setup, backswing, transition to downswing, downswing, and follow-through. This qualitative representation was complemented by Figure 8, which provided a biomechanical analysis of the golf swing, showing the club head's path and velocity, and how it envelops the inertia surface.
4.1. Detailed Analysis of the Golf Swing
Setup Position:
In the setup position, the golfer aligns their body with the target. This phase is critical for establishing a solid foundation for the swing. The Labanotation in Figure 9 shows the initial orientation, while the biomechanical data in Figure 8 depicts the club head's position relative to the body.
Backswing:
The backswing involves lifting the club upward and backward, rotating the torso and building potential energy. Labanotation captures the smooth, sustained, and indirect qualities of this movement, while the biomechanical analysis shows the path trajectory and velocity changes.
Transition to Downswing:
This phase marks the change in direction from backswing to downswing. It is characterized by a quick, strong, and indirect effort. The Labanotation highlights the dynamic shift, and the biomechanical analysis shows the corresponding acceleration of the club head.
Downswing:
The downswing is the most powerful phase, where the stored potential energy is released. The strong, direct, and quick qualities are captured in the Labanotation, and the biomechanical data shows the peak velocity and the enveloping inertia surface.
Impact and Follow-Through:
The impact and follow-through involve the club striking the ball and the subsequent continuation of the swing. The Labanotation represents the fluidity and control of this phase, while the biomechanical analysis provides data on the deceleration and final positioning of the club head.
4.2. Practical Implications and Applications
The integration of LMA with biomechanical analysis offers several practical benefits for golfers and coaches:
Enhanced Movement Analysis:
By combining qualitative and quantitative data, we can gain a deeper understanding of the intricacies of the golf swing. This comprehensive analysis helps identify areas for improvement and optimize performance.
Training and Rehabilitation:
The detailed representation of movement can be used in training programs to teach proper techniques and in rehabilitation settings to correct faulty movement patterns and prevent injuries.
Customization and Personalization:
The approach allows for personalized feedback based on individual movement patterns. Coaches can tailor training programs to address specific needs and goals of each golfer.
While the integration of LMA and biomechanical analysis provides valuable insights, there are limitations to consider. The study focused on a specific group of golfers, and the findings may not be generalizable to all skill levels or demographics. Additionally, the complexity of Labanotation may require specialized training to fully understand and utilize its benefits.
Future research should explore the application of this integrated approach to different sports and movement disciplines. Further studies could also investigate the long-term effects of using this method in training and rehabilitation programs.
By examining the Effort elements and biomechanical data of a golfer's swing, we provided a comprehensive analysis that bridges the gap between qualitative and quantitative methods. This integrated approach enhances our understanding of the golf swing, offering practical applications for improving performance and reducing the risk of injury. The findings underscore the importance of considering both the dynamic qualities of movement and the underlying biomechanics to achieve optimal results in sports and physical activities.
5. Conclusions
This study has demonstrated the value of integrating Laban Movement Analysis with biomechanical analysis to achieve a comprehensive understanding of the golf swing. By bridging qualitative and quantitative methods, we have provided a robust framework that enhances both the analysis and refinement of movement. The practical applications of this approach offer significant benefits for training, performance improvement, and injury prevention. Future research should continue to build on these findings, expanding their application and exploring new areas of study.
Coaches can use the detailed movement analysis to develop more effective training regimens that focus on improving specific aspects of the golf swing. This approach helps golfers understand the intricacies of their movements and make precise adjustments. The integration of LMA and biomechanical analysis can identify and correct faulty movement patterns that may lead to injuries. Rehabilitation programs can be tailored to address specific issues, promoting safer and more efficient recovery.
Future studies should explore the application of this integrated approach to other sports and movement disciplines. This will help determine its versatility and effectiveness in various contexts.
Author Contributions
Conceptualization, W.K.; writing—original draft preparation, W.K.; formal analysis, W.K.; writing—review and editing, A.H.V.; funding acquisition, A.H.V.; Laban interpretation and Labanotation, W.O.; resources, W.O.; writing—review and editing, W.O.; writing—review and editing, C.W. All authors have read and agreed to the published version of the manuscript.
Funding
Please add: This research was funded by PROCIENCIA under contract N° PE501080681-2022-PRO-CIENCIA Proyectos Especiales: Proyectos de Investigadores Visitantes.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Acknowledgments
The authors should like to acknowledge Miwon Kim, Sofia Camacho, and Professor Jorge Infante, for their help during data acquisition for this study. The first author should also like to thank Mr. Sangdo Kim for his stimulating discussions regarding the perfection of the golf swing.
Conflicts of Interest
The authors declare no conflicts of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.
References
- Cochran, A.J.; Farrally, M.R. Science and golf II: proceedings of the 1994 World Scientific Congress of Golf; Spon, 1994. [Google Scholar]
- Teu, K.K.; et al. The analysis of golf swing as a kinematic chain using dual Euler angle algorithm. Journal of Biomechanics 2006, 39, 1227–1238. [Google Scholar] [CrossRef] [PubMed]
- Horan, S.A.; et al. Thorax and pelvis kinematics during the downswing of male and female skilled golfers. Journal of Biomechanics 2010, 43, 1456–1462. [Google Scholar] [CrossRef] [PubMed]
- MacKenzie, S.; Sprigings, E. A three-dimensional forward dynamics model of the golf swing. Sports Engineering 2009, 11, 165–175. [Google Scholar] [CrossRef]
- MacKenzie, S.; Sprigings, E. Understanding the mechanisms of shaft deflection in the golf swing. Sports Engineering 2009, 12, 69–75. [Google Scholar] [CrossRef]
- Nesbit, S.M. A three dimensional kinematic and kinetic study of the golf swing. Journal of Sports Science and Medicine 2005, 4, 499–519. [Google Scholar] [PubMed]
- Sprigings, E.; Mackenzie, S.J. Examining the delayed release in the golf swing using computer simulation. Sports Engineering 2002, 5, 23–32. [Google Scholar] [CrossRef]
- Teu, K.; et al. Using dual Euler angles for the analysis of arm movement during the badminton smash. Sports Engineering 2005, 8, 171–178. [Google Scholar] [CrossRef]
- Wahl, C. Laban/Bartenieff movement studies: Contemporary applications; Human Kinetics, 2019. [Google Scholar]
- Laban, R.; Ullmann, L. The mastery of movement. 1971.
- Bartenieff, I.; Lewis, D. Body movement: Coping with the environment; Routledge, 2013. [Google Scholar]
- Fletcher, I.M.; Hartwell, M. Effect of an 8-week combined weights and plyometrics training program on golf drive performance. The Journal of Strength & Conditioning Research 2004, 18, 59–62. [Google Scholar]
- Kiat Teu, K.; Kim, W. Estimation of the axis of a screw motion from noisy data--a new method based on Plucker lines. J Biomech 2006, 39, 2857–2862. [Google Scholar] [CrossRef] [PubMed]
- Kim, W.; Kohles, S.S. A reciprocal connection factor for assessing knee-joint function. Computer Methods in Biomechanics and Biomedical Engineering 2011, 15, 911–917. [Google Scholar] [CrossRef] [PubMed]
- Berardi, G. Making connections: total body integration through Bartenieff fundamentals. Journal of Dance Medicine & Science 2004, 8, 91–91. [Google Scholar]
- Dell, C. A primer for movement description using effort-shape and supplementary concepts. (No Title), 1977.
- Davidson, J.; Hunt, K. Robots and screw theory: applications of kinematics and statics to robotics; Oxford University Press, 2004. [Google Scholar]
- Ball, R. A treatise on the theory of screws; Cambridge University Press, 1900. [Google Scholar]
- Hunt, K.H. Kinematic geometry of mechanism; Clarendon Press: Oxford, 1990. [Google Scholar]
- Ciblak, N. Analysis of Cartesian stiffness and compliance with applications, Georgia Institute of Technology, U.S.A, 1998.
- Zatsiorsky, V.M. Kinematics of Human Motion; Human Kinetics: Champaign, IL, 1998. [Google Scholar]
- MacKenzie, S.J. Understanding the role of shaft stiffness in the golf swing, in College of Kinesiology; University of Saskatchewan, 2005. [Google Scholar]
- Kim, W.; et al. Haptic Perception-Action Coupling Manifold of Effective Golf Swing. International Journal of Golf Science 2013, 2, 10–32. [Google Scholar] [CrossRef]
Figure 1.
a). Laban's Effort Graph. This Effort graph, created by Rudolf Laban, illustrates the organization of inner intent or motivation behind a movement. The graph delineates the polarities of the four Effort qualities: Weight, Time, Space, and Flow. Each Effort quality has two opposing characteristics: Weight Effort: Ranges from Light to Strong, indicating the force exerted in a movement. Time Effort: Ranges from Sustained to Sudden, reflecting the speed and acceleration of a movement. Space Effort: Ranges from Direct to Indirect, representing the focus and clarity of the movement's path. Flow Effort: Ranges from Free to Bound, describing the continuity and control of the movement.
Figure 1.
a). Laban's Effort Graph. This Effort graph, created by Rudolf Laban, illustrates the organization of inner intent or motivation behind a movement. The graph delineates the polarities of the four Effort qualities: Weight, Time, Space, and Flow. Each Effort quality has two opposing characteristics: Weight Effort: Ranges from Light to Strong, indicating the force exerted in a movement. Time Effort: Ranges from Sustained to Sudden, reflecting the speed and acceleration of a movement. Space Effort: Ranges from Direct to Indirect, representing the focus and clarity of the movement's path. Flow Effort: Ranges from Free to Bound, describing the continuity and control of the movement.

Figure 1.
b). Mapping Laban's Effort Actions to the Golf Swing. This figure illustrates the application of Laban's Effort Actions to the phases of a golf swing. The golf swing, a sophisticated biomechanical action, encapsulates these Effort elements in its execution. The swing begins with a preparatory phase where the golfer aligns with the target, transitioning to the backswing, and culminating in the powerful downswing. The diagrams show the integration of these Effort qualities within the spatial constructs (three planes: vertical, horizontal, sagittal) and the icosahedron, illustrating the complex and dynamic nature of the golf swing. The A-scale inclinations demonstrate the specific body alignments and movements that correspond to the different phases of the swing. The visual representations provide a qualitative lens through which to analyze and refine movement, enhancing both performance and understanding of the biomechanics involve.
Figure 1.
b). Mapping Laban's Effort Actions to the Golf Swing. This figure illustrates the application of Laban's Effort Actions to the phases of a golf swing. The golf swing, a sophisticated biomechanical action, encapsulates these Effort elements in its execution. The swing begins with a preparatory phase where the golfer aligns with the target, transitioning to the backswing, and culminating in the powerful downswing. The diagrams show the integration of these Effort qualities within the spatial constructs (three planes: vertical, horizontal, sagittal) and the icosahedron, illustrating the complex and dynamic nature of the golf swing. The A-scale inclinations demonstrate the specific body alignments and movements that correspond to the different phases of the swing. The visual representations provide a qualitative lens through which to analyze and refine movement, enhancing both performance and understanding of the biomechanics involve.

Figure 2.
This is the basic model Laban used to find his Effort Graph diagram with the three dimensions: (a) high/deep or the weight plane; (b) side-to-side or the space plane; (c) forward/backward or the time plane; (d) represents a two-dimensional projection of the Effort Cube, focusing on the movement within a single plane to simplify the analysis of dynamic qualities; (e) an X-marked schematic within the bounds of the Effort Cube, suggesting the intersection and interaction of Effort elements across different dimensions; (f) a clear view of the Effort Cube, emphasizing its structure and the confinement of movement dynamics within its boundaries; (g) displays a radial layout of various Effort elements and actions, illustrating the spectrum of possible movements and their classifications under Laban's system; and (h) integrates the Effort Cube with a human figure, mapping the movement dynamics to the body, illustrating how different Effort actions can be expressed physically within the three-dimensional space of the cube.
Figure 2.
This is the basic model Laban used to find his Effort Graph diagram with the three dimensions: (a) high/deep or the weight plane; (b) side-to-side or the space plane; (c) forward/backward or the time plane; (d) represents a two-dimensional projection of the Effort Cube, focusing on the movement within a single plane to simplify the analysis of dynamic qualities; (e) an X-marked schematic within the bounds of the Effort Cube, suggesting the intersection and interaction of Effort elements across different dimensions; (f) a clear view of the Effort Cube, emphasizing its structure and the confinement of movement dynamics within its boundaries; (g) displays a radial layout of various Effort elements and actions, illustrating the spectrum of possible movements and their classifications under Laban's system; and (h) integrates the Effort Cube with a human figure, mapping the movement dynamics to the body, illustrating how different Effort actions can be expressed physically within the three-dimensional space of the cube.

Figure 3.
This figure illustrates Laban's Effort Graph diagram, which integrates three key dimensions of movement: (a) high/deep or the weight plane, representing vertical movements and the distribution of weight; (b) side-to-side or the space plane, depicting lateral movements and spatial orientation; (c) forward/backward or the time plane, indicating movements related to timing and progression. (d) shows a two-dimensional projection of the Effort Cube, simplifying the analysis by focusing on movement within a single plane. (e) Presents an X-marked schematic within the bounds of the Effort Cube, highlighting specific movement patterns and the intersections of the three dimensions. This figure is crucial for understanding how the dynamic qualities of movement are analyzed and interpreted using Laban's framework. .
Figure 3.
This figure illustrates Laban's Effort Graph diagram, which integrates three key dimensions of movement: (a) high/deep or the weight plane, representing vertical movements and the distribution of weight; (b) side-to-side or the space plane, depicting lateral movements and spatial orientation; (c) forward/backward or the time plane, indicating movements related to timing and progression. (d) shows a two-dimensional projection of the Effort Cube, simplifying the analysis by focusing on movement within a single plane. (e) Presents an X-marked schematic within the bounds of the Effort Cube, highlighting specific movement patterns and the intersections of the three dimensions. This figure is crucial for understanding how the dynamic qualities of movement are analyzed and interpreted using Laban's framework. .
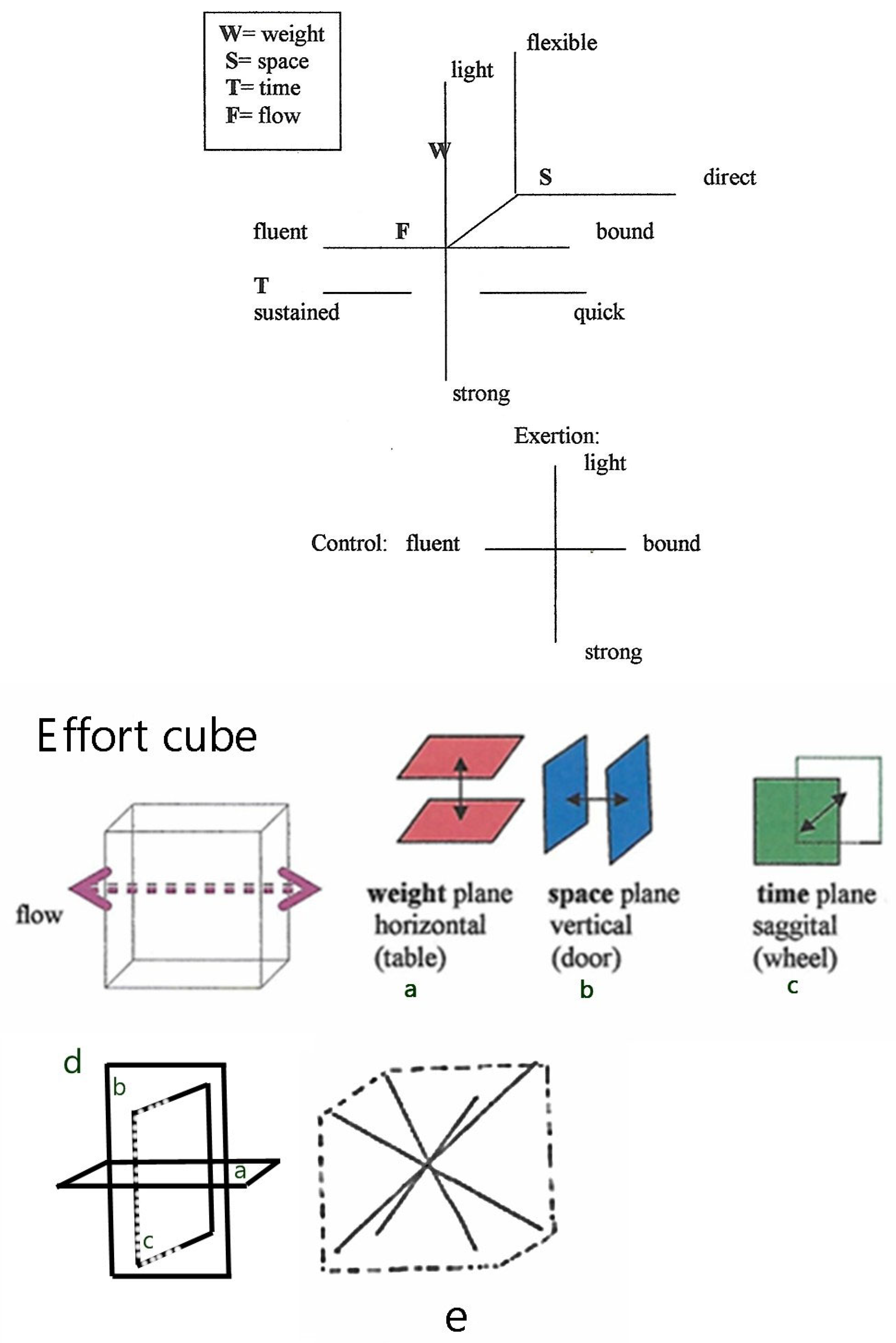
Figure 4.
This figure illustrates the grip reference frame attached at the center of the end of the club shaft. The spatial inertia tensor, computed with reference to the origin of the grip coordinate system, is depicted showing its principal axes and moments of inertia. This representation highlights how the inertia tensor transforms at any point along the wrist joint axis ‘A’, emphasizing the consistent eigenvalues irrespective of the positioning. The spatial arrangement allows us to explore how the inertia impacts the golfer’s control over the club during dynamic movements. The original values of quantities below the anatomical sketch that were originally published by [4] are used by permission of professor Sasho MacKenzie and are represented relative to the grip reference frame. (units in  ).
).
 ).
).
Figure 4.
This figure illustrates the grip reference frame attached at the center of the end of the club shaft. The spatial inertia tensor, computed with reference to the origin of the grip coordinate system, is depicted showing its principal axes and moments of inertia. This representation highlights how the inertia tensor transforms at any point along the wrist joint axis ‘A’, emphasizing the consistent eigenvalues irrespective of the positioning. The spatial arrangement allows us to explore how the inertia impacts the golfer’s control over the club during dynamic movements. The original values of quantities below the anatomical sketch that were originally published by [4] are used by permission of professor Sasho MacKenzie and are represented relative to the grip reference frame. (units in  ).
).
 ).
).
Figure 5.
Displayed here are the positions of reflective markers on a golfer at the moment the club addresses the ball. This setup was used to capture the biomechanical data originally recorded, detailing the anatomical landmarks critical for analyzing movement dynamics. The separate panel shows detailed placements on the hand, essential for understanding the grip dynamics and the resultant force transmission through the golfer's body during the swing.
Figure 5.
Displayed here are the positions of reflective markers on a golfer at the moment the club addresses the ball. This setup was used to capture the biomechanical data originally recorded, detailing the anatomical landmarks critical for analyzing movement dynamics. The separate panel shows detailed placements on the hand, essential for understanding the grip dynamics and the resultant force transmission through the golfer's body during the swing.

Figure 6.
This figure presents a three-dimensional spatiotemporal view of the ISA (solid lines) and the principal axes of inertia (e3 dashed lines) during a novice golfer’s swing. The paths projected onto the medial and superior sides illustrate the motion dynamics, showing the lack of alignment between perceived inertia and actual movement paths, indicative of the novice’s struggle with effective force management and synchronization. The arrow indicates where the subsequent axes have migrated at every 0.0333 second of time step (units in cm). Adapted from Kim et al. (2013), "Haptic Perception-Action Coupling Manifold of Effective Golf Swing," International Journal of Golf Science, 2(1), 10-32.
Figure 6.
This figure presents a three-dimensional spatiotemporal view of the ISA (solid lines) and the principal axes of inertia (e3 dashed lines) during a novice golfer’s swing. The paths projected onto the medial and superior sides illustrate the motion dynamics, showing the lack of alignment between perceived inertia and actual movement paths, indicative of the novice’s struggle with effective force management and synchronization. The arrow indicates where the subsequent axes have migrated at every 0.0333 second of time step (units in cm). Adapted from Kim et al. (2013), "Haptic Perception-Action Coupling Manifold of Effective Golf Swing," International Journal of Golf Science, 2(1), 10-32.

Figure 7.
Showcased here is the proficient golfer’s ability to synchronize her swing dynamics effectively, as evidenced by the alignment between the ISA (solid lines) and the e3 (dashed lines). This alignment demonstrates her adept perception-action coupling, allowing her to voluntarily harness the club’s inertia to optimize swing mechanics and energy flow, contrasted with the novice’s disjointed motion paths. Adapted from Kim et al. (2013), "Haptic Perception-Action Coupling Manifold of Effective Golf Swing," International Journal of Golf Science, 2(1), 10-32.
Figure 7.
Showcased here is the proficient golfer’s ability to synchronize her swing dynamics effectively, as evidenced by the alignment between the ISA (solid lines) and the e3 (dashed lines). This alignment demonstrates her adept perception-action coupling, allowing her to voluntarily harness the club’s inertia to optimize swing mechanics and energy flow, contrasted with the novice’s disjointed motion paths. Adapted from Kim et al. (2013), "Haptic Perception-Action Coupling Manifold of Effective Golf Swing," International Journal of Golf Science, 2(1), 10-32.

Figure 8.
This image embodies the application of Laban's Body-Effort-Space-Shape framework, illustrating how the golfer's body dynamically adapts to the contour of the club's inertia envelope during the downswing. The red dashed contour marks the principal axis of inertia of the club, serving as a guide that the golfer's movements mold around. This adaptive process, akin to a sculptor intuitively shaping clay, highlights the profound integration of body form with the evolving physical forces of the club, showcasing a sophisticated synchronization of movement and inertia for optimal swing efficiency.
Figure 8.
This image embodies the application of Laban's Body-Effort-Space-Shape framework, illustrating how the golfer's body dynamically adapts to the contour of the club's inertia envelope during the downswing. The red dashed contour marks the principal axis of inertia of the club, serving as a guide that the golfer's movements mold around. This adaptive process, akin to a sculptor intuitively shaping clay, highlights the profound integration of body form with the evolving physical forces of the club, showcasing a sophisticated synchronization of movement and inertia for optimal swing efficiency.
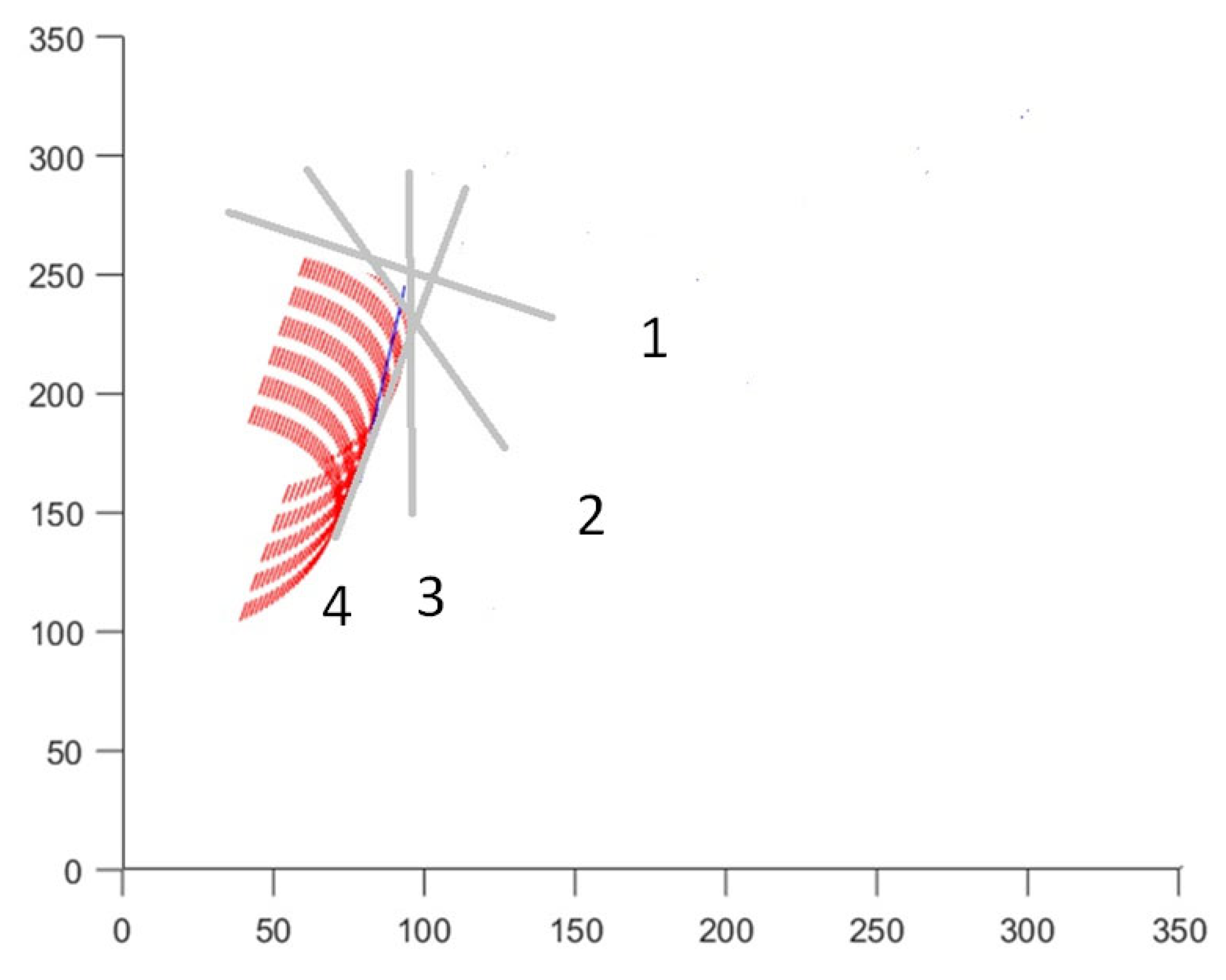
Figure 9.
Golf swing notation. This figure illustrates the golf swing using Labanotation. The notation captures successive postures of the body and the movements between these postures. Symbols represent various body parts and are read from the bottom up, in time. The center line indicates the midline of the body, while the columns represent the legs, arms, and other body segments. This method, originating with Rudolf Laban, can become highly detailed. The accompanying sketches and notes provide context and clarity for those unfamiliar with the notation (Appendix A).
Figure 9.
Golf swing notation. This figure illustrates the golf swing using Labanotation. The notation captures successive postures of the body and the movements between these postures. Symbols represent various body parts and are read from the bottom up, in time. The center line indicates the midline of the body, while the columns represent the legs, arms, and other body segments. This method, originating with Rudolf Laban, can become highly detailed. The accompanying sketches and notes provide context and clarity for those unfamiliar with the notation (Appendix A).

Table 1.
Mapping Laban Effort Actions to Golf Swing Phases. This table illustrates the association between specific phases of the golf swing and the corresponding Laban Effort Actions (see Figure 1(a) for the signs of the four different phases). Each phase is characterized by distinct Effort qualities, providing a comprehensive understanding of the movement dynamics involved.
Table 1.
Mapping Laban Effort Actions to Golf Swing Phases. This table illustrates the association between specific phases of the golf swing and the corresponding Laban Effort Actions (see Figure 1(a) for the signs of the four different phases). Each phase is characterized by distinct Effort qualities, providing a comprehensive understanding of the movement dynamics involved.
| Golf Swing Phase | Laban Effort Action | Effort Qualities | Description |
|---|---|---|---|
| Preparatory Phase | Float | Light, Sustained, Indirect | Aligning body and mind with the target direction. |
| Backswing | Glide | Light, Direct, Sustained | Smoothly lifting the club, building potential energy. |
| Transition to Downswing | Slash | Strong, Indirect, Quick | Converting potential energy into kinetic energy. |
| Downswing | Free Flow | Free Flow | Fluid, coordinated movement to maximize impact efficiency. |
Table 2.
This table provides demographic and golfing background information for the two female golfers participated in the study. It includes age, height, mass, golf handicap, experience, and annual rounds played, offering context for understanding the differences in their biomechanical data and performance outcomes.
Table 2.
This table provides demographic and golfing background information for the two female golfers participated in the study. It includes age, height, mass, golf handicap, experience, and annual rounds played, offering context for understanding the differences in their biomechanical data and performance outcomes.
| Participant | Age | Height | Mass | Handicap | Experience | Rounds |
|---|---|---|---|---|---|---|
| (years) | (cm) | (kg) | (years) | (per year) | ||
| A | 17 | 167 | 54 | 32 | 1 | 10 |
| B | 51 | 165 | 55 | 8 | 15 | 110 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated







