Measurement Error Analysis of Seawater Refractive Index Measurement Sensor Based on a Position-Sensitive Detector

Preprint

Article

Measurement Error Analysis of Seawater Refractive Index Measurement Sensor Based on a Position-Sensitive Detector

Altmetrics

Downloads

Views

Comments

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

25 June 2024

Posted:

26 June 2024

You are already at the latest version

Alerts

Abstract

The refractive index of seawater is one of the essential parameters in ocean observation, so it is necessary to achieve high-precision seawater refractive index measurements. A seawater refractive index measurement system based on position-sensitive detectors is an effective means to achieve high-precision measurement of seawater refractive index. However, in the actual seawater refractive index measurement process, the impact of the measurement error caused by the jitter signal obtained from the measurement on the measurement results cannot be ignored. In this paper, we theoretically analyse the causes of the long jitter signals during seawater refractive index measurement and quantify the influencing factors. Through the analysis, it can be seen that the angle between the two windows in the refractive index measurement area of seawater causes a great error on the measurement results, so the angle between the two windows should be controlled within 2.06° in order to effectively reduce the measurement error. At the same time, the factors affecting the measurement accuracy of the position-sensitive detector are analysed, and the factors leading to the reduction of the measurement accuracy of the position-sensitive detector are mainly the background light, the size of the photosensitive surface, and the change of the temperature of the working environment. For the above factors, we add a narrow-band filter with a bandwidth of 0.9nm in front of the photosensitive surface of the position-sensitive detector during the construction of the measurement system, so as to filter out stray light other than the signal light source. On the premise of ensuring the measuring range of the refractive index of seawater, a position-sensitive detector with a photosensitive surface size of 4 mm×4 mm was selected. In order to reduce the temperature variation of the working environment of the position-sensitive detector, the measurement system was partitioned. In order to validate the testing error range of the optimised test system, standard seawater samples under the same conditions were measured. It can be seen that the jitter signal of the measurement system has been reduced from 0.0022mm before the optimisation to 0.0011mm after the optimisation, and the deviation of the refractive index has been reduced by 1.09×10−8RIU. The experimental results show that the refractive index of seawater has been effectively reduced by adjusting the optical path and the structure of the seawater refractive index measurement system.

Keywords:

Subject: Physical Sciences - Optics and Photonics

1. Introduction

The measurement of the refractive index of seawater plays a pivotal role in ocean observations [1]. Indeed, the accurate measurement of the refractive index of seawater is of great importance in research areas such as seawater flow field, ocean climate prediction and seabed resource exploration [2,3]. The most recent definition of the thermodynamic equations of seawater (TEOS-10) was proposed by the UNESCO/IOC SCOR/IAPSO Working Group 127 (WG127). This definition is based on a Gibbs potential function of absolute salinity, temperature and pressure and focuses on the assessment of absolute salinity [4]. The traditional method of seawater salinity measurement is to utilise a conductivity sensor to ascertain the conductivity of seawater, after which the salinity is calculated by means of the formula [5,6]. Nevertheless, the silicates present in the oceans are non-ionic compounds that do not conduct electricity. Consequently, there is a bias in the measurement of seawater salinity using conductivity sensors [7]. In such a scenario, the use of an optical method for measuring the refractive index is a beneficial approach.

Among the various optical measurement techniques for seawater refractive index, the technique based on a position-sensitive detector has become a prominent approach for high-sensitive measurements due to its advantages, including minimal influence by environmental factors and contactless measurement. In 2003, Y. Zhao et al. proposed a fibre-optic sensor for remote monitoring of salinity in water for laboratory applications. The sensor is based on the detection of beam deflection caused by changes in the refractive index of seawater, and is capable of measuring salinity using a position-sensitive detector. The salinity measurement resolution was 0.012 g kg^-1, with a system instability of 0.9 per cent [8]. In 2009, D. Malardé et al. presented a compact optical refractive index sensor that has been applied to the in situ detection of the refractive index of seawater. The sensor is based on the principle of light refraction and employs a V-block optical structure. The device is capable of measuring the refractive index of seawater with a resolution of

4 \times 10^{- 7} R I U

[9]. A novel optical measurement structure, based on a position-sensitive detector, has been developed with the objective of enabling higher sensitivity seawater refractive index measurements on the order of 10^-9 RIU. This allows for the development of a high-sensitivity seawater refractive index measurement system. In 2024, a method for measuring the refractive index of seawater based on a PSD was proposed. A theoretical model was developed to illustrate the relationship between laser spot displacement and refractive index change, utilising a combination of a position-sensitive detector and laser beam deflection principles. The optical measurement method was employed to establish a seawater refractive index measurement system [10]. Nevertheless, a number of additional factors may contribute to inaccuracies in the measurement of the refractive index of seawater using a position sensitive detector. These include the configuration of the optical path within the region where the refractive index is being measured, the temperature of the system, the noise generated by the detector, and the uncertainty associated with the signal processing procedure. In the course of developing highly sensitive seawater refractive index measurement techniques, researchers have been striving to eliminate these measurement errors [11].

The current focus of research in the field of position-sensitive detector-based measurement systems is the analysis and reduction of the influence of various factors on the measurement accuracy of the position-sensitive detector. These include the output response linearity of the position-sensitive detector [12], the injection current [13], the laser spot size [14] and other factors. In the event of erroneous measurement results resulting from the presence of jitter signals, compensation systems are frequently employed in order to obtain more accurate measurement signals [15]. Consequently, more effective solutions must be developed to achieve more accurate measurements while maintaining the optimal condition of the position-sensitive detector.

Based on these new application requirements, an analysis was conducted to identify the underlying causes of the measurement system error, specifically with regard to the existing PSD-based seawater refractive index measurement system. Firstly, a theoretical analysis was conducted to identify the error factors generated in the optical path transmission process of the seawater refractive index measurement system based on a position-sensitive detector. In order to ensure the accuracy of the seawater refractive index gradient measurement, it is essential to guarantee that the laser incident window and the laser receiving window, which collectively constitute the measurement zone, are perfectly parallel to one another. Furthermore, it is of paramount importance that the laser is incident perpendicularly upon the measurement zone. Consequently, the impact of the angle between the two windows in the measurement area and the angle of laser incidence on the sensitivity of the refractive index gradient measurement were analysed. The findings indicate that the angle between the two windows should be maintained within a narrow range of 2.06° to ensure that the sensitivity of the refractive index gradient measurement is not compromised by its angle. And the angle of laser incidence should be maintained within a narrow range of 2.06°. Then, a theoretical analysis was conducted to investigate the factors influencing the internal environment of the seawater refractive index measurement system. The analysis revealed that the interior of the measurement system was affected by temperature and atmospheric pressure. Based on the results of the analysis, an experimental study was then carried out to verify the findings. Concurrently, an analysis was conducted to identify the factors influencing the performance of the position-sensitive detector. Based on this analysis, an improvement plan was developed to address the errors caused by these influencing factors, with the objective of ensuring that the position-sensitive detector operates at its optimal level. Finally, an experimental comparison and analysis was conducted to assess the stability of the seawater refractive index measurement system before and after reducing the error factor. The experimental results demonstrate that the jitter signal of the seawater refractive index measurement system has been reduced from

\pm 0.0022 mm

\pm 0.0011 m m

, and the deviation of the refractive index has been reduced by

1.09 \times 10^{- 8} R I U

. While the standard deviation of the seawater refractive index has been reduced from

10^{- 8}

10^{- 10} R I U

. The experimental results show that the refractive index of seawater has been effectively reduced by adjusting the optical path and the structure of the seawater refractive index measurement system.

2. Principle of the Refractive Index Optical Measurement System

The optical seawater refractive index measurement adopts the laser beam deviation technique, and the schematic diagram of seawater refractive index measurement system based on a position-sensitive detector is shown in Figure 1.

The light emitted by the laser passes through the laser-incidence window and reaches the area where the refractive index of seawater is measured. The refractive index gradient of seawater gives rise to a refraction phenomenon when the laser interacts with the seawater. Subsequently, the laser traverses the laser-receiving window, whereupon it is focused by the lens to a single point on the photosensitive surface of the PSD. Consequently, the coordinates of the laser spot are determined by the PSD.

The geometric theory of light refraction represents an approximation of the physical optics approach. It assumes that the measurement system optical path is a two-dimensional optical path that is isotropic in the x and y directions. However, since the optical path is deflected by the refractive index gradient in the y-direction when the laser enters the measurement zone, only the refractive index gradient in the y direction is considered. The refraction of light caused by inhomogeneity is proportional to the gradient of refractive index in each direction in the y-planes. Consequently, the deflection rate of light rays can be expressed as in [16], as follows:

\frac{\partial^{2} y}{\partial z^{2}} = \frac{1}{n} \frac{\partial n}{\partial y}

(1)

where n is the refractive index, z is the direction of light propagation, and the curvature of the refracted light

\frac{\partial^{2} y}{\partial z^{2}}

is represented by the gradient of the refractive index

\frac{\partial n}{\partial y}

of seawater.

The measured refractive index of the seawater can be expressed as follows [10]:

n = n_{0} + δ_{y} \frac{D_{y}}{f L}

(2)

where n₀ is the refractive index of the surrounding seawater, the

δ_{y}

is the displacement of the light ray in the direction of y axis, the

D_{y}

is the displacement on the PSD photosensitive surface, the L is the distance between the laser-incidence window and the laser-receiving window, the f is the focal length of the focusing lens.

As demonstrated by Equation (2), the sensitivity of a seawater refractive index measurement system based on a position-sensitive detector is contingent upon the length of the refractive index measurement zone, the focal length of the focusing lens, and the displacement on the PSD photosensitive surface. Therefore, the angle between the two windows that comprise the refractive index measurement area can result in the refraction of light during the transmission of the optical path, which in turn can lead to a deviation in the displacement on the PSD photosensitive surface. This can subsequently give rise to an error in the results of the refractive index measurement.

3. Study of the Errors Induced by the Optical Path of the Seawater Refractive Index Measurement System

During the transmission of the optical path of the seawater refractive index measurement system, an angle between the laser-incident window and the laser-receiving window that comprise the refractive index measurement area results in the laser light refraction phenomenon, which in turn leads to an error in the seawater refractive index measurement. The optical path transmission process is depicted in Figure 2. The laser is incident at a right angle to the main optical axis, and the first refraction occurs from the air medium through the first surface of the glass of the laser-incident window. The second refraction occurs from the glass of the laser-incident window to the refractive index measurement area of seawater. The third refraction occurs from the refractive index measurement area of seawater through the first surface of the laser-receiving window. Finally, the fourth refraction occurs from the glass of the laser-receiving window to the air medium. Following four refractions, the laser reaches the photosensitive surface of the PSD, at which point the position coordinates of the laser spot are obtained.

The law of refraction of light indicates that the angle of incidence

α

of the laser is refracted by the refractive index

n_{a}

of the air medium and the refractive index

n_{g}

of the window glass, resulting in the refractive angle

i_{1}

. This can be expressed as follows:

n_{a} \sin α = n_{g} \sin i_{1}

(3)

The laser is incident at an angle of incidence

i_{1}

from the window glass with a refractive index of

n_{g}

to the refractive index measurement area of seawater with a refractive index of

n_{s}

, producing an angle of refraction of

2 α - i_{2}

, and a refractive relation expression:

n_{g} \sin i_{1} = n_{s} \sin (2 α - i_{2})

(4)

The laser is incident at an angle of incidence

i_{2}

from the refractive index measurement area of seawater with a refractive index of

n_{s}

to the window glass with a refractive index of

n_{g}

, which produces an angle of refraction of

i_{3}

. The refractive relation expression is as follows:

n_{s} \sin i_{2} = n_{g} \sin i_{3}

(5)

The laser is incident at an angle of incidence

i_{3}

from a window glass with a refractive index of

n_{g}

to an air medium with a refractive index of

n_{a}

, producing an angle of refraction of

A

. The expression for the refraction relation is:

n_{g} \sin i_{3} = n_{a} \sin A

(6)

It can be further obtained from Equations (3) and (4):

n_{a} \sin α = n_{s} \sin (2 α - i_{2})

(7)

It can be further obtained from Equations (5) and (6):

n_{s} \sin i_{2} = n_{a} \sin A

(8)

The differentiation of Equations (7) and (8) can be obtained as follows:

{∆ n}_{s} ∆ i_{2} = ∆ n_{s} \tan (2 α - i_{2})

(9)

∆ n_{s} \sin i_{2} + n_{s} ∆ i_{2} \cos i_{2} = n_{a} ∆ A \cos A

(10)

Substitution of Equation (9) into Equation (10) and subsequent collation yields the following equation:

∆ n_{s} = \frac{n_{a} ∆ A \cos A}{\sin i_{2} + \cos i_{2} \tan (2 α - i_{2})}

(11)

Among others:

i_{1} = a r c \sin (\frac{n_{a} \sin α}{n_{g}})

(12)

i_{2} = 2 α - a r c \sin (\frac{n_{a} \sin α}{n_{s}})

(13)

i_{3} = a r c \sin (\frac{n_{s} \sin i_{2}}{n_{g}})

(14)

\cos A = \sqrt{1 - {(\frac{n_{g} \sin i_{3}}{n_{a}})}^{2}}

(15)

When the angle between the two windows is

2 α

and the length of the seawater refractive index measurement zone

L_{1}

, the laser is incident at the position

d_{1}

of the laser-incident window opening is known according to the trigonometric relationship:

d_{1} = \frac{2 \sin α}{L_{1}}

(16)

The position designated

d_{2}

, at which the laser exits the laser-incidence window, is defined as follows:

d_{2} = d_{1} - t_{i} \tan i_{1}

(17)

Where

t_{i}

is the thickness of the glass of the laser-incidence window.

The position of the laser light upon incidence upon the laser-receiving window following its passage through the refractive index measurement zone of seawater is designated as

d_{3}

, and it is expressed as follows:

d_{3} = d_{2} (\cos 2 α + \sin 2 α t a n i_{2})

(18)

The position designated

d_{4}

, at which the laser exits the laser-receiving window, is defined as follows:

d_{4} = d_{3} + t_{r} \tan i_{3}

(19)

Where

t_{r}

is the thickness of the glass of the laser-receiving window.

The position D of the received spot on the photosensitive surface can be expressed as follows:

D = d_{4} \cos α + ({L_{2} - d}_{4} \sin α) \tan (A - α)

(20)

Where

L_{2}

represents the distance between the apex of the second surface of the laser receiving window and the PSD photosensitive surface.

The differentiation of Equation (20) yields:

∆ D = ∆ d_{4} \cos α - ∆ d_{4} \sin α \tan (A - α) + ({L_{2} - d}_{4} \sin α) ∆ \tan (A - α)

(21)

Therefore,

\frac{∆ D}{∆ n_{s}}

can be expressed as follows:

\frac{∆ D}{∆ n_{s}} = \frac{∆ d_{4} \cos α - ∆ d_{4} \sin α \tan (A - α) + ({L_{2} - d}_{4} \sin α) ∆ \tan (A - α)}{n_{a} ∆ A \cos A} \times \frac{\sin i_{2} + \cos i_{2} \tan (2 α - i_{2})}{n_{a} ∆ A \cos A}

(22)

Among others:

∆ \tan (A - α) = \frac{2 ∆ A}{1 + \cos (2 A - 2 α)}

(23)

The relationship between the refractive index of the seawater and the angle between the two window glasses in the measurement area is shown in Figure 3. According to the existing refractive index optical measurement system can be known, the selection of k9 glass as seawater refractive index measurement area of the two window glass, so the window glass refractive index

n_{g} = 1.5168

, and the window thickness

t_{i} = t_{r} = 2 m m

. The length of the seawater refractive index measurement area

L_{1} = 100 m m

. The distance between the apex of the second surface of the laser receiving window and the PSD photosensitive surface

L_{2} = 300 m m

. The refractive index of air under ambient conditions at standard atmospheric pressure and a temperature of 25 ℃ is

n_{a} = 1.0003

. Initial refractive index of seawater at a salinity of 35 psu

n_{s} = 1.3381096

. The sensitivity of the PSD is

∆ D = 10^{- 3} m m

, and the angle between the two window glasses in the measurement area is set to be

0.1 ° \leq α \leq 4 °

. Given that the PSD-based optical measurement system for refractive index of seawater has a sensitivity of

9.93 \times 10^{- 9} R I U

, it is crucial to regulate the measurement error resulting from the angle between the two window glasses in the measurement area to be less than

9.93 \times 10^{- 9} R I U

, in order to ensure that this measurement error range is satisfied when the angle

2 α < 2.06 °

. It can be demonstrated that the measurement error range is satisfied when

∆ D / ∆ n_{s} < 107.875 μ m / μ R I U

. The refractive index measurement sensitivity changes by

3 \times 10^{- 10} R I U

for every 0.01° change in the angle between the two window glasses in the measurement area.

4. Noise Analysis and Measurement Error Analysis of the Position-Sensitive Detector

The operational principle of the PSD measurement position is shown in Figure 4. When the laser irradiates the photosensitive surface of the PSD, electrons are excited from the valence band to the conduction band through the photovoltaic effect, thus generating a photocurrent.The PSD is a PIN structure, with the light-sensitive surface being the P layer (a uniformly distributed resistive layer), the N layer being connected to the common electrode, and the electrodes on both sides being used to extract the positional signals.

When the centre of the PSD is taken as the reference point, the laser spot irradiates the PSD photosensitive surface. This results in the generation of corresponding photocurrents by electrodes 1 and 2. The relationship between the electrode output currents and the position of the incident spot can be expressed as follows:

The output current of electrode 1 is:

I_{1} = I_{0} \frac{\frac{L_{Y}}{2} - Y_{A}}{L_{Y}}

(24)

The output current of electrode 2 is:

I_{2} = I_{0} \frac{Y_{B}}{L_{Y}}

(25)

Where

I_{1}

is the output current of electrode 1,

I_{2}

is the output current of electrode 2,

I_{0}

is the total output photocurrent of electrode 1 and electrode 2,

L_{Y}

is the length of the photosensitive surface,

Y_{A}

is the distance from the incident position of the laser spot to the centre of the photosensitive surface, and

Y_{B}

is the distance from the incident position of the laser spot to electrode 1.

The positional resolution of the PSD is determined by the resistor length

L_{Y}

and the signal-to-noise ratio, and the following equation can be established according to Equation (25):

∆ Y = \frac{∆ I L_{Y}}{I_{0}}

(26)

Where

∆ I

is the amount of output current change,

∆ Y

is the tiny displacement.

In the case of infinitely small position displacements, the amount of noise contained in the output current signal

I_{2}

determines the position resolution. If the noise current of the PSD is

I_{n}

, the position resolution

∆ R

of the PSD can be expressed as:

∆ R = \frac{I_{n} L_{Y}}{I_{0}}

(27)

According to Equation (27), in order to improve the resolution of the position of the PSD, it can be done by decreasing the length of the photosensitive surface

L_{Y}

or increasing the signal photocurrent. In addition to this, PSD also has non-linear effects such as output current of electrodes at both ends and light intensity of incident spot, characteristic differences in signal amplification and processing in the subsequent processing circuit, and A/D conversion errors. Segmented optimisation BP networks and multilayer conjugate gradient optimisation algorithms are applied for nonlinear correction.

Noise exists in the PSD during operation, so the shot noise, thermal noise and operational amplifiers of the PSD are analysed according to the noise equivalent model of the PSD, and the root mean square of the total noise voltage is calculated for the case where the feedback resistor is considered.

The shot noise in PSD mainly originates from the photocurrent and dark current and can be expressed as:

I_{s} = \sqrt{2 q \times (I_{0} + I_{D}) \times B}

(28)

Where

q

is the electronic charge,

I_{D}

is the dark current,

B

is the bandwidths.

When the ratio of the interpole resistance to the feedback resistance is greater than 0.1, the feedback resistance is non-negligible, and the shot noise output voltage

V_{s}

is:

V_{s} = R_{f} \sqrt{2 q \times (I_{0} + I_{D}) \times B}

(29)

Where

R_{f}

is the feedback resistance.

In PSD, the random movement of charge carriers in the resistive material due to temperature changes causes thermal noise. The thermal noise current

I_{j}

generated by the inter-pole resistance is:

I_{j} = \sqrt{\frac{4 k T B}{R_{i e}}}

(30)

Where

k

is the Boltzmann constant,

T

is the absolute temperature at which the PSD operates, and

R_{i e}

is the inter-electrode resistance.

When the ratio of the interpole resistance to the feedback resistance is greater than 0.1, the feedback resistance is non-negligible, and the thermal noise output voltage,

V_{j}

, generated by the resistance between the poles can be expressed as follows:

V_{j} = R_{f} \sqrt{\frac{4 k T B}{R_{i e}}}

(31)

The operational amplifier equivalent noise input current

I_{e n}

can be expressed as:

I_{e n} = \frac{V_{e} \sqrt{B}}{R_{i e}}

(32)

Where

V_{e}

is the noise input voltage of the operational amplifier.

When the ratio of the interpole resistance to the feedback resistance is greater than 0.1, the feedback resistance is non-negligible, and the noise generated by the operational amplifier equivalent noise input voltage corresponds to the output voltage

V_{e n}

denoted as:

V_{e n} = (1 + \frac{R_{f}}{R_{i e}}) I_{e n} \sqrt{B}

(33)

The voltage

V_{R f}

corresponding to the thermal noise of the feedback resistor is expressed as:

V_{R f} = R_{f} \sqrt{\frac{4 k T B}{R_{i e}}}

(34)

The equivalent noise voltage

V_{i n}

of the operational amplifier is:

V_{i n} = R_{f} I_{n} \sqrt{B}

(35)

Where the noise current RMS value

I_{n}

of the PSD is expressed as:

I_{n} = \sqrt{I_{s}^{2} + I_{j}^{2} + I_{e}^{2}}

(36)

Therefore, the RMS value of the PSD noise voltage due to the operational amplifier equivalent noise input is expressed as:

V_{n} = \sqrt{V_{s}^{2} + V_{j}^{2} + V_{e n}^{2} + V_{R f}^{2} + V_{i n}^{2}}

(37)

According to the PSD used in the existing seawater refractive index measurement system, it can be seen that when the bias voltage is

5 V

and the load resistance is

1 k Ω

, the corresponding saturation photocurrent

I_{n}

is 10^-4 A and the bandwidth B is 10 Hz. In the operational amplifier, the feedback resistor

R_{f}

100 k Ω

, the typical value of the inter-polar resistor

R_{i e}

50 k Ω

, and the equivalent noise input voltage

V_{e}

is 0.003 V. And the electron charge

q

1.6 \times 10^{- 19} C

, the Boltzmann’s constant

k

1.38 \times 10^{- 23} J / K

. Figure 5a represents the curve of PSD noise current with temperature change from 20 to 25°C, and the noise current increases gradually with the increase of folding temperature. Figure 5b represents the PSD noise voltage with the operating temperature from 20 to 25 °C change curve, with the increase of fold temperature noise voltage gradually increases. Figure 5c represents the PSD position resolution with the operating temperature from 20 to 25 °C change curve, since the photosensitive side of the PSD is 4 mm long, and according to the formula (27) can be seen, with the increase of folding temperature PSD position resolution gradually increases, will lead to PSD measurement accuracy is reduced.

At the same time, there are some factors that can affect the PSD measurement accuracy during the refractive index measurement of seawater. When there is background light in the spot incident on the PSD photosensitive surface, this will result in an error in the position measurement. Therefore, a filter is added to the PSD photosensitive surface to filter out the background light other than the signal light. The spot size incident on the PSD photosensitive surface directly affects the repeatability of PSD positioning, and since the photocurrent is proportional to the irradiance on the PSD, the repeatability of PSD positioning is bound to be reduced when the spot size is large. In the seawater refractive index measurement system, a focusing lens is used to converge the light spots, thus ensuring the repeatability of PSD positioning. In the measurement system, it is difficult for the beam symmetry axis to always be parallel to the normal of the PSD photosensitive surface, so the projection of the circular spot on the PSD photosensitive surface is elliptical, which is equivalent to a large spot incidence, and the repeatability of the PSD positioning is necessarily reduced as a result.

5. Structural Optimisation of a Seawater Refractive Index Measurement System Based on a PSD

The seawater refractive index optical measurement system is based on a PSD (TEM Messtechnik GmbH, Hannover, German), and its optimised optical path structure is shown in Figure 6.

Due to the laser propagation in seawater there are light absorption and scattering phenomena, comprehensive consideration of seawater attenuation of light, choose the wavelength of 532nm laser as the signal light source. To ensure that the two window glasses in the measurement area remain parallel and that the laser is incident parallel to the main optical axis, the optical path of the measurement system is adjusted using a parallel light pipe. To reduce errors in the propagation of the optical path, the number of optical lenses has been reduced while maintaining the sensitivity of the refractive index measurement. To avoid position measurement errors caused by ambient background light, a narrow band filter with the same peak wavelength and a bandwidth of 0.9 nm was placed in front of the PSD photosensor to remove stray light outside the 532 nm measurement light. In order to improve the accuracy of PSD measurement, under the premise of ensuring the measurement range of seawater refractive index, the seawater refractive index measurement system selects the position sensitive detector with the size of

4 m m \times 4 m m

photosensitive surface as the measurement sensor. During the refractive index measurement process, the thermal noise and dark current of the PSD will change with the operating temperature, resulting in differences in the measurement accuracy of the PSD at different operating temperatures. Therefore, in the design of the structure of the seawater refractive index measurement system, the signal processing module that causes the temperature to change is processed in a partition with the PSD. The seawater refractive index measurement system was divided into two parts, an optical and an electrical section, to reduce the variation of the optical path and the PSD operating temperature. The optimised seawater refractive index measurement system is physically shown in Figure 7.

6. Stability Experiment of Seawater Refractive Index Measurement System

6.1. Experimental Setup

The stability of the seawater refractive index measurement system is an important indicator of reliability and reflects the accuracy of the system’s measurements. The stability experiment of seawater refractive index measurement system was carried out in an indoor glass water cylinder with constant temperature and pressure levels, and the experimental scenario is shown in Figure 8.

The seawater refractive index measurement system was mounted upside down, and the seawater refractive index detection area was immersed in standard refractive index seawater. The experiments were carried out using standard seawater from the National Centre for Marine Standards and Metrology of China for the Stability experiment of seawater refractive index measurement system. In the experiments, the sampling frequency of the seawater refractive index measurement system was 10kHz, and the salinity of standard seawater is 35.003 PSU, the temperature of the seawater samples was controlled to be 26 °C, the laboratory was a standard atmospheric pressure of 10.1325 dbar. The refractive index of standard salinity seawater is shown in Table 1.

6.2. Experimental Results of the Stability Experiment

In the experiments, the seawater refractive index measurement system employs a sampling time of 60 s. The position signal measured by the seawater refractive index measurement system before controlling the temperature of the PSD working zone by structural partitioning is shown in Figure 9 (a). The average value of the laser spot position data for this experiment was -0.0571 mm. According to the results of the seawater refractive index measurement experiment with standard salinity seawater, the jitter signal of the system was

\pm 0.0022 mm

. Based on the measurement sensitivity of the system, the refractive index deviation, due to stability, was calculated as

\pm 1.19 \times 10^{- 8} R I U

, since the system refractive index measurement sensitivity was

9.93 \times 10^{- 9} R I U

. After controlling the temperature of the PSD working zone by structural partitioning, the position signal measured by the seawater refractive index measurement system is shown in Figure 9b. The average value of the laser spot position data for this experiment was -0.0575 mm. According to the results of the seawater refractive index measurement experiment with standard salinity seawater, the jitter signal of the system was

\pm 0.0018 m m

. Based on the measurement sensitivity of the system, the refractive index deviation, due to stability, was calculated as

\pm 7.944 \times 10^{- 9} R I U

, since the system refractive index measurement sensitivity was

9.93 \times 10^{- 9} R I U

. The position signals measured by the seawater refractive index measurement system with the optimised angular structure between the two measurement windows are shown in Figure 9c. The average value of the laser spot position data for this experiment was -0.0574 mm. According to the results of the seawater refractive index measurement experiment with standard salinity seawater, the jitter signal of the system was

\pm 0.0011 m m

. Based on the measurement sensitivity of the system, the refractive index deviation, due to stability, was calculated as

\pm 9.93 \times 10^{- 10} R I U

, since the system refractive index measurement sensitivity was

9.93 \times 10^{- 9} R I U

By comparing and analysing the position signals obtained from seawater under the same conditions before and after the optimisation of the seawater refractive index measurement system, it can be seen that the jitter signal of the measurement system has been reduced from 0.0022mm before the optimisation to 0.0011mm after the optimisation, and the deviation of the refractive index has been reduced by

1.09 \times 10^{- 8} R I U

. The experimental results show that the refractive index of seawater has been effectively reduced by adjusting the optical path and the structure of the seawater refractive index measurement system.

7. Conclusions

In this paper, we theoretically analyse the causes of the long jitter signals during seawater refractive index measurement and quantify the influencing factors. Through the analysis, it can be seen that the angle between the two windows in the refractive index measurement area of seawater causes a great error on the measurement results, so the angle between the two windows should be controlled within 2.06° in order to effectively reduce the measurement error. At the same time, the factors affecting the measurement accuracy of the position-sensitive detector are analysed, and the factors leading to the reduction of the measurement accuracy of the position-sensitive detector are mainly the background light, the size of the photosensitive surface, and the change of the temperature of the working environment. For the above factors, we add a narrow-band filter with a bandwidth of 0.9nm in front of the photosensitive surface of the position-sensitive detector during the construction of the measurement system, so as to filter out stray light other than the signal light source. On the premise of ensuring the measuring range of the refractive index of seawater, a position-sensitive detector with a photosensitive surface size of

4 m m \times 4 m m

was selected. In order to reduce the temperature variation of the working environment of the position-sensitive detector, the measurement system was partitioned.In order to validate the testing error range of the optimised test system, standard seawater samples under the same conditions were measured. It can be seen that the jitter signal of the measurement system has been reduced from 0.0022mm before the optimisation to 0.0011mm after the optimisation, and the deviation of the refractive index has been reduced by

1.09 \times 10^{- 8} R I U

. The experimental results show that the refractive index of seawater has been effectively reduced by adjusting the optical path and the structure of the seawater refractive index measurement system.

Author Contributions

Conceptualization, G.Z.; validation, G.Z.; formal analysis, G.Z.; investigation, L.L.; resources, G.Z.; writing—original draft, G.Z.; supervision, X.C. and Y.Z.; project administration, L.L.; funding acquisition, Y.Z. All authors have read and agreed to the published version of the manuscript..

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data are contained within the article.

Conflicts of Interest

The authors declare no conflicts of interest.

References

Watada, S. Tsunami speed variations in density-stratified compressible global oceans. Geophys. Res. Lett. 2013, 40, 4001–4006. [Google Scholar] [CrossRef]
Yang, YP.; Liu, YH.; Wang, YH.; Zhang, HW.; Zhang, LH. Dynamic modeling and motion control strategy for deep-sea hybrid-driven underwater gliders considering hull deformation and seawater density variation. Ocean eng. 2017, 143, 66–78. [Google Scholar] [CrossRef]
Zhang, SW.; Li, LY.; Liu, YL.; Zhou, Y. Drift Error Compensation Algorithm for Heterodyne Optical Seawater Refractive Index Monitoring of Unstable Signals. Sensers 2023, 23, 8460. [Google Scholar] [CrossRef]
Pawlowicz, R.; Feistel, R. Limnological Applications of the Thermodynamic Equation of Seawater 2010 (TEOS-10). Limnol. Oceanogr. Methods 2012, 10, 853–867. [Google Scholar] [CrossRef]
Johnson, G.C.; Toole, J.M.; Larson, N.G. Sensor Corrections for Sea-Bird SBE-41 CP and SBE-41 CTDs. J. Atmosph. & Ocean. Technol. 2007, 24, 1117–30. [Google Scholar]
Grosso, P.; Le Menn, M.; De Bougrenet De La Tocnaye, J.L.; Wu, Z.Y.; Malardé, D. Practical versus absolute salinity measurements: New advances in high performance seawater salinity sensors. Deep Res. Part I Oceanogr. Res. Pap. 2010, 57, 151–156. [Google Scholar] [CrossRef]
Le Menn, M.; de la Tocnaye, J. L. de Bougrenet; Grosso, P.; Delauney, L.; Podeur, C.; Brault, P.; Guillerme, O. Advances in measuring ocean salinity with an optical sensor. Meas. Sci. Technol. 2011, 22, 115202. [Google Scholar] [CrossRef]
Zhao. Y.; Liao.Y.B.; Zhang.B.; Lai.SR. Monitoring technology of salinity in water with optical fiber sensor. J. Light. Technol. 2003, 21, 1334–1338. [Google Scholar] [CrossRef]
Malardé, D.; Wu, ZY.; Grosso, P.; de la Tocnaye, JLD. ; Le Menn, M. High-resolution and compact refractometer for salinity measurements. Meas. Sci. Technol. 2009, 20, 015204. [Google Scholar] [CrossRef]
Zhou, GL.; Li, LY.; Zhou, Y.; Chen, XY. High-Sensitivity Seawater Refraction Index Optical Measurement Sensor Based on a Position-Sensitive Detector. Sensers 2024, 24, 2050. [Google Scholar] [CrossRef] [PubMed]
Olyaee, S.; Nejad, S.M. Nonlinearity and frequency-path modelling of three-longitudinal-mode nanometric displacement measurement system. IET Optoelectron. 2007, 1, 211–220. [Google Scholar] [CrossRef]
Golnabi, H. Linearity in output signal of optical position-sensing detector systems. Opt. Laser Technol. 2000, 32, 311–316. [Google Scholar] [CrossRef]
Niu, FF.; Liu, ZY.; O’Neil, D.; Dashtgard, S.; Liu, JT. Study of a novel density well-logging tool using A position-sensitive detector. Appl. Radiat. Isotopes. 2019, 154. [Google Scholar] [CrossRef] [PubMed]
Cheng, SW.; Liu, J.; Li, Z.; Zhang, PC.; Chen, JQ.; Yang, HM. 3D error calibration of spatial spots based on dual position-sensitive detectors. Appl. Optics. 2023, 62, 933–943. [Google Scholar] [CrossRef] [PubMed]
Saha, A.; Das, S.; Suresh, M.; Kiran, VR. FPGA based self-vibration compensated two dimensional non-contact vibration measurement using 2D position sensitive detector with remote monitoring. Measurement. 2017, 111, 271–278. [Google Scholar] [CrossRef]
Burton, R Ashby. The application of Schlieren photography in fluid flow and heat transfer analysis. M.S. M.E., University of Texas, Austin, 1951.

Figure 1. Schematic diagram of seawater refractive index measurement system based on a position-sensitive detector.

Figure 2. The optical path transmission process is depicted.

Figure 3. Relationship between the measurement error of the refractive index measurement system and the angle between the two window glasses in the measurement area.

Figure 4. The operating principle of a position-sensitive detector.

Figure 5. (a) Relationship between operating temperature and PSD noise current. (b) Relationship between operating temperature and PSD noise voltage. (c) The effect of operating temperature on the positional resolution of the PSD.

Figure 6. Optical path diagram of a seawater refractive index measurement system after optical path optimisation.

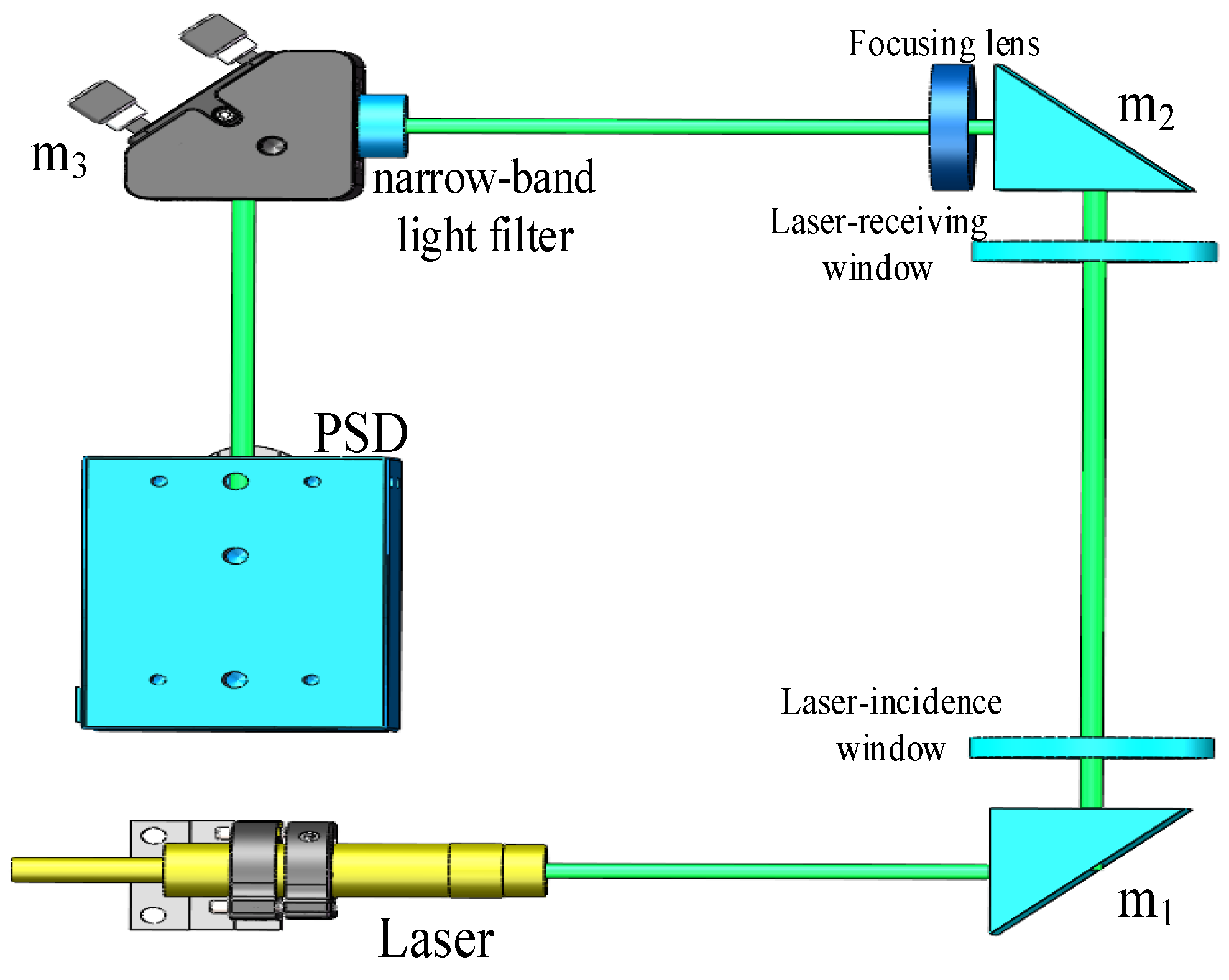
Figure 7. Optimised seawater refractive index measurement system.

Figure 8. Experimental setup for the Stability experiment of seawater refractive index measurement system.

Figure 9. (a) The position signal measured by the seawater refractive index measurement system before controlling the temperature of the PSD working zone by structural partitioning. (b) The position signal measured by the seawater refractive index measurement system after controlling the temperature of the PSD working zone by structural partitioning. (c) The position signals measured by the seawater refractive index measurement system with the optimised angular structure between the two measurement windows.

Table 1. Refractive index of standard salinity seawater.

Salinity (PSU)	Temperature (°C)	Pressure (dbar)	Refractive Index (RIU)
35.003	26.00	10.1325	1.3381096

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Measurement Error Analysis of Seawater Refractive Index Measurement Sensor Based on a Position-Sensitive Detector

Abstract

1. Introduction

2. Principle of the Refractive Index Optical Measurement System

3. Study of the Errors Induced by the Optical Path of the Seawater Refractive Index Measurement System

4. Noise Analysis and Measurement Error Analysis of the Position-Sensitive Detector

5. Structural Optimisation of a Seawater Refractive Index Measurement System Based on a PSD

6. Stability Experiment of Seawater Refractive Index Measurement System

6.1. Experimental Setup

6.2. Experimental Results of the Stability Experiment

7. Conclusions

Author Contributions

Funding

Institutional Review Board Statement

Informed Consent Statement

Data Availability Statement

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe