Is Dark Matter a Misinterpretation of a Perspective Effect?

Preprint

Article

Is Dark Matter a Misinterpretation of a Perspective Effect?

Altmetrics

Downloads

Views

Comments

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

26 June 2024

Posted:

28 June 2024

You are already at the latest version

Alerts

Abstract

Very recently, a straightforward way was proposed in order to understand the galaxy and galactic cluster world without using the very elusive dark matter concept. This way was called κ-model [1, 2, 3, 4]. The main idea is to save the form of the usual physical laws, especially the Newton’s laws of motion when gravity is weak, but only by applying a local renormalization procedure for the lengths, distances and velocities. This local renormalization appears as a correspondance principle in the κ-model. In this model the fundamental physical constants remain universal, i.e. are independent of the point and of time. The κ-model is Newtonian in essence but a relativistic extension can easily be built. The aim of the present paper is to detail the mathematical formalism supporting i

Keywords:

Subject: Physical Sciences - Astronomy and Astrophysics

1. Introduction

The classical laws of physics (for instance the Newtonian dynamics) have been defined at the meter scale. This scale goes from the sub-micrometric dimension to the scale of the solar system. However, we know that at the scale of the nanoscopic world these classical laws are no longer valid and the quantum field theory has to be used. Let us note that the ratio between the radius of an atom and the base unit length (meter) is

10^{- 10}

. Despite this statement, when we go now in the opposite direction, i.e. the macrocosmos world, the physicists use the same physical laws than those valid at the meter scale, with no changes, whereas the ratio between one meter and one parsec is

10^{- 16}

. The

κ

-model relies on the very simple suggestion that the matter does no longer behave on the same manner when the characteristic dimension of the region under study is very large (of the order of one parsec or more). The perception of an observer must then necessarily be changed [1, 2, 3, 4]. Our aim is also to reduce the modification compared to the Newtonian law at the minimal level. The main idea is that the environment of the observer modifies his perception, a bit like when this one or the observed object are immersed in different media with various refractive indices (even though the analogy can strongly be misleading). Furthermore the lengths and the velocities which are measured by him are differently renormalized following the mean densities surrounding him and the observed object. Let us note that it relates only to apparent effects, the unit of length (for instance the radius of a hydrogen atom) is obviously the same everywhere in the Universe. The renormalization coefficient, labelled

κ

, is linked to the local mean density

ρ

by a simple relationship

κ = 1 / (1 + L n (1 / ρ)))

assuming

ρ < 1

with appropriate normalization [2, 3]. In section 2 some basic mathematical concepts are recalled, section 3 develops the framework of the

κ

-model, in section 4 the

κ

-structure for Minkowski space is presented, Eventually in section 5 a few illustrative applications to astrophysics are supplied. A few didactic figures have also been added.

2. Some Basic Structures on $R^{n}$

2.1. Algebraic Structures

2.1.1. Affine and Vectorial Structure

When endowed with its usual vector space structure the set

R^{n}

of the real n-tuples will be noted

R^{n}

and when endowed with its affine structure it will be denoted simply by

R^{n}

. The affine structure being a 1-transitive action of the additive group

(R^{n}, +)

R^{n}

\forall x \in R^{n}, \forall u \in R^{n}, (x, u) \mapsto x + u .

When

x + u = y

we will sometimes write

u = y - x

2.1.2. Euclidean Structures

The vector space

R^{n}

is equipped with a Euclidean structureonce a scalar producton

R^{n}

has been selected. A scalar product is a definite positive bilinear symmetric form, in other words it is an application

〈 ., . 〉 : R^{n} \times R^{n} \to R

linear in each variable, symmetric and satisfying

\forall h \in R^{n}, 〈 h, h 〉 \geq 0

, the cancellation hapenning only when

h = 0

This means that in some base

B = (e_{1}, \dots, e_{n})

R^{n}

the matrix representing the bilinear form

〈 ., . 〉

i.e.the matrix

{(g_{i j})}_{1 \leq i, j \leq n}

where

g_{i j} = 〈 e_{i}, e_{j} 〉

I d_{n} = d i a g (1, \dots, 1)

which means that

g_{i j} = δ_{i j}

, the Kronecker symbol.

h = h^{i} e_{i}

and

k = k^{i} e_{i}

we have then

〈 h, k 〉 = h^{i} δ_{i j} k^{j} = \sum_{i = 1}^{n} h^{i} k^{i}

(1)

Such a base of

R^{n}

is an orthonormalbase (with respect to the particular scalar product considered).

The application

R^{n} \to R, h \mapsto ∥ h ∥ = \sqrt{〈 h, h 〉}

(2)

is a normon

R^{n}

in other words it fullfills the conditions :

- Positivity (

\forall x \in R^{n}, ∥ x ∥ \geq 0

)

- Separation (

\forall x \in R^{n}, ∥ x ∥ = 0 ⟺ x = 0

)

- Homogeneity (

\forall x \in R^{n}, \forall λ \in R, ∥ λ x ∥ = | λ | ∥ x ∥

)

- Subadditivity (

\forall x, y \in R^{n}, ∥ x + y ∥ \leq ∥ x ∥ + ∥ y ∥

)

To a scalar product is also associated a way to measure angles :

\forall h, k \in R^{n}, 〈 h, k 〉 = ∥ h ∥ ∥ k ∥ c o s (θ)

(3)

The linear transformations f of

R^{n}

respecting the scalar product

〈 ., . 〉

in other words such that

\forall h, k \in R^{n}, 〈 f (h), f (k) 〉 = 〈 h, k 〉

(4)

are called (vectorial) isometries.The set of vectorial isometries is a sub-group of the linear group

(G L (n), \circ)

called the orthogonal groupand denoted

O (n)

When the vectorial space

R^{n}

is equipped with a Euclidean structure, the affine space

R^{n}

is endowed with a distancedefined by

\forall x, y \in R^{n}, d (x, y) = ∥ x - y ∥ = 〈 x - y, x - y 〉 .

(5)

In other words we have

- Symmetry (

\forall x, y \in R^{n}, d (x, y) = d (y, x)

- Separation (

\forall x, y \in R^{n}, d (x, y) = 0 ⟺ x = y

- Triangle inequality (

\forall x, y, z \in R^{n}, d (x, z) \leq d (x, y) + d (y, z)

Furthermore this distance will be compatible with the affine structure :

- Invariance by translation :

\forall x, y \in R^{n}, \forall t \in R^{n}, d (x + t, y + t) = d (x, y)

- Homogeneity :

\forall λ \in R, \forall x \in R^{n}, \forall t, u \in R^{n}, d (x + λ t, x + λ u) = | λ | d (x + t, x + u)

The affine transformations with linear part in

O (n)

are the (affine) isometries.

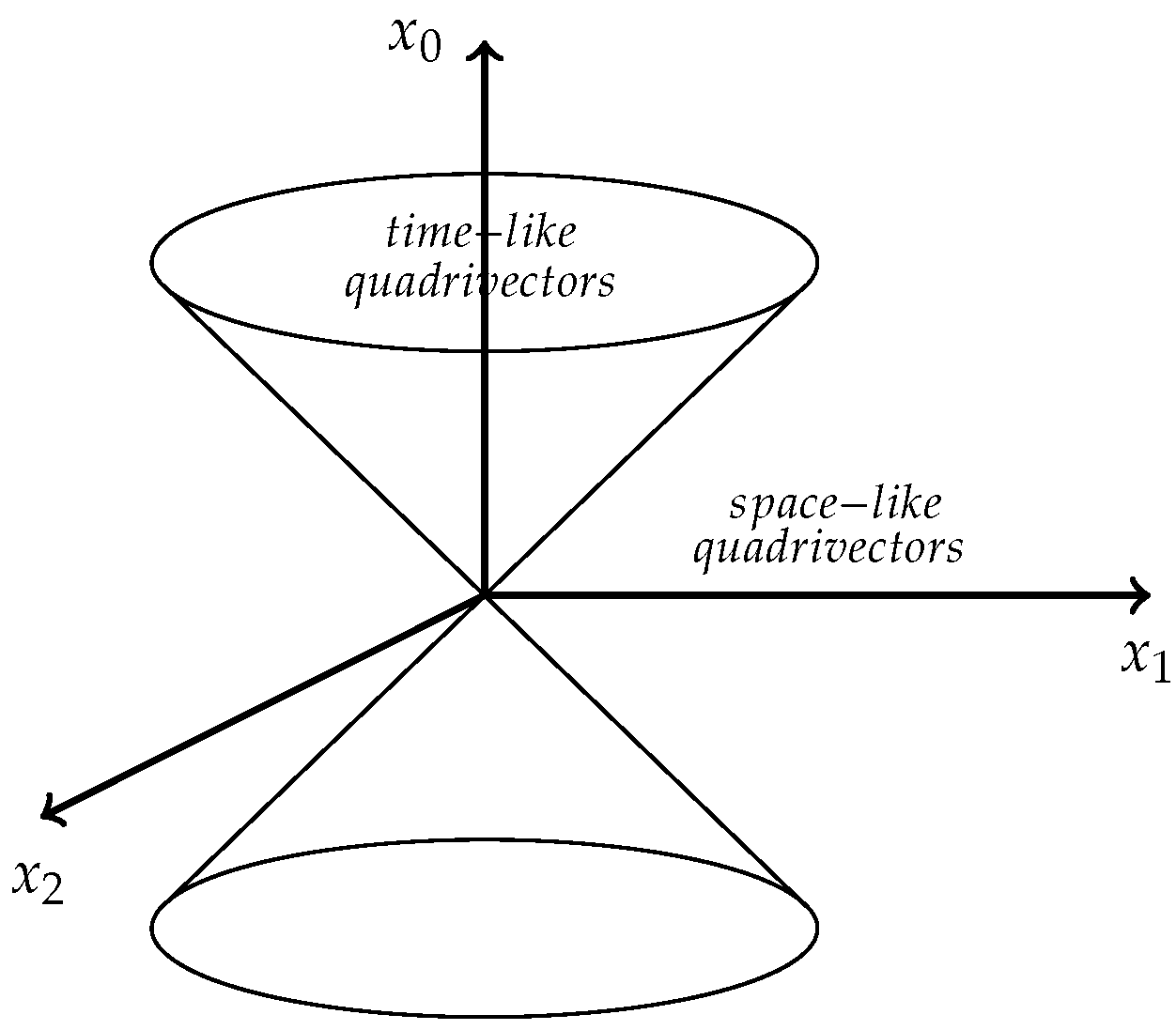
2.1.3. Minkowski Structure

A structure of Minkowski space on

R^{4}

is the choice of some symmetric bilinear form,

., .

R^{4}

with signature

(1, 3)

, that means that in some base

B = (e_{0}, e_{1}, e_{2}, e_{3})

R^{4}

the matrix associated to

., .

J = d i a g (1, - 1, - 1, - 1)

. In other words, if

h = h^{i} e_{i}

and

k = k^{i} e_{i}

we have then

h, k = h^{0} k^{0} - \sum_{i = 1}^{3} h^{i} k^{i} .

(6)

It is common to refer to vectors of a Minkowski space as "quadrivectors" and to

., .

as a Minkowki product.

According to the sign of

h, h

, a quadrivector

h

time-likewhen

h, h > 0

light-likewhen

h, h = 0

, the isotropy cone of

, .,

space-likewhen

h, h < 0

Figure 1. Minkowski space.

The linear transformations f of

R^{4}

respecting the Minkowski product M in other words such that

\forall h, k \in R^{4}, f (h), f (k) = h, k

(7)

are called Lorentz transformations.The Lorentz transformation is a sub-group of

(G L (4), \circ)

called the Lorentz groupand denoted

L

, it is straightforward to see that Lorentz transformation f respect quadrivector’s types.

When the space

R^{4}

is equipped with a Minkowski structure the points of the affine space

R^{4}

are usually called events.

Two events

e = (ε_{0}, ε_{1}, ε_{2}, ε_{3})

and

e^{'} = (ε_{0}^{'}, ε_{1}^{'}, ε_{2}^{'}, ε_{3}^{'})

are time-orientedwhen the unique quadrivector

u

such that

E^{'} = E + u

is time-like.

2.2. Topological Structure of $R^{n}$

A topologyon a set E is a way to give a meaning to expressions like "x and y are close" without having a way to measure a distance between x and y.

A standard way to do so is to select for each point x of E a set of parts of E,

N e i g h (x)

, called the set of the neighborhoodsof x. The sets

N e i g h (x)

fullfilling the conditions expected for such a notion :

† The set E must contain whatever is "close" to x :

\forall x \in E, E \in N e i g h (x)

† The point x is among what is close to x :

\forall x \in E, \forall V \in N e i g h (x), x \in V

† If two sets contain whatever is close to x their intersection too :

\forall x \in E, \forall V, W \in N e i g h (x), V \cap W \in N e i g h (x)

† If V contains whatever is close to x and W contains V, W contains whatever is close to x :

\forall x \in E, \forall V \in N e i g h (x),

V \subset W

then

W \in N e i g h (x)

† If V contains whatever is close to x then there exists W, containing also whatever is close to x, and such that V contain whatever is close to whatever points in W :

\forall x \in E, \forall V \in N e i g h (x), \exists W \in N e i g h (x) / \forall y \in W, V \in N e i g h (y)

Once a topology on E has been chosen an open setof E is a part O of E such that

\forall x \in O, O \in N e i g h (x)

Different topologies can be defined on

R^{n}

, the usual one is defined using the distance d defined in

(5)

. The set

B (x, r [= {y \in R^{n} / d (x, y) < r}

is the open ballwith center at

x \in R^{n}

and radius r. A neighborhood of x is any subset of

R^{n}

containing an open ball centered at x. We will also use this topology on

R^{4}

when equipped with its Minkowski affine structure even though, in that case, there is no distance directly linked to the topologic stucture.

The notion of topology allows correct definition of some very usefull "local" notions in particular the notion of continuity at a point for a function

f : E \to F

between two topological spaces and

x \in E

, f is continuous at xwhen

\forall W \in N e i g h (f (x)), \exists V \in N e i g h (x) / f (V) \subset W .

The affine orthogonal transformations (and the affine Lorentz transformations) are continuous on

R^{n}

(and on

R^{4}

)

In the Minkowsi space the set of light-like quadrivectors has two path connected components2. A Lorentz transformation is orthochronewhen the path connected components are respected, and antichronewhen the components are exchanged.

2.3. Differentiable Structure of $R^{n}$

R^{n}

being equipped with a scalar product and the associated norm defined in

(2)

and

R^{n}

with the distance defined in

(5)

2.3.1. Differential of a Function, Tangent Vectors at a Point

An application

f : R^{n} \to R^{p}

is differentiable at

x \in R^{n}

whenever

\forall h \in R^{n}, f (x + h) = f (x) + d f /_{x} (h) + o (h)

(8)

where

d f /_{x}

is continuous and linear from

R^{n}

R^{p}

3and

o (h) = ∥ h ∥ ε (h)

with

ε : R^{n} \to R^{p}

such that

ε (0) = 0

and

l i m_{h \to 0} ε (h) = 0

The linear application

d f /_{x} : R^{n} \to : R^{p}

is the differentialat x of f.

The application

d f : R^{n} \to L (R^{n}, R^{p})

is the differential of f.

At first glance, the definition of the differentiability of f at a point x, suggests that the vectors

h

are picked in the "same" space

R^{n}

independantly of the point x we are looking at, but this point of view would be barren if we want to go further.

To each point

x \in R^{n}

is associated a copy of

R^{n}

, called the tangent space to

R^{n}

at xand denoted

T_{x} R^{n}

, its elements are called tangent vectors to

R^{n}

at x.

With those definitions in mind, the definition of differentiability becomes

\forall h \in T_{x} R^{n}, f (x + h) = f (x) + d f /_{x} (h) + o (h)

(9)

where

d f /_{x} : T_{x} R^{n} \to T_{f (x)} R^{p}

is linear and

o (h) = ∥ h ∥ ε (h)

with

ε : T_{x} R^{n} \to T_{f (x)} R^{p}

satisfying

ε (0) = 0

and

l i m_{h \to 0} ε (h) = 0

We have to clarify the status of the differential application

d f : x \mapsto d f /_{x}

, for its arrival set had became unclear.

Set

T R^{n} = ⨆_{x \in R^{n}} {x} \times T_{x} R^{n} .

and

p : T R^{n} \to R^{n}; (x, h) \mapsto x

The set

T R^{n}

is the tangent bundle of

R^{n}

, it identifies to

R^{n} \times R^{n}

and then the projection p becomes the first projection of

R^{n} \times R^{n}

R^{n}

. The tangent bundle

T R^{n}

identifies also with the affine space

R^{2 n}

Now let

f : R^{n} \to R^{p}

be an application differentiable at each point of

R^{n}

, the application

T f : T R^{n} \to T R^{p}; (x, h) \mapsto (f (x), d f /_{x} (h))

is called the tangent applicationor differential applicationof f.

Furthermore the projection

p : T R^{n} \to R^{n}; (x, h) \mapsto x

is a (trivial) "fiber bundle", in that very simple case it just means that p is differentiable on

T R^{n}

The bitangent space to

R^{n}

(x, h)

T_{(x, h)} (T R^{n})

identifies with the product vector space

T_{x} R^{n} \times T_{h} R^{n}

then

p ((x, h) + (H, K)) = x + H = p (x, H) + H

and

d p_{(x, h)} (H, K) = H

The process of differentiation can be repeted indefinitelly, applications admitting differential at all orders are said of class

C^{\infty}

, the set of functions from

R^{n}

R

of class

C^{\infty}

is denoted

C^{\infty} (R^{n})

2.3.2. Tangent Vector Fields on $R^{n}$

A vector field

U

R^{n}

is a section of the tangent fiber bundle i.e. an application

U : R^{n} \to T R^{n} such that \forall x \in R^{n}, U (x) = (x, U (x)) \in T_{x} R^{n} .

As the first factor of a tangent vector fields is always known, in the sequels, we will note the tangent vector field

U

by its second factor

U

Let

(e_{1}, \dots, e_{n})

be the canonical base of

R^{n}

the tangent vector field

x \mapsto (x, e_{i})

will be denoted

\frac{\partial}{\partial x^{i}}

so that any tangent vector field on

R^{n}

has a unique expression

U = U^{i} \frac{\partial}{\partial x^{i}}

with

U^{1}, \dots, U^{n}

some functions from

R^{n}

R

The set of tangent vector fields of class

C^{\infty}

Γ (R^{n}) = {U^{i} \frac{\partial}{\partial x^{i}} / \forall i \in {1, \dots, n}, U^{i} \in C^{\infty} (R^{n})}

We clarify now the notation

\frac{\partial}{\partial x^{i}}

The set

C^{\infty} (R^{n})

, is endowed with a structure of real algebra by

- Addition defined by

\forall f, g \in C^{\infty} (R^{n}), \forall x \in R^{n}, (f + g) (x) = f (x) + g (x)

- Multiplication by a real scalar defined by

\forall (f, λ) \in C^{\infty} (R^{n}) \times R, \forall x \in R^{n}, (λ f) (x) = λ f (x)

- Inner multiplication defined by

\forall f, g \in C^{\infty} (M), \forall x \in M, (f . g) (x) = f (x) g (x)

The set

Γ (R^{n})

is endowed with a structure of

C^{\infty} (R^{n})

-modulus by

- Vector fields addition defined by

\forall U, V \in Γ (R^{n}), \forall x \in R^{n}, (U + V) (x) = U (x) + V (x)

- The multiplication by a real scalar defined by

\forall U \in Γ (R^{n}), \forall λ \in R, \forall x \in R^{n}, (λ U) (x) = λ U (x)

- Multiplication by a function defined by

\forall (f, U) \in C^{\infty} (R^{n}) \times Γ (R^{n}), \forall x \in R^{n}, (f U) (x) = f (x) U (x)

A derivation on the real algebra

C^{\infty} (R^{n})

is a linear application

D : C^{\infty} (R^{n}) \to C^{\infty} (R^{n})

satisfying

\forall f, g \in C^{\infty} (R^{n}), D (f g) = D (f) g + f D (g)

U \in Γ (R^{n})

correspond a derivation

D_{U}

C^{\infty} (R^{n})

where

\forall f \in C^{\infty} (R^{n}),

D_{U} (f) : R^{n} \to R; x \mapsto d f /_{x} (U (x))

Indeed we have

\forall f, g \in C^{\infty} (R^{n}), D_{U} (f g) = D_{U} (f) . g + f D_{U} (g)

(10)

and

\forall a, b \in R, \forall f, g \in C^{\infty} (R^{n}), D_{U} (a f + b g) = a D_{U} (f) + b D_{U} (g)

(11)

D_{U} (f) (x)

can be seen as the directional derivative of f in the direction of

U

at x.

The notations

\frac{\partial}{\partial x^{i}}

introduced previously for a tangent vector field are now clear :

If the expression of a tangent vector field is

U = U^{i} (x) \frac{\partial}{\partial x^{i}}

and

f \in C^{\infty} (R^{n})

for

x \in R^{n}

D_{U} (f) (x) = U^{i} (x) \frac{\partial f}{\partial x^{i}} (x)

(12)

The Lie bracket of two tangent vector fields

U = U^{i} \frac{\partial}{\partial x^{i}}

and

V = V^{i} \frac{\partial}{\partial x^{i}}

is defined by

[U, V] = (U^{j} \frac{\partial V^{i}}{\partial x^{j}} - V^{j} \frac{\partial U^{i}}{\partial x^{j}}) \frac{\partial}{\partial x^{i}}

(13)

we have

D_{[U, V]} = D_{U} \circ D_{V} - D_{V} \circ D_{U}

(14)

Endowed with this bracket the vector space

Γ (R^{n})

is a Lie algebra.

2.3.3. Covariant Derivation

A covariant derivationon

R^{n}

is an application

\nabla : Γ (R^{n}) \times Γ (R^{n}) \to Γ (R^{n}); (U, V) \mapsto \nabla_{U} V

satisfying

\forall a, b \in R, \forall U, V, W \in Γ (R^{n}), \forall f \in C^{\infty} (R^{n}),

\nabla_{a U + b V} W = a \nabla_{U} W + b \nabla_{V} W

(15)

\nabla_{U} (a V + b W) = a \nabla_{U} V + b \nabla_{U} W

(16)

\nabla_{f U} V = f \nabla_{U} V and \nabla_{U} (f V) = D_{U} (f) V + f \nabla_{U} V .

(17)

A covariant derivation, ∇, on

R^{n}

is entirely defined by the family of tangent vector fields

{(\nabla_{\frac{\partial}{\partial x^{i}}} \frac{\partial}{\partial x^{j}})}_{i, j = 1 \dots n} .

\nabla_{\frac{\partial}{\partial x^{i}}} \frac{\partial}{\partial x^{j}} = Γ_{i, j}^{k} \frac{\partial}{\partial x^{k}}

(18)

the functions

Γ_{i j}^{k}

are called Christoffelsymbols of ∇.

For

U = U^{i} \frac{\partial}{\partial x^{i}}

and

V = V^{i} \frac{\partial}{\partial x^{i}}

we have

\nabla_{U} V = U^{i} \frac{\partial V^{j}}{\partial x^{i}} \frac{\partial}{\partial x^{j}} + U^{i} V^{j} Γ_{i j}^{k} \frac{\partial}{\partial x^{k}}

(19)

2.3.4. Flat Covariant Derivation

The flat covariant derivation ∇ is the covariant derivation whose Christoffel symbols are all null, in that case we have

\nabla_{U} V = \sum_{i, j = 1}^{n} U^{i} \frac{\partial V^{j}}{\partial x^{i}} \frac{\partial}{\partial x^{j}}

\nabla_{U} V

can be seen as the derivative of

V

in the direction of

U

2.3.5. Covariant Derivation Along a Curve, Parallel Transport

Let

γ : R \to R^{n}; t \mapsto γ (t) = (γ_{1} (t), \dots, γ_{n} (t))

be a class

C^{\infty}

function.

A tangent vector field of

R^{n}

along

γ

is an application

U : R \to T R^{n}

such that

\forall t \in R, U (t) = (γ (t), U (t)) \in T_{γ (t)} R^{n} .

The set of tangent vector fields of class

C^{\infty}

along

γ

is denoted

Γ (γ)

, it is a

C^{\infty} (R)

-modulus.

The velocity

\dot{γ}

is the tangent vector field along

γ

defined by

t \mapsto \dot{γ} (t) = (γ (t), {\dot{γ}}^{i} (t) \frac{\partial}{\partial x^{i}})

(20)

Where

{\dot{γ}}^{i} = \frac{d γ^{i}}{d t}

. Its value depends only on the differential structure of

R^{n}

and

\forall t \in R, \forall h \in R, d γ /_{t} (h) = h . \dot{γ} (t)

Let ∇ be a covariant derivation on

R^{n}

. There is exactly one operator

\nabla_{\frac{d}{d t}}

4 on the

C^{\infty} (R)

-modulus of tangent vector fields along

γ

such that

\forall f \in C^{\infty} (R), \forall U \in Γ (γ), \nabla_{\frac{d}{d t}} (f . U) (t) = f^{'} (t) . U (t) + f (t) . \nabla_{\frac{d}{d t}} U (t)

and if

U

is the restriction to

γ

of a tangent vector field

V

R^{n}

\nabla_{\frac{d}{d t}} U (t) = \nabla_{\dot{γ} (t)} V (t)

For

U (t) = U^{i} (t) \frac{\partial}{\partial x^{i}}

we have

\nabla_{\frac{d}{d t}} U (t) = ({\dot{U}}^{k} (t) + Γ_{i j}^{k} U^{i} (t) U^{j} (t)) \frac{\partial}{\partial x^{k}}

(21)

When

R^{n}

is equipped with the flat covariant derivative 5, the covariant derivative of

U

with

U (t) = (γ (t), U^{i} (t) \frac{\partial}{\partial x^{i}})

along

γ

is the tangent vector field along

γ

defined by

\nabla_{\frac{d}{d t}} U (t) = (γ (t), {\dot{U}}^{i} (t) \frac{\partial}{\partial x^{i}})

(22)

A tangent vector field

U

along

γ

is said parallelwith respect to the covariant derivation ∇ whenever

\nabla_{\frac{d}{d t}} U = 0

. General results on differential equations insure that a parallel tangent vector field along

γ

is determined by its value at one point of the trajectory of

γ

. For

t_{0}, t_{1} \in R

the application

/ /_{t_{0}}^{t_{1}} : T_{γ (t_{0})} R^{n} \to T_{γ (t_{1})} R^{n}; (γ (t_{0}), U (γ (t_{0})) \mapsto (γ (t_{1}), U (γ (t_{1}))

where

U

is parallel along

γ

is called the parallel transport along

γ

between time

t_{0}

and

t_{1}

When ∇ is the flat covariant derivation for any class

C^{\infty}

curve

γ

the parallel vector fields along

γ

are simply the constant vector fields.

2.3.6. Acceleration

Let

γ : R \to R^{n}

be a class

C^{\infty}

function.

The covariant derivative of the vector field

\dot{γ}

along

γ

is the covariant accelerationof

γ

it is denoted

\ddot{γ}

, note that unlike the velocity,

\ddot{γ}

depends on the choice of a covariant derivation on

R^{n}

When a curve

γ

satisfies

\nabla_{\frac{d}{d t}} \dot{γ} = 0

it is called a geodesiccurve of the covariant derivation ∇, for the flat covariant derivation the geodesics are the parametrizations with constant velocity of straight lines.

2.4. Riemannian Structures on $R^{n}$

A Riemannian metricon

R^{n}

is an application G associating to each point

x \in R^{n}

a scalar product

G_{x}

T_{x} R^{n}

with the condition that

\forall U, V \in Γ (R^{n}), the application f : R^{n} \to R defined by f (x) = G_{x} (U (x), V (x)) is in C^{\infty} (R^{n}) .

The general expression of a Riemannian metric on

R^{n}

G_{x} = g_{i j} (x) d x^{i} d x^{j}

(23)

Where the coefficients

g_{i j} (x) \in C^{\infty} (R^{n})

are such that

\forall x \in R^{n},

the matrix

{(g_{i, j} (x))}_{1 \leq i, j \leq n}

is symmetric definite positive and

d x^{i}

is defined on

Γ (R^{n})

d x^{i} (U^{j} \frac{\partial}{\partial x^{j}}) = U^{i} .

Let G be a Riemannian metric G and

γ : I \to R^{n}

be a class

C^{\infty}

application defined on some interval of

R

, the real

∥ \dot{γ} {(t) ∥}_{G} = \sqrt{G_{γ (t)} (\dot{γ} (t), \dot{γ} (t))}

(24)

is the speedof

γ

at instant t with respect to the Riemannian metric G or G-speed,the application

t \mapsto (γ (t), ∥ \dot{γ} (t) ∥_{G})

is a scalar field along

γ

The G-lengthof

γ

L_{G} (γ) = \int_{a}^{b} {∥ \dot{γ} (t) ∥}_{G} d t .

(25)

The G-kinetic energyof

γ

E_{G} (γ) = \frac{1}{2} \int_{a}^{b} {∥ \dot{γ} (t) ∥}_{G}^{2} d t .

(26)

The G-length does not depend on the parametrization

γ

but the G-kinetic energy does.

We obtain a distance on

R^{n}

setting for

x, y \in R^{n}

d_{G} (x, y) = I n f {L_{G} (γ), γ differentiable with γ (a) = x, γ (b) = y} .

Though

d_{G}

is in general not associated to any norm on

R^{n}

, the topology of

R^{n}

induced by

d_{G}

is always the usual topology of

R^{n}

For

u, v \in T_{x} R^{n}

the G-angle,

θ_{G} (u, v))

, of

u

and

v

is defined by

G (u, v) = {∥ u ∥}_{G} {∥ v ∥}_{G} c o s (θ_{G} (u, v)) .

(27)

2.4.1. Levi-Civita Connection

Let G be a Riemannian metric on

R^{n}

, a covariant derivation ∇ on

R^{n}

is compatible with G when

\forall U, V, W \in Γ (R^{n}), D_{U} (G (V, W)) = G (\nabla_{U} V, W) + G (V, \nabla_{U} W)

(28)

For any Riemannian metric G on

R^{n}

there is exactly one torsion-free 6 covariant derivation compatible with G called the Levi-Civita connectionof G.

The Christoffel symbols of the Levi-Civita connection have expressions

Γ_{i j}^{k} = \frac{1}{2} g^{k ℓ} (\frac{\partial g_{i ℓ}}{\partial x^{j}} + \frac{\partial g_{j ℓ}}{\partial x^{i}} - \frac{\partial g_{i j}}{\partial x^{ℓ}})

(29)

where

{(g^{i j})}_{1 \leq i, j \leq n}

is the inverse matrix of

{(g_{i j})}_{1 \leq i, j \leq n}

2.4.2. Some Riemannian Metrics

1) The usual affine Euclidean structure of

R^{n}

can be seen as a Riemannian structure on

R^{n}

G^{E u c} = δ_{i j} d x^{i} d x^{j}

(30)

When

R^{n}

is equipped with the metric

G^{E u c}

the Levi-Civita connection is the flat covariant derivation ∇ defined previously. The distance

d_{G^{E u c}}

is the usual Euclidean distance and the angle measurement is the usual angle measurement. The geodesics are parametrizations of straightlines with constant velocities.

2) For

λ \in R_{+}^{*}

we put

G_{λ}^{E u c} = λ^{2} G^{E u c} = λ^{2} δ_{i j} d x^{i} d x^{j}

(31)

The Levi-Civita connection of

G_{λ}^{E u c}

is also the flat covariant derivation.

The distance associated is

d_{G_{λ}^{E u c}} = λ d_{G^{E u c}} .

(32)

The angles measurement associated satisfies

\forall x \in R^{n}, \forall u, v \in T_{x} R^{n}, θ_{G_{λ}^{E u c}} (u, v) = θ_{G^{E u c}} (u, v) .

(33)

The application

I d_{R^{n}}

from

(R^{n}, G^{E u c})

(R^{n}, G_{λ}^{E u c})

is a scaling.

The geodesics are straightlines parametrized with constant velocities.

For

G^{E u c}

as well as for

G_{λ}^{E u c}

the parallel transport is trivial, the parallel tangent vector fields are of the form

U = \sum_{i = 1}^{n} u^{i} \frac{\partial}{\partial x_{i}}

where

u^{i}

are constants.

3) For

μ \in C^{\infty} (R^{n})

such that

\forall x \in R^{n}, μ (x) \in] m, M [, (m > 0)

we put

G_{μ} = μ^{2} δ_{i j} d x^{i} d x^{j}

(34)

The Levi-Civita connection is not anymore the flat covariant derivation, a straightforward computation gives the expressions of the Christoffel symbols

Γ_{i j}^{k} = \frac{1}{μ} δ^{k l} (δ_{i l} \frac{\partial μ}{\partial x^{j}} + δ_{l j} \frac{\partial μ}{\partial x^{i}} - δ_{i j} \frac{\partial μ}{\partial x^{m}})

(35)

The geodesics are not anymore straightlines. The application

I d_{R^{n}}

from

(R^{n}, G^{E u c})

(R^{n}, G_{μ})

is conformal.

2.5. Pseudo-Riemannian Structure on $R^{4}$

A pseudo-Riemannian metricon

R^{4}

is an application G associating to each point

x \in R^{4}

a Minkowski product

G_{x}

T_{x} R^{4}

with the condition that

\forall U, V \in Γ (R^{4}), the application F : R^{4} \to R defined by G_{x} (U (x), V (x)) is in C^{\infty} (R^{4}) .

The general expression of a pseudo-Riemannian metric on

R^{4}

G_{x} = g_{i j} (x) d x^{i} d x^{j}

(36)

where the coefficients

g_{i j} \in C^{\infty} (R^{n})

are such that

\forall x \in R^{4},

the matrix

(g_{i j} (x))

is symmetric with signature

(1, 3)

Let

η_{i j}

be the coefficient of the matrix

d i a g (1, - 1, - 1, - 1)

. The usual affine Minkowski structure of

R^{4}

is a pseudo-Riemannian structure :

G^{M i n k} = η_{i j} d x^{i} . d x^{j}

(37)

It will be practical to limit the summation to

i \in {1, 2, 3}

. With this convention we have

G^{M i n k} = d x^{0} d x^{0} - δ_{i j} d x^{i} . d x^{j}

(38)

As in the Riemannian case, for any pseudo-Riemannian structure on

R^{4}

the Levi-Civita connection is the unique torsion-free covariant derivation satisfying

\forall U, V, W \in Γ (R^{4}), D_{U} (G (V, W)) = G (\nabla_{U} V, W) + G (V, \nabla_{U} W)

(39)

The Levi-Civita connection of

G^{M i n k}

is once again the flat covariant derivation.

For

λ > 0

we will consider later the pseudo-Riemannian metric defined by

G_{λ}^{M i n k} = d x^{0} d x^{0} - λ^{2} δ_{i j} d x^{i} d x^{j}

(40)

For this pseudo-Riemannian metric the Levi-Civita connection is still the flat covariant derivation.

3. Framework for the $κ$ -Model

3.1. $κ$ -Structure on a Riemannian Metric

A κ-structureon

R^{3}

or a Riemannian metric affected with a κ-effectis a couple

(G, κ)

where

† G = g_{i j} d x^{i} d x^{j}

is a fixed Riemannian metric on

R^{3}

† κ

is an application of class

C^{\infty}

from

R^{3}

] m, M [

with

m > 0

Then, when

(G, κ)

is a

κ

-structure on

R^{3}

we have :

1) On one hand two Riemannian metrics on

R^{3}

(R^{3}, G) and (R^{3}, G_{κ})

where

G_{κ} = κ^{2} g_{i j} d x^{i} d x^{j}

G_{κ}

is a metric conformal to G.

2) On the other hand to each point

a \in R^{3}

is associated the Riemannian metric

(R^{3}, G_{κ (a)})

which is a rescaling of G.

A point in

R^{3}

endowed with a

κ

-structure will be called a sitting-observer.

We can think of this situation as the trivial bundle

R^{3} \times] m, M [\to R^{3}

where each constant section

R^{3} \times {λ}

is equipped with the Riemannian metric

G_{λ}

, the function

κ

associating to each point a of

R^{3}

the constant section

R^{3} \times {κ (a)}

3.2. $κ$ -Structure on the Euclidean Metric

Let us equip

R^{3}

with a

κ

-structure

(G^{E u c}, κ)

, and choose once and for all a global system of coordinates

(σ^{1}, σ^{2}, σ^{3})

such that

G^{E u c} = δ_{i j} d σ^{i} d σ^{j}

. The distance associated is the Euclidean distance,

d_{E u c}

Each sitting-observer

a \in R^{3}

is equipped via

κ

with the flat Riemannian metric

G_{κ (a)}^{E u c}

defined by

\forall a \in R^{3}, \forall σ \in R^{3}, \forall u, v \in T_{σ} R^{3}, G_{κ (a)} (u, v) = κ^{2} (a) 〈 u, v 〉

(41)

from which a distance on

R^{3}

is deduced :

d_{a} (x, y) = κ (a) d_{e} (x, y) .

(42)

However there should be no confusion between the collection of flat Riemannian metrics

G_{κ (a)}^{E u c} = κ^{2} (a) \sum {(d σ^{i})}^{2}

which are rescalings of

(R^{3}, G^{E u c})

and the non-flat Riemannian metric

G_{κ} = κ^{2} \sum {(d σ^{i})}^{2}

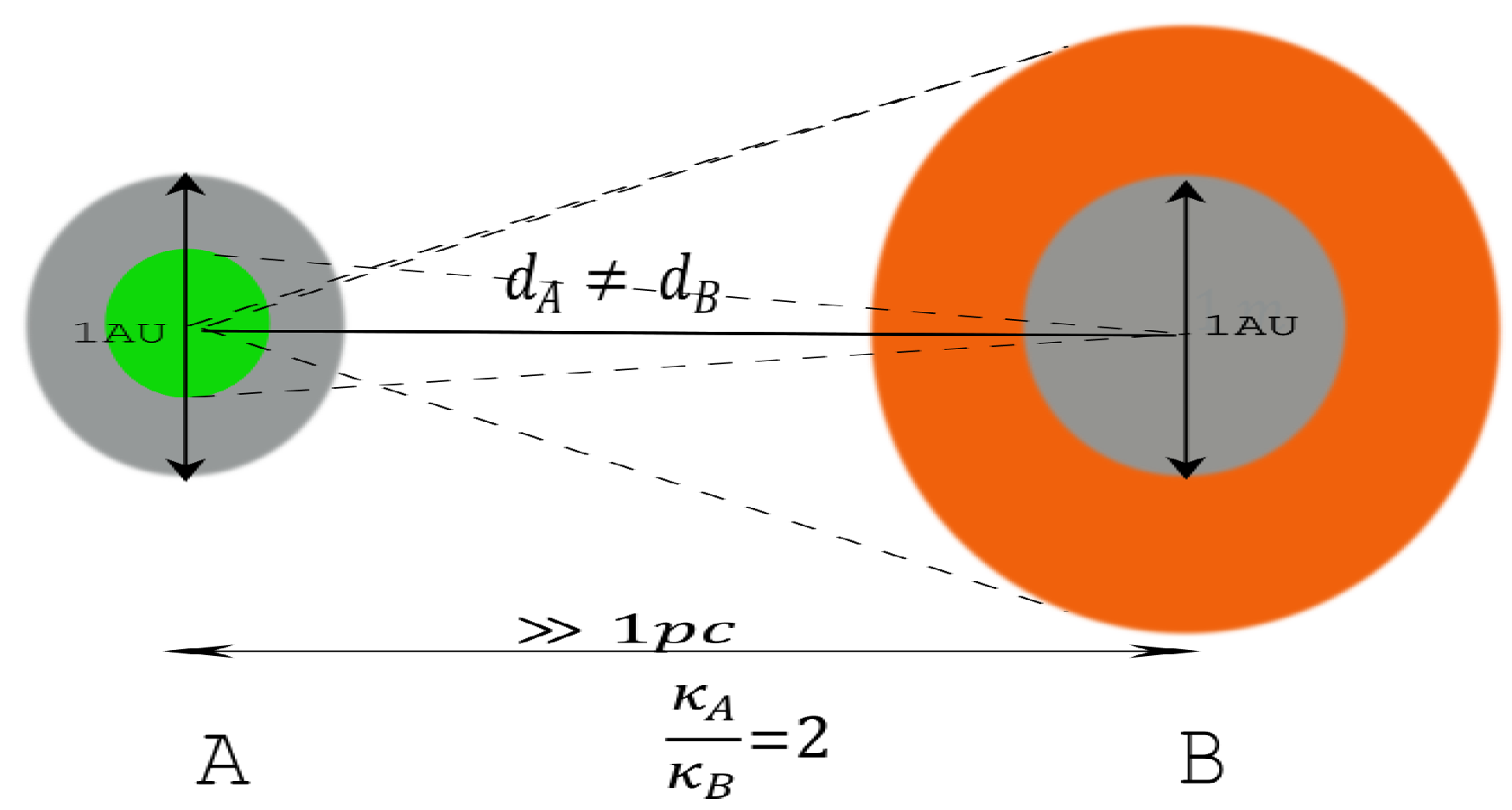
Figure 2. Circles with same radii and centers in

R_{μ}^{3}, R_{ν}^{3}

and

R_{λ}^{3}

with

λ > μ > ν

Figure 2. Circles with same radii and centers in

R_{μ}^{3}, R_{ν}^{3}

and

R_{λ}^{3}

with

λ > μ > ν

Any information retrieved using the flat Riemannian metric

G_{κ (a)}^{E u c}

R^{3}

will be called an observation made by the sitting-observera.

The application

I d : (R^{3}, d_{a}) \to (R^{3}, d_{b})

is not an isometry but it is nevertheless a scaling.Observations made by two sitting observers are linked, for example

\forall a, b \in R^{3}, \forall σ, σ^{'} \in R^{3}, d_{a} (σ, σ^{'}) = \frac{κ (a)}{κ (b)} d_{b} (σ, σ^{'}) .

(43)

while

\forall a, b \in R^{3}, \forall σ \in R^{3}, \forall u, v \in T_{σ} R^{3}, θ_{a} (u, v) = θ_{b} (u, v) .

(44)

This means that two sitting-observers will agree on angles measurements but not on length measurements. If they exchange their measurements two sitting-observers would desagree.

3.2.1. Speed Fields

Let

(G^{E u c}, κ)

be the euclidean metric on

R^{3}

affected with a

κ

-effect, let

γ : R \to R^{3}

be a smooth curve and

t \mapsto \dot{γ} (t)

be its velocity.

† The Euclidean-speedof

γ

is the scalar field along

γ

defined by

v_{E u c} (t) = {∥ \dot{γ} (t) ∥}_{G^{E u c}} .

(45)

† The a-speedof

γ

is the speed observed by a sitting-observer at a, it is the scalar field

along

γ

defined by

v_{a} (t) = {∥ \dot{γ} (t) ∥}_{G_{κ (a)}^{E u c}} = \sqrt{κ^{2} (a) 〈 \dot{γ} (t), \dot{γ} (t) 〉} = κ (a) v_{E u c} (t)

(46)

The a-velocityof

γ

is the tangent vector field along

γ

defined by

v_{a} (t) = κ (a) \dot{γ} (t)

(47)

so that the a-speed at time t is

∥ v_{a} {(t) ∥}_{G^{E u c}}

† The κ-speedof

γ

at time t is the speed observed by the sitting-observers coincident with

γ (t)

, it is the scalar field defined by

v_{κ} (t) = {∥ \dot{γ} (t) ∥}_{G_{κ (γ (t))}^{E u c}} = \sqrt{κ^{2} (γ (t)) 〈 \dot{γ} (t), \dot{γ} (t) 〉} = κ (γ (t)) v_{E u c} (t)

(48)

The

κ

-velocity of

γ

is the tangent vector field along

γ

defined by

v_{κ} (t) = κ (γ (t)) \dot{γ} (t) .

(49)

so that the

κ

-speed at time t is

∥ {\dot{v}}_{κ} {(t) ∥}_{G^{E u c}}

The relations between the different speeds is

\forall a, b \in R^{3}, v_{a} (t) = \frac{κ (a)}{κ (b)} v_{b} (t)

(50)

and

\forall a \in R^{3}, v_{κ} (t) = \frac{κ (γ (t))}{κ (a)} v_{a} (t)

(51)

A smooth curve

γ : R \to R^{3}

is κ-uniformwhen its

κ

-speed is constant, in other words when

d κ /_{γ (t)} (\dot{γ} (t)) ∥ \dot{γ} (t) ∥ + κ (γ (t)) \frac{< \ddot{γ} (t), \dot{γ} (t) >}{∥ \dot{γ} (t) ∥} = 0

(52)

〈 {G r a d}_{γ (t)} κ, \dot{γ} (t) 〉 v_{E u c} (t) + κ (γ (t)) \frac{< \ddot{γ} (t), \dot{γ} (t) >}{v_{E u c} (t)} = 0

(53)

3.2.2. $κ$ -Uniform Straight Lines

Let

σ

and

σ^{'}

be two distinct points of

R^{3}

. For any sitting-observer a the parametrization

γ : R \to R^{3}, t \mapsto σ + t (σ^{'} - σ)

(54)

is the geodesic passing through

σ

at time 0 and

σ^{'}

at time 1 with constant a-speed

v_{a} = d_{a} (σ, σ^{'})

Since

\forall t \in R, v_{κ} (t) = {∥ σ^{'} - σ ∥}_{κ (γ (t))}

is not a constant function, the smooth curve

γ

is not

κ

-uniform (unless

κ

is constant along it).

Nevertheless we can reparametrize the support of

γ

in a

κ

-uniform way.

Consider

c : R \to R^{3}

defined by

c (t) = σ + ϕ (t) u

(55)

where

† ϕ : R \to R

is some class

C^{\infty}

function with

ϕ (0) = 0 .

† u = \frac{1}{∥ σ^{'} - σ ∥} (σ^{'} - σ) \in R^{3}

The

κ

-speed of c is

v_{κ} (t) = κ (σ + ϕ (t) u) | ϕ^{'} (t) |

The parametrization c is

κ

-uniform with constant

κ

-speed V if and only if

\forall t \in R, | ϕ^{'} (t) | = \frac{V}{κ (σ + ϕ (t) u)}

(56)

Then

\forall t \in R, \frac{V}{M} \leq | ϕ^{'} (t) | \leq \frac{V}{m}

so the derivative

ϕ^{'} (t)

never cancels. If c is to reach

σ^{'}

at some positive time we have

\forall t \in R, ϕ^{'} (t) > 0

Let

K : R \to R

be the function defined by

K (θ) = \int_{0}^{θ} κ (σ + s u) d s

(57)

κ

is a strictly positive function, K is a strictly increasing bijective function from

R

to itself, let

χ

be its reciprocal.

The curve defined by

c (t) = σ + χ (t V) u

κ

-uniform with

κ

-speed V.

Indeed its

κ

-speed

v_{κ} (t) = κ (σ + χ (t V) u) χ^{'} (t V) = κ (σ + χ (t V) u) \frac{1}{K^{'} (χ (t V))} V = V

(58)

3.2.3. Velocity Fields and Covariant Accelerations

Let

γ : R \to R^{3}

be a smooth curve.

For a given sitting-observer a,

R^{3}

is equipped with

G_{κ (a)}^{E u c}

, the Levi-Civita connection is flat and the solutions of the equation of cancellation of covariant derivation of the a-velocity

\nabla_{\frac{d}{d t}} {\dot{v}}_{a} = 0

(59)

are the parametrizations with constant a-speed of straight lines, those are the a-geodesics.

Two sitting-observers a and b will agree on saying that a given curve is a geodesic but will observe two different speeds.

A straightforward computation gives the equation of cancellation of the flat covariant derivation of the

κ

-velocity

\nabla_{\frac{d}{d t}} {\dot{v}}_{κ} = κ (γ (t)) \ddot{γ} (t) + 〈 {G r a d}_{γ (t)} κ, \dot{γ} (t) 〉 . \dot{γ} (t) = 0

(60)

The flat covariant derivation is not the Levi-Civita of the metric

G_{κ}^{E u c}

so the equation

(60)

is not the equation of geodesics of

G_{κ}^{E u c}

. The solution curves are the parametrizations of straightlines with constant

κ

-speed.

This can be checked easily :

(60)

admits one unique solution with given initial conditions

(γ (0), \dot{γ} (0)

. Using an adapted frame we can assume that

γ (0) = O

and

\dot{γ} (0)

is colinear to

\frac{\partial}{\partial σ^{1}}

We have seen that the

κ

-uniform parametrization of the straight line

γ

such that

γ (0) = O

and

κ

-speed 1 is

γ (t) = (χ (t), 0, 0)

where

χ

is the reciprocal of the function K defined by

K (θ) = \int_{0}^{θ} κ (O + s \frac{\partial}{\partial x^{1}}) d s .

The velocity of

γ

writes

\dot{γ} (t) = χ^{'} (t) \frac{\partial}{\partial x^{1}} = \frac{1}{κ (γ (t))} \frac{\partial}{\partial x^{1}}

then

\ddot{γ} (t) = \nabla_{\frac{d}{d t}} \dot{γ} (t) = \frac{- \frac{\partial κ}{\partial x^{1}} (γ (t)) χ^{'} (t)}{κ^{2} (γ (t))} \frac{\partial}{\partial x^{1}} = - \frac{1}{κ {(γ (t))}^{3}} \frac{\partial κ}{\partial x^{1}} (γ (t)) \frac{\partial}{\partial x^{1}}

From where we find again relation

(60)

3.2.4. Laser Distance

Let

σ, σ^{'}

be two distinct points of

R^{3}

and

u = \frac{1}{d_{E u c} (σ, σ^{'})} (σ^{'} - σ)

. Consider

γ : R \to R^{3}; t \mapsto σ + t u

(61)

we have

γ (0) = σ, γ (d_{E u c} (σ, σ^{'})) = σ^{'} .

and as

\forall t \in R, \dot{γ} (t)

is the Euclidean-unit tangent vector

u

the

κ

-speed

v_{κ} (t) = κ (γ (t))

The laser distance

d_{l a s} (σ, σ^{'})

is defined by

G_{κ}^{E u c}

-length of

γ

between

σ

and

σ^{'}

d_{l a s} (σ, σ^{'}) = L_{G_{κ}^{E u c}} ([σ, σ^{'}]) = \int_{0}^{d_{E u c} (σ, σ^{'})} κ (σ + t u) d t .

(62)

This laser-distance can also be obtained with the

κ

-uniform parametrization of

[σ, σ^{'}]

with

κ

-speed equal to 1.

\tilde{γ} : R \to R^{3}; t \mapsto x = σ + χ (t) u

where

χ = K^{- 1}

with

K (θ) = \int_{0}^{θ} κ (x + s u) d s

L_{l a s} (σ, σ^{'})

is the "distance" obtained by compiling the observations of the speed made by sitting-observers along the straightline segment

[σ, σ^{'}]

(which is not a

G_{κ}^{E u c}

-geodesic).

In general, the application

(σ, σ^{'}) \mapsto d_{l a s} (σ, σ^{'})

is not a distance for it fails to satisfy the triangle inequality, but nevertheless

The positivity

\forall σ, σ^{'} \in R^{3}, d_{l a s} (σ, σ^{'}) \geq 0 .

The separation

\forall σ, σ^{'} \in R^{3}, d_{l a s} (σ, σ^{'}) = 0 ⟺ σ^{'} = σ

The symmetry

\forall σ, σ^{'} \in R^{3}, d_{l a s} (σ, σ^{'}) = d_{l a s} (σ^{'}, σ)

are satisfied.

3.2.5. Circular Motions

Let

γ : R \to R^{3}

be a parametrization of class

C^{\infty}

of some Euclidean circle

C

R^{3}

, in a well chosen orthonormal coordinates system

(σ^{1}, σ^{2}, σ^{3})

we have

γ (t) = R (cos θ (t), sin θ (t), 0))

(63)

with

R > 0

and

θ

some class

C^{\infty}

function.

For

θ \in R

, let us define the tangent vector fields

u (θ) = c o s (θ) \frac{\partial}{\partial σ^{1}} + s i n (θ) \frac{\partial}{\partial σ^{2}} and v (θ) = - s i n (θ) \frac{\partial}{\partial σ^{1}} + c o s (θ) \frac{\partial}{\partial σ^{2}} .

(64)

For a sitting-observer a, the apparent radius is

κ (a) R

, the a-velocity at time t is

v_{a} (t) = κ (a) R θ^{'} (t) v (θ (t))

(65)

While the

κ

-velocity at time t is given by

v_{κ} (t) = κ (γ (t)) R θ^{'} (t) v (θ (t)

(66)

The (covariant) a-acceleration of

γ

\nabla_{\frac{d}{d t}} {\dot{v}}_{a} (t) = κ (a) R [θ^{''} (t) v (θ (t)) - θ^{'} {(t)}^{2} u (θ (t))]

(67)

The (covariant)

κ

-acceleration of

γ

writes

\nabla_{\frac{d}{d t}} {\dot{v}}_{κ} (t) = \nabla_{\frac{d}{d t}} ((κ \circ γ) \dot{γ}) (t) = \frac{d (κ \circ γ)}{d t} (t) \dot{γ} (t) + κ \circ γ (t) \nabla_{\frac{d}{d t}} \dot{γ}

(68)

3.2.6. a-Uniform and $κ$ -Uniform Circular Motions

When a circular motion

γ

is a-uniform, in other words when for a sitting observer a the a-speed is a constant, the expression of

θ

has the form

θ (t) = φ + ω t

with

φ

and

ω

some constants. Changing the origin of time we may assume that

φ = 0

, and changing the orientation we may assume that

ω \geq 0

Then

γ (t) = R (c o s (ω t), s i n (ω t), 0)

(in polar coordinates

γ (t) = (R, ω t)

)

For any sitting-observer

a \in R^{3}

the motion will also be circular a-uniform with angular speed

ω

but with apparant radius of the trajectory

R_{a} = κ (a) R

The a-speed and the

κ

-speed writes

v_{a} (t) = R κ (a) ω and v_{κ} (t) = R κ (γ (t)) ω

(69)

The derivative of the

κ

-speed is

\frac{d v_{κ}}{d t} (t) = R^{2} ω^{2} 〈 {G r a d}_{σ (t)} κ, v (ω t) 〉 = R ω^{2} \frac{\partial g}{\partial θ} (σ, ω t)

(70)

where g is defined by

g (r, θ) = κ (r c o s (θ), r s i n (θ), 0) .

Of course if

κ

happens to have radial symmetry, in other words if

\frac{\partial g}{\partial θ} = 0

the motion is also

κ

-uniform.

3.3. $κ$ -Structure on a Minkowski Metric

Let us equip

R^{4}

with the Minkowski metric

G^{M i n k}

. We choose once and for all a global system of coordinates

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

such that the expression of the pseudo-metric is

G^{M i n k} = d σ^{0} d σ^{0} - δ_{i j} d σ^{i} d σ^{j}

Then

\forall e \in R^{4}, \forall u = u^{i} \frac{\partial}{\partial σ^{i}}, v = v^{i} \frac{\partial}{\partial σ^{i}} \in T_{e} R^{4},

G_{e}^{M i n k} (u, v) = u, v = u^{0} v^{0} - (u^{1} v^{1} + u^{2} v^{2} + u^{3} v^{3})

(71)

The choice of the global coordinates system provides a trivial foliation of

R^{4}

which leaves are the equivalence classes of the relation

(σ^{0}, σ^{1}, σ^{2}, σ^{3}) ≃ (σ^{' 0}, σ^{' 1}, σ^{' 2}, σ^{' 3})

when

(σ^{1}, σ^{2}, σ^{3}) = (σ^{' 1}, σ^{' 2}, σ^{' 3})

. Each leaves of that foliation will be called a sitting-observer relative to the chosen global coordinate system.To each point a in

R^{3}

is associated a unique sitting-observer

\bar{a}

Changing the choice for the coordinate system will of course change the sitting-observers.

A Minkowski metric affected with a

κ

-effect is a couple

((σ^{0}, σ^{1}, σ^{2}, σ^{3}), κ)

where

† (σ^{0}, σ^{1}, σ^{2}, σ^{3})

is a global coordinates system such as

G^{M i n k}

has expression

d σ^{0} d σ^{0} - δ_{i j} d σ^{i} d σ^{j} .

† κ : R^{3} \to] m, M [

with

m > 0

is a class

C^{\infty}

function.

When

((σ^{0}, σ^{1}, σ^{2}, σ^{3}), κ)

is a Minkowski metric affected with a

κ

-effect we have

On one hand two pseudo-metrics on

R^{4}

· The Minkowski metric

G^{M i n k} = d σ^{0} d σ^{0} - δ_{i j} d σ^{i} d σ^{j}

defined at

(71)

· The non-flat pseudo-metric

G_{κ} = d σ^{0} d σ^{0} - κ^{2} δ_{i j} d σ^{i} d σ^{j}

defined by

\forall e \in R^{4}, \forall u = u^{i} \frac{\partial}{\partial σ^{i}}, v = v^{i} \frac{\partial}{\partial σ^{i}} \in T_{e} R^{4},

G_{κ, e}^{M i n k} (u, v) = u, v_{κ (\bar{e})} = u^{0} v^{0} - κ^{2} (\bar{e}) (u^{1} v^{1} + u^{2} v^{2} + u^{3} v^{3})

(72)

On the other hand a collection of Minkowski metrics on

R^{4}

\forall a \in R^{3}

G_{κ (\bar{a})}^{M i n k} = d σ^{0} d σ^{0} - κ^{2} (\bar{a}) δ_{i j} d σ^{i} d σ^{j},

defined by

\forall e \in R^{4}, \forall u = u^{i} \frac{\partial}{\partial σ^{i}}, v = v^{i} \frac{\partial}{\partial σ^{i}} \in T_{e} R^{4},

G_{κ (\bar{a})}^{M i n k} (u, v) = u, v_{\bar{a}} = u^{0} v^{0} - κ^{2} (\bar{a}) (u^{1} v^{1} + u^{2} v^{2} + u^{3} v^{3})

(73)

Any information retrieved using the pseudo-metric

G_{κ (\bar{a})}^{M i n k}

R^{4}

is an observation made by a sitting-observer

\bar{a}

. For example, let e and

e^{'}

be two events, if

u

is the unique quadrivector such that

e^{'} = e + u

the sitting observer

\bar{a}

will interpret e and

e^{'}

separated by a time-like gap when

G_{κ (\bar{a})}^{M i n k} (u, u) > 0

. Another sitting-observer may interpret e and

e^{'}

separated by a space-like gap.

Figure 5. Deformation of the ℌfuture-coneℍ at e with

κ (\bar{a}) < κ (\bar{b}) < κ (\bar{c})

the ℌspace-componentℍ,

σ^{s}

represented in dimension 1.

Figure 5. Deformation of the ℌfuture-coneℍ at e with

κ (\bar{a}) < κ (\bar{b}) < κ (\bar{c})

the ℌspace-componentℍ,

σ^{s}

represented in dimension 1.

3.3.1. Quadrivelocities

Let

Γ : R \to R^{4}, τ \mapsto (Γ^{0} (τ), Γ^{1} (τ), Γ^{2} (τ), Γ^{3} (τ))

be a smooth worldline expressed in the coordinates system

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

, the triplet

(Γ^{1} (τ), Γ^{2} (τ), Γ^{3} (τ))

will be denoted

Γ^{s} (τ)

. The sitting-observer associated is

\bar{Γ} (s) (τ)

The quadrivelocity at proper time

τ

\dot{Γ} (τ) = {\dot{Γ}}^{0} (τ) \frac{\partial}{\partial σ^{0}} + {\dot{Γ}}^{i} (τ) \frac{\partial}{\partial σ^{i}} \in T_{Γ (τ)} R^{4}

(74)

where the dot is the derivation with respect to the proper time

τ

, and the summation is on indexes

i \in {1, 2, 3}

The quadrivelocity observed by a sitting observer

\bar{a}

, the

\bar{a}

-quadrivelocityis defined by

{\dot{Γ}}_{\bar{a}} (τ) = {\dot{Γ}}^{0} (τ) \frac{\partial}{\partial σ^{0}} + κ (\bar{a}) {\dot{Γ}}^{i} (τ) \frac{\partial}{\partial σ^{i}}

(75)

so that

\dot{Γ} (τ), \dot{Γ} {(τ)}_{\bar{a}} = {\dot{Γ}}_{\bar{a}} (τ), {\dot{Γ}}_{\bar{a}} (τ)

(76)

The quadrivelocity observed by the family of sitting-observers

{\bar{Γ}}_{s} (τ)

is the κ-quadrivelocity

{\dot{Γ}}_{κ} (τ) = {\dot{Γ}}^{0} (τ) \frac{\partial}{\partial σ^{0}} + κ ({\bar{Γ}}^{s} (τ)) {\dot{Γ}}^{i} (τ) \frac{\partial}{\partial σ^{i}}

(77)

so that

\dot{Γ} (τ), \dot{Γ} {(τ)}_{{\bar{Γ}}_{s} (τ)} = {\dot{Γ}}_{κ} (τ), {\dot{Γ}}_{κ} (τ)

(78)

We have

\dot{Γ} (τ) = {\dot{Γ}}^{0} (τ) (\frac{\partial}{\partial σ^{0}} + \frac{{\dot{Γ}}^{i} (τ)}{{\dot{Γ}}^{0} (τ)} \frac{\partial}{\partial σ^{i}})

(79)

If we put

v_{Γ} (τ) = \frac{{\dot{Γ}}^{i} (τ)}{{\dot{Γ}}^{0} (τ)} \frac{\partial}{\partial σ^{i}}

(80)

we get the equivalence

\dot{Γ} (τ), \dot{Γ} (τ) > 0 ⟺ 〈 v_{Γ} (τ), v_{Γ} (τ) 〉 < 1

(81)

When

\forall τ \in R, \dot{Γ} (τ), \dot{Γ} (τ) > 0

we say that

Γ

is a Minkowski-particle worldline.

Let

\bar{a}

be a sitting-observer we have

{\dot{Γ}}_{\bar{a}} (τ) = {\dot{Γ}}^{0} (τ) (\frac{\partial}{\partial σ^{0}} + κ (\bar{a}) \frac{{\dot{Γ}}^{i} (τ)}{{\dot{Γ}}^{0} (τ)} \frac{\partial}{\partial σ^{i}}) = {\dot{Γ}}^{0} (τ) (\frac{\partial}{\partial σ^{0}} + κ (\bar{a}) v_{Γ} (τ))

(82)

we get the equivalence

\dot{Γ} (τ), \dot{Γ} {(τ)}_{\bar{a}} > 0 ⟺ 〈 v_{Γ} (τ), v_{Γ} (τ) 〉 < \frac{1}{κ^{2} (\bar{a})}

(83)

When

\forall τ \in R, \dot{Γ} (τ), \dot{Γ} {(τ)}_{\bar{a}} > 0

we say that

Γ

is a

\bar{a}

-particle worldline.

We also have

{\dot{Γ}}_{κ} (τ) = {\dot{Γ}}^{0} (τ) (\frac{\partial}{\partial σ^{0}} + κ ({\bar{Γ}}_{s} (τ)) \frac{{\dot{Γ}}^{i} (τ)}{{\dot{Γ}}^{0} (τ)} \frac{\partial}{\partial σ^{i}}) = {\dot{Γ}}^{0} (τ) (\frac{\partial}{\partial σ^{0}} + κ ({\bar{Γ}}^{s} (τ)) v_{Γ} (τ))

(84)

we get the equivalence

\dot{Γ} (τ), \dot{Γ} {(τ)}_{κ} > 0 ⟺ 〈 v_{Γ} (τ), v_{Γ} (τ) 〉 < \frac{1}{κ^{2} ({\bar{Γ}}^{s} (τ))}

(85)

When

\forall τ \in R, \dot{Γ} (τ), \dot{Γ} {(τ)}_{κ} > 0

we say that

Γ

is a

κ

-particle worldline.

Let us put

v_{\bar{a}, Γ} (τ) = κ (\bar{a}) v_{Γ} (τ) and v_{κ, Γ} (τ) = κ ({\bar{Γ}}^{s} (τ)) v_{Γ} (τ)

(86)

for two sitting-observers

\bar{a}

and

\bar{b}

we have

v_{\bar{a}, Γ} (τ) = \frac{κ (\bar{a})}{κ (\bar{b})} v_{\bar{b}, Γ} (τ)

(87)

and

v_{κ, Γ} (τ) = \frac{κ ({\bar{Γ}}^{s} (τ))}{κ (\bar{a})} v_{\bar{a}, Γ} (τ)

(88)

Any of the Minkowskian metrics

G_{κ (\bar{a})}^{M i n k}

is flat, so their Levi-Civita connexions are all equal to the trivial covariant derivative. Therefore we have

\nabla_{\frac{d}{d τ}} \dot{Γ} = 0 ⟺ {\ddot{Γ}}^{i} (τ) = 0 for i = 0, 1, 2, 3

(89)

For any metric

G_{κ (\bar{a})}^{M i n k}

the geodesic worldlines are

σ : R \to R^{4}, τ \mapsto (σ^{0} (τ), σ^{1} (τ), σ^{2} (τ), σ^{3} (τ))

where the coordinate functions

σ^{i}

are affine functions.

3.3.2. Changing Coordinate Systems

Without κ-effect :

The choice of a "reference frame"

F = (O, B)

where

O = (x^{0}, x^{1}, x^{2}, x^{3})

is an event and

B = (\partial_{0}, \partial_{1}, \partial_{2}, \partial_{3})

is a base of

T_{O} R^{4}

such that for

u = u^{i} \partial_{i}

and

v = v^{i} \partial_{i}

two tangent vectors at O, we have

u, v = u^{0} v^{0} - (u^{1} v^{1} + u^{2} v^{2} + u^{3} v^{3}) .

determines a global system of coordinates

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

R^{4}

. For example we can choose

\partial_{i} = \frac{\partial}{\partial σ^{i}}

for

i = 0, 1, 2, 3

Consider

O^{'} : R \to R^{4}, T \mapsto (a_{0} T, a_{1} T, 0, 0)

, with

a_{0}, a_{1} \in R

, be a geodesic worldline. If we adjust a base

B^{'} = (\partial_{0}^{'}, \partial_{1}^{'}, \partial_{2}^{'}, \partial_{3}^{'})

T_{O^{'} (0)} R^{4}

so that the global coordinate system

(σ^{' 0}, σ^{' 1}, σ^{' 2}, σ^{' 3})

generated by the frame

F^{'} = (O^{'} (0), B^{'})

fullfills the conditions

(1) The coordinates functions of a geodesic worldline

R \to R^{4}

are

affine functions

(2) If

e \in R^{4}

has coordinates

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

satisfying

{(σ^{0})}^{2} - ({(σ^{1})}^{2} + {(σ^{2})}^{2} + {(σ^{3})}^{2}) = 0

its coordinates

(σ^{' 0}, σ^{' 1}, σ^{' 2}, σ^{' 3})

satisfy

{(σ^{' 0})}^{2} - ({(σ^{' 1})}^{2} + {(σ^{' 2})}^{2} + {(σ^{' 3})}^{2}) = 0

We obtain

\partial_{0}^{'} = γ (\partial_{0} + v \partial_{1}), \partial_{1}^{'} = γ (v \partial_{0} + \partial_{1}), \partial_{2}^{'} = \partial_{2}, \partial_{3}^{'} = \partial_{3}

(90)

where

v = \frac{a_{1}}{a_{0}} and γ = \frac{1}{\sqrt{1 - v^{2}}}

(91)

Then if e has coordinates

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

F

, its coordinates in

F^{'}

are

σ^{' 0} = γ (σ^{0} - v σ^{1}), σ^{' 1} = γ (- v σ^{0} + σ^{1}), σ^{' 2} = σ^{2}, σ^{' 3} = σ^{3} .

This is the

σ^{1}

-boost.

With the κ-effect :

Let

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

be an event and

\bar{O}

be the sitting-observer associated. We can choose a base

B_{\bar{O}} = (\partial_{0}, \partial_{1}, \partial_{2}, \partial_{3})

T_{O} R^{4}

such that for

u

and

v

two tangent vectors we have

u, v_{\bar{O}} = u^{0} v^{0} - (u^{1} v^{1} + u^{2} v^{2} + u^{3} v^{3})

Such a base is, for example, given by

\partial_{0} = \frac{\partial}{\partial σ^{0}}

and

\partial_{i} = \frac{1}{κ (\bar{O})} \frac{\partial}{\partial σ^{i}}

. Let

F_{\bar{O}}

be the frame

(O, B_{\bar{O}})

As previously, if we consider an inertial observer

O^{'}

passing at

T = 0

by a point of

\bar{O}

adjusting the coordinates system will give

\partial_{0}^{'} = γ_{\bar{O}} (\partial_{0} + v_{\bar{O}} \partial_{1}), \partial_{1}^{'} = γ_{\bar{O}} (v_{\bar{O}} \partial_{0} + \partial_{1}), \partial_{2}^{'} = \partial_{2}, \partial_{3}^{'} = \partial_{3}

(92)

where

v_{\bar{O}} = κ (\bar{O}) \frac{a_{1}}{a_{0}} and γ_{\bar{O}} = \frac{1}{\sqrt{1 - v_{\bar{O}}^{2}}}

(93)

We get the

\bar{O}

σ^{1}

-boost.

3.3.3. Observation of a Far Away Geodesic Worldline

Let

Γ : R \to R^{4}, τ \mapsto Γ (τ) = (Γ^{0} (τ), Γ^{1} (τ), Γ^{2} (τ), Γ^{3} (τ))

be a geodesic worldline.

Each sitting-observer

\bar{a}

interprets

Γ

as a geodesic worldline, the support of

Γ

is rectilinear and the quadrivelocity

\dot{Γ} (τ)

is a constant vector field

\dot{Γ}

along

Γ

Let

ψ : R \to R^{4}

be a reparametrization of this geodesic worldline in such a way that there exists a strictly positive constant

Λ

such that

\forall τ \in R, \dot{ψ} (τ), \dot{ψ} {(τ)}_{\bar{ψ (τ)}} = Λ

(94)

The support is still rectilinear but the quadrivelocity field

τ \mapsto \dot{ψ} (τ)

is no longer constant neverthless the

κ

-quadrivelocity is constant.

ψ

is a reparametrization of the geodesic worldline

Γ

, there exists a function

f : R \to R_{+}^{*}

, such that we have

\dot{ψ} (τ) = f (τ) \dot{Γ}

(95)

so we have

\dot{ψ} (τ), \dot{ψ} {(τ)}_{{\bar{ψ}}_{s} (τ)} = f^{2} (τ) \dot{Γ}, {\dot{Γ}}_{{\bar{ψ}}_{s} (τ)}

(96)

so condition

(94)

reformulates into

\forall τ \in R, f^{2} (τ) = \frac{Λ}{\dot{Γ}, {\dot{Γ}}_{{\bar{ψ}}_{s} (τ)}}

(97)

which can be fullfilled only when

\forall τ \in R, \dot{Γ}, {\dot{Γ}}_{{\bar{ψ}}_{s} (τ)} > 0

(98)

We also get for the worldline

ψ

〈 v_{κ} (τ), v_{κ} (τ) 〉 = \frac{1}{κ^{2} (ψ_{s} (τ))} (1 - \frac{Λ}{{\dot{ψ}}_{0} (τ)})

(99)

Let us remark that

v_{\bar{a}, ψ_{κ}} (τ) = \frac{κ (\bar{a})}{κ (\bar{ψ_{κ, s} (τ)})} v_{κ, σ_{κ}} (τ)

(100)

To a distant sitting-observer

\bar{a}

the worldline

ψ

appears rectilinear, even though it is not a a-geodesic worldline. For example, this means that if along a geodesic worldline

Γ

the function

κ

is decreasing we may get a reparametrization

ψ

Γ

such that

v_{κ, ψ}

is constant while

v_{\bar{a}, ψ}

is not. It is then possible that a distant sitting-observer a observes the

\bar{a}

-quadrivelocity of

κ

-particle worldline being time-like on some portion of the trajectory and space-like on some other portion.

4. Applications

Let us specify that these applications are just given here for illustrative purposes. Concrete and much more complex situations in the case of spiral galaxies and galactic clusters have been discussed elsewhere [2, 3, 4].

We need to clarify "where" the objects we will be considering and "where" the observers actually are. A

κ

-structure is conceived as the trivial bundle

R^{3} \times] m, M [\to R^{3}

where each constant section

R_{λ}^{3} = R^{3} \times {λ}

is equipped with the metric

G_{λ}^{E u c}

and the base space is equipped with the metric

G^{E u c}

. Each leaf

R_{λ}^{3}

of the bundle is accessible to sitting-observers a such that

λ = κ (a)

, those sitting-observers are subject to an illusion (associated to the surrounding density). The real space is the base space where the objets are, but no real observer can see the geometry of that space per se. Rather these observers see the base through the magnifying glass provided by both their own environment and that of the perceived object. Any potential observer in the base space accessing to

G^{E u c}

would be a "shadow-observer".

4.1. What a Sitting-Observer Sees, Size Measurement

Let a and b be two sitting-observers, as the Levi-Civita connections of

G_{κ (a)}^{E u c}

and

G_{κ (b)}^{E u c}

are both equal to the trivial covariant derivation, a and b will agree on which tangent vector fields along a smooth curve

γ

joining them are parallel : the constant vector fields. Assume that

κ (a) \neq κ (b)

and let

U

and

V

be two constant tangent vector fields along

γ

such that

d_{a} (a, a + U (a)) = {κ (a) ∥ U ∥}_{G^{E u c}} = κ (b) {∥ V ∥}_{G^{E u c}} = d_{b} (b, b + V (b))

we have

d_{a} (a, a + V (a)) = {κ (a) ∥ V ∥}_{G^{E u c}} \neq κ (b) {∥ U ∥}_{G^{E u c}} = d_{b} (a, a + U (a))

Figure 3. Discrepancy in size measurement:

d_{a} (a, a + U) = d_{b} (b, b + V)

, while

d_{a} (a, a + U) \neq d_{a} (b, b + V)

Figure 3. Discrepancy in size measurement:

d_{a} (a, a + U) = d_{b} (b, b + V)

, while

d_{a} (a, a + U) \neq d_{a} (b, b + V)

Practically, this means that two different sitting-observers, each one holding a stick, could agree on saying that the two sticks have the same length when they exchange their observations on their own stick, but if one of them use his own measurement system to measure both stick he would say that the two sticks have different lengths.

This can be illustrated on the following figure, two sitting-observers compare the radii of their own unit ball, a sees the unit ball hold by b bigger than his own.

Figure 4. Discrepancy in size measurement for a disk.

Another illustrative point of view appears very interesting. We assume here that there is no gravity. The base is equipped with an Euclidean metric. Consider a free particle emitted at some instant from the point a (Figure 5). Arrived at b the particle emits a photon in the direction of the observer A. Then the observer A sees the particle at the position b’. He measures the spectroscopic velocity

κ_{B} ∥ \dot{σ} ∥

(shown in orange). Likewise the observer B sees the particle starting from a at the position a’ and he measures the spectroscopic velocity

κ_{A} ∥ \dot{σ} ∥

κ

-aberration

In Figure 5 a linear variation of

κ

as a function of

σ

is assumed. Then the image of the straight line ab taken in the base (the real trajectory of the particle P) is represented by a multiplet of parallel straight lines ab’, a’b, ..., each of these lines being attached to a real observer. Let us note that the real trajectory of the particle P in the base space does not seem to be parallel to the corresponding multiplet of its images in the bundle. In fact there is no reason why this should be so. The base space is linked to the bundle by a projection which can diversely tilt any small portion of a trajectory, even though this tilt is fictitious (see also Figure 15, where the fictitious trajectory is shown as composed of a series of infinitesimal segments, each one of these segments being parallel to the real trajectory). Moreover the base space is not accessible to the real observers present in the bundle. Then an unreflected comparison of a vector in the base to the "same" vector in the bundle makes no sense regarding its apparent direction. In the base the vector

\dot{σ}

(denoted by

\dot{σ_{b}}

) is constant for a free particle, while in the bundle it is

κ \dot{σ}

that is a constant vector. In the sheet of the observer A, we have, respectively for the radial (spectroscopic) and (apparent) tangential components of the velocity, as seen by this observer (

d_{A} = 10 A U

)

v_{s p e c} = κ ∥ \dot{σ} ∥ v_{t} = κ_{A} \frac{\dot{κ}}{κ^{2}} \frac{d_{A}}{2}

(101)

the dot over

κ

denotes the Lagrangian time derivative of

κ

. We can verify that if

\dot{κ} = 0

, then the tangential velocity cancels out.

Overlapping images.

Let a be a sitting-observer,

Δ

be a straight line passing through a,

S

and

P

be two planes orthogonal to

Δ

, B be a disk and C a small concentric flattened annulus both in

P

and centered at

Δ \cap P

. Assume that

d_{E u c} (a, P) ≫ R_{B} = R_{C} ≫ d_{E u c} (a, S)

, where

R_{B}

is the radius of B and

R_{C}

the thickness of C, for example

d_{E u c} (a, P) ≫ 1 p c, R_{B} = R_{C} = 10 A U

and

d_{E u c} (a, S) =

a few meters.

The points of B are all affected with a

κ

coefficient

κ_{b}

and the points of C are all affected with a coefficient

κ_{c}

We have

\forall x \in B \cup C, d (a, x) ≃ d (a, P)

. Let

b \in B

and

c \in C

, we have

d_{b} (a, b) = \frac{κ_{b}}{κ_{a}} d_{a} (a, b)

and

d_{c} (a, c) = \frac{κ_{c}}{κ_{a}} d_{a} (a, c)

. Photons are emitted by b and c in direction of a, but of course each emitter estimates the direction of a with its own tools, the ratio

κ_{b} / κ_{c}

induces a magnification of the image received by a on a screen in the plane

S

, as shown in Figure 5.

To illustrate this phenomenon let us consider three cases, in the first case (Figure 6.a)

\frac{κ_{b}}{κ_{c}} = 1

, in the second case (Figure 5.b) we assume that

\frac{κ_{b}}{κ_{c}} > 1

(3 in the Figure 6.b), in the third case (Figure 6.c) we assume the

\frac{κ_{b}}{κ_{c}} < 1

(

1 / 3

in Figure 6.c)

κ_{b} = κ_{c}

there is no

κ

-effect, the observer a perceives an image with no magnification as shown on Figure 6.a. The second case is represented in Figure 5.b. it shows the modification of the image received by a, the image of the flattened annulus is stretched. The third case is represented in Figure 5.c, the image of the central disk is stretched and overlaps the image of the flattened annulus8.

4.2. The Circular Motion of a Test Mass $m = 1$ Around a Motionless MassM

4.2.1. Without $κ$ -Effect

Without

κ

-effect, in other words for any observer considering

R^{3}

endowed with the Euclidean metric

G^{E u c}

, the motion is the usual Newtonian motion.

Let us consider the elementary situation of a very massive point of mass M, located at the origin O of coordinates and surrounded by a spherical gas cloud of very weak density

ρ

with radial symmetry. Limiting us to the examination of a circular motion

t \mapsto σ (t)

of a test mass

m = 1

around this point, the dynamic equation is (assuming the gravitational constant

G = 1

)

\frac{d}{d t} (\dot{σ}) = - \frac{M (R)}{R^{2}} u (t)

(102)

where

u = \frac{σ (t) - O}{∥ σ (t) - O ∥}

is the radial

G^{E u c}

-unit vector at

σ (t)

and R is the constant

G^{E u c}

-distance from

σ (t)

to O and

M (R) = M + 4 π \int_{0}^{R} ρ (r) r^{2} d r

It is a very simple affair to show that the Newtonian speed

v_{N e w t, 0}

is that which would be measured by a fictive observer using the metric

G_{E u c}

. We find the trivial result

v_{N e w, 0} = {(\frac{M + 4 π \int_{0}^{R} ρ r^{2} d r}{R})}^{\frac{1}{2}} = \sqrt{\frac{M (R)}{R}}

(103)

The index 0 indicating that this is what is measured by the fictive observer. In the

κ

-model framework the Newtonian speed

v_{N e w, 0}

as defined above (103) is fictive, it cannot be measured by any real sitting-observer.

4.2.2. With $κ$ -Effect

Consider a local sitting-observer

a_{l o c}

, situated on the trajectory of the test mass. He sees the whole space endowed with the metric

G_{κ (a)}^{E u c}

, but of course this observer has access neither to

κ (a)

nor to

G^{E u c}

. As the

κ

-effect affects the distances but does not affect the masses, the apparent radius of the trajectory is

κ_{l o c} R

, while the mass M remains unchanged. Applying the Newtonian dynamics with this modified metric, the sitting-observer a measures the speed

v_{N e w, l o c} = \sqrt{\frac{M (R)}{κ_{l o c} R}} = \sqrt{\frac{κ_{E}}{κ_{l o c}}} v_{N e w t, E}

(104)

where

v_{N e w, E}

is the Newtonian velocity calculated (but not measured) by a terrestrial observer

a_{E}

We are aware that in astrophysics the radial velocity (the velocity component directed along the line of sight) is measured by spectroscopy while the component of the velocity projected on the sky plane is deduced from the measurement of the proper motions. Thus within the

κ

-model framework, a clear distinction must be made between these two components. We will call spectroscopic velocity the (radial) velocity measured by any observer. This velocity is independent of the sitting observer and is defined by

v_{s p e c} = v_{N e w, l o c} c o s (θ) = \sqrt{\frac{κ_{E}}{κ_{l o c}}} v_{N e w, E} c o s (θ)

(105)

where

θ

is the projection angle along the line of sight.

It is very commonly observed in the outskirts of the spiral galaxies that the density

ρ (r) \sim e x p (- r)

where r is the distance to the center of a galaxy, measured by a terrestrial observer, i.e.

κ_{E} R

. If we assume that

\frac{κ (r)}{κ_{E}} = \frac{1}{1 + L n (\frac{1}{ρ (r)})}

9, we get

\frac{κ (r)}{κ_{E}} ≃ \frac{1}{r}

for

r ≫ 1

. In this case we can conclude that

v_{N e w t, l o c}

becomes constant and by consequence

v_{s p e c}

becomes constant too. In other words, the observed flatness of the rotational curves of the spiral galaxies is correlated to the variation of the density in the disk as a decreasing exponential function of the radius r. A few concrete examples are given in Figure 8.

In contrast, the tangential velocity measured by a terrestrial observer

a_{E}

v_{t a n} = \frac{κ_{E}}{κ_{l o c}} v_{N e w t, l o c} s i n (θ) = \frac{κ_{E}}{κ_{l o c}} \sqrt{\frac{1}{κ_{l o c}}} v_{N e w t, 0} s i n (θ) .

(106)

4.3. An Analysis of the Spiral Substructure

The spiral galaxies are dominant in the Universe. It is therefore most interesting to understand the link existing between the observation of a tight spiral substructure as it would be seen by a fictive Newtonian observer (i.e. located in the Euclidean space without

κ

-effect), compared to the point of view of a sitting-observer, affected by the

κ

-effect. If the

κ

-effect is now taken into account (each real observer lives in the bundle and not in the base which is for them an unreachable place). In a spiral galaxy the density varies as

ρ (σ) ≃ e^{- σ}

and

\frac{1}{κ (σ)} ≃ 1 + σ

Without the

κ

-effect the equation supporting the spiral substructure is that of a tight logarithmic spiral of maximal extension equal to the unit

σ = e^{θ} (θ < 0)

(107)

With the

κ

-effect the equation becomes

\frac{σ}{1 + σ} = e^{θ}

(108)

σ = \frac{e^{θ}}{1 - e^{θ}}

(109)

For any siting-observer a

r = κ (a) \frac{e^{θ}}{1 - e^{θ}} θ < 0

(110)

While a hypothetical Newtonian observer would see a tightly coiled spiral, any sitting-observer sees a grand design spiral. Two distinct sitting-observers a and b see the same grand design spiral, but differing by a homothety ratio

\frac{κ (a)}{κ (b)}

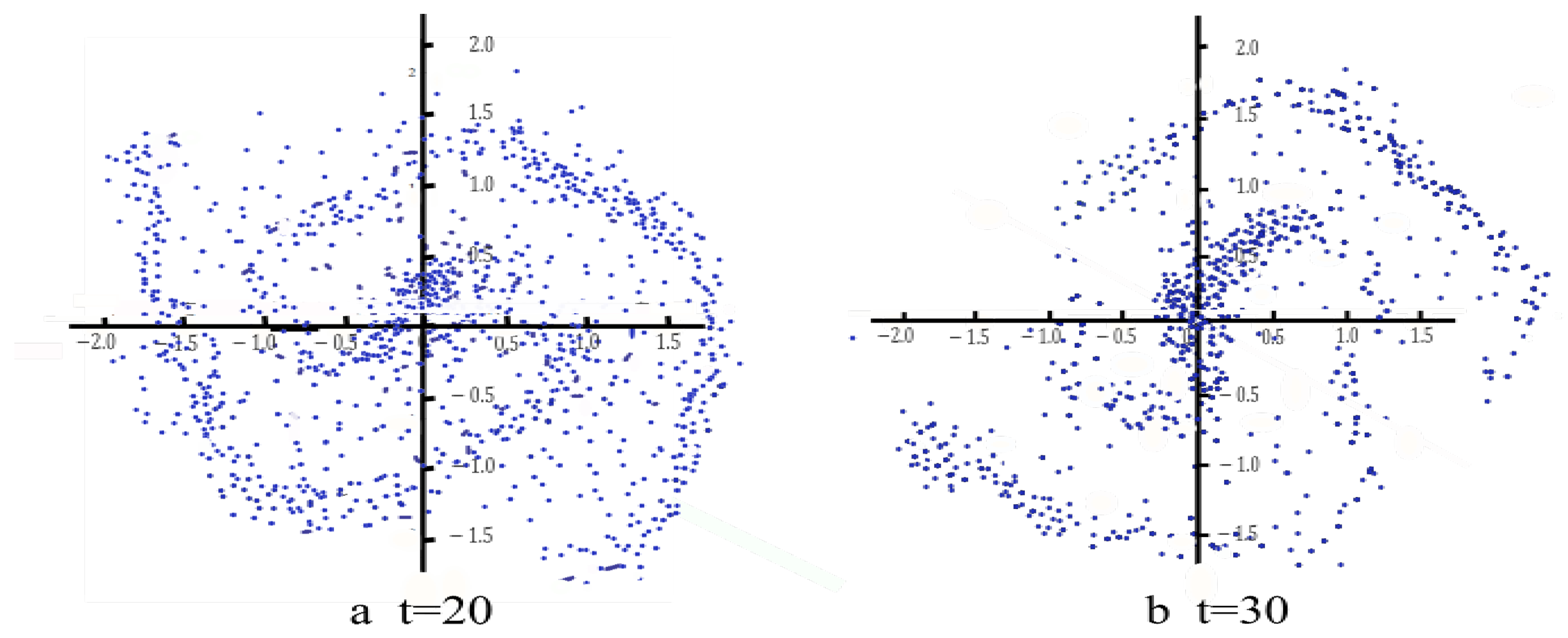
Figure 9. A toy example : A well-developed conservative spiral substructure seen in the bundle as opposed to its counterpart living in the base (a tightly coiled spiral).

Figure 10. A conservative grand design spiral produced by a numerical simulation in the

κ

-model framework (for the details see [2]). The elapsed time is given in the unit of 100 Myr. The Newtonian equivalent would be a much tighter spiral with a larger number of turns.

Figure 10. A conservative grand design spiral produced by a numerical simulation in the

κ

-model framework (for the details see [2]). The elapsed time is given in the unit of 100 Myr. The Newtonian equivalent would be a much tighter spiral with a larger number of turns.

A side-on galaxy

If we assume for simplicity that a terrestrial observer measures a constant thickness for a disk galaxy, then

δ_{E} (σ) = δ_{0}

In addition we have

\frac{κ}{κ_{E}} = \frac{1}{1 + κ_{E} σ}

Then the local thickness is

δ_{L} (σ) = \frac{κ}{κ_{E}} δ_{E} (σ) = \frac{δ_{0}}{1 + κ_{E} σ}

Without

κ

-effect a side-on galaxy, seen as a very extended flat disk of constant thickness by a terrestrial observer, would appear as a much more compact object (for

κ_{E} σ = 10, δ_{L} (σ) = \frac{δ_{0}}{11})

Figure 11. A side-on galaxy seen by a terrestrial observer (a) as opposed to its compact counterpart living in the base (b). On this illustrative example the terrestrial observer measures the density

ρ (r, z) = e x p (- r - (5 + r) | z |)

ρ (r, z) = e x p (- r - (5 + r) | z |)

4.4. Galaxy Clusters

The application of the

κ

-model to the galaxy clusters has been examined in the reference [3]. We have shown that the

κ

-model can greatly lower the major part of the dark matter content in the galaxy clusters. The current dark matter/baryons mass ratio amounts to approximately 10 in the outskirts of these objects, and the

κ

-model can strongly reduce this ratio within the much more acceptable range between 0 and 1. The fits are not optimal in the inner regions of the galaxy clusters, but by lowering the gas temperature in these regions, the problem can also be easily solved. An example is the well-studied COMA cluster (Figure 12).

4.5. The Bullet Cluster

The Bullet Cluster (1E 0657-56) consists of two colliding clusters of galaxies, where a clear separation appears between the stars (compact matter) and the hot gas (diffuse matter). It is a relatively rare situation, even though other cases exhibiting very similar properties are also known (for instance MACS J0025.4-1222). The Bullet Cluster is claimed to provide strong evidence for the existence of dark matter and, on the other hand, seems to severely challenge MOND. Yet the

κ

-model validates the test, when a comparison is made between the gravitational lensing diagrams coming from both dark matter and

κ

-model paradigms (Figure 13).

4.6. Translation of an Extended Object and $κ$ -Effect

We have assumed that the coefficient

κ

is linked to the average mass density,

ρ

, by the relationship

\frac{κ}{κ_{E}} = \frac{1}{1 + l n \frac{ρ_{E}}{ρ}}

(111)

where the index E designates the values associated to any baseline observer (for instance the terrestrial observer). The way we carry out this averaging operation at a given point, may depend on what we are observing. If we observe the inner motion of a little part of a galaxy, the observed zone must be affected with a coefficient

κ_{i}

deduced from an averaging of

ρ

on a "small" ball

U_{i}

with radius

δ_{i} ≃ 1 p c

; now if we observe a galaxy as a whole, taken in a galaxy cluster, we need to affect this galaxy with a coefficient

κ_{e}

, deduced from an average of

ρ

on a ball

U_{e}

, containing the whole galaxy with radius

δ_{e} ≃ 1 M p c

Let us consider a unit gaussian distribution,

w_{δ}

, centered on

r

(Figure 12)

w_{δ} (r^{'} - r) = {(\frac{δ}{π})}^{\frac{3}{2}} e^{{- [\frac{r^{'} - r}{δ}]}^{2}}

(112)

The convolution with any quantity gives the mean value of this quantity. For instance for the mean mass density

{\bar{ρ}}_{δ} (r) = \int_{U_{δ}} w_{δ} (r^{'} - r) ρ (r^{'}) d V

(113)

Figure 14. Averaging over a ball surrounding a star or a galaxy.

Figure 15. Stacking of balls covering a galaxy or a galaxy cluster.

The simultaneous consideration of both inner motions in a galaxy and global motion of the galaxy, as a whole in a galaxy cluster, will necessitate the consideration of two values of

κ

A sitting-observer in the observed galaxy correspond to the selection of two sheets in the bundle

R^{3} \times] m, M [\to R^{3}

or two different ways of measuring distances :

(R^{3}, G_{κ_{e}}^{E u c})

and

(R^{3}, G_{κ_{i}}^{E u c})

As previously the spectroscopic velocities, whether they are associated with internal motions in the galaxy or with global movement of the galaxy, are universally accessible, that means that spectroscopic velocities can therefore slide along a fiber without change.

Let us consider a galaxy moving in a cluster, and two small regions I and J of that galaxy moving into that galaxy.

Figure 16. Point of view of different observers.

With no κ-effect

The velocities of I and J decompose in sums

\dot{I} = {\dot{σ}}_{t} + {\dot{σ}}_{i n t}

and

\dot{J} = {\dot{σ}}_{t} + {\dot{σ}}_{i n t}^{'}

With κ-effect inside the galaxy

At I the coefficients

κ

are

κ_{e x t}

for translational movement and

κ_{i} (I)

for internal movements. An observer

{O ’}_{i}

travelling with the galaxy coinciding with I only perceives the internal movements, i.e. at I that observer measures

κ_{i} (I) {\dot{σ}}_{i n t}

. The fixed observer

O_{i}

measures the speed of the observer

{O ’}_{i}

which is

κ_{e} {\dot{σ}}_{t}

. Finally, the observer

{O ’}_{i}

transmits to

O_{i}

the internal velocity of I. The observer

O_{i}

makes then the sum to get the speed

κ_{e} {\dot{σ}}_{t} + κ_{i} (I) {\dot{σ}}_{i n t}

At J the coefficients

κ

are

κ_{e}

for the translation motion and

κ_{i} (J)

for the inner motions. The fixed observer

O_{j}

measures the speed

κ_{e} {\dot{σ}}_{t} + κ_{i} (J) {\dot{σ}}^{'}_{i n t}

With κ-effect outside the galaxy

For a sitting observer a out of the galaxy the coefficient

κ

is locally computed

κ (a)

. Cancelling the translation velocity of the galaxy, this observer only perceives the internal motions, resp.

κ_{i} {\dot{σ}}_{i n t}

at I and

κ_{j} {\dot{σ^{'}}}_{i n t}

at J. The fixed observer

O_{e}

measures the speed of the observer

{O ’}_{e}

which is obviously unique, i.e.

κ_{e} {\dot{σ}}_{t}

. On the other hand the observer

{O ’}_{e}

transmits to

O_{e}

the values of the internal velocities of I and J. The observer

O_{e}

then makes the respective sums :

at I

κ_{e} {\dot{σ}}_{t} + κ_{i} (I) {\dot{σ}}_{i n t}

at J

κ_{e} {\dot{σ}}_{t} + κ_{i} (J) {\dot{σ}}^{'}_{i n t}

4.7. The Relativistic Extension

In the world of galaxies and galaxy clusters, as both gravity and velocities are weak, a relativistic extension may not appear very useful. However this extension is needed at the cosmological level.

In general relativity, the background, i.e.

R^{4}

, is equipped with a pseudo-Riemannian metric. In some local coordinate system

(σ^{0}, σ^{1}, σ^{2}, σ^{3})

, the expression of the metric at point

φ

has the form

d s_{φ}^{2} = g_{00} (φ) {(d σ^{0})}^{2} + g_{11} (φ) {(d σ^{1})}^{2} + g_{22} (φ) {(d σ^{2})}^{2} + g_{33} (φ) {(d σ^{3})}^{2}

where

g_{i i}

are some functions of

φ

with

g_{00} > 0

and

g_{i i} < 0

for

i \in {1, 2, 3}

Hence the background is equipped with a pseudo-distance. An ideal observer having access to this pseudo-metric would use this metric for measurement of the space-time interval between two events,

φ_{1}

and

φ_{2}

, in other words, the pseudo-length of the

d s^{2}

-geodesic segment joining

φ_{1}

φ_{2}

For

i, j \in {1, 2, 3}

distinct, the Christoffel symbols are

Γ_{00}^{0} = \frac{g^{00}}{2} \frac{\partial g_{00}}{\partial σ^{0}}, Γ_{i 0}^{0} = Γ_{0 i}^{0} = \frac{g^{00}}{2} \frac{\partial g_{00}}{\partial σ^{i}}, Γ_{i i}^{0} = - \frac{g^{00}}{2} \frac{\partial g_{i i}}{\partial σ^{0}}, Γ_{i j}^{0} = 0

For

i \in {1, 2, 3}

and

j, k \in {0, 1, 2, 3} ∖ {i}

distinct (with no summation over i)

Γ_{i i}^{i} = \frac{g^{i i}}{2} \frac{\partial g_{i i}}{\partial σ^{i}}, Γ_{j i}^{i} = Γ_{i j}^{i} = \frac{g^{i i}}{2} \frac{\partial g_{i i}}{\partial σ^{j}}, Γ_{j j}^{i} = - \frac{g^{i i}}{2} \frac{\partial g_{j j}}{\partial σ^{i}}, Γ_{j k}^{i} = 0

In the

κ

-model each sitting-observer a associates to the background a modified pseudo-metric. The modification is a rescaling of the spatial coordinates of tangent quadrivectors with no change of time coordinate. Then the general form of modified pseudo-metric is

d s_{λ, φ}^{2} = g_{00} (φ) {(d σ^{0})}^{2} + λ^{2} (g_{11} (φ) {(d σ^{1})}^{2} + g_{22} (φ) {({d σ}^{2})}^{2} + g_{33} (φ) {(d σ^{3})}^{2})

Each current sitting-observer a is provided with a value for

λ = κ (a)

The modification of the pseudo-metric induces a modification of the Christoffel symbols. If we note

{\overset{λ}{g}}_{i j}

the coefficients of the modified metric

d s_{λ}^{2}

we have

{\overset{λ}{g}}_{00} = g_{00}, and for i \in {1, 2, 3}, {\overset{λ}{g}}_{i i} = λ^{2} g_{i i},

then

\overset{λ}{g}^{00} = g^{00} and \overset{λ}{g}^{i i} = \frac{1}{λ^{2}} g^{i i}

the Christoffel symbols of the modified metric and the initial metric are linked

\overset{λ}{Γ}_{00}^{0} = Γ_{00}^{0}, \overset{λ}{Γ}_{i 0}^{0} = Γ_{i 0}^{0}, \overset{λ}{Γ}_{i i}^{0} = λ^{2} Γ_{i i}^{0}, \overset{λ}{Γ}_{i j}^{0} = Γ_{i j}^{0} = 0

\overset{λ}{Γ}_{i i}^{i} = Γ_{i i}^{i}, \overset{λ}{Γ}_{j i}^{i} = Γ_{j i}^{i}, \overset{λ}{Γ}_{j j}^{i} = Γ_{j j}^{i}, \overset{λ}{Γ}_{00}^{i} = \frac{1}{λ^{2}} \overset{λ}{Γ}_{00}^{i}, \overset{λ}{Γ}_{j k}^{i} = Γ_{j k}^{i} = 0

This means that the supports of geodesic curves may differ from one observer to another.

The full background is also equipped with a modified pseudo-metric.

d s_{κ, φ}^{2} = g_{00} (φ) {(d σ^{0})}^{2} + κ^{2} (φ) (g_{11} (φ) {(d σ^{1})}^{2} + g_{22} (φ) {({d σ}^{2})}^{2} + g_{33} (φ) {(d σ^{3})}^{2})

If we note

{\overset{κ}{g}}_{i j}

the coefficients of the modified metric

d s_{κ}^{2}

we have

{\overset{κ}{g}}_{00} = g_{00}, and for i \in {1, 2, 3}, {\overset{κ}{g}}_{i i} = κ^{2} g_{i i},

then

\overset{κ}{g}^{00} = g^{00} and \overset{κ}{g}^{i i} = \frac{1}{λ^{2}} g^{i i}

the Christoffel symbols of this modified metric and the initial metric are linked (with no summation over i)

\overset{κ}{Γ}_{00}^{0} = Γ_{00}^{0}, \overset{κ}{Γ}_{i 0}^{0} = Γ_{i 0}^{0}, \overset{κ}{Γ}_{i i}^{0} = κ^{2} Γ_{i i}^{0} - \frac{1}{κ} \frac{\partial κ}{\partial σ^{0}}, \overset{κ}{Γ}_{i j}^{0} = Γ_{i j}^{0} = 0

\overset{κ}{Γ}_{i i}^{i} = Γ_{i i}^{i} + \frac{1}{κ} \frac{\partial κ}{\partial σ^{i}}, \overset{κ}{Γ}_{j i}^{i} = Γ_{j i}^{i} + \frac{1}{κ} \frac{\partial κ}{\partial σ^{j}}, \overset{κ}{Γ}_{j j}^{i} = Γ_{j j}^{i} - \frac{g^{i i} g_{j j}}{κ} \frac{\partial κ}{\partial σ^{i}},

\overset{κ}{Γ}_{00}^{i} = \frac{1}{κ^{2}} Γ_{00}^{i}, \overset{κ}{Γ}_{j k}^{i} = Γ_{j k}^{i} = 0

The geodesics of this pseudo-metric are not geodesics for any metric used by sitting-observers

4.7.1. Without Gravity

Without gravity the metric

d s^{2}

is Minkowskian, we have

g_{00} = η_{00} = 1, g_{11} = g_{22} = g_{33} = η_{11} = η_{22} = η_{33} = - 1 .

In this special case the

κ

-effect does not affect the Christoffel symbols, and then the geodesic equation has been considered in paragraph 3.3.3.

4.7.2. With Weak Gravity

Without $κ$ -effect

In the case of weak gravitational fields, the metric coefficients are slightly modified

For i \in {0, 1, 2, 3}, g_{i i} = η_{i i} becomes {\tilde{g}}_{i i} = η_{i i} + h_{i i}

With

h_{i i}

functions of the position and

| h_{i i} | ≪ 1

, then we have

{\tilde{g}}^{i i} ≃ η^{i i} - h^{i i}

Furthermore if we assume that the weak gravitation field is stationary, we have

for i \in {0, 1, 2, 3}, \frac{\partial h_{i i}}{\partial σ^{0}} ≃ 0

In this situation, for

i, j \in {1, 2, 3}

distinct, the Christoffel symbols are

Γ_{00}^{0} ≃ 0, Γ_{i 0}^{0} = Γ_{0 i}^{0} ≃ \frac{η^{00}}{2} \frac{\partial h_{00}}{\partial σ^{i}}, Γ_{i i}^{0} ≃ 0, Γ_{i j}^{0} = 0

For

i \in {1, 2, 3}

and

j, k \in {0, 1, 2, 3} ∖ {i}

distinct (with no summation over i)

Γ_{i i}^{i} ≃ \frac{η^{i i}}{2} \frac{\partial h_{i i}}{\partial σ^{i}}, if j \neq 0, Γ_{j i}^{i} = Γ_{i j}^{i} ≃ \frac{η^{i i}}{2} \frac{\partial h_{i i}}{\partial σ^{j}}, and Γ_{0 i}^{i} = Γ_{i 0}^{i} ≃ 0

Γ_{j i}^{i} = Γ_{i j}^{i} ≃ 0, Γ_{j j}^{i} ≃ - \frac{η^{i i}}{2} \frac{\partial h_{j j}}{\partial σ^{i}}, Γ_{j k}^{i} = 0

τ \mapsto γ (τ) = (γ^{0} (τ), γ^{1} (τ), γ^{2} (τ), γ^{3} (τ))

is a geodesic we have

(\frac{d}{d τ} {\dot{γ}}^{k} + Γ_{i j}^{k} {\dot{γ}}^{i} {\dot{γ}}^{j}) \frac{\partial}{\partial σ^{k}} = 0

(114)

where

{\dot{γ}}^{i} = \frac{d γ_{i}}{d τ}

For the first component we get

\frac{d}{d τ} ({\dot{γ}}^{0}) ≃ 0

(115)

In the case of low velocities, i.e.

{\dot{γ}}^{1}, {\dot{γ}}^{2}, {\dot{γ}}^{3} ≪ {\dot{γ}}_{0}

, the spatial components of the geodesic equation reduces to

\frac{d}{d τ} ({\dot{γ}}^{i}) + Γ_{00}^{i} {({\dot{γ}}^{0})}^{2} ≃ 0

(116)

For

i \in {1, 2, 3}

With $κ$ -effect

The metric coefficients are changed :

η_{i i} + h_{i i} \to κ^{2} (η_{i i} + h_{i i})

for

i \in {1, 2, 3}

. With the same hypothesis of weak stationary gravity and low speed, the geodesic equation simplifies (for eq. 117, see eq. 60)

\begin{matrix} \frac{d}{d τ} ({\dot{γ}}^{0}) & ≃ 0 \end{matrix}

(117)

\begin{matrix} \frac{d}{d τ} (κ [γ (τ)] {\dot{γ}}^{i}) + \overset{κ}{Γ}_{00}^{i} {({\dot{γ}}^{0})}^{2} & ≃ 0 \end{matrix}

(118)

for

i \in {1, 2, 3}

with

\overset{κ}{Γ}_{00}^{i} \sim \frac{1}{2 κ^{2}} \frac{\partial h_{00}}{\partial σ^{i}}

. Using the variable

γ^{0} (τ)

, for

i \in {1, 2, 3}

\frac{d γ^{i}}{d τ} = {\dot{γ}}^{0} \frac{d γ^{i}}{d γ^{0}}

and

\frac{d}{d τ} [κ [γ (τ)] \frac{d γ^{i}}{d τ}] + \overset{κ}{Γ}_{00}^{i} {({\dot{γ}}^{0})}^{2} = \frac{d}{d τ} [{\dot{γ}}^{0} κ [γ (τ)] \frac{d γ^{i}}{d γ^{0}}] + \overset{κ}{Γ}_{00}^{i} {({\dot{γ}}^{0})}^{2}

= \frac{d}{d τ} [{\dot{γ}}^{0}] . κ [γ (γ^{0})] \frac{d γ^{i}}{d γ^{0}} + {\dot{γ}}^{0} κ [γ (γ^{0})] \frac{d}{d τ} [\frac{d γ^{i}}{d γ^{0}}] + \overset{κ}{Γ}_{00}^{i} {({\dot{γ}}^{0})}^{2}

= ({\dot{γ}}^{0})^{2} \frac{d}{d γ^{0}} [κ [(γ^{0})] \frac{d γ^{i}}{d γ^{0}}] + \overset{κ}{Γ}_{00}^{i} {({\dot{γ}}^{0})}^{2}

Eventually

(118)

becomes

\frac{d}{d γ^{0}} [κ [γ (γ^{0})] \frac{d γ^{i}}{d γ^{0}}] = - \overset{κ}{Γ}_{00}^{i} = - \frac{1}{2 κ {[γ (γ^{0})]}^{2}} \frac{\partial h_{00}}{\partial σ^{i}} .

(119)

Let the Schwarzschild metric (with the attractive mass M situated at the origin of the coordinates and very far from the singularity). We have then

h_{00} = - \frac{2 M}{σ}

For any sitting-observer a, equipped with his own coordinates and located along the trajectory of the test mass, the motion equation is (

γ^{0} \equiv t

with the speed of light

c = 1

)

\frac{d}{d t} [\frac{κ [γ (t)]}{κ (a)} \frac{d r_{a}}{d t}] = - {(\frac{κ (a)}{κ [γ (t)]})}^{2} M \frac{r_{a}}{r_{a}^{3}}

(120)

where the right side of this equation can be compared to eq.

(60)

. Especially for a terrestrial observer equipped with his usual coordinates (

r (x, y, z)

)

\frac{d}{d t} [\frac{κ [γ (t)]}{κ_{E}} \frac{d r}{d t}] = - {(\frac{κ_{E}}{κ [γ (t)]})}^{2} M \frac{r}{r^{3}}

(121)

The motions perceived by two sitting-observers are homothetic to each other. All observers are equivalent and there is no priviliged observer.

Gravitational Tide

Let A and

A^{'}

be two particles submitted to the gravitational action of a massive particle of mass M and located at the origin of coordinates. The two particles are assumed to be very close to each other. Let

r

and

r^{'}

their respective position vectors. Putting

δ r = r^{'} - r

, the relative motion is given by the equation

\frac{d}{d t} [\frac{κ [γ (t)]}{κ_{E}} \frac{d}{d t} δ r] = - {(\frac{κ_{E}}{κ [γ (t)]})}^{2} M \frac{[r^{2} δ r - 3 (δ r . r) r]}{r^{5}}

(122)

Let us note that when

κ [γ (t)] < κ_{E}

, the self-interaction is strengthened.

Two "paradoxes" that emerge from the motion of free particles

For a free motion the equation is

\frac{d}{d t} [\frac{κ [γ (t)]}{κ_{E}} \frac{d r}{d t}] = 0

(123)

\frac{d}{d t} [\frac{d r}{d t}] = - \frac{d}{d t} [\frac{κ [γ (t)]}{κ_{E}}] \frac{d r}{d t}

(124)

i. A free particle (not submitted to any force) may exhibit an (apparent) auto-accelerated (or auto-decelerated) motion for a terrestrial observer. This result seems to be inconsistent with the first law of Newton. In fact the motion perceived by a terrestrial observer is fictitious. This motion is the image of a real motion taking place in the base. In the base the first law of Newton is obviously respected.

ii. Let two free particles A and

A^{'}

, sufficiently close to each other to share the same

κ

, moving along parallel straight lines in the base. Paradoxically enough, for a terrestrial observer these particles can now appear to move toward and away from each other (Figure 17), following the sign of

\dot{κ}

In other words the trajectories of these particles no longer appear as two absolutely continuous parallel lines. Each of these lines rather appears as a kind of Devil’s Staircase. The relative motion of these two particles reflects the apparent variation of the size of any astronomical object (a planetary system in a galaxy or a galaxy in a galaxy cluster), represented by a gray disk on Figure 15. The variation represented on the latter figure has been artificially magnified. In reality such a variation is very slow and it cannot be detected on the scale of a few hundred of millennia. In the base the velocity

\dot{σ}

(denoted

\dot{σ_{b}}

on Figure 17), measured by a fictitious observer, obeys to the equation (free particle)

\frac{d \dot{σ}}{d t} = 0

(125)

and

\dot{σ} = c o n s t

, whereas in the bundle the velocity

κ \dot{σ}

(measured by a local observer), obeys to the equation

\frac{d}{d t} [κ \dot{σ}] = 0

(126)

and in this case

\dot{σ} \propto κ^{- 1}

. If Figure 17 displays the projection of a moving disk on the sky plane, for instance as seen by a terrestrial observer E, then the measured velocity is

κ_{E} \dot{σ}

. For him the size of the disk (shown in orange) increases in a first phase and then decreases in a second phase. Likewise the velocity of the disk varies as

\frac{κ_{E}}{κ}

(increasing in a first phase and decreasing in a second phase). In fact the motion perceived by the terrestrial observer is fictitious, the real velocity is constant (free particle).

We have presented in this paper the formalism of the

κ

-model. Astonishingly this formalism is fairly straightforward when compared to other formalisms which try to modify the standard general relativity [5, 6, 7]. The

κ

-model is thus characterized by its relative simplicity. Furthermore any type of modified gravity necessarily leads to the introduction of new fields and surreptitiously to new particles. Thus all the various versions of modified gravity, with their lot of new unknown particles, even seems much more complex compared to the dark matter paradigm (Newtonian in essence), apparently for a very limited gain in the understanding. However the number of parameters in

5. Conclusion

We have presented in this paper the formalism of the

κ

-model. The basic hypotheses of this new paradigm are reasonably simple, and the

κ

-model exhibits some common points with the MOND theory [8, 9]. Thus, at least in its original form, MOND, relying on an unique universal parameter,

a_{0}

, also constitutes a straigthforward way to predictively generate the rotational curves of individual galaxies. Unfortunately some issues persist for MOND at the scale of the galactic clusters, even though some solutions have been proposed to circumvent the problem [7]. The

κ

-model has the same level of efficiency as MOND to fairly predict the profiles of the rotational curves of individual spiral galaxies [4], but moreover can also help to understand the physics of galactic clusters [3]. Other interesting ways have also been suggested to circumvent the dark matter conundrum [8, 9, 10, 11,12]. We have already noticed that the latter theories share some common points with the

κ

-model [4]. Eventually we can report that, in parallel, a study focusing on the cosmological implications of the

κ

-model is in progress.

Data Availability Statement

The authors confirm that the data supporting the findings of this study are available within the article and the reference list.

Acknowledgments

the authors wish to thank the anonymous reviewers for useful comments and suggestions.

Conflicts of Interest

The authors declare no conflict of interest.

References

Pascoli, G., and Pernas, L., 2020, hal.archives-ouvertes.fr/hal-02530737.
Pascoli, G. Astrophys. Space Sci. 2022; 367, 121.
Pascoli, G. Can. J. Phys. 2023; 101, 11.
Pascoli, G, 2024, Universe, 10(3), 151. [CrossRef]
Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophysical Journal 1983, 270, 365. [Google Scholar] [CrossRef]
Milgrom, M. Studies in History and Philosophy of Modern Physics 2020, 7, 170. [CrossRef]
Hodson, A.O., and Zhao, H., A&A, 2017, 598, A127. 2017, 598, A127. [CrossRef]
Varieschi, G.U., J.Appl.Math.Phys., 2018 06, 1247. [CrossRef]
Varieschi, G.U., Found.Phys., 2020, 50 11, 1608. [CrossRef]
Cesare V., Diaferio A., Matsakos T., and Angus, G., 2020, A&A, 637, A70. [CrossRef]
Varieschi, G.U. Universe 2021, 10, 387 r. [CrossRef]
Varieschi, G.U. Eur. Phys. J. Plus 2021, 136, 183. [CrossRef]

1	It is not relevant to note the particular scalar product one considers because the orthogonal groups of two different scalar products on $R^{n}$ are isomorphic.
2	A part C of a topological space E is said path connected when for any points a and b of C there is a continuous application $γ : [0, 1] \to E$ such that $γ (0) = a, γ (1) = b$ and $\forall t \in [0, 1], γ (t) \in C$ in other words a pathin C with source a and goal b
3	It is well-known linear applications between two finite dimensional normed vector spaces are always continuous, so the condition of continuity of $d f /_{x}$ can be omited in the definition.
4	The usual notation can be somehow tricky because the dependance on the curve $γ$ is not noted.
5	The application $U \circ γ : R \to T R^{n}$ can be seen as a section of the trivial vector bundle over $R$ with fibers $T_{γ (t)} R^{n}$ the covariant derivation along $γ$ is then the flat covariant derivative of $U \circ γ$ relative to the tangent field $\frac{d}{d t}$ over $R$ .
6	$\forall U, V \in Γ (R^{n}), \nabla_{U} V - \nabla_{V} U = [U, V]$ .
7	Let the action for a free particle $S = \int κ (σ) \sqrt{{(\frac{d σ}{d s})}^{2}} d s$ Applying the Hamilton’s Principle we find the geodesic equation (curve displayed in blue in Figure 5) $\frac{d}{d s} (κ \frac{d σ}{d s}) - \nabla κ = 0$
8	We have consider a non-continuous function $κ$ for a better visualisation of the overlapping, but if we had considered a continuous function $κ$ we would have found a "smooth" overlapping with three layers.
9	The detailed relationship is $\frac{κ (r)}{κ_{M}} = \frac{1}{1 + L n (\frac{ρ_{M}}{ρ (r)})}$ where $ρ_{M}$ denotes the maximal density in the galaxy (galaxy center). Here we assume that $ρ_{M} \sim ρ_{E}$ and the density $ρ$ is normalized to this value.

Figure 5. Two observers A and B separated by a very large distance

≫ 1 p c

. Very close to each of them an universal "atom" of small size

\sim 10 A U

is represented. The dashed straight line ab’ (resp. a’b) is a geodesic of the sheet of the observer A (resp. B) equipped with

κ_{A}

(resp.

κ_{B}

). Let us note that the norm of the vector

\dot{σ}

is a constant in the base, but this norm varies as

\frac{1}{κ}

in the respective sheets of the observers. On the other hand the curve ab" displayed in blue is a geodesic of the bundle equipped of a variable

κ

Figure 5. Two observers A and B separated by a very large distance

≫ 1 p c

. Very close to each of them an universal "atom" of small size

\sim 10 A U

is represented. The dashed straight line ab’ (resp. a’b) is a geodesic of the sheet of the observer A (resp. B) equipped with

κ_{A}

(resp.

κ_{B}

). Let us note that the norm of the vector

\dot{σ}

is a constant in the base, but this norm varies as

\frac{1}{κ}

in the respective sheets of the observers. On the other hand the curve ab" displayed in blue is a geodesic of the bundle equipped of a variable

κ

Figure 6. a : Images obtained without

κ

-effect, the annulus is represented in yellow; the central disk is represented in red. b : Images obtained with

k a p p a

-effect, the emitter in the annulus sees the observer a, three time closer. The gap in the image is a consequence of

κ

discontinuity at, the border between the annulus and the central disk. c : Images obtained with

k a p p a

-effect, emiter in the central disk see a three time closer, The image of the central disk overlaps the image of the annulus, the overlapping, region is colored in orange.

Figure 6. a : Images obtained without

κ

-effect, the annulus is represented in yellow; the central disk is represented in red. b : Images obtained with

k a p p a

-effect, the emitter in the annulus sees the observer a, three time closer. The gap in the image is a consequence of

κ

discontinuity at, the border between the annulus and the central disk. c : Images obtained with

k a p p a

-effect, emiter in the central disk see a three time closer, The image of the central disk overlaps the image of the annulus, the overlapping, region is colored in orange.

Figure 7. Circular Newtonian motion

Figure 8. Galaxy rotation velocity profiles: a. The Milky Way in the vicinity of the Sun, b. M33, c. NGC 1560, d. NGC6946. For the details see [2].

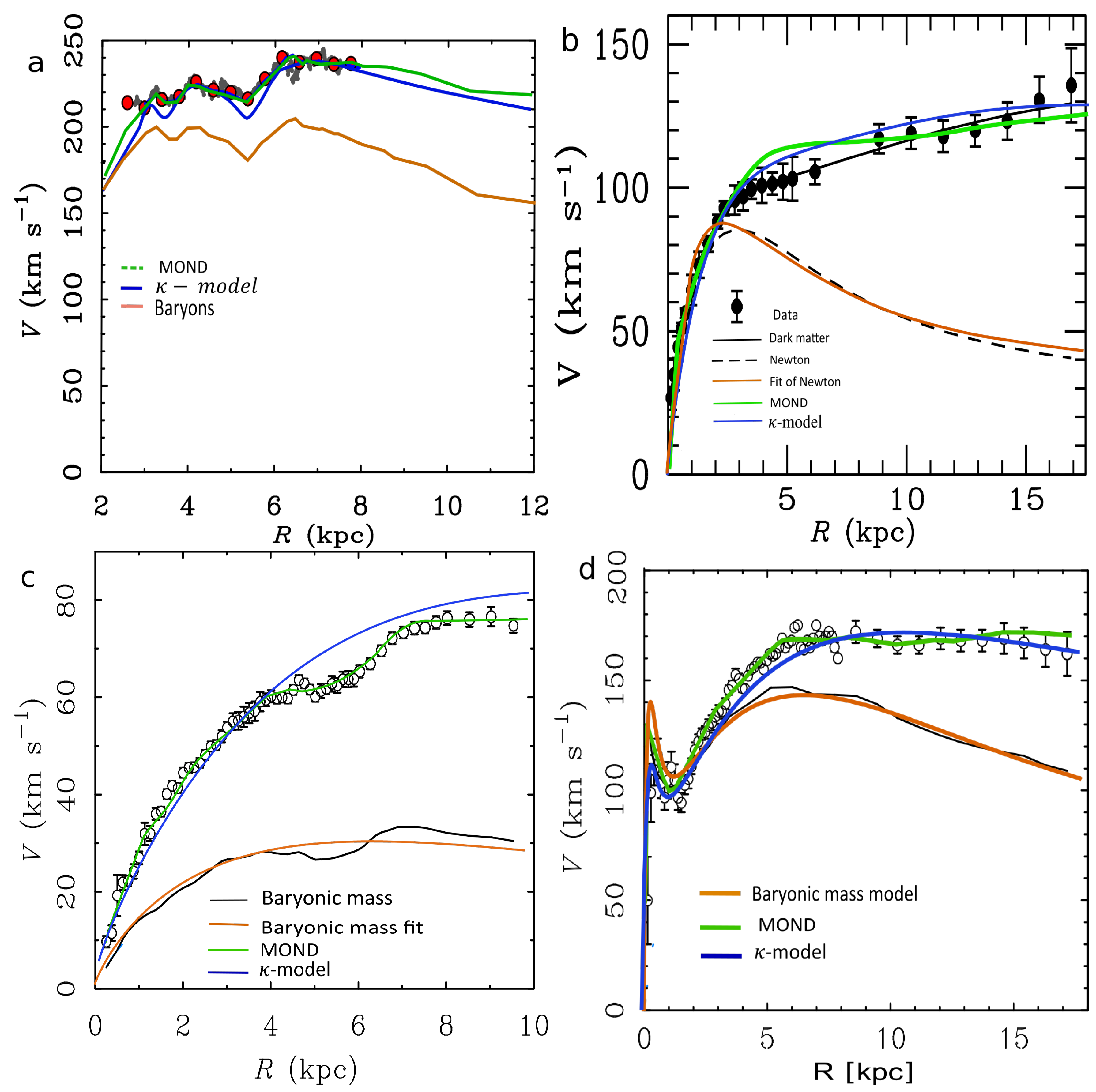
Figure 12. The short dashed blue curve is the Newtonian dynamic mass; the dashed-dotted cyan curve is the MOND dynamic mass. The dynamic mass for

κ

-model is displayed as the amber curve (dynamic mass with constant temperature

T = 8.38 k e V

) and green curve (assuming a non-isothermal temperature profile. The red long dashed curve is the ICM gas mass derived from X-ray observations (for the details see [3]).

Figure 12. The short dashed blue curve is the Newtonian dynamic mass; the dashed-dotted cyan curve is the MOND dynamic mass. The dynamic mass for

κ

-model is displayed as the amber curve (dynamic mass with constant temperature

T = 8.38 k e V

) and green curve (assuming a non-isothermal temperature profile. The red long dashed curve is the ICM gas mass derived from X-ray observations (for the details see [3]).

Figure 13. A comparison between the gravitational lensing diagrams resulting from the application of both dark matter and

κ

-model paradigms in the case of the Bullet Cluster (for the details see [3]).

Figure 13. A comparison between the gravitational lensing diagrams resulting from the application of both dark matter and

κ

-model paradigms in the case of the Bullet Cluster (for the details see [3]).

Figure 17. Apparent variation of a disk of constant size seen from the point of view of a terrestrial observer. The real size is the disk shown in gray.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Is Dark Matter a Misinterpretation of a Perspective Effect?

Abstract

1. Introduction

2. Some Basic Structures on R n

2.1. Algebraic Structures

2.1.1. Affine and Vectorial Structure

2.1.2. Euclidean Structures

2.1.3. Minkowski Structure

2.2. Topological Structure of R n

2.3. Differentiable Structure of R n

2.3.1. Differential of a Function, Tangent Vectors at a Point

2.3.2. Tangent Vector Fields on R n

2.3.3. Covariant Derivation

2.3.4. Flat Covariant Derivation

2.3.5. Covariant Derivation Along a Curve, Parallel Transport

2.3.6. Acceleration

2.4. Riemannian Structures on R n

2.4.1. Levi-Civita Connection

2.4.2. Some Riemannian Metrics

2.5. Pseudo-Riemannian Structure on R 4

3. Framework for the κ -Model

3.1. κ -Structure on a Riemannian Metric

3.2. κ -Structure on the Euclidean Metric

3.2.1. Speed Fields

3.2.2. κ -Uniform Straight Lines

3.2.3. Velocity Fields and Covariant Accelerations

3.2.4. Laser Distance

3.2.5. Circular Motions

3.2.6. a-Uniform and κ -Uniform Circular Motions

3.3. κ -Structure on a Minkowski Metric

3.3.1. Quadrivelocities

3.3.2. Changing Coordinate Systems

3.3.3. Observation of a Far Away Geodesic Worldline

4. Applications

4.1. What a Sitting-Observer Sees, Size Measurement

4.2. The Circular Motion of a Test Mass m = 1 Around a Motionless MassM

4.2.1. Without κ -Effect

4.2.2. With κ -Effect

4.3. An Analysis of the Spiral Substructure

4.4. Galaxy Clusters

4.5. The Bullet Cluster

4.6. Translation of an Extended Object and κ -Effect

4.7. The Relativistic Extension

4.7.1. Without Gravity

4.7.2. With Weak Gravity

5. Conclusion

Data Availability Statement

Acknowledgments

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe

2. Some Basic Structures on $R^{n}$

2.2. Topological Structure of $R^{n}$

2.3. Differentiable Structure of $R^{n}$

2.3.2. Tangent Vector Fields on $R^{n}$

2.4. Riemannian Structures on $R^{n}$

2.5. Pseudo-Riemannian Structure on $R^{4}$

3. Framework for the $κ$ -Model

3.1. $κ$ -Structure on a Riemannian Metric

3.2. $κ$ -Structure on the Euclidean Metric

3.2.2. $κ$ -Uniform Straight Lines

3.2.6. a-Uniform and $κ$ -Uniform Circular Motions

3.3. $κ$ -Structure on a Minkowski Metric

4.2. The Circular Motion of a Test Mass $m = 1$ Around a Motionless MassM

4.2.1. Without $κ$ -Effect

4.2.2. With $κ$ -Effect

4.6. Translation of an Extended Object and $κ$ -Effect