Preprint
Article
Surface Thermodynamic Properties of Styrene–Divinylbenzene Copolymer Modified by Supramolecular Structure of Melamine using Inverse Gas Chromatography
Altmetrics
Downloads
91
Views
18
Comments
0
A peer-reviewed article of this preprint also exists.
supplementary.docx (69.73KB )
This version is not peer-reviewed
Submitted:
16 July 2024
Posted:
17 July 2024
You are already at the latest version
Alerts
Abstract
The surface thermodynamic properties of polymers and copolymers modified by supramolecular structures are very used in several industrial processes, such as selective adsorption, paints, coatings, colloids, and adhesion applications. Background: Inverse gas chromatography at infinite dilution was proved to be the best technique to determine the surface properties of solid surfaces by studying the adsorption of some model polar and non-polar organic molecules adsorbed on solid surfaces by varying the temperature. Methods: The retention volume of adsorbed solvents is a precious parameter to obtain the London dispersive and polar free energies and the London dispersive surface energy of styrene–divinylbenzene copolymer modified by supramolecular structure of melamine using the Hamieh thermal model and our new methodology consisting in the separation of the two polar and dispersive free energy of interaction. This led to the determination of the polar acid and base surface energy, and the Lewis’s acid-base constants of the various solid materials. Results: all surface energetic properties of styrene–divinylbenzene copolymer modified by melamine at different percentages were determined as a function of temperature, following our new methodology. Conclusions: It was observed that the styrene–divinylbenzene copolymer exhibited the highest London dispersive surface energy which decreased when the melamine percentage increased. All materials presented higher Lewis’s basicity and this Lewis’s basicity increased with the percentage of melamine.
Keywords:
Subject: Chemistry and Materials Science - Materials Science and Technology
1. Introduction
Several papers were devoted in the last thirty years to two-dimensional network supramolecular structures due the important applications in the domain of molecular recognition and surface chemistry [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16]. When supported on solid surfaces, these heterocyclic compounds, such as melamine, cyanuric acid, and uracil and its derivatives, are likely to constitute supramolecular network structures capable of selective adsorbing various molecules, including enantiomers [10].
In the case of formed supramolecular structures on the surface of inert supports and porous polymer sorbents, it was showed that the properties of resulting adsorbents depend on the polarity of the surface and porosity of the initial adsorbents [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16]. The effect of the amount of the supported modifier on the properties of the obtained adsorbents was studied by Sukhareva et al. [3] and Guskov et al. [10,11,12,13,14,15,16] using the inverse gas chromatography technique. They studied the specific interactions between the various polymer adsorbents with some model organic solvents as well as the London dispersive energy of the various solid surfaces using Dorris-Gray method [17]. Whereas, it was proved in other works that the classic chromatographic method such as Schultz et al [18] and Dorris-Gray methods cannot be used for an accurate evaluation of the London dispersive surface energy of solid materials, because these methods were based on the hypothesis constant surface area organic solvents. In several studies [19,20,21,22,23], an important effect of the temperature on the surface area of organic molecules was highlighted and more accurate values of the London dispersive and polar components of the surface energy, and the Lewis acid-base properties of various solid substrates were obtained using the Hamieh thermal model. Furthermore, the polar and dispersive interactions between the organic solvents and several solid surfaces were determined by applying the new method [24,25] using the London interaction equation [26].
In this paper, we were interested in the determination of the surface thermodynamic properties of some porous polymers such as styrene–divinylbenzene copolymer modified by supramolecular structure of melamine using inverse gas chromatography (IGC) at infinite dilution [27,28,29,30,31,32,33,34,35,36,37,38,39,40] and our new methodology consisting in the correction of the London dispersive surface energy and the correct determination of polar free energy of adsorption of some polar organic probes as well as the Lewis’s acid-base parameters of the modified porous polymer.
2. Materials and Methods
2.1. Adsorbent and Materials
A porous polymer such as styrene–divinylbenzene copolymer (Dowex L-285, from Dow Chemical, Midland, USA), was used as the initial adsorbent for modification. Its specific surface area was 800 m2/g, with particle sizes of 250–500 μm. The chosen surface modifier was the melamine (from Vecton, St. Petersburg, Russia, 97%, CAS108-78-1), with percentage mass from 1% to 4% impregnated into the adsorbent surface by evaporation of aqueous solutions at 60 °C. The temperature choice was necessary to uniformly impregnate the modifier on the surface. Then the resulting sample was washed with high purity water to pH 7. The chemically pure organic solvents such as n-Hexane, n-heptane, n-octane, benzene, cyclohexane, toluene, ethanol, n-propanol, n-butanol, i-propanol, i-butanol, n-pentanol, i-pentanol, dichloromethane, and ethyl acetate (Chimreactivsnab, Russia) were used as the probes.
2.2. Inverse Gas Chromatography
The experimental determination of the retention time of organic molecules adsorbed on Dowex L-285 was carried out using inverse gas chromatography at infinite dilution with the help of a Chromos GC-1000 chromatograph (from Chromos, Russia) equipped with a flame ionization detector (FID). The solid particles were packed into stainless steel columns of 30 cm length and 3 mm internal diameter. The temperature of the column was 200°C and those of the injector and detector were 280°C. The flow rate of the nitrogen carrier gas was 30 mL/min. The mass of the sorbent packed into the column was equal to 1 g. All chromatographic columns were conditioned overnight at 200°C to remove any residual impurities. The probes in vapor phase were injected with a microsyringes at different temperatures to realize the infinite dilution and to satisfy the Henry’s law. The experiments were repeated three times and the error in the value of the retention volume did not exceed 2%.
2.3. Thermodynamic Methods
2.3.1. Dispersive and Polar Energies, and Lewis’s Acid-Base Parameters
The chromatographic measurements led to the determination of the net retention volume of the different probes adsorbed on the solid surfaces. This allowed determining the values of the free energy of adsorption of the adsorbed organic molecules as a function of temperature using the following equation:
Where is the absolute temperature, the perfect constant gas and a constant depending on the temperature and the interaction solvents-sorbent.
In the case of non-polar probes such as n-alkanes, is equivalent to the London dispersive energy of adsorption for all temperatures
When polar molecules are adsorbed on solid materials, is written as follows:
where is the free polar energy of the polar solvents.
To separate the two dispersive and polar contributions of the free energy of adsorption, one applied the new methodology recently published [24,25] that used the London dispersion interaction energy (Eq. 4)
Where is the Avogadro number, the dielectric constant of vacuum, and the respective deformation polarizabilities of the solid material denoted by S and the organic molecule denoted by X, separated by a distance , and and their corresponding ionization energies.
Previous equations led to Eq. 5:
The new chosen chromatographic interaction parameter was given by:
For non-polar molecules such as n-alkanes, the representation of of these molecules is given by:
where is the slope of the non-polar straight line.
Using the distance between the straight line of n-alkanes and the representative point of a polar molecule, it was possible to determine the free polar energy of the polar molecule, at any temperature:
The polar enthalpy and entropy of polar probes adsorbed on porous polymers can be deduced from relation 9 if the linearity of is assured.
2.3.1. London Dispersive Surface Energy, and Lewis’s Acid-Base Surface Energies
The London dispersive surface energy of the different sorbents was determined using the Fowkes relation [43] and applying the Hamieh thermal model that gave the surface area of organic molecules as a function of the temperature [19,20,21,22,23]:
where is a constant depending only on the temperature and the solid material.
The total surface energy of material is then given by
where represents the total polar (or acid-base) contribution of the surface energy.
Using Van Oss et al.’s method [44], it was possible to determine of the different polymers. This method consists in the determination of the Lewis acid and base surface energies of the solid particles. By choosing two monopolar solvents such as ethyl acetate (B) and dichloromethane (A), characterized by the following parameters:
and using the expression of polar free energy of the polar molecules, given by relation 15:
the Lewis acid and base surface energies of the solid surfaces were deduced from Eqs. 16:
The polar (or acid-base) surface energy and the total surface energy of different materials were then obtained using Eqs. 16:
3. Results
3.1. Variations of of Adsorbed Probes against the Temperature
The experimental determination of the net retention volume of organic molecules adsorbed on Dowex L-285 modified by different percentages of melamine as a function of temperature led to the variations on with the temperature for the various organic solvents (Table S1). The obtained results led drawing the curves of of the different organic molecules on Figure 1 for different temperatures as well as the variations of (--) of n-alkanes adsorbed solid materials as a function of temperature.
where
The curves presented in Figure 1 showed an important variation of of the various organic solvents as a function of temperature and the percentage of melamine on the porous polymer Dowex L-285. It seems that these variations were stabilized after 3% melamine percentage. Figure 1 (d) also showed a decrease in the values of (--) of n-alkanes for the different percentages of melamine.
However, the variations of of the different probes adsorbed on solid materials alone cannot describe the polar and dispersive free energies of adsorption. In the next sections, a new methodology was applied to better determine the London dispersive surface energy and the surface thermodynamic parameters of interaction of the examined porous polymers with the model organic molecules.
3.2. London Dispersive Surface Energy of Dowex L-285 with Different Melamine Percentages
The new expressions of the surface area of organic solvents were obtained using the Hamieh thermal model [19,20,21,22,23]. The slopes of straight-lines representing the variations of of n-alkanes adsorbed on Dowex L-285 with different percentages of melamine as a function of using the London dispersive surface energy of n-alkanes [19,20,21,22,23], gave the values of the London dispersive surface energy of the various porous polymers for the different temperatures. The variations of the London dispersive surface energy of materials when the temperature and the percentage of melamine varied were presented in Figure 2. A linear decrease of was observed for all materials when the temperature increased (Figure 2a). The highest values of were obtained for the copolymer Dowex L-285 without any addition of melamine. An important difference in the behavior of different materials was observed in Table 1 showing different values of of Dowex L-285 modified by melamine percentages, the London dispersive surface entropy , the extrapolated values of London dispersive surface energy at 0K and 298.15K, and the temperature maximum .
A minimum in the different values of , , , and was obtained for 2% of melamine proving a change in the surface groups of the copolymer due to the effect of this minimum percentage on the different surface parameters of Dowex L-285. Figure 2b highlighted the presence of such minimum of melamine. The obtained curves of of solid materials presented in Figure 2b at different temperatures clearly showed a minimum of corresponding to 2% of melamine.
The values of extrapolated London dispersive surface energy at room temperature are limited between 140 and 230 mJ/m2 for the different melamine percentages. In a recent paper [45], Guskov et al. obtained mJ/m2 using Dorris-Gray (DG) method and mJ/m2 applying the linear free energy relationship (LFER) coupled with the Dorris-Gray calculation (DG-LFER) for the same copolymer Dowex L-285 modified with 1% melamine, while our new methodology gave mJ/m2. The error committed in the case of DG method reached 28%, whereas, this error did not exceed 3% for DG-LFER method. The value obtained by our new method is very close to that obtained by Guskov et al. using DG-LFER calculation. However, the advantage of the new methodology using the Hamieh thermal model was to give the expressions of for the different percentages of melamine. For Dowex L-285 modified by 1% of melamine, one obtained the following relation:
The obtained values of of the styrene–divinylbenzene copolymer as a function of temperature were correlated to the thermal conductivity of this copolymer. Indeed, Yamamoto and Kambe [46] determined the thermal conductivity of this material by the following relation:
The results in Table 1 and Equation 19 led to the expression of the thermal conductivity as a function of :
Equation 20 clearly showed that the thermal conductivity of Dowex L-285 decreased when the London dispersive surface energy increased or when the temperature decreased. This study confirmed the previous results on the presence of a strong relation between the thermal conductivity of some graphene and carbon materials and its London dispersive surface energy obtained in a recent paper [47].
3.3. Polar Free Interaction Energy of Dowex L-285 Modified by Melamine with the Polar Probes
The free polar energy of adsorption of polar organic probes adsorbed on the different solid materials was determined against the temperature using our new approach, equations 5-8 and Table S1. The obtained results were presented in Table S2.
The curves of of the various polar organic probes adsorbed on Dowex L-285, drawn in Figure 3 as a function of temperature showed linear variations for the various melamine percentages. The obtained straight-lines of function of the temperature easily led to the polar enthalpy ( and entropy ( values of the adsorbed polar solvents.
Several conclusions can be deduced from the curves of Figure 3:
- -
-
It was observed that the free polar energies of interaction between the solids and the organic solvents can be globally classified in increasing order of interaction energy with the various polar probes at all temperatures as follows:cyclohexane < benzene < toluene < ethyl acetate < i-butanol < n-butanol < ethanol < n-propanol < dichloromethane < i-propanol.
- -
-
The highest free interaction energy was obtained for 4% melamine on Dowex L-285 for the following polar molecules in increasing order:cyclohexane < benzene < toluene < n-butanol < ethanol < n-propanol.
- -
- However, a maximum of free interaction energy was observed for 2% melamine on Dowex L-285 for the other polar solvents such as dichloromethane, i-propanol, and i-butanol.
- -
- It was proved that the alcohol molecules exchanged the maximum free interaction energy with the various solid surfaces, and especially, with 4% melamine, while the minimum interaction was observed in the case of adsorption on Dowex L-285.
- -
- The above results showed that the modification of the copolymer Dowex L-285 by melamine increased the polar free interaction.
The above results were confirmed by the curves of presented in Figure 4 as a function of the percentage of melamine on Dowex L-285 at different temperatures, where the maximum of the free interaction energy was shown for all polar solvents in Figure 4. Different non-linear variations of the free interaction energy were observed for the various polar molecules when the melamine percentage increased, but with a global increasing tendency of .
In order to better understand the polar behavior of the different solid surfaces, it was necessary to determine the surface polar enthalpy and entropy of adsorption, as well as the Lewis’s acid-base constants of the solid materials. This was developed in the next section.
3.4. Polar Enthalpy and Entropy of Adsorption, and Lewis Acid-Base Parameters of Dowex L-285 Modified by Melamine
The polar enthalpy ( and entropy ( of the polar solvents adsorbed on the copolymer Dowex L-285 modified by different melamine percentages, were deduced from the variations of using Table S2, Figure 3, and Equation 9. The curves in Figure 5 showing the approximated linear variations of and versus of the polar solvents adsorbed on the different solid materials led to the values of the Lewis enthalpic and and entropic and acid–base constants of Dowex L-285 with different melamine percentages. The results are presented in Table 2 which clearly showed an amphoteric behavior of the different solid surfaces with a Lewis’s basicity higher than their Lewis’s acidity for all solid substrates.
The values of the Lewis’s acid and base constants of different materials were obtained using Equations 10 and Figure 5. The slopes of straight-lines of and against determined the Lewis acid constant and , while the ordinates at origin led to the Lewis base constant and . The results in Table 2 showed that Dowex L-285 exhibited the highest acid character, whereas, the copolymer modified by 4% of melamine had the highest basicity. The results presented in Figure 6 showed that the basicity of solid materials largely increased when the melamine percentage increased, while a brutal decrease of the acidity was observed when Dowex L-285 was modified by 1% of melamine. A sleight increase of the acidity was found when the melamine percentage passed from 2% to 4%. The same conclusions were observed for the entropic acid-base constants. The total acid-base parameters SK = KA+KD and SK = ωA+ ωD. were given in Table 2. It was proved that the total acido-basicity increased when the melamine percentage increased. It seemed that a pallier was obtained for all acid-base parameters of solid surfaces after a 3% of melamine. In fact, the presence of six nitrogen atoms in the supramolecular structure of melamine increased its donor interaction of electrons when added to the structure of styrene–divinylbenzene copolymer. This increased the basicity of Dowex L-285 when the melamine percentage increased, while the Lewis’s acidity decreased because of the strong basic sites of melamine that certainly interacted with the acidic sites of the copolymer by decreasing the total acidity of the solid materials.
2.3. Polar Acid-Base Surface Energies of Dowex L-285 Modified by Melamine
The different polar surface energies such as the polar acid and base surface energies of Dowex L-285 modified by different melamine percentages were determined using the method of Van Oss et al. [44]. To do that, the free polar energy of the different polar given in Table 3 were used to determine the values of and at different temperatures, knowing the values of polar acid and base surface energies of two chosen polar solvents such as dichloromethane and ethyl acetate molecules.
Using Equations 14 and 16, the values of in Table 3, and the Hamieh thermal model, one obtained the values of and of the various solid surfaces as a function of temperature. The polar acid-base surface energy was then deduced from the relation . This led to the total surface energy of the different solid surfaces using relation .
The variations of , , , , and of Dowex L-285 with different percentages of melamine were given in Table S3. Whereas, Figure 7 gave the linear variations of polar surface energy components of Dowex L-285 modified by different melamine percentages as a function of temperature. The results showed that the highest polar surface energy values were obtained with the Lewis base surface energy for all materials, while the lowest values were highlighted with the Lewis acid surface energy, then confirming the highest basic character of Dowex L-285 at different melamine percentages. Furthermore, the maximum values of the Lewis base surface energy and the polar acid-base surface energy were obtained when using Dowex L-285 modified by 2% of melamine, followed by that of 4% of melamine.
By applying the Hamieh thermal model giving the values of the surface area of organic molecules adsorbed on the different solid surfaces as a function of temperature and the results giving in Table S3 and Figure 7, we gave in Figure 8 the variations of the polar surface energy of the adsorbed polar probes against the temperature using the Fowkes relation [43]. The highest values of the polar surface energy were obtained with dichloromethane and ethanol adsorbed in the various solid materials, whereas, the lowest values were obtained in the case of adsorption of cyclohexane and benzene. These results are in perfect agreement with the polarity of the above organic molecules.
2.4. Determination of the Average Separation Distance H
The separation distance between the organic molecules and the solid surfaces was determined as a function of the temperature, using Equations 7 and 8 and the previous experimental results. The obtained curves were plotted in Figure 9. It was observed that the separation distance increased when the temperature increased proving the effect of the thermal agitation on the separation distance between molecules and solid substrates. The results showed that the separation distance presented values comprised between 5.5 Å and 6.1 Å with the highest value in the case of 2% of melamine.
4. Conclusions
The surface thermodynamic properties of styrene–divinylbenzene copolymer (Dowex L-285) modified by a supramolecular structure of melamine at different percentages were characterized using the inverse gas chromatography at infinite dilution. The new methodology based on the Hamieh thermal model was used for accurate determination of the London dispersive surface energy of the different materials as a function of the temperature and the melamine percentage. A linear decrease of the London dispersive surface energy of materials was noticed when the temperature increased, with the highest value obtained for the copolymer Dowex L-285. The results showed an important difference in the behavior of different materials when the melamine percentage varied. This study highlighted a linear correlation between the thermal conductivity of the styrene–divinylbenzene copolymer and its London dispersive surface energy, confirming similar relations previously obtained with graphene and carbon materials.
The application of our new methodology using the London dispersion interaction on the styrene–divinylbenzene copolymer modified by melamine led to the determination of the polar free energy, enthalpy, and entropy of adsorption of polar solvents on the various solid surfaces as well the Lewis’s acid-base constants. It was proved that all used materials exhibited basic behavior largely higher than their acid behavior and the basic character increased with the melamine percentage in good agreement with the increase of nitrogen atom number at the surface of solid materials. The determination of the various components of polar acid and base surface energies of the different materials led to conclude that the highest polar surface energy values were obtained with the Lewis base surface energy for all materials, while the lowest values were obtained with the Lewis acid surface energy, confirming the highest basic character of Dowex L-285 at different melamine percentages. It was also showed that the maximum values of the Lewis base surface energy and the polar acid-base surface energy were obtained when using Dowex L-285 modified by 2% of melamine which showed the highest separation distance between surface materials and organic solvents.
Supplementary Materials
The following supporting information can be downloaded at the website of this paper posted on Preprints.org, Table S1. Values of (kJ/mol) of n-alkanes adsorbed on silica particles as a function of the temperature. Table S2. Variations of of different polar solvents adsorbed on Dowex L-285 and various melamine percentages as a function of temperature. Table S3. Values of , , , , and of Dowex L-285 and the different percentages of melamine on the copolymer.
Author Contributions
“Conceptualization, T.H. and V.G.; methodology, T.H.; software, T.H.; validation, T.H. and V.G.; formal analysis, T.H. and V.G.; investigation, T.H. and V.G.; resources, V.G.; data curation, V.G.; writing—original draft preparation, T.H.; writing—review and editing, T.H.; visualization, T.H. and V.G.; project administration, T.H. and V.G.; funding acquisition, V.G. All authors have read and agreed to the published version of the manuscript.”
Funding
This research received no external funding.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
The data presented in this study are available in the article and Supplementary Materials.
Conflicts of Interest
The authors declare no conflicts of interest.
References
- Gus´kov, V.Y.; Bilalova, R.V.; Kudasheva, F.K. Adsorption of organic molecules on a melamine-modified porous polymer. Russ Chem Bull, 2017, 66, 857–861. [CrossRef]
- Zaitsev, S.Yu.; Vereschetin, V.P.; Gromov, S.P.; Fedorova, O.A.; Alfimov, M.V.; Huesmann, H.; Möbius, D. Photosensitive supramolecular systems based on amphiphilic crown ethers, Supramolecular Science, 1997, 4 (3-4), 519-524, . [CrossRef]
- Sukhareva, D.A.; Gus´kov, V.Y.; Karpov, S.I.; Kudasheva, F. Kh. Adsorption of organic molecules on the highly ordered MCM-41 sorbent modified by different amounts of melamine. Russ Chem Bull 66, 958–962 (2017). [CrossRef]
- Gainullina, Y.Y.; Gus’kov, V.Y.; Timofeeva, D.V. Polarity of Thymine and 6-Methyluracil-Modified Porous Polymers, According to Data from Inverse Gas Chromatography. Russ. J. Phys. Chem. 93, 2477–2481 (2019). [CrossRef]
- Gus’kov, Vladimir Yu., Yulia F. Shaihitdinova, R. A. Zil’berg, Vladimir A Kraikin and Valery N. Maistrenko. “Intermolecular interactions between polyarylenephthalides surface and organic compounds of different nature by inverse gas chromatography data, 2019, 19 (2), 229-236. Corpus ID: 145851861, . [CrossRef]
- Gus’kov, V.Y., Ivanov, S.P., Khabibullina, R.A. et al. Gas chromatographic investigation of the properties of a styrene-divinylbenzene copolymer modified by 5-hydroxy-6-methyluracil. Russ. J. Phys. Chem. 86, 475–478 (2012). [CrossRef]
- J.-M. Lehn, Supramolecular Chemistry. Concepts and Perspectives, VCH, Weinhiem, 1995, 271 pp., . [CrossRef]
- Guo, Fang, Eugene Y. Cheung, Kenneth D. M. Harris and Venkateswara Rao Pedireddi. “Contrasting Solid-State Structures of Trithiocyanuric Acid and Cyanuric Acid.” Crystal Growth & Design, 2006, 6, 846-848. [CrossRef]
- U. P. Singh, S. Kashyap, H. J. Singh, B. K. Mishra, P. Roy, A. Chakraborty, Effect of adenosine on the supramolecular architecture and activity of 5-fluorouracil, J. Mol. Struct., 2012, 1014, 47-56, . [CrossRef]
- Gus'kov, V. Y., Gainullina, Y. Y., & Kudasheva, F. K. Razdelenie enantiomerov mentola, kamfena i kamfory na 5-gidroksi-6-metiluratsile v usloviyakh gazovoi khromatografii. Analitika i kontrol, 2014, 18(2), 178-181.
- Breckenridge, W. H.; Ayles, V. L.; Wright, T. G. Evidence for Emergent Chemical Bonding in Au+−Rg Complexes (Rg = Ne, Ar, Kr, and Xe), The Journal of Physical Chemistry A, 2008, 112 (18), 4209-4214, . [CrossRef]
- Gus’kov, V. Y.; Ivanov, S. P.; Khabibullina, R. A.; Garafutdinov, R. R.; Kudasheva, F. K. Gas chromatographic investigation of the properties of a styrene-divinylbenzene copolymer modified by 5-hydroxy-6-methyluracil, Russian Journal of Physical Chemistry A, 2012, 86, 475-478.
- Gus’kov, V. Y.; Gainullina, Y. Y.; Ivanov, S. P.; Kudasheva, F. K. Properties of the surface of a porous polymer modified with 5-fluorouracil, according to data of gas chromatography. Russian Journal of Physical Chemistry A, 2014, 88, 1042-1046.
- Gus’kov, V. Y.; Gainullina, Y. Y.; Ivanov, S. P.; Kudasheva, F. K. Porous polymer adsorbents modified with uracil. Prot. Met. Phys. Chem. Surf., 2014, 50, 55-58.
- Gus’kov, V. Y.; Ivanov, S. P.; Kudasheva, F. K. Sorbts. Khromat. Protsessy [Sorption and Chromatographic Processes], 2012, 12, 916 (in Russian).
- Godlewski, S.; Tekiel, A.; Piskorz, W. ; Zasada, F.; Prauzner-Bechcicki, J. S.; Sojka, Z.; Szymonski, M. Supramolecular ordering of PTCDA molecules: The key role of dispersion forces in an unusual transition from physisorbed into chemisorbed state. ACS Nano. 2012, 6 (10), 8536-8545. [CrossRef]
- Dorris, G.M.; Gray, D.G. Adsorption of n-alkanes at zero surface coverage on cellulose paper and wood fibers. J. Colloid Interface Sci. 1980, 77, 353–362.
- Schultz, J.; Lavielle, L.; C. Martin, C. Propriétés de surface des fibres de carbone déterminées par chromatographie gazeuse inverse, J. Chim. Phys., 1987, 84 (2), 231-237.
- Hamieh, T. Study of the temperature effect on the surface area of model organic molecules, the dispersive surface energy and the surface properties of solids by inverse gas chromatography. J. Chromatogr. A 2020, 1627, 461372.
- Hamieh, T.; Ahmad, A.A.; Roques-Carmes, T.; Toufaily, J. New approach to determine the surface and interface thermodynamic properties of H-β-zeolite/rhodium catalysts by inverse gas chromatography at infinite dilution. Sci. Rep. 2020, 10, 20894.
- Hamieh, T. New methodology to study the dispersive component of the surface energy and acid–base properties of silica particles by inverse gas chromatography at infinite dilution. J. Chromatogr. Sci. 2022, 60, 126–142. [CrossRef]
- Hamieh, T. Inverse Gas Chromatography to Characterize the Surface Properties of Solid Materials. Chem. Mater. 2024, 36, 5, 2231–2244. [CrossRef]
- Hamieh, T. Some Irregularities in the Evaluation of Surface Parameters of Solid Materials by Inverse Gas Chromatography. Langmuir 2023, 39, 17059–17070. [CrossRef]
- Hamieh, T. New Progress on London Dispersive Energy, Polar Surface Interactions, and Lewis’s Acid–Base Properties of Solid Surfaces. Molecules 2024, 29, 949. [CrossRef]
- Hamieh, T. London Dispersive and Lewis Acid-Base Surface Energy of 2D Single-Crystalline and Polycrystalline Covalent Organic Frameworks. Crystals 2024, 14, 148. [CrossRef]
- London, F. The general theory of molecular forces. Trans. Faraday Soc. 1937, 33, 8–26. [CrossRef]
- Conder, J. R.; Young, C. L. Physical measurements by gas chromatography. Eds: John Wiley & Sons, Chichester, New York, 1979, 632 pages.
- Sawyer, D.T.; Brookman, D.J. Thermodynamically based gas chromatographic retention index for organic molecules using salt-modified aluminas and porous silica beads, Anal. Chem. 1968, 40, 1847-1850. [CrossRef]
- Dorris, G.M.; Gray, D.G. Adsorption of n-alkanes at zero surface coverage on cellulose paper and wood fibers, J. Colloid Interface Sci. 1980, 77, 353-362.
- Fowkes, F.M. in: Surface and interfacial aspects of biomedical polymers, Vol. I, pp. 337-372, Ed: J. D. Andrade, Plenum Press, New York, 1985.
- Schultz, J.; Lavielle, L.; Martin, C. The role of the interface in carbon fibre-epoxy composites. J. Adhes. 1987, 23, 45-60.
- Saint-Flour, C.; Papirer, E. Gas-solid chromatography. A method of measuring surface free energy characteristics of short glass fibers. 1. Through adsorption isotherms, Ind. Eng. Chem. Prod. Res. Dev. 1982, 21, 337-341, . [CrossRef]
- Saint-Flour, C.; Papirer, E. Gas-solid chromatography: method of measuring surface free energy characteristics of short fibers. 2. Through retention volumes measured near zero surface coverage, Ind. Eng. Chem. Prod. Res. Dev. 1982, 21, 666-669, . [CrossRef]
- Donnet, J.-B.; Park, S.; Balard, H. Evaluation of specific interactions of solid surfaces by inverse gas chromatography, Chromatographia. 31 (1991) 434–440.
- Chehimi, M.M.; Pigois-Landureau, E. Determination of acid–base properties of solid materials by inverse gas chromatography at infinite dilution. A novel empirical method based on the dispersive contribution to the heat of vaporization of probes, J. Mater. Chem. 4 (1994) 741–745.
- Brendlé, E.; Papirer, E. A new topological index for molecular probes used in inverse gas chromatography for the surface nanorugosity evaluation, 1. Method of Evaluation, J. Colloid Interface Sci. 194 (1997) 207–216.
- Brendlé, E.; Papirer, E. A new topological index for molecular probes used in inverse gas chromatography for the surface nanorugosity evaluation, 2. Application for the Evaluation of the Solid Surface Specific Interaction Potential, J. Colloid Interface Sci. 194 (1997) 217–2224.
- Przybyszewska, M.; Krzywania, A.; Zaborski, M.; Szynkowska, M.I. Surface properties of zinc oxide nanoparticles studied by inverse gas chromatography, J. Chromatogr. A, 2009, 1216, 5284-5291.
- Bakaoukas, N.; Sevastos, D.; Kapolos, J.; Koliadima, A.; Karaiskakis, G. Characterization of polymeric coatings in terms of their ability to protect marbles and clays against corrosion from sulfur dioxide by inverse gas chromatography, Int. J. Polym. Anal. Charact., 2013, 18, 401-4013.
- Rückriem, M.; Inayat, A.; Enke, D.; Gläser, R.; Einicke, W-D.; Rockmann, R. Inverse gas chromatography for determining the dispersive surface energy of porous silica. Colloids Surf A Physicochem. Eng. Asp. 2010, 357, 21–26.
- Gutmann, V. The Donor-acceptor Approach to Molecular Interactions, Plenum. Publisher: Springer New York, NY, 1978, 279 pages.
- Riddle, F.L.; Fowkes, F.M. Spectral shifts in acid-base chemistry. Van der Waals contributions to acceptor numbers, Spectral shifts in acid-base chemistry. 1. van der Waals contributions to acceptor numbers. J. Am. Chem. Soc. 1990, 112 (9), 3259-3264, . [CrossRef]
- Fowkes, F.M. in: Surface and interfacial aspects of biomedical polymers, Vol. I, pp. 337-372, Ed: J. D. Andrade, Plenum Press, New York, 1985.
- Van Oss, C.J.; Good, R.J.; Chaudhury, M.K. Additive and nonadditive surface tension components and the interpretation of contact angles. Langmuir 1988, 4, 884. [CrossRef]
- Gus’kov, V. Y., Gainullina, Y. Y.; Gabdulmanova, A. F.; Gareeva. A. N. Dispersive surface free energy of adsorbents modified by supramolecular structures of heterocyclic compounds.” Chimica Techno Acta (2024), Guskov, Corpus ID: 269795422, . [CrossRef]
- Yamamoto, O; Kambe, H. Thermal conductivity of cross-linked polymers. A comparison between measured and calculated thermal conductivities, Polymer Journal, 1971, 2 (5), 623-628.
- Hamieh, T. Thermal Surface Properties, London Dispersive and Polar Surface Energy of Graphene and Carbon Materials Using Inverse Gas Chromatography at Infinite Dilution. Molecules, 29(12), 2871. [CrossRef]
Figure 1.
Effect of the percentage of melamine on the values of of different adsorbed organic solvents at various temperatures: T = 453.15K (a), T = 463.15K (b), T = 473.15K (c), and variations of (--) of n-alkanes adsorbed on solid surfaces as a function of temperature (d).
Figure 1.
Effect of the percentage of melamine on the values of of different adsorbed organic solvents at various temperatures: T = 453.15K (a), T = 463.15K (b), T = 473.15K (c), and variations of (--) of n-alkanes adsorbed on solid surfaces as a function of temperature (d).
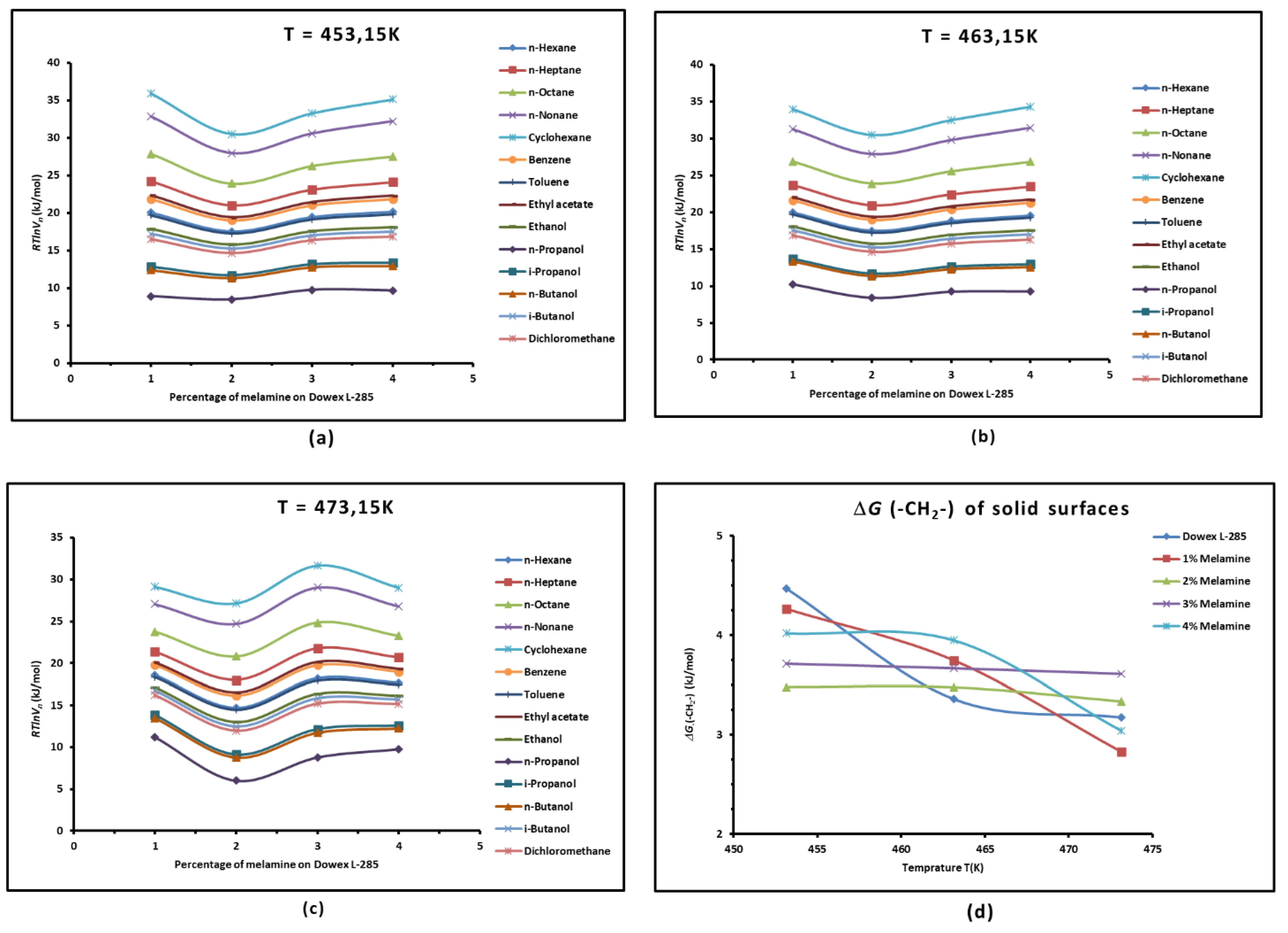
Figure 2.
Variations of the London dispersive surface energy of materials as a function of the temperature (a) and the percentage of melamine (b).
Figure 2.
Variations of the London dispersive surface energy of materials as a function of the temperature (a) and the percentage of melamine (b).

Figure 3.
Evolution of the free polar energy of the various polar organic probes adsorbed on the different solid materials as a function of temperature for different melamine percentages. (a): dichloromethane, (b): cyclohexane, (c): benzene, (d): toluene, (e): ethyl acetate, (f): ethanol, (g): n-propanol, (h): i-propanol, (i): n-butanol, and (j): i-butanol.
Figure 3.
Evolution of the free polar energy of the various polar organic probes adsorbed on the different solid materials as a function of temperature for different melamine percentages. (a): dichloromethane, (b): cyclohexane, (c): benzene, (d): toluene, (e): ethyl acetate, (f): ethanol, (g): n-propanol, (h): i-propanol, (i): n-butanol, and (j): i-butanol.


Figure 4.
Variations of the free polar energy of polar organic probes adsorbed on the different solid materials as a function of melamine percentage on Dowex L-285 at various temperatures. (a): dichloromethane, (b): cyclohexane, (c): benzene, (d): toluene, (e): ethyl acetate, (f): ethanol, (g): n-propanol, (h): i-propanol, (i): n-butanol, and (j): i-butanol.
Figure 4.
Variations of the free polar energy of polar organic probes adsorbed on the different solid materials as a function of melamine percentage on Dowex L-285 at various temperatures. (a): dichloromethane, (b): cyclohexane, (c): benzene, (d): toluene, (e): ethyl acetate, (f): ethanol, (g): n-propanol, (h): i-propanol, (i): n-butanol, and (j): i-butanol.


Figure 5.
Variations of and against of polar solvents adsorbed on Dowex L-285 modified by different percentages of melamine.
Figure 5.
Variations of and against of polar solvents adsorbed on Dowex L-285 modified by different percentages of melamine.

Figure 9.
Evolution of the separation distance (in Å) between the organic solvents and Dowex L-285 modified by melamine supramolecule as a function of the temperature.
Figure 9.
Evolution of the separation distance (in Å) between the organic solvents and Dowex L-285 modified by melamine supramolecule as a function of the temperature.
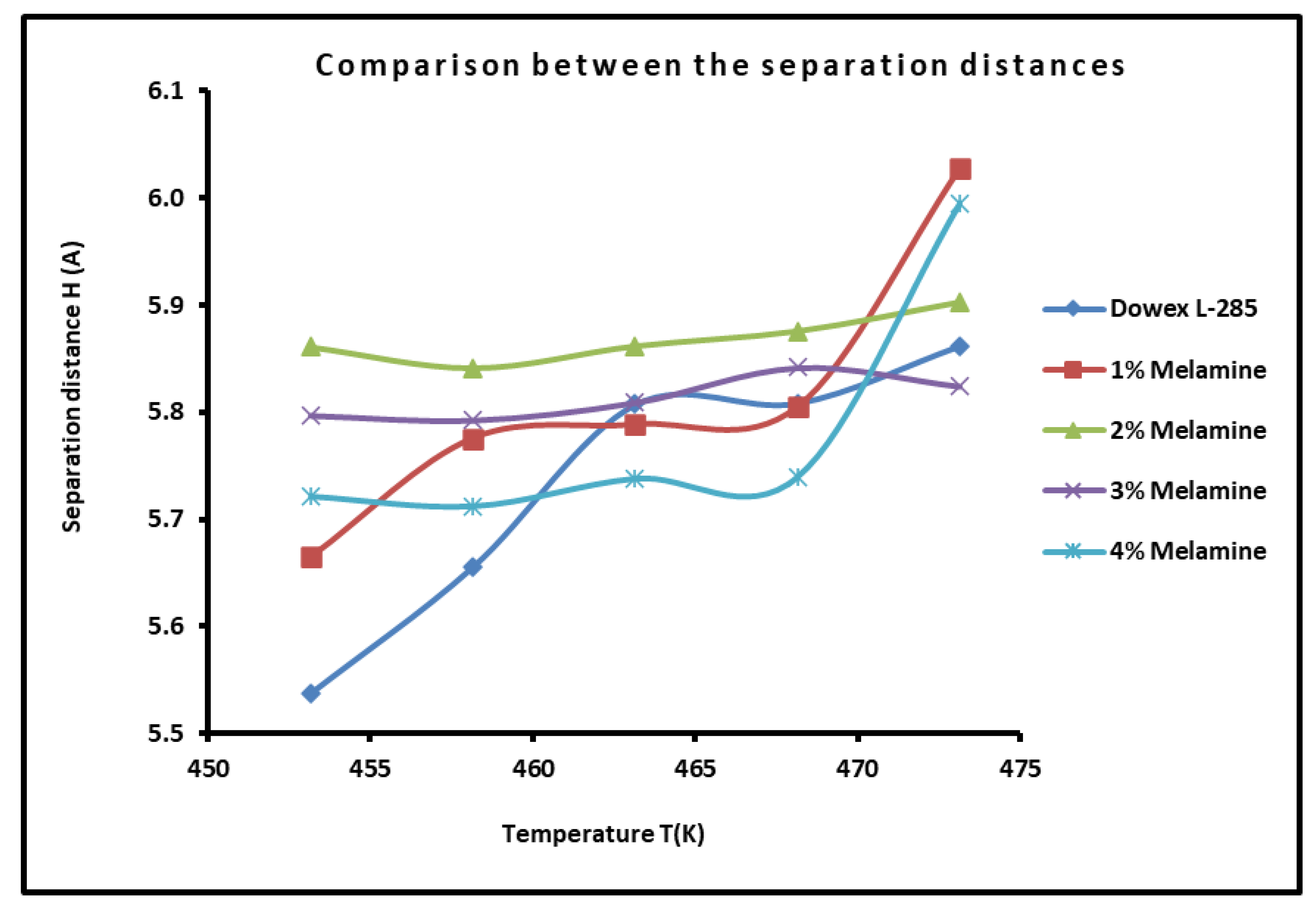
Figure 6.
Curves of the Lewis’s enthalpic and entropic acid-base constants , , , , the acid-base ratios, and the corresponding parameters SK = KA+KD and SK = ωA+ ωD. as a function of melamine percentage.
Figure 6.
Curves of the Lewis’s enthalpic and entropic acid-base constants , , , , the acid-base ratios, and the corresponding parameters SK = KA+KD and SK = ωA+ ωD. as a function of melamine percentage.

Figure 7.
Variations of acid and base surface energy components, and total energy () of Dowex L-285 modified by different melamine percentages as a function of temperature: Dowex L-285 (a), 1% Melamine (b), 2% Melamine (c), 3% Melamine (d), 4% Melamine (e), base surface energy (f), acid surface energy (g), acid-base surface energy (h), total surface energy (i).
Figure 7.
Variations of acid and base surface energy components, and total energy () of Dowex L-285 modified by different melamine percentages as a function of temperature: Dowex L-285 (a), 1% Melamine (b), 2% Melamine (c), 3% Melamine (d), 4% Melamine (e), base surface energy (f), acid surface energy (g), acid-base surface energy (h), total surface energy (i).


Figure 8.
Variations of polar surface energy () of the different polar solvents adsorbed on Dowex L-285 modified by different melamine percentages as a function of temperature: Dowex L-285 (a), 1% Melamine (b), 2% Melamine (c), 3% Melamine (d), 4% Melamine (e).
Figure 8.
Variations of polar surface energy () of the different polar solvents adsorbed on Dowex L-285 modified by different melamine percentages as a function of temperature: Dowex L-285 (a), 1% Melamine (b), 2% Melamine (c), 3% Melamine (d), 4% Melamine (e).

Table 1.
Linear expressions of of Dowex L-285 modified by different percentages of melamine, regression coefficients, London dispersive surface entropy , extrapolated values of London dispersive surface energy at 0K and 298.15K, and the temperature maximum
Table 1.
Linear expressions of of Dowex L-285 modified by different percentages of melamine, regression coefficients, London dispersive surface entropy , extrapolated values of London dispersive surface energy at 0K and 298.15K, and the temperature maximum
| Solid material |
(mJ/m2) |
R² |
(mJ m−2 K−1) |
(mJ/m2) |
(mJ/m2) |
(K) |
|---|---|---|---|---|---|---|
| Dowex L-285 | = -0.835T + 482.43 | 0.9980 | -0.835 | 482.43 | 233.47 | 577.8 |
| 1% Melamine | = -0.907T + 496.21 | 0.9972 | -0.907 | 496.21 | 225.79 | 547.1 |
| 2% Melamine | = -0.656T + 341.59 | 0.9590 | -0.656 | 341.59 | 146.12 | 521.0 |
| 3% Melamine | = -0.827T + 439.96 | 0.9608 | -0.827 | 439.96 | 193.51 | 532.3 |
| 4% Melamine | = -0.876T + 473.64 | 0.9735 | -0.876 | 473.64 | 212.40 | 540.6 |
Table 2.
Values of the Lewis’s acid-base constants , , , , the acid-base ratios, and the linear regression coefficient R2 relative to Dowex L-285 modified by different melamine percentages with the corresponding parameters SK = KA+KD and SK = ωA+ ωD.
Table 2.
Values of the Lewis’s acid-base constants , , , , the acid-base ratios, and the linear regression coefficient R2 relative to Dowex L-285 modified by different melamine percentages with the corresponding parameters SK = KA+KD and SK = ωA+ ωD.
| Material | KA | KD | KD/KA | KA+KD | R2 | 10−3ωA | 10−3ωD | ωD/ωA | 10−3 (ωA+ ωD) | R2 |
|---|---|---|---|---|---|---|---|---|---|---|
| Dowex L-285 | 0.550 | 0.844 | 1.53 | 1.393 | 0.999 | 1.05 | 0.55 | 0.5 | 1.59 | 0.9968 |
| 1% Melamine on Dowex L-285 | 0.164 | 1.455 | 8.85 | 1.620 | 0.9783 | 0.09 | 2.81 | 30.2 | 2.90 | 0.9316 |
| 2% Melamine on Dowex L-285 | 0.160 | 2.137 | 13.38 | 2.297 | 0.9989 | 0.22 | 4.00 | 18.5 | 4.21 | 0.9520 |
| 3% Melamine on Dowex L-285 | 0.193 | 3.263 | 16.90 | 3.456 | 0.9938 | 0.22 | 6.30 | 28.0 | 6.52 | 0.9695 |
| 4% Melamine on Dowex L-285 | 0.218 | 3.401 | 15.58 | 3.619 | 0.9276 | 0.34 | 5.00 | 14.8 | 5.34 | 0.9702 |
Table 3.
Values of for dichloromethane and ethyl acetate adsorbed on Dowex L-285 modified with different melamine percentages at different temperatures.
Table 3.
Values of for dichloromethane and ethyl acetate adsorbed on Dowex L-285 modified with different melamine percentages at different temperatures.
| Dichloromethane | |||||
| T(K) | Dowex L-285 | 1% Melamine | 2% Melamine | 3% Melamine | 4% Melamine |
| 453.15 | 15.274 | 15.609 | 24.349 | 9.737 | 16.294 |
| 458.15 | 15.239 | 15.559 | 24.294 | 9.352 | 16.452 |
| 463.15 | 15.204 | 15.509 | 24.239 | 8.967 | 16.012 |
| 468.15 | 15.169 | 15.459 | 24.184 | 8.582 | 15.989 |
| 473.15 | 15.134 | 15.409 | 24.129 | 8.197 | 12.318 |
| Ethyl acetate | |||||
| T(K) | Dowex L-285 | 1% Melamine | 2% Melamine | 3% Melamine | 4% Melamine |
| 453.15 | 10.106 | 8.503 | 9.667 | 10.567 | 39.887 |
| 458.15 | 9.593 | 8.378 | 9.507 | 10.314 | 38.842 |
| 463.15 | 9.079 | 8.253 | 9.347 | 10.060 | 37.077 |
| 468.15 | 8.566 | 8.128 | 9.187 | 9.807 | 35.814 |
| 473.15 | 8.052 | 8.003 | 9.027 | 9.553 | 30.163 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated







