Preprint
Article
p-Numerical Semigroups of Triples from the Three-Term Recurrence Relations
Altmetrics
Downloads
82
Views
24
Comments
0
A peer-reviewed article of this preprint also exists.
This version is not peer-reviewed
Submitted:
23 July 2024
Posted:
24 July 2024
You are already at the latest version
Alerts
Abstract
Many people, including Horadam, have studied the numbers $W_n$, satisfying the recurrence relation $W_n=u W_{n-1}+v W_{n-2}$ ($n\ge 2$) with $W_0=0$ and $W_1=1$. In this paper, we study the $p$-numerical semigroups of the triple $(W_i,W_{i+2},W_{i+k})$ for integers $i,k(\ge 3)$.
For a nonnegative integer $p$, the $p$-numerical semigroup $S_p$ is defined as the set of integers whose nonnegative integral linear combinations of given positive integers $a_1,a_2,\dots,a_\kappa$ with $\gcd(a_1,a_2,\dots,a_\kappa)=1$ are expressed in more than $p$ ways. When $p=0$, $S=S_0$ is the original numerical semigroup. The largest element and the cardinality of $\mathbb N_0\backslash S_p$ are called the $p$-Frobenius number and the $p$-genus, respectively.
Keywords:
Subject: Computer Science and Mathematics - Mathematics
MSC: 11D07; 20M14; 05A17; 05A19; 11D04; 11B68; 11P81
1. Introduction
We consider the sequence , satisfying
where u and v are positive integers with . The values of depend on the values of u and v. If , is the n-th Fibonacci number [1]. If and , is the n-th Jacobsthal number [2,3]. If and , is the n-th Pell number [4]. However, for simplicity, if we do not specify the values of u or v, we will simply write for .
This type of number sequence has been well known to many people by Horadam’s series of studies ([5,6,7,8,9]) in the 1960s. Because of this fact, this sequence is sometimes called the Horadam sequence. Horadam himself used the recurrence relation . But recently more people (see, e.g., [10,11]) have used the recurrence relation and such works are still due to Horadam. In general, the initial values are arbitrary, but because of some simplifications, we set and . According to [6], this sequence has long exercised interest, as seen in, for instance, Bessel-Hagen [12], Lucas [13], and Tagiuri [14], and, for historical details, Dickson [15]. However, it is deplorable that quite a few papers are publishing results that have already been obtained by these authors as new results, either because they are unaware of their or the following important results, or even if they are ignoring them.
Given the set of positive integers (), for a nonnegative integer p, let be the set of integers whose nonnegative integral linear combinations of given positive integers are expressed in more than p ways. For a set of nonnegative integers , the set is finite if and only if . Then there exists the largest integer in , which is called the p-Frobenius number. The cardinality of is called the p-genus and is denoted by . The sum of the elements in is called the p-Sylvester sum and is denoted by . This kind of concept is a generalization of the famous Diophantine problem of Frobenius since is the case when the original Frobenius number , the genus and the Sylvester sum are recovered. We can call the p-numerical semigroup. Strictly speaking, when , does not include 0 since the integer 0 has only one representation, so it satisfies simply additivity, and the set becomes a numerical semigroup. For numerical semigroups, we refer to [16,17,18]. For the p-numerical semigroup, we refer to [19].
We are interested in finding any closed or explicit form of the p-Frobenius number, which is even more difficult when . For three or more variables, no concrete example had been found. Most recently, we have finally succeeded in giving the p-Frobenius number as closed-form expressions for the triangular number triplet ([20]), for repunits ([21,22]).
2. Preliminaries
We introduce the Apéry set (see [23]) below in order to obtain the formulas for , , and technically. Without loss of generality, we assume that .
Definition 1.
Let p be a nonnegative integer. For a set of positive integers with and we denote by
the p-Apéry set of A, where each positive integer satisfies the conditions:
Note that is defined to be 0.
It follows that for each p,
Even though it is hard to find any explicit form of as well as and , by using convenient formulas established in [24,25], we can obtain such values for some special sequences after finding any regular structure of . One convenient formula is on the power sum
by using Bernoulli numbers defined by the generating function
and another convenient formula is on the weighted power sum ([26,27])
by using Eulerian numbers appearing in the generating function
with and . Here, is a nonnegative integer and . From these convenient formulas, many useful expressions are yielded as special cases. Some useful ones are given as follows. The formulas (3) and (4) are entailed from and , respectively.
Lemma 1.
Let κ, p and μ be integers with and . Assume that . We have
3. Main Results
We use the following properties repeatedly. The proof is trivial and omitted.
Lemma 2.
For , we have
First of all, if i is odd and then by (1) and (7),
Hence, . Therefore, from now on, we consider the case only when i is even and k is odd, or when i is odd, with .
3.1. The Case k Is Odd
When k is odd, we choose non-negative integers and as
where if due to (5), otherwise is the largest integer, satisfying
More directly, when i is even (and k is odd),
When i is odd (and k is odd),
Note that if ([2]), then always .
In particular, if i is even and
If , then by (5) . So, when i is even, by (8) (8). Thus, we get
When and i is odd, by and , there exists an integer h such that . By , . Thus,
Thus, we get
We use the following identity.
Lemma 3.
For , we have
Assume that (The case is discussed later). Then the elements of the (0-)Apéry set are given in Table 1. Here, we consider the expression
or simply the position .
We shall show that all the elements in Table 1 constitute the sequence in the vertical y direction. However, if i is odd and i is even, the situation of this sequence is different. In short, if i is odd, the sequence appears continuously, but if i is even, the sequence is divided into u subsequences.
First, let i be odd. Then by , we have
By (7), we get
Hence,
Thus, the element at () cannot be an element of but as the same residue modulo , where . Next, by Lemma 3, we have
Thus, the element at () cannot be an element of but .
Therefore, the sequence is divided into the longer parts with length and the shorter parts with length : Namely, the longer part is of the subsequence
with the next element at . The shorter part is of the subsequence
with the next element at . Since , all elements in are different modulo .
Next, let i be even. Then by , we have
Hence,
with (). By the determination of in (11), we see that . So, by using the relations (14). Thus, each subsequence is given as the following points. For
with next element is at , coming back to the first one, because of Lemma 3. In addition, by (8), all terms of the above subsequence are
Since , this is equivalent to (). Therefore, there is no overlapped element among all subsequences. By (9), the total number of terms in each subsequence is
as expected.
By Table 1, the candidates of the largest element of are at or at . Since is equivalent to , by Lemma 1 (2), if , then
If , then
3.1.1. The Case k Is Odd with
When k is odd and , we get and . Hence, the elements of the (0-)Apéry set are given in Table 2.
Similarly to the case , when i is odd, so , the sequence simply becomes one sequence by combining all the subsequences with length and with length . When i is even, so , the sequence consists of u subsequences with the same length .
By Table 2, the largest element of is at . Hence,
In fact, this is included in the case where and .
3.2. The Case k Is Even
When k is even (so i is odd), we choose non-negative integers q and r as
where . Note that is an integer for even k.
Note that because otherwise i is also even. Then the elements of the (0-)Apéry set are given in Table 3.
Similarly to the case where k is odd in (14), we have
Thus, the element at () cannot be an element of but as the same residue modulo . The sequence is divided into the longer parts with length and one shorter part with length r: Namely, the longer part is of the subsequence
with the next element at . One shorter part is of the subsequence
with the next element at . Notice that similarly to Lemma 3, we have
Since , all elements in are different modulo . Then by , we have
By Table 3, the candidates of the largest element of are at or at . Since is equivalent to , by Lemma 1 (2), if , then
If , then
Notice that may occur in some cases. For example, . In this case, both of the two formulas are valid, yielding the Frobenius number .
4. The Case where
It is important to see that the elements of are determined from those of .
4.1. When k Is Odd
4.1.1. When
The corresponding relations from to are as follows. See Table 4.
[The first u rows]
by Lemma 3 and
respectively. Note that when , the second corresponding relation does not exist. This also implies that all the elements at and can be expressed in terms of in at least two ways.
[Others]
by the identity (13). This also implies that all the elements at can be expressed in at least two ways.
By Table 4, there are four candidates to take the largest value of . Namely, the values at
If , one of the elements at and at is the largest. In this case, if , then
If , then
If , one of the elements at and at is the largest. In this case, if , then
If , then
Examples.
When , the first identity is applied:
Indeed, there are two representations in terms of as
which is the largest element of . In fact, the second, the third and the fourth identities yield the smaller values
respectively.
When , the second identity is applied:
In fact, the first, the third and the fourth identities yield the smaller values
respectively.
When , the third identity is applied:
In fact, the first, the second and the fourth identities yield the smaller values
respectively.
When , the fourth identity is applied:
In fact, the first, the second and the third identities yield the smaller values
respectively.
4.1.2. When
The similar corresponding relations to the case are also applied for . When , the elements of the first u rows of the main area (the second block from the left) correspond to fill the gap below the left-most block:
The other elements of the main area correspond to those in the block immediately to the right to go up the u row:
The elements of the stair areas correspond to those in the block immediately to the right in the form as it is to go up the row:
See Table 5. We can also show that all these elements have at least three distinct representations in terms of .
From Table 5, there are six candidates to take the largest element of . These elements are indicated as follows.
 |
In conclusion, if and , then
If and , then
If and , then
If and , then
In general, for an integer , it is sufficient to compare two elements at both ends. See Table 6. If and , then
If and , then
If and , then
If and , then
The positions of the elements of below the left-most block and the positions of in the right-most block are arranged as shown in Table 6.
This situation is continued as long as . However, when , the shape of the block on the right side collapses. Thus, the regularity of taking the maximum value of is broken. Hence, the fourth case holds until and other cases hold for .
Theorem 1.
When , the first identity is applied. Since and , for we have
Namely, the corresponding element for each integer is at (). However, for , the p-Frobenius numbers can be computed neither by the above formula nor by any other closed formulas. Namely, the real value is , corresponding to , though the formula gives 1274931, corresponding to .
4.2. When k Is Even
4.2.1. When
Similarly to the odd case where k is odd, the elements of can be determined from those of . When , there are corresponding relations as follows.
[The first row ]
with
due to (15). Note that when the second corresponding relation does not exist. This also implies that all the elements at and can be expressed in terms of in at least two ways.
[Others]
by the identity (13). This also implies that all the elements at can be expressed in at least two ways.
By Table 7, there are four candidates to take the largest value of . Namely, the values at
If , one of the elements at and at is the largest. In this case, if , then
If , then
If , one of the elements at and at is the largest. In this case, if , then
If , then
4.2.2. When
The situation is similar for . From Table 8, there are six candidates to take the largest element of . These elements are indicated as follows.
 |
 |
In conclusion, if and , then
If and , then
If and , then
If and , then
In general, for an integer , it is sufficient to compare two elements at both ends. See Table 9. If and , then
If and , then
If and , then
If and , then
The positions of the elements of below the left-most block and the positions of in the right-most block are arranged as shown in Table 6.
This situation is continued as long as . However, when , the shape of the block on the right side collapses. Namely, we cannot take the value at . Thus, the regularity of taking the maximum value of is broken. Hence, the fourth case holds until , and other cases hold for .
Theorem 2.
Let i be an integer and k be even with . Let q and r be determined as (15). For , if and , then
If and , then
If and , then
If and , then for
Example
When , we have and . So, the elements of , where , are given as in Table 10. The largest element is at , which comes from the second identity. Thus,
Notice that the right-most element is at and the block of the right side is empty. Therefore, the formula does not hold for . In fact, , corresponding to , though the formula gives 63914, corresponding to .
5. p-Genus
5.1. The Case Where k Is Odd
Let k be odd. For a non-negative integer p, the areas of the p-Apéry set can be divided into three parts: the stairs part (left), the stairs part (right) and the main part. By referring to Table 6 (with Table 4 and Table 5), we can compute
Here, we used the relation (9) to simplify the expression. In addition, by , we have
By Lemma 1 (3), we have
Since the z value of the right-most side must be non-negative, . Namely, the above formula is valid for .
Example
When , by
for we have for
However, for , the p-genus cannot be obtained by the above formula. The real values are given by
though the formula gives
5.2. The Case Where k Is Even
Similarly to the case for k is odd, when k is even, by referring to Table 9 (with Table 7 and Table 8), we can compute
Here, we used the relation (15) to simplify the expression. In addition,
are all positive integers. By Lemma 1 (3), we have
Theorem 3.
Example
Let . So, . Then for by the formula we have
However, contrary to the fact that , the formula gives 50746.
6. Final Comments
The original numbers studied by Horadam satisfy the recurrence relation . From this point of view, almost all the above identities hold by replacing v by , though the condition is necessary. For example, the identities of (7) and (8) are replaced by
respectively. For example, when , by for by the first identity of Theorem 1, we have
When , by for by the first identity of Theorem 2, we have
Horadam also studied the number with arbitrary initial values and . However, with arbitrary initial values many identities (e.g., (7)) do not hold as they are. Hence, the situation becomes too complicated.
Author Contributions
writing—original draft preparation, T.K.;writing—review and editing, J.M.; All authors have read and agreed to the published version of the manuscript.
Funding
This research received no external funding.
Data Availability Statement
Data are contained within the article.
Conflicts of Interest
The author declares no conflict of interest.
| 1 | There was a typo, but it was corrected in [31]. |
References
- Komatsu, T.; Ying, H. The p-Frobenius and p-Sylvester numbers for Fibonacci and Lucas triplets. Math. Biosci. Eng. 2023, 20, 3455–3481. [Google Scholar] [CrossRef] [PubMed]
- Komatsu, T.; Pita-Ruiz, C. The Frobenius number for Jacobsthal triples associated with number of solutions. Axioms 2023, 12, 98. [Google Scholar] [CrossRef]
- Komatsu, T.; Laishram, S.; Punyani, P. p-numerical semigroups of generalized Fibonacci triples. Symmetry 2023, 15, 852. [Google Scholar] [CrossRef]
- Komatsu, T.; Mu, J. p-numerical semigroups of Pell triples. J. Ramanujan Math. Soc.
- Horadam, A.F. A generalized Fibonacci sequence. Am. Math. Mon.
- Horadam, A.F. Generating functions for powers of a certain generalized sequence of numbers. Duke Math. J. 1965, 32, 437–446. [Google Scholar] [CrossRef]
- Horadam, A.F. Basic properties of a certain generalized sequence of numbers. Fibonacci Quart. 1965, 3, 161–177. [Google Scholar]
- Horadam, A.F. Special properties of the sequence wn(a,b;p,q). Fibonacci Quart. 1967, 5, 424–434. [Google Scholar]
- Horadam, A.F. Tschebyscheff and other functions associated with the sequence {wn(a,b;p,q)}. Fibonacci Quart. 1969, 7, 14–22. [Google Scholar]
- Belbachir, H.; Belkhir, A. On some generalizations of Horadam’s numbers. Filomat 2018, 32, No–14. [Google Scholar] [CrossRef]
- Kocer, G. E.; Mansour, T.; Tuglu, N. Norms of circulant and semicirculant matrices with Horadam’s numbers. Ars Comb. 2007, 85, 353–359. [Google Scholar]
- Bessel-Hagen, E. Repertorium der hSheren Mathematik. Leipzig, 1929, p. 1563.
- Lucas, E. Théorie des Nombres. Paris, 1961.
- Tagiuri, A. Sequences of positive integers. Periodico di Matematiche Storia, Series 2 1901, 3, 97–114. [Google Scholar]
- Dickson, L.E. History of the theory of numbers. Vol. I: Divisibility and primality; Chelsea Publishing Co., New York, 1966, Chapter 17.
- Assi, A.; D’Anna, M.; Garcia-Sanchez, P.A. Numerical semigroups and applications. 2nd extended and revised edition, RSME Springer Series 3. Cham: Springer (2020).
- Rosales, J.C.; Garcia-Sanchez, P.A. Finitely generated commutative monoids. Nova Science Publishers, Inc., Commack, New York, 1999.
- Rosales, J.C.; Garcia-Sanchez, P.A. Numerical semigroups. Developments in Mathematics, 20. Springer, New York, 2009.
- Komatsu, T.; Ying, H. p-numerical semigroups with p-symmetric properties. J. Algebra Appl. 2024, (Online ready), 2450216. [Google Scholar] [CrossRef]
- Komatsu, T. The Frobenius number for sequences of triangular numbers associated with number of solutions. Ann. Comb. 2022, 26, 757–779. [Google Scholar] [CrossRef]
- Komatsu, T. The Frobenius number associated with the number of representations for sequences of repunits. C. R. Math. Acad. Sci. Paris 2023, 361, 73–89. [Google Scholar] [CrossRef]
- Komatsu, T.; Laohakosol, V. The p-Frobenius problems for the sequence of generalized repunits. Results Math. 2023, 79, 26. [Google Scholar] [CrossRef]
- Apéry, R. Sur les branches superlinéaires des courbes algébriques. C. R. Acad. Sci. Paris 1946, 222, 1198–1200. [Google Scholar]
- Komatsu, T. Sylvester power and weighted sums on the Frobenius set in arithmetic progression. Discrete Appl. Math. 2022, 315, 110–126. [Google Scholar] [CrossRef]
- Komatsu, T. On the determination of p-Frobenius and related numbers using the p-Apéry set. Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. RACSAM 2024, 118, Article–58. [Google Scholar] [CrossRef]
- Komatsu, T.; Zhang, Y. Weighted Sylvester sums on the Frobenius set. Irish Math. Soc. Bull. 2021, 87, 21–29. [Google Scholar] [CrossRef]
- Komatsu, T.; Zhang, Y. Weighted Sylvester sums on the Frobenius set in more variables. Kyushu J. Math. 2022, 76, 163–175. [Google Scholar] [CrossRef]
- Brauer, A.; Shockley, B.M. On a problem of Frobenius. J. Reine. Angew. Math. 1962, 211, 215–220. [Google Scholar]
- Selmer, E.S. On the linear diophantine problem of Frobenius. J. Reine Angew. Math. 1977, 293–294, 1–17. [Google Scholar]
- Tripathi, A. On sums of positive integers that are not of the form ax+by. Am. Math. Mon. 2008, 115, 363–364. [Google Scholar] [CrossRef]
- Punyani, P.; Tripathi, A. On changes in the Frobenius and Sylvester numbers. Integers 2018, 18B, Paper. [Google Scholar]
Table 1.
for odd k
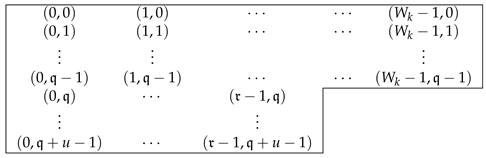 |
Table 2.
when
 |
Table 3.
for even k
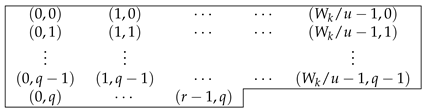 |
Table 4.
() for odd k
 |
Table 5.
() for odd k
 |
Table 6.
for odd k
 |
Table 7.
() for even k
 |
Table 8.
() for even k
 |
Table 9.
for even k
 |
Table 10.
for
 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated






