Prerpints.org logo
Preprint
Article

Investigating the Influence of Fiber Content and Geometry on the Flexural Response of Fiber-Reinforced Cementitious Composites.

Altmetrics

Downloads

103

Views

35

Comments

0

A peer-reviewed article of this preprint also exists.

supplementary.xlsx (23.89KB )

This version is not peer-reviewed

Submitted:

27 July 2024

Posted:

29 July 2024

You are already at the latest version

Alerts
Abstract
This research investigates fiber-reinforced cementitious composites, with a particular emphasis on the geometric properties of soft micro and macro fibers. These fibers are characterized by a lower elastic modulus and larger aspect ratios than steel fibers. The composites were manufactured using a cement-to-sand ratio of 1:2.5, with 20% fly ash serving as a partial substitute. In order to attain varying strengths, two water-to-binder ratios (0.55 and 0.60) were implemented. Synthetic fibers, such as carbon and polypropylene, and natural sisal fibers, were introduced in volumes varying from 0.4% to 2.27%, with aspect ratios ranging from 71 to 3750. The flexural strength and behavior were evaluated using 75x75x380 mm prisms, while the compressive strength was measured using 50 mm cubes. Load-deflection curves were developed to investigate fracture behavior. The behavior of specimens reinforced with fibers post-cracking is dependent upon the matrix compressive strength, fiber type, quantity, and aspect ratio. A regression analysis was conducted on data from this study and previous publications to develop an equation that predicts the ratio of modulus of rupture (MOR) to initial fracture strength. The variables are matrix strength and fiber characteristics. The results reveal that carbon fiber-reinforced composites (CFRCCs) exhibit considerable brittleness post-cracking, despite exhibiting a flexural strength that is two to three times greater than that of control specimens. When workability is not compromised, the maximal load capacity and deflection are improved by increasing the fiber volume and aspect ratio. The maximal load stress is more significantly influenced by volumes of fibers than by aspect ratio.
Keywords: 
Subject: Engineering  -   Civil Engineering

Introduction

Fiber-reinforced concrete is a specific type of concrete that is reinforced by using fiber materials resulting in increased toughness, ductility, and resistance to cracking. It has been observed (Ravikumar et al., 2015) that the effectiveness of fiber-reinforced concrete depends on several parameters such as the type, amount of fiber, their geometric properties, and the orientation of the fibers inside the concrete. The addition of fibers of any material type (Polymeric, metallic or natural) into the concrete, modify the mechanical properties of the composite and effectively fixes the cracking problems
Modern research and development efforts have led to significant advancements in FRC technology (Hao et al., 2023), (Tabatabaei et al., 2013) , (Talikoti & Kandekar, 2019). The use of high-performance fibers, such as carbon and aramid fibers, has enabled fiber-reinforced composites (FRC) to meet the demanding requirements of contemporary infrastructure and building projects.
The fiber content in Fiber Reinforced Concrete (FRC) is a critical factor in determining its properties. The addition of fibers to concrete has been demonstrated to improve many mechanical characteristics, including tensile strength, flexural strength, toughness, and impact resistance. Nevertheless, it is important to understand that this inclusion might also result in a decrease in the workability of the concrete.
The investigations (Yazıcı et al., 2007) and (Soufeiani et al., 2016) show how the volume percentage of fibers affects the properties of FRC. Their findings indicate that the fiber's shape and kind determine the optimal fiber volume in concrete. An example of this is when concrete mixtures are strengthened with basalt fibers and have a greater concentration of fibers, namely about 0.40 % of 12 mm fibers. In such cases, it is common for the fibers to clump together, resulting in what is known as fiber balling. This issue results from fibers clumping and making it harder to get a homogenous mix during the concrete mixing process, therefore affecting the workability of the mixture.
It has been noticed (Soylu & Bingöl, 2019) that up to an adequate fiber concentration of 8%, adding steel fibers into Slurry Infiltration Fiber Reinforced Concrete (SIFCON) significantly increases its compressive and flexural strengths. Beyond this amount of fiber, strength was decreased because the mortar's restricted flow through closely packed fibers. It has been shown that both compressive and flexural strengths increase with increasing aspect ratio of the fiber, where at an aspect ratio of 80, the greatest results were obtained. The optimal volume fraction of fibers in concrete varies depending on factors such as the type and size of the fibers (Liu et al., 2020). Additionally, it has been reported by (M. A. Mashrei et al., 2018) that the flexural strength of concrete increase with the addition of polypropylene fibers up to 0.3% , regardless of the presence or absence of reinforcing steel. The results revealed that the best percentage of polypropylene fiber for flexure is between 0.2% and 0.3%. The study done by (Maksum et al., 2020)shown that the addition of steel fibers has a substantial influence on the split tensile and flexural strength of concrete. The magnitude of this effect depends on the aspect ratio (l/d ratio) and volume fraction (Vf) of the fibers.
The ACI-code 544 committee (ACI Committee 544, 2001) has shown that incorporating elongated fibers with a high aspect ratio into typical Steel Fiber Reinforced Concrete (SFRC) can enhance the material's post-peak load behavior. Nevertheless, it is important to realize that the inclusion of lengthy fibers might also present difficulties with the even distribution and dispersion of these fibers inside the concrete matrix.
The most important factors that significantly govern the tensile strength of concrete are the fiber content and fiber aspect ratio, in addition to compressive strength, which influences the tensile strength of fiber reinforced concrete (Musmar, 2013). The observed relationship between an increase in aspect ratio and a rise in tensile strength may be explained by the fact that increased aspect ratios indicate a greater extent of fiber surface area in contact with the matrix, typically resulting in enhanced bonding between the fiber and matrix(N. Banthia & J. Trottier, 1995). increased level of contact generally leads to a more robust connection between the fiber and the matrix, hence enhancing the tensile strength. Furthermore, it is important to mention that extensive fibers with a substantial aspect ratio have been discovered to be more effective in bridging cracks that may develop within the matrix (A.E. Naaman & H.W. Reinhardt, 1996).These factors collectively impact the tensile characteristics of concrete that is reinforced with fibers. However, exceeding a particular limit in the aspect ratio could cause difficulties in establishing even distribution over the matrix, perhaps leading to aggregation and therefore diminishing the strength (Alrawashdeh & Eren, 2022).
The study conducted on the influence of steel fiber aspect ratio and fiber type on flexural performance of UHPFRC. Research has demonstrated that the utilization of long straight or twisted steel fibers leads to higher ductility compared to the use of short straight steel fibers. The study demonstrated that the flexural strength could be maximized when using steel fibers with aspect ratios ranging from 30 to 50 (Yoo & Yoon, 2016). The improved dispersion and alignment of fibers inside the geopolymer concrete matrix were identified as the main factors contributing to this phenomenon. However, the advantages begin to decline when problems like with balling and fiber clumping arise(S. K. Lee et al., 2023)
Within the domain of Fiber-Reinforced Concrete (FRC), the scholarly community universally recognizes the significance of certain fiber kinds and amounts in enhancing mechanical characteristics. more evidence to emphasize the importance of the interaction between fibers and the matrix provided as a crucial factor in determining the performance of fiber-reinforced composites (FRC) (Lin et al., 2023). Similarly (Amin & Gilbert, 2019)emphasized the importance of this contact in enabling the transmission of stress from the fibers to the concrete matrix, a crucial aspect in enhancing the toughness of fiber-reinforced concrete (FRC). According to (Y. Lee et al., 2010), the cracking strength of Fiber-Reinforced Concrete (FRC) is mostly governed by the strength of the concrete matrix. Furthermore, the post-cracking behavior of fiber-reinforced concrete (FRC) is influenced by the properties of the fiber reinforcement and the strength of the bond between the fiber and the matrix.
Numerous studies and reports have looked at the influence of fiber properties on the mechanical properties and behavior of fiber-reinforced cementitious composites (FRCCs), with a focus on steel fibers (RILEM TC 162-TDF, 2000; Singh, 2017). According to the American Concrete Institute (ACI Committee 544, 2001), SFs are defined as discrete, short lengths of steel that have an aspect ratio (the ratio of length to diameter) in the range of 20 to 100. However, there is a significant gap in understanding the impact of aspect ratio of synthetic microfibers like carbon, micro polypropylene and natural fibers such as sisal as well. Because of their tiny size, these microfibers have aspect ratios that surpass steel fibers by several times. A critical question arises: do these higher aspect ratios of flexible synthetic fibers have a consistent growing rate of influence on the mechanical properties of FRCC as steel fibers, and do they have distinct effects on mixture workability? It is vital to explore the influence of these soft fibers which have lower elastic moduli than rigid steel fibers.
The significance of this work is that to investigates the impact of such tiny diameter carbon and micro polypropylene fibers on the flexural performance of FRCCs. The study aims to investigate the impact of different fiber types (carbon, micro, macro polypropylene and sisal fibers) on the compressive and flexural strength, as well as the initial cracking resistance and post-cracking behavior of cementitious composites reinforced with fibers. The flexural strength and behavior of fiber-reinforced cementitious composites are estimated by incorporating an innovative formula derived from statistical regression analysis of the current data and data collected from the previous literature. The purpose of this equation is to determine the extent of the influence that different volume fractions and aspect ratios of these soft fibers have on the first cracking stress and maximum flexural stress of FRCC.

Experimental Work

Materials

Ordinary Portland cement type I available in the region and brought from Bazian cement factory plant was used in this investigation. Physical properties and chemical composition of the cement satisfied the requirements of EN-197-1.
Locally available natural fine aggregate (NFA) was used as fine aggregate in the mix. The water absorption and specific gravity based on ASTM C128 (2015) in the saturated surface dry condition (SSD) were 1.3 % and 2.69, respectively. The maximum size of fine aggregates was 2.36 mm. Grading and properties of sand is shown in Table 1: The fine aggregates were washed and dried in a laboratory for several hours until saturated and surface dry (SSD) condition were obtained.
Fly ash was added 20 % by weight of total cementitious composite. Specific gravity of fly ash was 2.2 and the chemical composition are listed in Table 2.
Fibers: The carbon fiber involved in this study was SikaWrap FX-50C type which is unidirectional cord commonly used for strengthening the structures. The diameter of the monofilament fiber is 0.008 mm and various length was prepared by cutting the cord the following lengths were obtained; 5mm, 10 mm, 15 mm, 20 mm , 20 mm and 25mm and thus the aspect ratio of the fiber, defined as the ratio of the length to diameter of the fiber, is calculated to be 625, 1250, 1875, 2500, 3125 and 3750. The following characteristics of carbon fiber provided by the manufacturer: Tensile strength,4000 MPa , modulus of elasticity, 240 kN/mm2 and the density was 1.825 gm/cm3.
Two types of Polypropylene fiber was used in this study (Micro and Macro fiber) , provided by local Sika company also. Micro PP fiber commonly added to concrete mixtures to control the plastic shrinkage of concrete. The fiber was type SikaFiber PPM®-12 . The diameter of monofilament fiber provided was 0.032 mm , fiber length was 12 mm, and density of the fiber ~ 0.91 gm/cm3, hence the aspect ratio was calculated to be 375. The tensile strength of the fiber also provided was ranged between 467 to 548 N/mm2. However macro polypropylene fibers was SikaFiber® Force-60 which is synthetic fiber for structural use in shotcrete and concrete. The equivalent diameter of fibers was 0.84 mm and the length was 60 mm , the aspect ratio of the fiber calculated to be 71.4. The tensile strength of the PP macro polypropylene fiber provided was 550 MPa , and the tensile modulus of elasticity was 8.5 kN/mm2. Figure 1 Showing the shape and type of fibers used.
Local companies employed sisal fibers to reinforce gypsum boards. Agave sisalana, a plant that is found in tropical regions such as Kenya and is renowned for its exceptional strength-to-weight ratio, is the source of these fibers. The dimensions of individual sisal fibers was measured using digital vernier calipers. The equivalent diameter was determined to be 0.156 mm, with a density of approximately 1.22 g/cm³. In order to investigate the various aspect ratios of 256, 320, 384, 448, and 512, the fibers were prepared in a variety of lengths, including 40 mm, 50 mm, 60 mm, 70 mm, and 80 mm. The laboratory conducted tensile strength testing on three monofilaments, which resulted in an average of 279 N/mm².

Mix Proportions and Preparation of Samples

The experimental work consisted of preparing four groups of cementitious composites that have the same mix proportion of cement, sand, and fly ash ratio with the variability of volume fraction of fibers , aspect ratio and type of fibers as a reinforcing material and also varied with w/b ratio, as shown in Table 3. The use of superplasticizer depended on the workability of the mix, in which the rates was slightly varied . Each group had three specimens with a size of 50 mm cubes to follow the characteristics of the composite in terms of the compressive strength and prisms of size 75 × 75 × 380 mm, to measure first cracking strength and post cracking behavior of fiber reinforced cementitious composites. The groups G1, G2, and G3 were made up of a cement-based composite of five subgroups combined with carbon fiber at a 0.6 w/b ratio. The volume percentages of carbon fibers in G1 and G3 were (0.41, 0.82, 1.23, 1.63 2.05)%, in addition to a reference sample without fiber. G1 fibers have a constant length of 5 mm, whereas G3 fibers have a length of 3mm, resulting in an L/D aspect ratio of around 625 and 375, respectively. Furthermore, G2 featured carbon fibers, however at varying lengths of fibers, the aspect ratios achieved were 1250, 1875, 2500, 3125, and 3750, while the volume fraction of fibers remained constant at around 1%. Cement-based composite of G4 and G5 with the same mix percentage except the w/b ratio was 0.55, and polypropylene (PP) fiber was used, with micro-PP as a reinforcing material for G4 and macro-PP fiber for G5. Sisal fibers was introduced to the cementitious composite G6 and G7 as a reinforcing material with volume fractions varied from 0.41 to 2.03 % and different lengths where the aspect ratio calculated by dividing the length to equivalent diameter ratio was from 128 to 512 .The density of fresh composite examined ranged from 2130 to 2200 kg/m3.Table 3 displays the kind of fiber, the number of fibers by volume and weight for each batch, and the aspect ratio for each of the four groups of cementitious composite.

Test Variables and Specimens

Flowability

The flowability of each mix mentioned in Table 3 was measured using the flow table test, in accordance with the ASTM C1437(ASTM C 1437 – 07, 2007).
Compressive Strength
The specimens undergo compressive strength testing in accordance with the ASTM standard for cement mortar, namely the ASTM C109 standard (ASTM C 109/C 109M – 08, 2009). A computerized compression machine with a maximum load-carrying capability of 2,000 kN was employed to assess three similar specimens measuring 50 × 50 × 50 mm for each mixture. The compressive strength values were measured at the age of 28 days.

Flexural Strength

A flexural strength test was conducted on prisms of 75 × 75 × 380 mm, in accordance with the guidelines outlined in (ASTM C1018-97, 2017). The test was performed after a curing period of 28 days. The flexural strength that includes first cracking strength and maximum post cracking strength and load deflection curve of the specimens was evaluated by the utilization of a typical third-point bending test as shown in Figure 3.
Preprints 113497 g002
Figure 2. Testing Setup for Evaluating the Flexural Strength and Behavior of FRCC Specimens Using Four-Point Bending Test.
Figure 2. Testing Setup for Evaluating the Flexural Strength and Behavior of FRCC Specimens Using Four-Point Bending Test.
Preprints 113497 g002

Results and Discussion

Flowability

The flowability of the selected mixes demonstrated in Table 4 was evaluated using the flow table test. The results of the study indicated that an increase in both the volume fraction and aspect ratio of fibers had a negative impact on flowability in all of the mixtures examined. At first, the decrease in flowability was small, but once the volume percentage beyond a particular threshold, the deterioration in flowability became more significant. Regarding the aspect ratio, it was observed that the mixes made with carbon fibers exhibited favorable flowability up until an aspect ratio of 3125 corresponding to the mix G2M10 . However, it is worth noting that carbon fibers exceeded this point experienced a notable decline in flowability beyond this point.

Compressive Strength

Figure 4 show how fiber volume percentages and aspect ratios (l/d) affect the compressive strength of fiber-reinforced cementitious composites (FRCC). The study found that as the volume percent of fibers increased, compressive strength decreased, a pattern that was constant across carbon and polypropylene microfibers with l/d= 375. However, while there is a similar pattern of decreasing compressive strength as seen in mixtures with l/d of fibers of 675, there is no precipitous fall in compressive strength when the volume fraction hits 2.06%. This discrepancy emphasizes the different impacts of l/d, although increasing aspect ratio might reduce compressive strength due to inadequate compaction and flowability difficulties as resulted for FRCC with fibers having aspect ratio of 375, higher aspect ratios often result in enhanced tensile strength and a more solid connection , this is a reason for the mixes of FRCC with aspect ratio of 625. Critical thresholds were established at 1.6% for carbon fibers and 1.81% for polypropylene fibers of l/d =375, beyond which compressive strength decreases by 25% for carbon fibers and 15% for polypropylene fibers. This reduction is due to the fibers' lower elastic modulus when compared to plain mortar, which has a major influence on the composite's total elastic modulus (Abd Elmoaty et al., 2022) support these findings by noticing a loss in compressive strength with the presence of lightweight fibers such as polypropylene, with declines of 9.48% and 14.45% after 7 and 28 days, respectively, for combinations containing 4% fibers. At increasing volume fractions, the fibers have a lower modulus of elasticity and less interfacial bonding strength, which accounts for this pattern. It has been shown(BAŞSÜRÜCÜ et al., 2022) that adding steel fibers in certain quantities can improve compressive strength. Their findings show that short steel fibers at 0.4% and 0.8% volumes can boost strength by 5.84% and 9.98%, respectively, after 28 days, most likely owing to improved tension management between the matrix and fibers, which delays fracture onset.
The influence of Kevlar fiber on the compressive strength of concrete was carefully assessed (Y.-F. Li et al., 2023). The researchers discovered that Kevlar fiber-reinforced concrete (KFRC) specimens with a 0.5% weight ratio of Kevlar fiber had the maximum compressive strength in both quasi-static and dynamic testing, overcoming those with higher fiber ratios of 1.0% and 1.5%. This suggests that a 0.5% weight ratio of Kevlar fiber is ideal for increasing the compressive strength of concrete under both static and high-strain rate dynamic loading.
Figure 5 shows how varied aspect ratios of carbon fibers with equal volume fractions impact the compressive strength of Fiber Reinforced Cementitious Composites (FRCC). The study examines carbon fibers ranging in length from 10 to 30 mm, rising in 5 mm increments, yielding aspect ratios between 1250 and 3750. Compressive strength diminishes by 30% at an aspect ratio of 3125, and 40% at the maximum aspect ratio of 3750. This decrease comprises a 20% drop due to the inclusion of 1% adjusted fiber content, with an additional 10% and 20% loss as the aspect ratio becomes 3125 and 3750, respectively. It can be observed, the compressive strength of composites decreases with an increasing volume fraction (Vf %) of sisal fibers. At 2.0 % fiber volume fraction, the compressive strength reduces to about 32.0 MPa. This change represents a decrease in compressive strength by approximately 31.0%

Equivalent Elastic Bending Stress versus Deflection

The load resistance, is influenced by the dimensions of the specimen and its span. Thus, in order to facilitate comparisons with findings from various scholarly articles, it is more suitable to express the load-deflection curves in terms of the equivalent elastic bending stress-versus deflection(Naaman, 2018). The stress can be calculated for any load on the load-deflection curve using the calculation provided in the (ASTM C1609/C1609M-12, 2009)standard for the third point loading arrangement as follow;
f = P L b d 2  
Where ;
( f ) : is the equivalent elastic bending stress; (P): is the applied load ;( b) is the width of specimen ; (h) is the depth of the specimen and (L) is the span between the supports c/c.
Figure 6 shows parts of the prisms and their fracture places. It is evident that the fractures do not always appear exactly in the center. Several of the cracks are found around the edges of the middle spans, showing that Fiber Reinforced Concrete (FRC) responds differently than regular concrete. This strength variation can be connected to areas with densely packed fiber bundles, which may increase the structural integrity of those sections. As a result, the central line of the span may not be the weakest point. However, all of the cracks originated within the middle span of the prism.
Figure 7a; displays the flexural stress versus deflection curve for carbon fiber reinforced mortar samples with fibers of 5 mm in length. Initially, the curve is intended to grow linearly, indicating the material's elastic behavior, until reaching a peak representing the final flexural strength. The peak flexural stresses measured for the series G1M1 to G1M5 were 4.61, 5.07, 6.22, 9.26, and 9.59 MPa, respectively. These results exceed the first crack formation stress, which was calculated to be 3.8 MPa using the flexural strength of the control samples without fibers. Furthermore, the findings show that fiber-reinforced specimens have a larger deflection by approximately 2 times as a maximum at peak stress, indicating the increased toughness provided by the addition of carbon fibers when compared to their non-fiber-reinforced specimens.
The insertion of fibers with a low elastic modulus into a concrete or mortar matrix reduces the total elastic modulus of the composite material (Naaman, 2008). Following the peak stress, the material's load bearing capacity declined significantly, indicating a response subsequent to cracking.
The exhibited stress-deflection curve indicates a primarily brittle failure mode, with material breaking followed by little to no plastic deformation. It is worth noting, however, that the flexural strength increased by two to three times when compared to non-fiber specimens, with only a single fracture appearing prior to reaching the peak load. Following this peak, there was a quick drop in load-bearing capability, with residual strength varying between 1 and 2.5 MPa depending on the fiber volume percent in the mixture. Following fracture creation, the specimens maintained structural integrity, rather than breaking into two different pieces, as seen in the adjacent figure.
Figure 6b; shows the impacts of several carbon fiber lengths within the mortar, ranging from 10 mm to 30 mm and incremented by 5 mm for each mix configuration. Throughout the studies, the fiber concentration is constantly about 1% by volume. This picture most likely depicts a succession of curves, each corresponding to a particular fiber length. All of these curves show a continuous tendency, with flexural stress reaching a peak that far surpasses the flexural strength of the control specimen. By interpolating the values of fiber volume fractions between 0.81% and 1.23%, one can approximate the maximal stress value for a 1% fiber volume fraction in Figure 6b, this value is slightly greater than the maximum tension detected in specimens reinforced with a 1% volume of fibers at aspect ratio of 625.
This tendency emphasizes the importance of the aspect ratio in determining the composite material's flexural capabilities as well as its post-crack performance. One distinguishing aspect of these curves is the more gradual post-peak decline in bending stress. This is in contrast to the behavior observed in the group with an aspect ratio of 625, which showed a more rapid drop in stress. As the aspect ratio increases to 2500 and higher, the stress decrease along the descending branch becomes less dramatic, suggesting improved material characteristics.
At an aspect ratio of 3750, an anomaly is discovered in which the flexural strength drops more quickly, showing higher brittleness in comparison to lower aspect ratios. This increased brittleness is mostly due to a considerable drop in the mix's flowability and compressive strength produced by the fibers' excessive length. These lengthy fibers most likely hampered the efficient compaction process during specimen preparation.
Specifically, specimens with aspect ratios of 2500 and 3125 initially had a quick reduction in load-bearing capacity from peak values of roughly 44% and 28%, respectively, to 4.24 and 5.55 MPa, but followed by a more steady fall.
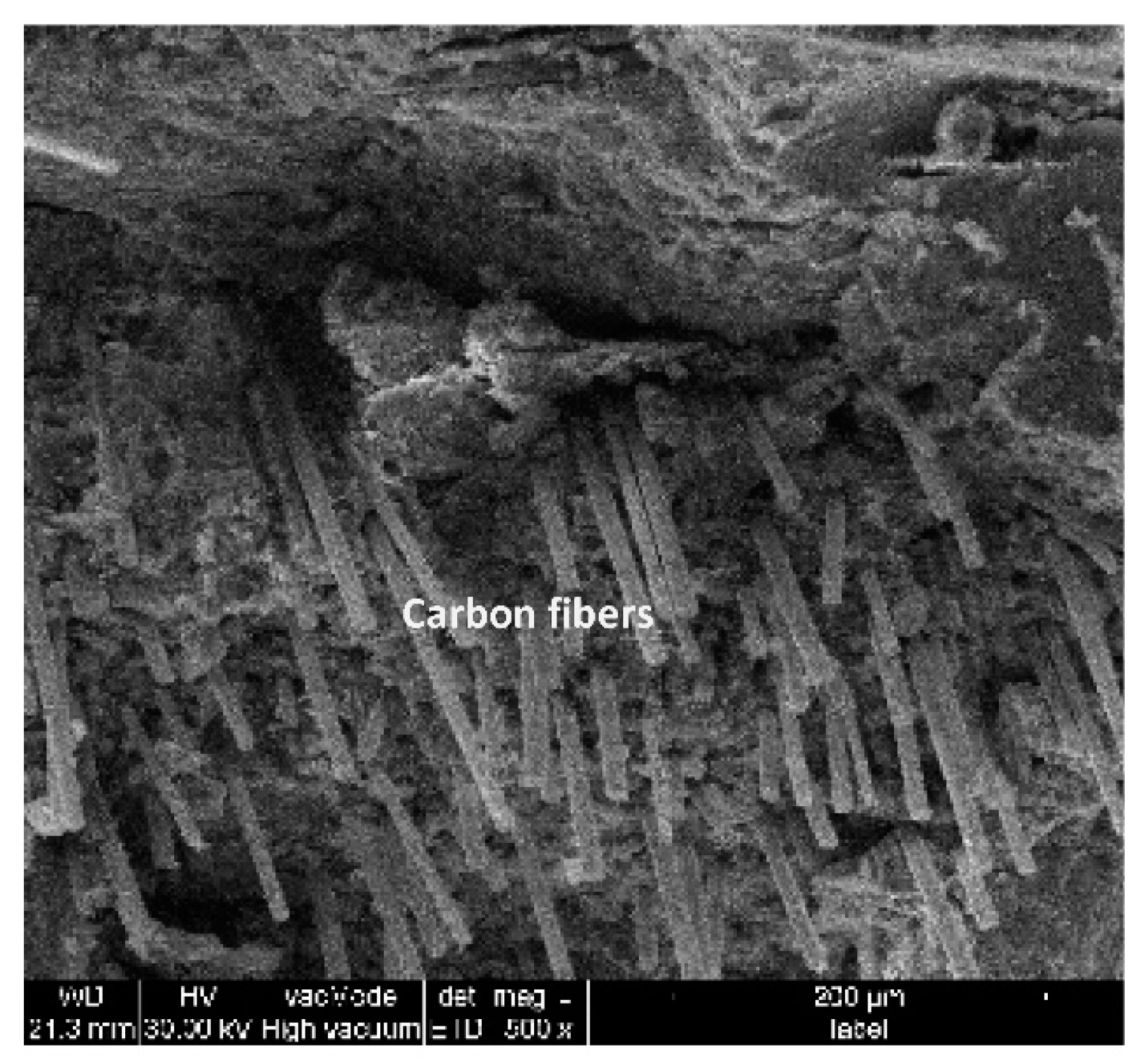
This trend is due to the greater inter fiber bonding at higher aspect ratios. A higher aspect ratio usually enhances load transmission efficiency between the fiber and the cementitious matrix. Figure 10 shows SEM images of carbon fibers mixed into a cement-based composite, resulting in short tubular structures with minimal gaps. These fibers have a high aspect ratio and small diameters, resulting in excellent adherence to the cement matrix. Under flexural stress, they increase the composite's structural integrity; beyond a maximum flexural load, they cause brittle failure due to the rupture of the majority of the fibers. This improved load transfer leads to better fracture bridging inside the matrix, which increases the composite's strength, and deflection at failure (Yoo et al., 2017).
Figure 7c; shows the bending stress vs deflection curve for mortar reinforced with 3 mm carbon fibers. This curve is predicted to follow a pattern similar to that shown in Figure 6a, beginning with a linear section showing elastic behavior and peaking above the first cracking stress or flexural strength observed in non-fiber specimens. After peak, load capacity decreases significantly, decreasing to between 0.5 and 1.5 MPa. Notably, specimens with a 2.07% fiber volume percentage, although having a lower peak stress, but it showed a steady drop, indicating a more ductile failure style. When we examine these lines, which have a lower length-to-diameter ratio (l/d) than those in Figure 6a at the same fiber volume percent, we can see that the peak stress is lower. This demonstrates the role of the aspect ratio in increasing the flexural strength of Fiber Reinforced Cementitious Composites (FRCC).
Figure 8a,b provides a detailed evaluation of the effect of polypropylene (PP) fibers on the mechanical response of composite materials. The study focuses on the deflection behavior and flexural characteristics at different volume fractions and aspect ratios. The data given in the figures examines the effects of various volume fractions of PP fibers ranging from 0.45% to 2.27%. Figure 7a shows that micro-PP fibers have an aspect ratio of roughly 375, whereas macro-PP fibers have an aspect ratio of 71. The findings show a linear connection between deflection and stress, up to the limit of proportionality (LOP). The LOP values recorded ranged from 4.37 to 5.17 MPa, with the exception of two mixes (G4M18, G4M19) that were less than 4 MPa. This observation underscores the material's early elastic reaction.
Preprints 113497 g007
Figure 8. Equivalent elastic bending stress versus deflection of Polypropylene fiber reinforced mortar prisms (a) with different volume fraction of fibers at L/d=375(micro polypropylene) (b) different volume fractions of fibers at L/d=71 (macro polypropylene).
Figure 8. Equivalent elastic bending stress versus deflection of Polypropylene fiber reinforced mortar prisms (a) with different volume fraction of fibers at L/d=375(micro polypropylene) (b) different volume fractions of fibers at L/d=71 (macro polypropylene).
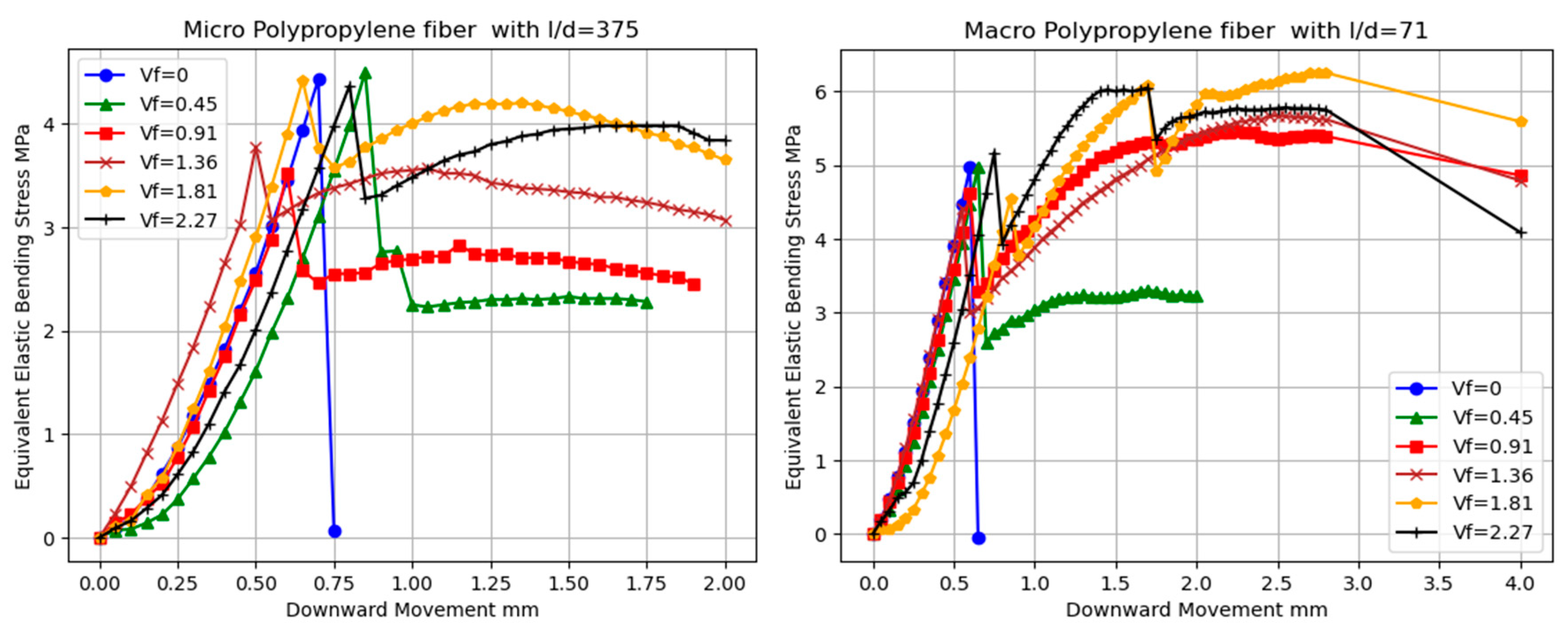
Preprints 113497 g007
Preprints 113497 g008
Figure 9. Equivalent elastic bending stress versus deflection of sisal fiber reinforced mortar prisms (a) with different volume fraction of fibers at L/d=128 (b) different aspect ratio of fibers at volume fraction=1.44 %.
Figure 9. Equivalent elastic bending stress versus deflection of sisal fiber reinforced mortar prisms (a) with different volume fraction of fibers at L/d=128 (b) different aspect ratio of fibers at volume fraction=1.44 %.
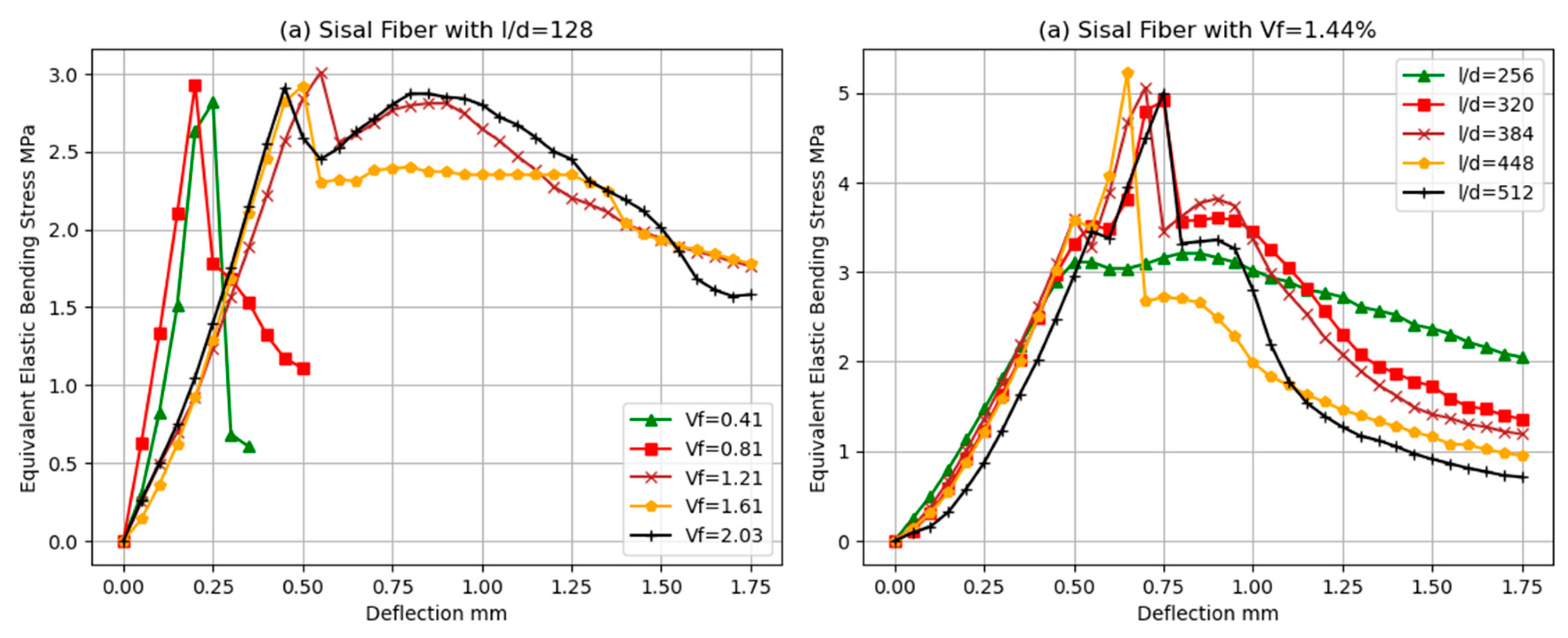
Preprints 113497 g008
Preprints 113497 g009
Figure 10. SEM of carbon fibers in a cement matrix ruptured at a maximum flexural load.
Figure 10. SEM of carbon fibers in a cement matrix ruptured at a maximum flexural load.
Preprints 113497 g009
Following the limit of proportionality (LOP), there was a significant drop in load, with varying magnitudes depending on the fiber volume percentage. It is worth noting that there is an inverse connection between fiber content and drop quantity, indicating that increased fiber content increases the material's ability to withstand cracking and structural failure. In specimens reinforced with micro fibers, the ratio between the maximum flexural stress (MOR) and the first cracking stress (LOP) was found to be less than one, indicating the presence of deflection softening behavior. However, when specimens with volume fractions of 1.36% and 1.81% were considered, the aforementioned ratio tended to unity, demonstrating a favorable link between fiber content and improved flexural behavior. Specimens with a volume fraction of 2.27% exhibited distinctive behavioral features. The substantial volume percentage of fibers had a detrimental influence on the mix's workability, making complete compaction and thorough mixing difficult to achieve. As a result, the percentage decrease in compressive strength was about 33% , emphasizing the need of carefully considering the interaction between fiber content, workability, and strength. Figure 11 displays a scanning electron microscope (SEM) image of a micro polypropylene (PP) fiber-reinforced matrix. The image shows several fibers that have completely debonded from their original positions within the matrix. This debonding leads to increased deflection under stress. The total percentage of fibers that remain effective in resisting the applied load after cracking has decreased, which reduces the overall load-carrying capacity of the material.
Figure 8b; shows the relationship between equivalent bending stress and deflection for samples reinforced with macro polypropylene fibers. The findings show that deflection hardening occurs equally across these specimens, as the maximal bending stress (MOR) exceeds the limit of proportionality (LOP). This trend is consistent across all samples, with the exception of those having 0.45% volume percentage of macro polypropylene fibers. The MOR-to-LOP ratio of 0.66 for these individual specimens is less than 1, indicating a considerable divergence from the overall trend seen. Furthermore, each of these specimens shows significant deflection while maintaining their flexural strength. This separates them from other examples that have been strengthened with carbon fibers. Despite having a lower length-to-diameter ratio (l/d), macro polypropylene fibers, with longer fibers, greatly improve the flexural strength and flexibility of the specimens compared to micro PP fibers. This discovery emphasizes the importance of the volume percentage and the presence of indentations on the surface of macro polypropylene fibers could enhance the interaction between the fibers and the cement matrix of macro polypropylene fibers in improving the deflection capacity and post-cracking stress behavior of the composite. The interfacial bond strength is an important aspect in better flexural performance, this highlighted the relevance of fiber qualities other than aspect ratio in designing fiber-reinforced concrete mixes customized to specific structural needs. Figure 12 showing SEM of macro PP fibers reinforced cementitious composite it is shown that the fibers us partially debonded from the matrix.
Figure 9a,b provide a detailed evaluation of the effect of sisal fibers on the flexural behavior of the cementitious composite. Figure 9a shows how increasing the percentage volume of sisal fibers improves the bending stress obtained by the mortar composite, up to a first cracking point; beyond this point, there is a sudden drop in the load, which decreases with an increase in the volume fraction of fibers, particularly when the volume of fibers exceeds 0.81%. Following that decrease, the fibers were able to withstand the imposed stress, and the flexural capacity was gradually raised until it reached the maximum flexural capacity, or MOR. The ratio of MOR to first cracking strength was changed from 0.8 to 1 for specimens reinforced with fiber volumes greater than 0.81%. Following the post-peak load, the load gradually lowered.
Figure 9b shows the effect of sisal fiber (L/d) on the flexural behavior of fiber-reinforced composites with a constant fiber volume percentage (Vf = 1.44%). Up to the point of initial cracking stress, the behavior of all curves is essentially linear and identical, implying that the composite structure acts consistently regardless of fiber aspect ratio. After the first cracking stress, each curve shows a significant decrease in stress that is approximately identical across all analyzed aspect ratios, demonstrating a consistent failure process despite changes in fiber length, with minor variances representing the influence of varied aspect ratios. The highest flexural stress after cracking is recorded at L/d = 384, indicating an optimum relationship between fiber length and matrix binding at this ratio. For larger aspect ratios, such as L/d = 448 and 512, the curves show a more notable decrease and poorer stress recovery, highlighting possible concerns such as increased micro-cracking caused by diminished fiber-matrix contact. This is supported by SEM pictures as shown in Figure 13, which reveal non-uniform dispersion and gaps leading to micro-cracks.

Modulus of Rupture

Figure 14 shows how integrating fibers affects the MOR, illustrating how the MOR corresponds with the fiber volume percentage in the mix. For mortars without fibers and a small volume fraction, the MOR is within certain limitations. At first, the inclusion of fibers decreases the MOR (modulus of rupture), suggesting that a little amount of fibers does not significantly enhance the ability to bear loads right before failure, as shown in both micro and macro polypropylene fibers. However, when the volume percentage of fibers reaches a critical threshold, which may be viewed as the minimum volume fraction of fibers, there is an observed increase in the modulus of rupture (MOR). The minimum volume fraction of fibers required to avoid a sudden breakdown may be estimated by calculating the ratio of the tensile strength of the fiber to the tensile strength of the matrix. For prisms reinforced with polypropylene fibers, this value is roughly 0.9%, whereas for prisms reinforced with carbon fiber, it is about 0.1%. Both macro and micro polypropylene fibers exhibited a lower modulus of rupture at 0.41%. However, as the volume fraction increased, the modulus of rupture increased for macro PP fibers, while for micro PP fibers, it remained lower than the first cracking strength even when the volume fraction reached 0.82%. This difference can be attributed to the lower interfacial bond strength of short and smooth micro polypropylene fibers compared to long and deformed macro polypropylene fibers.
However, the addition of carbon fiber, even at a small volume fraction of 0.41%, resulted in a flexural strength that was somewhat higher than the critical threshold. This enhancement signifies the participation of the fibers in connecting and bridging the fractures of the prisms, hence enhancing the composite's capacity to withstand forces following breaking.
Notably, specimens reinforced with carbon fiber had a peak stress that exceeded the flexural strength of control specimens across all fiber volume fractions. This emphasizes the distinct advantages of carbon fibers, such as their higher aspect ratio and tensile strength, over PP fibers in reinforcing mortars.
It has been derived by Naaman (Naaman, 2018) that the post cracking strength of fiber reinforced cement based products under direct tension depends on the fiber content , aspect ratio and interfacial bond strength between fibers and the matrix ( Ï„ ) as shown in the equation below;
σ P c = Λ . Ï„ . L d   V f
Where;
Λ : The coefficient is the result of multiplying numerous factors that determine the effectiveness of the fiber. The equation accurately predicts the tensile strength after cracking and can be utilized to predict the modulus of rupture for specimens under flexural stress by analyzing the stress distribution across the section of the members. This prediction takes into consideration various characteristics of fibers, such as fiber content, aspect ratio, and interfacial bond strength between the fiber and matrix.
However, measuring the interfacial bond strength between fiber and matrix in fiber-reinforced concrete (FRC) is challenging because fiber parameters (e.g., material, texture, shape) have a major influence on bond strength, resulting in inconsistent findings and measurement issues. Furthermore, the stress distribution among cement fibers and the entrance of air spaces during mixing and compaction introduce heterogeneity into bond strength measurements. Despite numerous estimating approaches, the absence of a worldwide agreed standard for measuring bond strength makes comparisons complicated between studies.
In this study, rather than focusing on the interfacial bond strength between fiber and matrix, two closely related properties to the bond strength was introduced: fiber tensile strength and matrix compressive strength. This study looks at how various factors affect the flexural strength of fiber-reinforced cementitious composite (FRCC). The primary variable of interest is the ratio of maximum post-cracking stress to initial cracking stress which is called as the limit of proportionality (MOR/LOP). This ratio correlated in relation to several independent variables, including fiber volume percentage (Vf), fiber aspect ratio (L/d), matrix compressive strength (C), and fiber material tensile strength (T). The information gathered was submitted to multi-logistic regression analysis using statistical techniques for predicting non-linear curves. The overall model structure is shown below:
M O R L O P = b 0 ∗ V f b 1   ∗ L d b 2   ∗ C b 3 ∗   T b 4
Figure 15 was generated by calculating the ratio of MOR to LOP using the measured flexural strength, as well as the anticipated ratios resulting from this model's output, for all laboratory specimens and extra data obtained from the literature, which totaled 96 instances. 33 of the above instances were derived from this study's experimental work, with the other data points coming from previous literature (G. Ramakrishna & Sundararajan Thirumalai, 2005)(BAŞSÜRÜCÜ et al., 2022), (B. Li et al., 2018), (Yoo et al., 2017). The figure displays a linear connection with the MOR to LOP ratio as the dependent variable and( V f 0.478 ∗ L d 0.038 ∗ C 0.161 ∗ T 0.253 ) as independent variable, the relation approximately linear with a coefficient of determination R2= 0.84 and sum of residual squares ∑ O b s − P r e d 2 = 8.13 , the slope of the best fit line determined is 0.095.
The prediction equation indicates that fiber characteristics, specifically fiber quantity and aspect ratio, have varying effects. Unlike steel fibers, synthetic soft fibers are not rigid, may be folded in a variety of directions, and can be crushed by shear force. As a result, the aspect ratio has far less effect than the fiber content.

Conclusions

The study looks at the impact of fiber content, shape, and type of synthetic nonrigid fibers on the mechanical properties of fiber-reinforced cementitious composites (FRCC). The key results include:
  • Carbon fiber composites have stronger flexural strength but poorer ductility than polypropylene. Stress behavior varies greatly depending on fiber type and aspect ratio, affecting the composite's mechanical performance.
  • Increasing fiber volume reduces compressive strength by 20 % for carbon fiber at 1.66 % and decrease by15% for polypropylene fiber at volume fraction 1.81% beyond this point compressive strength decreased notably.
  • Adding additional fibers improves peak load deflection in composites reinforced with carbon and macro polypropylene fibers. However, composites containing micro polypropylene and natural sisal fibers fibers have nearly the same and less first cracking load and less deflection as the fiber-less cementitious matrix, despite the fact that they enhance toughness.
  • Carbon fiber with an aspect ratio of 625 increased modulus of rupture by about 3 times at volume fraction more than 1.66 % compared to the cementitious matrix without fibers.
  • Macro polypropylene at volume fraction greater than 0.8 % contribute to deflection hardening behavior that was attributed to higher interfacial bond strength between fiber and whereas micro-PP fibers with higher aspect ratio tend to display deflection softening following cracking at all volume fractions.
  • According to the results and the model to predict the ratio of modulus of rupture to first cracking strength, the role of increasing volume fraction is much higher than the role of aspect ratio to enhance the properties of the composite.

Author Contributions

The corresponding author was entirely responsible for all parts of this research paper. This involves conception, technique, data gathering and analysis, as well as paper writing.

Funding

This study received no particular grants from public, commercial, or non-profit funding entities. There was no financial assistance for the study's development, execution, or publishing.

Data Availability Statement

The experimental work conducted as part of this research and supplementary data obtained from the literature were the sources of all datasets utilized in this study. In total, 96 instances were analyzed, with 33 instances originating from the experimental work of this study and the remaining data points sourced from the following references: (G. Ramakrishna & Sundararajan Thirumalai, 2005) ,(BAŞSÜRÜCÜ et al., 2022) , (B. Li et al., 2018), and (Yoo et al., 2017). The datasets generated and/or analyzed during the current study are available from the corresponding author upon appropriate request.

Acknowledgements

I am deeply appreciative of the invaluable support provided by the Civil Engineering Department staff, particularly those in the Concrete Laboratory and Soil Laboratory, during the preparation and testing of materials.

Conflicts of Interest

The authors declare that they have no competing interests.

References

  1. Abd Elmoaty, A. E. M., Morsy, A. M., & Harraz, A. B. (2022). Effect of Fiber Type and Volume Fraction on Fiber Reinforced Concrete and Engineered Cementitious Composite Mechanical Properties. Buildings, 12(12). [CrossRef]
  2. ACI Committee 544. (2001). Report on Fiber Reinforced Concrete, ACI 544.1R-96.
  3. A.E. Naaman, & H.W. Reinhardt. (1996). Chapter Characterization of high performance fiber reinforced cement composites - HPFRCC (A. E. Naaman & H. W. Reinhardt, Eds.; 1st Edition).
  4. Alrawashdeh, A., & Eren, O. (2022). Mechanical and physical characterisation of steel fibre reinforced self-compacting concrete: Different aspect ratios and volume fractions of fibres. Results in Engineering, 13, 100335. [CrossRef]
  5. Amin, A., & Gilbert, R. I. (2019). Steel Fiber-Reinforced Concrete Beams—Part II: Strength, Ductility and Design. ACI Structural Journal, 116(2). [CrossRef]
  6. ASTM C 109/C 109M – 08. (2009). Compressive Strength of Hydraulic Cement Mortars (Using 2-in. or [50-mm] Cube Specimens)1. ASTM Book of Standards, vol. 4.01, Cement; Lime; Gypsum. West Conshohocken, PA: ASTM International.
  7. ASTM C 1437 – 07. (2007). Standard Test Method for Flow of Hydraulic Cement Mortar. ASTM Book of Standards, vol. 4.01, Cement; Lime; Gypsum. West Conshohocken, PA: ASTM International.
  8. ASTM C1018-97. (2017). Standard Test Method for Flexural Toughness and First-Crack Strength of Fiber-Reinforced Concrete (Using Beam With Third-Point Loading). ASTM Book of Standards, vol. 4.05, Cement; Lime; Gypsum. West Conshohocken, PA: ASTM International.
  9. ASTM C1609/C1609M-12. (2009). Standard Test Method for Flexural Performance of Fiber-Reinforced Concrete (Using Beam With Third-Point Loading)1. ASTM Book of Standards, vol. 4.05, Cement; Lime; Gypsum. West Conshohocken, PA: ASTM International.
  10. BAŞSÜRÜCÜ, M., FENERLİ, C., KINA, C., & AKBAŞ, Ş. D. (2022). Effect of Fiber Type, Shape and Volume Fraction on Mechanical and Flexural Properties of Concrete. Journal of Sustainable Construction Materials and Technologies, 7(3), 158–171. [CrossRef]
  11. G. Ramakrishna, & Sundararajan Thirumalai. (2005). Impact strength of a few natural fibre reinforced cement mortar slabs: A comparative study. Cement and Concrete Composites, 27(5), 547–553.
  12. Hao, Y., Shi, C., Bi, Z., Lai, Z., She, A., & Yao, W. (2023). Recent Advances in Properties and Applications of Carbon Fiber-Reinforced Smart Cement-Based Composites. Materials, 16(7), 2552. [CrossRef]
  13. Lee, S. K., Oh, T., Banthia, N., & Yoo, D.-Y. (2023). Optimization of fiber aspect ratio for 90 MPa strain-hardening geopolymer composites (SHGC) with a tensile strain capacity over 7.5%. Cement and Concrete Composites, 139, 105055. [CrossRef]
  14. Lee, Y., Kang, S.-T., & Kim, J.-K. (2010). Pullout behavior of inclined steel fiber in an ultra-high strength cementitious matrix. Construction and Building Materials, 24(10), 2030–2041. [CrossRef]
  15. Li, B., Xu, L., Shi, Y., Chi, Y., Liu, Q., & Li, C. (2018). Effects of fiber type, volume fraction and aspect ratio on the flexural and acoustic emission behaviors of steel fiber reinforced concrete. Construction and Building Materials, 181, 474–486. [CrossRef]
  16. Li, Y.-F., Huang, Y.-R., Syu, J.-Y., Tsai, Y.-K., & Huang, C.-H. (2023). A study on mechanical behavior of Kevlar fiber reinforced concrete under static and high-strain rate loading. International Journal of Protective Structures, 14(3), 407–437. [CrossRef]
  17. Lin, C., Kanstad, T., Jacobsen, S., & Ji, G. (2023). Bonding property between fiber and cementitious matrix: A critical review. Construction and Building Materials, 378, 131169. [CrossRef]
  18. Liu, T., Yang, Y., Chen, Z., Li, Y., & Bai, R. (2020). Optimization of fiber volume fraction to enhance reinforcing efficiency in hybrid fiber reinforced strain hardening cementitious composite. Cement and Concrete Composites, 113, 103704. [CrossRef]
  19. M. A. Mashrei, Ali A. Sultan, & Alaa M. Mahdi. (2018). EFFECTS OF POLYPROPYLENE FIBERS ON COMPRESSIVE AND FLEXURAL STRENGTH OF CONCRETE MATERIAL. International Journal of Civil Engineering and Technology (IJCIET) , 9(11), 2208–2217.
  20. Maksum, M., Alrasyid, H., Irmawan, M., & Piscesa, B. (2020). Effect of steel fiber volume fraction to the tensile splitting strength of concrete cylinder. IOP Conference Series: Materials Science and Engineering, 930(1). [CrossRef]
  21. Musmar, M. (2013). Tensile strength of steel fiber reinforced concrete. Contemporary Engineering Sciences, 6, 225–237. [CrossRef]
  22. N. Banthia, & J. Trottier. (1995). Test Methods for Fexural Toughness Characterization of Fiber Reinforced Concrete: Some Concerns and a Proposition. Engineering Materials.
  23. Naaman, A. E. (2008). High Performance Fiber Reinforced Cement Composites. In High-Performance Construction Materials (pp. 91–153). WORLD SCIENTIFIC. [CrossRef]
  24. Naaman, A. E. (2018). Fiber Reinforced Cement and Concrete Composites. Techno Press 3000.
  25. Ravikumar, C. S., Ramasamy, V., & Thandavamoorthy, T. S. (2015). Effect of Fibers In Concrete Composites. International Journal of Applied Engineering Research, 10(1), 419–430.
  26. RILEM TC 162-TDF. (2000). Test and design methods for steel fibre reinforced concre. Materials and Structures/M, 33, 75–81.
  27. Singh, H. (2017). Steel Fiber Reinforced Concrete. Springer Singapore. [CrossRef]
  28. Soufeiani, L., Raman, S. N., Jumaat, M. Z. Bin, Alengaram, U. J., Ghadyani, G., & Mendis, P. (2016). Influences of the volume fraction and shape of steel fibers on fiber-reinforced concrete subjected to dynamic loading – A review. Engineering Structures, 124, 405–417. [CrossRef]
  29. Soylu, N., & Bingöl, A. F. (2019). Research on effect of the quantity and aspect ratio of steel fibers on compressive and flexural strength of SIFCON. Challenge Journal of Structural Mechanics, 5(1), 29. [CrossRef]
  30. Tabatabaei, Z. S., Volz, J. S., Gliha, B. P., & Keener, D. I. (2013). Development of Long Carbon Fiber-Reinforced Concrete for Dynamic Strengthening. Journal of Materials in Civil Engineering, 25(10), 1446–1455. [CrossRef]
  31. Talikoti, R., & Kandekar, S. (2019). Strength and Durability Study of Concrete Structures Using Aramid-Fiber-Reinforced Polymer. Fibers, 7(2), 11. [CrossRef]
  32. Yazıcı, Ş., İnan, G., & Tabak, V. (2007). Effect of aspect ratio and volume fraction of steel fiber on the mechanical properties of SFRC. Construction and Building Materials, 21(6), 1250–1253. [CrossRef]
  33. Yoo, D.-Y., Kim, S., Park, G.-J., Park, J.-J., & Kim, S.-W. (2017). Effects of fiber shape, aspect ratio, and volume fraction on flexural behavior of ultra-high-performance fiber-reinforced cement composites. Composite Structures, 174, 375–388. [CrossRef]
  34. Yoo, D.-Y., & Yoon, Y.-S. (2016). A Review on Structural Behavior, Design, and Application of Ultra-High-Performance Fiber-Reinforced Concrete. International Journal of Concrete Structures and Materials, 10(2), 125–142. [CrossRef]
Preprints 113497 g001
Figure 1. Various types of fibers used for the preparation of specimens.
Figure 1. Various types of fibers used for the preparation of specimens.
Preprints 113497 g001
Preprints 113497 g003
Figure 4. Percentage decrease in compressive strength of FRCC versus volume fraction of different types of fibers at different aspect ratios.
Figure 4. Percentage decrease in compressive strength of FRCC versus volume fraction of different types of fibers at different aspect ratios.
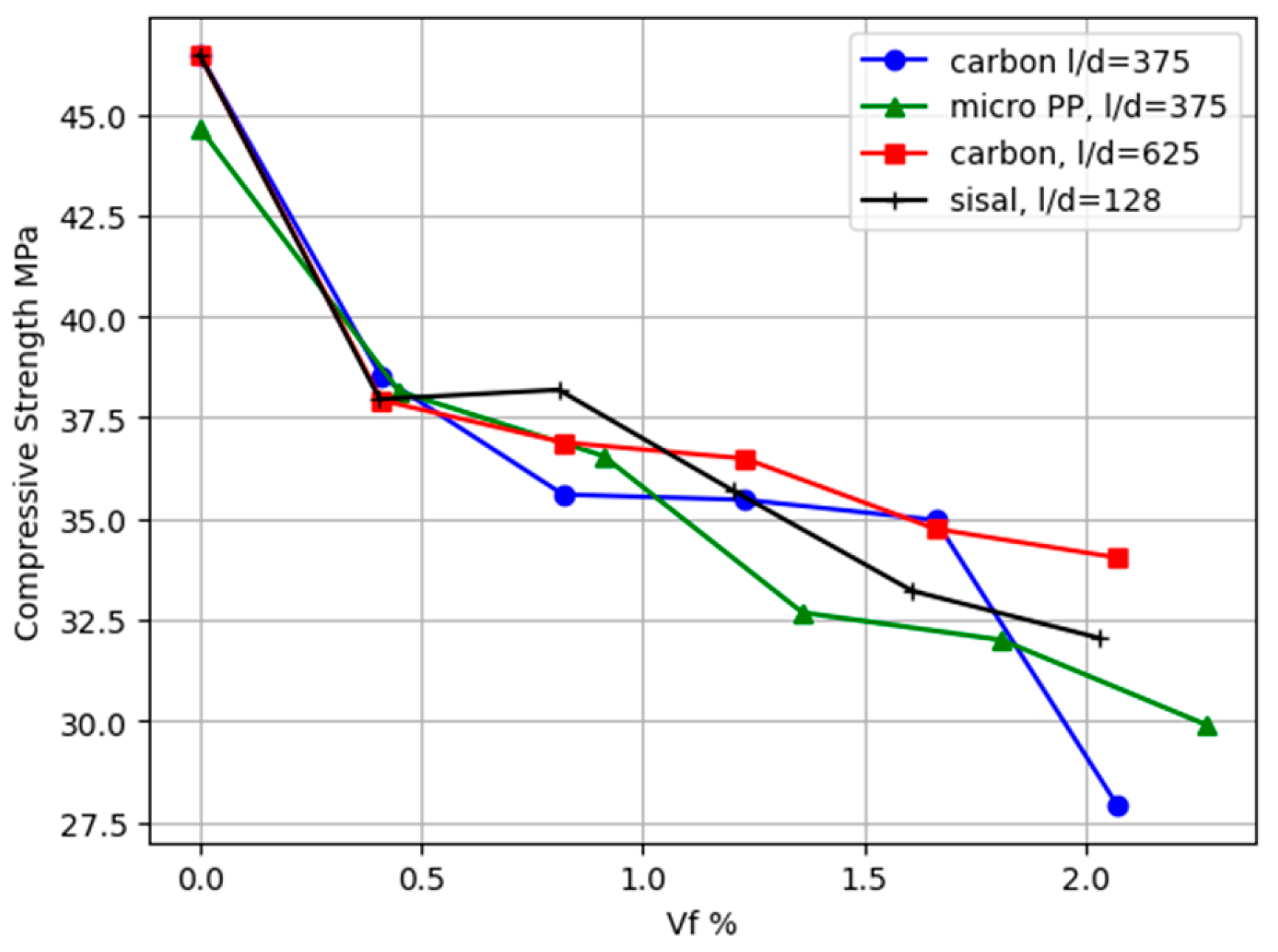
Preprints 113497 g003
Preprints 113497 g004
Figure 5. Percentage decrease in compressive strength of FRCC versus the aspect ratio of fibers.
Figure 5. Percentage decrease in compressive strength of FRCC versus the aspect ratio of fibers.
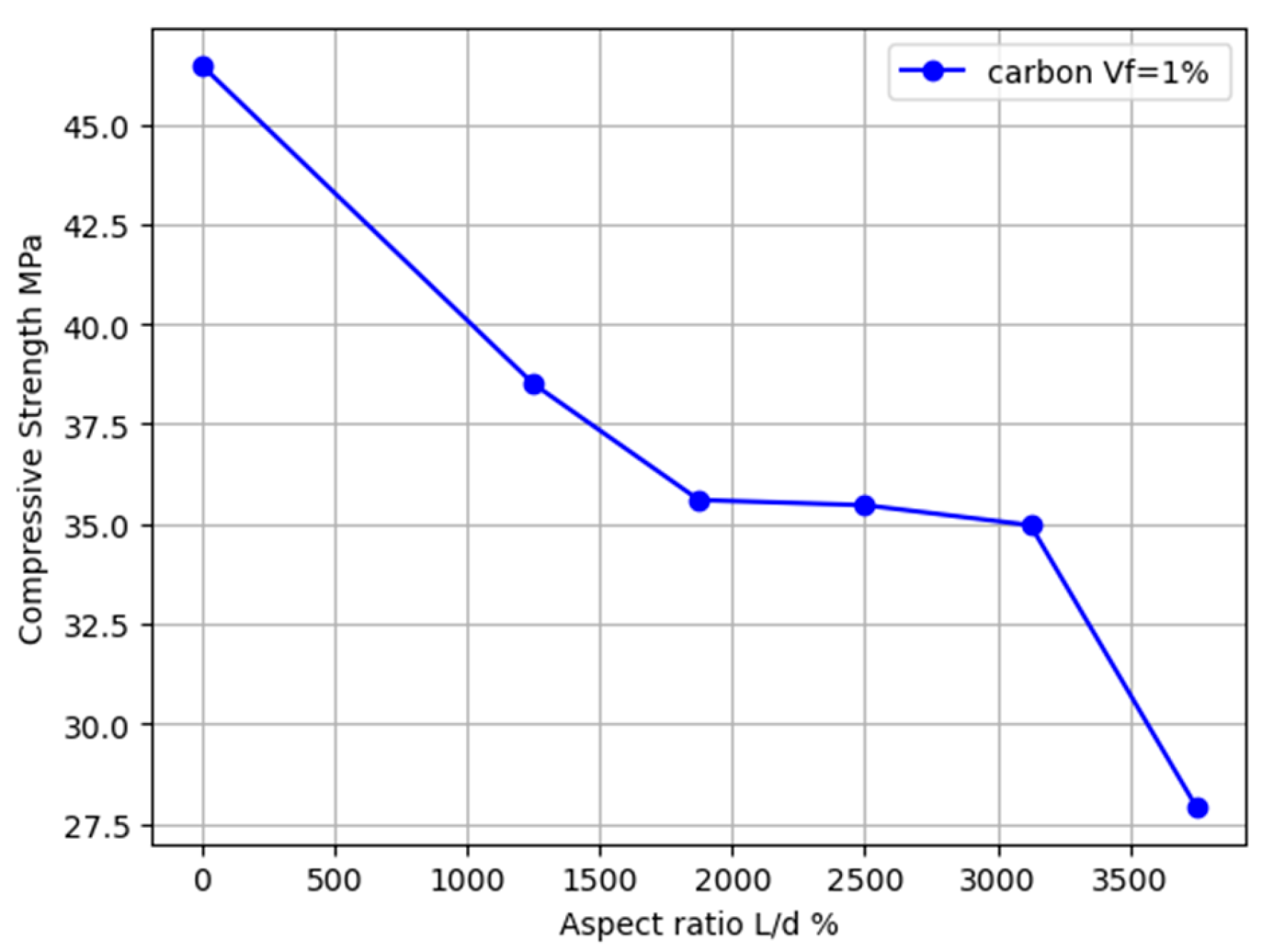
Preprints 113497 g004
Preprints 113497 g005
Figure 6. Part of the prisms showing the mode of failure and cracks location.
Figure 6. Part of the prisms showing the mode of failure and cracks location.
Preprints 113497 g005
Preprints 113497 g006
Figure 7. Equivalent elastic bending stress versus deflection of carbon fiber reinforced mortar prisms (a) with different volume fraction of fibers and L/d =625 (b) for different aspect ratios at Vf=1% , (c ) with different volume fraction of fibers and L/d =375.
Figure 7. Equivalent elastic bending stress versus deflection of carbon fiber reinforced mortar prisms (a) with different volume fraction of fibers and L/d =625 (b) for different aspect ratios at Vf=1% , (c ) with different volume fraction of fibers and L/d =375.
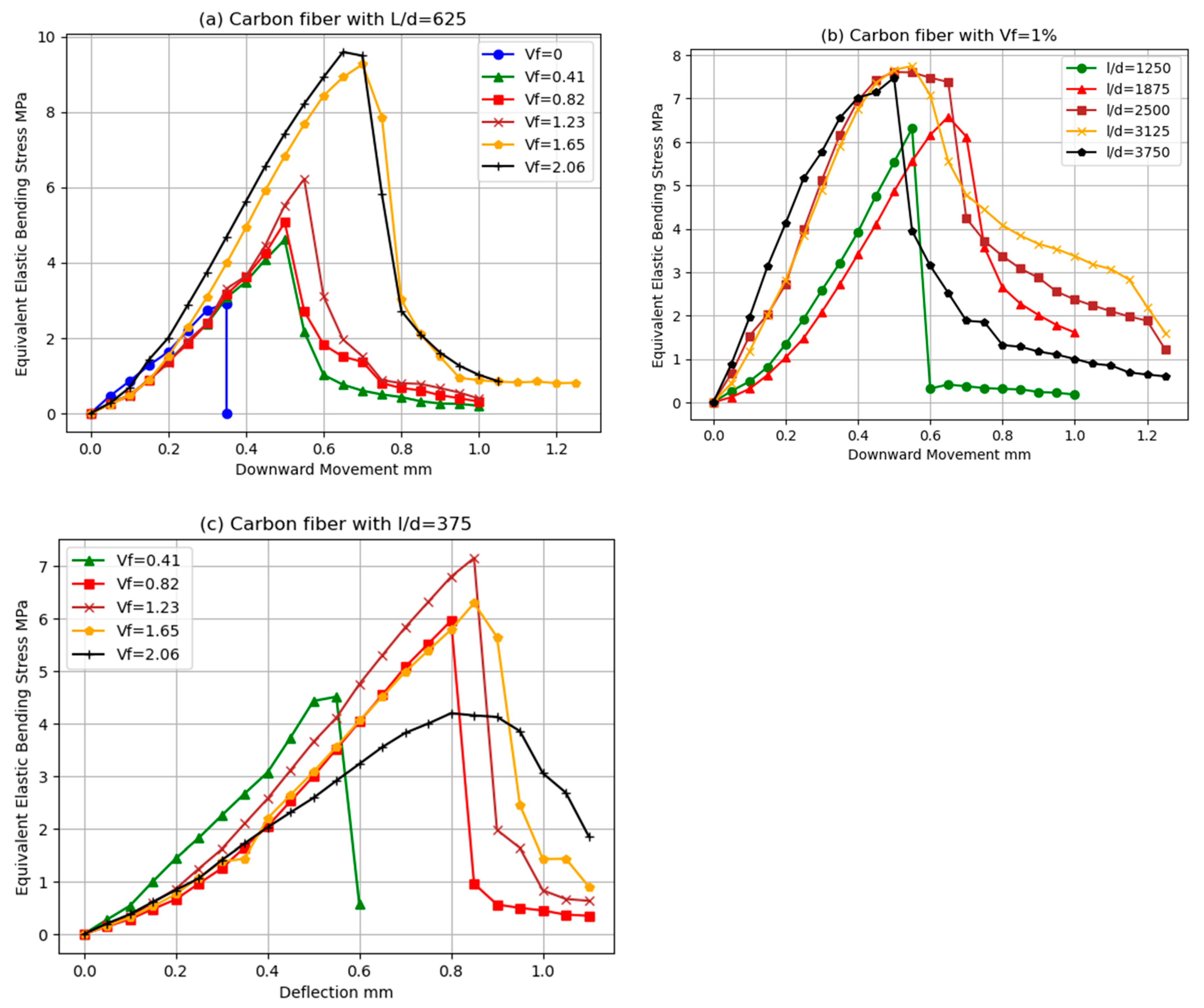
Preprints 113497 g006
Preprints 113497 g010
Figure 11. SEM of micro PP fibers in a cement matrix some of them debonded at a maximum flexural load.
Figure 11. SEM of micro PP fibers in a cement matrix some of them debonded at a maximum flexural load.
Preprints 113497 g010
Preprints 113497 g011
Figure 12. SEM of macro PP fibers in a cement matrix, many fibers where partially debonded from the matrix.
Figure 12. SEM of macro PP fibers in a cement matrix, many fibers where partially debonded from the matrix.
Preprints 113497 g011
Preprints 113497 g012
Figure 13. SEM shows sisal fibers in a cement matrix that have partially debonded due to very small fractures in the matrix and breaking in the fiber.
Figure 13. SEM shows sisal fibers in a cement matrix that have partially debonded due to very small fractures in the matrix and breaking in the fiber.
Preprints 113497 g012
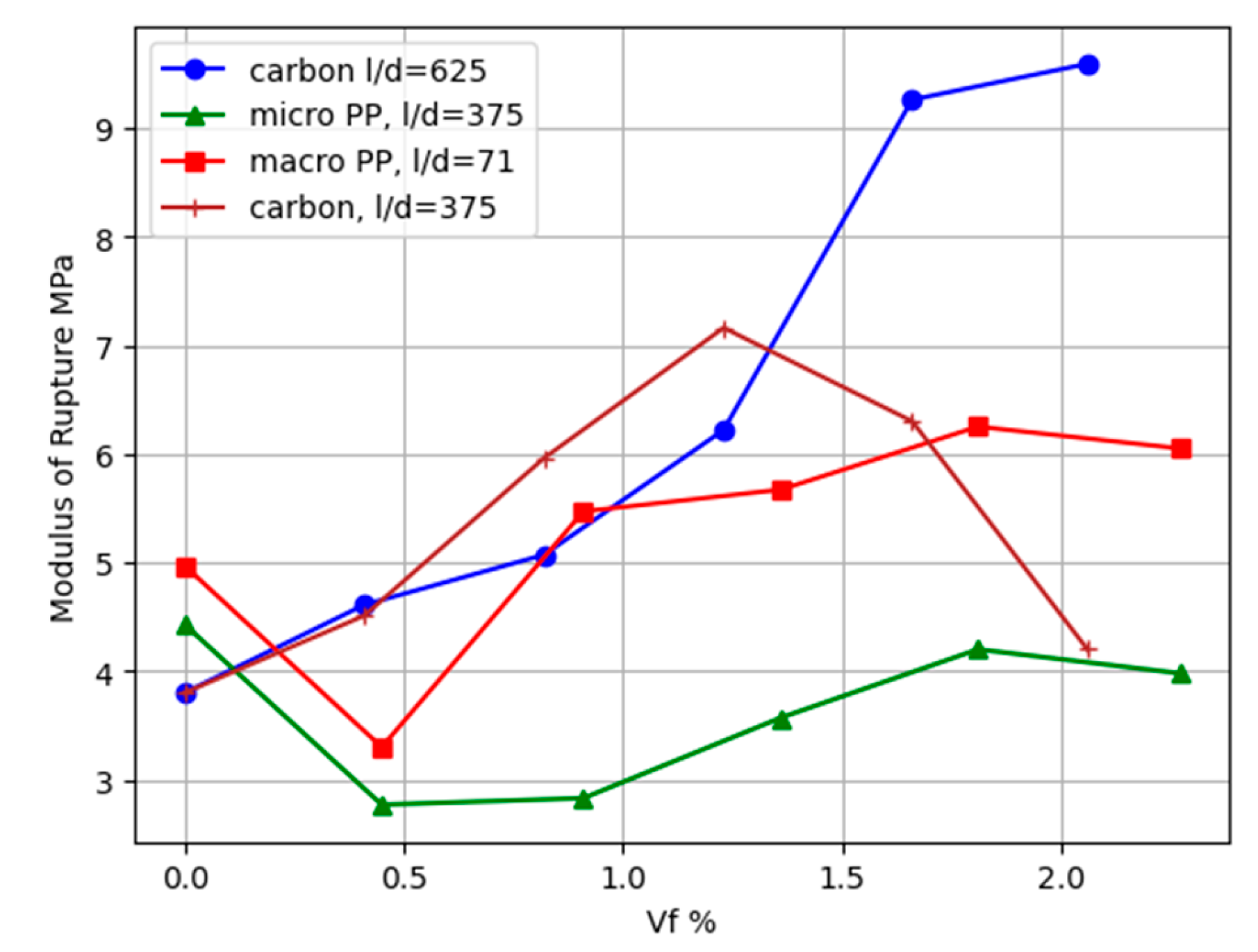
Preprints 113497 g013
Figure 14. Effect of volume fraction of fibers on the maximum equivalent elastic bending stress (Modulus of rupture) of FRCC reinforced with carbon and polypropylene fibers.
Figure 14. Effect of volume fraction of fibers on the maximum equivalent elastic bending stress (Modulus of rupture) of FRCC reinforced with carbon and polypropylene fibers.
Preprints 113497 g013
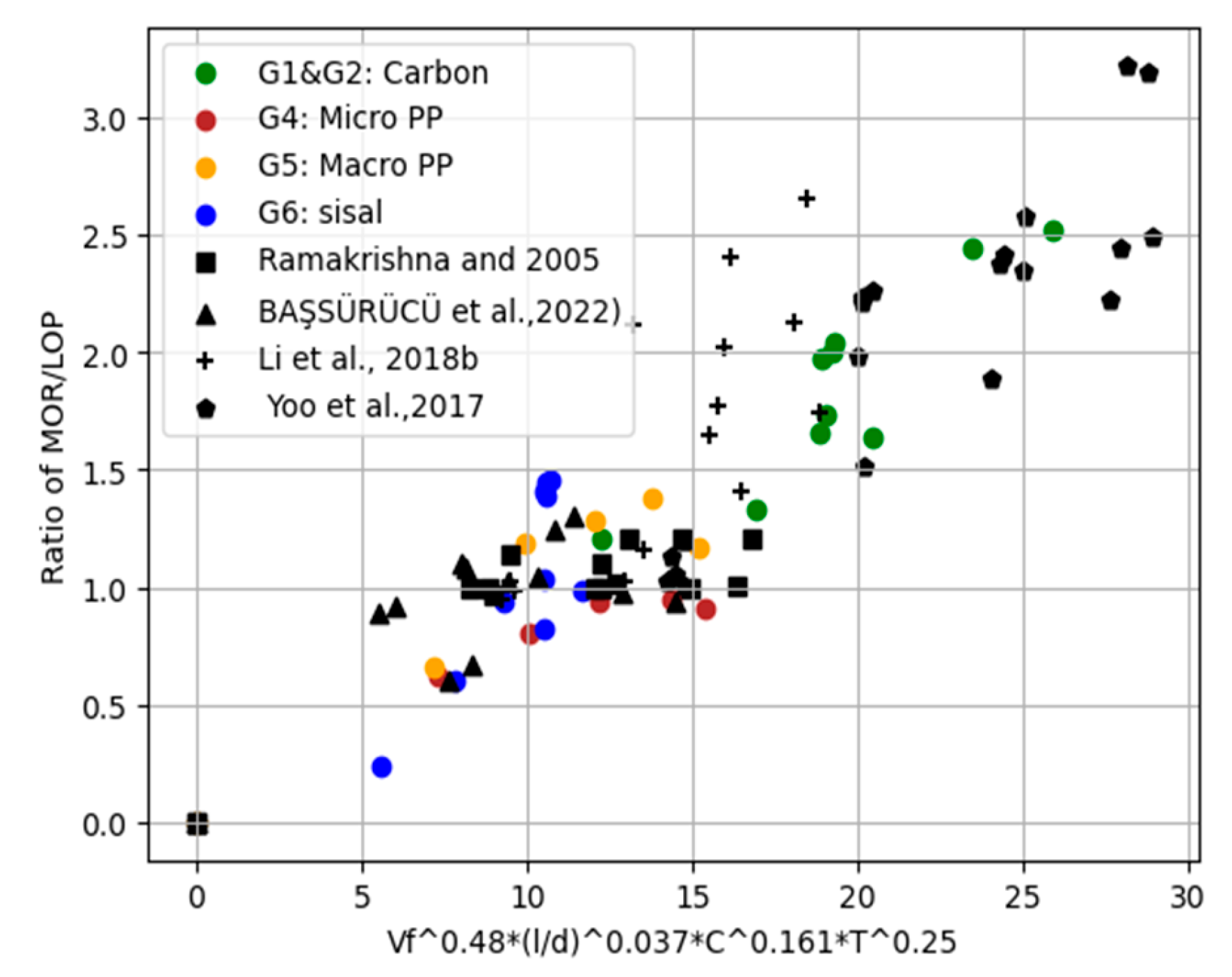
Preprints 113497 g014
Figure 15. Regression Model Fit: Comparison with Collected Data.
Figure 15. Regression Model Fit: Comparison with Collected Data.
Preprints 113497 g014
Table 1. Grading and Physical properties of the sand.
Table 1. Grading and Physical properties of the sand.
Sieve Size (mm) % Passing Physical Properties
5 100 Bulk Specific gravity (SSD): 2.69
2.36 99 Water absorption: 1.3 %
1.18 68 Moisture content (stock): 1.0 %
0.6 51 Fineness Modulus: 2.64
0.30 15
0.15 3
Table 2. Chemical composition of fly ash.
Table 2. Chemical composition of fly ash.
Oxides MgO Al2O3 SiO2 P2O5 Sulfur K2O CaO TiO2 Fe2O3 Sr Zr
Percent 3.967 25.077 60.160 0.250 0.249 0.542 3.57 0.203 5.51 0.18 0.151
Table 3. Volume fraction, aspect ratio and type pf fiber used for the preparation of the cementitious mixtures.
Table 3. Volume fraction, aspect ratio and type pf fiber used for the preparation of the cementitious mixtures.
Group No. Mix designation w/b Type of fiber Volume fraction
Vf %		 Weight /Batch
(kg/m3)		 Aspect ratio L/d
1 G1M0 0.60 None 0.0 0.00 0
G1M1 0.60 Carbon 0.41 7.47 625
G1M2 0.60 Carbon 0.82 14.94 625
G1M3 0.60 Carbon 1.23 22.41 625
G1M4 0.60 Carbon 1.66 30.29 625
G1M5 0.60 Carbon 2.06 37.59 625
2 G2M6 0.60 Carbon 1.00 18.23 1250
G2M7 0.60 Carbon 1.00 18.23 1875
G2M8 0.60 Carbon 1.00 18.23 2500
G2M9 0.60 Carbon 1.00 18.23 3125
G2M10 0.60 Carbon 1.00 18.23 3750
3 G3M11 0.60 Carbon 0.41 7.47 375
G3M12 0.60 Carbon 0.82 14.94 375
G3M13 0.60 Carbon 1.23 22.41 375
G3M14 0.60 Carbon 1.66 30.29 375
G3M15 0.60 Carbon 2.06 37.59 375
4 G4M16 0.55 Plain 0 0 0
G4M17 0.55 PP (Micro) 0.45 4.09 375
G4M18 0.55 PP (Micro) 0.91 8.28 375
G4M19 0.55 PP (Micro) 1.36 12.37 375
G4M20 0.55 PP (Micro) 1.81 16.47 375
G4M21 0.55 PP (Micro) 2.27 20.65 375
5 G5M22 0.55 Plain 0 0 0
G5M23 0.55 PP(Macro) 0.45 4.09 71
G5M24 0.55 PP(Macro) 0.91 8.28 71
G5M25 0.55 PP(Macro) 1.36 12.37 71
G5M26 0.55 PP(Macro) 1.81 16.47 71
G5M27 0.55 PP(Macro) 2.27 20.65 71
6 G6M28 0.60 Sisal 0.406 27 128
G6M29 0.60 Sisal 0.811 53 128
G6M30 0.60 Sisal 1.206 79 128
G6M31 0.60 Sisal 1.609 106 128
G6M32 0.60 Sisal 2.027 133 128
7 G6M33 0.60 Sisal 1.44 95 256
G6M34 0.60 Sisal 1.44 95 320
G6M35 0.60 Sisal 1.44 95 384
G6M36 0.60 Sisal 1.44 95 448
G6M37 0.60 Sisal 1.44 95 512
Table 4. Percentage of flow for selected mixes of fiber reinforced cementitious composites.
Table 4. Percentage of flow for selected mixes of fiber reinforced cementitious composites.
Carbon fibers Group No. G1M0 G1M1 G1M3 G1M5 G2M6 G2M7 G2M9 G2M10
% Flow 135 119 105 77 84 77 65 34
Polypropylene
Micro & Macro		 Group No. G3M12 G3M14 G3M15 G4M18 G4M19 G4M21
% Flow 133 90 77 96 82 68
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated