Prerpints.org logo
Preprint
Article

On the Strong Composition Dependence of the Martensitic Transformation Temperature and Heat in Shape Memory Alloys

Altmetrics

Downloads

62

Views

52

Comments

0

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

29 July 2024

Posted:

30 July 2024

You are already at the latest version

Alerts
Abstract
General derivation of the well-known Ren-Otsuka relation, 1/α (dTo)/dx=-α/β (where To, x, α and β(>0) are the transformation temperature, the composition, as well as the composition and temperature coefficient of the critical shear constant, c’, respectively) for shape memory alloys, SMAs, is provided based on the similarity of interatomic potentials in the framework of dimensional analysis. A new dimensionless variable, to(x)=(To (x))/(Tm (x) ), describing the phonon softening (where Tm is the melting point) is introduced. The dimensionless values of the heat of transformation, H, and entropy, S as well as the elastic constants c’, c44, A=c44/c' are universal functions of to(x) and have the same constant values at to(0) within sub-classes of host SMAs having the same type of crystal symmetry change during martensitic transformation. The ratio of (dto)/dx and α has the same constant value for all members of a given sub-class and relative increase of c’ with increasing composition should be compensated by the same decrease of to. In the generalized Ren-Otsuka relation the anisotropy factor, A appears instead of c’ and α as well as β are the differences of the corresponding coefficients for the c44 and c’ elastic constants. The obtained linear relation between h and to rationalizes the observed empirical linear relations between the heat of transformation measured by DSC (QAM) and the martensite start temperature, Ms.
Keywords: 
Subject: Physical Sciences  -   Chemical Physics

1. Introduction

It is well-know that the martensitic transformation temperature has strong composition dependence in shape memory alloys, SMAs, [1,2,3,4,5] and for instance, 1at% composition change can alter the transition temperature by about 100K. In addition, the change of the content of defects (e.g., the change of concentration of vacancies by quench) can also have a similar effect [1]. The explanation of the above effect, even after the publication of a semi-quantitative derivation in [1], is still under discussion in the literature (see e.g., [4,6,7,8,9,10,11,12,13]). In addition, it was found (see e.g., Figure 5 in [3] and Figure 5b in [4]) that the transformation heat was a common linear function of the martensite start temperature, Ms, in some different SMAs. We will also discuss this relation and will derive it.
It was shown in [1], form a Landau-type model of first order phase transformations, that the martensitic transformation, MT, occurs at a critical elastic (basal plane shear) constant, c’ (=(c11 − c12)/2) and the value of it is constant at the transformation temperature, To. Using that the elastic constant has strong composition, x, and temperature dependence, it was concluded in [1] that the constancy of c’ at To demands that the transformation temperature must exhibit an opposite effect, i.e., if c’ increases, To should decrease with increasing composition. Thus, they arrived at the requirement
c ′ x , T = c o n s t .   ,   at   T = T o .
Assuming that the composition and temperature dependence of c’ can be expressed as
c ′ x , T = c o ′ 1 + α Δ x 1 + β Δ T , )
where c o ′ is the value of c’ at To,
α = 1 c ′ d c ′ d x   and   β = 1 c ′ d c ′ d T
the following relation was obtained:
Δ T o Δ x = d T o d x = − α β   ,
or, using the definition of α,
d T o d c ′ = − 1 β C ′
It was also shown in [3] that, taking also into account that typically β =   4 % 100 K = 4 · 10 − 4 1 K [1,2,3,5,13] and α = 4 − 10 % a t % = 4 − 10   [1,11], d T o d x has a negative sign and can be given as
d T o d x = − 100 − 250 K a t % .
This means that the transformation temperature is strongly affected by even a small change in composition or by quench. Investigating the validity of predictions (3) and (4) in [1], experimental data obtained in Cu-based shape memory alloys [2,3] as well as in Ti50Ni30Cu20 alloys [11] were used, implicitly suggesting that (3) and (4) can be general predictions for all SMAs, although according to their derivation c’ and d T o d x  can have different constant values at To(0) in different alloy systems. Experimental data supported this expectation: the values of c’ and the d T o d x slopes were slightly but definitely different in different SMAs [2,3,7,14,15,16,17]. For instance it was shown in [14] that the value of c’ at To was 30% smaller in Ti50Ni30Cu20 alloy than in binary NiTi. Furthermore, it was demonstrated that in Ni2MnGa d T o d x was even positive (and α was negative) [7,15], and e.g., the value of d T o d x in TiPd-based shape memory alloys [17] with different alloying elements varied by about a factor of four (i.e., it changed between -15 K/at% and -60K/at%) by changing the type of the third alloying element. Since the softening of the corresponding elastic moduli is a key characteristics for martensitic transformations in SMAs, and not only c’, but c44 (belonging to non-basal plane shear) can also show softening, the above Ren-Otsuka relations are expected to be valid only if c’ has phonon softening behaviour and c44 is practically independent of the temperature [11,12,13,18]. This latter assumption is, in a good approximation, valid for Cu-based alloys [5,18] or for Ti50Ni30Cu20 [14] but e.g., in binary NiTi both above moduli have phonon-softening-related temperature and composition dependence [11,12,13,18] and it was concluded (see e.g., [14]) that the transformation temperature is more sensitive to the variation of c44 than to that of c’.
In this paper we provide a different, general derivation of relations of type (3) and (4), based on the law of corresponding states, LCS, for metals with phonon softening. It will be shown that the general forms of (3) or (4), which contains the composition and temperature dependence of both c’ and c44, are just the consequence of the similarity of interatomic potentials [19]. For the derivation of it one can avoid the use of phrasing like “critical value at the transition temperature” [1,4,5], or “criticality of the austenite”, which can be typical formulation for second order phase transformations [20]. This is in line with the Ren-Otsuka approach in which also no complete mode softening (if e.g., c’ → 0) is required [18] for first order phase transformations. In addition, since the explanation of the strong composition dependence of the heat of transformation, ΔH, and the linear relation between ΔH and Ms are still the question under debate [3,4,6,7,8,21] (e.g., in [21] it was concluded that composition dependence of ΔH “remains to be rationalized”), these will also be discussed.
The organization of the paper is as follows. Basic relations for the dependence of the transformation heat ( Δ H ), entropy (ΔS), the shear constants c’, c44 and the anisotropy constant, A = c 44 c ′ on t o = T o T m ( T m   is the meting point) are given in Chapter 2. The validity of the derived linearized relations between h = Δ H k T m and t o is demonstrated on the examples of binary NiTi alloy (where the concentration, x, denotes the deviation from the stoichiometric (50/50at%) composition on the Ni-rich side), in Cu74.08Al13.13Be2.79 alloys (where x shows the increase of the Be content from xBe=2.79at%), in Ti50-xNi40+xCu10 (where x changes between 0at% and 1.2 at%) as well as in Ni2MnGa alloys (where x is the Ni excess in at% from the stoichiometric composition). In Chapter 3 our predictions will be compared with other experimental data. Chapter 4 contains the conclusions.

2. Derivation of the Basic Relations

2.1. Law of Corresponding States for Phonon Softening Systems

The LCS is the consequence of similarity of interatomic potentials [19], which can be written in general as:
Φ= εf(r1/ro,…rN/ro),
where ri is the position vector of the particle i (N is the number of atoms). Its form along a given direction can be represented by a periodic function with a period of a and with an energy parameter of ε=fmax-fmin (e.g., similar to a sinus type function with wavelength λ=ao and amplitude A = ε 2 , where ao is the nearest neighbour distance). This shape is similar for all solids of the same bonding type (e.g., for metals and metallic alloys) and crystal structure, forming so-called similarity classes, i. e. f is the same function of its arguments within a similarity class.
In order to derive useful relations between different physical quantities one has to start from the fundamental theorem of dimensional analysis (“Pi theorem”) [22]. This is based on the dimensional homogeneity. It is well known that in physics there are fundamental and derived quantities. The fundamental quantities are dimensionally independent and their number, n, is finite. It is easy to show that e.g., the mass, m, the energy, ε, the length, ao, and the Boltzmann constant, k, can be taken as dimensionally independent quantities (the physical dimension of none of them can be combined from the others), forming the basis of the dimensional analysis [19,22]. According to the fundamental theorem, if Q denotes a (derived) physical quantity, then it can be given in the following form [19]:
Q= maεbaockdq(q1,…,qg-n).
here q and its variables q1,…,qg-n are dimensionless. This also means that q=Q/maεbaockd is dimensionless, i.e., the exponents a, b, c and d should be chosen such a way that the above dimensional combination of the fundamental quantities should give the dimension of Q. Furthermore, the number of independent dimensionless variables qi in principle is equal to g-n, where g is the number of variables present in the physically meaningful equation under investigation [22]. It can be shown that, neglecting quantum effects, and considering macroscopic (thermodynamic) quantities, in most of the cases the only plausible variables are the dimensionless pressure and temperature [19,23,24]:
Q= maεb aockdq(t,p),
where the dimensionless temperature and pressure are given by t=kT/ε and p=pa3/ε. Furthermore, in accordance with eqn. (6), q should be the same function for all members of the similarity class in question. It was shown that, using scaling parameters kTm ( ∝ ε ), Ω ( ∝ a o 3 ), m and k, the relations derived from (8) provided nice agreement with experimental data at p≅0 for most metals and alloys (see for instance relations for the diffusion and point defect properties [19,23,24,25]), even for binary alloys using composition dependent melting points, Tm(x), molar volume, Ω(x), and mass m(x). As it follows from (8), for the activation energy of diffusion at p ≅ 0
Q D T m = c o n s t . ,
which is the well-known thumb rule for self-diffusion in normal metals [23,25] (QD is independent of T, according to the well-known Arrhenius-type T-dependence of the diffusion coefficient). On the other hand, for bcc metals showing phonon softening behaviour in form of a curved Arrhenius functions (anomalous behaviour), introduction of one new dimensionless parameter, ξ, in the argument of QD was needed [23,26]. According to [26,27] the curved Arrhenius plot can be described by the following temperature dependence of QD:
Q D T m = Q D T m n 1 − T o ′ / T m T / T m ,
where Q D T m n is the constant obtained in normal metals and T o ′   was called in [26] as “hypothetical critical temperature”. In addition, it was shown in [26] that at a fixed (low) temperature the Q D T m ratio showed a linear dependence on the phonon softening parameter, ξ =   v T v L 111 , where ξ is the ratio of the transversal and longitudinal sound velocities along 111 direction. i.e., ξ ~ T o ′ .
Since phonon softening is a key characteristics for martensitic transformations in SMAs, let us also introduce formally this parameter, denoted by ξ too, for shape memory alloys. Thus, we will use (8) in the form
Q = m a k T m b Ω c k d q t , ξ
at atmospheric pressure ( t = T T m , p r ≅ 0 ). Accordingly, the equilibrium transformation temperature, To, can be given as
T o = T m ϑ t o , ξ ,
i.e.,
t o = ϑ t o , ξ .
(13) means that a universal relation should exist between ξ and to. Thus, T o T m can be considered as a good measure of ξ at t = t o and in the following ξ will be replaced by t o = T o T m . It is well-known that To has a strong composition dependence and thus to should also have similar behaviour.

2.2. Dependence of the Reduced Transformation Heat and Entropy on the Transformation Temperature

Let us first consider in general the to-dependence of the heat of transformation, h = Δ H k T m , and transformation entropy
Δ H k T m = h t , t o ≅ h t o ,
and
Δ S k = s t , t o ≅ s t o .
Here we used that Δ H and ΔS are usually independent of the temperature, t. Relation (14) means that h should depend universally on to only and should have the same constant value at to(0). Consequently for instance the η h = 1 h d h d t o derivative should also have the same constant value for all SMAs at to(0).
Thus, for shape memory alloys we can plot Δ H k T m versus t o = T o T m , for NiTi (from [21] with T m ≅ 1583   K ), CuAlBe alloys (from [3] with T m ≅ 1353   K ), Ni2MnGa (from [15] with T m ≅ 1403   K ) as well as for Ti50-xNi40+xCu10 (from [4] with T m ≅ 1550   K ) as it is shown in Figure 1. (In these plots Q = − Q A M ≅ Δ H and T o ≅ M s + A f 2   assumptions were implicitly assumed, where Q A M ( < 0 ) is the transformation heat measured by DSC as well as Ms and Af are the martensite start and austenite finish temperatures, respectively).
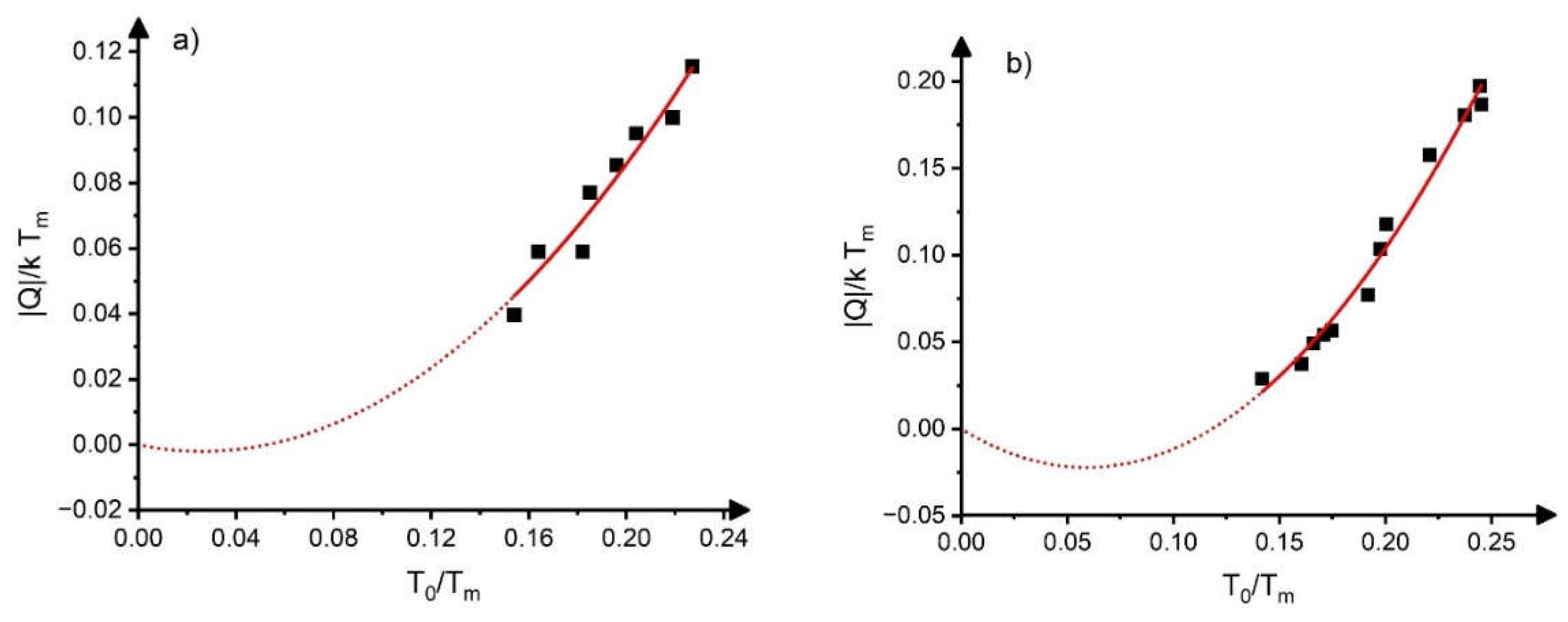
It can be seen that, these are linear functions and the slopes of Q k T m versus T o T m are 0.83, 0.16, 1.77 as well as 0.30 for NiTi, CuAlBe, Ni2MnGa and Ti50-xNi50+xCu10, respectively. Furthermore, η h t o 0 = 1 h d h d t o = T m Δ H d Δ H T m d t o = 8.0 for NiTi, 5.2 for the CuAlBe, 59   for Ni2MnGa and 4.2   for Ti50-xNi40+xCu10 systems, respectively (see also Table 1).
From the results shown in Figure 1 it can be seen that the h(to), in the investigated parameter ranges, can be well approximated by straight lines. The corresponding slopes as well as the values of ηh at to(0) (see also Table 1), although it would be expected that h has the same dependence on to for all SMAs, are characteristically different. The main difference between the above four alloys is that they have different type of symmetry change during the martensitic transformation: (B2(bcc)/B19’(monclinic), in NiTi, B2(bcc)/18R(rombohedral), in CuAlBE, L21(bcc)/tetragonal, in Ni2MnGa (where the structure of the tetragonal phase can be complex being non-modulated or modulated [7]) as well as B2(bcc)/B19(orhorombic) in Ti50-xNi40+xCu10, respectively. Thus, plausibly we have to make distinction between SMAs on the basis of the type of the symmetry change during the martensitic transformation: groups of alloys, having the same symmetry change, form different similarity sub-classes.
The above classification is also supported by the following arguments: since by definition the dimensionless transformation entropy is given by
s = Δ S k = Δ H k T o = Δ H / T m k t o ,
and the transformation entropy, as it is well-known [3], is also different for different sub-classes, i.e., it depends on the structure (symmetry) of the martensite. Furthermore, the fact that Δ H k T m is approximately a linear function of T o T m would dictate that the reduced entropy, s, should be independent of to (and thus from x) within a sub-class, and has different constant values for different sub-classes. In addition the function h(to) should go through the origin.
It can be seen that it is quite well fulfilled for CuAlBe and Ti50-xNi40+xCu10, where the Q k T m versus T o T m function goes through the origin, while the linear extrapolation of the fitted straight lines have definite intercept values for binary NiTi and binary Ni2MnGa alloys. In the case CuAlBe and Ti50-xNi40+xCu10 alloys it also means that the slopes in Figure 1 should be equal to the (constant) entropy as calculated from the DSC data at to(0): Δ S e x p k = 0.15   and Δ S e x p k = 0.30 . It can be seen the DSC data and the above slopes indeed agree very well (the slopes are 0.16 as well as 0.30 , respectively). In addition the experimental data of [3,4] confirm that s is an indeed constant, i.e., independent of to, in these alloys and se≅s (see also the Appendix A). In the case of NiTi and Ni2MnGa the slopes provide different values than those of the experimental values of the entropies (calculated as Δ S e x p = Q A → M + Q M → A 2 T o ) at to(0) (see also the data in Table 1). In addition, the s e = Δ S e x p k values show approximately a linear dependence on to as it is illustrated in Figure 2. Thus, both the linear dependence of s e and the different slopes of the Q k T m versus to plots from s e t o 0 can be related to i) the se(to) function is not constant, but linear function of to ( s e = s e o + β s ( t o − t o 0 ) and/or ii) the approximations used when the Q k T m versus t o is plotted instead of Δ H k T m versus t o . As it is discussed in the Appendix A i) has the dominating effect and Q k T m is a quadratic function of to and the slope of the fitted linear relation between Q k T m and t o in a certain interval, is given approximately by s e t o 0 + t o 0 β s = s e o +   t o 0 β s .
Since h has composition dependence via its universal to-dependence a general relation should exist between its composition derivative and d t o d x :
1 h d h d x = 1 h d h d t o d t o d x = η h d t o d x .
According to this the universal constants η h can also be calculated from the ratio of the slopes giving the composition dependence of h and to (see also Table 1).
It is worth emphasizing that the found linear universal dependence of h on to can also explain that even if both Δ H and To has non-linear dependence on x, Δ H versus To can be linear (see e.g., Figure 2a and 2b in [4] for binary NiTi where the x-dependence of both Ms and Δ H is non-linear, but Δ H versus Ms is a linear function. (Regarding the validity of assumption Ms≅To, see our comments in Chapter 3.)
Before considering the corresponding expressions for the reduced (dimensionless) c’, c44 and A it is worth recalling that besides the Boltzmann constant the other three scaling parameters can also have composition dependence. But it can be easily accepted that the composition dependence of m, Ω and Tm is week as compared to the composition dependence of To in SMAs. Indeed in shape memory host alloys the value of the atomic mass and volume is expected to have only a few percent change upon alloying. Furthermore, for the composition dependence of Tm we can take as examples of binary NiTi system as well as the CuAlBe systems, in which 1 T o d T o d x ≅ − 0.278 1 a t % = − 27 , 8 and 1 T m d T m d x = − 1 1583 K 21 K 1 a t % = − 0 , 013 1 a t % = − 1.13 [21] as well as 1 T o d T o d x ≅ − 21.7 and 1 T m d T m d x ≅ 0   [3]. Thus, for the sake of simplicity in the following only To will be considered composition dependent.

2.3. Derivation of General Relation for the Composition Dependence of the Transformation Temperature

Let us consider the elastic constant, c’ given in the following form:
c ′ = k T m Ω γ c ′ t , t o x .
where γ c ′ is universal function of t and to
γ c ′ t , t o x
and at t=to
γ c ′ t = t o , t o x = c o n s t .
This corresponds to relation (1) in dimensionless form and the to-dependence is the consequence of the phonon softening, with an additional conclusion that the constant is expected to be the same within a sub-class but different in different sub-classes. From (19) (using that 1 γ c ′ d γ c ′ d t o = 1 c ′ d c ′ d t o and 1 γ c ′ ∂ γ c ′ ∂ x = 1 c ′ d c ′ d x for composition independent Tm and Ω)
1 c ′ d c ′ d t o = 1 c ′ d c ′ d x d x d t o
According to (19), in the γ c ′ = c o n s t . condition the constant value is the limit of the bilinear function of γ c ′ t , x taken at t=to(0). Introducing   t ′ = t − t o , as a new variable in the vicinity of to, the to-dependence stems from the t’-dependence and it is given by
1 c ′ d c ′ d t o = 1 c ′ d c ′ d t d t d t ′ d t ′ d t o = − 1 c ′ d c ′ d t
Combination of (21) and (22) leads to
d t o d x = − α T m β .
This is just the relation (3) and, by the same arguments, which were used in [1], relation (4) is also valid, i.e.,
d T o d c ′ = − 1 β c ′ .
In addition, η c ′ = 1 γ c ′ d γ c ′ d t o = 1 c ′ d c ′ d t o , should have the same constant value at to(0) within a sub-class, i.e.,
η c ′ = 1 c ′ d c ′ d t o = − 1 c ′ d c ′ d t = − β T m .
Thus, the value of η c ′ can be calculated from experimental data on β and Tm (see also Table 3). In addition from (23)
1 α d t o d x = − 1 β T m = 1 η c ′ ,
and, since the right hand side is the same negative constant value within a sub-class, we get that the ratio of slopes expressing the composition dependence of to and c’ is constant, i.e., a relative increase of c’ with increasing composition should be compensated by the same relative decrease of to (β>0). This is a more quantitative statement than simply saying: the lower α the higher d T o d x is (see [1]).
As we summarized in the introduction, eqn. (4) and thus (24) can be valid only if c’ shows phonon softening while c44 does not. The same results as above can be obtained by replacing c’ with c44 (with β describing now the phonon-softening-caused temperature dependence of c44): in this case the softening of c’ should be neglected. This conclusion is in line with the comments of [12], where it was mentioned that in (24) “…c can be either c44 or c’….”.
Following the same procedure as above for c’ we can write for the dimensionless anisotropy factor, A
A = γ A t ,   t o x ,
and
T m A d A d T o = η A ,
respectively ( η A = 1 A d A d t o ), and η A and A = γ A are constants at t o 0 . Thus, (see also (21) and (22)) finally
d t o d x = − α T m β ,
or
d t o α d x = − 1 T m β = c o n s t .
is obtained where
α = α c 4 − α c ′ = 1 c 44 d c 44 d x − 1 c ′ d c ′ d x
and
β = β c 4 − β c ′ = 1 c 44 d c 44 d T − 1 c ′ d c ′ d T .
(29) is the generalized Ren-Otsuka relation with generalized α and β. If the composition and temperature dependence of c44 can neglected (or normal, i.e., it does not show phonon softening and its contribution to the martensitic transformation can be neglected) then one gets back the original relation (3). For phonon softening systems the temperature coefficient contains two contributions: the positive one describes the direct phonon softening contribution while the small, usually negligible, negative one is related to the usual (normal) anharmonicity-related softening of the crystal and the first one dominates in the vicinity of To. It can be noted that the anharmonicity-related temperature and composition dependence was also neglected in [1]. Furthermore, if both c’ and c44 have phonon softening in the above difference the “normal” anharmonicity-related contributions approximately cancel out.
It is worth mentioning that the above approach, namely that the constancy of the anisotropy constant is the best starting point for finding generalized relation on the composition dependence of To, can also be confirmed from the general form of the Landau expansion of the free energy, F, as used in [18]. This paper, instead of using only two strains (basal plane shear, e1, basal plane suffle, η in eqn. (1) of [1]), contained three ones: e1, η, and the 001 1 1 ¯ 0 non-basal plane shear, e2 (and c’, ω η 2 as well as c44 are the corresponding energy terms, respectively). Now it is easy to show that minimizing F with respect to both e2 and η strains one can get, besides the condition (1) (and ω η 2 T , x = c o n t s . : see eqns. (7a and b) in [1]), that c44(T,x) has to be constant too at T=To. Now, the ratio of eqn. (1) and c44(T,x)=const. gives that A=γA=const. at to(0) (see eqn. (27)). Of course if there is no phonon softening in c44 (i.e., if its to-dependence can be neglected) then only the phonon softening of c’ occurs and the original Ren-Otsuka relation can be approximately valid (like to the case of the Ti50-xNi40+xCu10 or CuAlBe alloy with large and increasing anisotropy by approaching to To). On the other hand the NiTi alloys represent the other limit, when both c’ and c44 shows phonon softening with small value of A and decreasing tendency of A with approaching to To [18] (i.e.,  η A > 0 , see also Table 1 and the discussion below).

3. Comparison with Experimental Data

Some general features of the to-dependence of the reduced characteristic quantities were already analysed in the previous chapter and it led to the conclusion that the host SMAs can be divided into sub-classes (having the same type of symmetry change during MT) within which the above quantities have the same constant values at to(0). In this chapter, besides summarizing these, we also consider other SMAs to support the conclusions based on data analysed in Chapter 2.2. Furthermore, the reduced values of c’, c44 and A will be collected and compared with the data available in the literature.
It has to be noted that in many publications the composition dependence of To and Ms is taken to be the same, and similarly to [1], we can also assume it here. Ms can be given as M s = T o − d o + e o − Δ s c , where do and eo denote the first derivatives of the dissipative and elastic energies per unit volume, during the cooling process at the beginning of the transformation and Δ s c is the entropy change per unit volume during cooling [29]. The second term in Ms is in fact determines the dissipation and elastic energy accumulation, i.e., the above assumption means that we neglect the composition dependence of these terms, although in a more refined treatment this should be necessary to take into account, since e.g., according to [8,9] the dissipative energy (the integral of do) also shows a composition dependence. It is also worth mentioning that in general the x-dependence of To(x) and the transformation heat, ΔH(x) are not strictly linear, but have a small downward curvature [8,21,30], but for the sake of simplicity we neglect this moderate x-dependence of the slopes. Furthermore, it is also worth emphasizing that, in the light of the results obtained in the previous chapter it is indeed not expected that the values of the slopes d t o d x = 1 T m d T o d x should have the same value even within a given sub-class: while according to equation (26) 1 α d t o d x quantity should have the same value (see also Table 3 below).
Table 1 contains the values of those dimensionless constants at to(0) which are predicted to be the same within a given subclass; h, ηh, s, γ c ′ , γ c 44 ,   A≡γA and η A = 1 A d A d t o = T m A d A d T . Table 2 contains the most important input parameters (Tm, T o T m , c’, c44, βc’, βc44,αc’,αc44 and   1 T m d T o d x ) used in the calculation of data given in Table 1. It has to be noted that that most of the experimental data suffer from relatively large errors for the elastic parameters (typically between 15-25%), and there is a lack of reliable data especially for the composition dependence of c’ and c44. Table 3 shows the estimated parameters related to the composition dependence of the transformation temperature.
Table 1 contains the summary of the parameters predicted to be the same within the five sub-classes, represented by the NiTi, Ti45-xNi50+xCu5, Ti50-xNi40+xCu10, Ni2MnGa, Cu-Al-Be, CuZn, CuZnAl as well as Cu68Al28Ni4 alloys. It can be seen that indeed the estimated values are characteristically different for the sub-classes. Furthermore, data for Cu-based alloys with B2/18R transformation (6th, 7th and 8th rows) are rather similar demonstrating that these quantities have the same constant values within a certain sub-class, as predicted. Regarding the CuAlNi (with B2/2H transformation) the constants are also not much different from the values of the above Cu-based alloys, suggesting that these two sub-classes behave similarly. On the other hand, the observation concluded in [3] support that the CuAlNi belongs to a different sub-class. In Figure 5 of [3], where the transformation heat ( Δ H A → M ) was plotted versus the Ms temperature, the slope of the straight lines were slightly, but definitely different for CuAlNi (1.59 J/molK) from the common slope belonging to the fitted line on data of CuAlBe and CuZnAl (1.30 J/molK; see also Figure 3 below). From the above slopes η h = T m Δ H d Δ H d T o ≅ T m Δ H d Δ H d M s 5.2 and 4.8, respectively. It can be seen, as expected, that 5.2 is in a very good agreement with the value given in Table 1 for CuAlBe from eqn. (17) (4.9), while for CuAlNi the agreement is still acceptable (from eqn. (17) 3.9 was obtained). While this example illustrates also the experimental scatter (which is still in the range of the differences between 5.2 and 4.9 as well as 4.8 and 3.9), since the difference of the above slopes obtained on the basis of large number of experimental data collected in [3] was definite, one can confirm that the CuAlNi belongs to a different sub-class.
Interestingly in Ni2MnGa the sign of both α as well as of 1 T m d T o d x are even negative (see Table 2) and thus the relations (4) and (5) (as it was also mentioned in [7]) remain valid, since the sign of β is still the same as for other phonon softening alloys (i.e., it is positive).
One additional comment supporting that bcc metals (and alloys), with phonon softening, behave differently than the “normal” metals can be made. According to the above results βTm should be universal constant (at t=1, i.e., at Tm) for all “normal” metals while its value can be different for phonon softening systems and in addition it should be different for different sub-classes of SMAs. The most salient result is that indeed β>0, belonging to phonon softening elastic constants in SMAs. On the other hand, it is negative e.g., for Ag, Au and Cu [41] (and βTm is approximately constant for temperatures larger than the Debye temperature for all “normal” metals [40]: ~ − 0.56 ).
Table 3 contains the comparison of the predicted values of η A − 1 = 1 α T m d T o d x as well as η A − 1 = − 1 β T m (columns 4th and 5th), as calculated at To(0) from the experimental data given in Table 2. It can be seen that the signs in all cases are correct. Note, (as it is also mentioned in the caption of Table 3) that for NiTi only the generalized relation provides the correct sign. Furthermore, as it can be seen from columns 5th and 6th, the agreement between the value of − α β and the experimental data for d T o d x is also satisfactory, taking into account the uncertainties of the experimental values of α and β at present. As an example we can mention the case of CuAlBe alloys. Here the temperature dependence of the elastic constants is well known (as it is also shown in Table 2) and this slope does not change with the composition [2]. On the other hand the composition dependence of c’ and c44 at room temperature and at the transformation temperature (see Figure 2 in [3]) is remarkably different (αc’ is about 10 at room temperature and about 0.7 at To or the composition dependence of A is given by η A =-9.3 as well as -1.0, respectively). In the Tables above the room temperature value of αc’ was taken, since the reported value at To would lead to about an order of magnitude smaller value, although a value between 0.7 and 10 (and closer to 10) would lead a better agreement (αc’≅6.7 would lead to exact agreement between − α β and the experimental d T o d x value).
Finally it is also worth adding that the constancy of 1 α T m d T o d x provides an explanation of the conjecture proposed already in 1988 by Verlinder and Delaey [45]: “the Ms temperatures of all the alloys can be correlated with an expression similar to that given for the composition dependence of c’… ” i.e.,  1 T m d T o d x ~ α . In addition they expressed that “similar calculations and conclusions as those presented in this paper for the two observations concerning the composition dependence of c’ and Ms could be made for the other alloy systems, providing the necessary experimental data are available.”
Finally, since on the Q k T m versus to plots the intercepts I, depends also on the position of the fitted to-interval for systems in which the entropy has a linear to-dependence and thus Q k T m versus to is quadratic function (see the Appendix A), it would be worth to compile these plots in a common plot of Q k T m − I versus to. Furthermore, since the Q k T m versus Ms plots are more commonly used in the analysis of experimental data (see e.g., [3,4]), Figure 3a and 3b show the Q k T m versus to as well as Q k T m − I versus M s T m plots. It can be seen in the compiled plots in Figure 3b that the only difference between the straight lines is that their slopes are different for different sub-classes of SMAs. Thus, this is a nice illustration of our prediction that the slopes the Q k T m versus to plots should be different for SMAs with different symmetry changes during MTs. It is so even if one takes into account that
i) in those systems where the entropy has an intrinsic to-dependence the slopes differ from the s e t o 0 = Δ S e x p k ≅ Q k T o values (shown in the fifth column of Table 1), and ii) the slopes of Q k T m − I versus M s T m are obviously slightly different from those of the Q k T m − I versus to plots (for instance the slopes are 1.11 as well 0.83 in NiTi or 0.160 and 0.156 in CuAlBe, respectively).

4. Conclusions

-
It is shown that the application of the law of corresponding states for martensitic transformations of shape memory alloys with phonon softening requires the introduction of a new dimensionless phonon softening parameter, which is proportional to t o = T o T m .
-
Both the dimensionless heat and entropy of transformation, ( h = Δ H k T m and s = Δ S k ) are universal functions of to, and the composition dependence of them are determined by the composition dependence of to (or To, since the composition dependence of Tm can be neglected).
-
The slopes of the linearized h versus to plots were different for SMAs with different symmetry changes during martensitic transformation forming sub-classes.
-
Within a given sub-class the normalized parameters like the c’ elastic constant or the anisotropy constant ( γ = c ′ Ω k T m   a n d   A = c 44 c ′ ) are the same constants at T o T m = t o 0 ,
-
From the above property of A the generalized Ren-Otsuka relation is obtained with generalized α and β parameters ( α = α c 4 − α c ′ = 1 c 44 d c 44 d x − 1 c ′ d c ′ d x as well as β = β c 4 − β c ′ = 1 c 44 d c 44 d T − 1 c ′ d c ′ d T , respectively, where these are different from zero only for parameters showing phonon softening).
-
It is shown that 1 α d t o d x is the same constant within a given sub-class.
-
The obtained linear relation between Δ H and To rationalizes the observed empirical linear relations between the heat of transformation measured by DSC (QA→M) and the martensite start temperature, Ms.

Author Contributions

“Conceptualization, D.L. Beke; methodology, D.L. Beke, A.A. Azim; validation, D.L. Beke; formal analysis, A.A. Azim: data curation, A.A. Azim; writing—original draft preparation, D.L. Beke; writing—review and editing, D.L. Beke; visualization, A.A. Azim.; supervision, D.L. Beke; project administration, D.L. Beke.; funding acquisition, D.L. Beke. All authors have read and agreed to the published version of the manuscript.”

Funding

Please add: “This research received no external funding”

Institutional Review Board Statement

“Not applicable”.

Informed Consent Statement

“Not applicable.”

Data Availability Statement

Data will be available upon request..

Conflicts of Interest

“The authors declare no conflicts of interest.”

Appendix A The Slope and Intercept of Q k T m Versus to Plots

The heats of transformation measured by a calorimeter for cooling, as well as heating (A→M, and M→A, respectively) contains additional terms arising from elastic energy accumulation and dissipation losses (non-chemical energy terms) [3,29]:
Q = − Q A M = − Δ H A M − E A M − D A M = Δ H − E − D ,
and
Q M A = Δ H M A − E A M + D A M = Δ H − E + D .
Here Δ H = − Δ H A M = Δ H M A 0 is the transformation enthalpy and E A M = − E M A = E > 0 and D(>0) denotes the elastic and dissipative energy, assuming that D is the same in both directions and that the same elastic energy is released during heating what was accumulated during cooling (which explains the negative sign in (A2)). Thus, using the Tong-Wayman approximation ( T o ≅ M s + A f 2 ) we can write from (A1),
Q k T m = Δ H k T m − E + D k T m = s t o − E + D k T m ,
where the definition of the transformation entropy, s = Δ S k = Δ H k T o , was also used. Furthermore, the experimental values of s are calculated from the relation s e = Δ S e x p k ≅ Q k T o as
s e = Δ S e x p k ≅ Q k T o ≅ s − E + D k T o .
Combination of (A3) and (A4) gives
Q k T m = s e t o .
Thus, for the slopes of Q k T m versus to as well as of se versus to we get
d Q k T m d t o = s e ,
and
d s e d t o = 0 ,
if se is constant.
We have seen in the main text that for CuAlBe and Ti50-xNi40+xCu10 alloys se was indeed independent of to, as well as Q k T m versus to was linear and went through the origin and s e ≅ d Q k T m d t o . In addition, since E + D k T m is typically not larger than about 10-20% of Δ H k T m and, using data from Table 1 in the main text Δ H k T m ≅ 0.09 in Ti50-xNi40+xCu10 as well as 0.03 in CuAlBE and thus E + D k T m ≅ 0.018 − 0.009 as well as 0.002-0.001, respectively (while s e = 0.3   and 0.15, respectively). Thus, se≅ s holds too.
For the NiTi and Ni2MnGa systems the condition (A7) did not fulfil and Table A1 shows the corresponding values of the slopes and the intercepts of the Q k T m versus to as well as s e versus to plots. In these alloys there is a well-defined linear dependence of se on to, which can be attributed to other than vibrational contributions (which are fully related to the symmetry change during the martensitic transformation [3,15]) and for instance in [15] the above dependence was interpreted by magnetic contribution to se in Ni2MnGa. Thus, we can write for such an intrinsic dependence
s e = s e o + β s t o − t o o = s e o − β s t o o + β s t o ,
where s e o = s e t o 0 and t o o = t o 0 are constants. Putting (A8) into (A5) we get
Q k T m = t o s e o + β s t o − t o o = s e o − β s t o o t o + β s t o 2 .
and thus
d Q k T m d t o = s e o − β s t o o + 2 β s t _ o = s e o + β s 2 t _ o − t o o ,
where t _ o is the median value of the interval where the linear fit was made to the Q k T m versus to plot. It can be seen from (A9) that Q k T m is a quadratic function of to as it is illustrated in Fig. A1 and the linear fit is made in the certain to interval with to median value (to≅0.20 and 0.21 for NiTi as well as Ni2MnGa, respectively). Thus, the intercepts of the Q k T m versus to have no physical meaning (and depend on the position of the fitted range). In contrast, the slopes of the Q k T m versus to as well as s e versus to plots are relevant parameters and as one can check it from the data collected in Table A1 they form a consistent set, in accordance with eqns. (A8) and (A9).
Table A1. Slope and intercepts of the Q k T m versus to as well as s e versus to plots for NiTi and Ni2MnGa alloys.
Table A1. Slope and intercepts of the Q k T m versus to as well as s e versus to plots for NiTi and Ni2MnGa alloys.
Alloy slope of Q k T m versus to intercept of Q k T m versus to slope of se versus to intercept of se versus to s e o = ≅ Q k T o t o 0 = t o o t _ o
NiTi 0.83±0.10 -0.08±0.02 3.0±0.7 -0.11±0.13 0.50 0.23 0.20
Ni2MnGa 1.78±0.10 -0.25±0.02 6.4±0.5 -1.1±0.1 0.20 0,15 0.21
Preprints 113601 g0a1
Figure A1. Q k T m versus to plots for NiTi (a) and Ni2MnGa (b). The fitted curves show the fit with eqn. (A9) and both fitting parameters are in very good agreement with data given in Table A1 (obtained from the linear of se versus to): s e o − β s t o o = − 0.15 and s e o − β s t o o = − 0.75 as well as β s = 2.9 and β s = 6.4 , for NiTi and Ni2MnGa systems, respectively.
Figure A1. Q k T m versus to plots for NiTi (a) and Ni2MnGa (b). The fitted curves show the fit with eqn. (A9) and both fitting parameters are in very good agreement with data given in Table A1 (obtained from the linear of se versus to): s e o − β s t o o = − 0.15 and s e o − β s t o o = − 0.75 as well as β s = 2.9 and β s = 6.4 , for NiTi and Ni2MnGa systems, respectively.
Preprints 113601 g0a1

References

  1. X. Ren, K. Otsuka, (2000) Why does the Martensitic Transformation temperature strongly depends on composition, Mat. Sci. Forum, 327-328:429-432. [CrossRef]
  2. LI. Manosa, M. Jorado, A. Planes, J. Zaretsky, T. Lograsso, C. Stassis (1994) Elastic constants of bcc Cu–Al–Ni alloys, Phys. Rev. 49B:9969-72. [CrossRef]
  3. Planes, LI. Manosa, D. Rios-Jara, J. Ortin, (1992) Martensitic transformation of Cu-based shape-memory alloys: Elastic anisotropy and entropy change, Phys. Rev. 45B:7633-39. [CrossRef]
  4. J. Frenzel, A Wieczorek, J, Opahle, R. Dantz, G. Eggeler, (2015) On the effect of alloy composition on martensite start temperatures and latent heats in Ni–Ti-based shape memory alloys, Acta Mater 90:213-23. [CrossRef]
  5. K. Otsuka, X. Ren, (2005) Physical metallurgy of Ti–Ni-based shape memory alloys, Prog. Mater. Sci. 50:511-678. [CrossRef]
  6. T. Chakraborty, J. Rogal, R. Drautz, (2016) Unraveling the composition dependence of the martensitic transformation temperature: A first-principles study of Ti-Ta alloys, Phys. Rev. B 94:224104-1-9. [CrossRef]
  7. Ch. Li, H. Luo, Q. Hu, R. Yang, B. Johansson, L. Vitos, (2010) First-principles investigation of the composition dependent properties of Ni2+xMn1−xGa shape-memory alloys, Phys. Rev. B82: 024201-1-9. [CrossRef]
  8. N. Resnina, S. Belyayev, A. Sibirev, I. Ponikariva, A. Ivanov, R. Bikhaev, T. Rebrov, M. Starodubova, S. Berezovskaya, M. Kalitskaya, A. Bazlov, V. Andreev, V. Kalganov, (2023) The influence of the chemical composition of the Ti-Hf-Zr-Ni-Cu-Co shape memory alloys on the structure and the martensitic transformations, J. of Alloys and Comp. 968:172040-1-14. [CrossRef]
  9. S. Vedamanickam, P. Vageeswaran, B. Jacob, (2023) Theoretical analysis and design of Ti-based shape memory alloys correlating composition and electronic properties to transformation temperatures for high temperature applications, MaT. Sci. & Eng. B296:116681-1-14. [CrossRef]
  10. Z. Wu, J.W. Lawson, O. Benafan, (2023) Origin of the asymmetry in martensitic phase transitions in off-stoichiometric NiTi near equiatomic compositions, Phys. Rev. 108:L140103. [CrossRef]
  11. J.M. Lu, Q.M. Hu, R. Yang, (2008) First-principles investigations of point defect behaviour and elastic properties of Ti-Ni Alloys, Mater.Res. Soc. Simp. Proc. 1128:U09-03. [CrossRef]
  12. J.M. Lu, Q.M. Hu, R. Yang, (2008) Composition-dependent elastic properties and electronic structures of off-stoichiometric TiNi from first-principles calculations, Acta Mater. 56:4913-20. [CrossRef]
  13. X. Lin, X.Q. Tu, B.Q. Liu, J. M. Song, W. Luo, Y. Lei, G.A. Sun, B. Chen, Q.M. Hu, (2017) Composition-dependent elastic properties in TiNi-Nb from first principle calculations, J of All. and Comp. 706:260-266. [CrossRef]
  14. X. Ren, K. Taniwaki, K. Otsuka, T. Suzuki, K. Tanaka, Yu.I. Chumljakov, T. Ueki, (1999) Elastic constants of Ti50Ni30Cu20 alloy prior to martensitic transformation, Phil. Mag. A79:31-41. [CrossRef]
  15. V.V. Khovailo, K. Oikawa, T. Abe, T. Takagi, (2003) Entropy change at the martensitic transformation in ferromagnetic shape memory alloys Ni2+ xMn1− xGa, J. of Appl. Phys. 93:8483-8485. [CrossRef]
  16. V.V. Khovailo, V.D. Buchelnikov, R. Kainuma, V.V. Koledov, M. Ohtsuka, V.G. Shvarov, T. Takagi, S.V. Taskaev, A.N. Vasiljev, (2005) Phase transitions in Ni2+xMn1-xGa with a high Ni excess Phys. Rev. B72 (2005):224408-1-10. [CrossRef]
  17. H. Hosoda. K. Enami, A. Kamio, K, Inoune, (1996) Alloys design of PdTi-based shape memory alloys based on defect structures and site preference of ternary elements, J of Intelligent Materials Systems and Structures 7:312-320. [CrossRef]
  18. X. Ren, K. Otsuka, (1998) The role of softening in elastic constants c44 in martensitic transformations, Scripta Mater., 38: 1669-75. [CrossRef]
  19. D.L. Beke, G. Erdélyi, F.J. Kedves, (1981) The law of corresponding states for metals, J. Phys. Chem. Solids, 42:163-170. [CrossRef]
  20. J.A. Krumhansl, (1992) Landau models for structural phase transitions: Are soft modes needed? Sol. Stat. Comm. 84:251-254. [CrossRef]
  21. J. Frenzel, E.P. George, A. Dlouhy, C.H. Somsen, M.F.-X. Wagner, G. Eggeler (2010) Influence of Ni on martensitic phase transformations in NiTi shape memory alloys, Acta Mat. 58:3444–3458. [CrossRef]
  22. C.M. Focken: Dimensional Methods and Their Applications, Erward Arnold, and Co. London, 1953.
  23. D.L. Beke, (1989) Theoretical background of empirical laws for diffusion, Defect and Diffusion Forum Vols. 66-69:127-156. [CrossRef]
  24. D.L. Beke, “Tracer Diffusion in Homogeneous and Heterogeneous Alloys” in “Diffusion in Solids. Unsolved Problems” (ed. G. E Murch), Trans.Tec. Publ. Ltd. Zurich (1992) p. 31-53.
  25. D. LeClaire: Diffusion in Body-Centered Cubic Metals, ed. by J. A. Wheeler, Jr. and F. R. Winslow, Am. Soc. Met., Metals Park, Ohio, (1965), 3.
  26. Herzig, Ch., Köhler, U., (1987) Anomalous Self-Diffusion in BCC IVB Metals and Alloys, Materials Science Forum 15-18:301-322. [CrossRef]
  27. J.M. Sanchez, D. de Fontaine, (1975) Model for Anomalous Self-Diffusion in Group-IVB Transition Metals Phys. Rev. Letters., 35:227-230. [CrossRef]
  28. X. Ren, N. Miura, J. Zhang, K. Otsuka, K. Tanaka, M. Koiwa, T. Suzuki. Yu.I. Chumljakov, M. Asahi, (2001) A comparative study of elastic constants of Ti–Ni-based alloys prior to martensitic transformationMat. Sci. and Eng. A312:196-206. [CrossRef]
  29. D.L. Beke, L. Daróczi, N.M. Samy, L.Z. Tóth, M.K. Bolgár, (2020) On the thermodynamic analysis of martensite stabilization treatments, Acta Mater. 200:490-501. [CrossRef]
  30. K. Niitsu, Y. Kimura, X. Xu, R. Ksinuma, (2015) Composition dependences of entropy change and transformation temperatures in Ni-rich Ti–Ni system, Shap. Mem. Superelasticity 1:124-131. [CrossRef]
  31. WS. Ko, S. B. Maisel, B. Grabowski, J. B. Jeon, J. Neugebauer, (2017) Atomic scale processes of phase transformations in nanocrystalline NiTi shape-memory alloys, Acta Materialia 123:90-101. [CrossRef]
  32. P. Sedlák, M. Janovská, L. Bodnárová, O. Heczko, H. Seiner, (2020) Softening of Shear Elastic Coefficients in Shape Memory Alloys Near the Martensitic Transition: A Study by Laser-Based Resonant Ultrasound Spectroscopy, Metals, 10:1383-1-13. [CrossRef]
  33. Mercier, K.N. Melton, G, Gremaud, J. Hagi, (1980) Single-crystal elastic constants of the equiatomic NiTi alloy near the martensitic transformation, J. appl. Phys., 51:1833-34. [CrossRef]
  34. C-M. Li, H-B. Luo, Q-M. Hu, R. Yang, B. Johansson, L. Vitos, (2011) Site preference and elastic properties of Fe-, Co-, and Cu-doped NiMnGa shape memory alloys from first principles, Phys. Rev. B84:024206-1-10. [CrossRef]
  35. P. Sedlák, M. Janovská, P. Bodnarová, O. Heczko, (2020) Softening of shear elastic coefficients in shape memory alloys near the martensitic transition: A study by laser-based resonant ultrasound spectroscopyMaterials, Mat. 10:1383-1-13. [CrossRef]
  36. P. Zhao, L. Dai, L. Cullen, M. Wuttig, (2007) Magnetic and Elastic Properties of Ni49.0 Mn23.5 Ga27.5 Premartensite, Metallurgical and Materials Transactions 38A:745-751. [CrossRef]
  37. W. Arneodo, M. Ahlers, (1974) The martensitic transformation in β Cu-Zn, Acta Met. 22:1475-1480. [CrossRef]
  38. M. Ahlers, (1986), Martensite and equilibrium phases in Cu-Zn and Cu-Zn-Al alloys, Progress in Mat. Sci. 30:135-186. [CrossRef]
  39. Planes, R. Romero, M. Ahlers, (1990) The martensitic transition temperature in ternary Cu-Zn-Al alloys. Influence of the L21 structure, Acta Met. Mat. 38:757-763. [CrossRef]
  40. G.Guenin, M. Morin, P.T. Gobin, W. Dejonghe, L. Delaey, (1977) Elastic constant measurements in β Cu-Zn-Al near the martensitic transformation temperature, Scripta Mat. 11:1071-1075. [CrossRef]
  41. V, Recarte, J.I. Perez-Lanadazábal, M,I, Nó, J. San Juan, (2004) Study by resonant ultrasound spectroscopy of the elastic constants of the β phase in Cu-Al-Ni shape memory alloys, Mat. Sci. Eng. A 70:488-491. [CrossRef]
  42. B. Verlinder, L. Delaey, (1988) On the elastic constants and Ms-temperatures in β-Hume-Rothery alloys, Acta Met. 36:1771-1779. [CrossRef]
  43. T. Castan. A. PLanes, (1988) Elastic constants of bcc shape-memory binary alloys: Effect of the configurational ordering, Phys. Rev B 38:7959-7965. [CrossRef]
  44. Y.A. Chang, L. Himmel, (1966) Temperature dependence of the elastic constants of Cu, Ag, and Au above room temperature, J. of Appl Phys. 37:3567–3572. [CrossRef]
  45. B. Verlinder, L. Delaey, (1988) Beta-Phase stability and martensitic nucleation in hume-rothery alloys, Metallurgical Trans. 19A:207-216. [CrossRef]
Preprints 113601 g001aPreprints 113601 g001b
Figure 1. Q k T m versus T o T m in binary NiTi (a), in CuAlBe (b) in Ni2MnGa alloys (c) as well as in Ti50-xNi40+xCu10 (d) (on the basis of data published in [3,4,15,21], respectively. The slopes, are 0.83, 0.16, 1.77 as well as 0.30, respectively.
Figure 1. Q k T m versus T o T m in binary NiTi (a), in CuAlBe (b) in Ni2MnGa alloys (c) as well as in Ti50-xNi40+xCu10 (d) (on the basis of data published in [3,4,15,21], respectively. The slopes, are 0.83, 0.16, 1.77 as well as 0.30, respectively.
Preprints 113601 g001aPreprints 113601 g001b
Preprints 113601 g002
Figure 2. Δ S k versus T o T m in binary NiTi alloys [21] (a) and in Ni2MnGa alloys (b) [15].The slopes, d Δ S k d t o   , are 3.0 and 6.4, respectively.
Figure 2. Δ S k versus T o T m in binary NiTi alloys [21] (a) and in Ni2MnGa alloys (b) [15].The slopes, d Δ S k d t o   , are 3.0 and 6.4, respectively.
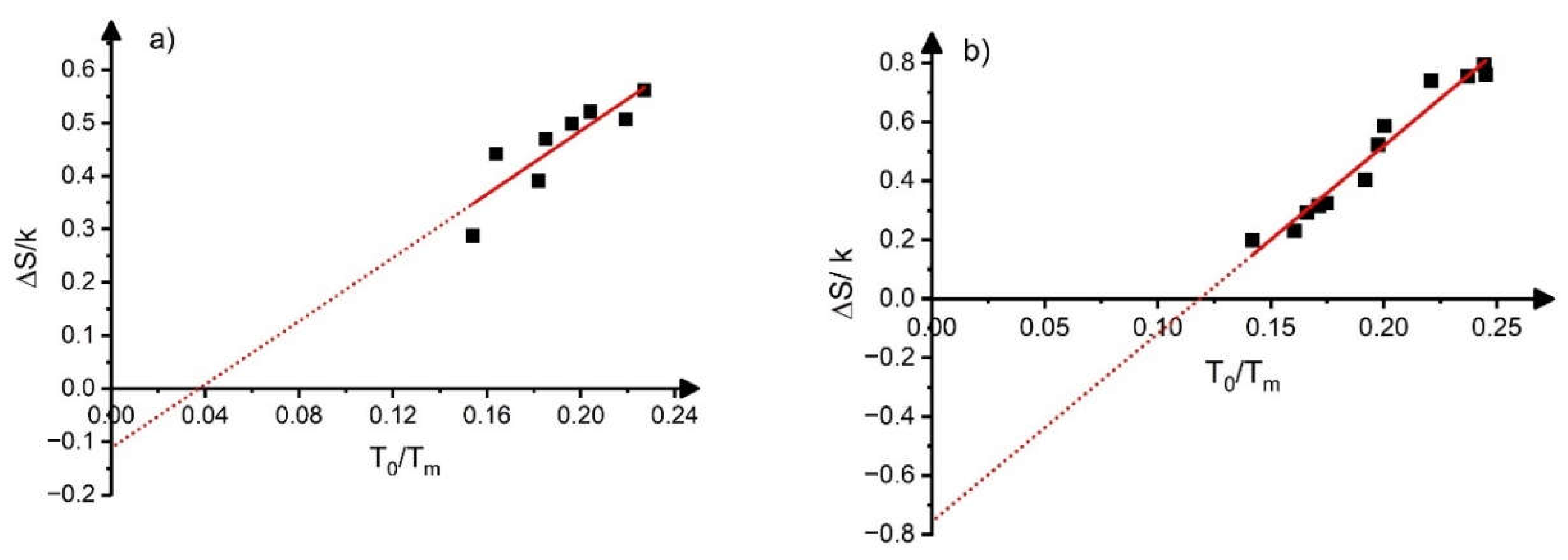
Preprints 113601 g002
Preprints 113601 g003
Figure 3. Q k T m versus M s T m (a) as well as Q k T m − I versus M s T m (b) plots (where I is the values of the intercepts in Figure 1.
Figure 3. Q k T m versus M s T m (a) as well as Q k T m − I versus M s T m (b) plots (where I is the values of the intercepts in Figure 1.
Preprints 113601 g003
Table 1. Experimental dimensionless parameters at to(0) in different sub-classes of SMAs, (the references are given in the first column). For calculation of the dimensionless values the melting points were estimated from the corresponding phase diagrams (see also Table 2) and we assumed that the atomic volume is the same for all alloys ( Ω = Ω N i T i = 8.4 · 10 − 6 m 3 m o l [31]). In the third and fifth columns values of η h , calculated from the to-dependence of h (from eqn. (14) and Figure 1)), as well as from eqn. (21), respectively, are shown for comparison.
Table 1. Experimental dimensionless parameters at to(0) in different sub-classes of SMAs, (the references are given in the first column). For calculation of the dimensionless values the melting points were estimated from the corresponding phase diagrams (see also Table 2) and we assumed that the atomic volume is the same for all alloys ( Ω = Ω N i T i = 8.4 · 10 − 6 m 3 m o l [31]). In the third and fifth columns values of η h , calculated from the to-dependence of h (from eqn. (14) and Figure 1)), as well as from eqn. (21), respectively, are shown for comparison.
sub-class/alloy · H 0 k T m 0 η h 1 h d h d t o η h  
e q n .21 		· S e x p 0 k
γ c ′
γ c 44 A η A = = T m A d A d T
B2/B19’
binary Ni50+xTi50-x [4,5,14,21,28,30,32]		 0.12 8.0 8.7 0.5 9.2 18 2.0 -2.9
B2/B19’
Ti45-xNi45+xCu5 (0≤x≤+1.2at%) [4]		 0.12 5.3 4.9 0.56
 - - - -
B2/B19
Ti50-xNi40+xCu10 (0≤x≤+1.2at%) [4,28]		 0.09 4.2
 3.8
 0.37 9.6
 28
 2.4 -5.3
L21/tetragonal*
Ni2+xMnxGa
[7,15,16,32,33,34,35,36]		 0.03 59 60 0.20
 9.4 80 8.4 -3.1



B2/18R

 CuAlBe [2,3] 0.03 5.2 4.9 0.15 5.3 71.4 13.7 -1.0
CuZn [5,14,37,38,40,42]
 0.04 4.8
 2.7 0.16 - - 11 -0.8
CuZnAl [2,3,37,38,39,40] 0.04 3.9 12 0.16 5.2 70.5 13.6 -1.0
B2/2H
Cu68Al28Ni4 [2,3,35,41]		 0.04 4.8 3.9 0.19 5.7 116 19 -2.85
Table 2. Experimental input parameters.
Table 2. Experimental input parameters.
sub-class/alloy Tm (K) T o 0 T m 0 c’ (Gpa) c 44
(GPa)		 β c ′ T m β c 44 T m α c ′ α c 44 1 T m d T o d x 1 h d h d x
B2/B19’
binary Ni50+xTi50-x [4,5,14,21,28,30,32]		 1583 0.23 14.4 28.6 2.5 5.4 -4 10 - 6.3 -55
B2/B19’
Ti45-xNi50+xCu5 (-2≤x≤+2) [4]		 1583 0.26 - - - - - - -45 -4.3
B2/B19
Ti50-xNi40+xCu10 (0≤x≤+1.2at%) [4,14,27,28]		 1550 0.22
 14.5
 34.53
 3.9 -1.4 - - -62 -2.0
L21/tetragonal
Ni2+xMnxGa
[7,15,16,32,33,34,35,36]		 1403 0.15 12.8 107 3.1 ~0 -15* ~ 0 * 1.9 115




B2/18R

 CuAlBe [2,3] 1353 0.20 7.0 95 0.46 -0.52 10 ~ 0 -10.8 -53
Cu1-xZnx (0.38≤x≤0.50) [5,14,37,3840]
 1048 0.22 9.0 82 0.34 -0.46 6.5** ~0 -6.1 -17
CuZnAl
[2,3,37,38,39,40]		 1210 0.17 6.2 86 0.52 -0.48 3.5 ~ 0 -6.1 -75
B2/2H
Cu68Al28Ni4 [2,3,35,41]		 1353 0.18 7.4
 140 0.65
 -2.2 4.5 ~ 0
-7.4 -29
CuAlNi: data for elastic constants and their T-dependence are averages of those published in [35,40], which deviate from the given average values by about ±18%. It is worth mentioning that in a recent paper [32] the temperature dependence of c’ and c44 was investigated in the very near vicinity of To in NiTi, Ni2MnGa and CuAlNi and except the result for NiTi, their data provides about an order of magnitude larger values for βc’ than those given in Table 1 above. Data with upper index * are from theoretical papers cited also in the first column. Data for αc’ indexed by ** are estimated from the empirical relation proposed by Veringen and Delaey [42] (see also [43]).
Table 3. Estimated parameters related to the composition dependence of the transformation temperature. The last column shows the experimental values of d T o d x for comparison. Since in Table 2, except the binary NiTi alloy, in all cases β c 44 < 0 (i.e., c44 did not show phonon softening) the original Ren Otsuka relation (eqn. (3)) with β c ′ and α c ′ was used. For NiTi the generalized relation (eqn. (29)) with and β = β c 4 − β c ′ and α = α c 4 − α c ′ was used ((eqn. (3) would lead even a positive value for −   α β , i.e., the predicted value for d T o d x would be wrong: −   α β = 2533 ).
Table 3. Estimated parameters related to the composition dependence of the transformation temperature. The last column shows the experimental values of d T o d x for comparison. Since in Table 2, except the binary NiTi alloy, in all cases β c 44 < 0 (i.e., c44 did not show phonon softening) the original Ren Otsuka relation (eqn. (3)) with β c ′ and α c ′ was used. For NiTi the generalized relation (eqn. (29)) with and β = β c 4 − β c ′ and α = α c 4 − α c ′ was used ((eqn. (3) would lead even a positive value for −   α β , i.e., the predicted value for d T o d x would be wrong: −   α β = 2533 ).
sub-class/alloy β T m α − 1 β T m 1 α d t o d x − α β d T o d x
ÍB2/B19’
binary Ni50+xTi50-x 		2.9 14 -0.35
 -0.45 -7642 -9973
L21/ tetragonal
Ni2+xMnxGa
 3.1 -15 -0.32 -0.13 6788
 2666



B2/18R

 CuAlBe 0.62 10 -1.6 -1.1 -21823 -14612
CuZn
 0.34 6.5 -2.9 -0.94 -8552 -6393
CuZnAl
 0.52 3.5 -1.9 -1.7 -8144 -7381
B2/2H
Cu68Al28Ni4 		0.65 4.5 -1.5 -1.6 -9370 -10012
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated