Probabilistic Assessment of Safety Performance of Composite Pile Foundation Using Symmetrical FEM Reliability Method

Preprint

Article

Probabilistic Assessment of Safety Performance of Composite Pile Foundation Using Symmetrical FEM Reliability Method

Altmetrics

Downloads

Views

Comments

Yi Wang^*,

Fenghui Dong^*

Yi Wang^*,

Fenghui Dong^*

This version is not peer-reviewed

Submitted:

16 August 2024

Posted:

20 August 2024

You are already at the latest version

Alerts

Abstract

In this paper, aiming at the safety evaluation of ultimate bearing safety capacity of composite pile bridge foundation, the symmetrical reliability method with forward and inverse analysis con-sidering the randomness of parameters is proposed to evaluate the safety performance of com-posite pile foundation. The proposed method, considering the randomness of parameters, was used to calculate the safety factor of ultimate bearing capacity of composite pile foundation to meet the prescribed target reliability requirements. Through the example calculation of the safety coefficient of pile foundation, the results show that the statistical characteristics of random vari-ables that affect the safety coefficient of pile foundation have a significant impact. The degree of the safety coefficient depends on the probability distribution type and distribution parameters of the random parameters, among which the more important is the variation characteristics of the random variables. The stronger the parameter variability, the lower the safety level of the struc-ture. During design and evaluation, it is necessary to measure the safety reserve of pile founda-tions in accordance with engineering practice to ensure the safe construction and well operation of the pile.

Keywords:

Subject: Engineering - Civil Engineering

1. Introduction

The pile foundation of bridges is a very widely used foundation form in civil engineering [1,2,3]. At present, reinforced concrete piles (especially bored piles), which are the most widely used bridge structures, have high horizontal stiffness and can generate significant additional forces such as temperature when used in integral bridges; Meanwhile, due to the weak tensile performance of concrete piles, the pile foundation is prone to cracks and has poor durability [4,5,6].

Although domestic and foreign scholars have conducted extensive research on the mechanical performance of reinforced beams and derived various forms of ultimate bearing capacity formulas, these formulas often only meet the fitting requirements of deterministic test results [7,8,9]. To determine whether the bearing capacity formula of reinforced beams meets the requirements of reliability, a design method for components based on reliability theory has been established [10,11]. Some scholars have conducted research on the reliability of reinforced components [12,13,14]. There are many factors that affect the force of the pile foundation, and most of them have great uncertainty. Therefore, researching the influence of each factor on the reliability of the pile foundation is of great significance.

Stochastic analysis provides an effective analysis method for considering the random effect of parameters in the research of the safety of pile foundation of bridges [15,16,17]. The significance of studying the probability safety coefficient of bridge pile foundation lies in evaluating the stability and safety of pile foundation, providing scientific basis for bridge design and construction. Specifically, the significance of studying the probability safety factor of pile foundations includes the following aspects. Firstly, improve the safety of bridges: bridges are an important component of transportation, and their safety is directly related to the safety of people’s lives and property. By studying the probability safety factor of bridge pile foundation, the stability and bearing capacity of the bridge can be evaluated, potential safety hazards can be identified in a timely manner, and corresponding measures can be taken to improve the safety of the bridge. Secondly, optimize bridge design: The study of the probability safety factor of bridge pile foundation can provide scientific basis for bridge design. By analyzing and evaluating the probability safety factor of pile foundations, reasonable pile design parameters such as pile length, pile diameter, and pile spacing can be determined, thereby optimizing the design scheme of bridges and improving their bearing capacity and stability. Thirdly, guiding Bridge Construction: The study of the probability safety factor of bridge pile foundation can also provide guidance for bridge construction. By evaluating the probability safety factor of the pile foundation, reasonable construction methods and processes can be determined to avoid damage to the pile foundation during the construction process and ensure the construction quality and safety of the bridge. And then, reduce engineering risks: Bridge engineering is a complex project that involves the comprehensive effects of multiple factors. By studying the probability safety factor of bridge pile foundation, the risk of bridge engineering can be evaluated and controlled, reducing engineering risks, and ensuring the smooth progress of the project.

The structure of this paper is as follows. Firstly, a description is given of the forward and inverse symmetrical reliability method used in this paper. Based on this, a reliability based probabilistic safety factor evaluation method for pile foundations is proposed. Finally, a practical engineering case is used to illustrate the correctness of the research method in this paper, providing theoretical guidance for the safe construction and optimization design of pile foundations.

2. Symmetrical Reliability Method

2.1. Forward Reliability Theory

Structural reliability is of great significance for ensuring the safety of pile foundation structures, improving economic benefits, and achieving sustainable development. In the process of designing, constructing, and maintaining pile foundations, attention should be paid to improving the reliability of the structure to ensure the stability and safety of the system or structure.

Reliability refers to the probability of a system operating normally within a certain period of time, usually described by a reliability function. The reliability function is a function of influencing factors that represents the probability of a system operating normally within a given constraint condition. According to the characteristics and requirements of the system, suitable functional functions can be selected to calculate the reliability of the system, and corresponding optimization and improvement can be carried out based on the calculation results.The limit state of a structure can be described using the limit state equation, which is expressed as:

g=g(R,S)=R-S=0

(1)

where g- the limit state function,

R- is the resistance,

S - the effect of action.

The calculation of reliability index is as follows

β = \frac{μ_{R} - μ_{S}}{\sqrt{σ_{R}^{2} + σ_{S}^{2}}}

(2)

where

μ_{R}

- mean value of random variables of resistance,

μ_{S}

- mean value of random variables of effect,

σ_{R}^{}

- the standard deviation of random variables of resistance,

σ_{S}^{}

- the standard deviation of random variables of effect.

2.2. Inverse Reliability Theory

The safety factor, as a deterministic variable, is a very important parameter in the evaluation and design of pile foundation structures. The probability safety factor is the correlation between the value of the safety factor and the reliability index, and the safety factor is calculated through the reliability index.

For a target reliability index

β^{T}

, the inverse problem can then be stated as [18,19]:

Given

β^{T}

Find: d or/and r

(3)

Subject to:

β (X, k, r) = β^{T}

and

G (X, k, r) = 0

where G - the limit state function,

X- the vector of basic random variables,

k - the vector of design deterministic parameters,

r - the vector of the design parameters of random variables.

The equation

β (X, k, r) = β^{T}

can be written as an ordinary nonlinear equation

f = β (X, k, r) - β^{T}

(4)

with the Newton-Raphson iterations applied to arrive at

f = 0

The iterative formula for the safety factor can be obtained from formula (2) as

K_{}^{j + 1} = K_{}^{j} + \frac{β_{T} - β_{}^{j}}{{\frac{\partial β}{\partial K} |}_{K^{j}}}

(5)

Equation (8) is selected as the convergence criterion of the reliability inverse analysis method adopted in this paper,

| K^{j + 1} - K^{j} | \leq ε

(6)

where,

ε

is the convergence criterion value.

2.3. Implementation Steps

Step 1: Set initial values for random variables and iteration of surrogate parameters.

Step 2: Set j = 1, and calculate

β_{}^{j}

and

{\frac{\partial β}{\partial K} |}_{K^{j}}

Step 3: Using

β_{}^{j}

and

{\frac{\partial β}{\partial K} |}_{K^{j}}

to calculate the safety factor of pile foundation and to update K value.

Step 4: Check the convergence criteria and perform cyclic iterations until convergence.

3. Reliability Model of Safety Factor of Pile Foundation

The expression for the deterministic safety factor of pile foundation is as follows:

K = \frac{R}{S}

(7)

Where,

K

- the safety factor of the pile foundation,

R

- the characteristic value of the pile,

S

- the combination of the standard value of the action effect of the pile.

The standard value of the vertical ultimate load-carrying capacity of a single pile is calculated by the following formula (10)

Q_{k} = Q_{s k} + Q_{p k} = u \sum q_{s i k} l_{i} + p_{s k} A_{p}

(8)

Where,

Q_{k}

- the standard value of the pile,

Q_{s k}

- the standard value of the total ultimate lateral friction resistance,

Q_{p k}

- the standard value of the total ultimate end resistance,

u

- the perimeter of the pile,

q_{s i k}

- the standard value of the unit limit friction resistance of the

i

-layer soil on the pile side,

l_{i}

- the thickness of the

i

-layer soil on the pile side,

p_{s k}

- the standard value of the unit limit end resistance of the soil,

A_{p}

- the area of the bottom of the pile.

Since the ultimate load-carrying capacity of a single pile is related to various uncertain factors, equation (12) cannot cover all the uncertain factors, so a random variable is introduced to describe the uncertainty of the calculation model, as shown in equation (13):

Q_{u} = χ Q_{k}

(9)

where,

Q_{u}

- the measured value of the vertical ultimate load-carrying capacity of the single pile of bridge structures,

χ

- the random variable coefficients of the uncertainty calculation model.

According to the inverse reliability theory, when evaluating the safety factor of the vertical load-carrying capacity of the pile foundation of bridges, the load-carrying capacity safety factor is taken as an unknown parameter. From equations (7) and (9), it can be known that the calculation expression of the pile foundation of bridges stability safety factor is as follows:

K = \frac{Q_{u}}{S}

(10)

And then,

Z = Q_{u} - K S_{}

(11)

Combining Equation (8) and Equation (11), the functional function for the analysis of the probabilistic safety factor of the vertical load-carrying capacity of the pile foundation can be constructed as:

Z = χ (u \sum q_{s i k} l_{i} + p_{s k} A_{p}) - K S

(12)

4. Application

The ultra-high performance concrete composite pile foundation is used under the pier columns of an actual bridge engineering. Establish a solid model of the pile foundation and bearing platform using the universal finite element software ANSYS, and simulate it using SOLID45 elements. The soil spring was simulated using the COMBIN14 element, with each soil spring element using a single degree of freedom analysis option. The two nodes of the element coincide and are located at the same position as the peripheral nodes of the pile foundation. The spring element stiffness is calculated based on the soil investigation results. In order to facilitate the application of loads and boundary conditions, reference points were established at the top section of the column and the bottom section of the pile cap, and rigid coupling constraints were established between all points in the corresponding section and the reference points. The finite element model is shown in Figure 1.

The ultimate bearing capacity of the foundation soil under the cushion cap can be calculated using the modified Hansen formula, as follows:

f_{d} = 0.5 ψ N_{γ} ζ_{γ} γ B + N_{q} ζ_{q} γ_{0} D + ψ N_{c} ζ_{c} c_{d}

(13)

Where

c_{d} = \frac{λ c_{k}}{γ_{c}}

(14)

φ_{d} = \frac{λ φ_{k}}{γ_{φ}}

(15)

where

ψ

was correction factor, related to the design value of internal friction angle

φ_{d}

N_{γ}

N_{q}

N_{c}

are bearing capacity factor, related to design value of internal friction angle

φ_{d}

;

ζ_{γ}

ζ_{q}

ζ_{c}

was the shape coefficient of the cushion cap;

B

wasthe width of the cushion cap;

D

was burying depth for bearing platform;

γ

wasweight of the soil below the bottom surface of the bearing platform;

γ_{0}

was weighted average weight of each layer of soil above the bottom surface of the bearing platform;

c_{d}

was design value of cohesive force of foundation soil;

c_{k}

was the standard value of foundation soil cohesion;

φ_{k}

was Standard value of internal friction angle of foundation soil;

λ

was correction coefficient for the standard value of shear strength index of soil, which was 0.8;

γ_{c}

was partial coefficient of soil cohesion, which was 2.7;

γ_{φ}

was partial coefficient of internal friction angle of soil, which was 1.2

Among the above parameters, the geometric parameters of the pile cap and pile body, as well as the coefficients related to the geometric parameters, as well as the thickness of each layer of soil and the weight of the soil layer and pile cap, can be regarded as deterministic. The correction coefficients and bearing capacity coefficients related to

c

and

φ

, as well as load indicators of each soil layer

f_{s i}

and

f_{p}

, which have significant variability and both are considered random variables.

The settlement control expressed by various basic random variables conforms to the limit state equation for checking the overall bearing capacity of the pile foundation as follows:

\begin{matrix} Z = χ [n (U_{P} \sum_{}^{} f_{s i} l_{i} + f_{p} A_{p}) + (0.5 ψ N_{γ} ζ_{γ} γ B + N_{q} ζ_{q} γ_{0} D + ψ N_{c} ζ_{c} c_{d}) A_{c}] \\ - K [(\bar{S_{G}} + \bar{S_{Q}}) - G] \end{matrix}

(16)

A bridge bored pile has a diameter of 1.2m and a length of 22m. The load effect on the top of the pile is 3000kN. The design parameters of each soil layer are shown in Table 1. The statistical characteristics of random variable indicators for foundation soil are shown in Table 2.

Putting the above parameter values into formula (16), the structural overturning stability function can be obtained as:

Z = x (1) \cdot (1492.88 x (2) + 1244.07 x (3) + 3317.52 x (4) + 2488.14 x (5)) - 3000 \cdot K \cdot x (6)

(17)

The values of the statistical parameters of each random variable in formula (19) are shown in Table 3.

4.1. Analysis Results of Safety Factor

In this example, the target reliability index of the bridge is 3.5, the calculated safety factor of the vertical load-carrying capacity of the pile foundation of bridges is 1.224, and the safety factor calculated based on the deterministic model is 2.848.

By comparing the safety factor calculation results of probabilistic safety factors and deterministic models, it can be found that using the principle of reliability to calculate the safety factor from a probability perspective can fully consider the randomness of parameters, which has a significant impact on the calculation results of probabilistic safety factors, mainly reflected in the fact that the variability of parameters directly reduces the safety reserve of the structure. Therefore, the result of the probability safety factor is smaller than the calculation result of the deterministic model. This will prompt us to consider the impact when designing and evaluating the pile safety.

4.2. Impact of Target Reliability Indicators

The target reliability index is used to ensure the safety of bridge pile foundation reliability, and the size of the target reliability index has a significant impact on the value of the pile foundation safety coefficient. In order to study the quantitative impact of target reliability indicators on the safety factor of pile foundations, different values of target reliability indicators are taken and each type of target reliability indicator is calculated separately. The value range of the structural target reliability index is 1~4, in order to analyze the influence of the target reliability on the safety factor of the vertical load-carrying capacity of the pile foundation of bridges. The specific calculation results are shown in Table 4.

Through analysis, it can be found that the target reliability index has a significant impact on the value of the safety coefficient of pile foundation structures, and the size of the safety coefficient is inversely proportional to the target reliability index. That is to say, the larger the value of the target reliability index, the smaller the calculated safety factor, and the magnitude of the safety factor corresponds one-to-one with the structural reliability index. Therefore, the determination of the probability safety coefficient of pile foundation structures and the functional relationship between the target reliability indicators can provide effective means for structural safety assessment and design.

4.3. Influence of Parameter Uncertainty on Safety Factor

In order to study the impact of parameter randomness on the safety factor of pile foundation structures, the mean and variability of random variables are changed when the target reliability index is set. The structural target reliability index is taken as 3.5, the mean value of each random variable is increased by -15%~+15%, and the variation coefficients is taken as 0.07, 0.1, 0.15, 0.2, 0.25, the calculation results of the overturning stability safety factor are shown in Table 6 and Table 7 and Figure 2 and Figure 3.

By analyzing the influence of the mean value of random variables on the safety factor, it can be known that the safety factor increases with the increase of the mean value of

χ

q_{s 1 k}

q_{s 2 k}

q_{s 3 k}

p_{s k}

, and decreases with the increase of the mean value

S

. By analyzing the influence of random variable coefficients of variation on the safety factor, it can be known that the safety factor decreases with the increase of the variability of

χ

q_{s 1 k}

q_{s 2 k}

q_{s 3 k}

p_{s k}

and

S

. Comprehensive analysis of the influence of parameter uncertainty on the safety factor shows that the random variable coefficients

χ

that describes the uncertainty has the greatest influence on the safety factor, which needs to be determined carefully.

4. The Influence of the Initial Value of the Safety Factor Iteration

Since the initial value

K^{0}

of the safety factor of the vertical load-carrying capacity of the pile foundation of bridges is arbitrarily selected in the process of reliability inverse analysis, it is necessary to analyze the influence of the initial value

K

on the safety factor of the vertical load-carrying capacity of the pile foundation of bridges. When the initial values

K

are 1.05, 1.10, 1.15, 1.20, 1.25, and 1.30, the corresponding safety factor calculation results are shown in Figure 4 (the abscissa in the figure represents the number of iterations).

Through analysis, it can be found that the calculation of the safety factor of pile foundation structures requires multiple iterations. By setting different initial iteration values, the final results all converge to the same value. This indicates that the setting of the initial iteration value only affects the iteration process and number of iterations, and has no effect on the final result value.

6. Conclusions

This paper proposes a probability analysis method for the safety evaluation of pile foundation structures. This method calculates the probability safety factor by combining forward and inverse reliability theory. The calculated safety factor can simultaneously consider the randomness of the target reliability index and design parameters of the structure. The method proposed in this article is analyzed through an engineering example and the following conclusions are obtained:

(1) A reliability model for the safety factor of pile foundation has been established, which is based on a functional function and takes into account the target reliability indicators of the structure.

(2) The target reliability index of a structure has a significant impact on the value of the safety factor of pile foundations. The larger the target reliability index, the smaller the structural safety factor. The smaller the target reliability index, the greater the structural safety factor.

(3) The randomness of design parameters also has a significant impact on the value of safety factors. Overall, the greater the variability of parameters, the smaller the safety factor of the structure. The smaller the variability of parameters, the greater the safety factor of the structure.

(4) The method proposed in this paper has no effect on the final result when calculating the safety factor, indicating that the method proposed in this paper is suitable for calculating the safety factor of pile foundation.

Author Contributions

Methodology, Yi Wang; Writing – review & editing, Fenghui Dong.

Funding

Natural Science Foundation of Jiangsu Province (Grant No. BK20200793).

Data Availability Statement

The data used to support the findings of this study are available from the corresponding author upon request.

Acknowledgments

The authors wish to express their sincere to the Natural Science Foundation of Jiangsu Province (Grant No. BK20200793).

Conflicts of Interest

The authors declare no conflict of interest.

References

Zhang, Y.; Zheng, H.; Li, G. Experimental Study of UHPC-RC Composite Pile Connected by Shear Keys. Structural Engineering International 2022, 32, 189—202. [Google Scholar] [CrossRef]
Shin, Y.; Langford, T.; Cho, K.; et al. Applicability of Concrete–Steel Composite Piles for Offshore Wind Foundations. Energies 2021, 16, 4794. [Google Scholar] [CrossRef]
Jiang, K.; Gong, W.; Xu, J.; et al. Effects of inclined bedrock on dissimilar pile composite foundation under vertical loading. Geomech. Eng. 2022. [Google Scholar] [CrossRef]
Heimann, M.; Schmidt, H.; Tran, N.L.; Graubner, C.A. Reliability of highly stressed UHPC-columns. Beton-Und Stahlbetonbau 2013, 108, 2–12. [Google Scholar] [CrossRef]
Kwon, K.; Parkkeunhee, Y.; Tae, C.; et al. k-uhpc reliabilitybased performance assessment and prediction of tendon corrosion in kuhpc bridges. Journal of the Korean Society of Safety 2018. [Google Scholar] [CrossRef]
Tran, N.L.; Grziwa, U.; Graubner-Ing, C.A. Spatial Variability of Material Properties and Its influence on Structural Reliability of UHPC Columns[C]//Concrete - Innovation and Design (fib Symposium).2015.
Lu, D.; Yalan, X.; Xudong, S. Fatigue reliability assessment of light-weighted steel-UHPC composite bridge deck. Zhongnan Daxue Xuebao (Ziran Kexue Ban)/Journal of Central South University (Science and Technology) 2018, 49, 711–717. [Google Scholar] [CrossRef]
Roberson, M.M.; Inman, K.M.; Carey, A.S.; Howard, I.L.; Shannon, J. Probabilistic neural networks that predict compressive strength of high strength concrete in mass placements using thermal history. Computers & Structures 2022, 259, 106707. [Google Scholar] [CrossRef]
Stewart, M.G.; Li, J. Risk-based assessment of blast-resistant design of ultra-high performance concrete columns. Structural Safety 2021, 88, 102030. [Google Scholar] [CrossRef]
Zai, J.; Lu, Z.; Huang, G. Reliability analysis of designed method of composite pile foundation according to ultimate bearing capacity of single pile. Rock and Soil Mechanics 2004, 25, 1483–1486. [Google Scholar]
Li, M.; Li, J. Reliability analysis of settlement control for composite pile foundation. Journal of Hefei University of Technology 2004, 27, 698–701. [Google Scholar]
Zhang, L.; Han, J. The probability analysis method of the bearing capacity of composite pile foundation. Journal of Shenyang Agricultural University 2001, 32, 433–435. [Google Scholar]
Xiong, Q. Reliability analysis of ultimate bearing capacity of composite pile foundation. Journal of Civil, Architectural and Environmental Engineering 1998, 20, 28–32. [Google Scholar]
Li, J.; Lou, X.; Jia, F. Safety and reliability of composite pile foundation designed by controlling settlement and bearing capacity. Journal of Building Structures 2003, 24, 86–90. [Google Scholar]
Cheng, J.; Xiao, R. Main Cable Safety Factors Assessment of Long-span Suspension Bridges Based on Inverse Reliability Method. China Journal of Highway and Transport 2007, 20, 58–61. [Google Scholar]
Jiang, W.; Lv, D. Stability design of concrete filled steel tubular arches using inverse finite element reliability method. Journal of Building Structures 2013, 34, 119–124. [Google Scholar]
Lou, C.; Dong, F. Study of overturning stability safety factor of continuous girder bridges during cantilever construction. Engineering Journal of Wuhan University 2017, 50, 264–268. [Google Scholar]
Der Kiureghian, A.; Zhang, Y.; Li, C.C. Inverse reliability problem. Journal of Engineering Mechanics, ASCE 1994, 120, 1154–1159. [Google Scholar] [CrossRef]
Li, H.; Foschi, R.O. An inverse reliability method and application. Structural Safety 1998, 20, 257–270. [Google Scholar] [CrossRef]

Figure 1. Finite element model of composite pile foundation.

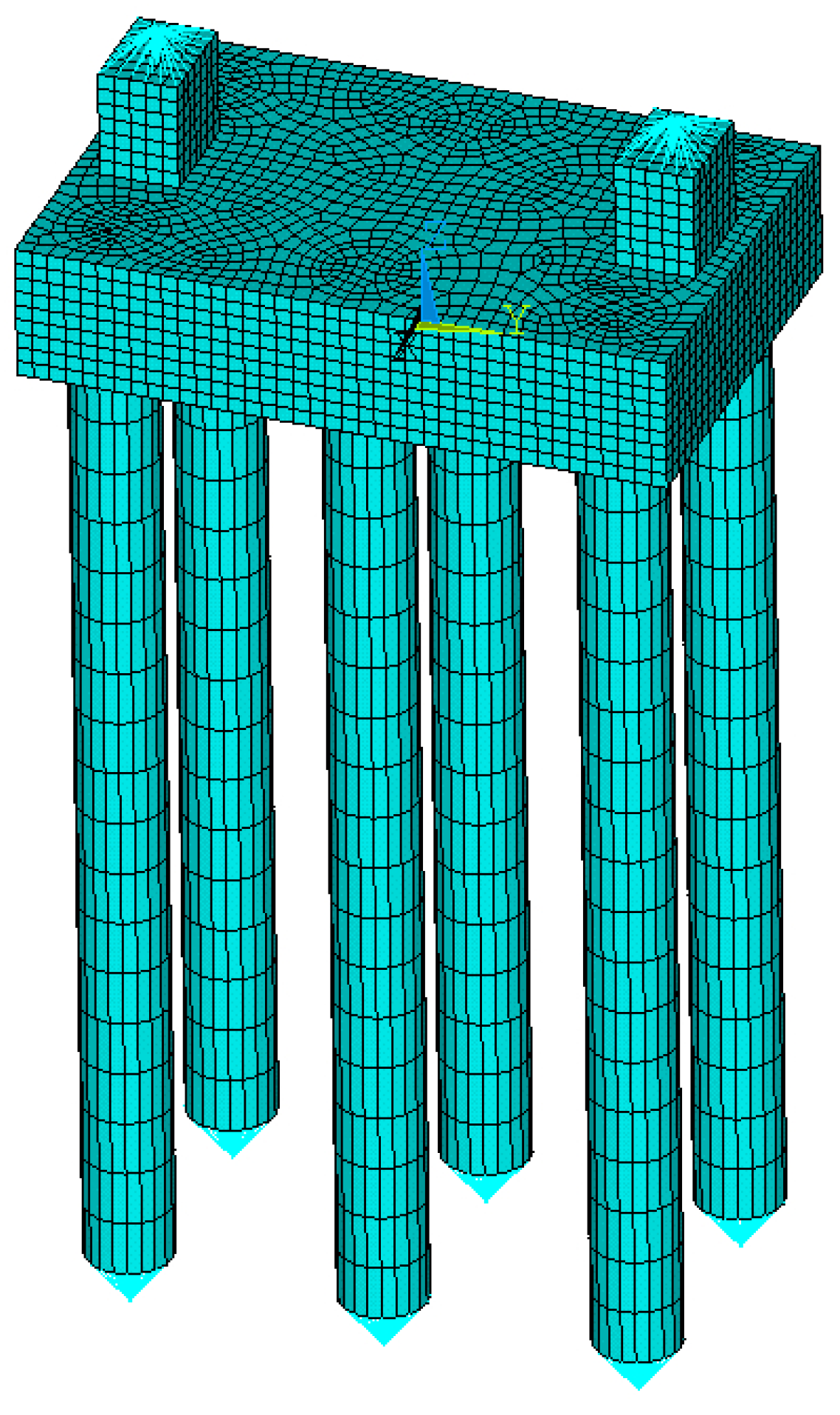
Figure 2. Effect of mean value of random variables.

Figure 3. Effect of coefficients of variation of random variables.

Figure 4. Effect of initial value K of safety factors.

Table 1. Design parameters of soil layers.

Structural layer	Thickness/m	Ultimate pile side friction resistance/kPa	Ultimate pile end friction resistance/kPa
Soil layer1	6	60	-
Soil layer2	6	50	-
Soil layer3	10	80	-
Pile end soil	-	-	2000

Table 2. Statistical properties of random variables of foundation soil.

Layer order	$c / k P a$		$φ / \circ$		f_si/kPa		$f_{p} / k P a$
Layer order	Mean value	coefficient of variation	Mean value	coefficient of variation	Mean value	coefficient of variation	Mean value	coefficient of variation
2	16.8	0.45	10.4	0.20	12	0.11	-	-
3	9.1	0.33	15.3	0.15	12	0.26	-	-
4	7.0	-	7.5	-	12	0.16	-	-
5₁	11.2	-	12.0	-	33.6	0.18	800	0.25
5₂	5.0	-	25.0	-	40	0.23	1100	0.31
6	30.8	-	19.5	-	48	0.20	1200	0.29

Table 4. Influence of reliability index on safety factor of vertical load-carrying capacity of pile foundation of bridges.

Target reliability index	1	1.5	2	2.5	3	3.5	4
Safety factor	2.3174	2.0745	1.8449	1.6275	1.4212	1.2240	1.0332

Table 6. Effect of mean value of random variables.

Mean	Safety factor
	x(1)	x(2)	x(3)	x(4)	x(5)	x(6)
	$χ$	$q_{s 1 k} / k P a$	$q_{s 2 k} / k P a$	$q_{s 3 k} / k P a$	$p_{s k} / k P a$	$S / k N$
-15%	1.0401	1.1894	1.1947	1.1561	1.1698	1.4402
0	1.2240	1.2240	1.2240	1.2240	1.2240	1.2240
+15%	1.4078	1.2577	1.2526	1.2905	1.2771	1.0640

Table 7. Effect of coefficients of variation of random variables.

Coefficients of variation	Safety factor
	x(1)	x(2)	x(3)	x(4)	x(5)	x(6)
	$χ$	$q_{s 1 k} / k P a$	$q_{s 2 k} / k P a$	$q_{s 3 k} / k P a$	$p_{s k} / k P a$	$S / k N$
0.07	1.6135	-	-	-	-	1.2240
0.1	1.5117	-	-	-	-	1.1945
0.15	1.2240	1.2289	1.2274	1.2478	1.2377	1.1342
0.2	-	1.2240	1.2240	1.2240	1.2240	-
0.25	-	1.2175	1.2195	1.1907	1.2057	-

Table 3. Random variables.

No.	Random variable	Probability distribution type	Mean	coefficients of variation
x(1)	$χ$	Normal	1	0.15
x(2)		Normal	1	0.2
x(3)	$q_{s 2 k} / k P a$	Normal	1	0.2
x(4)	$q_{s 3 k} / k P a$	Normal	1	0.2
x(5)	$p_{s k} / k P a$	Normal	1	0.2
x(6)	$S / k N$	Normal	1	0.07

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer

Probabilistic Assessment of Safety Performance of Composite Pile Foundation Using Symmetrical FEM Reliability Method

Abstract

1. Introduction

2. Symmetrical Reliability Method

2.1. Forward Reliability Theory

2.2. Inverse Reliability Theory

2.3. Implementation Steps

3. Reliability Model of Safety Factor of Pile Foundation

4. Application

4.1. Analysis Results of Safety Factor

4.2. Impact of Target Reliability Indicators

4.3. Influence of Parameter Uncertainty on Safety Factor

4. The Influence of the Initial Value of the Safety Factor Iteration

6. Conclusions

Author Contributions

Funding

Data Availability Statement

Acknowledgments

Conflicts of Interest

References

MDPI Initiatives

Important Links

Subscribe