Preprint
Article
Negotiating Darwin’s Barrier: Evolution Limits Our View of Reality, AI Breaks Through
Altmetrics
Downloads
53
Views
96
Comments
0
This version is not peer-reviewed
Submitted:
19 September 2024
Posted:
19 September 2024
You are already at the latest version
Alerts
Abstract
Physics is imagined in the human brain which was formed in Darwin's Evolution by responding to certain threats for the survival of our evolutionary ancestors. The struggle to survive prevented us from wasting resources by paying attention to parts of reality that had no survival impact. These parts are of unknown scope and dimension. Can we negotiate this Darwin's barrier? As to reality within our reach, we suffer from imbalance between information absorption capacity and information analysis capacity which resulted in ignoring the overflow of information to match human capacity to make sense of it. Also James Clerk Maxwell suggested that reality has small scale phenomena beyond our human reach. Since we have no brain other than what Darwin equipped us with, this barrier looked unsurmountable. But lo and behold: much as we have built vehicles to carry us further than our biological legs, so we now build artificial intelligence machines that carry us beyond our Darwinist barrier. Physics may transform to building AI capability to ride on. This capability may require a more universal geometry and material representation to fit AI inference engines. Suggesting Depth Unbound Reality Architecture (DURA). AI connects raw data to its conclusion potential, by-passing a storied theory of nature. The objective of physics ahead may be to build theory-replacement AI machines.
Keywords:
Subject: Physical Sciences - Theoretical Physics
1.0. Introduction
For bio entities to evolve to human beings they need to face a threat climate of very narrow parameters. It must be a threat that allows the “evolvers” to adjust through generational selection, and stay alive as a community. Threat that is either too mild, or too abrupt will not enable evolution. We, humans, the pinnacle of our evolutionary ladder, we owe our development to the threat climate that propelled us into who we are. And what is more consequential, the features of this particular generational threat are the only features of reality we were motivated to develop sensors for and think about. All other aspects of reality, namely aspects that had no survival impact on us and on our evolutionary ancestors, presented no incentive for us to build sensory organs for, not to speak of preparing processing devices for.
This depressing conclusion of blindness to unknown parts of reality has been reached by our Darwinist brain. It is a fatal blow to our aspirations for understanding reality in its fullness and in its essence. Alas, Darwin also taught us to be ever hopeful, and so we are, planning to meet this challenge and find a way to open our curious eyes and be blind no more.
But before we address our Darwinist blindness we are drawn to realize that in this particular evolutionary path leading to us, humanity, we experienced a fundamental imbalance between how easy it is for us to develop sensory organs to read reality, as opposed to how difficult it is for us to process recorded information and use it to guide our actions to promote our survival. This imbalance compelled us to abandon the full conclusion-potential carried by the vast amount of sensory information we collect, and make do with a fraction thereto. In other words, we suffer from blurry vision. This challenge may have found its answer in the emerging technology of artificial intelligence.
Let reality be expressed with n measurements. nb of them have no survival impact on the evolving community (the “evolvers”), and hence the evolvers are blind towards them. So only the other measurements are regarded: nr = n - nb. Evolution so happened that the evolvers don’t have the capacity to process all the nr measurements of reality which they absorb. It was necessary to recede from the full detail of recorded information, and use some means to reduce this raw data input to a quantity that our evolving brain can handle. Human beings used two methods to bridge this imbalance: (i) disregarding some measurements, (nd) and (ii) grouping some measurements, and representing such groups with a single representative measurement. As a result the non-disregarded measurements nr - nd are grouped to ng representative measurements, which are few enough for the capacity of the evolvers to timely process.
The “magic” of the Darwinist operation is that the evolvers will eventually identify the best set of nd measurements to be ignored, and the best way to group the other (nr - nd) measurements to ng measurements which the evolvers can process.
While this Darwinist optimization is indeed very powerful, it still retains inherent blurriness. The ignored measurements (nd) offer insight into reality, which is ignored, and the grouping of the other measurements confers the loss of the pre-grouping wealth of information about the nature of reality.
So while Darwin works well enough to build a successful evolutionary ladder and put us on the top rung, it does not satisfy our burning desire to not just survive, not even just thrive in our reality home, but more than that: we aspire to understand where is it that we find ourselves. We aspire to replace this blurriness of vision with sharpness of vision.
We have no reason to assume that threats to humanity are limited to the subclass of threats not too mild and threats not too harsh, which are the only threats with evolutionary relevance. So assume we must that reality is filled with features that pose no survival impact on us, and are lurking next to us, or far from us, but undetected by us. This conclusion is a big gut punch to our aspirations to know reality. This article addresses these aspirations.
The disturbing phenomenon of large and small numbers. Any natural law that involves a very large or very small number is suspected to be non fundamental because such numbers reflect a forethought, design which modern science denies. Numbers showing up in “reality generating formulas”, scientists insist, must be small. Come to think about it, all particular numbers showing up in a natural law, large or small, are hard to stomach. If the universe is” Godless” then nothing but randomness can be comfortably explained. And to the extent that a natural law is particular and involves arbitrary large or small numeric values, it runs into no plausible explanation on the part of the universe rather a plausible explanation on the part of the observers. The layers and layers of evolution from the early forms of life have impacted arbitrary imprints on the evolving consciousness so that it became guided by it to practice innovation and discovery along the track that extends itself linearly from history, suffering from blindness to all other tracks.
Cracking Darwin’s Egg: One picturesque rendition of the message herein is that humanity hitherto was incubating in “Darwin’s egg”, and now it is cracking this egg with the newfound tool of AI, going outside to the world without.

1.1. Innovation Science
The thesis herein is developed through the use of new methods of innovation science -- the generic elements of innovation. In particular the use of InnovationSP [1] . Accordingly the two recent monumental upheavals of physics are the realization that probability is in the core of reality and the audacity with respect to space, promoting it from passive markings to an active actor. Extracting from these upheavals, one would further upgrade space into “unbound state”, and promote probability calculus to power up inference engines, applied over reams of raw data, striving towards the ambitious aim of voiding the need for representative equations as intermediaries between raw experimental and observational data and its full-fledged inferential treasure.
1.2. Literature Survey
Reference [3,4,5,6,7,8,9,10,11] represents the interest that is growing in the impact of artificial intelligence on physics. It is mostly on teaching physics, next on helping with physics computations, and even into AI written scientific papers. The notion that artificial intelligence will void the need for a scientific theory was not found. Also no mention was found of the thought to construct space from a finite number of points with rational unbound distances between the points so that any other space used in physics becomes an unbound space, bound to the a particular framework of the case in point.
2.0. Deblurring
Blurring of reality is happening on a personal every day level, and up to the full depth of science.
Being approached by a stranger one quickly reads myriads of facial features ahead, and instantly groups the readings of the curved eyebrows, the tight lips, and squinting eyes into a single reading: threat. This reading is then processed by the brain along with some other parameters to conclude on a course of action. The human brain cannot relate to myriads of facial features ahead and extract all the inferential message therein. Time is of the essence, processing capacity is limited (compared to information acquisition capacity), so grouping takes place. The myriad of facial details are lost, left is the summary reading: a very angry and threatening face was there to be dealt with.
Similar examples of data grouping to match processing capacity are plentiful as we go along on our day. However, in this presentation I wish to focus on the other end of this Darwinist grouping: how science is developed.
In 1873 James Clerk Maxwell suggested [13] that there are classes of phenomena affected by influences whose physical magnitude is too small to be taken account of by a finite being but which may produce results of the highest importance. Maxwell might have been the pioneer of the notion of inherent blurriness.
If one feeds a supervised learning AI program with sufficient number of cases where in each case one throws a rock in stationary air at a given temperature, in an arbitrary, but recorded direction and speed, and is also specifying the weight, the shape, and the surface of the rock, and for each such case the flying trajectory will be specified, then the AI program would accurately predict the landing place and even the trajectory of any rock for which the AI program would be given the pre-throwing parameters that have been recorded for the reference cases.
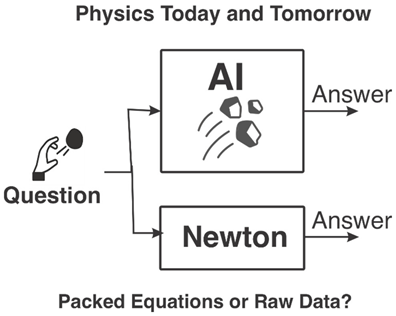
What is of interest in this AI capability is that it was not given Newton’s laws of motion. It applies generic learning techniques and it performs in a way that would deny an observer the knowledge of whether the AI machine relied on Newton’s law or not.
A strong argument in favor of the “Newton way” is that these equations were derived from one set of observations (say, throwing rocks) and then were applied over a completely different set (say, cosmology). AI can’t do it! That is true, but AI is a young solution, give it time. When steam engines made their appearance they lagged behind fast horses. But people did not focus on improved equestrian diet, rather they tinkered with the new machine.
The tacit premise of modern science is that if a finite number of observations appears consistent to the degree of measurement accuracy with a certain mathematical equation, then it indicates that reality obeys this formula, which we were smart enough to discover. Newton’s laws served humanity remarkably well: roads, bridges, houses, ships, trains, airplanes -- all designed and built under this almost ‘religious’ belief that reality reads and obeys Newton’s “Bible”. Imagine how cumbersome it would have been to try and test countless constructions and arrangements until we build a bridge that does not collapse. As things happen, we trust our simple equations and make life so much easier with it.
Indeed, as far as survival is the point, the ability to ignore countless random configurations until building a stable bridge is a great advantage. Albeit, when we indulge our aspirations to learn the reality we live in, we must admit the possibility of blurry vision. In fact, Newton’s formula was proven “blurry”. Centuries later Albert Einstein has pointed to Newtonian blurry vision at its contours. It did not change much in construction practice, but it enlightened us as to the reality we find ourselves in.
Come to think about it, Einstein too used a concise mathematical formula that relates to Newtonian math as a private case, and much like we did with Newton, we build a daring picture of space, reality, galaxies, the big bang, all on the new equations we now regard as the “law of the land”.
Formulas and mathematical equations offer by their nature a blurry vision of reality they describe. They summarize the raw data that gave them birth, and ignore some measure of inferential content they carry.
What is more alarming is that many scientists, tend to regard the equations we carve, much the same way our ancestors regarded the big wooden or stone statues they carved. Men of antiquity danced around their overpowering handiwork, regarding them as God. Our most brilliant scientists are saying about one mathematical framework or another: the beauty here is so compelling that it is without a doubt that the universe surrenders to this beauty and complies with its dictates.
It is easy to understand this sentiment. Come to think about it, the simplicity of Newton’s laws united heaven and earth, past and future, large and small. It was so dramatically useful, so broadly applicable. We can’t but be thankful to the math that described reality for us. In fact many people of note are claiming that the universe is nothing but a mathematical entity [2] The entire body of physics is searching for the ‘lost ark’ -- the single simple formula for ‘everything’.
Equations represent grouping of the raw data that we may read in reality. Grouping is lossy in terms of information content, so, to the extent that we wish to extend beyond survival concerns and aspire to understanding the world we live in, we must seek to undo grouping, avoid information loss, abandon our beloved equations, and do what the AI machine did in the beginning of this section: ignorant of any so called natural law it predicted the behavior of a thrown rock by inferring directly from the raw relevant data.
The attitude of hanging on to the natural law equations is attractive because it seems to seal the deal. Once we have inked out the elegant equation we believe we have nothing more to glean from experimental data. Maxwell’s equations, for example, say everything worth saying about electromagnetic phenomena. No need to measure them any longer, at least not for the purpose of gaining more fundamental insight. The psychological attachment to the notion that the natural law equation is a dictum that cannot be deviated from is so strong that no researcher will secure funding for a project to keep measuring electromagnetic phenomena for either (i) hidden refined behavior, or (ii) for a quick passing wave of deviation from the equations. Neither would one is likely to get funded for suggesting that a given set of natural laws are valid only under certain conditions, yet unknown.
It is anathema for a scientist to contemplate a law of nature that ‘takes a break,’ disappears for a very brief moment in which a new, yet undiscovered formula comes into play. Einstein famously assumed that laws of nature look the same everywhere. All these assumptions come from a psychological sense of natural harmony, symmetry, beauty. It is so gratifying to expect that the universe is operated in ways consistent with what we, the evolvers, call beautiful.
Isn’t it a tad suspicious that nature rolls out according to formulas that incorporate a sufficiently small number of parameters which we humans can be cognizant of in parallel?
A modern imperative over nature is the notion of symmetry. Evolution has driven us humans to be partial to symmetry so we readily assume that nature will respect symmetry because we do. Our brightest scientists are left undaunted by lack of scientific evidence; they hail and promote a super symmetry view of reality.
We did not have an alternative to theories and simple equations until recently but with the rise of AI, we can aspire to minimize the blurriness and use the ungrouped, raw data as the input for our inference engines.
2.1. Mathematical Expression of Blurriness
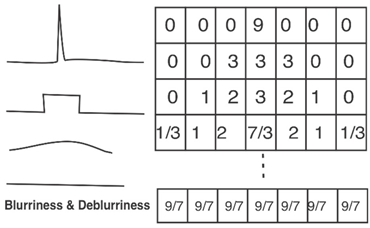
“Data as it really is” is said to be measured with some blurriness by the foremost sensors touching this data. These sensors then feed data to several levels of processors until a ‘ready for analysis’ data is generated. One particular way to handle this mathematically is given in []. A brief account is jotted here. Let raw data be presented as a series of values x0n, x02, ..... x0m.
The data is said to be measured with blurriness effect resulting in readings: x1n, x12, ..... x1m.
This measured data is blurred again and again and after i times it looks like this: xin, xi2, ..... xim.
where
xij =. (xi-1j-1 + xi-1j + xi-1j+1)/3
The x series gets flatter and flatter each round blurriness until at round t it is totally flat:
for every i=n, n+1....m. for j= n, n+1,...m
xti = (Σ x0j)/ (m-n)
At a certain level k < t. the data is analyzed. One assumes blurriness and builds an array of likely candidates for the pre-blurred data x0n, x02, ..... x0m. By building similar pre-blurred candidates for other similar measurement an AI machine will point to the most likely pre blurred data and analyze it. This is done under the approach that scientific measurements see a blurred reality and must use its tools to counter this loss.
2.2. Chaos Theory and Blurriness
De-blurriness as mathematically described in 2.1 is a means to overcome the challenge of chaos arising from measuring reality too minutely. Henry Poincarè failed to apply Newton’s laws to the three body problem because it required too high resolution analysis. By measuring reality sufficiently roughly (blurred vision) we spare ourselves the confusion post by chaos behavior, but we deprive ourselves from insight regarding reality. Fortunately today AI machines are well adjusted to analyze the complexity of chaos.
3.0. Dark Reality
All the parts of reality that had and have no impact on human survival, are not being sensed, detected, or even suspected by humans and are for us dark reality.
Unlike deblurring of knowledge, dark reality offers no threads on which to pull to unravel the hidden knowledge. It is a much bigger task. The suggested strategy is to go backwards to the points of evolution where decisions have been made to go in one direction (that led to the present state) and explore at those forks of the road the outcome associated with the other choices. This strategy is too preliminary at this point, and will be discussed in a coming version or a subsequent writing on this topic.
3.1. On the Possibility that “There is Nothing There”
Curiosity drives us to explore the parts of reality that are immaterial for our survival, but one cannot count out the possibility that there is nothing there. The only theory of reality that can survive the absence of a purposeful designer is reality without features. Every particular feature, like say the fixed speed of light, brings up the question: why so, who selected this measurement, for what purpose? If reality were to be a perfect random expression of events then reality would be featureless and unchallenged by ‘why so’. How can reality be a perfect random stream of events while we humans see it feature-full? A newly developed cipher, Replica, [15] offers an answer. In it a reader is using a template to discriminate between incoming ciphertext letters, thereby interpreting the inflow according to the template. If the inflow is perfectly randomized the built-in reader template will discriminate within the input flow and interpret it as non-randomized and as template-wise. Darwin’s evolution built such a reading template in us, and hence we interpret a perfectly randomized and featureless reality, as feature loaded, and rules-compliant. It is not reality that needs to be explored, it is our reading template. Side note: the first and second commandments in the holy Bible say: “I am your God, and you can’t, and should not attempt, to attribute any features to me”. Quite consistent with the possibility that we are barking at the wrong tree, reality has no secrets, our Darwinist reading template does.
4.0. Scaffolding for a Richer Reality
A sculptor prepares a scaffolding to fit in it the big block of marble they bring from the mountains and intend to carve to a piece of art. Natural sciences in general and physics in particular refer to space as such a scaffolding for holding the elements of reality.
It was Albert Einstein who pioneered the idea that space needs to be generalized from its Newtonian straight forward construction into a reality-defining factor where curvature and deformation are introduced in order to describe observations that defied any other explanation. By using space malleability Einstein managed to offer a plausible explanation to the mystery of gravity.
How much further can we advance by allowing space to absorb any and all observational oddities? Would it be too much to further abstract the analysis by regarding reality as comprised of space and content -- two building blocks?
To serve that aim we further lay out space to be defined in its most abstract form: a set of s locations with 0.5s(s-1) distances between them.
We choose to limit these distances to rational numbers because irrational numbers rely on the ambiguity of continuity, while rational numbers maintain the clarity of the natural count.
We define the content of space as a set of occupants where each occupant occupies a single location in the space.
The (s-1) distances from a given location to the other (s-1) locations is the full and only definition of a location. No attributes of size, shape, color, fabric etc. are not given, nor needed. The space (the set of s locations, by habit also called “points”) is internally defined by the distances between all its points which we refer to as locations. This internal mutual definition is apart from any external observer offering their own characterization.
We can define now an unbound space (or unbound geometry) as a space where the 0.5s(s-1) distances thereto are all of arbitrary values, and every distance in the unbound space can assume any positive rational value whatsoever, unencumbered, unconditioned, uninfluenced by any of the other 0.5s(s-1)-1 distances.
Returning to the scaffolding analogy we allow for a scaffolding configuration of any size, shape, or complexity. This unbound space relates to Euclidean and non-Euclidean spaces as private cases; as an unbound space after bounds have been laid. Note that an unbound space does not have to abide by the triangle inequality. Namely the distance from location A to location B can be larger than the sum of distances from location A to location C and location C to location B.
Space serves as the place of happening, happening happens through space travelers. Space is the residential territory where residents reside, roam and move about. The most that our imagination enables us so far is to abstract reality to residences (space), and residents (occupants of space). As we strive to construct a scaffolding that would hold whatever reality is there to be held, we insist on unbound space, and in parallel insist on unspecified space occupants. Occupants are minimally defined as entities that occupy one location or another of space.
The occupants of the space are entities identified through their residence -- the identity of the location in which they reside. No other attributed is needed, none supplied. Since we use the location address as the means to identify the occupant, if two occupants oa and ob have a mutual distance d(oa, ob) > 0 but share distance values with all other locations in the space then they will be seen as a single occupant because they are not distinguishable within the space.
So far we defined a space comprising s locations with specified 0.5s(s-1) distances (rational numbers) between them, where from the point of view of the space it houses o ≤ s occupants (one occupant per location).
Repeating: neither the locations, nor the occupants are defined beyond the above definition.
We regard the combined entities of space and occupants as an Unbound Reality Architecture (URA).
4.1. Rethinking Time
Albert Einstein robbed us from a very pleasing and relaxing notion: a universal clock. Space has shape and complexity, material has fabric and complexity. By contrast time comes to us through the most elementary mental notion: counting: 1,2,3,... It would be advantageous to have a reality associated with a universal clock. Is there a chance? Einstein, arbitrarily, we dare say, decided to crown the speed of light as the universal constant, and in order to accommodate it he robbed us from an unstretchable space and a universal time. The mathematics is beautiful, the puzzle fit perfectly, and we are all at awe, as if a master painter pained the skies. Alas, our sense of beauty is a product of the randomized evolutionary track that brought us here. If evolution were to start again, it might yield different outcome with a different sense of beauty. If randomness is the core of reality then any specificity is a private case, not a general one.
A universal clock is such a simple and attractive feature that on the occasion of returning to the drawing board and re-thinking space, we may try to redesign it with a universal ticking clock in tact. We expect reality to evolve, to change, and do so in a clear single direction. Granted we may speak like this because we are so ingrained with the concept of time, but be it as it may, we introduce a reality-wide counter: time
We envision a reality wide clock ticking 1,2, 3, ... and its count is seen clearly with no distortion by all the locations in the space. With our definition of space this is easy to do without unsettling old man Einstein. The reality wide counter will be ticking in a. dedicated location in space -- location T. The unbound distance between point T and any other point in space will be the same. So even under Einstein’s argument about the speed of information, the universal clock will be shared, same value throughout the space.
We define speed. An occupant o is found in location y in space at time ty. At time tz occupant o is found in location z which is at a distance dyz from location y when o arrives there. We say then that occupant o moved from location y to location z at a speed V:
V = dyz/(tz - ty)
Note that no actual movement is recorded. Occupant o is seen in location y at time count ty, is not seen anywhere in times: ty+1, ty+2, ..... tz-1, then it is observed at location z at time tz.
When an occupant hops from location y1, to y2, ... yt then it does so by moving at a succession of speeds V1, V2, ... Vt-1 where:
Vi = dii+1 /( ti+1 - ti )
And the average speed V1t:
V1t = (Σ dii+1) / (tt - t1)
4.2. Unbound Space
We define unbound space as a set S of s locations (points) with d* distances: d* = 0.5s(s-1) all non-negative rational numbers: dij for i,j = 1,2,...s. The self distance dii is zero:
dii = 0
At any universal time count t the d* distances are unbound. Each distance is not limited in any way by any of the other d*-1 distances, nor by any distance values at any time count t’ < t.
Location i ∈ S is fully and completely defined by the d* distances from it to all other locations in the set (the space).
At time point t’ the unbound space U’ is comprised of s’ locations with 0.5s’(s’-1) distances between them. At time point t” the unbound space U” is comprised of s” locations with 0.5s”(s”-1) locations between them. The two spaces overlap such that location i’ ∈ U’ is associated with location i” in U”.
We define the space evolution table, (SET), E, as the table that lists the overlapping locations in U’ and U”. This table looks as follows:
s’i -- s”i
s’j - ø
ø -- s”k
s’j - ø
ø -- s”k
Namely s’i was mapped to s”i, sj in U’ disappeared and is not appearing in U”, s”k shows up in U” but has no match in U’.
So defined we call this unbound space ‘flat’. It can be depicted on a flat surface. Nonetheless we can map any Cartesian multidimensional space onto this flat unbound space.
We now add the notion of ‘depth’.
4.2.1. Deep Unbound Space
Since a location x on unbound space U of s locations is fully defined by its s-1 distances to the other s locations in U, one can add a point x’ such that:
dx’i = dxi. for i=1,2,...s; i≠ x’
From the point of view of the other s-1 locations points x and x’ are one and the same. Alas, point x and x’ may be related through a non-zero distance 0 < dx,x’. In fact there may be an arbitrary number g of locations w1, w2, ..... wg for which:
dwj,i = dwk,i. for i=1,2,...s. i≠wj and j,k = 1,2,...g
The space U will be blind to the fact that there are g locations where there appears to be only one.
The g locations that appear as one for set U may be regarded as ‘depth set’ of locations, G, relative to set U. In the depth set G, the g locations are mutually defined through 0.5g(g-1) distances, much as U is defined.
The depth set of locations may be larger, smaller or same size as the set in which it hides. We use the term hides because from the point of view of the ‘super state’, U, the depth state is hidden by virtue of all its locations sharing the same set of distances with all the members of U.
Every location in U may spawn a depth space of any size.
Any location in a depth set can spawn a depth state relative to the depth state of which it is a member. This can continue iteratively indefinitely.
This depth-loaded unbound space, (DUS), is designed as a disengagement of the legacy idea of space, impressed upon us by the three dimensional world we appear to be living in. It is a proposition (one of many) to express the intuitive notion of roaming area for elements of reality, making this area as unbound, as non pre-constrained as possible.
Shared Reality: all the occupants in a depth set are seen as one by the hosting set, and from the point of view of the depth set the hosting set is a shared reality. All the occupants in the hosting set have the same distances as seen by the occupants of the depth set. In other words, the hosting set is the shared reality for all the members of the depth set.
Depth and Complexity: The deep space is the way complexity is represented in the unbound space. It is open ended. A single occupant in the host set may become as complex as desired through its structure in the depth set, what is more, depth set are iterative.
4.2.2. Line, Straight lines, Functions, Dimensions, Circles
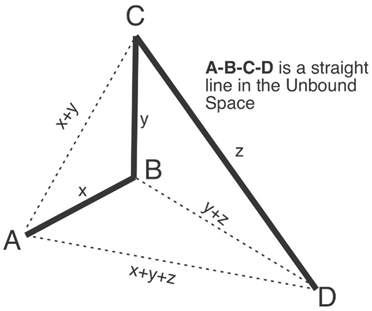
A series of locations L1, L2, ..... Ln is considered a well-ordered line, or simply line.
If L1 = Ln than the line is considered closed.
A line is said to be a straight line if:
for all values of i,j = 1, 2, ...n
dij = di,i+1 + di+1,i+2 + ... dj-1,j
Obviously points marked on an Euclidean straight line are regarded a straight line in an unbound space.
Two (or more) straight lines A, B are considered beams projecting from the same origin location o, if:
where a1 and b1 are the first locations in lines A and B respectively. Two lines A and B will intersect in location s if for some point ai and some point bj it holds: s = ai = bj Two beams on an unbound space, A and B, both defined with n locations are spread in an angle α if the Euclidean triangles drawn from d(o, ai), d(o, bi), and d(ai, bi) computes to having an angle α opposite the ai-bi segment for all values i=1,2,...n.
o = a1 = b1
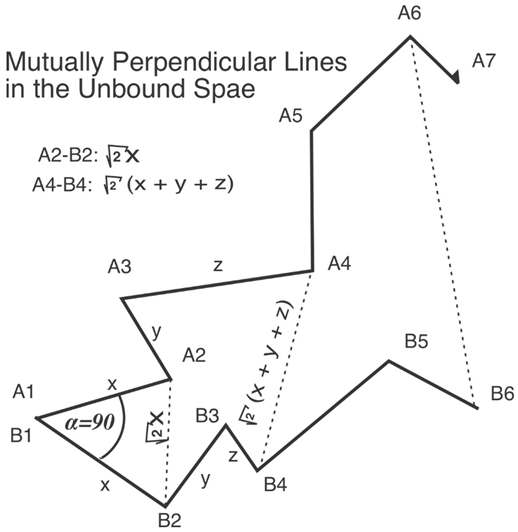
Similarly for two intersecting straight lines.
In the case where α=90o the two intersecting lines or two beams are considered perpendicular to each other.
Since the distance between locations ai, bi is rational, and for α to be exactly 90 degrees the distance on an Euclidean plane is an irrational measure, square root of 2, then the perpendicular lines on the unbound space will be said to be perpendicular to the measure of the distinction (error) between a selected rational distance and the square root of two.
N straight lines which are mutually perpendicular to each other will represent an Euclidean N dimensional space.
A function y=f(x) will be represented with a line F on the unbound space by drawing two perpendicular lines X and Y, such that when from any point of F one draws a perpendicular line towards line X such that the intersection (shared location) is x’ and when from the same point on F one draws a perpendicular line towards line Y that intersects Y at point y’, then it holds that y’ = f(x’).
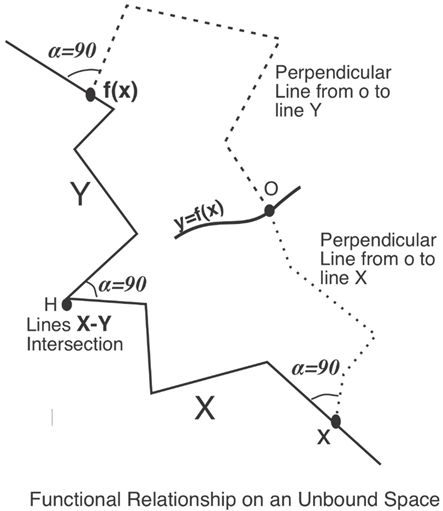
Any line L comprising n point L1, L2, ..... Ln can be regarded as a circle by adding a location L0 to the unbound space such that:
d(L0, Li) = d(L9, Lj) for all combinations of i,j=1,2,....n
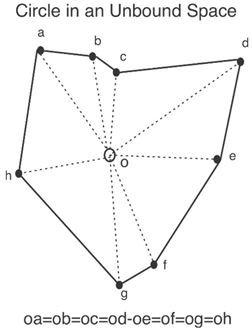
A subset S comprising S1, S2, ..... Sn locations in an unbound set U is considered a multi dimensional circle by adding to the space U a center for that circle S0 such that:
d(S0, Si) = d(S9, Sj) for all combinations of i,j=1,2,....n
4.2.3. Combined Curved Spaces
An unbound space can be built as a compendium of classic curves spaces. Let F be an Euclidean flat surface, P be a positive curvature surface, and N be a negative curved surface. Let us mark f points on F, p points on P, and n points on N. These f+p+n points may be cast as f+p+n locations in an unbound space where the distances between the f points comply with a flast surface, the distances between the p points comply with a positive surface, and the distances between the n points comply with the negative curvature surface. These f+p+n points may define an unbound space. The distances between the f points and the p points, or between the n points and the f point, or between the n points and the p points are not constrained in any way.
The three surfaces may intersect in a single point that will be part of the three spatial options.
It works backwards too, an unbound space U is comprised of three parts F’, P’, and N’.
U = F’ + P’ + N’
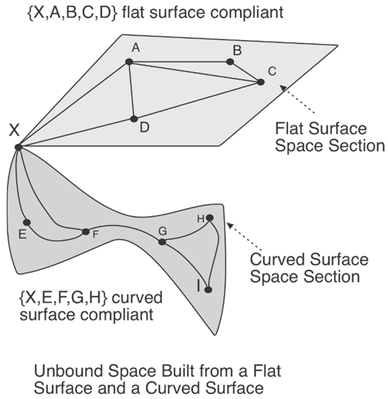
Part F’ becomes bound by fitting into a flat surface, part P’ becomes bound by fitting into a positive curvature surface, and part N’ becomes bound by a negative curvature surface.
U =. Crv0(F’) + Crvp(P) + Crvn(N’)
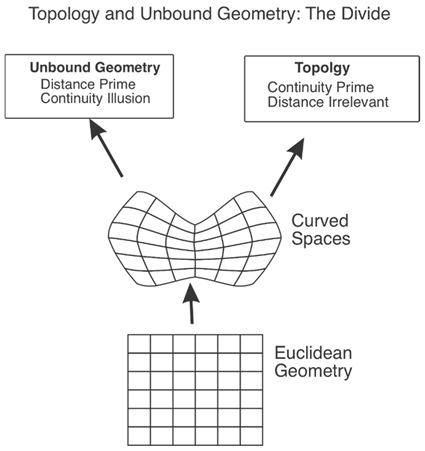
4.2.4. Topology v. Unbound Geometry
Projective geometry and topology emerged from classical geometry regarding Euclidean continuity as prime, while distances were thought of as irrelevant. Continuity and disconnects defined a new picture of space and geometry. Unlike physical lines which can always be represented as blurred discrete items of sufficiently small size, the mathematical notion of continuity is absolute, namely infinite: it cannot be represented with discrete elements. It was considered a triumph of human imagination over factual observation. Continuity gave us irrational numbers, gradation of infinity and calculus per se. The products of topology are very visual and well becoming, alas continuity is essentially less sharp and not as clear as the count of natural numbers. Thus the pursuit of clarity offers a better route towards hidden knowledge. At the very least Unbound Geometry should stand shoulder to shoulder with topology.
4.3. Occupants
Space is the place where things happen. Happening is expressed through entities that occupy locations in space and move to different locations as the universal clock ticks away. Having defined the unbound space, we now set to define space occupation.
An occupant is identified by the location it occupies at a given time point. Much as the location is the building block of space, so the entity that occupies a single location is the building block of the content of space -- the set of occupants.
We can define an unbound space U as an empty space, in which there are no occupants. We can then place an occupant, O, in location x in U, and do so at time clock tx. Entity O may show up in location y at time point ty. If between tx and ty O did not appear in any location in U then we say that O traveled from location x to location y at speed v = dxy/(ty-tx) where dxy is the distance from location x to location y.
As described space U was empty from tx+1 to ty-1. A ty O is showing up in location Y. The space is associated with a space evolution table (SET) which indicates that occupant O which occupied location x at time point tx is occupying location y at time point ty.
We thus define an unbound reality model in which occupants of space locations can appear, disappear, change locations, totally unbound except that no two occupants may reside in the same location (as seen by the location defining space).
So defined we have a framework, which is also called scaffolding which is designed to represent any situation in physics we are interested in, from macro cosmos to microcosmos. Our aim is to represent the experimental and observational data at our disposal in a framework so broad that should we encounter a surprising new observational data, we will not have to rebuild the framework, the space, nor to add dimensions and such like. Our unbound space, and unbound reality model will be able to log and accommodate any future measurement surprise. It might not work, but this is the objective.
4.3.1. Bodies
A body is a collection of occupants. Two bodies are considered one and the same if they are defined through the same list of occupants on an unbound space at a given point of time t. Two bodies may partially overlap if there are some occupants that belong to both bodies. In general bodies are taken as distinct, in as much as not having any occupants in common.
Rigid Bodies: We identify rigid bodies, as a bodies where the distances between their constituent occupants are fixed over time. The distances from the constituents of the body to the other locations in the space may change over time.
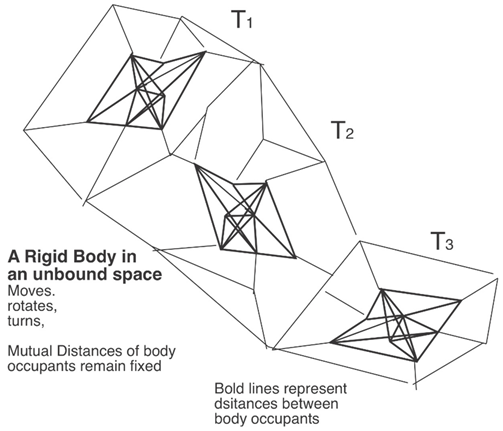
4.3.2. Mass
An occupant X in an unbound space U is said to have a mass Mx where Mx represents the count of occupants in the depth spaces that spawn from occupant X.
Example: if point x in unbound space U is in fact a depth subspace D that comprises d locations and m occupants where q occupants among the m spawn each depth spaces that contain each q occupants, then the mass of point x as seen from U is: (d-m) + mq
4.3.3. Flavors
Occupants like locations are set to be uniform and indistinguishable except that locations are defined through their set of distances to other locations in the space, and occupants are defined by the location they occupy. To the extent that flavors will be desired for occupants, they will be arranged through depth spaces, where each occupant in an unbound space will be in fact a depth space of particular attributes. This occupant will look singular to the space it is in, but will be projecting a flavor owing to the way it is structured in the respective depth space.
Flavors may be needed to represent the equivalent of positive and negative versus neutral basic element.
4.4. Motion
Changes, events, activity is the ‘life’ of reality. In our unbound reality motion is expressed through appearance or disappearance of occupants in space locations.
An occupant o will appear in location A at time point ta. This occupant was not recorded in the unbound reality at any time before ta. This is regarded as occupant birth.
An occupant o occupying location D may carry out an act of disappearance at time point td, such that at time point td+1 location A will not be occupied by occupant o
Disappearance as described can be permanent, namely occupant o will not reappear in the relevant unbound reality, or it may be temporary, namely occupant o will reappear as occupying location A at time poin ta.
The latest case is an act of motion. The occupant moves from location D to location A. It takes the occupant a travel time Δt(D→A) = ta - td, in which the occupant travels the distance from location D to location A; d(D,A).
We define the velocity or speed of the motion, V(D→A) as:
V(D→A) = d(D,A)/(ta - td)
An occupant o may move on a line L: L1, L2, ..... Ln with variable speeds. V1, V2, ..... Vn, where:
Vi = d(Li+1, Li)/(ti+1- ti)
The motion of occupant o on line L will be called uniform if:
Vi = Vj. for all i,j=1,2, ....n
The motion of occupant o on line L will be an accelerated motion if:
Vi < Vj. for all i < j. for i=1,2, ....n-1, and j = 1,2,...n
And reverse for decelerated motion.
Occupants may be moving at constant speed (at any speed). Let the fixed speed be Vf. To accomplish the fixed speed the distance between location of departure, D and location of arrival, A, d(D,A) will be marked according to the relation:
d(D,A) = Vf * (ta - td)
Where ta and td are the times of arrival and departure respectively.
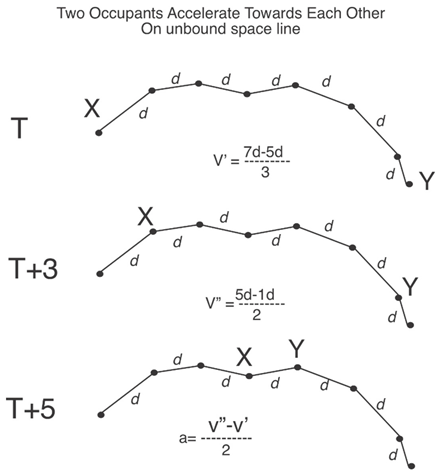
This value for the distance between locations A and D is valid only for time ta. If D spawns depth space then all the locations of the depth space share distance from all locations in the super space, included distance from location D. There is no limit on the number of fixed velocity occupants, nor to the value of their velocity.
An occupant traveling along a closed line in fixed speed may serve as a circular clock.
Two occupants X and Y of masses Mx and My respectively may experience a decrease in their mutual distance d=dXY(t) that is commensurate with Newtonian attraction. In that case X and Y will be said to experience a mutual force F = G*Mx*My/d2, which will generate accelerations F/Mx and F/My on X and Y respectively.
A symmetric definitions will apply to electrical rejection forces between bodies X and Y.
4.4.1. Motion of Rigid Bodies
A rigid body, comprising r occupants with fixed 0.5r(r-1) distances between them is occupying r locations at time td: L1, L2, ..... Lr. At a later time ta the body occupies locations L’1, L’2, ..... L’r. The mutual distances between the body occupants are the same at time td and at time ta -- as follows from the rigidity of the body.
If
then the motion of the rigid body is said to be taking place in a uniform region of the unbound space, and the motion of the body is said to be straight.
d(Li, L’i) = d(Lj, L’j). for all i,j = 1,2,..t
A rigid body can move in a circular way around a center of rotation C if for every occupant i of the rigid body it holds that the distance d(Li, L’i), is the size of the arc defined by the two straight lines: C-Li. and C-L’i and the angle α between them. The value of α is the same for i=1,2,...t.
If C is occupied by an occupant which is part of the rigid body then the rigid body is circulating around itself.
A rigid body may circulate or rotate in a fixed speed where the occupants that are further from the center C are moving proportionally faster than occupants who are close to C.
Body to Body Attraction: A body X comprising x occupants X1, X2, ..... Xx, and a body Y comprising y occupants: Y1, Y2, ..... Yy may experience Newtonian attraction expressed as mutually diminishing distances if all the distances between every occupant in X versus every occupant in Y will be diminished at the same rate. If these distance reductions are manifest over an unbound line, such that the occupants occupy successive locations in a pace described by some acceleration a= ΔV/Δt, then body X is said to be attracted to body Y with a force Fx =. Mx * (ΔV/Δt). where Mx is the mass of body X. And similarly for Y. Reverse symmetry for mutual body rejection.
Anti-Occupants: Since the spread of occupants in space is described without any attributes neither to occupants, not to their location, it is therefore possible to flip occupants to non-occupants and vice versa.
5.0. The Turn of AI
When science discovered that a few concise equations describe what is happening around, it led to enormous sense of power. The fear of God abated. When Napoleon asked the preeminent scientist Pierre Simin Laplace where God fitted into his scientific work, Laplace, arrogantly replied: “Sir, I have no need of that hypothesis.”‘ Karma: now comes AI and declares there is no need for natural law equations in our new world view. Data speaks, raw, detailed, unreducted. In past centuries we built steam engines to carry us much further than our biological (Darwin incubated) limbs allowed; in the past decade we build inferential engines to carry us much further than our biological (Darwin incubated) mind allows.
We come to recognize that our picture of the universe, large and small was an extension of our daily experience, not very large, not very small. We deal with small stones we through and watch their trajectory, we deal with water flow, with waves; we deal with energy, and mild speeds, and we do build on these daily experiences to construct models of the very large and the very small. We further use our sense of beauty and intuition to guide us as to how reality in far away places is like. On further thought this imposition of our daily experience on the far and away creates a filtered and distorted view of reality, and the further we dig into this filtered and distorted view the further we move from a fair picture of how reality is as viewed from a place not contaminated by these distortions.
To remove these distortions to the extent possible, we turn to the emerging technology of AI. Focusing first on the ground floor of AI -- supervised learning -- we envision a knowledge base which is used to come up with answers to related questions -- replacing the answers we computed from our equations. It is a much more laborious method. The equations offer a shortcut, without them the full body of details must be put to work to answer each question. Unsupervised learning and reinforcement learning are next in line.
Progress with the AI approach is much faster because there is no fixed set of approved equations like we have today. Each observation adds to the database and refines the result.
To do it right we need (i) optimally represent the inference database, (ii) apply an effective inference engine, and (iii) develop use strategy for (a) practical purposes (convenience, survival), and (b) fundamental curiosity -- how things are beyond our distorted view of them, (iv) set up an on going improvement strategy to do better and better with our AI capability.
5.1. Representing the Raw Database
AI inference works best over a uniform situation wherein all the cases and instances which are part of the raw data are cast onto the same framework, F, which determines a target function, Y, through n parameters, or facotrs x1, x2, ..... xn:
Y = F(x1, x2, ..... xn)
The data is packed into m cases:
where M is AI analyzed to predict Y0 for a question case x1,0, x2,0, ..... xn,0 which is not part of the database but has an array of similarities with the contents of the database.
M={ Yi = F(x1,1, x2,1, ..... xn,1)} for i=1,2,...m
The uniformity of F is critical for the efficacy of the AI learning. And therefore it is important to fit the experimental results, the observations, regarding the behavior of reality into a framework that would be broad enough to allow all the variety of observations to fit in the same framework. That was the motivation for the deep unbound reality architecture (DURA) presented here.
The space representations used today in physics, Euclidean, Non-Euclidean, all metric spaces, of any number of dimensions as well as the emerging quantum spaces, are all representable with DURA. In fact they all can be defined as a set of constraints on DURA. The AI inference will therefore start with DURA and gradually apply limitations on it to become a reality architecture as reflected from the growing database.
5.2. Apply the Effective Inference Engine
The preliminary instances of experimental work have been done using the BiPSA methodology in which the neural network is applied to provide its inferential conclusions as a series of answers to binary questions. It is a much longer process than non-binary questions but it has its advantages.
The limitation on AI today and in the foreseeable future is energy and time. These two resources are in greater and greater demand the more work AI is asked to do. So it looks like that the progress of the deep understanding of natural science in the immediate future will be bound by the availability of energy and by our patience with time.
5.3. Return to Equations
When adults explain complicated matters to children they use simplified language. Similarly when AI inference engines communicate to humans they strive to develop simplified equations and formulas to put humans at ease. So that when AI analyses dynamical systems as in the rock throwing example, they may reduce the complexity of the algorithms to the familiar Newtonian equations that are humanly digestible. Similarly with respect to cosmology and microcosmos.
5.4. Progress without Paying with Clarity
A lot of progress in mathematics and science has been claimed at the expense of clarity. Clarity is axiomatic, it cannot be explained in a “clear” way. It is very important as a shared communication platform. The clearer a concept, the more broadly it is shared. And after all, the body of science and mathematics rely on widespread consensus -- clarity. So while a great deal of progress has been gained for a moderate loss of clarity, following InnovationSP : innovation science methodology, one explores the proposition that same progress can be claimed without losing clarity. One of the fundamental notions where clarity was compromised is continuity. A continuous Euclidean line is a series of points. Alas, non-dimensional points when added one next to the other are still non-dimensional. This clarity gap gave us irrational numbers, and established velocity as a fundamental notion. The unbound space models are free of irrational numbers. The models (DURA) replace the intuitive notion of velocity with a sequence of states, punctuated by a universal clock. It is not yet clear if this attempt can replicate the progress achieved with the cost of clarity, but it is worth looking into it.
5.5. Developing Use Strategy
In the large picture DURA is to be used both for practical purposes and for curiosity satisfaction. It should be easier to apply for the first category because the latter is quite open. Also AI is more effective in a supervised learning mode than in unsupervised mode. At this stages AI is used only to test how close the inference comes to results expected from solid theory.
Sideways Illustration: Given a series of three sequential measurements: 12, 18, 24. What is the ‘theory’ for the next measurements? The natural ‘human’ theory is to add 6, namely: 12, 18, 24, 30, 36, 42. Subsequent measurements validate the theory. Alas the series is governed by the formula:
Xn = Pn+2 + Pn+3
Where Xi is the i-th measurement and Pi is the i-th prime number. However for the 6 first measurements the true theory overlaps with the natural human expectations. This results in human confidence in their theory. The 7th measurements is where the ‘theories’ differ: 48 v. 52. In nominal science the “add 6” theory would be regarded as the established ‘law’ until the 7th measurement is taken. In an AI setting the human theory, the true theory, and perhaps some other theories will be regarded each with a certain probability to be the right one. No pretense to discover the ‘right law’. And when the 7th measurement comes forth the probability of the true theory is rising.
6.0. Review and Outlook
The more we know the more we believe that we know almost all there is to know, and while we are open to learn more and more details about nature, we are not prepared to accommodate the premise that our entire edifice of science is wrong headed, and that there are vast parts of reality that are undetected and unsuspected but are what is actually there and by ignoring these parts we are locked into a deformed and an approximate picture of reality.
This bold proposition to do away with theories of nature and tight neat equations that rule the world is a hard sale. We are so deeply ingrained with the configuration of having a story about particles and waves, mass and energy, space and time and then attaching to this story a quantitative equation that can be used to predict measurements and understand reality. It is very challenging for us to embrace the idea that nature does not run according to a script that we who were incubated in Darwin’s egg came up with. Reality does not confine itself to descriptions that make sense to us.
It is extremely demanding of us humans to consider the proposition that stories about particles and waves, energy momentum etc., are limiting us to linear evolution and foreclose on us the off-sides: the turns and roads in the territory of reality which did not introduce themselves to us during our Darwinist incubation process.
How painful is it to wrestle with the weirdness of quantum physics where our common sense is at odds with the mathematical beauty that rules the field. No, we are not quite ready for the conclusion that scientific theories and mathematical equations offer blurry vision of reality, and should be replaced by the emerging technology of artificial intelligence where combined with the newly discovered power of quantum computing will be able to provide the service and insight formerly provided by nice concise equations.
The objective of physics ahead may be to build theory-replacement AI machines and theory replacement raw databases that together will work efficiently and revealingly towards the twin goals of supporting human survival and indulging human desire to understand the reality which we call home.
References
- Innovation Solution Protocol, www.InnovationSP.net.
- Metaverse Oriented Geometry. https://www.researchgate.net/publication/360084946_Metaverse_Oriented_Geometry.
- “Improving AI System Awareness of Geoscience Knowledge: Symbiotic Integration of Physical Approaches and Deep” Shijie Jiang, Yi Zheng, Dimitri Solomatine . https://agupubs.onlinelibrary.wiley.com/doi/full/10.1029/2020GL088229. [CrossRef]
- “On scientific understanding with artificial intelligence”. Mario Krenne et al. https://www.nature.com/articles/s42254-022-00518-3. [CrossRef]
- “AI-assisted superresolution cosmological simulations”. Yin Lia,b,1 https://www.pnas.org/doi/pdf/10.1073/pnas.2022038118.
- “Machine Learning for Topology Optimization: Physics-based Learning through an Independent Training Strategy” Fernando V. Senhora et al. https://www.sciencedirect.com/science/article/pii/S0045782522003036. [CrossRef]
- “Integrating Physics-Based Modeling With Machine Learning: A Survey” JARED WILLARD∗ et al. https://beiyulincs.github.io/teach/fall_2020/papers/xiaowei.pdf.
- “Machine Learning in the Search for New Fundamental Physics” Georgia Karagiorgi, et al. https://arxiv.org/pdf/2112.03769.
- “Artificial intelligence: A powerful paradigm for scientific research” Yongjun Xu et al. https://www.sciencedirect.com/science/article/pii/S2666675821001041.
- “The death of the short-form physics essay in the coming AI revolution” Will Yeadon, https://iopscience.iop.org/article/10.1088/1361-6552/acc5cf/meta.
- “Development of multimodal machine learning potentials: toward a physics-aware artificial intelligence” T Zubatiuk, et al. https://pubs.acs.org/doi/abs/10.1021/acs.accounts.0c00868.
- “On scientific understanding with artificial intelligence”. M Krenn et al. https://www.nature.com/articles/s42254-022-00518-3.
- “Complexity, A Guided Tour”. Melanie Mitchell, Oxford University Press 2009.
- “ Science needs reason to be trusted” Sabrine Hossenfelder, Nature Physics volume 13, pages316–317 (2017). [CrossRef]
- “Replica: An Improved Communication Concealment Cipher”, US Patent 11,716,192.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
MDPI Initiatives
Important Links
© 2024 MDPI (Basel, Switzerland) unless otherwise stated





