Preprint
Article
Proof of Goldbach's Conjecture
This version is not peer-reviewed.
Submitted:
26 September 2024
Posted:
29 September 2024
You are already at the latest version
Abstract
The paper presents two novel mathematical approaches to prove Goldbach's conjecture. The conjecture states that every even number greater than or equal to 6 can be expressed as the sum of two odd prime numbers. We propose that the conjecture has only four genetic codes, and as long as one of them remains valid, the conjecture continues to hold. Through these new mathematical ideas, we aim to prove the validity of Goldbach's conjecture comprehensively.
Keywords:
Goldbach Conjecture
; Prime Numbers
; Mathematical Proof
; Number Theory
; Odd Prime Numbers
; Composite Numbers
CONTENT
Section 1,a new mathematical idea to prove Goldbach's conjecture----3
Section 2, Insufficient approximation formula of the number of primes in the [n, 2n]-------------------34
Section 3, Goldbach's conjecture 2n=pi+pj,the basic properties of identity 2n=q+(2n-q) and Hou Shao-sheng's theorem--------43
Section 4, 4 inferences of Hou Shaosheng's theorem-----------------67
Section 5, The 4 genetic codes of the conjecture, the proof of Goldbach's conjecture---------------------83
Section 6, Summary of the proof process of Goldbach conjecture----176
References--------------------------------------------------------183
In 1977, the sufficient and necessary condition theorem of the conjecture was proved, and in 2016, it was proved that the conjecture has only four genetic codes, and as long as one genetic code exists, the conjecture continues to be true! Hou Shaosheng has theoretically proved that the genetic code Impossible to interrupt! This is the fundamental guarantee and mystery of the conjecture!
No scientist had theoretically proved that the genetic code of living things Impossible to interrupt.The sad history of the Chinese nation without mathematical theorems is over forever!! Hou Shaosheng invites mathematicians from all over the world to review and wait to answer any questions from the experts.
By Hou Shaosheng
侯 绍 胜 著
Section 1, New mathematical idea to prove Goldbach's conjecture
Abstract In 1742, the German mathematician Goldbach, in a letter to Euler, the world's great mathematician, put forward two conjectures, in slightly modified language, which can be expressed as follows: A: Any even number that is not less than 6 is the sum of two odd prime numbers (that is“”). B: Any odd number that is not less than 9 is the sum of three odd prime numbers.
This became known as Goldbach's conjecture. Euler hopes future mathematicians will prove the conjecture.Since conjecture B is A corollary to conjecture A, the conjecture mentioned in the following article refers to conjecture A, and the proof conjecture refers to proving conjecture A.Since there are an infinite number of n's, Goldbach's conjecture is an infinite conjecture. The difficulty in proving the conjecture is that you have to prove it for every even 2n. The title of this paper is: New Mathematical Ideas to Prove Goldbach's Conjecture. Now, this paper presents two new mathematical ideas to prove conjectures. They belong to two different mathematical idea systems,this paper discusses the topic in the end.
2n, 2 (n+1), Goldbach's conjecture is true, so Goldbach's conjecture has a genetic code just like humans and living things.
This paper proves that Goldbach conjecture has only 4 kinds of genetic code, and as long as there is 1 kind of genetic code, the conjecture can be maintained.Hou Shaosheng proved in theory that there are at least three kinds of genetic codes exist, which proved Goldbach's conjecture completely and comprehensively in theory.
The genetic code of Goldbach's conjecture, which is theoretically proved to be impossible to interrupt, is a major breakthrough in mathematical theory.No one has theoretically proved that the genetic code of living things to be impossible to interrupt.
In the follow-up paper, 8 mathematical examples are randomly given. With these 8 mathematical examples, all the theories above are tested. The examples are completely consistent with the theories.
[Document ID] A [Document number]
1.1.1. Goldbach Conjecture is briefly Introduced.
Goldbach's conjecture is expressed mathematically as: 2n=pi+pj, pi≤pj,(3≤n∈N).
p represents the odd prime number, pi and pj respectively represent the ith odd prime number and the jth odd prime number, N represents the set of natural numbers.
Someone checks every even number up to 3 × 106 one by one, the conjecture is valid.
Since there are infinitely many even numbers, Goldbach's conjecture is an infinite conjecture. Goldbach's conjecture can never be proved by computer verification alone.
In 1900, German mathematician Hilbert held in Paris the second International Congress of Mathematics speech, Goldbach conjecture as the past legacy of one of the most important problems, introduced to the 20th century mathematicians to solve. In 1912, at the Fifth International Mathematical Congress held in Cambridge, the German mathematician Landes, in his speech, the conjecture of A as one of the four unsolved problems in prime number theory was recommended. In 1921, the British mathematician Hardy said at the mathematical Congress held in Copenhagen that the difficulty of guessing A could be compared with any unsolved mathematical problem. Goldbach's conjecture is therefore not only number theory, but also one of the most famous and difficult problems in mathematics.
In March 2000, Faber and Bloomsbury jointly offered a $1 million reward for information, but no one has been able to prove the theory.
In 1963, Wang Yuan, Pan Seung-dong and Barbain all proved "1+4".
In 1965, A Vinogradoff, Buchtabb and the Italian mathematician Pombini proved "1+3".
In 1966, Chen Jingrun proved "1+2". He showed that any sufficiently large even number can be expressed as the sum of two numbers, one of which is prime, the other is either prime or the product of two prime numbers. This result is called Chen's theorem in the world, and is considered to be the "pinnacle of sieve development".
It is generally acknowledged in the world mathematical circle that it is impossible to prove the conjecture by the improvement of the past method, and a new mathematical thought is necessary to prove the conjecture. The Chinese Academy of Mathematics even believes that it is difficult to find new mathematical ideas to prove conjectures in several decades, even hundreds or thousands of years, let alone prove conjectures.
1.1.2. The study of the Goldbach Conjecture Is in a Dark Freezing Period
The history of proving Goldbach's conjecture can be divided into three stages.
The first period, from 1742 to 1920, involved many mathematicians in the verification of Goldbach's conjecture. But there is no theory to prove the conjecture.
The second period was from 1920 to 1977. In 1920, mathematicians proved 9+9, and many others followed until 1966, when Chen Jingrun proved 1+2. There has been no progress since.
The third period is from 1977 to the present. In 1977, Hou Shaosheng proved the sufficient and necessary condition theorem of Goldbach conjecture. And based on this theorem, after 29 years of preparation, the monograph "Proof of Goldbach Conjecture· Proof of Fermat's last conjecture " was published in 2006 in 1000 volumes. In 2007, two old professors at Sun Yat-sen University praised the proof of Goldbach's conjecture.
This proof of Goldbach's conjecture in the book is called proof Method 1.
In 2016, Hou completed the second proof of Goldbach's conjecture. And this proof, and the proof in the book, belongs to two different systems of mathematical ideas. A few years ago, I have posted this Chinese certificate on the Internet. At least tens of thousands of people have seen it, but so far no one can deny it.
The second proof of Goldbach's conjecture is called proof Method 2.
Wang Yuan was the president of the Chinese Academy of Mathematics and an academician of the Chinese Academy of Sciences. Wang Yuan has won the First Prize in China Natural Science, the Chen Jiageng Prize in Physical Science and the He Liang and He Li Prize in Mathematics. In 1963, he proved 1+4, and he signed and published Chen Jingrun's 1+2. He is the author of Wang Yuan on Goldbach conjecture. It is very appropriate to say that Wang Yuan is the representative figure of this stage.
In 1992, 4 academicians of the Chinese Academy of Sciences: Wang Yuan, Chen Jingrun, Pan Chengdong, Yang Le, held a press conference.For another 1,000 years, they said, it would be difficult to find a new mathematical idea to prove Goldbach's conjecture, let alone prove it!
In 2006, on CCTV4, Wang Yuan said, "I firmly oppose Chinese attempts to prove Goldbach conjecture! Because it's impossible!
Because of Wang Yuan's authoritative position, the proof of Goldbach's conjecture entered a dark frozen period: almost all magazines did not review the proof of conjecture! Almost all mathematicians consider conjectures unprovable! Even the reviewers hired by the magazine found no errors and dared not publish a proof of Goldbach's conjecture.
Hou once made a written agreement with West China Science and Technology Magazine: If the expert they hired could raise a problem and Hou could not solve it, Hou would send 10,000 yuan to the magazine; Otherwise, the proof of Hou Shao-sheng's Goldbach conjecture must be published in full. The magazine hired professors from two prestigious universities to review the paper and found no problems. The president of Western China Science and Technology magazine said that if it was published to you, it would be equivalent to the explosion of an atomic bomb in China, which would shake the whole of China and affect the whole world. We could not afford the responsibility. Not answering the phone after that.
There is no long night that will not end! There is never a day when the sun does not rise!
Now the proof of the Goldbach's conjecture: "The nature of the identity 2n=q + (2n-q) determines that the Goldbach's conjecture must be true", translated into English, about 180 pages of A4 paper. The vast majority of reviewers are not willing to review, afraid that after I admit, others find a mistake. To facilitate the magazine and reviewers, Hou had to publish a new mathematical idea to prove Goldbach's conjecture, with the support of some experts. After receiving some expert support, I will publish follow-up paper.
If any expert is willing to review the proof method 2, Hou will send you "the nature of the identity 2n=q+ (2n-q) determines that Goldbach's conjecture must be true." Any questions you may have are welcome. If there is no problem, please recommend and cooperate to publish.
1.1.3. The Proof of Goldbach's Conjecture Has Dawned
Hou Shaosheng's research, from the very beginning, focused on new discoveries, on linking theory with practice, on theoretical abstraction and mathematical proof of known facts, and always worked around sufficient and necessary conditions.
Sufficient and necessary conditions (n±Δ are all prime numbers) are not only the action guide for Hou Shaosheng to prove 1+1, but also the theoretical basis for judging whether Hou has proved 1+1.
In 1977, Hou Shaosheng proved the sufficient and necessary condition theorem of conjectures.However, the difficult task put forward, scared Hou a cold sweat. After 23 years of theoretical preparation, it was not until August 2000 that the first draft of the proof was completed.
Professors at more than 10 universities in China have reviewed Hou's manuscripts. Including Wang Tianze, China's first doctor of number theory trained by Chen Jingrun. Wang Tianze asked a question, Hou answered, he never asked a question again. But no university magazine dared to publish it!
Hou Shaosheng requested Anyang City mathematical Society to organize Anyang city mathematicians to review. From September 2004 to March 2005, after half a year of review, the mathematics society finally reported to the Anyang Municipal government in a red-headed document, admitting that no mistakes had been found. It is quite possible that Hou has proved the world-famous Goldbach conjecture, and the municipal government is requested to give high support and attention. We hope that the municipal government will hire famous experts at home and abroad to review it.
Henan Provincial Mathematical Society doubted the level of Anyang City mathematical Society, in 2005, Henan Provincial Mathematical Society reviewed again,Finally, the document admitted Hou Shaosheng's proof. And to recommend the document to the Chinese Mathematical journal and the Chinese science journal, I hope the journal published, let mathematicians around the world comment. The Chinese Mathematical Journal, however, requested that Wang Tianze's name be put first and Hou Shaosheng's name be put second, because Wang Tianze was an established mathematician. Goldbach Conjecture is the most famous mathematical problem in the world, Hou Shaosheng is not famous, the name in the first place, will not organize experts to review. Hou immediately accused them of using their power for personal gain and falsifying history.
On November 24, 2005, the editorial office of Today Science Yuan Magazine sent a book publication notice.
The notice said that the manuscript of "Proof of Goldbach's Conjecture" was submitted by our press to two academicians of the Institute of Mathematics of the Chinese Academy of Sciences for review. There was no objection, and it was decided to publish it officially by the national publishing house, Changzheng Press or Guangming Daily Press.
We think that the publication of the manuscript is of great significance for promoting the study of mathematics.
When you receive the notice of publication, please hurry up and raise the publication fund of thirty thousand yuan only so that the book can be published as soon as possible.
I hope you can continue your efforts to climb the scientific peaks and play an even bigger role in becoming a mathematical country.
China Association for Science and Technology
Editorial Department of Today Science Yuan Magazine (with official seal)
November 24, 2005.
Hou Shaosheng asked Anyang association for science and technology Association for support dozens, even hundreds of times, but there was no support. This time, the book publishing notice finally caused the attention of the city Association for Science and Technology, to the city leaders did a report.
After a debate, the Standing Committee of Anyang Municipal Committee finally decided to support Hou Shaosheng with 30,000 yuan and let Hou publish the book.
In 2006, Proof of Goldbach's Conjecture and Proof of Fermar's Last Conjecture was published by Guangming Daily Press in 1000 copies. One thousand Chinese books have been circulated to date, and no one has been able to deny the proof.
This proof of Goldbach's conjecture in the book is simply called proof Method 1.
Hou Shaosheng sent his monograph "The Proof of Goldbach's Conjecture and the Proof of Fermat's Last Conjecture" to Wang Yuan and others several times by registered mail. He also asked Wang Yuan's friends to bring books to Wang Yuan in the hope that Wang Yuan would ask questions. However, until his death, Wang Yuan did not respond.
In 2013, Hou Shaosheng1, Ma Linjun2 et al published "A New Mathematical idea to Prove Goldbach's Conjecture" and "Defects of Eratosthenes screen method ,and Ideal final screening method" in Beijing Today Science and Technology Journal.
Chen Jiazhong, vice president of the magazine and chief editor of the magazine, is very close to Wang Yuan. I asked Chen Jiazhong to give this issue to Wang Yuan. Wang Yuan promised, must give a statement. But until Wang died, he did not respond.
In April 2007, Li Baitian, a professor at Sun Yat-sen University, called Hou and said: 'Someone on the Internet said they had proved Goldbach's conjecture. We have reviewed almost every proof on the Internet and none of them is correct.' It only takes a few minutes, a few hours at most, to find their essential mathematical error. Now we need to review your testimony. Do you agree?
Hou Shaosheng and Li Baitian telephone agreement: if Li Baitian put forward a problem, Hou Shaosheng can not solve, Li Baitian can declare Hou Shaosheng's proof is wrong! If such a question cannot be raised, Li must confirm Hou's proof in writing.
In the afternoon of the same day, Hou Shaosheng gave Professor Li Baitian two books and asked Professor Li to invite another professor to review them. A month later, according to Li Baitian's request, another three books were given to Li Baitian.
Li Baitian and Ma Linjun, both born in 1932, began to teach at Sun Yat-sen University in 1957 and began to study Goldbach Conjecture. After more than six months of review, they have a question. Hou answered the question.Finally, the two old professors spoke highly of Hou Shaosheng's proof in writing.
(Note: The other three, not professors, did not sign. One of the three, Li Baitian's university classmate, also wrote a proof of Goldbach's conjecture and asked the two old professors to support it. The two old professors refused, pointing out that she had not solved the fundamental mathematical problem of conjecture. The researcher, who participated in the review of Hou's proof.
Written testimonies of the two old professors are as follows:
Certifying Materials
In the past six months, several other professors and I have carefully studied Hou Shaosheng's Proof of Goldbach Conjecture (hereinafter referred to as Proof). And raised a question, other than that, we haven't found anything else. Hou Shaosheng's answers to the questions raised were satisfactory and prompt beyond our expectation, which made us deeply impressed. His solution, together with part of the original text, satisfactorily proves that there are prime numbers with single digits of 1, 3, 7 and 9 in any interval [n,2n] (19≤n∈N). This is a very big problem in number theory, and one that is extremely difficult to prove. We are ready to recommend the relevant content for publication in the Journal of Sun Yat-sen University.
We believe that Hou Shaosheng proved that the mathematical thought of "1+1" not only conforms to the essence of the existing mathematical theory, but also has a series of innovations. The mathematical idea of how to prove "1+1" has been correctly answered. For more than 260 years, "1+1" can not be proved, and there is no correct mathematical thinking is the root cause. Famous mathematicians such as Wang Yuan and Chen Jingrun once expected to produce new mathematical ideas in the process of studying "1+1", Hou Shaosheng has already given answers to this question.
Hou Shaosheng and Wang Shunqing proved that all the composite numbers whose single digits are 1, 3, 7 and 9 are only the values of 10 functions. This is the theoretical basis for his proof of "1+1". Thus they easily answered the most fundamental distribution law of prime numbers: All integers whose values the 10 functions, and whose single digits are 1, 3, 7, and 9, are prime. They and 2 and 5 are all prime numbers, and others integers whose single digit are 1, 3, 7, and 9 are composite number.
Hou and Wang's theorems in the range of composite numbers, the theorems outside the range of composite numbers, and the theorems of sufficient and necessary conditions that positive integers are prime numbers have essentially answered the quantitative relation between any composite number and other composite numbers, and the quantitative relation between any composite number and other prime numbers, positive integers are necessary and sufficient conditions for prime numbers.
Since the conception of man and nature, man has been studying three relationships, that is, the relationship within man, the relationship within nature, and the relationship between man and nature.
Similarly, prime and composite numbers have been the basic objects of number theory since the conception of prime and composite numbers. The relationship between prime numbers, composite numbers, prime numbers and composite numbers is the basic question that number theory should answer, the first question.
It is now fair to say that Hou and Wang have answered these questions. This is a major breakthrough in the research of basic theories and a few big golden eggs laid in the process of "1+1" research. At the same time, it lays a reliable theoretical foundation for proving "1+1".
Hou Shaosheng divided n (≥3) into prime number and composite number at first, and when n is prime, "1+1" does not need to be proved. When n is composite, he divides it into 16 classes. Then for each class of composite numbers n, it is proved that there are values of the function △ (> 0), so that n±△ is a prime number, so 2n = (n+△) +(n-△) is the sum of two prime numbers.
Here he created an entirely new sieve: when he wanted to prove that a certain class of composite numbers n, such as n = (10a+3) (10b+11), must exist △ such that n±△ are all prime, he first screened out all the composite numbers {f = (10x+3) (10y+11)}. This makes the problem much simpler to solve (prove).
Then, in view of the particularity of n = (10a+3) (10b+11), the specific expression (function) of △ is given to prove that the value of △ must exist, so that n±△ is a prime number.
Hou shaosheng creatively used 8 equations (some 12) equations to form the equation system, making the equations both interrelated and restricting each other.
With its association (common solution), each desired value of △ is netted. Filter out all values that are not needed by its constraint. It is the culmination of the "1+1" screening method!
After he proved the case of n = (10a+3) (10b+11) in detail, he could prove the case of n = (10a+7) (10+9), n = (10a+7) (10+11), n = (10a+3) (10+9) in the same way. Should be detailed, he has been detailed, should be simple, he has been simple.The same is true for the other 12 types of n.
We should recommend Hou's proof to the Chinese Academy of Sciences. However, we are nearly 80 years old, and many diseases, every day to take several medicine, and the journey is long and bumpy, I am afraid that the heart is willing to help but the power is insufficient! The above words are left for the reference of future referees and readers. In order to show our contribution to science, for the revival of the Chinese nation's heart.
We have been studying "1+1" for decades, and Hou's research results are of the highest level to our knowledge. It has already established the basis for the research of expert meetings and deserves the support and help of the government.
Department of Mathematics, Sun Yat-sen University: Li Baitian, Ma Linjun
At CUHK on 15 November 2007.
Note: Professor Li Bai-tian has passed away; Professor Ma Lin-jun, 2023 Spring Festival, in good health, loud voice, clear mind.
Since 2015, Professor Ma Linjun has been repeatedly reviewing the proof of the second Goldbach conjecture, which is simply called proof Method 2. He affirmed that the proof was correct!
Proof Method 2, a few years ago, has been posted online, and no one has asked questions yet.
1.1.4. The Proof of Goldbach's Conjecture Cannot be Completed by Mathematical Induction
In the recent 12 years of research, Hou Shaosheng focused on the decomposition law of 2n=q+(2n-q). Because, when q and (2n-q) both take odd primes, 2n is decomposed into the sum of two odd primes, which is Goldbach's conjecture. So Goldbach's conjecture is only part of 2n=q+(2n-q). So we must find and prove the decomposition law of 2n=q+(2n-q). The decomposition law of 2n=q+(2n-q) is Hou Shaosheng's theorem.
On the other hand, Hou Shao-sheng always focused on: suppose that the conjecture is true when 2n, why is the conjecture also true when 2 (n+1)? If the conjecture is true for 2n, it can be proved that the conjecture is also true for 2 (n+1), which proves that Goldbach's conjecture must be true. This looks like mathematical induction, but it's a lot more complicated than mathematical induction.
Mathematical induction, we have induction 1 and induction 2. Mathematical induction has definite implementation steps. Since n in 2n is all the integers not less than 3, this presents an insurmountable difficulty in proving the conjecture by mathematical induction.
For example, if n is an odd prime, 2n=n+n, the conjecture is valid; To prove that k=n+1, the conjecture is also true, because 2(n+1)=n+2+n, but n+1 is not an odd prime, and we can't be sure that 2+n is an odd prime, so we can't continue to prove it.
Another example is that if 2n=pi+pj is true, and both pi and pj are odd prime numbers, To prove that the 2 (n+1) conjecture is also true , 2 (n+1) =2n+2=pi+2+pj, because there is no certainty that at least one of (pi+2) and (2+pj) is odd prime numbers, the proof cannot be completed.
The above practice tells us that it is impossible to prove Goldbach's conjecture by mathematical induction.
The previous checking calculation tells us that every even number which is no less than 6 and no more than 3×106 satisfies the sufficient and necessary condition theorem of conjectures.
Previous calculations tell us that Goldbach conjecture is probably correct. However, since there are an infinite number of even numbers that are not less than 6, it is impossible to prove the conjecture completely with concrete checking calculations.
To prove the conjecture, one must make a new discovery, and abstract the discovery into a new mathematical proposition, and then prove the new mathematical proposition into a new mathematical theorem.
The necessary and sufficient condition theorem of the conjecture is proved below. And prove that the sufficient and necessary condition theorem can not be violated.
Therefore, as a proof of Goldbach's conjecture, it should answer all the questions raised by the sufficient and necessary condition theorem, or prove that the proof of Goldbach's conjecture satisfies the sufficient and necessary condition theorem.
We know that the mathematical expression of Goldbach's conjecture is 2n=pi+pj, and we know that 2n=pi+pj is only a part of 2n=q+(2n-q), so we should study the relationship between 2n=pi+pj and 2n=q+(2n-q).
The research on the necessary and sufficient conditions for the establishment of 2n=pi+pj, the relationship between 2n=pi+pj and 2n=q+(2n-q), and the relationship between the establishment of the conjecture at 2n and the establishment of the conjecture at 2 (n+1) are the main line of thinking throughout the proof method 2.
It is this main line that establishes connections between sections and forms a logical system where each step is based on theorems or axioms and leads to the final goal.
This paper emphasizes that a proof satisfying the theorems of sufficient and necessary conditions is a correct proof, and a proof not satisfying the theorems of sufficient and necessary conditions must be wrong.This is the core idea of Proof Method 1.
A professor asked: the proof that does not satisfy the sufficient and necessary condition theorem must be wrong, why? Please look at the following: The sufficient and necessary condition theorem cannot be violated.
If the conjecture is true for 2n, why is it true for 2 (n+1)? For 2n and 2 (n+1), both conjectures are true, which is a necessary and sufficient condition for Goldbach's conjecture to be valid continuously.
In order to uncover the relationship between the 2n conjecture and the 2 (n+1) conjecture, after 45 years of repeated research, we write: The nature of the identity 2n=q +(2n-q), q≤(2n-q), determines that Goldbach's conjecture must hold.
The nature of the identity 2n=q+(2n-q), q ≤ (2n-q), determines that Goldbach's conjecture must hold. This is the core idea of proof method 2.
1.2. Meaning (Definition) of Mathematical Symbols in the Paper
In order to express our mathematical ideas briefly and accurately, we introduce the following mathematical symbols.
The meanings (definitions) of mathematical symbols in this paper are as follows:
N represents the set of natural numbers.
3≤n∈N indicates that n is a positive integer not less than 3.
2∣n, represents 2 divides n; or n is even.
2n, represents 2 cannot divide n; or n is not even, is odd.
(x) represents the number of prime numbers that do not exceed x (x is a positive integer).
num(2n=pi+pj), represents the number of 2n=pi+pj. p represents the odd prime number, and i, j, to distinguish different p. Also, under the convention, pi≤pj.
num(2n=pi+pj), read as: number of 2n=pi+pj . The same goes for the others.
"num" is an abbreviation for "number".
num(2n=pi+pj),It's a symbol that combines number with something. The thing after the number is 2n=pi+pj. It's like 3 eggs, 5 apples. 3, 5 is number, eggs, apples, things.
For example, when n=25,2n=2×25=3+47=7+43=13+37=19+31.
So, num(2×25=pi+pj)=4.
The thing after 4 is 2×25=pi+pj. So there are 4 different 2×25=pi+pj.
num(2n=ht+hu), represents the number of 2n=ht+hu, The thing after this number is 2n=ht+hu. h represents the odd composite number, t, u is to distinguish different h. It is also agreed that ht≤hu.
For example, when n=25,2n=2×25=ht+hu=15+35=25+25. Therefore, num(2×25=ht+hu) =2. There are 2 different 2×25=ht+hu.
num(2n=ps+hr), represents the number of 2n=ps+hr , The thing after this number is 2n=ps+hr. It is also agreed that ps<hr.
For example: the when n = 25, 2 n = 2×25 = ps+hr=5+45=11+39=17+33=23+27.
So,num(2×25=ps+hr)=4. There are 4 different 2×25=ps+hr.
num(2n=hk+pd),represents the number of 2n=hk+pd , The thing after this number is 2n=hk+pd. It is also agreed that hk<pd.
For example, when n=25,2n=2×25=hk+pd=9+41=21+29.
So, num(2×25=hk+pd)=2.there are 2 different 2×25=hk+pd.
num(3≤p≤n), represents the number of odd prime p in the interval [3,n]. The thing after this number is the odd prime p in the interval [3,n].
This definition states that num(3≤p≤n) is not only a number, and that this number also carries things: the odd prime p in the interval [3,n].
For example: when n=25,num(3≤p≤25)=8, so there are 8 odd prime numbers in the interval [3,25] : 3,5,7,11,13,17,19,23.
num(3≤h≤n), represents the number of odd composite h in the interval [3,n], the thing after this number is the odd composite number h in the interval [3,n].
This definition states that num(3≤h≤n) is not only a number, and that this number also carries things: the odd composite number h in the interval [3,n].
For example: when n=25,num(3≤h≤25)=4, so there are 4 odd composite in the interval [3,25] : 9,15,21,25 .
num(2n=q+(2n-q)), represents the number of 2n=q+(2n-q) , the thing after this number is 2n=q+(2n-q). q≤2n-q, 3≤q, q is odd.
For example: when n=25, num(50=q+(50-q))=12, so there are 12 of 2n=q+(2n-q) :2×25=q+(2n-q)= 3 + (50-3) = 5 + (50-5) = 7 + (50-7) = 9 + 11 + (50-9) = (50-11) = 13 + (50-13) = 15 + (50-15) = 17 + (50-17) = 19 + (50-19) = 21 + 23 + (50-21) = (50-23)
= 25 + (50 - 25).
In this paper, the concepts of number are as follows:
1、Positive integers: 1,2,3,4, ···, 10,11,12,···, called positive integers.
2、Natural numbers: Positive integers and 0 are collectively called natural numbers. Non-negative integers are natural numbers.
3、 Negative integers: -1,-2,-3,-4, ···, -10,-11,-12, ···, called negative integers.
4、Integers: Positive integers, negative integers, and 0 are collectively called integers.
5、Prime: Integers greater than 1 and divisible only by 1 and itself, such as 2, 3, 5,7,11.Every prime number has and only has 2 divisors: 1 and itself. 1 is not prime.
composite number: A (non-zero) natural number can be expressed as the product of two (or more) natural numbers that do not include itself and 1. For example , 4,6,8,9,10,12,14,15, are composite number. xxxxx
7、Even number: An integer that is divisible by 2, and 0 is also an even number. For example, 2,4,6,8.
8、Odd number: Integers that are not divisible by 2, such as 1,3,5,7,9.
9、Odd primes: Prime numbers other than 2. For example, 3,5,7,11,13.
10、Odd composite number: A composite number that is not divisible by 2. For example, 9,15,21,25,27.
11、 Even composite number: An even number other than 0,2. For example, 4,6,8,10.
12、Prime after odd composite: Let h be an odd composite, If h+2 is an odd prime, the odd prime is called Prime after odd composite, or a prime after composite number. For example, 11,17,23,29, are a prime after composite number.
13、Sister primes: Let p be an odd prime. If p+2 is an odd prime, p and (p+2) are called sister primes. For example, 3,5,7, are the 3 sister prime ; 11,13; 17,19; They're both twin prime numbers.
14、Odd prime after odd prime: Let p be an odd prime, if p+2 is an odd prime, this odd prime is called odd prime after odd prime, or is called prime after prime. For example, 5,7,13,31, are odd prime numbers after odd prime numbers.
The odd prime number after the odd prime number is the larger of the sister primes.
15、Independent odd primes: Let p be an odd prime. If p-2,p+2, are both odd composite numbers, this odd prime p is called an independent odd prime.
For example, 23,37,47, are independent odd prime numbers. Independent odd prime numbers are odd prime numbers after composite numbers.
This concludes the introduction of mathematical notation.
Our subject is the Goldbach conjecture 2n=pi+pj,What are the necessary and sufficient conditions of the conjecture?
1.3. Sufficient and Necessary Condition Theorems of Conjectures, New Mathematical Ideas to Prove Conjectures
In 1977, In an unpublished manuscript, Hou proved the necessary and sufficient conditions of the Goldbach's conjecture. And so he was determined to prove Goldbach's conjecture.
1.3.1. The Necessary and Sufficient Condition Theorem of the Conjecture
Theorem 1 (necessary and sufficient conditions of the conjecture):
2n=p1+p2, (3≤n∈N, p1,p2, all are odd primes), the sufficient and necessary condition is that there is a non-negative integer △, make that n- △, n + △, are all odd primes.
Proof Goldbach's conjecture is mathematically expressed as 2n=p1+p2,
(3≤n∈N, p1,p2, are all odd prime).
1:When n is an odd prime,△=0, 2n=(n- 0)+(n+0)=n+n.n+n , is the sum of two odd prime numbers, so the theorem holds.
2:When n is not an odd prime, the proof is as follows:
First prove sufficiency, as follows:
Because: n-△,n+△,are all prime numbers,
So, we could say n-△=p1, n+△=p2.
And because n-△ + n+△=2n, is the identity, order n-△=p1, n+△=p2, substitute in The identity,to get:
p1+p2=2n.
Sufficiency has been verified.
n-△, n+△, with respect to n symmetry,as shown below.

Let's prove the necessity.
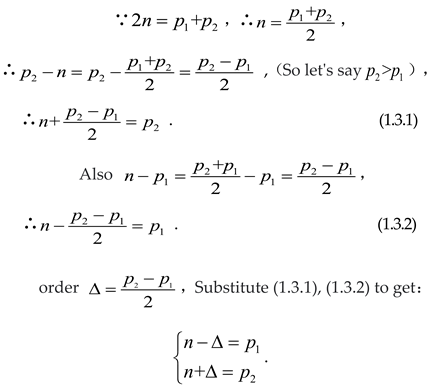
Necessity proved.
Theorem 1 has been proved.
Note that sufficient and necessary conditions are one and the same: both are n-△,n+△, are all prime numbers. It should also be noted that, n-△,n+△,It's two odd prime numbers that are symmetric about n, that is, p1 and p2 are two odd prime numbers that are symmetric about n.
The above theorem was written by Hou Shaosheng in 1977. According to this theorem, if n is an odd prime, the conjecture needs no proof. So, the key to proving the conjecture is to prove an infinite number of composite numbers, n.
In 2n=pi+pj, pi and pj are symmetric about n, it's not obvious. After proving the sufficient and necessary condition theorem for 2n=pi+pj, it turns out that pi and pj are symmetric about n.
The relationship between 2n=pi+pj and 2n=(n-△)+(n+△) is very simple, pi=(n-△), pj=(n+△).
Some readers may ask: What are the conditions for n-△,n+△ to be both odd prime numbers?
The answer is as follows: Here is only to introduce the content of sufficient and necessary condition theorems and theorems. The theorem itself does not require an answer as to what condition △ satisfies.
What is the condition that△satisfies ? It's a very deep, very complex mathematical problem. This problem is discussed in detail in the proof of Goldbach's conjecture (Proof method 1). For readers who are interested in this problem, please review the two parts of Proof (Proof method 1) : "Decomposition formula of Odd composite numbers, Determination of prime Numbers, distribution of prime Numbers and a New screening method" and "Relationship between prime numbers and composite numbers, the condition that positive integers are prime Numbers". The length of each of these two parts is relatively large.
In Proof Method 1, what is the condition that △ is satisfied without answering? Goldbach's conjecture cannot be proved.
The present proof is the proof of Goldbach's conjecture (proof method 2).
In proof method 2, the Goldbach conjecture can be proved without
answering what the conditions satisfy by △.
Method 1 and Method 2 of proof are two different theoretical systems, mathematical idea and methods, which differ greatly.
Proof 2 is not only much shorter than Proof 1, but it answers questions that proof 1 can't answer.
For example, what is the close relationship between 2n being true and 2 (n+1) being true? That is, if 2n is good, why 2(n+1)is good? Proof 1 doesn't answer that question. However, proof 2 answers the question completely.
It works for 2n, why does it work for 2 (n+1)? This is the core question that must be answered in this paper, which is the mathematics idea throughout this paper.
However, there are other problems in which Proof 1 has shown remarkable ability. For example, the reader asked, What are the conditions for satisfaction? In Proof method 1, mathematical formulas are used to make accurate solutions.
For example, how to determine a positive integer is an odd prime number, how to determine a positive integer is an odd composite number? This is a question that no theory in the world can answer. However, in Proof Method 1, the answer is given thoroughly in theory.
For another example, how to improve the world famous sieve of Eratosthenes , in proof method 1, not only in theory, but also in practical examples, give a thorough answer. There are more problems, which will not be addressed here. In short, different theoretical systems can solve different problems. Each has its advantages and disadvantages.
A professor asked: Why can't the sufficient and necessary condition theorem of conjecture be violated? See our answers.
1.3.2. The sufficient and Necessary Condition Theorem of the Conjecture Cannot be Violated
The sufficient and necessary condition theorem of the conjecture can not be violated. The proof is as follows:
Because
2n=(n-△)+(n+△) (1.3.3)
is an identity.
In the (n-△)、(n+△), as long as there is a not odd prime, then (n-△)+(n+△), is not the sum of two odd prime Numbers. Since 2n=(n-△)+(n+△), 2n is no longer the sum of two odd prime numbers, so Goldbach's conjecture cannot be established at this time.
This proves that, (n-△), (n+△), If I have a number that's not an odd prime, or does not satisfy the sufficient and necessary conditions, Goldbach conjecture cannot be established. That is, the necessary conditions cannot be violated.
If (n-△) and (n+△) are both odd prime numbers, then (n-△)+(n+△) is already the sum of two odd prime numbers. Since 2n=(n-△)+(n+△), 2n is already the sum of two odd prime numbers, so Goldbach's conjecture holds.
This proves that when (n-△) and (n+△) are both odd prime numbers, the conjecture must be true if sufficient and necessary conditions are satisfied.
It has been proved above that a proof satisfying the sufficient and necessary condition theorem is a correct proof; A proof that does not satisfy the sufficient and necessary theorems must be a false proof!
The core mathematical idea of the proof of Goldbach's conjecture (Method 1) is to study around sufficient and necessary conditions and answer all the questions raised by sufficient and necessary conditions.
Try to prove that num(2n=pi+pj)≥1, which is the core mathematical idea of proving method 2.
1.3.3. Num(2n=pi+pj) ≥1, Satisfies the Sufficient and Necessary Condition Theorem of the Conjecture
To prove method 2, as long as num(2n=pi+pj)≥1 is proved, it is proved that there is at least one 2n=pi+pj, because 2n is already the sum of two odd prime pi, pj, so Goldbach's conjecture has been proved.
In proof method 1, the sufficient and necessary condition theorem of 2n=pi+pj is obtained from 2n=pi+pj. Now, in proof method 2, as long as 2n=pi+pj exists is proved, the sufficient and necessary condition theorem of 2n=pi+pj can be obtained from 2n=pi+pj, which indicates that proof method 2 satisfies the sufficient and necessary condition theorem of 2n=pi+pj.
1.3.4. New ideas 1 in Mathematics and New ideas 2 in Mathematics are two different theoretical systems
This paper presents two new mathematical ideas for proving conjectures.
The mathematical ideas of proving method 1 is called new mathematical ideas 1 of proving conjecture , simply called new mathematical ideas 1.
The mathematicalideas of proving method2 is called new mathematicalideas 2 of proving conjecture , simply called new mathematicalideas2.
New mathematical ideas1,the details are as follows.
The new mathematical idea 1, based on the necessary and sufficient condition theorem of Goldbach's conjecture, is to answer all the questions raised by the necessary and sufficient condition theorem.
Undoubtedly, a proof satisfying the necessary and sufficient condition theorem is a correct proof, because it satisfies the sufficient condition for the conjecture to hold. So, if we follow the sufficient and necessary theorem, we can prove 1+1.
There is no doubt that a proof that does not satisfy the necessary and sufficient condition theorem is a false proof, because it does not satisfy the necessary conditions for the conjecture to hold up.
Because a sufficient condition and a necessary condition are the same condition, so not satisfying the necessary condition is not satisfying the sufficient condition. It certainly shouldn't be true if it doesn't satisfy sufficient conditions.
The above are the core mathematical ideas of New Ideas 1 in Mathematics .
The sufficient and necessary condition theorem for the conjecture to be valid points out that: 2n=p1+p2, (3≤n∈N, p1,p2, all are odd primes), the sufficient and necessary condition is that there is a non-negative integer △, make that n- △, n + △, are all odd primes.
Since there are infinite number n , it is impossible to find a △ for each n and make n-△ and n+△ all prime without new discoveries. It was this question that surprised Hou Shao-sheng out of a cold sweat!
The key question is whether we can divide an infinite number of n into finite types, that is, to divide the infinite Goldbach conjecture into smaller conjectures, and then prove it one by one.
Around this problem, Hou Shaosheng analysis and research problems, the whole 23 years! In March 2000, the thinking suddenly enlightened, wisdom suddenly burst out! I finally found a way to divide an infinite number of n into a finite number of types.
After the paper, Reference 8, Hou Shaosheng1, Wang Shunqing2, published in March 2002: the factorization formula of odd composite numbers, prime distribution and screening method.
A, this paper proves that the units digit is an odd composite of 1,3,7,9, and there are only 10 factorization formulas.
The 10 formulas are as follows.
f1=f1(x,y)=(10x+3)(10y+7), f2=f2(x,y)=(10x+9)(10y+9), f3=f3(x,y)=(10x+11)(10y+11);
f4=f4(x,y)=(10x+3)(10y+11),f5=f5(x,y)=(10x+7)(10y+9);
f6=f6(x,y)=(10x+3)(10y+9), f7=f7(x,y)=(10x+7)(10y+11);
f8=f8(x,y)=(10x+3)(10y+3), f9=f9(x,y)=(10x+7)(10y+7), f10=f10(x,y)=(10x+9)(10y+11).
Here x,y∈N.
This paper proves that: these 10 odd composite number factorization formula, one more will not do, not one less! Their domain are uniform, and they're all natural numbers. The structure of every formula is the product of two factors.
The single digit is odd composite number of 1, and there are 3 decomposition formulas.
The single digit is odd composite number of 3, and there are 2 decomposition formulas.
The single digit is odd composite number of 7, and there are 2 decomposition formulas.
The single digit is odd composite number of 9, and there are 3 decomposition formulas.
These 10 formulas lay the theoretical foundation for determining whether an integer is prime or not! Before that, there was probably no theory in the world that could solve this problem! How to determine whether an integer is prime is one of the most basic and important questions that number theory must answer.
More than 1, single digits are 1,3,7,9 integer, If it is not the functional value of the 10 formulas, is the prime number!
An integer m greater than 1, with units digits of 1, is prime if it is not a function of f1, f2, f3! If m is a function of f1, or f2, or f3 , m is composite number!
The same goes for the others.
In this paper, we give a method to determine whether a positive integer m is prime by using equations.
On this basis, a simple and feasible new screening method is proposed to find the distribution of prime numbers. As an example, all prime numbers with 1 as single digit in the interval [1000, 2000] are selected.
Reference 13 states that, Hou Shaosheng1, Ma Linjun2, Li Baitian3 , Qin Jianmin4, Zhang Kaida5, 2013, Published: Eratosthenes sieve method defects and Ideal Final sieve method.
In this paper, it not only points out the shortcomings of Eratosthenes screening method, but also develops Eratosthenes screening method to the ideal final screening method by using 10 formulas. The ideal final screening method is used to determine whether a positive integer m is prime.
We know that the units digit is a positive integer of 0,2,4,5,6,8, all of which are composite numbers , except 2,5, which is prime.
Greater than 1, the units digit is a positive integer of 1,3,7,9, either composite or odd prime. The ones digit is the composite number of 1,3,7, and 9, and they're all functions value of 10 formulas! As long as we know the law of composite numbers, we indirectly know the law of odd prime numbers.
B, integers can be divided into 17 classes
The 10 odd composite number formulas let us know: all odd composite number whose single digits are 1,3,7,9 can be divided into 10 types, which are the function values of the 10 formulas respectively. Plus odd composite numbers with 5 as units digits, plus 5 classes of even numbers with 0,2,4,6,8 as units digits, all composite numbers, can be divided into 16 classes!
Primes as 1 class of integers, plus 16 classes of composite numbers, all integers greater than 1, can be divided into 17 classes!
C, Goldbach's conjecture can be divided into 17 small conjecture
The division of 17 classes of integers is the theoretical basis for dividing Goldbach conjecture into 17 small conjecture and proving them separately. The mathematical idea of dividing Goldbach's conjecture into 17 small conjectures and proving them separately is very important! Goldbach conjecture is an infinite conjecture, like a huge cake, impossible to eat in one bite! We cut it into 17 pieces and eat it piece by piece.
When n is an odd prime, 2n=n+n. Since n+n is already the sum of two odd prime numbers, Goldbach's conjecture naturally holds. So when n is an odd prime, Goldbach's conjecture doesn't need to be proved! So, to prove the conjecture, all you have to do is prove each of the 16 composite numbers, n.
When n=f1(a,b)=(10a+3)(10b+7),to prove that 2n=2f1(a,b)=2(10a+3)(10b+7) can be expressed as the sum of two odd prime numbers, we sieve out all composite numbers except f1(a1,b1), f2(a2,b2), f3(a3,b3), and eliminate all kinds of interference. Make the problem relatively simple; Then it is proved by simultaneous equations that 2f1(a,b) can be expressed as the sum of two odd prime numbers.
The same goes for the others.
That's the basic content of New Ideas 1 in Mathematics .
Having a new idea 1 in mathematics is not the same as having completed the proof of Goldbach's conjecture. To complete the proof of the conjecture, a great deal of in-depth and detailed work must be carried out. Since we are only introducing new mathematical ideas 1, and not completing the proof of the conjecture, we will stop there. If you want to study how to prove Goldbach Conjecture, please refer to Hou Shaosheng's book "Proof of Goldbach Conjecture " in reference 10.
1.3.4.2. New Ideas 2 in Mathematics , briefly described below.
2n=q+(2n-q), called Hou Shaosheng's identity. The nature of the identity determines that Goldbach's conjecture holds true!
2n=q+(2n-q), When q and (2n-q) both take odd primes, 2n is decomposed into the sum of two odd primes, which is Goldbach's conjecture.
2n=q+(2n-q), when q is even, (2n-q) is also even, and 2n is decomposed into the sum of 2 even numbers, which has nothing to do with Goldbach's conjecture, so q cannot be even.
2n=q+(2n-q), when q=1, (2n-1) is odd, 2n=1+(2n- 1), which has nothing to do with Goldbach's conjecture, so q cannot be 1.
2n=q+(2n-q), 3≤q must be set, and q is odd. In order to avoid the duplication between q and (2n-q), then set q≤(2n-q), which is q≤n. So 3≤q≤n, q is odd.
If 3≤q≤n and q is odd, q can only take odd prime numbers or odd composite numbers in the interval [3, n]. (2n-q) can only take odd prime numbers or odd composite numbers in the interval [n, 2n-3].
The following conventions: 3≤q≤n, q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And:pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
2n=q+(2n-q), there are only4 decomposition forms as follows:
First: 2n=odd primes in the [3,n] + odd primes in the [n,2n-3]. This is the conjecture.
The mathematical formula is as follows: 2n=pi+pj.
The number of 2n=pi+pj is expressed as num(2n=pi+pj).
Second: 2n=odd prime numbers in the [3,n] + odd composite numbers in the [n,2n-3].
The mathematical formula is as follows: 2n=ps+hr.
The number of 2n=ps+hr is expressed as num(2n=ps+hr).
Third: 2n=odd composite numbers in the [3,n] + odd composite numbers in the [n,2n-3].
The mathematical formula is as follows: 2n=ht+hu.
The number of 2n=ht+hu is expressed as num(2n=ht+hu).
Fourth: 2n=odd composite numbers in the [3, n] + odd prime numbers in the [n, 2n-3].
The mathematical formula is as follows:2n=hk+pd.
The number of 2n=hk+pd is expressed as num(2n=hk+pd).
So, the number of (2n=q+(2n-q))=
the number of the first+ the number of the second
+ the number of the third+ the number of the fourth.
The above equation is referred to as Hou Shaosheng's theorem . The mathematical formula for this theorem includes all the possibilities for 2n to be decomposed into the sum of two odd numbers. 2n=p1+p2 is only a part of Hou Shaosheng's theorem. Hou Shaosheng's theorem is the theoretical basis of proving our conjecture.
Hou Shaosheng's theorem is expressed as follows:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)(3.3.0) is the number of the relevant section when the formula is proved.
The 4 corollary formulas of Hou Shaosheng's theorem are as follows:
num(3≤p≤n)=num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Wherein, it is agreed that: 3≤q≤n, q is odd.
pi, pj, ps, pd , are odd prime; ht, hu, hr, hk, are odd composite number.
And:pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3, n]; pj, hu, hr, pd∈[n, 2n-3].
The 4 formulas in the corollary of Hou Shaosheng's theorem are the main theory for us to prove the conjecture.
The 4 formulas uncover num(3≤p≤n), num(3≤h≤n), num(n≤p≤2n-3),
num(n≤h≤2n-3), quantitative relationship between num(2n=pi+pj), num(2n=ps+hr), num(2n=ht+hu), num(2n=hk+pd). It lays a theoretical foundation for further research.
In "The Meaning (definition) of mathematical symbols in Papers is as follows:", when n=25, we give:
num(2×25=pi+pj)=4. num(2×25=ht+hu)=2. num(2×25=ps+hr)=4.
num(2×25=hk+pd)=2.
num(3≤p≤25)=8. num(3≤h≤25)=4. num(50=q+(50-q))= 12 .
Please verify the mathematical formula of Hou Shaosheng's theorem with the above data:
num(2n=q+(2n-q))= num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
Please verify the 4 corollary formulas of Hou Shaosheng's theorem.
The reader can randomly give n=a,(3≤a∈N), and find the above data from the actual situation. Then verify the mathematical formula of Hou Shaosheng's theorem, verify the 4 corollary formulas.
We carefully studied the 4 mathematical formulas and found that: if the conjecture is valid for 2n, there are only 4 kinds of genetic code among the 4 mathematical formulas. As long as there is 1 kind of genetic code, the conjecture is guaranteed to be valid for 2 (n+1). The existence of genetic code is a decisive factor in the persistence of Goldbach's conjecture.
Goldbach conjecture at 2n, we use 2n=pi+pj; let k=n+1, Goldbach conjecture at 2k, we use 2k=pi++pj+, pi+, pj+, are all odd primes.
Definition: let qi and qj are odd primes, and 2k=qi+qj. At this point, we call (define) 2k=qi+qj, which is the source of 2k=pi++pj+.
2k=pi++pj+, as long as there is a source, the Goldbach conjecture at 2k is true.
Regarding the source of 2k=pi++pj+, the subsequent paper has theorem 11 answers as follows:
Theorem 11: If the conjecture at 2n is true, then 2k=pi++pj+ has the following4 sources. As long as one source exists, the conjecture at 2k is guaranteed to remain true.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+;
Each of these 4 sources is a theorem. Because they have the same form, they're put together as a theorem.
The statement of 4 sources, from the conditions of the theorem to the conclusions of the theorem, is very direct, so no longer proved.
Now, let's test theorem 11 with a mathematical example.
Theorem 11 theoretically proves that 2k=2(n+1)=pi++pj+ has 4 sources. Now, let's test theorem 11 with a mathematical example.
Example A. 2n=2×25=50
=3+47=5+45=7+43=9+41=11+39=13+37=15+35=17+33=19+31=21+29=23+27=25+25.
Theorem 11 proves that 2k=2(n+1)=pi++pj+ has4 sources. Of these4 sources, in ExampleA, three have been fully verified. See below for specific verification.
Verify the first source of 2k=2(n+1)=pi++pj+ as follows:
num(2×25=pi+pj)=4.
The four 2×25=pi+pj are as follows:
2×25=pi+pj=3+47=7+43=13+37=19+31.
2n=pi+pj, (pi+2) are odd prime numbers: (3+2).
2k=2(n+1)=(pi+2)+pj .2k is the sum of two odd prime numbers: 2k=(3+2)+47;
Verify the second source of 2k=2(n+1)=pi++pj+, as follows:
2n=pi+pj,(pj+2)is an odd prime number, no. No(pj+2)is an odd prime because n=25, which is relatively small. Other examples show that (pj+2) is an odd prime number. So that doesn't negate theorem 11.
Verify the third source of 2k=2(n+1)=pi++pj+, as follows:
num(2n=ps+hr)=4.
The four 2×25=ps+hr are: 2×25= 5+45=11+39=17+33=23+27.
2n=ps+hr,(hr+2)are odd prime numbers: (45+2); (39+2); (27+2).
2k=2(n+1)=ps+(hr+2). 2k is the sum of two odd prime numbers:
2k=5+(45+2); 2k=11+(39+2); 2k=23+(27+2).
Verify the fourth source of 2k=2(n+1)=pi++pj+ , as follows:
num(2n=hk+pd)=2.
These 2 number of 2n=hk+pd are as follows: 2n=9+41; 2n=21+29;
2n=hk+pd ,(hk+2)are odd prime numbers: (9+2); (21+2).
2k=2(n+1)=(hk+2)+pd . 2k is the sum of 2 odd prime numbers:
2k=(9+2)+41. 2k=(21+2)+29.
The above facts show that 3 of the 4 sources of 2k=2(n+1)=pi++pj+ appear in this example. Above we get 6 2k=2(n+1)=pi++pj+ . These six are as follows:
2k=(3+2)+47 ;2k=5+(45+2); 2k=11+(39+2); 2k=23+(27+2);
2k=(9+2)+41; 2k=(21+2)+29.
Where: 2k= (3+2) +47, with 2k=5+ (45+2), repeat.2k=11+ (39+2), repeat with 2k= (9+2) +41.2k=23+ (27+2), repeat with 2k= (21+2) +29.Only 2k=5+47=11+41=23+29 is not repeated.
Example B: 2k=2×26=pi++pj+=5+47=11+41=23+29.
After comparison, the 3 non-repeated 2k is exactly the same as the actual situation of 2k=2×26=pi++pj+ in instance B.
The reader should recognize that theorem 11 proves that 2k=2(n+1)=pi++pj+ has 4 sources. These 4 sources have repeated situations in the examples. Would rather repeat, never appear missing! This happens to be an expression of absolute rigor in theory.
At this point, the only questions readers should ask are:
Is it possible that none of the 4 if conditions in theorem 11 exist? This is the most central issue, and this is the issue that we are most concerned about. As a proof of the conjecture, this question must be answered.
As an answer to the core question, there are theorems 17, 18, and 19, as follows:
Theorem 17: If the conjecture is true at 2n and k (=n+1) is composite, then at least one of (pi+2), (hr+2) is an odd prime p,ensuring that the conjecture is true at 2k.
Theorem 18: If the conjecture is true at 2n and k (=n+1) is composite, then at least oneof (hr+2) is an odd prime p , ensuring that the conjecture is true at 2k.
Theorem 19: If the conjecture is true at 2n and k (=n+1) is composite, then at least one of (hk+2) is an odd prime p, ensuring that the conjecture is true at 2k.
Theorems 17, 18, 19 prove that 3 of the 4 genetic codes must exist without interruption. This ensures that Goldbach's conjecture continues to hold true.
The proof of theorems 17, 18, 19, which is very complicated and very long, will not be covered here. After publishing them, expect experts to review them carefully.
Theorem 11, in theory, gives 4 sources of 2k=pi++pj+.
The 4 sources of 2k=pi++pj+ essentially answer the close relationship between the 2n conjecture and the 2k conjecture.
These 4 sources,as long as one exists,that makes sure 2k time is true!This is the decisive factor and fundamental guarantee of the continuity of the conjecture. So the4 sources are essentially the4 genetic codes of Goldbach's conjecture.
Theorems 17,18, and 19, as long as one of them is correct, the proof of the conjecture is complete. We, as authors, believe that each of these 3 theorems is true!
In the subsequent paper, eight random examples of 2n=q+(2n-q) are given to test all our above theories with these 8 examples, and the examples are completely consistent with the theory.
So that's New Ideas 2 in Mathematics.
Having the new mathematical idea 2 does not equal to complete the proof of Goldbach's conjecture. To complete the proof of Goldbach's conjecture, a great deal of painstaking and meticulous work must be done. Every step of this work is in the innovation of mathematical theory and mathematical method!
Summary of this paper: The title of this section is: New mathematical idea to prove Goldbach's conjecture. Therefore, after proving the sufficient and necessary condition theorems of conjectures, the paper emphasizes that the proof satisfying the sufficient and necessary condition theorems is the correct proof, and the proof not satisfying the sufficient and necessary condition theorems must be wrong. Emphasize that necessary conditions cannot be violated.This is the core content of the new Mathematical Ideas 1.
It is also pointed out that as long as num(2n=pi+pj)≥1 is proved, Goldbach's conjecture is proved, and the sufficient and necessary condition theorem of the conjecture is satisfied.This is the core of New Ideas 2 in mathematics.
Finally, the paper also points out that New Ideas 1 in Mathematics and New Ideas 2 in Mathematics, are two different theoretical systems.
To be sure, it is impossible to deny the above new mathematical idea of proving the conjecture. I hope that real mathematicians recommend the publication of this paper and let mathematicians around the world comment on it, please make a mathematician's contribution.
For references, see the end.
Section 2, Insufficient approximation formula of the number of primes in the [n, 2n]
Hou Shao-Sheng1, Ma Lin-Jun2
(1 Anyang Mathematical Society, Henan Province, China; 2 Department of Mathematics, Sun Yat-sen University, Guangzhou City, Guangdong Province, China)
Hou Shao-Sheng Email: ayhss@163.com.
Abstract: There are prime numbers in the interval [n,2n], (3≤n∈N), which is a necessary condition for the establishment of Goldbach conjecture. In this section, we give the insufficient approximation formula of the number of primes in the interval [n,2n]. And prove that there must be prime numbers in the interval [n,2n], 3≤n.
Key words [n, 2n] interval; the number of prime;formula ;
Insufficient approximation value.
[Document ID] A [document number]
2.1.1. Definition of the Insufficient Approximation Value
Definition: If the approximation is less than the true value, we say the approximation is an insufficient approximation.
If the approximation is greater than the true value, we say the approximation is an excess approximation.
There are prime numbers in the interval [n,2n], (3≤n∈N), which is a necessary condition for Goldbach's conjecture to be established. In this section, we first derive the formula for the number of primes in the interval [n,2n], and then prove that there must be primes in the interval [n, 2n], 3≤n.
The mathematical dictionary introduces the theorem of prime numbers. The prime number theorem is as follows: If (x) represents the number of primes not exceeding x ( x is a positive integer), then there is =
This theorem says that when x is very large, is very close to . When x is large, ≈; It shows that the distribution of prime numbers has a certain law.
On the other hand, we see: the average density of the distribution of prime numbers should be close to ÷x=. That means that as x gets bigger , smaller and smaller , the average density of the distribution of prime numbers gets smaller and smaller.
Also because n!+2, n!+3, n!+4,... ,n!+n, are composite numbers, which gives the illusion that the number of prime numbers in the interval [n,2n] is likely to decrease as n increases! However, this is not consistent with the facts as people know them.
When n≤5000, you can find through the experiment that in the interval [n,2n], with the increase of n, the number of prime numbers is increasing! So, in the interval [n, 2n], as n increases, does the number of prime numbers increase? Or fewer and fewer? Still no pattern?
We have proved that the necessary and sufficient conditions for Goldbach's conjecture are that for every integer n of no less than 3, there is at least a non-negative integer △, so that n-△ and n+△ are all odd prime! It is known that n-△, n+△, is two odd primes about n symmetry. And the n+△ is just within the [n, 2n] interval.
If the number of prime numbers in the interval [n,2n] increases with the increase of n, then the probability that the conjecture is true increases. On the contrary, the probability of the conjecture being true is decreasing!
For the reasons mentioned above, it is necessary to answer the regularity of the number of primes in the interval [n,2n].
2.1.2. Theorem 2. Let num[n≤p≤2n] Represent the Number of Prime p in the Interval [n, 2n]. Then
num[n≤p≤2n]≈- =- .
(Note: Theorem 1 is the necessary and sufficient condition theorem of Goldbach's conjecture.)
(Note: num is short for number.)
Proof: The prime number theorem states that if (x) represents the number of primes that does not exceed x (x is a positive integer), then there is =
The prime number theorem shows that when x is large, with being very close to. Can be regarded as:≈, ≈.
We use num[n≤p≤2n] to represent the number of prime p in the interval of [n, 2n], so has:
num[n≤p≤2n]=(2n)-(n-1)
≈- (2.1.0)
num[n≤p≤2n] ≈- (2.1.1)
=2(-). (2.1.2)
Let x≥10 be a real number and the function(-), when x is an integer not less than 10, its function value is (-) . Now, by studying the derivative of (-) , we will study the tendency of (-) as n increases.
Let x≥10 be a real number because:
(-)′= ()′-()′
=-=-
=--+
=-+-+
=-+(+)(-)+
=(-)[1-(+)]+
>(-)[1-(+)]
>0. (2.1.3)
Note: Because when x≥10, ->0; And 1-(+)>0.
We know from (2.1.3) that 2(-) is an increasing function of n, so num[n≤p≤2n] is, on the whole, an increasing function of n.
Here it is not written as: so num[n≤p≤2n] is an increasing function of n because: ≈, Instead of: .

(2.1.1), (2.1.4) is the calculation formula of num[n≤p≤2n].
2.2. Example 1. Calculate num[50 000≤p≤2×50 000] and Compare with the Exact Value.
Solution: num[50 000≤p≤2×50 000]

=8686-4621
=4065.
Introduction to Number Theory by Hua Luogeng p87:
π (2×50000)=9592, π (50000)=5133.
num[50 000≤p≤2×50 000]
= 9592- 5133
=4459.
4065÷4459=91.16%.
This example shows that the value calculated by the approximate formula is less than the true value! Accounts for 91.16% of the true value.
This example illustrates that the approximation degree when n is not less than 50000 already exceeds 91%.
2.3. Example 2. Calculated num[10 000 000≤p≤2×10 000 000] and Compared With the True Value
Solution:num[10 000 000≤p≤2×10 000 000]

=1 189 700-620 429
=569 271.
Introduction to Number Theory by Hua Luogeng p87:
π (2×10000000)=1 270 607, π (10000000)=10000000.
num[10 000 000≤p≤2×10 000 000]
= 1 270 607- 664 579
=606 028.
569 271÷606 028=93.93%.
This example illustrates that the values calculated with the approximate formula are less than the true value. Accounting for 93.93% of the true value.
This example illustrates that when the n is no less than 10 000 000, the approximation has exceeds 93%.
The above two examples illustrate that the accuracy increases as n increases with the approximate formula. This is a valuable property. This is consistent with the prime number theorem.
The above two examples show that the value calculated by the approximate formula, less than the true value, is an insufficient approximation value. It's better to know the insufficient approximation of the number of primes in [n,2n] than to know the excess approximation of the number of primes in [n,2n]. Knowing the less than approximate value of the number of primes in the interval [n,2n], is to know the minimum of the number of primes in the [n, 2n] interval.
If you only know the excess approximation of the number of primes in the interval [n,2n],which is almost meaningless. For example, we tell you that the maximum number of the number of primes in the interval [n,2n] is no more than 0.5n, which makes almost no sense.
In the interval [n,2n], the number of primes increases with the increase of n, which provides a good condition for the establishment of the conjecture.
2.4. A conclusion: let num[n≤p≤2n] represent the number of prime p in the [n, 2n] interval. If 10≤n , then num[n≤p≤2n]≥ 3.
Proof if 10≤n≤5000, it is not difficult to verify one by one.
If 5000≤n, it can be computationally verified like the two examples in 2.2 and 2.3.
If 5000≤n, or n→∞, we give the following proof.
π(x) represents the number of primes not exceeding x (x is a positive integer), π(2x) represents the number of primes not exceeding 2x, and π[n, 2n] represents the number of primes in the [n, 2n] interval, then there:

that is, let π [x,2x]=c, and c is a constant and cannot hold, In particular, c=3, which cannot hold.
The proof is over
Summary: The title of this section is the Insufficient approximation formula for the number of primes in the [n, 2n]. We use the prime number theorem to give a formula for the number of primes in the [n, 2n] interval. And with 2 practical examples to test the calculation formula. The inspection shows that as n increases, the results of the approximate formula get closer and closer to the true value.
Finally, we prove a conclusion: let num[n≤p≤2n] represents the number of prime p in the [n, 2n] interval, if 10≤n, then num[n≤p≤2n]≥3. It is shown that there must be prime number in the [n, 2n-3] interval, 10≤n.
Also because
num[3≤p≤6]=2, num[4≤p≤8]=2, num[5≤p≤10]=2,
num[6≤p≤12]=2, num[7≤p≤14]=3, num[8≤p≤16]=2,
num[9≤p≤18]=3, num[n≤p≤2n]≥3, 10 ≤n.
So it can be concluded that there are at least 2 primes, in the
[n, 2n] interval,3≤n.
The approximation formula is much more important than the conclusion that there are at least 2 prime numbers in the interval [n, 2n] and 3≤n. Since the formula can calculate the number of primes in any [n, 2n] interval, the conclusion is the result of the calculation.
There are at least two prime numbers in the interval [n,2n], 3≤n. It's been a conjecture for centuries, and today it's been proved once and for all.
In reviewing the above results, Professor Ren Wei consulted a large amount of data. In particular, he found that Hardy, a famous mathematician, had proved that there were prime numbers in the interval [n,2n]. This is certainly an important result. However, Hardy merely pointed out that there are prime numbers in the interval [n,2n].
Simply knowing that there are primes in the interval [n,2n] is different from knowing the formula for calculating the insufficient approximation of the number of primes in the interval [n,2n]. Because of the calculation formula, we can calculate the less than approximate value of the number of primes in any [n,2n] interval.There are primes in the interval [n,2n],this conclusion does not have this ability.
It should be said that we have taken Hardy's results to a whole new historical stage, and pushed them to a point where they are approaching their limits.
However, the accuracy of the calculation formula of the approximate value of the number of prime numbers in the interval [n,2n] has not reached the ideal degree. Especially when n is relatively small, the accuracy of the calculation needs to be improved.
Take measures to make 100≤n, the accuracy of the calculation is more than 95%, is a worthy direction! Hou Shaosheng wants other mathematicians to finish the job!
There must be prime numbers in the interval [n,2n], which satisfies a necessary condition for the conjecture to be true.
It is far from enough to prove Goldbach's conjecture just to know the necessary and sufficient theorems of the conjecture, and to know that there must be prime numbers in the interval [n,2n].
Goldbach's conjecture 2n=pi+pj is only a part of Hou Shaosheng's identity 2n=q+(2n-q). In order to fully grasp the law of 2n=pi+pj, we must study the basic law of 2n=q+(2n-q). Therefore, the next section will study the basic law of Hou Shaosheng's identity.
For references, see the end.
Section 3, Goldbach's conjecture 2n=pi+pj,The basic properties of identity 2n=q+(2n-q) and Hou Shao-sheng's theorem
Hou Shao-Sheng1, Ma Lin-Jun2
(1 Anyang Mathematical Society, Henan Province, China; 2 Department of Mathematics, Sun Yat-sen University, Guangzhou City, Guangdong Province, China)
Hou Shao-Sheng Email: ayhss@163.com.
Abstract 2n=q+(2n-q), we call it Hou Shaosheng's identity, that is, when the variable q takes any value, this equation is always true.
Let 3≤q≤n, and q be odd. When q takes all the odd numbers in the interval [3,n], (2n-q) takes all the odd numbers in the interval [n,2n-3].
So, 2n=q+(2n-q) contains all the possibilities for 2n to be decomposed into the sum of two odd numbers.
Goldbach's conjecture 2n=pi+pj is only a part of Hou's identity 2n=q+(2n-q).
In order to fully grasp the law of 2n=pi+pj, we must study the basic law of 2n=q+(2n-q). The basic law of 2n=q+(2n-q) is Hou Shaosheng's theorem.
The basic task of this section is to introduce Hou Shaosheng's identity and prove Hou Shaosheng's theorem. Then the mathematical meaning of the mathematical formula of Hou Shaosheng's theorem is explained.
Key words Identity; Odd number; Odd prime numbers; Odd composite number.
[Document ID] A [Document number]
3.1. Basic Properties of the Identity 2n=q+(2n-q)
2n=q+(2n-q), the most basic property is: 2n=q+(2n-q) is a mathematical identity, that is, regardless of any value of the variable q, this equation is always true.
3.1.1. 2n=q+(2n-q), which includes all possibilities for factorization of 2n into the sum of two odd numbers
2n=q+(2n-q), when 3≤q≤n, q can take any odd number in the interval [3, n]; Also, (2n-q), can take any odd number in the interval [n, 2n-3].
So, 2n=q+(2n-q) contains all the possibilities for 2n to be decomposed into the sum of two odd numbers.
So Goldbach's conjecture 2n=pi+pj is only part of the case of 2n=q+(2n-q).
Similarly, 2n=1+ 2,2 n=1+3,... It's all part of 2n=q+(2n-q). 1, here, is an odd prime number. 2 means the product of not more than 2 odd primes, 3 means the product of not more than 3 odd primes,... . Chen's proof of 1+2 is 2n=1+2 here.
Since 2n=q+(2n-q) contains all the possibilities of factorizing 2n into the sum of two odd numbers, 2n=q+(2n-q) contains all the laws of factorizing 2n into the sum of two odd numbers. So, as long as we grasp the law of 2n=q+(2n-q), this makes us grasp the whole law of 2n=pi+pj, no exceptions will occur.
In order to fully grasp the law of 2n=pi+pj, we must make a comprehensive study of 2n=q+(2n-q). So 2n=q+(2n-q) is the research object of this part, our purpose is to master the basic law of 2n=q+(2n-q), so as to grasp the law of 2n=pi+pj more comprehensively.
The basic law of 2n=q+(2n-q) is Hou Shao-sheng's theorem, which is the only theoretical basis for proving the conjecture in this paper.
3.1.2. To Study the Relationship between 2n=q+(2n-q) and 2n=pi+pj, it MUST BE ASSumed that 3≤q≤n, and q is odd.
Since we are looking at the relationship between 2n=q+(2n-q) and 2n=pi+pj, we should first exclude cases that are unrelated to this.
When both q and (2n-q) take odd primes, 2n=q+(2n-q), 2n is decomposed into the sum of two odd primes, which is Goldbach's conjecture 2n=pi+pj.
When q is even, (2n-q) is also even, 2n=q+(2n-q), and 2n is decomposed into the sum of 2 even numbers, This has nothing to do with Goldbach's conjecture. To rule this out, it is agreed that q cannot be even.
When q=1, (2n-1) is odd, 2n=1+ (2n-1), which has nothing to do with Goldbach's conjecture. To rule this out, the convention cannot be that q=1.
To rule out q being even and q=1, it is necessary to set 3≤q and q to be odd.
In order to avoid repetition between q+ (2n-q) and (2n-q) +q, it is necessary to set q≤(2n-q), that is, q≤n.
It has been set above that 3≤q, q is odd, and q≤n.
So we must set 3≤q≤n, and q is odd.
Let 3≤q≤n, q is odd, this is our basic prerequisite for studying the relationship between 2n=q+(2n-q) and 2n=pi+pj. In later papers, if it is not specifically stated, whether or not the paper mentions that 3≤q≤n, q is odd, this condition always exists.
3.1.3. num(3≤p≤n)=num(0≤p≤n)
After 3≤q≤n, q is odd, q can only take odd prime numbers or odd composite numbers in the interval [3, n]; (2n-q) can only take odd prime numbers or odd composite numbers in the interval [n, 2n-3].
Note: Since there are no odd primes or odd composite numbers in the interval [0, 2], taking odd prime or odd composite number in the interval [0, n] is, in fact, taking odd prime or odd composite number in the interval [3, n].
So: q takes the odd prime number or odd composite number in the interval [3, n];
And q are odd prime numbers or odd composite numbers in the interval [0, n];
These two expressions are different, but their mathematical nature is exactly the same.
In our mathematical notation, it is:
num(3≤p≤n)=num(0≤p≤n) (3.1.3.1)
Where, num(3≤p≤n) represents the number of odd primes p in the interval [3,n];
num(0≤p≤n) represents the number of odd primes p in the interval [0,n].
So, when you see num(3≤p≤n), you can assume that num(3≤p≤n) is num(0≤p≤n). However, it must be said that num(3≤p≤n) is absolutely accurate.
Similar to:
num(3≤h≤n)=num(0≤h≤n) (3.1.3.2)
Where, num(3≤h≤n) represents the number of odd composite number h in the interval [3,n];
num(0≤h≤n) represents the number of odd composite number h in the interval [0,n].
It must be said that num(3≤h≤n) is absolutely accurate. h is the first letter of the word of composite number in Hanyu Pinyin.
It should also be noted that 2n-q is actually a function of q, 3
≤
q
≤
n, q is odd.
Because 3≤q≤n, 2n-q≤2n-3, that is, 2n-q cannot take the only odd number in the interval [2n-2, 2n] : 2n-1.
Therefore: (2n-q) can only take odd prime numbers or odd composite numbers in the interval [n, 2n-3].
Because in the interval [2n-2, 2n], there is only one odd number: 2n-1. This 2n-1, which is an odd composite number, or an instance of an odd prime number, exists. 2n-1, odd composite number, odd prime number, depending on the specific n. This brings complexity to our research.
If 2n-1,is an odd composite number or an odd prime number, you're not sure, the following mathematical expression :
num(n≤p≤2n-3)=num(n≤p≤2n)
There are problems.
It is because of the above problems that, in order to be correctly represented, we must use the following mathematical notation.
num(n≤p≤2n-3) represents the number of odd prime numbers p in the interval [n,2n-3];
num(n≤h≤2n-3) represents the number of odd composite number h in the interval [n,2n-3].
3.1.4. q,(2n-q), is two odd numbers symmetric about n, 3≤q≤n, q is odd.
Note that q and (2n-q), are two odd numbers that are symmetric about n, 3≤q≤n, q is odd.
q,(2n-q), with respect to n-symmetry, can be proved as follows:
∣n-q∣=∣(2n-q)-n∣.
∣n-q∣ is the absolute value of n-q, which is the distance between point n and point q.
Since the distance from point q to point n is equal to the distance from point 2n-q to point n, q,(2n-q), is symmetric about point n.
3.1.5. n-△, n+△, symmetric about n; q, (2n-q), symmetric about n.
In 2n=pi+pj, we emphasize that only n-△, n+△, are odd prime numbers, and n-△=pi, n+△=pj, 2n is the sum of two odd prime numbers.
In 2n=q+(2n-q), we emphasize that 2n is the sum of two odd prime numbers only when q, (2n-q), are both odd prime numbers.
When n-△, n+△, are all odd prime numbers, and q, (2n-q), are all odd prime numbers, there must be: q=n-△, 2n-q= n+△.
In the picture below, q=n-△, 2n-q=n+△.
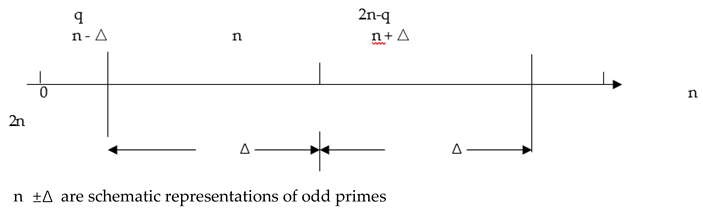
And q, (2n-q), regarding n symmetry
We notice that the distance from point q to origin 0 is q. The distance from point 2n-q to point 2n is q.
3.1.6. 2n=q + (2n-q) has only the following 4 specific decomposition forms:
2n=pi+pj;2n=ps+hr;2n=ht+hu;2n=hk+pd.
Where: pi,ps,ht,hk∈[3,n];pj,hr,hu,pd∈[n,2n-3].
That is: pi≤pj;ps<hr;ht≤hu;hk<pd.
The odd number before the plus sign is less than or equal to the odd number after the plus sign.
p is the first letter of prime; h is a composite number, the first letter of Chinese Pinyin.
All the odd prime numberspin the interval [3,n] are divided into two parts:pi,ps.
pi, combined with the odd prime number pj in the interval [n,2n-3], to form 2n=pi+pj;
ps, combined with the odd composite number hr in the interval [n,2n-3], to form 2n=ps+hr;
All odd composite numbershin the interval [3,n] are divided into two parts: ht,hk.
ht, combined with the odd composite number hu in the interval [n,2n-3], to form 2n=ht+hu;
hk, combined with the odd prime pd in the interval [n,2n-3] to form 2n=hk+pd;
In the process described above,
The odd prime numbers p in the interval [n,2n-3] are also divided into two parts: pj,pd.
The odd composite numbershin the interval [n,2n-3] are also divided into two parts: hu,hr.
The following is a specific analysis as follows:
First: 2n= sum of two odd primes. The mathematical expression is 2n=pi+pj, where pi≤pj .
This is Goldbach's conjecture. pi∈[3,n],pj∈[n,2n-3].
Second: 2n= sum of odd primes and odd composite numbers. The mathematical expression is 2n=ps+hr , where ps<hr. ps∈[3,n],hr∈[n,2n-3].
Third: 2n= sum of 2 odd composite numbers. The mathematical expression is 2n=ht+hu, where ht≤hu. ht∈[3,n],hu∈[n,2n-3].
Fourth: 2n= Sum of odd composite numbers and odd prime numbers. The mathematical expression is 2n=hk+pd , where hk<pd. hk∈[3,n],pd∈[n,2n-3].
In addition to the above 4 factorization forms, 2n is not factorized into the sum of two odd numbers.
3.1.7. Summary of Interval Problems
In this paper, the use of interval symbols, there are two categories.
The first kind: num(3≤p≤n) is used to represent the number of odd prime p in the interval [3,n];
num(3≤h≤n) is used to represent the number of odd composite h in the interval [3,n];
The interval used here is the [3,n] closed interval.
The second kind : num(n≤p≤2n-3) represents the number of odd prime numbers p in the interval [n,2n-3];
num(n≤h≤2n-3) is used to represent the number of odd composite number h in the interval [n,2n-3];
The interval used here is the [n,2n-3] closed interval.
Another interval that appears in the paper is [n,2n]. It should be noted that [n,2n] has only 3 more positive integers than [n,2n-3] : 2n-2,2n-1,2n. Of these 3 positive integers, only 2n-1 is odd. And there are practical examples of 2n-1 being odd prime numbers and 2n-1 being odd composite numbers.
We're looking at the range of values of 2n-q on the number line. Since 3≤q≤n, [n,2n-3] must be used, and [n,2n] cannot be used.
However, Section 2 of the paper uses [n,2n] in the title of the insufficient approximation formula of the number of odd primes in the interval [n,2n]. This is because, first of all, it is relatively simple to derive the insufficient approximation formula of the number of odd primes in the interval [n,2n]. Then it is easier to prove that there must be odd primes in the interval [n,2n-3].
In short, where [n,2n] is used and where [n,2n-3] is used depends on the specific mathematical problem. Because of the complexity of Goldbach's conjecture, because the specific mathematical problems are different, it is impossible to use a mathematical notation that applies to the whole paper.
3.2.8. mathematical examples and expansions of Hou Shaosheng's identity.
The following eight examples provide mathematical facts for readers to test our theory with concrete examples. The example itself does not constitute a proof of the theorem and Goldbach's conjecture, but it helps the reader to understand the mathematical formula of the theorem and has the function of showing that Goldbach's conjecture may be correct.
Here are eight specific mathematical examples. For readers to check the relevant formulas used in the paper. The example 3 used in the paper is the example 3 below.
Underlined numbers are odd prime numbers, and ununderlined numbers are odd composite numbers.
Eight examples, are given randomly, and then the results of testing, not deliberately arranged. Attached are 8 examples for readers to test the relevant theorems and formulas in the paper.
The number after the formula is the number in the relevant chapter when the formula was first proved in the paper.
In the following formula, there are:
pi, pj, ps, pd, are all odd prime numbers; ht, hu, hr, hk, are all odd composite numbers.
And pi≤pj, ht≤hu, ps≤hr, hk≤pd.The rule is: in the sum of two numbers, the former is less than the latter.
Example 1. 2×152=304
=1+303=3+301=5+299=7+297=9+295=11+293=13+291=15+289=17+287=19+285
=21+283=23+281=25+279=27+277=29+275=31+273=33+271=35+269=37+267
=39+265=41+263=43+261=45+259=47+257=49+255=51+253=53+251=55+249
=57+247=59+245=61+243=63+241=65+239=67+237=69+235=71+233=73+231
=75+229=77+227=79+225=81+223=83+221=85+219=87+217=89+215=91+213
=93+211=95+209=97+207=99+205=101+203=103+201=105+199=107+197
=109+195=111+193=113+191=115+189=117+187=119+185=121+183=123+181
=125+179=127+177=129+175=131+173=133+171=135+169=137+167=139+165
=141+163=143+161=145+159=147+157=149+155=151+153.
In this example:
num(2×152=pi+pj)=10. num(2×152=ht+hu)=24. num(2n=ps+hr)=25.
num(2n=hk+pd)=16. num(3≤p≤301)=61. num(3≤h≤301)=89.
num(2×152=q+(2n-q))=75.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 2. 2×52=104
=1+103=3+101=5+99=7+97=9+95=11+93=13+91=15+89=17+87=
=19+85=21+83=23+81=25+79=27+77=29+75=31+73=33+71=35+69
=37+67=39+65=41+63=43+61=45+59=47+57=49+55=51+53.
In this example:
num(2×52=pi+pj)=5. num(2×52=ht+hu)=5. num(2n=ps+hr)=9.
num(2n=hk+pd)=6. num(3≤p≤101)=25. num(3≤h≤101)=25.
num(2×52=q+(2n-q))=25.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 3. This example 3 is the example used in the following paper. In this paper, the mathematical meaning of each formula (3.3.0), (4.0.1), (4.0.2), (4.0.3), (4.0.4) is explained by this example 3. 4 sources of 2k=2(n+1)=pi++pj+ are verified; 4 sources of 2k=2(n+1)=ps++hr+ are verified. 4 sources of 2k=2(n+1)=ht++hu+ are verified. 4 sources of 2k=2(n+1)=hk++pd+ are verified.
It is more convincing to test the above problems with one example than to test the above problems with several examples, this example to test this problem, and that example to test that problem. This is one of the reasons why Example 3 is used repeatedly; The second is Example 3, which is of moderate size and easy to use.
Readers are welcome to use other examples to verify all the formulas in the paper.
Example 3. 2×51=102
=1+101=3+99=5+97=7+95=9+93=11+91=13+89=15+87=17+85=
=19+83=21+81=23+79=25+77=27+75=29+73=31+71=33+69=35+67
=37+65=39+63=41+61=43+59=45+57=47+55=49+53=51+51.
In this example:
num(2×51=pi+pj)=8. num(2×51=ht+hu)=9. num(2n=ps+hr)=6.
num(2n=hk+pd)=2. num(3≤p≤99)=24. num(3≤h≤99)+1=26.
num(2×51=q+(2n-q))=25.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 4. 2×50=100
=1+99=3+97=5+95=7+93=9+91=11+89=13+87=15+85=17+83=
=19+81=21+79=23+77=25+75=27+73=29+71=31+69=33+67=35+65=
37+63=39+61=41+59=43+57=45+55=47+53=49+51.
In this example:
num(2×50=pi+pj)=6. num(2×50=ht+hu)=6. num(2n=ps+hr)=8.
num(2n=hk+pd)=4. num(3≤p≤97)=24. num(3≤h≤97)=24.
num(2×50=q+(2n-q))=24.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 5. 2×49=98
=1+97=3+95=5+93=7+91=9+89=11+87=13+85=15+83=17+81=19+79
=21+77=23+75=25+73=27+71=29+69=31+67=33+65=35+63=37+61
=39+59=41+57=43+55=45+53=47+51=49+49.
In this example:
num(2×49=pi+pj)=3. num(2×49=ht+hu)=4. num(2n=ps+hr)=11. num(2n=hk+pd)=6. num(3≤p≤95)=23. num(3≤h≤95)+1=25.
num(2×49=q+(2n-q))=24.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 6. 2×48=96=
=1+95=3+93=5+91=7+89=9+87=11+85=13+83=15+81=17+79=19+77
=21+75=23+73=25+71=27+69=29+67=31+65=33+63=35+61=37+59
=39+57=41+55=43+53=45+51=47+49.
In this example:
num(2×48=pi+pj)=7. num(2×48=ht+hu)=7. num(2n=ps+hr)=7.
num(2n=hk+pd)=2. num(3≤p≤93)=23. num(3≤h≤93)=23.
num(2×48=q+(2n-q))=23.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 7. 2×40=80
=1+79=3+77=5+75=7+73=9+71=11+69=13+67=15+65=17+63=19+61
=21+59=23+57=25+55=27+53=29+51=31+49=33+47=35+45=37+43=39+41.
In this example:
num(2n=pi+pj)=4. num(2n=ht+hu)=3. num(2n=ps+hr)=7.
num(2n=hk+pd)=5. num(3≤p≤77)=20. num(3≤h≤77)=18.
num(2×40=q+(2n-q))=19.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Example 8. 2×19=38
=1+37=3+35=5+33=7+31=9+29=11+27=13+25=15+23=17+21=19+19.
In this example:
num(2n=pi+pj)=2. num(2n=ht+hu)=0. num(2n=ps+hr)=5.
num(2n=hk+pd)=2. num(3≤p≤35)=10. num(3≤h≤35)=7.
num(2×19=q+(2n-q))=9.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
End of example.
In the 8 examples, the number with an underscore is an odd prime; Ununderlined numbers are odd composite numbers. The statistical results of the formula in this example are also given. Readers can review these statistical results and also use these results to verify the formulas of Hou Shaosheng's theorem and the formulas derived from Hou Shaosheng's theorem mentioned below.
The purpose of this paper is to prove Goldbach's conjecture. It has already been pointed out in the preface that the mathematical representation of Goldbach's conjecture is 2n=pi+pj. However, 2n=pi+pj is only part of 2n=q+(2n-q). In order to fully grasp the law of 2n=pi+pj, we must study the law of 2n=q+(2n-q). The law of 2n=q+(2n-q) is Hou Shaosheng's theorem.
The expansion of Hou's identity, depending on whether the end of the expansion is even or odd, has two different manifestations.
If the end of the expansion is even, we call it the identity 1.
If the end of the expansion is odd, we call it the identity 2.
The expansion of identity 1 is as follows:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n-1+(2n-n+1).(2∣n) .
2∣n, which means n is even.
The expansion of identity 2 is as follows:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n+(2n-n); (2n).
2n, means that n is odd.
If the reader wants to know:
Why is the end of the identity 1: n-1+(2n-n+1), (2∣n).
Why the end of the identity 2 is: n+(2n-n), (2n).
Please look at the end of identity 1 and identity 2 in 3.5. Why are they different?
After our research, we found that although the final representation of identity 1 and identity 2 are not exactly the same, they have a common general formula, which is 2n=q+(2n-q).
Most importantly, identity 1 and identity 2 have a common property, which is the following Hou Shaosheng theorem. Therefore, the common properties of identity 1 and identity 2 are combined into a Hou Shao-sheng theorem. However, when describing the conditions of Hou Shaosheng's theorem, we must also describe identity 1 and identity 2 respectively. In describing their common properties, only one common representation is needed. Thus, the following representation of Hou Shaosheng's theorem is formed.
Above, we have made necessary preparations for proving Hou Shaosheng's theorem. Next, we prove Hou Shaosheng's theorem.
3.3. Theorem 3 (Hou Shaosheng theorem)
Following
Identity 1:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n-1+(2n-n+1).(2∣n) .
Identity2:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n+(2n-n); (2n).
There must be:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
In the above formula, the convention is: 3≤q≤n,q is odd.
pi, pj, ps, pd, are all odd prime numbers; ht, hu, hr, hk, are all odd composite numbers.
And: pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
Proof Now prove Hou Shaosheng's theorem as follows:
As we all know, 1 is odd, but it is not prime. 2 is the smallest prime number, the only prime number in an even number. All prime numbers except 2 are odd prime numbers. All odd numbers greater than 1 are either odd prime numbers or odd composite numbers.
Because we are studying the Goldbach conjecture, if m is a positive integer, obviously 2m+(2n-2m) is the sum of two even numbers, not the sum of two odd numbers, and certainly not the sum of two odd prime numbers, so 2m+(2n-2m) has nothing to do with Goldbach's conjecture. So delete the form of 2m+(2n-2m) from the expansion of 2n=q+(2n-q).
To remove the form 2m+(2n-2m), we agree that in the identity 2n=q+(2n-q),q denotes odd.
When q=1, (2n-1) is odd, and 2n=1+(2n-1).1+(2n-1), not the 2 odd primes sum of. So 2n=1+(2n-1), it cannot be a part of Goldbach's conjecture 2n=pi+pj. Therefore, 1+(2n-1) needs to be excluded, for which we need to agree that q > 1. q > 1, which is odd, so q≥3.
To rule out q=1 and q being even, it is necessary to set 3≤q and q to be odd.
In order to avoid repetition between q+ (2n-q) and (2n-q) +q, it is necessary to set q≤ (2n-q), that is, q≤n.
It has been set above that 3≤q, q is odd, and q≤n.
So we must set 3
≤
q
≤
n, and q is odd.
After setting 3≤q≤n, and q is odd, q can only take odd numbers in the interval [3, n].
(2n-q) can only take odd number in the interval [n, 2n-3].
Note that in the identity 2n=q+(2n-q), q is an odd number not less than 3, which excludes q=1.
num(2n=q+(2n-q)) Indicates the number of 2n=q+(2n-q).
Under the condition 3≤q≤n, 2n=1+(2n-1) cannot be one of 2n=q+(2n-q),
so 2n=1+(2n-1) is not included in calculating num(2n=q+(2n-q)).
2n-1 could be an odd prime number, or it could be an odd composite number. Since num(2n=q+(2n-q)) does not include 2n=1+(2n-1), 2n-1 must be excluded when calculating the number of odd primes p.
After excluding 2n-1, therefore, when calculating the number of odd primes p in the interval [0,2n], we should calculate the number of odd primes p in the interval [3,2n-3]. Therefore, num(3≤p≤2n-3) must be used.
num(3≤p≤2n-3) indicates the number of odd primes p in the interval [3,2n-3].
We use q to represent odd numbers, and q≥3.
num(2n=q+(2n-q)) is used to represent the number of 2n=q+(2n-q).
In 2n=q+(2n-q), we agree that 3
≤
q
≤
(2n-q), that is, 3
≤
q
≤
n, q is
odd.
Under this convention, q takes all odd numbers in the interval
[3, n], and (2n-q) takes all odd numbers in the interval [n, 2n-3].
We agree that q≤(2n-q) is reasonable. Because 2n=q+(2n-q)=(2n-q)+q.
If q≤(2n-q) is not agreed, after considering 2n=q+(2n-q), 2n=(2n-q)+q is also considered, forming a repetition.
For example, 2n=3+(2n-3), and 2n=(2n-3)+3, form a redundant
repetition;
2n=5+(2n-5), with 2n=(2n-5)+5, and so on.
After we agree that q≤(2n-q), we can avoid the above redundant
repetition.
What are the numbers of 2n=q+(2n-q)?
The raising of this question is the breakthrough to prove Hou Shao-sheng's theorem.
Since q≤(2n-q), that is, q≤n, q is odd. And since 3≤q≤n, so the number of q is the number of all odd numbers in the interval [3, n]. Further, the number of q is also the number of q+(2n-q), which is also the number of 2n=q+(2n-q).
The above instructions are applicable to the whole paper and will not be repeated.
Now we have to ask, how many 2n=q+(2n-q)?
How does the number of 2n=q+(2n-q) relate to the number of 2n=pi+pj?
How does the number of 2n=q+(2n-q) relate to the number of 2n=ht+hu?
How does the number of 2n=q+(2n-q) relate to the number of 2n=ps+hr?
How does the number of 2n=q+(2n-q) relate to the number of 2n=hk+pd?
In 2n=q+(2n-q), and by convention q≤(2n-q); So q and 2n-q could both be odd prime numbers, or they could both be odd composite numbers, or one could be odd prime numbers, and the other could be odd composite numbers.
Note: odd numbers greater than 1 are either odd prime numbers or odd composite numbers.
When both q and (2n-q) are odd prime numbers, 2n=q+(2n-q) is 2n=pi+pj, pi≤pj.
When both q and (2n-q) are odd composite numbers, 2n=q+(2n-q) is 2n=ht+hu, ht≤hu.
When q is an odd prime and (2n-q) is an odd composite, 2n=q+(2n-q) is 2n=ps+hr, ps<hr.
q is an odd composite number, and when (2n-q) is an odd prime, 2n=q+(2n-q) is 2n=hk+pd, hk<pd.
Where: pi, pj, ps, pd, are all odd prime numbers; ht, hu, hr, hk, are all odd composite numbers.
So num(2n=pi+pj), num(2n=ht+hu), num(2n=ps+hr), num(2n=hk+pd)are all part of num(2n=q+(2n-q)), and there is no repetition between any of the two.
Furthermore, there is no other combination of q and (2n-q) except 2n=pi+pj, 2n=ht+hu, 2n=ps+hr, 2n=hk+pd.
And so there must be:
num(2n=q+(2n-q))= num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
(3.3.0) Proved.
(3.3.0) is the mathematical formula of Hou Shaosheng's theorem.
Hou Shaosheng's theorem is described in words as follows:
The number of (2n =q+(2n-q)) is equal to
number of (2n=pi+pj) + number of (2n=ht+hu)
+number of(2n=ps+hr)+ number of(2n=hk+pd).
Hou Shaosheng's theorem is described in words as follows:
The number of 2n factorized into the sum of two odd numbers is equal
to the number of 2n factorized into the sum of two odd primes, plus the number of 2n factorized into the sum of two odd composite numbers, plus the number of 2n factorized into the sum of odd primes and odd composite numbers, plus the number of 2n factorized into the sum of odd composite numbers and odd prime number.
Theorem 3, complete proof.
3.4. (3.3.0) Mathematical meaning of the formula
(3.3.0) The mathematical meaning of the formula is as follows:
(3.3.0) Established, not only is the quantity on both sides of the equal sign equal. There are deeper mathematical implications, as follows.
(3.3.0) is true, that is, the number of odd numbers q in the interval [3,n] on both sides of the equal sign is equal.
On the left side of the equal sign, num(2n=q+(2n-q)) represents the number of all odd q in the interval [3,n]; As an equation, the right side of the equal sign must also be the number of all odd numbers q in the interval [3,n]. pi,ht,ps,hk, they are all odd numbers in the interval [3,n], and there are no other odd numbers.
(3.3.0) is established, which can also be understood as: that is, the number of odd numbers (2n-q) in the interval [n,2n-3] on both sides of the equal sign is equal,3≤q≤n.
On the left side of the equal sign, num(2n=q+(2n-q)) represents the number of all odd numbers (2n-q) in the interval [n,2n-3]; As an equation, the right side of the equal sign must also be the number of all odd numbers (2n-q) in the interval [n,2n-3]. pj,hu,hr,pd, they are all odd numbers in the interval [n,2n-3], and there are no other odd numbers.
(3.3.0) The formula gives:
num(2n=q+(2n-q)),
num(2n=pi+pj), num(2n=ht+hu), num(2n=ps+hr), num(2n=hk+pd),
the quantitative relationship between them has laid a reliable theoretical foundation for the study and proof of Goldbach's conjecture. Goldbach's conjecture, which is the conjecture num(2n=pi+pj)≥1, is only a part of Hou Shaosheng's theorem.
Identity 1:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n-1+(2n-n+1).(2∣n) .
Identity 2:
2n=1+(2n-1)= 3+(2n-3)=5+(2n-5)=7+(2n-7)=9+(2n-9)=11+(2n-11)=
=13+(2n-13)=15+(2n-15)=17+(2n-17)=19+(2n-19)=21+(2n-21)=23+(2n-23)=
=25+(2n-25)=…=q+(2n-q)=…=n+(2n-n); (2n).
It's like the endless Pacific Ocean. Goldbach's conjecture 2n=pi+pj is only a part of 2n=q+(2n-q). It's impossible to study Goldbach's conjecture directly from identity 1, identity 2.
Hou Shaosheng's theorem covers all cases where 2n is decomposed into the sum of two odd numbers. This provides a reliable theory for us to study Goldbach's conjecture as a whole.
The mathematical formula of Hou Shaosheng's theorem is the theoretical basis for us to prove Goldbach's conjecture. The following series of reasoning processes begin with the mathematical formula of this theorem. Therefore, the mathematical formula of this theorem does not allow any error and must be absolutely correct. The above reasoning process should be said to be absolutely rigorous. However, whether our understanding is completely in line with mathematical reality needs to be verified by mathematical practical examples.
Above, we give the mathematical meaning of the mathematical formula of Hou Shaosheng theorem. Next, we use two of the eight mathematical examples in 3.2 for validation.
Example 1 below is Example 1 of the 8 mathematical examples in 3.2. Now test (verify) the formula (3.3.0) with the relevant data in Example 1.
Example 1. 2×152=304
=1+303=3+301=5+299=7+297=9+295=11+293=13+291=15+289=17+287=19+285
=21+283=23+281=25+279=27+277=29+275=31+273=33+271=35+269=37+267
=39+265=41+263=43+261=45+259=47+257=49+255=51+253=53+251=55+249
=57+247=59+245=61+243=63+241=65+239=67+237=69+235=71+233=73+231
=75+229=77+227=79+225=81+223=83+221=85+219=87+217=89+215=91+213
=93+211=95+209=97+207=99+205=101+203=103+201=105+199=107+197=109+195
=111+193=113+191=115+189=117+187=119+185=121+183=123+181=125+179
=127+177=129+175=131+173=133+171=135+169=137+167=139+165=141+163
=143+161=145+159=147+157=149+155=151+153.
In this example:
num(2×152=pi+pj)=10. num(2×152=ht+hu)=24. num(2n=ps+hr)=25.
num(2n=hk+pd)=16. num(3≤p≤301)=61. num(3≤h≤301)=89.
num(2×152=q+(2n-q))=75.
The above data fully satisfy the following formula (3.3.0) :
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
Specific tests are as follows:
num(2n=q+(2n-q))=num(2×152=q+(2n-q))=75.
num(2n=pi+pj)=num(2×152=pi+pj)=10.
num(2n=ht+hu)= num(2×152=ht+hu)=24.
num(2n=ps+hr)= num(2×152=ps+hr)=25.
num(2n=hk+pd)=num(2×152=hk+pd)=16.
Plug the above numbers into (3.3.0) :
75=10+24+25+16.
Now both sides of the equal sign are 75.
So the formula (3.3.0) is exactly the same as example 1.
Example 2 below is Example 2 of the 8 mathematical examples in 3.2. Now check (validate) the formula (3.3.0) with the relevant data in Example 2.
Example 2. 2×52=104
=1+103=3+101=5+99=7+97=9+95=11+93=13+91=15+89=17+87=
=19+85=21+83=23+81=25+79=27+77=29+75=31+73=33+71=35+69
=37+67=39+65=41+63=43+61=45+59=47+57=49+55=51+53.
In this example:
num(2×52=pi+pj)=5. num(2×52=ht+hu)=5. num(2n=ps+hr)=9.
num(2n=hk+pd)=6. num(3≤p≤101)=25. num(3≤h≤101)=25.
num(2×52=q+(2n-q))=25.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
Specific tests are as follows:
num(2n=q+(2n-q))= num(2×52=q+(2n-q))=25.
num(2n=pi+pj)=num(2×52=pi+pj)=5.
num(2n=ht+hu)= num(2×52=ht+hu)=5.
num(2n=ps+hr)=num(2×52=ps+hr)=9.
num(2n=hk+pd)=num(2×52=hk+pd)=6.
Plug the above numbers into (3.3.0):
25 = 5 + 5 + 9 + 6.
25 on both sides of the equal sign, so equal.
So the formula (3.3.0) is exactly consistent with example 2.
Readers are welcome to verify the formula (3.3.0) with other examples.
3.5. Why are the ends of identity 1 and identity 2 different?
First of all, it should be clear that we are now talking about the end of the expansion of 2n=q+(2n-q). In proving Hou Shaosheng's theorem, it has been made clear that q is odd and must be 3≤q≤n. It can be seen that in the expansion of Hou Shao-sheng's identity, it is not necessary to consider the case of q=0, q=1. However, 2n=1+(2n-1) is still written in the expansion, so that the reader can think about the problem. However, when considering the range of values of (2n-q), it must still be: q is odd, and 3≤q≤n. That is, the value range of (2n-q) is [n,2n-3].
In 3.3, the proof of Theorem 3 (Hou Shaosheng's theorem), it has been
given that:
The end of the identity 1 is: n-1+(2n-n+1), (2∣n).
The end of the identity 2 is: n+(2n-n), (2n).
Let's first talk about the end of the identity 1, why it's n-1+(2n-n+1),(2∣n).
2∣n, which means n is even. Since n is even, n-1 is odd.
First, it is clear that n-1+(2n-n+1) must be one of the manifestations of q+(2n-q).
Under the condition that n-1 is odd, n-1+(2n-n+1) is the representation of q+(2n-q). Now, q=n-1, which is consistent with the requirement that q is odd.
Note that this is the time to discuss the specific manifestation of the expansion of q+(2n-q). Not to study the range of values of (2n-q).
Now let's say n=20.
2n=2×20. Now write 2n=2×20 in the form of Hou Shaosheng's identity
as follows:
2n=2×20=1+39=3+37=5+35=7+33=9+31=11+29
=13+27=15+25=17+23=19+21. (3.5.1)
In (3.5.1), 1+39 can be deleted.
2×20 expansion can only end in 19+21.
Otherwise, the next is: 21+19=23+17.
From the commutative law of addition, 19+21=21+19, 17+23=23+17.
However, since we already have 19+21, 21+19 is superfluous.Since we already have 17 + 23, 23 +17 is superfluous.
Because we already have 1+39=3+37=5+35=7+33=9+31=11+29
= 13+27=15+25=17+23=19+21.
So 21+19=23+17=... =37+3=39+1, is superfluous.
This means that 21+19=23+17=... =37+3=39+1. This arrangement is redundant and repetitive in this article. So it should be deleted.
So the end of a 2×20 expansion can only be 19+21. It has to be 19 <n=20.
Because the general term formula for the identity 1 is: 2n=q+(2n-q), where 3≤q≤n,q is odd. So when n is even, if the end is n+(2n-n), then q=n, contradicting the convention that q is odd.
Therefore, when n is even, the end of the expansion can only be: n-1+(2n-n+1), when q=n-1, is odd, in accordance with the convention that q is odd.
Therefore, the end of the identity 1 can only be: n-1+(2n-n+1). It cannot be: n+(2n-n).
Let's discuss why the end of the identity 2 is: n+(2n-n), (2n).
2n means that n is odd.
Under the condition that n is odd, n+(2n-n) corresponds to the expression q+(2n-q). Now q=n, which is consistent with the convention that q is odd.
Now let's say n=21.
2n=2×21. Now write 2n=2×21 in the form of Hou Shaosheng's identity
as follows:
2n=2×21=1+41=3+39=5+37=7+35=9+33=11+31
=13+29=15+27=17+25=19+23=21+21. (3.5.2)
In (3.5.2), the general term formula remains: 2n=q+(2n-q), where 3≤q≤n and q is odd.
In (3.5.2), 1+41 can be deleted.
Because we already have 2×21=1+41=3+39=5+37=7+35=9+33=11+31
=13+29=15+27=17+25=19+23=21+21.
So 2×21=23+19=……=37+5=39+3=41+1.it is redundant and should be deleted.
So the end of a 2×21 expansion can only be 21+21. it has to be: q=21=n.
Because the general term formula for the identity 2 is: 2n=q+(2n-q), where 3≤q≤n,q is odd. So when n is odd, if the end is n+(2n-n), then q=n, which is consistent with the convention that q is odd.
If the end of the expansion is: n-1+(2n-n+1), then q=n-1,(n-1) is even, not in accordance with the convention that q is odd.
Therefore, the end of the identity 2 can only be: n+(2n-n). It cannot be: n-1+(2n-n+1).
Brief summary: The title of this section is: Goldbach's conjecture 2n=pi+pj, Fundamental Properties of the identity 2n=q+(2n-q) and Hou Shao-sheng's Theorem. In this section, we first define Hou Shao-sheng's identity, discuss the basic properties of 2n=q+(2n-q), and study the value interval of q and (2n-q), and the symmetry between q and (2n-q) about n. Then we give 8 mathematical examples from the paper. Eight examples are provided to verify Hou Shaosheng's theorem for readers.Hou Shaosheng's theorem is proved. In the following papers, we will use the examples to verify the relevant theorems.
The main task of this section is to prove Hou Shaosheng's theorem. We have given detailed proof. This theorem is the theoretical basis for proving our conjecture.
The secondary task of this section is to explain the meaning of the mathematical formula of Hou Shaosheng's theorem. We've given instructions.
Previously, we proved Hou Shaosheng's theorem. The mathematical formula of this theorem involves 5 variables: num(2n=q+(2n-q)), num(2n=pi+pj), num(2n=ht+hu), num(2n=ps+hr), num(2n=hk+pd).
5 variables, in a formula, constrain each other, making it difficult to prove the conjecture. Moreover, the quantitative relation of a certain side of Hou Shaosheng's identity is not specific and clear.
for example:
What is the relationship between the number of odd primes p in the interval [3, n] and num(2n=pi+pj)?
What is the relationship between the number of odd primes p in the interval [3, n] and num(2n=ht+hu)?
What is the relationship between the number of odd primes p in the interval [3, n] and num(2n=ps+hr)?
What is the relationship between the number of odd primes p in the interval [3, n] and num(2n=hk+pd)?
What is the relationship between the number of odd composite h in the interval [3, n] and num(2n=ht+hu)?
What is the relationship between the number of odd composite h in the interval [3, n] and num(2n=ps+hr)?
What is the relationship between the number of odd composite h in the interval [3, n] and num(2n=hk+pd)?
What is the relationship between the number of odd primes p in the interval [n, 2n-3] and num(2n=pi+pj)?
What is the relationship between the number of odd primes p in the interval [n, 2n-3] and num(2n=ht+hu)?
What is the relationship between the number of odd primes p in the interval [n, 2n-3] and num(2n=ps+hr)?
What is the relationship between the number of odd primes p in the interval [n, 2n-3] and num(2n=hk+pd)?
None of this is clear to us. In order to clarify these problems, we must analyze the mathematical formula of Hou Shaosheng's theorem. The result of the study is the following inference of Hou Shaosheng's theorem.
The inference of Hou Shaosheng's theorem has 4 mathematical formulas, which are the deepening and refinement of a certain aspect of Hou Shaosheng's theorem. Each mathematical formula, in some aspect, gives a specific quantitative relationship between the quantities concerned.
The 4 mathematical formulas in the inference of Hou Shaosheng's theorem are the main mathematical theories to prove our conjectures. Next, we prove 4 inferences of Hou Shaosheng's theorem.
For references, see the end.
Section 4, 4 inferences of Hou Shaosheng's theorem
Hou Shao-Sheng1, Ma Lin-Jun2
(1 Anyang Mathematical Society, Henan Province, China; 2 Department of Mathematics, Sun Yat-sen University, Guangzhou City, Guangdong Province, China)
Hou Shao-Sheng Email: ayhss@163.com.
Abstract The mathematical formula of Hou Shaosheng's theorem is as follows:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
This formula includes:
num(2n=q+(2n-q)),num(2n=pi+pj),num(2n=ht+hu),num(2n=ps+hr),num(2n=hk+pd).Formulas contain too much mathematical content. It is very inconvenient to prove Goldbach's conjecture directly by formula. So it is necessary to derive the 4 inferences of Hou Shaosheng's theorem.
The 4 inferences of Hou Shaosheng's theorem are as follows:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n,q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
The basic task of this section is to prove the 4 inferential formulas of Hou Shaosheng's theorem. The second is to explain the mathematical meaning of the 4 inferential formulas. And the 4 inferential formulas are verified by mathematical examples.
The 4 inferential formulas are the main theoretical tools for proving Goldbach's conjecture.
Therefore, this section is the central link between the preceding and the following.
Keyword Inference of theorem; odd number; odd prime number;
odd composite number.
[Document code] A [Document number]
4.0 Theorem 4, (Four corollaries of Hou Shaosheng's theorem).
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
always have:
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n,q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
The 4 mathematical formulas in the inference of Hou Shaosheng's theorem can be described in words as follows:
(4.0.1) is stated as follows: The number of odd primes p in the interval [3,n] is equal to the number of 2n decomposed into the sum of two odd primes, plus the number of 2n decomposed into the sum of odd primes and odd composite numbers.
(4.0.2) is stated as follows: the number of odd composite number h in the interval [3, n] is equal to the number of 2n decomposed into the sum of two odd composite number, plus the number of 2n decomposed into the sum of odd composite number and odd prime.
(4.0.3) is stated as follows: The number of odd primes p in the interval [n,2n-3] is equal to the number of 2n decomposed into the sum of two odd prime number, plus the number of 2n decomposed into the sum of odd composite number and odd prime.
(4.0.4) is stated as follows: The number of odd composite number h in the interval [n,2n-3] is equal to the number of 2n decomposed into the sum of two odd composite number,plus the number of 2n decomposed into the sum of odd prime number and odd composite number.
Proof: Because we agreed: pi≤pj, ht≤hu, ps<hr, hk<pd.
So there must be a causal relationship:
If 2n=pi+pj, because 3≤pi≤pj, therefore pi≤n; n≤pj≤2n-3.
If 2n=ps+hr, because 3≤ps<hr, therefore ps<n; n<hr≤2n-3.
If 2n=ht+hu, because 3≤ht≤hu, therefore ht≤n; n≤hu≤2n-3.
If 2n=hk+pd, because 3≤hk<pd, therefore hk<n; n<pd≤2n-3.
From the above we can see:
The only odd prime numbers in the interval [3,n] are: pi and ps. So (4.0.1) is true.
The only odd composite numbers in the interval [3,n] are: ht and hk. So (4.0.2) is true.
The only odd prime numbers in the interval [n,2n-3] are: pj and pd. So (4.0.3) is true.
The only odd composite numbers in the interval [n,2n-3] are: hu and hr. So (4.0.4) is true.
End of proof.
There are 4 mathematical formulas for the inference of Hou Shaosheng's theorem. These 4 mathematical formulas are the theorem in
num(3≤p≤n), num(3≤h≤n), num(n≤p≤2n-2), num(n≤h≤2n-2),
4 quantitative deepening and refinement.
In these 4 quantities, more specific is given:
num(2n=pi+pj), num(2n=ps+hr), num(2n=ht+hu), num(2n=hk+pd),
the relationship between. It plays an important role in proving Goldbach's conjecture.
Let's look at the mathematical implications of these 4 mathematical formulas.
4.1 (4.0.1), (4.0.2), (4.0.3), (4.0.4) the mathematical meaning of the formula
(4.0.1), (4.0.2), (4.0.3), (4.0.4), not only means that the quantity on both sides of the equal sign is equal, but also has a deeper mathematical meaning, as follows.
4.1.1 (4.0.1) Mathematical meaning of the formula
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr). (4.0.1)
The mathematical meaning of (4.0.1) is: The number of all odd primes p in the interval [3,n] is equal to the number of pi in num (2n=pi+pj) + the number of ps in num (2n=ps+hr). pi,ps are all the odd prime numbers p in the interval [3,n]. This is the most basic mathematical meaning of (4.0.1).
(4.0.1) True, not only is the quantity on both sides of the equal sign equal. There are deeper mathematical implications, as follows.
(4.0.1) is true, that is, the number of odd primes p in the interval [3,n] on both sides of the equal sign is equal. On the left side of the equal sign, num (3≤p≤n) represents the number of all odd prime numbers p in the interval [3,n]; As an equation, the right side of the equal sign must also be the number of all the odd primes p in the interval [3,n]. pi,ps, is all the odd prime numbers p in the interval [3,n]. This is the most basic mathematical meaning of (4.0.1).
(4.0.1) indicates that all the odd prime numbers p in the interval [3,n] are divided into pi and ps,2 parts.
pi is combined with the odd prime number pj in the interval [n,2n-3] to form 2n=pi+pi;
ps is combined with the odd composite number hr in the interval [n,2n-3] to form 2n=ps+hr.
There must be no other possibility than 2n=pi+pj and 2n=ps+hr.
pi,ps, are all part of the odd prime number p in the interval [3,n]. One is written as pi and the other as ps to distinguish between two different combinations: 2n=pi+pj, 2n=ps+hr. If we write them all as p, it will be difficult when we need to specify pi, or when we need to specify ps.
(4.0.1) True, contains two meanings:
First, the numbers on both sides of the equal sign are equal;
Second, things on both sides of the equal sign have the same name, and are the odd prime p in the interval [3,n].
If one of the two is missing, it cannot be an equation. This mathematical meaning is completely consistent with that in primary school arithmetic, only the numbers with the same name can be added or subtracted, and only the numbers with the same name can be equal.
The above properties of (4.0.1) play an important role in the process of proving the conjecture.
The above properties of (4.0.1) have been tested with 8 examples in the paper.
4.1.2 (4.0.2) Mathematical meaning of the formula
num (3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd). (4.0.2)
The mathematical meaning of (4.0.2) is: The number of all odd composite number h in the interval [3,n] is equal to the number of ht in num (2n=ht+hu) + the number of hk in num (2n=hk+pd). ht, hk are all the odd composite numbers h in the interval [3,n]. This is the most basic mathematical meaning of (4.0.2).
(4.0.2) True, not only is the quantity on both sides of the equal sign equal. There are deeper mathematical implications, as follows.
(4.0.2) is true, that is, the number of odd composite number h in the interval [3,n] on both sides of the equal sign is equal. On the left side of the equal sign, num (3≤h≤n) represents the number of all odd composite h in the interval [3,n]; As an equation, the right side of the equal sign must also be the number of all the odd composite h in the interval [3,n]. ht,hk, is all the odd composite numbers h in the interval [3,n].
(4.0.2) indicates that all the odd composite number h in the interval [3,n] are divided into ht and hk,2 parts.
ht is combined with the odd composite number hu in the interval [n,2n-3] to form 2n=ht+hu;
hk is combined with the odd prime number pd in the interval [n,2n-3] to form 2n=hk+pd.
There must be no other possibility than 2n=ht+hu and 2n=hk+pd.
In (4.0.2),ht, hk, is all the odd composite numbers h in the interval [3,n].
ht,hk, are all odd composite numbers h in the interval [3,n]. One is written as ht and the other as hk to distinguish between two different combinations: 2n=ht+hu, 2n=hk+pd. If we write both h, it will be difficult when we need to specify ht, or when we need to specify hk.
(4.0.2) True, contains two meanings:
First, the numbers on both sides of the equal sign are equal;
Second, things on both sides of the equal sign have the same name, and are the odd composite number h in the interval [3,n].
If one of the two is missing, it cannot be an equation. This mathematical meaning is completely consistent with that in primary school arithmetic, only the numbers with the same name can be added or subtracted, and only the numbers with the same name can be equal.
The above properties of (4.0.2) have been tested with 8 examples in the paper.
4.1.3 (4.0.3) The mathematical meaning
num (n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd). (4.0.3)
The mathematical meaning of (4.0.3) is: The number of all odd prime p in the interval [n,2n-3] is equal to the number of pj in num (2n=pi+pj) + the number of pd in num (2n=hk+pd). pj , pd are all the odd prime numbers p in the interval [n,2n-3]. This is the most basic mathematical meaning of (4.0.3).
(4.0.3) indicates that all the odd prime number p in the interval [n,2n-3] are divided into pj and pd,2 parts.
Pj is combined with the odd prime number pi in the interval [3,n] to form 2n=pi+pj;
pd is combined with the odd composite number hk in the interval [3,n] to form 2n=hk+pd.
There must be no other possibility than 2n=pi+pj and 2n=hk+pd.
In (4.0.3), pj, pd, is all the odd prime numbers p in the interval [n,2n-3].
pj, pd, are all odd prime numbers p in the interval [n,2n-3]. One is written pj and the other pd to distinguish between two different combinations: 2n=pi+pj, 2n=hk+pd. If we write them all as p, it will be difficult when we need to specify pj, or when we need to specify pd.
(4.0.3) is true, that is, the number of odd primes p in the interval [n,2n-3] on both sides of the equal sign is equal. On the left side of the equal sign, num (n≤p≤2n-2) represents the number of all odd prime numbers p in the interval [n,2n-3]; As an equation, the right side of the equal sign must also be the number of all the odd primes p in the interval [n,2n-3].
(4.0.3) True, contains two meanings:
First, the numbers on both sides of the equal sign are equal;
Second, things on both sides of the equal sign have the same name, and are the odd prime p in the interval [n,2n-3].
If one of the two is missing, it cannot be an equation. This mathematical meaning is completely consistent with the mathematical idea that only numbers with the same name can add or subtract, and only numbers with the same name can be equal in elementary school arithmetic.
The above properties of (4.0.3) have been tested with 8 examples in the paper.
4.1.4 (4.0.4) The mathematical meaning
num (n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
The mathematical meaning of (4.0.4) is: The number of all odd composite number h in the interval [n,2n-3] is equal to the number of hu in num(2n=ht+hu) + the number of hr in num (2n=ps+hr). hu, hr are all the odd composite numbers h in the interval [n,2n-3]. This is the most basic mathematical meaning of (4.0.4).
(4.0.4) indicates that all the odd composite numbers h in the interval [n,2n-3] are divided into hu and hr,2 parts. hu combines with the odd composite number ht in the interval [3,n] to form 2n=ht+hu; hr is combined with the odd prime ps in the interval [3,n] to form 2n=ps+hr.
There must be no other possibility than the above two: 2n=ht+hu, 2n=ps+hr.
In (4.0.4),hu, hr, are all the odd composite numbers h in the interval [n,2n-3].
The odd composite number h in the interval [n,2n-3], one written hu and the other written hr, is to distinguish between two different combinations: 2n=ht+hu, 2n=ps+hr. If we write both h, it will be difficult when we need to specify hu, or when we need to specify hr.
(4.0.4) is true, that is, the number of odd composite number h in the interval [n,2n-3] on both sides of the equal sign is equal. Before the equal sign, num (n≤h≤2n-3) indicates the number of all odd composite number h in the interval [n,2n-3]; As an equation, after the equal sign, it must also be the number of all the odd composite number h in the interval [n,2n-3].
(4.0.4) True, contains two meanings:
First, the numbers on both sides of the equal sign are equal;
Second, things on both sides of the equal sign have the same name, and are the odd composite number h in the interval [n,2n-3].
If one of the two is missing, it cannot be an equation. This mathematical meaning is completely consistent with the mathematical idea that only numbers with the same name can add or subtract, and only numbers with the same name can be equal in elementary school arithmetic.
The above properties of (4.0.4) have been tested with 8 mathematical examples in the paper.
The above meanings of the 4 formulas play a very important role in the process of proving the conjecture.
4.2 Verify the formula (3.3.0), (4.0.1), (4.0.2), (4.0.3), (4.0.4) with a mathematical example.
The following example, 3, is the third of the eight mathematical examples in 3.2: Instance 3. Now test (verify) formula (3.3.0), (4.0.1), (4.0.2), (4.0.3), (4.0.4) with the relevant data in Example 3.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=9+93=11+91=13+89=15+87=17+85=
=19+83=21+81=23+79=25+77=27+75=29+73=31+71=33+69=35+67
=37+65=39+63=41+61=43+59=45+57=47+55=49+53=51+51.
Note: Numbers underlined, or red numbers, odd prime numbers. Numbers without underscores, or blue numbers, odd composite numbers.
In the example above, the data is as follows:
num(2n=q+(2n-q))=25.
These 25 number of 2n=q+(2n-q) are as follows:
2n=3+99; 2n=5+97; 2n=7+95; 2n=9+93; 2n=11+91;
2n=13+89; 2n=15+87; 2n=17+85; 2n=19+83; 2n=21+81;
2n=23+79; 2n=25+77; 2n=27+75; 2n=29+73; 2n=31+71;
2n=33+69; 2n=35+67; 2n=37+65; 2n=39+63; 2n=41+61;
2n=43+59; 2n=45+57; 2n=47+55; 2n=49+53; 2n=51+51.
num(2×51=pi+pj)=8.
These 8 number of 2×51=pi+pj, as follows:
2n=5+97; 2n=13+89; 2n=19+83; 2n=23+79; 2n=29+73;
2n=31+71; 2n=41+61; 2n=43+59;
num(2×51=ht+hu)=9.
These 9 number of 2n=2×51=ht+hu are as follows:
2n=9+93; 2n=15+87; 2n=21+81; 2n=25+77; 2n=27+75;
2n=33+69; 2n=39+63; 2n=45+57; 2n=51+51.
num(2n=ps+hr)=6.
These 6 number of 2n=ps+hr are as follows:
2n=3+99; 2n=7+95; 2n=11+91; 2n=17+85; 2n=37+65;
2n=47+55;
num(2n=hk+pd)=2.
These 2 number of 2n=hk+pd are as follows:
2n=35+67; 2n=49+53;
num(3≤p≤51)=14.
The 14 odd prime numbers in the interval [3,51] are as follows:
3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47
.
num(n≤p≤2n-3)=10.
The 10 odd prime numbers in the interval [51, 99] are as follows:
97, 89, 83, 79, 73, 71, 67, 61, 59, 53.
num(3≤h≤n)= num(3≤h≤51)=11.
The 11 odd composite numbers h in the interval [3,51] are as follows:
9, 15, 21, 25, 27, 33, 35, 39, 45, 49, 51
num (n≤h≤2n-3)=15.
The 15 odd composite numbers h in the interval [51, 99] are as follows:
99, 95, 93, 91, 87, 85, 81, 77, 75, 69, 65, 63, 57, 55, 51.
After testing one by one, the above data fully satisfy each of the following formulas:
(3.3.0), (4.0.1), (4.0.2), (4.0.3), (4.0.4).
num (2n=q+(2n-q))= num (2n=pi+pj)+ num (2n=ht+hu)
+ num (2n=ps+hr)+ num (2n=hk+pd). (3.3.0)
num (3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr). (4.0.1)
num (3≤h≤n)= num(2n=ht+hu)+ num(2n=hk+pd); (4.0.2)
num (n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num (n≤h≤2n-3)= num(2n=ht+hu)+ num(2n=ps+hr). (4.0.4)
After testing (verification), the above data fully satisfy each of the above formulas. In the paper, there are other examples, the reader is also free to use examples to test (verify) the above formula.
Now check (verify) the mathematical meaning of the formula (4.0.1) as follows:
From example 3, statistics show that:
num(3≤p≤n)= num(3≤p≤51)=14.
The 14 odd prime numbers p are:
3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47.
num(2n=pi+pj)=8.
These 8 number of 2×51=pi+pj are:
2×51=5+97, 2×51=13+89, 2×51=19+83, 2×51=23+79, 2×51= 29+73, 2×51=31+71, 2×51=41+61, 2×51=43+59.
8 of these pi are: 5,13,19,23, 29,31,41,43.
num(2n=ps+hr)=6.
The 6 number of 2×51=ps+hr are:
2×51=3+99, 2×51=7+95, 2×51=11+91,
2×51=17+85, 2×51=37+65, 2×51=47+55.
6 of these ps are: 3, 7, 11, 17, 37, 47.
The above 8 of pi and 6 of ps are all the odd prime numbers in the interval [3,51].
Arrange the above 8 of pi and 6 of ps in order from small to large to get:
3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47.
These 14 odd prime numbers are the 14 odd prime numbers obtained by statistics from example 3.
This example, once again, illustrates the mathematical meaning of (4.0.1) :
The number of all odd primes p in the interval [3,n] is equal to the number of pi in num (2n=pi+pj) + the number of ps in num (2n=ps+hr). pi, ps are all the odd prime numbers p in the interval [3,n]. This is the most basic mathematical meaning of (4.0.1).
(4.0.1) True, not only is the quantity on both sides of the equal sign equal. There are deeper mathematical implications, as follows.
(4.0.1) is true, that is, the number of odd primes p in the interval [3,n] on both sides of the equal sign is equal. On the left side of the equal sign, num (3≤p≤n) represents the number of all odd prime numbers p in the interval [3,n]; As an equation, the right side of the equal sign must also be the number of all the odd primes p in the interval [3,n]. pi,ps, is all the odd prime numbers p in the interval [3,n]. This is the most basic mathematical meaning of (4.0.1).
(4.0.1) indicates that all the odd prime numbers p in the interval [3,n] are divided into pi and ps,2 parts.
pi is combined with the odd prime number pj in the interval [n,2n-3] to form 2n=pi+pj;
ps is combined with the odd composite number hr in the interval [n,2n-3] to form 2n=ps+hr.
There must be no other possibility than 2n=pi+pj and 2n=ps+hr.
pi,ps, are all part of the odd prime number p in the interval [3,n]. One is written as pi and the other as ps to distinguish between two different combinations: 2n=pi+pj, 2n=ps+hr. If we write them all as p, it will be difficult when we need to specify pi, or when we need to specify ps.
(4.0.1) True, contains two meanings:
First, the numbers on both sides of the equal sign are equal;
Second, things on both sides of the equal sign have the same name, and are the odd prime p in the interval [3,n].
If one of the two is missing, it cannot be an equation. This mathematical meaning, and primary school arithmetic, only the number with the same name can be added or subtracted , only the number with the same name can be equal, completely consistent.
The above properties of (4.0.1) play an important role in the process of proving the conjecture.
Please use Example 3 to test (verify) the mathematical meaning of (4.0.2), (4.0.3), (4.0.4) for yourself.
The mathematical meanings of (4.0.1),(4.0.2),(4.0.3), and (4.0.4) are tested and repeated here because their mathematical meanings play a very important role in the proof of conjectures. I want the reader to remember their mathematical implications.
You may ask: num (3≤p≤51)=14, are both sides equal?
Answer: Equal. num(3≤p≤51), 14, followed by the name p, is already implied after num(3≤p≤51) and 14.
Above, we give inferences of Hou Shaosheng's theorem at 2n. Now we give the inference of Hou Shaosheng's theorem at 2k, k= n+1.
4.3 The inference of Hou Shao-sheng's theorem in 2k time.
Definition: 2k=q+(2k-q), we call it 2k time Hou Shao Sheng identity.
Where: 3≤q≤k; q is odd.
The inference of Hou Shaosheng's theorem at 2k is as follows.
Theorem 5: 2k The inference of Hou Shaosheng's theorem is as follows:
num (3≤p≤k)= num(2k=pi++pj+)+ num(2k=ps++hr+). (4.3.1)
num (3≤h≤k)= num(2k=ht++hu+)+ num(2k=hk++pd+). (4.3.2)
num (k≤p≤2k-3)= num(2k=pi++pj+)+ num(2k=hk++pd+). (4.3.3)
num (k≤h≤2k-3)= num(2k=ht++hu+)+ num(2k=ps++hr+). (4.3.4)
Among them, the agreement:
pi+, pj+, ps+, pd+, are all odd prime numbers; ht+, hu+, hr+, hk+, are all odd composite numbers.
And pi+≤pj+, ht+≤hu+, ps+≤hr+, hk+≤pd+.
pi+, ps+, ht+, hk+, ∈[3,k]; pj+, hr+, hu+, pd+, ∈[n,2k-3].
Proof By replacing n with k in the Hou Shaosheng identity, we can
get the Hou Shaosheng identity at 2k.
Replace n in Hou Shao-sheng's theorem (theorem 4) with k, and at the same time
Replace 2n in 2n=pi+pj with 2k, pi, and pj with pi+, pj+;
Replace 2n in 2n=ps+hr with 2k,ps, hr with ps+, hr+;
Replace 2n in 2n=ht+hu with 2k, ht, hu with ht+,hu+;
Replace 2n in 2n=hk+pd with 2k, hk, and pd with hk+, pd+.
The formula (4.3.1), (4.3.2), (4.3.3), (4.3.4) can be obtained.
Please note that: (4.3.1), (4.3.2), (4.3.3), (4.3.4), are directly derived from Hou Shaosheng's theorem at 2k, so the expression of these 4 equations is always the same, and it has no relationship with whether num (2n=pi+pj) =0; It does not matter whether num(2k=pi++pj+) =0.
It has nothing to do with whether the conjecture is true. In other words, even if num (2n=pi+pj) =0, num (2k=pi++pj+) =0, (4.3.1), (4.3.2), (4.3.3), (4.3.4), this is still true.
Special emphasis is placed on:
2k=ht++hu+, where ht+ and hu+ are odd composite numbers.
2k=ps++hr+, where ps+ is an odd prime and hr+ is an odd composite number.
num (k≤h≤2k-3) equals the number of hu+ + the number of hr+.
We call (4.3.4) the standard representation of num (k≤h≤2k-3). In this formula, ht+ must be an odd composite number in the interval [3,k]; ps+ must be an odd prime in the interval [3,k]. hu+, hr+ must be odd composite numbers in the interval [k,2k-3].
End of proof
5.4 num(k≤ h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+).(4.3.4)
Another independent proof of certainty
Proof We want to prove that (4.3.4) must be true when hu+, hr+, are both odd composite numbers in the interval [k,2k-3].
To prove that (4.3.4) holds, it is necessary to prove that the quantities on both sides of the equality sign are equal and that the names of things on both sides of the equality sign are the same.
On the left side of the equal sign, num (k≤h≤2k-3) represents the number of all odd composite numbers in the interval [k,2k-3].
Let hx be any odd composite number in the interval [k,2k-3]. w is an odd number in the interval [3,k].
Set again:
2k-hx=w (4.4.1)
2k is a definite constant. For any hx, there is always w to make (4.4.1) true.
Order hx to take each odd composite number in the interval [k,2k-3] in order from smallest to largest.
2k-hx , either an odd composite number, or an odd prime number, there are only two possibilities.
If 2k-hx is odd composite number, then 2k-hx=ht+ , where ht+ is odd composite number. And write hx as hu+ , that is, hx=hu+, hu+ odd composite number.
So we get 2k=ht++hu+.
The number of (2k=ht++hu+) is denoted by num(2k=ht++hu+).
If 2k-hx is an odd prime, then 2k-hx=ps+, and ps+ is an odd prime. And write hx as hr+, that is, hx=hr+, hr+ odd composite number.
So we get 2k=ps++hr+.
The number of (2k=ps++hr+) is denoted by num (2k=ps++hr+).
Since hx is any odd composite number in the interval [k,2k-3], now hx is written either hu+ or hr+, so hu+, hr+ is all odd composite numbers in the interval [k,2k-3].
Because hu+, hr+ , are all odd composite numbers in the interval [k,2k-3].
So there must be:
num (k≤h≤2k-3)= num(2k=ht++hu+)+ num(2k=ps++hr+). (4.3.4)
Now num (k≤h≤2k-3) is the total number of odd composite numbers in the interval [k, 2k-3].
num(2k=ht++hu+)+ num(2k=ps++hr+)is also the number of all odd composite numbers in the interval [k,2k-3].
On both sides of the equal sign, the quantity is the same, the name of the thing is the same. So (4.3.4) must be true.
(4.3.4) Must be established, end of proof.
(4.0.1), (4.0.2), (4.0.3), (4.0.4); (4.3.1), (4.3.2), and (4.3.3) can be proved by referring to the proof method of (4.3.4).
brief summary: The title of this section is: 4 Inferences of Hou Shaosheng's Theorem.
Therefore, proving the 4 inferences of Hou Shaosheng's theorem is the main task of this section. We have proved 4 mathematical formulas in the inference of the theorem. Then the mathematical meanings of the 4 mathematical formulas are explained. Finally, 4 mathematical formulas in the theorem are tested by mathematical examples.
The test shows that the mathematical example is completely consistent with the mathematical formula of Hou Shaosheng's theorem and the 4 mathematical formulas in the inference.
The 4 mathematical formulas in inference are the main mathematical tools we use to prove conjectures. The mathematical meanings of the 4 mathematical formulas presented in this section will play an important role in proving the conjecture. The 4 formulas have made the necessary theoretical preparation for the proof of the conjecture.
Finally, we prove 4 inferences of Hou Shaosheng's theorem in 2k time. And give an independent proof of (4.3.4); We also pointed out: (4.0.1),(4.0.2),(4.0.3),(4.0.4); (4.3.1), (4.3.2), (4.3.3) can be proved by referring to the method of proving (4.3.4).
The theorems of sufficient and necessary conditions of conjectures make clear the mathematical thought of proving conjectures: a proof that satisfies the theorems of sufficient and necessary conditions must be a correct proof, and a proof that does not satisfy the theorems of sufficient and necessary conditions must be a wrong proof.
The formula for calculating the insufficient approximation of the number of odd primes in the interval [n,2n] proves that there must be odd primes in the interval [n,2n]. One of the necessary conditions for the hypothesis to be valid is satisfied.
Hou Shao-sheng's identity 2n=q+(2n-q) contains all the changes of Goldbach's conjecture 2n=pi+pj. We have proved Hou Shaosheng's theorem, which has laid a reliable theoretical foundation for proving the conjecture.
This section proves 4 inferences of Hou Shao-sheng's theorem. Concrete theories are prepared for the proof of the conjecture. Now we should prove our conjecture.
For references, see the end.
Section 5,The 4 genetic codes of the conjecture,
the proof of Goldbach's conjecture
Hou Shao-Sheng1, Ma Lin-Jun2
(1 Anyang Mathematical Society, Henan Province, China; 2 Department of Mathematics, Sun Yat-sen University, Guangzhou City, Guangdong Province, China)
Hou Shao-Sheng Email: ayhss@163.com.
Abstract The mathematical formula of Hou Shaosheng's theorem is as follows:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
The 4 inferences of Hou Shaosheng's theorem are as follows:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n,q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
4 inferential formulas provide a reliable theoretical basis for proving Goldbach's conjecture.
The basic task of this section is to uncover the 4 genetic codes that make Goldbach's conjecture valid, based on 4 inferential formulas. Moreover, as long as one genetic code exists, Goldbach's conjecture will continue to hold true. The paper proved that there were at least three of the four genetic codes, which proved Goldbach's conjecture once and for all.
Keyword Goldbach conjecture; Continue to be established;
genetic code.
[Document ID] A [Document number]
5.0. Theorem 6. If n Is an Odd Prime, then 2n=n+n, the Conjecture is True
Proof Since 2n=n+n, if n is an odd prime, then n+n is already the sum of two odd prime numbers, so the conjecture works.
As for Goldbach's conjecture 2n=pi+pj, some sources say that the computer has verified that 6≤2n≤1010, and Goldbach's conjecture is valid. As we have already said, if n is an odd prime, then the conjecture naturally holds. Therefore, in the following study of the conjecture, n is generally assumed to be composite number and relatively large, for example, 1000≤2n.
5.1 Let num(3≤p≤2k-3)=num(3≤p≤2n-3)+1, k=n+1,
Then at 2k,the conjecture must be true.
(After that, if not specified, always set k=n+1)
Theorem 7.If num(3≤p≤2k-3)=num(3≤p≤2n-3)+1, where k=n+1, then 2k must can be expressed as the sum of two odd prime numbers, that is, if 2k=2 (n+1), Goldbach's conjecture must be true.
Proof because
num(3≤p≤2k-3)=num(3≤p≤2n-3)+1.
And k=n+1, so we have:
num(3≤p≤2k-3)
=num(3≤p≤2n-1)
=num(3≤p≤2n-3)+1 (5.1.1)
(5.1.1) indicates that the number of odd prime p in the interval
[3,2n-1] is 1 more than that in the interval [3,2n-3].
There are only two more integers in the interval [3,2n-1] than in the interval [3,2n-3] : 2n-2,2n-1. Obviously, 2n-2 is even and 2n-1 is odd number. So, one extra odd prime can only be 2n-1.
Because k=n+1, 2k-3=2n-1. So in the figure below, 2k-3 and 2n-1 are the same point.
as shown in the figure: In the interval [3,2n-1], there are only two more integers than in the interval [3,2n-3] : 2n-2,2n-1.

So there must be:
2k=2(n+1)=3+(2n-1). (5.1.2)
Since 3 and (2n-1) are both odd prime numbers, so for 2k=2(n+1), the conjecture is true.
Theorem 7 has been proved.
5.2. The Application Value of Theorem 7
The above proof reveals an important secret: let k=n+1, if (2n-1) is an odd prime number, regardless of whether n is odd or even, then 2k must can be expressed as the sum of two odd prime numbers, that is, 2k conjecture must be true.
If (2n-1) is an odd prime, then 2k=2 (n+1) must can be expressed as the sum of two odd prime numbers, that is, 2k must be true.
This theorem provides a theoretical basis for supposing (2n-1) to be an odd composite number when proving conjectures by classification.
If (2n-1) is an odd prime, then 2k=2 (n+1) must can be expressed as the sum of two odd prime numbers, So the conjecture must be true at 2k.
This situation, in practice, is not uncommon.
See 1:
When n=3, 2n-1=2×3-1= 5, 5 is an odd prime number.
So we have 2k=2 (n+1)
=2×4= (2×3-1)+3.
See 2:
When n=4, 2n-1=2×4-1= 7, 7 is an odd prime.
So we have 2k=2 (n+1)
=2×5= (2×4-1)+3.
See 3:
when n=6, 2n-1=2×6-1=11,11 is an odd prime.
So we have 2k=2(n+1)
=2×7=(2×6-1)+3.
See 4:
When n=7, 2n-1=2×7-1= 13,13 is an odd prime number.
So we have 2k=2(n+1)
=2×8=(2×7-1)+3.
Above, we give 4 examples. These 4 examples show that the situation expressed by theorem 7 exists in practice.
However, not every n applies to theorem 7. For example, n=5, 2n-1=9. 9 is not an odd prime and does not satisfy that (2n-1) is an odd prime, so it does not apply to theorem 7.
If (2n-1) is not an odd prime number, for such n, to prove that 2 (n+1), the conjecture must hold, we need to apply a series of subsequent theories, and gradually solve.
5.3 In proving the conjecture, we can assume that the conjecture holds for 2n and that k=(n+1) is composite number.
If not specified, always set k=n+1 thereafter.
If n is an odd prime, 2n=n+n, then obviously the conjecture works.
And since (2n-1) is an odd prime,2k=3+(2n-1),2k is the sum of two odd prime numbers.
That is, as long as (2n-1) is an odd prime, for such n we already have: 2(n+1)=2k=3+ (2n-1),2(n+1) is already the sum of two odd prime numbers.
So, if we assume that the conjecture is true for 2n and to prove that the conjecture must be true for 2k, we can assume that (2n-1) is odd composite. As for (2n-1) being an odd composite number, whether this condition must be used depends on the specific situation. Put this condition here in case you need it.
And we know that for 6≤2n≤106, our conjecture is true. So we can assume that 2n is true.
Let 3≤n, k=n+1, and if k is an odd prime, then the conjecture works at 2k. So we'll work on the conjecture Always set k=n+1, and k is composite number.
Therefore, in proving the conjecture later, we can assume that the conjecture holds for 2n, and that k(=n+1) is composite number. As long as you can prove that the 2k conjecture is true under these conditions, then the conjecture must be true.
k(=n+1) is a composite number, which can be divided into k(=n+1) is an odd composite number and an even composite number.
(2n-1) is composite number, and only odd composite numbers are possible.
Please read this section carefully, and do not ignore it because it has no proof of theorems and no derivation of formulas. Actually, this section is very important. Because this section is the link between the previous and the next, is the most important logical process in the proof of conjecture.
5.4 Minimum and maximum values of num(2k=pi++pj+).
Theorem 8. Suppose that the conjecture is true for 2n, that is:
num(3≤p≤n)=num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num(n≤p≤2n-3)=num(2n=pi+pj)+ num(2n=hk+pd); (4.0.3)
num(2n=pi+pj)≥1.
A: If one of (pi+2), (pj+2), (hr+2), (hk+2) is an odd prime, then the conjecture holds for 2k, k=n+1.
B: And (pi+2), (pj+2), (hr+2), (hk+2) are the sum of the number of odd primes, which is the maximum value of num(2k=pi++pj+). To wit:
num(2k=pi++pj+)≤num((pi+2)∈P)+num((pj+2)∈P)
+num((hr+2)∈P)+num((hk+2)∈P)
(pi+2)∈P means that pi+2 is an odd prime, num((pi+2)∈P) means that pi+2 is the number of odd prime numbers, and the same applies to the rest. (P is the set of odd prime numbers).
(pi, pj, ps, pd, pi+, pj+, are all odd prime numbers).
C: And num((pj+2)∈P)+num((hr+2)∈P), is the minimum value of
num(2k=pi++pj+). To wit:
num((pj+2)∈P)+num((hr+2)∈P) ≤ num(2k=pi++pj+).
Proof Firstly, part of the inference of Hou Shao-sheng's theorem (Theorem 4) is quoted as follows:
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+ num(2n=ht+hu)
+ num(2n=ps+hr)+ num(2n=hk+pd). (3.3.0)
always have:
num (3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr); (4.0.1)
num (n≤p≤2n-3)= num(2n=pi+pj)+ num(2n=hk+pd). (4.0.3)
(1) The following proves theorem content A, as follows:
because
2k=2n+2
=(pi+2)+pj =pi+(pj+2) . (5.4.1)
So, as long as one of (pi+2) is an odd prime, or one of (pj+2) is an odd prime, 2k is already the sum of two odd prime numbers, so at 2k, the conjecture is true.
also because
2k=2n+2
=ps+hr+2=ps+(hr+2). (5.4.2)
That is to say, as long as one of (hr+2) is an odd prime, 2k is already the sum of two odd prime numbers, so at 2k, the conjecture is true.
(hr+2) is an odd prime number, which exists in all 8 examples. And the larger num (2n=ps+hr), the more odd prime numbers (hr+2) are.
also because
2k=2n+2
=hk+pd+2=(hk+2)+pd. (5.4.3)
That is to say, as long as one of (hk+2) is an odd prime, 2k is already the sum of two odd prime numbers, So at 2k, the conjecture is true.
At lest one of (hk+2) is an odd prime number, which exists in all 8 examples. And the larger num(2n=hk+pd)is, the more odd primes (hk+2) are.
Note that if num (2n=pi+pj) =m, and m is a positive integer, then there are m number of 2n=pi+pj, then there are m number of pi, then there are m of pj, and so on.
From (4.0.1), (4.0.3) we can see that if there is a pi+2=pi+1 that is an odd prime, or a pj+2=pj+1 that is an odd prime, or a hr+2=p that is an odd prime, or a hk+2=p that is an odd prime, then the conjecture must be true at 2k.
We have noticed that when (pi+2), (pj+2), (hr+2), (hk+2) are odd prime numbers,then
2k=(pi+2)+pj, 2k=pi+(pj+2), 2k=ps+(hr+2), 2k=(hk+2)+pd,
Every 2k is the sum of two odd prime numbers. As long as one of them exists, it proves that the 2k time conjecture is true.
Theorem content A proves the end.
(2) Theorem content B is proved as follows:
From the above, if (pi+2), (pj+2), (hr+2), (hk+2) are odd prime numbers, then 2k=(pi+2)+pj, 2k=pi+(pj+2), 2k=ps+(hr+2), 2k=(hk+2)+pd,
Each 2k is the sum of two odd prime numbers. As long as one of them exists,
it proves that the 2k time conjecture is true.
And (pi+2), (pj+2), (hr+2), (hk+2) are the sum of the number of odd primes, which is the maximum value of num(2k=pi++pj+). To wit:
num(2k=pi++pj+)≤num((pi+2)∈P)+num((pj+2)∈P)
+num((hr+2)∈P)+num((hk+2)∈P)
This provedtheoremcontentB.
(3) The following proof theorem content C, as follows:
Let num((pi+2) ∈P) indicate that pi+2 is the number of odd prime numbers.
Let num((pj+2)∈P)=m1, (m1 is a non-negative integer), that is, there are m1 odd primes of pj+2.
If 1 number of pj+2 is an odd prime, then 1 number of pi+(pj+2) is the sum of 2 odd prime numbers, and there is 1 of 2k=pi++pj+.
Let num((pj+2) ∈P)=m1, that is, there are m1 number of pj+2 which is odd prime numbers,then there are m1 number of pi+(pj+2) is the sum of two odd prime numbers, there are m1 number of 2k=pi++pj+.
Replacing m1 with num((pj+2)∈P) in the above sentence gives the following statement:
If num((pj+2)∈P). there are num((pj+2)∈P) of pj+2 which is odd prime, there are num((pj+2)∈P) of pi+(pj+2) which is the sum of two odd primes, there are num((pj+2)∈P) of 2k=pi++pj+ . The same goes for others.
Let num((hr+2)∈P)=m2, (m2 is a non-negative integer),that is, there are m2 number of hr+2 which is odd prime numbers,then there are m2 number of hr+2 that is odd prime numbers.
If one number of hr+2 is an odd prime, then there is one number of ps+(hr+2) is the sum of two odd prime numbers, and there is one 2k=pi++pj+ .
If m2 number of hr+2 is odd primes,then there are m2 number of ps+(hr+2) is the sum of two odd primes, and there are m2 number of 2k=pi++pj+ .
If num((hr+2)∈P), there are num((hr+2)∈P) of hr+2 which is odd prime, there are num((hr+2)∈P) of ps+(hr+2) which is the sum of two odd primes, there are num((hr+2)∈P) of 2k=pi++pj+ . The same goes for others.
We notice that since there is no repetition between pi,ps , there is no repetition between pi+(pj+2),ps+(hr+2).
So num((pj+2)∈P)+num((hr+2)∈P)is the minimum value of
num(2k=pi++pj+).
so has:
num((pj+2)∈P)+num((hr+2)∈P) ≤ num(2k=pi++pj+).
This proved theorem content C.
Theorem 8 has been proved.
5.5 Theorem 9. If num (2n=ps+hr) =0, Goldbach's conjecture must be true.
Proof Because the 2n conjecture is true, there must be:
num(3≤p≤n)= num(2n=pi+pj)+ num(2n=ps+hr). (4.0.1)
Among num(2n=pi+pj)≥1.
However, it is possible that num(2n=ps+hr)=0.
If num(2n=ps+hr)=0,
there must be: num(3≤p≤n)=num(2n=pi+pj).
This shows that pi in (2n=pi+pj) is all the odd prime numbers p in the interval [3,n].
Let the smallest odd prime in pi be p1, then p1=3, that is, p1 is the first odd prime 3 in the interval [3,n]. So at least one of pi+2 is an odd prime.
Because at least one of pi+2 is an odd prime, and because:
num(2n=pi+pj)= num(2n+2=pi+2+pj)
= num(2k=(pi+2)+pj).
We know that there are sister primes in the interval [3,n].
Let the ith odd prime be pi, and pi+2=pi+1 is the odd prime, and pi+1 is the (i+1)th odd prime.
Here, pi is the ith odd prime number, and pi+1 is the (i+1)th odd prime number. pi, pi+1 is a sister prime number.
Then:
2k=(pi+2)+pj
=pi+1+pj.
So 2k time is a good guess.
From the above proof, we can see that when num(2n=ps+hr)=0, Goldbach's conjecture must be true.
Therefore, when proving the conjecture below, it can be assumed that num (2n=ps+hr) ≥1, And just consider that k=(n+1) is composite. Since k is an odd prime, the conjecture is obviously true at 2k.
Theorem 9, complete proof.
5.6 Theorem 10. If pi+2is an odd prime, or hr+2is an odd prime, Goldbach's conjecture must be true.
Proof Since k=n+1 is composite number, there must be:
num(3≤p≤k)=num(3≤p≤n) (5.6.1)
Quote (4.0.1) as follows:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr). (4.0.1)
From (5.6.1) and (4.0.1), there must be:
num(3≤p≤k)= num(3≤p≤n)
=num(2n=pi+pj)+num(2n=ps+hr)
=num(2n+2=pi+2+pj)+num(2n+2=ps+hr+2) (5.6.2)
=num(2k=pi+2+pj)+num(2k=ps+hr+2), k=n+1. (5.6.3)
From (5.6.3) :
2k=pi+2+pj
=(pi+2)+pj
=pi+(2+pj)
If one of(pi+2)is an odd prime, then(pi+2)+pj is already the sum of 2 odd prime numbers. So 2k=(pi+2)+pj, 2k is already the sum of 2 odd prime numbers. So the 2k time conjecture works.
If one of(2+pj)is an odd prime, then pi+(2+pj)is already the sum of 2 odd prime numbers. So 2k=pi+(2+pj), 2k is already the sum of 2 odd prime numbers. So the 2k time conjecture works.
From (5.6.3) :
2k=ps+hr+2
=ps+(hr+2)
If one of(hr+2)is an odd prime, then ps +(hr+2)is already the sum of 2 odd prime numbers. So 2k=ps+(hr+2), 2k is already the sum of 2 odd prime numbers. So the 2k time conjecture works.
This proves that if there is a pi+2 that is an odd prime, or a 2+pj that is an odd prime, or a hr+2 that is an odd prime, then the conjecture must be true at 2k. That Goldbach's conjecture must be true.
Theorem 10 is proved.
Theorem 10 proves that if pi+2 is an odd prime, or hr+2 is an odd prime, Goldbach's conjecture must be true. However, this theorem is not answered: there must be pi+2 which is an odd prime numbers, or there must be hr+2 which is an odd prime numbers, to ensure that Goldbach's conjecture is true.
The task that pi+2 must be an odd prime, or that hr+2 must be an odd prime, requires theorem 17.
If someone checks every even number up to 3× one by one, Goldbach's conjecture is true. This fact tells us that Goldbach's conjecture is true for every even number in the interval [6, 3×].
Let's say 2n,2(n+1), no less than 6, no greater than 3×, why is the conjecture true for 2n, and true for 2(n+1)? It works for 2n, it works for 2(n+1), so what's the secret? The result of our research is the following 4 major theoretical questions raised by the authors.
5.7 Four major theoretical questions raised by the author; The four genetic codes of Goldbach's conjecture.
Goldbach's conjecture at 2n is expressed as 2n=pi+pj; Goldbach's conjecture for 2 (n+1), we use 2(n+1)=pi++pj+, pi+, pj+, are odd prime numbers.
In the study of 4 major theoretical problems, the term 2(n+1)=pi++pj+ is involved. To do this, we need to give it a definition.
Definition: Let qi and qj both be odd prime numbers, and 2(n+1)=qi+qj, then we say (define) 2(n+1)=qi+qj, which is the source of 2(n+1)=pi++pj+.
We're working on Goldbach's conjecture. We have to ask: what is the nature of Goldbach's conjecture? Some people would say that the essence is: as long as 3≤n, then 2n=pi+pj can be true. We say this answer is correct, but it misses the point.
So, what's the point? The key is: why is the conjecture true for 2n, and true for 2(n+1)? Or, if 2n=pi+pj is true, 2k=pi++pj+ where does come from? 2k=pi++pj+ How is composed?
This part will reveal the most mysterious and essential recurrence relation of Goldbach's conjecture. The recursion relation here means that if the conjecture is true at 2n, it must lead to the conjecture being true at 2 (n+1).
5.7.1 Question 1: Assuming 2n is true, where does 2k=pi++pj+ come from? Or, why do assumptions persist?
We have theorem 11 to answer as follows:
Theorem 11: If the 2n conjecture is true, then2k=pi++pj+ has the following 4 sources, as long as 1 source exists, the 2k conjecture is guaranteed to continue to be true.
The 4 sources of2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+.
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+.
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+.
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
Each of these 4 sources is a theorem. Because they have the same form, they're put together as a theorem.
4 sources of expression, very simple, are what if, then what. If what is the condition of the theorem, then what is the conclusion of the theorem. From the condition of the theorem, to the conclusion of the theorem, there is no other intermediate link, causality, very direct, so it is no longer proved.
At this point, the only question the reader should ask is, is it possible that none of the 4 ifs exist? This is the most central issue, and this is the issue that we are most concerned about. As a proof of the conjecture, this question must be answered.
As an answer to the core question, theorem 17 will prove:
If the Goldbach conjecture holds for 2n and k (=n+1) is composite number, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture holds for 2k.
Theorem 11, theoretically gives 4 sources of 2k=pi++pj+.
Note that 2k=ht+2+hu, either 2k=(ht+2)+hu, or 2k=ht+(2+hu), cannot be the sum of 2 odd prime numbers. So it can't be one of 2k=pi++pj+, so 2k=pi++pj+, there are only 4 sources above.
The 4 sources of 2k=pi++pj+ essentially answer the close relationship between the 2n conjecture and the 2k conjecture.
As long as one of these 4 sources exists, 2k time conjecture must be true!
These 4 sources are the decisive factors and fundamental guarantees for the continuation of the conjecture.
These 4 sources are the 4 genetic codes of Goldbach's conjecture.
These 4 sources are the fundamental mystery of the persistence of Goldbach's conjecture.
Note that the first 2k=(pi+2)+pj of the 4 sources. when (pi+2) is an odd prime, because pi, (pi+2), are both odd primes, so pi, (pi+2), is a sister prime, and pi is the smaller of the sister primes.
The second 2k=pi+(pj+2) of the 4 sources , when (pj+2) is an odd prime, since pj, (pj+2), are both odd primes, so pj, (pj+2), is a sister prime, and pj is the smaller of the sister primes.
The third 2k=ps+(hr+2) of the 4 sources, originally hr is an odd composite number, when (hr+2) is an odd prime, ps+(hr+2) becomes the sum of 2 odd primes. The significance of this event is that it connects 2n=ps+hr with 2k=ps+(hr+2), completing the transition from the non-Goldbach conjecture (2n=ps+hr) to Goldbach's conjecture(2k=pi++pj+)!
The fourth 2k=(hk+2)+pd of the 4 sources, originally hk is an odd composite number, and when (hk+2) is an odd prime number, (hk+2)+pd becomes the sum of 2 odd primes. The significance of this event is that it connects 2n=hk+pd with 2k=(hk+2)+pd, completing the transition from the non-Goldbach conjecture (2n=hk+pd) to Goldbach's conjecture(2k=pi++pj+)!
So, two of the 4 sources of 2k=pi++pj+ are realized with the help of the smaller of the sister primes; Two are realized by means of the non-Goldbach conjecture.
Question 1.1: As n increases, the number of (2n=pi+pj) increases in a wave.
2k=2(n+1)=pi++pj+ has 4 sources, which is the fundamental reason why num(2n=pi+pj) gradually waves up as n increases.
In some materials, especially at
https://en.wikipedia.org/wiki/Goldbach’s_conjecture the odd primes slash the intersection of graphics, further enriches our perceptual knowledge. Although these data know that num (2n=pi+pj) increases with the increase of n, they do not indicate the reason for the gradual increase.
Numerous researchers of Goldbach's conjecture, through a limited number of mathematical examples, have found that as n increases, the number of (2n=pi+pj) gradually increases in a wave pattern. This discovery is a contribution and deserves recognition. However, no one knows that 2k=2(n+1)=pi++pj+ has 4 sources, so no one has mathematically explained the gradual increase in the number of (2n=pi+pj).
To explain the gradual increase in num(2n=pi+pj), we use the following 2 mathematical formulas.
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
Let's say k=n+1, and let's compare num(3≤p≤n), num(3≤p≤k).
If k is composite number, whether k is odd composite number or even composite number, there is:
num(3≤p≤n)=num(3≤p≤k).
That is to say, if k is composite number, num(3≤p≤n) does not change as n increases by l from n to k. That is, num(3≤p≤n) does not change.
When n changes to k,num(3≤p≤n)=num(3≤p≤k), it is known from (4.0.1) that the total number of num(2n=pi+pj) +num (2n=ps+hr) does not change. However,num(2n=pi+pj), num(2n=ps+hr), can be changed. This change will cause a wave change in num(2n=pi+pj), num(2n=ps+hr).
If k is an odd prime, there is always:
num(3≤p≤n)+1=num(3≤p≤k).
That is to say, if k is an odd prime, num(3≤p≤n), because n increases by 1, and as n changes from n to k, num(3≤p≤k) increases by 1 compared to num(3≤p≤n), the change occurs.
When n changes to k,num(3≤p≤k) increases by 1 compared with
num(3≤p≤n), and it can be seen from (4.0.1) that the total number of num(2n=pi+pj)+ num(2n=ps+hr)increases by 1. num (2n=pi+pj), num (2n=ps+hr), must change. It is likely to increase num (2n=pi+pj).
⑴ If the conjecture is true for 2n, it is accepted that
1≤num (2n=pi+pj). There is no doubt that the greater num (2n=pi+pj), the greater the number of (2n=pi+pj). The more 2n=pi+pj, the more pi+2, the more pj+2. Not every pi+2, not every pj+2, is an odd prime, but the more chances pi+2, pj+2, is an odd prime, the more pi+2, pj+2, is an odd prime.
If pi+2, pj+2, are odd prime numbers, each 2k=(pi+2)+pj, each 2k=pi+ (2+pj), becomes one of(2k=pi++pj+). Causes an increase in the number of (2k=pi++pj+).
⑵ Assuming that the conjecture is true for 2n, in general, 1≤
num(2n=ps+hr). The greater num (2n=ps+hr), the greater the number of (2n=ps+hr). The more we have (2n=ps+hr), the more we have (hr+2). Although not every (hr+2) is an odd prime, the more chances (hr+2) is an odd prime, the more (hr+2) is an odd prime.
If (hr+2) is an odd prime, every 2k=ps+(hr+2) becomes one of(2k=pi++pj+). Causes an increase in the number of (2k=pi++pj+).
2n=ps+hr, not one of (2n=pi+pj). If 2k=ps+(hr+2) becomes one of (2k=pi++pj+), this is a process from 2n=ps+hr to(2k=pi++pj+). This is an important factor that the num(2k=pi++pj+)of increase .
⑶ Assuming that the conjecture is true for 2n, in general, 1≤num (2n=hk+pd). There is no doubt that the greater num (2n=hk+pd), the greater the number of (2n=hk+pd). The more (2n=hk+pd) there is, the more (hk+2) there is. Although not every (hk+2) is an odd prime, the more chances (hk+2) is an odd prime, the more (hk+2) is an odd prime.
If (hk+2) is an odd prime, every 2k=(hk+2)+pd becomes one of(2k=pi++pj+). Causes an increase in the number of (2k=pi++pj+).
2n=hk+pd, not one of (2n=pi+pj). If 2k=(hk+2)+pd becomes one of (2k=pi++pj+), this is a process from 2n=hk+pd to(2k=pi++pj+). This is an important factor that the num(2k=pi++pj+)of increase .
Theorem 11 theoretically proves that 2k=2(n+1)=pi++pj+ has 4 sources. Now, let's test theorem 11 with a mathematical example.
Verification question 1: 2k=2(n+1)=pi++pj+, there are four sources.
The following example, 3, is the third of the 8 mathematical examples in 3.2: Instance 3. Now verify the 4 sources of 2k=pi++pj+ with example 3.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=9+93=11+91=13+89=15+87=17+85=
=19+83=21+81=23+79=25+77=27+75=29+73=31+71=33+69=35+67
=37+65=39+63=41+61=43+59=45+57=47+55=49+53=51+51.
Note: Numbers underlined are prime numbers. If the number is not underlined, it is a composite number.
Theorem 11 proves that 2k=2(n+1)=pi++pj+ has 4 sources. These 4 sources are fully confirmed in Example 3. See below for specific verification.
Verify the first source of 2k=2(n+1)=pi++pj+as follows:
num(2×51=pi+pj)=8.
These 8 number of 2×51=pi+pj are as follows:
2×51 =5+97 =13+89 =19+83 =23+79 =29+73 =31+71 =41+61 =43+59.
2n=pi+pj, (pi +2)are odd prime numbers:
(5+2); (29+2);(41+2).
2k=2(n+1)=(pi+2)+pj. 2k is the sum of 2 odd prime numbers, such as:
2k=(5+2)+97 ;2k=(29+2)+73 ; 2k=(41+2)+61.
Verify the second source of2k=2(n+1)=pi++pj+, as follows:
2n=pi+pj,(pj+2)are odd prime numbers:
(71+2);(59+2).
2k=2(n+1)=pi+(pj+2), 2k is the sum of 2 odd prime numbers, which is:
2k=31+(71+2); 2k=43+(59+2).
Note: (29+2)+73, repeated with 31+(71+2). (41+2)+61, repeat with 43+(59+2). We're shading the duplicators.
Verify the third source of 2k=2(n+1)=pi++pj+, as follows:
num(2n=ps+hr)=6.
The 6 number of 2×51=ps+hr are: 2×51= 3+99= 7+95= 11++91= 17+85= 37+65= 47+55.
2n=ps+hr,(hr+2) are odd prime numbers: (99+2); (95+2); (65+2).
2k=2(n+1)=ps+(hr+2), 2k is the sum of 2 odd prime numbers, which is:
2k=3+(99+2); 2k=7+(95+2); 2k=37+(65+2).
Verify the fourth source of 2k=2(n+1)=pi++pj+, as follows:
num(2n=hk+pd)=2.
These 2 number of 2n=hk+pd are as follows:
2n=35+67; 2n=49+53;
2n=hk+pd, (hk+2)are odd prime numbers: (35+2).
2k=2(n+1)=(hk+2)+pd, 2k is the sum of 2 odd prime numbers, which is:
2k=(35+2)+67.
(The character shading is repeated and can be ignored.)
For example, the above facts are taken as examples:
(5+2)+97, like 7+(95+2), is the sum of the same 2 numbers, and 7+(95+2) is the repeat.
37+(65+2)and (35+2)+67 are the sum of the same 2 numbers, and (35+2)+67 is the repeat.
The above facts show that the 4 sources of 2k=2(n+1)=pi++pj+ all appear in Example 3, and the theory and practice are completely consistent.
Above we get 9 number of 2k=2(n+1)=pi++pj+, of which there are 4 repetitions. So there are no repetitions(2k=pi++pj+),only 5.
After comparison, these 5 are exactly the same as the actual situation in example 2 (2(51+1)).
Us from(2n=2×51=102=1+101=3+99=······=49+53=51+51.), got 2k=
2(51+1)=pi++pj+ is all about.
The reader should appreciate that the 4 sources would rather repeat the same data (with character shading), and never give up the opportunity to ensure that the conjecture continues to hold.
The appearance of the duplicate data explains the 4 sources and provides multiple guarantees for the establishment of Goldbach's conjecture. If only one(2k=pi++pj+)occurs once, the conjecture is guaranteed. If(2k=pi++pj+)occurs m times in total, the conjecture is guaranteed m times. This is one of the mysteries of the persistence of conjecture.
Since(2k=pi++pj+)has only 4 sources,(2k=pi++pj+)can occur at most 4 times for the same (2k=pi++pj+). Because in the same source, there can be no duplication. For example, 2k=2(n+1)=pi++pj+ the fourth source: 2n=hk+pd, when one (hk+2) is an odd prime, 2k= (hk+2) +pd, forming a 2k=pi++pj+, it is impossible to appear 2 identical 2k=(hk+2)+pd.
5.7.2 Question 2: Where does 2k=ps++hr+ come from? Will the 4 sources of 2k=2(n+1)=pi++pj+ use up 2n resources and hinder the source of 2k=ps++hr+?
We have theorem 12 answers as follows:
Theorem 12: If the conjecture is true for 2n, then 2k=ps++hr+has4sources:
If one of(pj+2)is an odd composite number, then 2k=pi+(pj+2) becomes one of 2k=ps++hr+.
If one of (hr+2) is an odd composite number, then 2k=ps+(hr+2) becomes one of 2k=ps++hr+.
If one of (ps+2) is an odd prime, then 2k=(ps+2)+hr becomes one of 2k=ps++hr+ .
If one of (ht+2) is an odd prime, then 2k=(ht+2)+hu becomes one of 2k=ps++hr+.
Each of the 4 sources of 2k=ps++hr+ above is a theorem. Because the causal relationship between the condition and the conclusion is very direct, it can no longer be proved.
2k=ps++hr+ There are only 4 sources mentioned above. Because 2k=hk+2+pd, neither 2k=(hk+2)+pd, nor 2k=hk+(2+pd), can be the sum of 1 odd prime + 1 odd composite number. So it can't be one of 2k=ps++hr+, it can't be a source of it.
2k=2(n+1)=ps++hr+ has 4 sources, which is why num(2n=ps+hr) is gradually increasing in waves.
The reason why num(2n=ps+hr) gradually increases in waves can still be explained by mathematical formulas. In this regard, we see above (Problem 1.1: As n increases, the number of (2n=pi+pj) increases in a wave.) Examples have been given, which are omitted here for the sake of shortening space.
Theorem 12, in theory, proves that 2k=2(n+1)=ps++hr+ has 4 sources. Let's test theorem 12 with a mathematical example.
Verification question 2: 2k=2(n+1)=ps++hr+ 4 sources.
Example 3 below, the third of the 8 mathematical examples in 3.2, now uses example 3 to verify the 4 sources of 2k=ps++hr+.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=······=41+61=43+59=45+57=47+55=49+53=51+51.
The number is underlined. The number is an odd prime. None digits with underscores are odd composite numbers.
Theorem 12 proves that 2k=2(n+1)=ps++hr+ has 4 sources. These 4 sources are fully confirmed in Example 3. See below for specific verification.
Verify the first source of 2k=2(n+1)=ps++hr+, as follows.
num(2×51=pi+pj)=8.
These 8 number of 2×51=pi+pj as follows:
2×51=5+97=13+89=19+83=23+79=29+73=31+71=41+61=43+59.
2n=pi+pj, (pj+2)are odd composite numbers:
5+(97+2)=13+(89+2)=19+(83+2)=23+(79+2)=29+(73+2)=41+(61+2).
Verify the second source of 2k=2(n+1)=ps++hr+, as follows.
num (2n=ps+hr)=6.
These 6 number of 2n=ps+hr are as follows:
2×51=3+99=7+95=11+91=17+85=37+65=47+55.
2n=ps+hr,(hr+2) are odd composite numbers:
11+(91+2)=17+(85+2)=47+(55+2).
Verify the third source of 2k=2(n+1)=ps++hr+, as follows.
num(2n=ps+hr)=6.
These 6 number of 2n=ps+hr are as follows:
2×51=3+99=7+95=11+91=17+85=37+65=47+55.
2n=ps+hr,(ps+2) are odd prime numbers:
(3+2)+99=(11+2)+91=(17+2)+85.
(3+2)+99, repeat with 5+(97+2), (11+2)+91 repeat with 13+(89+2),
(17+2)+85 is repeated with 19+(83+2).
Verify the fourth source of 2k=2 (n+1) =ps++hr+, as follows.
num(2×51=ht+hu)=9.
These 9number of 2n=2×51=ht+huare as follows:
2n=9+93; 2n=15+87; 2n=21+81; 2n=25+77; 2n=27+75;
2n=33+69; 2n=39+63; 2n=45+57; 2n=51+51.
2n=ht+hu, (ht+2) are odd prime numbers:
(9+2)+93=(15+2)+87=(21+2)+81=(27+2)+75=(39+2)+63=(45+2)+57.
(The character shading is repeated and can be ignored.)
The above facts show that the 4 sources of 2k=2(n+1)=ps++hr+ are exactly consistent with mathematical examples.
Us from(2n=2×51=102=1+101=3+99=······=49+53=51+51.), got it
num(2(51+1)=ps++hr+)=9. The data is exactly the same as in example 2
(2(51+1)).
Above, we have completed the verification task of 2k=2 (n+1) =ps++hr+ 4 sources.
In order to facilitate later references if necessary, we make the following summary.
Summary:
Us from(2n=2×51=102=1+101=3+99=······=49+53=51+51.), got it
num(2(51+1)=ps++hr+)=9.
These 9 number of 2(51+1)=2(n+1)=ps++hr+ as follows.
From the first source of 2k=2(n+1)=ps++hr+, as follows:
2n=pi+pj, and pj+2 are odd composite numbers:
5+(97+2)=13+(89+2)=19+(83+2)=23+(79+2)=29+(73+2)=41+(61+2).
From the second sourceof2k=2(n+1)=ps++hr+, as follows:
2n=ps+hr,(hr+2) are odd composite numbers:
11+(91+2)=17+(85+2)=47+(55+2).
From the third source of 2k=2(n+1)=ps++hr+,as follows:
2n=ps+hr, (ps+2) are odd prime numbers:
(3+2)+99=(11+2)+91=(17+2)+85.
From the fourth source of 2k=2(n+1)=ps++hr+, as follows:
2n=ht+hu, (ht+2) are odd prime numbers:
(9+2)+93=(15+2)+87=(21+2)+81=(27+2)+75=(39+2)+63=(45+2)+57.
(The character shading is repeated and can be ignored.)
Us from(2n=2×51=102=1+101=3+99=······=49+53=51+51.), got it
num(2(51+1)=ps++hr+)=9.The data is exactly the same as in example 2
(2(51+1)).
From the above 4 sources, the obtained 2k=2(n+1)=ps++hr+ is summarized as follows:
5+(97+2)=13+(89+2)=19+(83+2)=23+(79+2)=29+(73+2)=41+(61+2).
11+(91+2)=17+(85+2)=47+(55+2).
(3+2)+99=(11+2)+91=(17+2)+85.
(9+2)+93=(15+2)+87=(21+2)+81=(27+2)+75=(39+2)+63=(45+2)+57.
The shadow is the duplicator.
Please pay attention to 1, the following:
5+(97+2)=13+(89+2)=19+(83+2)=23+(79+2)=29+(73+2)=41+(61+2).
2k=pi +(pj+2) is the sum of an odd prime number and an odd composite number.
Please pay attention to 2, the following:
11+(91+2)=17+(85+2)=47+(55+2).
2k=ps+(hr+2) is the sum of an odd prime number and an odd composite number.
It should also be noted that there is no repetition between 2k=pi+(pj+2)and 2k=ps+(hr+2)! And they're all part of 2k=2(n+1)=ps++hr+ . This example shows that in 2k=2(n+1)=ps++hr+ , if you want to reject 2k=pi+(pj+2), or reject 2k=ps+(hr+2), there is no reason.
5.7.3 Question 3: 2k=ht++hu+ Where does come from? We have theorem 13 answers as follows.
Theorem 13: If the conjecture holds for 2n, then2k=ht++hu+ has the following 4 sources:
If one of (ps+2) is odd composite number, then 2k=(ps+2)+hr becomes one of 2k=ht++hu+.
If one of (ht+2) is odd composite number, then 2k=(ht+2)+hu becomes one of 2k=ht++hu+.
If one of (hu+2) is odd composite number, then 2k=ht+(hu+2) becomes one of 2k=ht++hu+.
If one of (pd+2) is odd composite number, then 2k=hk+(pd+2) becomes one of 2k=ht++hu+.
Above, 2k=ht++hu+ 4 sources, each proposition is a theorem. Because the causal relationship between the condition and the conclusion is very direct, it can no longer be proved.
Above, we give 4 sources for 2k=ht++hu+. This is why num(2n=ht+hu), the wave form, increases rapidly as n increases! It is still possible to explain the reason for the rapid increase of num(2n=ht+hu) in a mathematical formula, but for the sake of compression, it is omitted here.
2k=ht++hu+, there are only 4 sources mentioned above. Because 2k=pi+2+pj, neither 2k= (pi+2) +pj, nor 2k=pi+ (2+pj), can be the sum of one odd composite number plus one odd composite number. So it can't be a source of 2k=ht++hu+.
Theorem 13 theoretically proves that 2k=2(n+1)=ht++hu+ has 4 sources. Let's test theorem 13 with a mathematical example.
Verification question 3: 2k=2(n+1)=ht++hu+ 4 sources.
Example 3, which is the third of the 8 mathematical examples in 3.2, is now used to verify 2k=2(n+1)=ht++hu+ 4 sources.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=······=41+61=43+59=45+57=47+55
=49+53=51+51.
Theorem 13 proves that 2k=2(n+1)=ht++hu+ has 4 sources. These 4 sources are fully confirmed in Example 3. See below for specific verification.
Verify the first source of 2k=2(n+1)=ht++hu+, as follows.
num(2×51=ht+hu)=9.
These 9number of2n=2×51=ht+huare as follows:
2n=9+93; 2n=15+87; 2n=21+81; 2n=25+77; 2n=27+75;
2n=33+69; 2n=39+63; 2n=45+57; 2n=51+51.
2n=ht+hu, and (ht+2) are odd composite numbers:
(25+2)+77=(33+2)+69.
Verify the second source of 2k=2(n+1)=ht++hu+, as follows.
2n=ht+hu, (hu+2) are odd composite numbers:
9+(93+2)=27+(75+2)=39+(63+2).
Verify the third source of 2k=2(n+1)=ht++hu+, as follows.
num(2n=ps+hr)=6.
These 6 number of 2n=ps+hr are as follows:
2n=3+99; 2n=7+95; 2n=11+91; 2n=17+85; 2n=37+65;
2n=47+55;
2n=ps+hr, (ps+2) are odd composite numbers:
(7+2)+95=(37+2)+65=(47+2)+55.
Verify the fourth source of 2k=2(n+1)=ht++hu+, as follows.
num(2n=hk+pd)=2.
These two number of 2n=hk+pd are as follows:
2n=35+67; 2n=49+53;
2k=hk+pd, and (pd+2) are odd composite numbers:
35+(67+2)=49+(53+2).
(The character shading is repeated and can be ignored.)
The above facts show that the 4 sources of 2k=2(n+1)=ht++hu+ are exactly consistent with mathematical examples.
Us from (2n=2×51=102=1+101=3+99=······=49+53=51+51.), obtained the num(2(51+1)=ht++hu+)=5. The data is exactly the same as in example 2 (2 (51+1)).
We should note that 2k=2(n+1)=ht++hu+ 4 sources, in mathematical practice, it is preferable to repeat the data, but never give up the data that should be.
5.7.4 Question 4: Where does2k=hk++pd+ come from? We have theorem 14 answers as follows.
Theorem 14: If the conjecture is true for 2n,2k=hk++pd+ has the following 4 sources:
If one of (pi+2) is odd composite number , then 2k=(pi+2)+pj becomes one of 2k=hk++pd+;
If one of (hu+2) is odd prime, then 2k=ht+(hu+2) becomes one of 2k=hk++pd+;
If one of (hk+2) is odd composite number, then 2k=(hk+2)+pd becomes one of 2k=hk++pd+;
If one of (pd+2) is odd prime, then 2k=hk+(2+pd) becomes one of 2k=hk++pd+;
Above, there are 4 sources of 2k=hk++pd+, each of which is a theorem. Because the causal relationship between the condition and the conclusion is very direct, it can no longer be proved.
Above we give 4 sources of 2k=hk++pd+. This is why num (2n=hk+pd) waves gradually increase as n increases! The reason for the gradual increase of num (2n=hk+pd) wave can still be explained by mathematical formula, but for the sake of space, it is omitted here!
2k=hk++pd+, there are only 4 sources mentioned above. Because 2k=ps+2+hr, neither 2k=(ps+2)+hr, nor 2k=ps+ (2+hr), can be the sum of one odd composite number plus one odd prime number. So it can't be a source of 2k=hk++pd+.
Theorem 14 theoretically proves that 2k=2(n+1)=hk++pd+ has 4 sources. Let's verify theorem 14 with a mathematical example.
Verification question 4: 2k=2(n+1)=hk++pd+ 4 sources.
Example 3 below, which is the third of the 8 mathematical examples in 3.2, is now used to verify 2k=2(n+1)=hk++pd+ 4 sources.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=······=41+61=43+59=45+57=47+55
=49+53=51+51.
Theorem 14 theoretically proves that 2k=2(n+1)=hk++pd+ has 4 sources. These 4 sources are fully confirmed in Example 3. See below for specific verification.
Verify the first source of 2k=2(n+1)=hk++pd+, as follows.
num(2×51=pi+pj)=8.
These 8 number of 2×51=pi+pj, as follows:
5+97=13+89=19+83=23+79=29+73=31+71=41+61=43+59.
2n=pi+pj, (pi+2) are odd composite numbers:
(13+2)+89=(19+2)+83=(23+2)+79=(31+2)+71=(43+2)+59.
Verify the second source of 2k=2(n+1)=hk++pd+, as follows.
num(2×51=ht+hu)=9.
These 9 number of 2n=2×51=ht+hu are as follows:
2n=9+93; 2n=15+87; 2n=21+81; 2n=25+77; 2n=27+75;
2n=33+69; 2n=39+63; 2n=45+57; 2n=51+51.
2n=ht+hu, (hu+2) are odd prime numbers:
15+(87+2)=21+(81+2)=25+(77+2)=33+(69+2)=45+(57+2)=51+(51+2).
Verify the third source of 2k=2(n+1)=hk++pd+, as follows.
num(2n=hk+pd)=2.
These two number of 2n=hk+pd are as follows:
2n=35+67; 2n=49+53;
2n=hk+pd, and (hk+2) are odd composite numbers: (49+2)+53.
Verify the fourth source of2k=2(n+1)=hk++pd+, as follows.
num(2n=hk+pd)=2.
These two number of 2n=hk+pd are as follows:
2n=35+67; 2n=49+53;
2n=hk+pd, (pd+2) is an odd prime number, not one in this case. However, this cannot be used to negate the existence of odd prime numbers (pd+2), much less to negate the theorem 14. It is now confirmed by example 2 that there are indeed (pd+2) odd prime numbers.
Example 2. 2×52=104
=1+103=3+101=5+99=7+97=9+95=11+93=13+91=15+89=17+87=
=19+85=21+83=23+81=25+79=27+77=29+75=31+73=33+71=35+69
=37+67=39+65=41+63=43+61=45+59=47+57=49+55=51+53.
In this case, num(2n=hk+pd)=6.
These 6 number of 2n=hk+pd are as follows:
2n=15+89, 2n=21+83, 2n=25+79, 2n=33+71, 2n=45+59, 2n=51+53.
2n=hk+pd, (pd+2) are odd prime numbers: 33+(71+2), 45+(59+2).
The above facts show that the four sources of 2k=2(n+1)=hk++pd+ are exactly consistent with mathematical examples.
Us from(2n=2×51=102=1+101=3+99=······=49+53=51+51.), obtained the num(2(n+1)=2(51+1)=hk++pd+)=6. The data is exactly the same as in example 2 (2(51+1)).
We should note that 2k=2(n+1)=hk++pd+ 4 sources, in mathematical examples, it is preferable to repeat the data, but never give up the data that should be.
Four big theoretical questions, and that concludes.
5.8 Summary of 4 major theoretical problems and 4 Genetic code problems
The 4 major theoretical problems are the core theoretical problems in the proof of Goldbach conjecture, which is a major breakthrough and great harvest in our study of Goldbach conjecture.
Goldbach's conjecture holds, essentially, because it holds for 2n, and it still holds for 2(n+1).
The proof of Goldbach's conjecture must answer: If the conjecture is true for 2n, why is it still true for 2(n+1)? What is the close relationship between the establishment of 2n and the establishment of 2k?
4 major theoretical questions are theoretically answered: the close relationship between 2n holds and 2k(k=n+1) holds.
Theorem 11 answers: If the conjecture is true at 2n, then 2k=pi++pj+ has 4 sources, and as long as one source exists, the conjecture will continue to be true at 2k. This is the most important discovery and central theory in the proof of the conjecture.
Theorem 12 answers: If the conjecture is true for 2n, then 2k=ps++hr+ has 4 sources.
Theorem 13 answers: If the conjecture is true for 2n, then 2k=ht++hu+ has 4 sources.
Theorem 14 answers: If the conjecture is true for 2n, then 2k=hk++pd+ has four sources.
The 4 major theoretical questions not only theoretically answer the close relationship between the establishment of 2n and the establishment of 2k(k=n+1), but also accept the strict test of case 3 and case 2.
Inspection instructions:
2k=pi++pj+ has 4 sources; fully unified with complex mathematical examples.
2k=ps++hr+ has 4 sources; fully unified with complex mathematical examples.
2k=ht++hu+ has 4 sources; fully unified with complex mathematical examples.
2k=hk++pd+ has 4 sources; fully unified with complex mathematical examples.
The test shows that Hou Shaosheng's theorem is correct, the inference of Hou Shaosheng's theorem is correct, and the theory of four sources is correct. Readers are welcome to test with other examples.
Above, we have made all kinds of theoretical preparations for proving Goldbach's conjecture, but only the above theoretical preparations are not enough, we must also study the classification of odd prime numbers, we must also study the relationship between all odd composite numbers and the number of odd prime numbers in the interval [3,n]. It is also necessary to study the relationship between all the odd composite numbers and the number of odd primes in the interval [n, 2n-3].
Professor Liang Yixing, who was the dean of the School of Mathematics at Xiamen University before he retired, and Professor Zhu Huilin, once asked the question. They, along with Ma Linjun, a professor at Sun Yat-sen University, and Zeng Hongbiao, who graduated from Wuhan University with a master's degree in mathematics and is a professor in the United States,
raised similar questions in the first draft of their paper, forcing Hou to dig deeper. After six years and hundreds of revisions, the present proof was finally formed.
I sincerely thank Professors Ma Linjun, Zeng Hongbiao, Liang Yixing and others for their questions and important contributions. My sincere thanks to Dr. Liu Xiaoqian and Li Canzhang, who graduated from the Chinese Academy of Mathematics, for their 6-7 years of repeated review.
Let's first define three new concepts about odd prime numbers.
5.9 Odd prime after odd composite number, odd prime after odd prime number, independent odd prime number.
Definition 1: Let h be an odd composite number, if h+2 is an odd prime p, that is, if h+2=p, this paper calls this kind of odd primes p are odd primes after odd composite numbers,or prime numbers after odd composite numbers.
Definition 2: Let pi be an odd prime, if pi+2 is an odd prime p, that is, if pi+2=p, this paper calls this kind of odd primes p are odd primes after odd primes, or prime numbers after odd primes.
Definition 3: Let p be an odd prime number, and if p-2, p+2, are both odd composite numbers, then the odd prime p is called an independent odd prime number, or independent prime number. The independent prime must be the prime after the odd composite number.
According to the above definition, all odd prime numbers greater than 3 can be divided into twokind:
The first kind, An odd prime after an odd composite number.
For example, 9+2=11,15 +2= 17,21 +2=23, 27+2=29. 11,17, 23, 29, that's the prime after the odd composite number.
The secondkind,anodd prime numbers afteranodd prime numbers.
For example, 3+2=5, 5+2= 7, 11+2= 13, 17+2=19. 5, 7, 13, 19, that's the prime after the odd prime.
In fact, if pi+2=pi+1, usually piand pi+1are called sister primes.
If pi is the ith odd prime number, pi+1 is the (i+1)th odd prime number, And pi+2=pi+1, then pi+1 is an odd prime after an odd prime.
3 is an odd prime number, but it is neither an odd prime after an odd composite number nor an odd prime after an odd prime. 3 is the first odd prime number.
5.10 3,5,7 is the only three sister prime number.
Theorem 15: 3,5,7 is the only 3 sister primes, and there can be no other 3 odd primes that are 3 sister primes.
Proof 3,5,7, is the only 3 sister primes, that is,there can be no three odd primes other than 3,5,7 that are 3 sister primes.
The proof for this conclusion is as follows:
Suppose pi, pi+2, pi+4, are three sister primes, 3 < pi .
Because pi is an odd prime number, so pi =3m+1; Or pi=3m+2.
If pi=3m+1, then pi+2=3m+3=3(m+1), which contradicts that pi+2 is an odd prime.
If pi=3m+2, then pi+4=3m+6=3(m+2), which contradicts that pi+4 is an odd prime.
Theorem 15, complete proof.
5.11 [3, n] The number of odd primes after odd composite numbers in the interval.
The number of odd primes after odd composite numbers in the interval [n, 2n-3].
Theorem 16A: (ht+2) and (hk+2), contain all the odd primes p after odd composite numbers in the interval [3, n]. After 11≤n, in the interval [3, n], there is at least one odd prime p are odd primes after odd composite numbers. After 50≤n, in the interval [3, n], 50% or more of the number of odd primes p are odd primes after odd composite numbers.
Theorem 16B: (hu+2) and (hr+2) contains all the odd primes p after odd composite numbers in the interval [n, 2n-3]. In the interval [n,2n-3], 50% or more of the number of odd primes p are odd primes after odd composite numbers.
Note: ht, hk, hu and hr here are ht, hk, hu and hr in Hou Shaosheng's theorem.
Theorem 16C: Of (ht+2) and (hk+2), at least one is an odd prime.
Of (hu+2) and (hr+2), at least one is an odd prime.
Theorem 16D: Let h be an odd composite number in the interval [n, 2n-3], and if h+2 is an odd prime and 2n-1 is an odd composite number, then there must be odd primes (h+2) in the interval [n, 2n-3].
ProofWe first quote the inference of Hou Shaosheng's theorem (theorem 4) as follows:
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
always have:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n, q is odd. n≤2n-q≤2n-3;
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And: pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
Please note that: (4.0.1), (4.0.2), (4.0.3), (4.0.4), are directly derived from Hou Shao-sheng's theorem, so the expression of these 4 equations will always be the same, regardless of whether num (2n=pi+pj) =0. It has nothing to do with whether the conjecture is true. In other words, even if the num(2n=pi+pj)=0, (4.0.1), (4.0.2), (4.0.3), (4.0.4), still is established.
According to (4.0.2),ht, hk are all the odd composite numbers h in the interval [3, n];
According to (4.0.4), hu, hr, are all the odd composite numbers h in the interval [n, 2n-3].
Since 3(2m+1) is an odd composite number, and (m is a positive integer), it follows that the odd composite number h in the interval [3,n] and [n,2n-3] is abundant. Because ht, hk is all the odd composite numbers h in the interval [3,n]; hu, hr, is all the odd composite numbers h in the interval [n,2n-3], so ht, hk, hu, hr, is rich, and it follows that (ht+2), (hk+2), (hu+2), (hr+2) is rich.
Theorem 16A is as follows.
Theorem 16A: (ht+2) and (hk+2), contain all the odd primes p after odd composite numbers in the interval [3, n]. After 11≤n, in the interval [3, n], there is at least one odd prime p are odd primes after odd composite numbers. After 50≤n, in the interval [3, n], 50% or more of the number of odd primes p are odd primes after odd composite numbers.
Theorem 16A is proved as follows:
From the concept can be known:
If h is all the odd composite numbers in the interval [3, n], then h+2 contains all the odd prime numbers p after the odd composite numbers in the interval [3, n].
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
(4.0.2) The mathematical meaning of the formula is:
The only odd composite numbers in the interval [3, n] are: ht, hk; And the number of odd composite h in the interval [3, n] is equal to the number of ht + the number of hk.
That is,ht,hk, is all the odd composite numbers h in the interval [3, n].
Therefore, (ht+2) and (hk+2) contain all the odd primes p after odd composite numbers in the interval [3, n].
This proves the statement of theorem 16A:
(ht+2) and (hk+2), contain all the odd primes p after odd composite numbers in the interval [3, n].
The following must be proved:
In the interval [3,n], 50% or more of the number of odd primes p are odd primes after odd composite numbers, 50≤n.
The proof is as follows:
The interval [3, n] is divided into two intervals [3, 50] and
[50, n].
A: There are a total of 14 odd prime numbers in the interval [3, 50]. As follows:
3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47.
Among them, odd prime number after odd composite number, there are a total of 7. As follows:
11,17,23,29,37, 41,47.
The actual inspection shows that 50% of the number of odd primes p in the interval [3, 50] are odd primes after odd composite numbers.
B: In the interval [50, n], there are no 3 sister primes. So, any odd prime number is either one of the two sister primes or an independent odd prime number.
Because, of the 2 sister odd primes (pi, pi+2), (pi+2 is an odd prime), there must be a pi, and only one pi is an odd prime after the odd composite number;
Any independent odd prime number p is an odd prime after an odd composite number.
Therefore, in the interval [50, n], 50% or more of the number of odd primes p are odd primes after odd composite numbers, 50<n.
By synthesizing the above A and B, we can see that in the interval [3, n], 50% or more of the number of odd prime numbers p is the odd prime number after the odd composite number, 50<n.
This proves that:
After 50≤n, in the interval [3, n], 50% or more of the number of odd primes p are odd primes after odd composite numbers.
Since 11 is an odd prime after an odd composite number, after 11≤n, there is at least one odd prime after an odd composite number in the interval [3, n].
At this point, the contents of theorem 16A are proved.
Theorem 16B is as follows:
(hu+2)and (hr+2), contain all the odd primes p after odd composite numbers in the interval [n, 2n-3]. In the interval [n, 2n-3], 50% or more of the number of odd primes p are odd primes after odd composite numbers, 10≤n.
Note: ht, hk, hu and hr here are ht, hk, hu and hr in Hou Shaosheng's theorem.
Theorem 16B is proved as follows:
First quote (4.0.4) as follows:
num(n≤h≤2n-3)=num (2n=ht+hu) +num (2n=ps+hr). (4.0.4)
The mathematical meaning of (4.0.4) is:
The only odd composite numbers in the interval [n, 2n-3] are: hu and hr; And the number of odd composite h in the interval [n, 2n-3] is equal to the number of hu + the number of hr.
That is, hu, hr, is all the odd composite numbers h in the interval [n, 2n-3].
Let all odd composite numbers in the interval [n, 2n-3] be h, then h+2 contains all odd primes after odd composite numbers in the interval [n, 2n-3].
Therefore, (hu+2) and (hr+2) contain all the odd primes p after the odd composite numbers in the interval [n, 2n-3].
This proves a statement in theorem 16B:
(hu+2) and (hr+2), contain all the odd primes p after odd composite numbers in the interval [n, 2n-3].
Let's prove another sentence from theorem 16B:
In the interval [n, 2n-3], 50% or more of the number of odd primes p are odd primes after odd composite numbers, 10≤n.
All odd numbers q in the interval [n, 2n-3] are either odd prime numbers p or odd composite numbers h. In 2.1.2, theorem 2 has been proved: 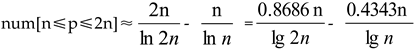
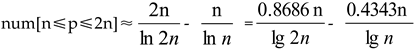
If n=10 is substituted into this formula, num[10≤p≤20] > 2 is obtained.
The actual test shows that num[10≤p≤20]=4, because 11,13,17, 19, ∈[10,20].
We have shown that num[n≤p≤2n] is an increasing function of n, n→∞. The calculation can prove that as long as 10≤n, there is
num[n≤p≤2n] > 2. This shows that after 10≤n, there are at least three odd prime numbers in the interval [n, 2n].
Since 3,5,7 is the only 3-sister odd prime number, there can be no 3-sister odd prime numbers in the interval [n, 2n], 3 < n. Therefore, when we talk about sister odd prime numbers below, we refer to 2 sister odd prime numbers unless otherwise specified.
Let 10<n, considering that n=pi,pi+2=pi+1, there are possible special cases, in the interval [n, 2n-3], as long as the number of odd primes is not less than 3, the odd prime p after the odd composite number must exist. This is because if n=pi, n+2=pi+2=pi+1, then pi, pi+1, is already a 2 sister prime, since there is no 3-sister prime, then the third odd prime pi+2, must be an odd prime after an odd composite number. That is, between pi+1 and pi+2 , there is at least one odd composite number h.
Since there are no 3-sister primes in the interval [n, 2n-3] after 10<n, there are either 2 sister primes or no 2 sister primes in the interval [n, 2n-3]. This shows that for every two prime numbers arranged from smallest to largest, at least one is an odd prime after an odd composite number.
This means that 50% or more of the number of odd primes p in the interval [n, 2n-3] are odd primes after odd composite numbers, 10≤n.
This proves another statement in theorem 16B:
In the interval [n, 2n-3], 50% or more of the number of odd primes p are odd primes after odd composite numbers, 10≤n.
At this point, the contents of theorem 16B are proved.
Let's prove theorem 16C.
Theorem 16C is as follows:
At least one of the (ht+2) and (hk+2) is an odd prime.
In both (hu+2) and (hr+2), at least one is an odd prime.
Theorem 16C is proved as follows.
Theorem 16A states that: (ht+2) and (hk+2) contain all the odd primes p after odd composite numbers in the interval [3, n].
Since 11 is an odd prime after an odd composite number, so long as 11≤n, then the odd prime p after odd composite number in the interval [3,n] must exist. These odd prime numbers p after odd composite numbers are all formed by odd composite numbers h+2.
Since (ht+2) and (hk+2) contain all the odd primes p after the odd composite numbers in the interval [3, n], at least one of (ht+2) and (hk+2) is an odd prime.
At least one of the (ht+2) and (hk+2) is an odd prime. Proof complete.
The following proof:
In both (hu+2) and (hr+2), at least one is an odd prime.
The proof is as follows.
Theorem 16B states that: (hu+2) and (hr+2) contain all the odd primes p after odd composite numbers in the interval [n, 2n-3]. In the interval [n, 2n-3], 50% or more of the number of odd primes p are odd primes after odd composite numbers.
Since (hu+2) and (hr+2) contain all the odd primes p after the odd composite numbers in the interval [n, 2n-3], at least one of (hu+2) and (hr+2) is an odd prime number.
In both (hu+2) and (hr+2), at least one is an odd prime. Proof complete.
Theorem 16C, complete proof.
Let's prove theorem 16D.
Theorem 16D, as follows.
Let h be an odd composite number in the interval [n, 2n-3], and if h+2 is an odd prime and 2n-1 is an odd composite number, then there must be an odd prime h+2 in the interval [n, 2n-3].
Theorem 16D is proved as follows:
Since h is an odd composite number in the interval [n, 2n-3], h≤2n-3, and therefore h+2≤2n-1. Since 2n-1 is assumed to be odd composite number, if h+2 is odd prime, must be h+2 < 2n-1. Since 2n-2 is even, there must be h+2≤2n-3. That is, if h+2 is an odd prime, then the odd prime h+2≤2n-3 . It's the odd prime h+2 in the interval [n, 2n-3].
Theorem 16D, complete proof.
Theorem 16 will play an important role in the proof of Goldbach's conjecture.
Finally, we emphasize that theorem 16 is a fundamental theorem. This theorem uncovers the transition from the odd composite number h to the odd prime h+2 after the odd composite number.
Theorem 16, finished proving.
5.12 Theorem 17: If the conjecture is true at 2n and k (=n+1)
is composite number, then at least one of (pi+2), (hr+2) is an
odd prime, which guarantees that the conjecture is true at 2k.
Proof:To prove theorem 17, first quote the theorem that will be used, and then prove theorem 17.
(1) Quote theorem 11 as follows:
Theorem 11: If the 2n conjecture is true, then 2k=pi++pj+ has the following 4 sources, as long as one of the sources exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
If the Goldbach conjecture holds for 2n, 2k=pi++pj+ has 4 sources, so long as one source exists, the conjecture will continue to hold for 2k. So, to prove the conjecture, you just need to prove the existence of a source.
Conversely, if the Goldbach conjecture is true at 2n, and if it is not true at 2k, then none of the 4 sources of 2k=pi++pj+ can exist.
As a proof by contradiction, if the conjecture is not valid when 2k is assumed, it is assumed that: 2k=pi++pj+ 4 sources, none of which exist; As long as the contradiction can be found according to rigorous mathematical reasoning, it is proved that 2k=pi++pj+ 4 sources, at least one of the existence. That proves the 2k conjecture. That's the mathematical idea behind proving Theorem 17.
(2) The inference of Hou Shaosheng's theorem is quoted as follows:
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
There is always:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=gsh(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n, q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And: pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
Under our theoretical system, if the conjecture is true for 2n, it is accepted that the above (4.0.1), (4.0.2), (4.0.3), (4.0.4) are also true. Therefore, the simultaneous holding of (4.0.1), (4.0.2), (4.0.3), (4.0.4) is also the theoretical basis for our proof of theorem 17, in particular the admission that: 1≤num(2n=pi+pj).
(3)If the conjecture is true for 2n and k (=n+1) is composite number, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture must be true for 2k.
The proof is as follows.
Because 2k=k+k, if k is an odd prime number, 2k is already the sum of two odd prime numbers, and the guess is natural stand.
So, as long as it can be shown that k is composite number, the 2k conjecture must be true, then Goldbach's guess If you want it, it will be true.
Therefore, in the following proof, it is not distinguished whether k is an even composite number or an odd composite number. Just assume k
It's composite number.
Specific proof is as follows:
The reference (4.0.1) is as follows.
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr) (4.0.1)
If the conjecture is true for 2n, it is accepted that (4.0.1) is true, where: 1≤num(2n=pi+pj).
(4.0.1), is the object of our study below.
Since k (=n+1) is composite number, it is obtained by (4.0.1) :
num(3≤p≤k)
=num(3≤p≤n)
=num(2n=pi+pj)+num(2n=ps+hr) (4.0.1)
=num(2n+2=pi+2+pj)+num(2n+2=ps+hr+2)
=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
that's it:
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
From (4.0.1) to (5.12.1), each step of the derivation process is based on mathematical definitions, or mathematical axioms, or mathematical theorems. So (5.12.1) must be true.
We know from (5.12.1) that as long as one of (pi+2) is an odd prime, there must be at least one 2k=(pi+2)+pj, and 2k is the sum of two odd prime numbers (pi+2), pj, to ensure that the conjecture must be true at 2k.
If none (pi+2) is an odd prime, then every (pi+2) is an odd composite number.
It is also known from (5.12.1) that as long as one of (hr+2) is an odd prime, there must be at least one 2k= ps+(hr+2), and 2k is the sum of two odd prime numbers ps, (hr+2), which guarantees that the conjecture must be true at 2k.
If none (hr+2) is an odd prime, then every (hr+2) is an odd composite number.
Therefore, whether at least one of (pi+2) is an odd prime number is one of the keys to proving Goldbach's conjecture.
Similarly, whether at least one of (hr+2) is an odd prime is another key to proving Goldbach's conjecture.
A sufficient condition for at least one of (pi+2) to be an odd prime is that at least one in pi is the lesser of the sister primes.
Note: If pi is the smaller of the sister primes, there must be (pi+2) that is odd primes.
Depending on whether at least one pi in (2n=pi+pj) is the smaller of the sister primes, pi can be divided into 2 classes.
first kind: In pi, at least one pi is the smaller of the sister primes. So at least one (pi+2) is an odd prime, which guarantees that the conjecture is true at 2k.
For this category, there is no need to write a written argument.
second kind: In pi, none of the pi is the lesser of the sister primes, so none (pi+2) is an odd prime; So every(pi+2)is an odd composite number.
For the second kind of pi, we must prove that at least one of (hr+2) is an odd prime. So there is at least one 2k=ps+(hr+2), where 2k is the sum of two odd prime numbers ps, (hr+2), which guarantees that the conjecture is true at 2k.
It can be obtained from (5.12.1) :
num(3≤p≤k)-num(2k=ps+(hr+2))=num(2k=(pi+2)+pj) (5.12.2)
By (5.12.1) must be true, we can see that (5.12.2) must be true.
That is to say, both sides of the equal sign must have the same number and the same name.
However, if (pi+2) is not an odd prime, then (5.12.2) must not be true. This proof uses the mathematical implication that if (pi+2) is not an odd prime, (5.12.2) must not be true to prove theorem 17. The concrete proof is as follows.
In (5.12.2), according to our convention, ps≤(hr+2), (pi+2)≤pj, so ps, (pi+2), are within the interval [3, k].
(hr+2), pj, both in the range [k, 2k-3]. pi, pj, ps are odd prime numbers.
(pi+2),(hr+2),whether it is an odd prime number or an odd composite number, is what needs to be discussed below.
The following, through the way of debating questions and answers, proves that 2k, Goldbach conjecture must be true.
Question 1: Is at least one (hr+2) an odd prime?
If at least one (hr+2) is an odd prime, then the Goldbach conjecture holds for 2k.
This is because 2k=ps+(hr+2), with at least one 2k, is already the sum of an odd prime ps and an odd prime (hr+2). So at 2k, Goldbach's conjecture is established.
If there is not at least one (hr+2) that is an odd prime,that is, every (hr+2) is an odd composite number.
Next, we continue our argumentative question and answer under the assumption that each (hr+2) is an odd composite number.
Question 2: Under the assumption that every (hr+2) is an odd composite number, is at least one (pi+2) an odd prime number?
If at least one (pi+2) is an odd prime, then the Goldbach conjecture holds for 2k.
This is because 2k=(pi+2)+pj, with at least one 2k, is already the sum of an odd prime (pi+2) and the odd prime pj. So at 2k, Goldbach's conjecture is established.
If there is not at least one (pi+2) that is an odd prime,So every (pi+2) is an odd composite number.
From problem 1 and problem 2, we can see that the conditions for the following discussion are: every (pi+2), every (hr+2) is an odd composite number.
Next, we continue our argumentative question and answer under the assumption that each (pi+2) and each (hr+2) are odd composite numbers.
Each (pi+2) is an odd composite number, that is, the smaller of the sister primes that do not exist in pi.
Question 3: Under the assumption that every (pi+2) and every (hr+2) are odd composite numbers, does (5.12.2) still hold?
(5.12.2), is a mathematical equation, both sides of the equal sign, must have the same number, the name of the thing must be the same.
num(3≤p≤k)-num(2k=ps+(hr+2))=num(2k=(pi+2)+pj) (5.12.2)
From the derivation process of 5.12.2), we can know that (5.12.2) must be established and (5.12.2) should be established.
However, under the assumption that every (pi+2), every (hr+2), is an odd composite number, (5.12.2) cannot be held. This means the hypothesis is not valid! (pi+2), (hr+2), at least one of them is an odd prime number! So theorem 17 is true. That's the logic below.
If one (pi+2) is an odd prime, or one (hr+2) is an odd prime, then Goldbach's conjecture is established.
To prevent us from proving the conjecture, we must assume that every (pi+2) is odd composite number and every (hr+2) is odd composite number.
Next, we proceed with the inference under the assumption that each (hr+2) and each (pi+2) are odd composite numbers.We shall show that the assumptions here contradict the results obtained by further inference. This is proof by contradiction.
Now analyze (5.12.2) as follows.
On the left side of the equal sign, num(3≤p≤k) is the number of all the odd prime numbers p in the interval [3, k].
Every ps is an odd prime, so it's known. There is no doubt.
Each (hr+2), according to the above assumptions, is an odd composite number.
So every 2k=ps+(hr+2), every 2k is the sum of an odd prime ps and an odd composite number (hr+2).
Since ps is a partial odd prime in the interval [3, k], num (2k=ps+(hr+2)) is the number of partial odd prime ps in the interval [3, k]. It is also the number of odd composite numbers (hr+2) in the interval [k, 2k-3].
So, num(3≤p≤k)-num (2k=ps+(hr+2)) is the number of all odd primes p in the interval [3, k], minus the number of partial odd primes ps in the interval [3, k].
Therefore,num(3≤p≤k)-num (2k=ps+(hr+2)) is the number of partial odd prime numbers p in the interval [3, k].
(5.12.2), is a mathematical equation. On the right side of the equals sign, num(2k=(pi+2)+pj), must also be the number of partial odd prime p in the interval [3, k]. This is a necessary condition for the establishment of (5.12.2).
num(2k=(pi+2)+pj), must also be the number of partial odd prime numbers p in the interval [3, k]; It follows that every (pi+2) must be an odd prime p in the interval [3, k].However, this contradicts the previous: it must be assumed that every (pi+2) is odd composite number and every (hr+2) is odd composite number.
The proof of theorem 17 is now completely complete. However, for the reader to see more, the inferences continue.
If one of (pi+2) is not an odd prime p in the interval [3, k],
num(2k=(pi+2)+pj) cannot be the number of partial odd prime p in the interval [3, k].
num(2k=(pi+2)+pj)represents the number of(2k=(pi+2)+pj)according to the definition (1.2 Meaning of mathematical symbols in the paper).
The number of (2k=(pi+2)+pj) is the number of (pi+2) in the interval [3, k], which is also the number of pj in the interval [k, 2k-3].
Since every (pi+2) must be an odd prime p in the interval [3, k], the conjecture holds for 2k. As a proof of the conjecture, this is quite the end of it.
Above, we have proved that at 2k, Goldbach's conjecture holds.
Our main logical procedure for proving the conjecture is: In order to prevent proving Goldbach's conjecture, we must assume that every
(pi+2), every (hr+2), is an odd composite number; Under this assumption, num (2k=(pi+2)+pj) must be the number of odd composite numbers (pi+2) in the interval [3, k]. However, this contradicts the necessary condition for (5.12.2) to hold: num (2k=(pi+2)+pj) must be the number of odd primes p in the interval [3, k], so (pi+2) must be odd primes! Therefore, the assumption that every (pi+2), every (hr+2), is an odd composite number cannot be held. So at least one of (pi+2), (hr+2), is an odd prime. This is the main logical process for proving theorem 17. This proof, in fact, is proof by contradiction.
The proof of theorem 17 ends here.
(3) The role of sister primes in the proof of Goldbach's conjecture.
Some readers may ask: The key step in your proof is to assume that every (pi+2) is an odd composite number, and every (hr+2) is an odd composite number, which follows: num (2k=(pi+2)+pj) is the number of odd composite numbers (pi+2) in the interval [3, k].This contradicts the fact that num (2k=(pi+2)+pj) must be the number of odd prime numbers p in the interval [3, k].
Asking 1: If you don't assume that every pi+2 is an odd composite number, does theorem 17 still hold?
Answer: True.If we do not assume that every (pi+2) is an odd composite number, that is, at least one of (pi+2) is an odd prime, which is consistent with theorem 17, then at least one of (pi+2), (hr+2), is an odd prime number.
A sufficient condition for each (pi+2) to be odd composite number is that pi is either the largest of the (3,5,7) sister primes, or the larger of the other sister primes, or an independent odd prime. If every (pi+2) is an odd composite number, then at least one of (hr+2) is an odd prime number, as theorem 17 states.
A sufficient condition for (pi+2) to be an odd prime is that pi is the lesser of the (3,5,7) sister primes 3, or 5, or the lesser of the other sister primes.
As long as one (pi+2) is an odd prime, 2k=(pi+2)+pj, 2k is already the sum of two odd prime numbers, and Goldbach's conjecture is guaranteed.
Above is the role of sister primes in the proof of Goldbach's conjecture.
Below, we first demonstrate with example 8 that every (pi+2) is an example of an odd composite number and does exist.The main proof procedure of theorem 17 above is verified with example 8.
Since instance 8, each of which (pi+2) is a odd composite number, has certain particularity, and then use instance 3 to verify the main proof process of theorem 17 above. Example 3 is the example 3 used many times in the paper.
(4) Each of these (pi+2) is an example of an odd composite number that does exist.
Some readers wonder if there are actual examples: every one of them (pi+2) is an odd composite number.
Answer: Each of these (pi+2) is an example of an odd composite number that does exist.
Take a look at example 8 below.
Example 8. 2×19=38
=1+37=3+35=5+33=7+31=9+29=11+27=13+25=15+23=17+21=19+19.
In this example:
num(2×19=q+(2n-q))=9. num(19≤p≤2×19-3)=4.
num(19≤h≤2×19-3)=5.
num(2n=pi+pj)=2. num(2n=ht+hu)=0. num(2n=ps+hr)=5.
num(2n=hk+pd)=2. num(3≤p≤35)=10. num(3≤h≤35)=7.
num(3≤p≤19)=7. num(3≤h≤19)=2.
The above data fully satisfy the following formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Note that num(2n=ht+hu)=0. It indicates that 2n is small. When 2n is slightly larger, num(2n=pi+pj), num(2n=ht+hu), num(2n=ps+hr), and
num(2n=hk+pd) should all be no less than 1.
num(3≤p≤19)=7 > num(3≤h≤19)=2. That also means that 2n is small. When 2n is larger, the value should be: num(3≤p≤n)≤num(3≤h≤n).
num(3≤p≤n)≤num(3≤h≤n) indicates that the number of odd primes in the interval [3,n] is not greater than the number of odd composite numbers.
look again
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr) (4.0.1)
num(3≤p≤19)=7. The seven odd prime numbers are: 3, 5, 7, 11, 13, 17, 19.
num(2×19=pi+pj)=2. The 2 number of(2×19=pi+pj) are:
2×19=7+31, 2×19=19+19.
num(2n=ps+hr)=5. The 5 number of (2×19=ps+hr)are as follows:
2×19=3+35; 2×19=5+33; 2×19=11+27; 2×19=13+25; 2×19=17+21.
The above data are in full agreement with the formula (4.0.1). The test is as follows:
Num(2n=pi+pj)+num(2n=ps+hr)
=2+5
=7=num(3≤p≤19).
The test shows that the above data are in full agreement with the formula (4.0.1).
Of particular note: num(2×19=pi+pj)=2. These two number of
(2×19=pi+pj) are:
2×19=7+31; 2×19=19+19.
This means that the two of pi in(2×19=pi+pj)are 7 and 19.
7 is the largest prime of the sister primes (3,5,7).
19 is the larger prime of the sister primes (17,19).That is, in the pi of instance 8, there is no smaller of the sister
primes.
We notice that in the pi of example 8, there is no smaller of the sister primes because num(2×19=pi+pj)=2. In other words, it's because n=19 is relatively small.
Look again:
7+2=9. 9 is an odd composite number.
19+2=21. 21 is an odd composite number.
That is, in example 8, every (pi+2) is an odd composite number, not an odd prime number.
Example 8 tells us that there are instances where each (pi+2) is an odd composite number. This shows that it is impossible to prove Goldbach's conjecture by proving that at least one of (pi+2) is an odd prime number.
Example 8 tells us that every pi in (2n=pi+pj) is the greater of the sister primes, or the largest of (3,5,7), and therefore every (pi+2) is an odd composite number, and such practical examples exist. So the smaller of the sister primes, all in ps of (2n=ps+hr), there are practical examples of this.
In Example 8, num(2n=ps+hr)=5. The five number of (2n=ps+hr) are as follows:
2×19=3+35; 2×19=5+33; 2×19=11+27; 2×19=13+25; 2×19=17+21.
The five of ps are as follows:
3; 5; 11; 13; 17.
3,5, is the smaller of the (3, 5, 7) sister primes.
11 is the smaller of the (11, 13) sister primes.
17, is the smaller of the (17, 19) sister primes.
In the interval [3, n], which in this case is the interval [3, 19], all the odd primes are as follows:
3, 5, 7, 11, 13, 17, 19.
The smaller of the sister primes are: 3, 5, 11, 17. They're all in ps. 13 is the greater of the (11, 13) sister primes. However, this cannot be denied: the smaller of the sister primes are all in ps.
Example 8 tells you and me that it is never possible to prove Goldbach's conjecture by proving that at least one of (pi+2) is an odd prime.
Since it is impossible to prove that at least one of (pi+2) is an odd prime, we prove theorem 17 that at least one of (pi+2) and (hr+2) is an odd prime! This completes the proof of Goldbach's conjecture.
(6) Verify theorem 17 and its main proof process with example 8.
(6.1) Verify theorem 17 with example 8.
Theorem 17: If the conjecture is true at 2n and k (=n+1) is compositenumber, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Example 8 is as follows:
Example 8. 2×19=38
=1+37=3+35=5+33=7+31=9+29=11+27=13+25=15+23=17+21=19+19.
In Example 8: num(2×19=pi+pj)=2. The two number of (2×19=pi+pj) are: 2×19=7+31;2×19=19+19.
This means that the two of pi in(2×19=pi+pj)are 7 and 19.
7 is the largest of the sister primes (3,5,7); 19 is the greater of
the sister prime numbers (17,19).
7+2=9. 9 is an odd composite number.
19+2=21, 21 is an odd composite number.
That is, in example 8, each pi in(2n=pi+pj)is either the largest of the sister primes, Or 7, the largest of the sister primes (3,5.7). Or, in pi, there is no smaller of the sister primes. So every pi+2 is an odd composite number.
Since every (pi+2) is an odd composite number, theorem 17 tells us that at least one of (hr+2) is an odd prime number, which guarantees that the conjecture holds for 2k.
Whether this is actually the case, the test is as follows:
In Example 8, num(2n=ps+hr)=5. The five number of(2n=ps+hr)are as
follows:
2×19=3+35; 2×19=5+33; 2×19=11+27; 2×19=13+25; 2×19=17+21.
The five number of ps are as follows:
3; 5; 11; 13; 17.
The five number of hr are as follows:
35; 33; 27; 25; 21.
Each hr+2 is as follows:
35+2=37; 33+2=35; 27+2=29; 25+2=27; 21+2=23.
hr+2, is an odd prime number, as follows:
35+2=37; 27+2=29; 21+2=23.
Since every (pi+2) is an odd composite number, according to theorem 17, at least one of (hr+2) is an odd prime number, which guarantees that the conjecture is true at 2k.
In example 8, three number of (hr+2) are odd prime numbers: 35+2=37; 27+2=29; 21+2=23.
The result of verifying theorem 17 with example 8 shows that the actual situation of example 8 is completely consistent with theorem 17.
(6.2) Verify the main proof process of theorem 17 with example 8.
(5.12.2), is a mathematical equation, both sides of the equal sign, should have the same quantity, the same name of things.
num(3≤p≤k)-num(2k=ps+(hr+2))=num(2k=(pi+2)+pj) (5.12.2)
Obviously,num(3≤p≤k)-num(2k=ps+(hr+2)), on the left side of the equals sign, is the number of partial odd prime p in the interval [3, k], without any doubt.
(5.12.2), is a mathematical equation where num(2k=(pi+2)+pj), on the right side of the equal sign, must also be the number of partial odd primes p in the interval [3, k]. This is a necessary condition for the establishment of (5.12.2).
Since num(2k=(pi+2)+pj)must also be the number of partial odd prime numbers p in the interval [3, k], every (pi+2) must be an odd prime number p in the interval [3, k]; Otherwise, num(2k=(pi+2)+pj) cannot be the number of partial odd prime p in the interval [3, k]. Since each (pi+2) is an odd prime p in the interval [3, k], the conjecture is established at 2k. Thus, (5.12.2), the necessary condition for the establishment is: 2k, the conjecture is established.
If one of (pi+2) is an odd prime, or one of (hr+2) is an odd prime, then Goldbach's conjecture is established.
To prevent us from proving the conjecture, we must assume that every (pi+2) is odd compositenumberand every (hr+2) is odd compositenumber.
In Example 8, num(2×19=pi+pj)=2. The two number of (2×19=pi+pj)are:
2×19=7+31;2×19=19+19.
Since num(2×19=pi+pj)=2. There are 2 number of (pi+2).
These 2 number of (pi+2) are: (7+2), (19+2).
(7+2)=9. 9 is an odd composite number.
(19+2)=21, 21 is an odd composite number.
Because instance 8 only has these 2 number of (pi+2), which are (7+2)= 9,9 is odd composite number; (19+2)=21, 21 is an odd composite number. So every (pi+2) is an odd composite number. So num(2k=(pi+2)+pj), is the number of odd composite numbers (pi+2) in the interval [3, k]; Contradicts
num(2k=(pi+2)+pj), which must be the number of odd prime p in the interval [3, k]. This contradiction illustrates: the assumption that every (pi+2) is odd composite number and every (hr+2) is odd composite number is not true. That is, (pi+2), (hr+2), at least one of them is an odd prime.
The above verification results are in complete agreement with the proof process of theorem 17.
Verify theorem 17 with example 8, and this is the end.
(7) Verify theorem 17 and its main proof process with example 3.
(7.1) Verify theorem 17 with example 3.
Since in instance 8, each (pi+2) is an odd composite number and has particularity, we will use example 3 to verify theorem 17.
Example 3. 2×51=102
=1+101=3+99=5+97=7+95=9+93=11+91=13+89=15+87=17+85=19+83=21+81
=23+79=25+77=27+75=29+73=31+71=33+69=35+67=37+65=39+63=41+61
=43+59=45+57=47+55=49+53=51+51.
Theorem 17: If the conjecture is true at 2n and k (=n+1) is compositenumber, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
In Example 3, num(2×51=pi+pj) =8.
These 8 number of 2×51=pi+pj, as follows:
2n=5+97; 2n=13+89; 2n=19+83; 2n=23+79; 2n=29+73;
2n=31+71; 2n=41+61; 2n=43+59;
Among, 8 number of pi are as follows:
5, 13, 19, 23, 29, 31, 41, 43.
In the 8 number of pi above, pi+2, are odd prime numbers as follows:
5+2=7; 29+2=31; 41+2= 43.
So, 2k=2(n+1)=(pi+2)+pj, 2k is the sum of two odd prime numbers, as
follows:
2k=(5+2)+97; 2k=(29+2)+73; 2k=(41+2)+61.Any one of the above three 2k is sufficient to guarantee the Goldbach
conjecture for 2k=2 (n+1)!
The above fact shows that in 2n=pi+pj, as long as there is a pi+2, which is an odd prime number, then 2k=2(n+1), Goldbach's conjecture must be true.
In 2n=pi+pj, a sufficient condition for one of pi+2 to be an odd prime is that one of pi is the smaller of the sister primes.
Similarly, in 2n=pi+pj, as long as there is a pj+2, which is an odd prime number, then 2k=2(n+1), Goldbach's conjecture must be true.
In 2n=pi+pj, pi is either the smaller of the sister primes, or it is not; pi must be one of them.
pi is not the smaller of the sister primes, and there are only two possibilities: pi is the larger (or largest) of the sister primes, or it is an independent odd prime. In both cases, pi+2 is not an odd prime, it's an odd composite number.
In Example 3 :num(2n=ps+hr) =6.
These 6 number of 2n=ps+hr are as follows:
2n=3+99; 2n=7+95; 2n=11+91; 2n=17+85; 2n=37+65;
2n=47+55;
Among them, 6 number of hr, as follows:
99;95;91; 85;65;55.
In the 6 number of hr above, hr+2, are odd prime numbers as follows:
99+2=101; 95+2=97; 65+2=67.
So, 2k=2(n+1)=ps+(hr+2), 2k is the sum of two odd prime numbers, as follows:
2k=3+(99+2); 2k=7+(95+2); 2k=37+(65+2).
Any one of the above three 2k is sufficient to guarantee the Goldbach conjecture for 2k=2 (n+1)!
We see that in example 3, pi has the smaller of the sister primes; There are also the larger of the sister primes. This is completely different from example 8.
Example 3: In pi, at least oneofpiis the smaller prime of the sister primes, so at least oneof(pi+2) is an odd prime. So there is at least one 2k=(pi+2)+pj, where 2k is the sum of two odd primes (pi+2), pj, which guarantees that the conjecture holds for 2k.
The above logic tells us that in pi, as long as one piis the smaller prime of the sister primes, the 2k time conjecture is guaranteed to be true. Thus, the proof of the conjecture only needs to prove that in pi, no piis a smaller prime number, and the conjecture still holds at 2k.
In the proof of theorem 17, assuming that every (pi+2) is an odd composite number, essentially assuming that no pi is a smaller prime of sister prime, we still prove that the 2k conjecture holds. The others, of course, at least one of pi is the smaller prime of the sister primes, and the conjecture is certainly true, and no further proof is needed.
(5.12.2), is a mathematical equation, both sides of the equal sign, should have the same quantity, the same name of things.
num(3≤p≤k)-num(2k=ps+(hr+2))=num(2k=(pi+2)+pj) (5.12.2)
Obviously, num(3≤p≤k)-num(2k=ps+(hr+2)) is the number of partial odd prime p in the interval [3, k], without any doubt.
(5.12.2), is a mathematical equation where num (2k=(pi+2)+pj), on the right side of the equal sign, must also be [3, k] the number of odd primes p in the interval. Each (pi+2) in (2k=(pi+2)+pj) must be an odd prime p in the interval [3, k]. Because, if there is a (pi+2) that is not an odd prime p in the interval [3, k], num(2k=(pi+2)+pj), it cannot be the number of odd prime p in the interval [3, k].
If there is a (pi+2) that is an odd prime p in the interval [3, k], then Goldbach's conjecture is established. If any (pi+2) is not an odd prime p in the interval [3, k], then any (pi+2) is an odd composite number h in the interval [3, k]; num (2k=(pi+2)+pj) is the number of odd composite number h in the interval [3, k]. This contradicts num (2k=(pi+2)+pj), which must be the number of odd prime numbers p in the interval [3, k].
If some (pi+2) is an odd prime p in the interval [3, k]; The others (pi+2) are odd composite numbers h in the interval [3, k]; num(2k=(pi+2)+pj)
is not the number of odd primes p in the interval [3, k]. This contradicts num(2k=(pi+2)+pj), which must be the number of odd prime numbers p in the interval [3, k]. Example 3 falls into this category.
After the above verification, we know that the case of example 3 is still included in the proof of theorem 17.
(pi+2) in (2k=(pi+2)+pj), there are only three cases.
In the first case, each (pi+2) is a odd composite number h in the
interval [3, k]. Example 8 falls into this category.
In the second case, some of (pi+2) are odd prime numbers p in the interval [3, k]; Others of (pi+2), are odd composite number h in the interval [3, k]; Example 3 falls into this category.
Since some of (pi+2) are odd prime numbers p in the interval [3, k], the conjecture holds at 2k.
In the third case, each (pi+2) is an odd prime p in the interval
[3, k]. There must be 2k=2n+2=(pi+2)+pj. 2k is already the sum of two odd prime numbers. This is already included in the proof of theorem 17.
After the above test, we know that: (pi+2), the three possible cases, all included in the proof of theorem 17. Therefore, the proof process of theorem 17 is absolutely rigorous and absolutely comprehensive! Verify theorem 17 with example 3, and this is the end.
(8)Readers may raise the following questions, and answer them as follows.
(8.1) Some experts ask why you use 2k=(pi+2)+pj instead of 2k=(pi+1)+(pj+1)?
The answer is as follows: 2n+2=2k=(pi+2+pj) =(pi+1)+ (pj+1), which is numerically valid. However, since pi and pj are both odd prime numbers, pi+1 and pj+1 are both even numbers. So 2k=(pi+1)+ (pj+1), is 2k expressed as the sum of two even numbers. This has nothing to do with the 2k conjecture. In other words, 2k=(pi+1)+ (pj+1) is meaningless for the study of Goldbach's conjecture. So we're not going to consider the form 2k=(pi+1)+ (pj+1).
Above is the answer to the question (8.1).
(8.2) Some experts ask, you use 2k=(pi+2)+pj, in (2k=(pi+2)+pj), did not lose the prime number 3?
The answer is as follows: In order to answer the questions of the experts on this subject, we have previously added some findings on sister prime numbers.
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr) (4.0.1)
We know that there must be sister primes in the interval [3,n].However,it is not certain that pi must contain the smaller of the sister primes.
If the mth odd prime is pm, and pm+2=pm+1, pm+1 is the (m+1)th odd prime. We call pm the smaller of the sister prime numbers; pm+1 is the larger of the sister primes.
If at least one of the pi is the smaller of the sister primes, then it is immediately certain that at least one of (pi+2) is an odd prime.
So there is at least one 2k=(pi+2)+pj, 2k is already the sum of two odd prime numbers (pi+2), pj. So Goldbach's conjecture is established.
Example 8, in pi, none is the smaller of the sister primes; The smaller of the sister primes, all in ps.
Example 8 tells us that it is never possible to prove that at least one of pi is the smaller of the sister primes.
The existence of example 8 has brought endless difficulties and troubles to the proof of Goldbach's conjecture. The above theorem 17 and its proof successfully avoid this endless difficulty and annoyance.
Now we can answer your questions. Experts ask, if you use 2k=(pi+2)+pj, don't you lose the prime 3?
The answer is as follows: If you think that one pi is the smaller of the sister primes, then at least one (pi+2) is already an odd prime, then the conjecture is established.
If you think that no pi is the lesser of the sister primes, you think that every pi is the greater of the sister primes, so (pi+2) are all odd composite numbers.
Under the above assumption, each (pi+2) is an odd composite number.
So, where is the odd prime number 3? Under the above assumption, it
should be said with certainty that 3 is one of the odd prime numbers ps. Also, the smaller of the sister primes are in ps.
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
Because 3 is one of the odd prime numbers ps, it is not thrown away. The smaller of each sister prime, including 3,5, is in ps and is not thrown away.
The above is the answer to the question (8.2).
(8.3) Some experts say that in (5.12.1), num (2k=(pi+2)+pj) represents the number of odd prime pi. Does not represent the number of pi+2. Answer as follows: Do you think num(2k=(pi+2)+pj) represents the number of odd primes pi, not (pi+2). First of all, your understanding is inconsistent with the definition of the meaning of mathematical symbols in (1.2 the meaning of mathematical symbols in the paper), so it is wrong.
Second, you're not treating pi+2 as a number. See: (3+2) =5, (5+2) =7, (7+2) =9, (9+2) =11,..., 5,7,9,11... is a number. You interpret a parenthesis (pi+2) as two numbers. This contradicts the intention of the paper. If you say so, you only look at pi in (pi+2). So, let me ask you, where did the +2 go?
In this article, we always treat the parentheses (pi+2) and similar representations as a number. Look at the text before you ask your question, in bold bold:
Question 1: Is at least oneof(hr+2) an odd prime?
Question 2: Under the assumption that every (hr+2) is an odd composite
number, is at least oneof(pi+2) an odd prime number?
Fromquestion1 andquestion2, we can see that the basis of the following discussion is: every (pi+2), every (hr+2) is an odd composite number.
Question 3: Is (5.12.1) still true under the assumption that every (pi+2), every (hr+2), is an odd composite number?
This proves that the assumption that every (pi+2), every (hr+2), is an odd composite number is not valid! It proves that at least one of (pi+2), (hr+2), is an odd prime. So at 2k, Goldbach's conjecture works.
You see, the above (hr+2), (pi+2), every time they are mentioned, are they not as a number?
The above is the answer to the question (8.3).
(8.4) The expert asks: (pi+2), (hr+2), at least one of which is an odd prime, is this consistent with the previous theorem?
The answers are as follows. Theorem 11: If the 2n conjecture is true, then 2k=pi++pj+ has the following 4 sources, as long as one of the sources exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of(pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
Theorem 11 simply says:If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
It is not certain that at least one of (pi+2), (hr+2), is an odd prime number; There is no guarantee that at least one 2k=pi++pj+ exists; There's just no guarantee that Goldbach's conjecture is true.
So, at least one of (pi+2), (hr+2) is an odd prime, which is a step further than theorem 11. Absolutely sure, at least one 2k=pi++pj+ exists, which is a completely positive conclusion. It's exactly the same as theorem 11, but it goes one step further and makes a completely positive conclusion.
The above is the answer to the question (8.4).
(8.5) The reader asks:In each of the eight mathematical instances in the paper, is at least one of them (pi+2), (hr+2) an odd prime?
Answer as follows:We examined each of the eight mathematical examples. Every mathematical instance, in (pi+2), (hr+2), has at least one odd prime.
Earlier, we did a written test for Example 3 and Example 8, which the reader has reviewed.
Readers are welcome to write their own new mathematical examples, test theorems 17. And we hope you will publish your examples and test results. It is best to send your examples and test results to Hou Shaosheng.
Example 3 is shown as follows.
Example 3. 2n=2×51=102
=1+101=3+99=5+97=7+95=······=41+61=43+59=45+57=47+55
=49+53=51+51.
Example 3, above, is the third of the eight mathematical examples in the paper: Example 3. Now use example 3 to verify that at least one of the (pi+2), (hr+2) numbers is an odd prime.
Verify the first source of 2k=2(n+1)=pi++pj+as follows:
num(2×51=pi+pj)=8.
These 8 number of 2×51=pi+pj are:
2×51= 5+97 = 13+89 = 19+83 = 23+79
= 29+73 = 31+71 = 41+61 = 43+59.
2n=pi+pj,(pi+2) are odd prime numbers:
(5+2)+97 =(29+2)+73 =(41+2)+61.
This verifies that at least one of (pi+2) is an odd prime.
For every (pi+2) that is an odd prime, there is a 2k=(pi+2)+pj, which becomes one of 2k=pi++pj+.
For example, since 2×51=5+97, and(5+2)is an odd prime,
So 2×(51+1)=(5+2)+97.
2×(51+1) is already the sum of two odd prime numbers.
For example, since 2×51=29+73, and (29+2) is an odd prime,
So 2×(51+1)=(29+2)+73.
2×(51+1) is already the sum of two odd prime numbers.
......
This verifies that for every (pi+2) that is an odd prime, there is a 2k=(pi+2)+pj, which becomes one of 2k=pi++pj+.
Verify the third source of2k=2(n+1)=pi++pj+ as follows:
num(2n=ps+hr)=6.
The 6 number of 2×51=ps+hr are:
2×51= 3+99 = 7+95 = 11+91 = 17+85 = 37+65 = 47+55.
2n=ps+hr,(hr+2) are odd prime numbers: 3+(99+2)= 7+(95+2)
= 37+(65+2).
This verifies that (hr+2) is an odd prime number.
For each (hr+2) that is an odd prime, there is a 2k=ps+(hr+2), which becomes one of 2k=pi++pj+.
For example, since 2×51= 3+99, and (99+2)is an odd prime,
So 2×(51+1)=3+(99+2).
2×(51+1) is already the sum of two odd prime numbers.
For example, since 2×51=7+95, and (95+2)is an odd prime,
So 2×(51+1)=7+(95+2).
2×(51+1) is already the sum of two odd prime numbers.
......
This verifies that if one of (hr+2) is an odd prime, then 2k=ps+(hr+2)
becomes one of 2k=pi++pj+.
The reader should appreciate that the four sources of 2k=pi++pj+ would rather repeat the same data, (Character under shadow), and never give up the opportunity to ensure that the conjecture continues to hold.
The appearance of the duplicate data explains the four sources, which provides multiple guarantees for the establishment of Goldbach's conjecture. If only one(2k=pi++pj+)occurs once, the conjecture is guaranteed. If(2k=pi++pj+)occurs m times in total, the conjecture is guaranteed m times. This is one of the mysteries of the persistence of conjecture.
Since(2k=pi++pj+)has only four sources,(2k=pi++pj+)can occur at most four times for the same(2k=pi++pj+). Because in the same source, there can be no duplication.
Above is the answer to the question (8.5).
(9)Some notes on (5.12.1).
(5.12.1) is as follows:
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
Note: num(3≤p≤k) indicates the number of odd primes p in the interval [3, k].
In (5.12.1), (pi+2), ps, are in the interval [3, k].
pj, (hr+2), are all in the interval [k, 2k-3].
pj, ps is an odd prime number.
(pi+2), (hr+2), whether it is an odd prime number or an odd composite
number, is what needs to be discussed below.
The inference of Hou Shaosheng's theorem when quoting theorem 5:2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
Among them, it is agreed:
pi+, pj+, ps+, pd+, are all odd prime numbers; ht+, hu+, hr+, hk+, are all odd composite numbers.
And: pi+≤pj+, ht+≤hu+, ps+≤hr+, hk+≤pd+.
pi+, ht+, ps+, hk+, ∈[3,k]; pj+, hu+, hr+, pd+, ∈[n,2k-3].
(9.1) Note 1: If one of (pi+2) is an odd prime in the interval
[3, k], one of (hr+2) is odd composite number in the interval [k, 2k-3]. In this case,
num(3≤p≤k)=num(2k=(pi+2)+pj)+ num(2k=ps+(hr+2)) (5.12.1)
Among:
2k=(pi+2)+pj becomes one of 2k=pi++pj+.
2k=ps+(hr+2) becomes one of 2k=ps++hr+.
(9.2) Note 2: Where does all 2k=pi++pj+ in (4.3.1) come from?
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
Theorem 11: If the 2n conjecture is true, then 2k=pi++pj+ has the
following four sources, as long as one of the sources exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
(9.3) Note 3: If one of (pi+2)is an odd prime in the interval [3, k], one of (hr+2) is odd prime number in the interval [k, 2k-3], where
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
Among:
2k=(pi+2)+pj becomes one of 2k=pi++pj+.
2k=ps+(hr+2) becomes one of 2k=pi++pj+.
2k=(pi+2)+pj, 2k=ps+(hr+2), are all part of num(2k=pi++pj+).
Moreover, it is possible that (pi+2)=ps and pj=(hr+2).
If (pi+2)=ps, pj=(hr+2), then 2k=(pi+2)+pj, 2k=ps+(hr+2), are repeated.
(9.4) Note 4: Expert asked: Does 2k=ps++hr+ in (4.3.1) no longer exist?
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
In general, 2k=ps++hr+, there is: 1≤num(2k=ps++hr+).
num(2k=ps++hr+)=0. only when k is small.
For example, k=5, 2k=10=1+9=3+7=5+5.
In this example, 2×5=3+7, 2×5=5+5. Both are one of 2k=pi++pj+.In this example, num(2k=ps++hr+)=0.
Where does 2k=ps++hr+ in (4.3.1) come from?Theorem 12:
If the conjecture is true for 2n, then 2k=ps++hr+ has four
sources:
If one of (pj+2) is an odd composite number, then 2k=pi+(pj+2) becomes
one of 2k=ps++hr+;
If one of (hr+2) is an odd composite number, then 2k=ps+(hr+2) becomes
one of 2k=ps++hr+;
If one of (ps+2) is an odd prime, then 2k=(ps+2)+hr becomes one of
2k=ps++hr+;
If one of (ht+2) is an odd prime, then 2k=(ht+2)+hu becomes one of
2k=ps++hr+.
(9.5) Note 5: If one of (pi+2) is an odd composite number in the interval [3, k], one of (hr+2) is odd composite number in the interval [k, 2k-3], where
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
Among:
2k=(pi+2)+pj becomes one of 2k=hk++pd+.This 2k=hk++pd+ must be transferred
from (5.12.1) to (4.3.2), (4.3.3) to become one of num (2k=hk++pd+).It is a process of returning to the source.
2k=ps+(hr+2) becomes one of 2k=ps++hr+. This 2k=ps++hr+ must be transferred
from (5.12.1) to (4.3.1), (4.3.4) to become one of num (2k=ps++hr+).It is a process of returning to the source.
Similar situations encountered should be dealt with in this way, and will not be discussed too much.
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
(9.6) Note 6: The reader asks: Where does all of 2k=hk++pd+ come from?
Theorem 14: If the conjecture is true for 2n, 2k=hk++pd+ has the following 4 sources:
If one of (pi+2) is odd composite, then 2k=(pi+2)+pj becomes one of 2k=hk++pd+;
If one of (hu+2) is odd prime, then 2k=ht+(hu+2) becomes one of 2k=hk++pd+;
If one of (hk+2) is odd composite, then 2k=(hk+2)+pd becomes one of 2k=hk++pd+;
If one of (pd+2) is an odd prime, then 2k=hk+(2+pd) becomes one of 2k=hk++pd+.
(9.7) Note 7: If one of(pi+2) is an odd composite number in the interval [3, k], one of (hr+2) is odd prime number in the interval [k, 2k-3], where
num(3≤p≤k)=num(2k=(pi+2)+pj)+num(2k=ps+(hr+2)) (5.12.1)
Among:
2k=(pi+2)+pj becomes one of 2k=hk++pd+.
2k=ps+(hr+2) becomes one of 2k=pi++pj+.
(9.8)Note 8: Where does all 2k=ht++hu+ in (4.3.2) come from?
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
Theorem 13: If the conjecture holds for 2n, then 2k=ht++hu+ has the following four sources:
If one of (ps+2) is odd composite, then 2k=(ps+2)+hr becomes one of 2k=ht++hu+.
If one of (ht+2) is odd composite, then 2k=(ht+2)+hu becomes one of 2k=ht++hu+.
If one of (hu+2) is odd composite, then 2k=ht+ (hu+2) becomes one of 2k=ht++hu+.
If one of (pd+2) is odd composite, then 2k=hk+ (pd+2) becomes one of 2k=ht++hu+.
A statement on theorem 17, over.
5.13 Theorem 18: If the conjecture is true at 2n and k (=n+1) is composite, then at least oneof(hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
To prove theorem 18, first quote the related theorem and then prove theorem 18.
Proof: (1) Quote theorem 11 as follows:
Theorem 11: If the 2n conjecture is true, then 2k=pi++pj+ has the following four sources, as long as one of the sources exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
If the Goldbach conjecture holds for 2n, 2k=pi++pj+ has four sources, so long as one source exists, the conjecture will continue to hold for 2k. So, to prove the conjecture, you just need to prove the existence of a source.
Conversely, if the Goldbach conjecture is true at 2n, and if it is not true at 2k, then none of the 4 sources of 2k=pi++pj+ can exist.
As a proof by contradiction, if the conjecture is not valid for 2k , that is,2k=pi++pj+ 4 sources, none of which exist;As long as the contradiction can be found according to rigorous mathematical reasoning, it is proved that 2k=pi++pj+ 4 sources, at least one of the existence. That proves the 2k conjecture. That's the mathematical idea proving theorem 18.
(2) The inference of Hou Shaosheng's theorem is quoted as follows:
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
There is always:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n, q is odd.
pi, pj, ps, pd are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And: pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
Under our theoretical system, if the conjecture is true for 2n, it is accepted that the above (4.0.1), (4.0.2), (4.0.3), (4.0.4) are also true. Therefore, the simultaneous holding of (4.0.1), (4.0.2), (4.0.3), (4.0.4) is also the theoretical basis for our proof of theorem 18, in particular the admission that: 1≤num(2n=pi+pj).
(3) Quote theorem 5 (theinferenceof Hou Shaosheng's theorem at 2k) as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
Quote (4.0.4) as follows.
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
(4.0.4) indicates that hu, hr, are the all odd composite numbers in the interval [n,2n-3].
(4.0.4) Indicates that num(n≤h≤2n-3) is equal to the number of odd composite number hu + the number of odd composite number hr.
If n in (4.0.4) is replaced by k, we get:
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
(4.3.4), is k time, Hou Shaosheng theorem of inference formula.
(4.3.4) It is stated that num(k≤h≤2k-3) is equal to the number of
odd composite number hu+ + the number of odd composite number hr+.
(4)At least one of (hu+2) and (hr+2) is an odd prime number.
Quote theorem 16C as follows:
At least one of the (ht+2) and (hk+2) is an odd prime.
In both (hu+2) and (hr+2), at least one is an odd prime.
(5) Theorem 18: If the conjecture is true at 2n and k (=n+1) is composite, then at least oneof(hr+2) is an odd prime, which guarantees that the conjecture must be true at 2k.
The proof is as follows.
(5.1) Assuming that 2n-1 is an odd prime number, the conjecture must hold for 2k.
If 2n-1 is an odd prime, then it must be: 2k=2(n+1)=3+(2n-1).
So 2k is the sum of the odd prime number 3 and the odd prime number 2n-1, and the conjecture holds for 2k. So let's say that 2n-1 is an odd prime number, so we don't need to talk about it anymore.
Now let's say that 2n-1 is odd composite, and the conjecture must be true.
(5.2) Suppose that 2n-1 is the odd composite number, and the number relationship between the odd composite number h in the interval [n, 2n-3] and [k, 2k-3] is as follows.
A If n is an odd composite number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3).
B If n is an odd prime number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: To the right of the above equation, -1, is to subtract an odd composite number (2n-1) = (2k-3).
C If n is even, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note:To the right of the above equation, -1, is to subtract an odd composite number (2n-1)=(2k-3).
The proof is as follows.
Please refer the reader to the picture below.
[n, 2n-3] has one more integer n than [k, 2k-3], but two fewer
integers: 2n-2, 2n-1.
Note: n+1=k, where K is composite number; 2n-1=2k-3, 2n-1, which is
odd composite number.
n is an even number, an odd prime number, and an odd composite
number, which need to be studied separately.
Since we are dealing with the number of odd composite number h in the intervals [n,2n-3] and [k,2k-3], we have nothing to do with the even numbers n, 2n-2. It has nothing to do with odd prime p.

A If n is odd composite number, since 2n-1 is also odd composite number, the number of odd composite number h in the interval [n,2n-3] and [k,2k-3] is equal; Except for n and (2n-1), these two odd composite numbers, the other odd composite numbers h are the same. so has:
num(n≤h≤2n-3)=num(k≤h≤2k-3).
B If n is an odd prime, since 2n-1 is an odd composite number, then [n,2n-3] is 1 less than the number of odd composite number h in the interval [k,2k-3]; 1, refers to an odd composite number (2n-1); The other odd composite numbers are the same. So there are:
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: To the right of the above equation, -1, is to subtract an odd composite number (2n-1) = (2k-3).
C If n is even, since 2n-1 is an odd composite number, [n,2n-3] is 1 less than the number of odd composite number h in the interval [k,2k-3]; 1, refers to an odd composite number (2n-1); The other odd composite numbers are the same. so has:
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: To the right of the above equation, -1, is to subtract an odd composite number (2n-1) = (2k-3).
A,B,C, three conclusions have been proved.
The following, for A,B,C, 3 conclusions, respectively prove the conjectures are valid.
(5.3) Suppose that 2n-1 is an odd composite number;
A If n is an odd composite number, there must be
num(n
≤
h
≤
2n-3)=num(k
≤
h
≤
2k-3).
Under these conditions, the conjecture must be true.
The proof is as follows.
From num(n≤h≤2n-3)=num(k≤h≤2k-3), there must be:
num(k≤h≤2k-3)
=num(n≤h≤2n-3)
=num(2n=ht+hu)+num(2n=ps+hr) (4.0.4)
=num(2n+2=ht+2+hu)+num(2n+2=ps+2+hr)
=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)). (5.13.1)
That's it :
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
The derivation process of each step above has mathematical axioms, theorems, definitions, and bases, so (5.13.1) must be true!
Our aim is to prove that there must be (2+hr) that is odd prime numbers. Thus 2k=ps+(2+hr), and at least one 2k is the sum of the odd prime ps and the odd prime (2+hr).
The proof is as follows:
Theorem 16C states that at least one of (2+hu) and (2+hr) is an odd prime number.
The following proof by contradiction shows that at least oneof(2+hr) is an odd prime number.
Theorem 16C states that at least one of (ht+2) and (hk+2) is an odd prime number.
In both (hu+2) and (hr+2), at least one is an odd prime.
Suppose each (2+hr) is an odd composite number. According to theorem 16C, at least one of (2+hu) is an odd prime number.
Now analyze (5.13.1) as follows.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
On the left side of the equal sign, num(k≤h≤2k-3) is the number of all the odd composite number h in the interval [k, 2k-3].
(5.13.1), is a mathematical equation, on the right of the equals sign,
num(2k=ht+(2+hu))+ num(2k=ps+(2+hr)),
It must also be the number of all odd composite number h in the interval
[k, 2k-3]. This is a necessary condition for (5.13.1) to be established.
It can be seen that num(2k=ht+(2+hu)), num(2k=ps+(2+hr)), must be the number of partial odd composite numbers h in the interval [k, 2k-3]. Otherwise, it contradicts the mathematical principle that only numbers with the same name can be equal, and only numbers with the same name can be added or subtracted.
num(2k=ht+(2+hu))represents the number of (2k=ht+(2+hu))according to the definition (1.2 Meaning of mathematical symbols in the paper).
number of (2k=ht+(2+hu)) is the number of ht in the interval [3, k]; Of course is also the number of (2+hu) in the interval [k, 2k-3].
Since num(2k=ht+(2+hu))must be the number of partial odd composite numbers h in the interval [k, 2k-3], every (2+hu) must be the odd composite number h in the interval [k, 2k-3]; Otherwise, num(2k=ht+(2+hu)) cannot be the number of odd composite number h in the interval [k, 2k-3].
Each (2+hu) must be an odd composite number h in the interval [k, 2k-3]; Contradicted by the fact that at least oneof(2+hu) is an odd prime.
Therefore, the assumption that every (2+hr) is an odd composite number is not true. So at least oneof(2+hr) is an odd prime.
At least oneof(2+hr) is an odd prime, which guarantees that at 2k, the conjecture must be true.
A If n is an odd composite number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3).
Under these conditions, the conjecture must be true. Proof is finished.
(5.3.1) Answer 9 relevant questions
The following 9 questions, experts and readers, are likely to be asked, and we offer to answer them below.
Problem 1: (2+hr) is an odd prime number, which also contradicts (2+hu), (2+hr), which must both be odd composite numbers. How do you solve it?
Answer 1: If some (2+hr) are odd prime numbers, then 2k=ps+(2+hr), 2k is already the sum of odd prime numbers ps and odd prime numbers (2+hr). 2k=ps+(2+hr), already one of the four sources of 2k=pi++pj+ .
To be more specific, 2k=ps+(2+hr) is the third of the four sources of 2k=pi++pj+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
Theorem 11: The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
At this point, 2k=ps+(2+hr) should be removed from (5.13.1). After copying, transfer to position of(2k=pi++pj+)in (4.3.1), (4.3.3). Make it part of num(2k=pi++pj+). Because 2k=pi++pj+ has four sources, 2k=ps+(2+hr), if k is relatively large, can only be part of num(2k=pi++pj+), not all of it.
2k=pi++pj+ in (4.3.1) and (4.3.3) is formed after the convergence of the four sources of 2k=pi++pj+.
To quote Theorem 5: The inference of Hou Shaosheng's theorem in 2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
Note that we moved 2k=ps+(2+hr) from (5.13.1), copied it, and moved it to position of(2k=pi++pj+)in (4.3.1), (4.3.3). Make it part of
num(2k=pi++pj+). This process, after proving 2k, does not affect the proof that the conjecture is established.
Problem 2: If some (2+hr) are odd composite numbers, then 2k=ps+(2+hr), 2k is already the sum of odd prime ps and odd composite numbers (2+hr). 2k=ps+(2+hr) is already one of 2k=ps++hr+.
To be more specific, 2k=ps+(2+hr) is the second of four sources for 2k=ps++hr+.
Theorem 12: If the conjecture is true for 2n, then 2k=ps++hr+ has four sources:
If one of (pj+2) is an odd composite number, then 2k=pi+(pj+2) becomes one of 2k=ps++hr+;
If one of (hr+2) is an odd composite number, then 2k=ps+(hr+2) becomes one of 2k=ps++hr+;
If one of (ps+2) is an odd prime, then 2k=(ps+2)+hr becomes one of 2k=ps++hr+;
If one of (ht+2) is an odd prime, then 2k=(ht+2)+hu becomes one of 2k=ps++hr+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+ num(2k=ps+(2+hr)) (5.13.1)
If some (2+hr) are odd composites number, 2k=ps+(2+hr) is left in the position of 2k=ps+(2+hr) in (5.13.1) as part of 2k=ps++hr+. The four sources of 2k=ps++hr+, when brought together, replace the position of 2k=ps+(2+hr) in (5.13.1).
Problem 3: If some (ps+2) are odd prime numbers, then 2k=(ps+2)+hr, 2k is already the sum of odd prime numbers (ps+2) and odd composite numbers hr. 2k=(ps+2)+hr, already one of 2k=ps++hr+.
To be more specific, 2k=(ps+2)+hr is the third of the four sources for 2k=ps++hr+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=(ps+2)+hr is retained in the position of 2k=ps+(2+hr) in (5.13.1), becoming one of the four sources of 2k=ps++hr+.
The four sources of 2k=ps++hr+, when brought together, replace the position of 2k=ps+(2+hr) in (5.13.1).
Problem 4: If some (ps+2) are odd composite numbers, then 2k=(ps+2)+hr, 2k is already the sum of odd composite numbers (ps+2) and odd composite numbers hr. 2k=(ps+2)+hr, already one of 2k=ht++hu+.
To be more specific, 2k=(ps+2)+hr is the first of four sources for 2k=ht++hu+.
Theorem 13: If the conjecture holds for 2n, then 2k=ht++hu+ has the following four sources:
If one of (ps+2) is an odd composite number, then 2k=(ps+2)+hr becomes one of 2k=ht++hu+.
If one of (ht+2) is an odd composite number, then 2k=(ht+2)+hu becomes one of 2k=ht++hu+.
If one of (hu+2) is odd composite number, then 2k=ht+(hu+2) becomes one of 2k=ht++hu+.
If one of (pd+2) is odd composite number, then 2k=hk+(pd+2) becomes one of 2k=ht++hu+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=(ps+2)+hr is retained in the position of 2k=ht+(2+hu) in (5.13.1), becoming one of the four sources of 2k=ht++hu+.
The four sources of 2k=ht++hu+, when aggregated, replace the position of 2k=ht+(2+hu) in (5.13.1).
Problem 5: If some (2+hu) are odd prime numbers, then 2k=ht+(2+hu), 2k is already the sum of odd composite numbers ht and odd prime numbers (2+hu). 2k=ht+(2+hu) is already one of 2k=hk++pd+.
To be more specific, 2k=ht+(2+hu) is the second of the four sources of 2k=hk++pd+.
Theorem 14: If the conjecture is true for 2n, 2k=hk++pd+ has the following four sources:
If one of (pi+2) is odd composite , then 2k=(pi+2)+pj becomes one of 2k=hk++pd+;
If one of (hu+2) is an odd prime, then 2k=ht+(hu+2) becomes one of 2k=hk++pd+;
If one of (hk+2) is odd composite, then 2k=(hk+2)+pd becomes one of 2k=hk++pd+;
If one of (pd+2) is an odd prime, then 2k=hk+(2+pd) becomes one of 2k=hk++pd+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=ht+(2+hu) should be removed from (5.13.1), copied, and transferred to(2k=hk++pd+) in (4.3.2), (4.3.3). Make it part of
num(2k=hk++pd+). Because 2k=hk++pd+ has four sources, 2k=ht+(2+hu), in the case of k is relatively large, can only be a part of num(2k=hk++pd+), not all.
2k=hk++pd+ in (4.3.2) and (4.3.3) is formed after the convergence of four sources of 2k=hk++pd+.
To quote Theorem 5: The inference of Hou Shaosheng's theorem in 2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
Problem 6: If some (2+hu) are odd composite numbers, then 2k=ht+(2+hu), 2k is already the sum of odd composite numbers ht and odd composite numbers (2+hu). 2k=ht+(2+hu), already one of 2k=ht++hu+.
Specifically, 2k=ht+(2+hu) is the third of the four sources for 2k=ht++hu+.
Theorem 13: If the conjecture holds for 2n, then 2k=ht++hu+ has the following four sources:
If one of (ps+2) is odd composite number, then 2k=(ps+2)+hr becomes one of 2k=ht++hu+.
If one of (ht+2) is odd composite number, then 2k=(ht+2)+hu becomes one of 2k=ht++hu+.
If one of (hu+2) is odd composite number, then 2k=ht+(hu+2) becomes one of 2k=ht++hu+.
If one of (pd+2) is odd composite number,then 2k=hk+(pd+2) becomes one of 2k=ht++hu+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+ num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=ht+(2+hu) should be retained in the position of 2k=ht+(2+hu) in (5.13.1). Also copy, move 2k=ht+(2+hu) to the position 2k=ht++hu+ in (4.3.2), (4.3.4) and become part of num(2k=ht++hu+). Because 2k=ht++hu+ has four sources, 2k=ht+(2+hu), in the case of k is relatively large, can only be a part of num(2k=ht++hu+), not all.
To quote Theorem 5: The inference of Hou Shaosheng's theorem in 2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
2k=ht++hu+ in (4.3.2) and (4.3.4) is formed after the convergence of four sources of 2k=ht++hu+. When the four sources of 2k=ht++hu+ are aggregated, the position of 2k=ht+(2+hu) in (5.13.1) is replaced.
Problem 7: If some (ht+2) are odd prime numbers, then 2k=(ht+2)+hu, 2k is already the sum of odd prime numbers (ht+2) and odd composite numbers hu. 2k=(ht+2)+hu, already one of 2k=ps++hr+.
Specifically, 2k=(ht+2)+hu is the fourth of the four sources for 2k=ps++hr+.
Theorem 12: If the conjecture is true for 2n, then 2k=ps++hr+ has four sources:
If one of (pj+2) is an odd composite number, then 2k=pi+(pj+2) becomes one of 2k=ps++hr+;
If one of (hr+2) is an odd composite number, then 2k=ps+(hr+2) becomes one of 2k=ps++hr+;
If one of (ps+2) is an odd prime, then 2k=(ps+2)+hr becomes one of 2k=ps++hr+;
If one of (ht+2) is an odd prime, then 2k=(ht+2)+hu becomes one of 2k=ps++hr+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=(ht+2)+hu should be retained in the position of 2k=ps+(2+hr) in (5.13.1). Also copy, move 2k=(ht+2)+hu to 2k=ps++hr+ in (4.3.1), (4.3.4) and become part of num(2k=ps++hr+). Because 2k=ps++hr+ has four sources, 2k=(ht+2)+hu, if k is larger, can only be part of
num(2k=ps++hr+), not all of it.
To quote Theorem 5: The inference of Hou Shaosheng's theorem in 2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
2k=ps++hr+ in (4.3.1) and (4.3.4) is formed after the convergence of four sources of 2k=ps++hr+. When the four sources of 2k=ps++hr+ are aggregated, the position of 2k=ps+(2+hr) in (5.13.1) is replaced.
Problem 8: If some (ht+2) are odd composite numbers, then 2k=(ht+2)+hu, 2k is already the sum of odd composite numbers (ht+2) and odd composite numbers hu. 2k=(ht+2)+hu, already one of 2k=ht++hu+.
To be more specific, 2k=(ht+2)+hu is the second of four sources for 2k=ht++hu+.
Theorem 13: Assuming the conjecture is true for 2n, 2k=ht++hu+ has the following four sources:
If one of (ps+2) is odd composite number, then 2k=(ps+2)+hr becomes one of 2k=ht++hu+.
If one of (ht+2) is odd composite number, then 2k=(ht+2)+hu becomes one of 2k=ht++hu+.
If one of (hu+2) is odd composite number, then 2k=ht+(hu+2) becomes one of 2k=ht++hu+.
If one of (pd+2) is odd composite number, then 2k=hk+(pd+2) becomes one of 2k=ht++hu+.
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+ num(2k=ps+(2+hr)) (5.13.1)
At this time, 2k=(ht+2)+hu should be retained in the position of 2k=ht+(2+hu) in (5.13.1). Also copy, move 2k=(ht+2)+hu to 2k=ht++hu+ in (4.3.2), (4.3.4) to become part of num(2k=ht++hu+). Because 2k=ht++hu+ has four sources, 2k=(ht+2)+hu, in the case of k is relatively large, can only be a part of num(2k=ht++hu+), not all.
To quote Theorem 5: The inference of Hou Shaosheng's theorem in 2k is as follows:
num(3≤p≤k)=num(2k=pi++pj+)+num(2k=ps++hr+). (4.3.1)
num(3≤h≤k)=num(2k=ht++hu+)+num(2k=hk++pd+). (4.3.2)
num(k≤p≤2k-3)=num(2k=pi++pj+)+num(2k=hk++pd+). (4.3.3)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
2k=ht++hu+ in (4.3.2) and (4.3.4) is formed after the convergence of four sources of 2k=ht++hu+. When the four sources of 2k=ht++hu+ are aggregated, the position of 2k=ht+(2+hu) in (5.13.1) is replaced.
Question 9: With 2k=ht++hu+ replace (5.13.1) 2k=ht+(2+hu),With 2k=ps++hr+ to replace (5.13.1) 2k=ps+(2+hr) after, (5.13.1) will evolve into (4.3.4).
num(k≤h≤2k-3)=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.1)
num(k≤h≤2k-3)=num(2k=ht++hu+)+num(2k=ps++hr+). (4.3.4)
The evolution process described above is extremely complex. It is a process of great differentiation, great reorganization, recombination, and reintegration. Generally speaking, from the great differentiation to the return of the team, the following eight situations are involved.
A: From 2n=pi+pj, to 2n+2=pi+2+pj, to 2k=(pi+2)+pj; It must be studied separately that (pi+2) is an odd prime number; (pi+2) is an odd composite number.
If pi+2 is an odd prime; 2k=(pi+2)+pj, will return to 2k=pi++pj+.
If pi+2 is odd composite; 2k=(pi+2)+pj, will return to 2k=hk++pd+.
B: From 2n=pi+pj, to 2n+2=pi+2+pj, to 2k=pi+(2+pj). It is necessary to study separately that (2+pj) is an odd prime number and (2+pj) is an odd composite number.
If (2+pj) is an odd prime; 2k=pi+(2+pj), will return to 2k=pi++pj+.
If (2+pj) is odd composite number; 2k=pi+(2+pj), will return to 2k=ps++hr+.
C: From 2n=ps+hr, to 2n+2=ps+2+hr, to 2k= (ps+2) +hr. It must be studied separately that (ps+2) is an odd prime number and (ps+2) is an odd composite number.
If (ps+2) is an odd prime; 2k=(ps+2)+hr, will return to 2k=ps++hr+.
If (ps+2) is odd composite number; 2k=(ps+2)+hr, will return to 2k=ht++hu+.
D: From 2n=ps+hr, to 2n+2=ps+2+hr, to 2k=ps+(2+hr). It must be studied separately that (2+hr) is an odd prime number and (2+hr) is an odd composite number.
If (2+hr) is an odd prime; 2k=ps+(2+hr), will return to 2k=pi++pj+.
If (2+hr) is odd composite number; 2k=ps+(2+hr), will return to 2k=ps++hr+.
E: From 2n=ht+hu, to 2n+2=ht+2+hu, to 2k= (ht+2) +hu. It must be studied separately that (ht+2) is an odd prime number and (ht+2) is an odd composite number.
If (ht+2) is an odd prime; 2k=(ht+2)+hu, will return to 2k=ps++hr+.
If (ht+2) is an odd composite number;2k=(ht+2)+hu,will return to 2k=ht++hu+.
F: From 2n=ht+hu, to 2n+2=ht+2+hu, to 2k=ht+(2+hu). We must study separately that (2+hu) is an odd prime number and (2+hu) is an odd composite number.
If (2+hu) is an odd prime; 2k=ht+(2+hu), will return to 2k=hk++pd+.
If (2+hu) is an odd composite number; 2k=ht+(2+hu), will return to 2k=ht++hu+.
G: From 2n=hk+pd, to 2n+2=hk+2+pd, to 2k=(hk+2)+pd. It must be studied separately that (hk+2) is an odd prime number and (hk+2) is an odd composite number.
If (hk+2) is an odd prime; 2k=(hk+2)+pd, will return to 2k=pi++pj+.
If (hk+2) is an odd composite number; 2k=(hk+2)+pd, will return to 2k=hk++pd+.
H: From 2n=hk+pd, to 2n+2=hk+2+pd, to 2k=hk+(2+pd). It must be studied separately that (2+pd) is an odd prime number and (2+pd) is an odd composite number.
If (2+pd) is an odd prime; 2k=hk+(2+pd), will return to 2k=hk++pd+.
If (2+pd) is an odd composite number; 2k=hk+(2+pd), will return to 2k=ht++hu+.
In the above 8 cases, return to 2k=pi++pj+, in 4 cases. In these four cases, there are four sources of 2k=pi++pj+, and only these four sources.
Back at 2k=ps++hr+, there are 4 scenarios. In these four cases, there are four sources of 2k=ps++hr+, and only these four sources.
Back to 2k=ht++hu+ , there are 4 scenarios. In these four cases, there are four sources of 2k=ht++hu+, and only these four sources.
Back to 2k=hk++pd+, there are 4 cases. These four cases, previously called 2k=hk++pd+ 4 sources, and only these 4 sources.
Question 10: The above answers to the nine questions have been very comprehensive. The answer to 9 questions, essentially applicable: Theorem 17, theorem 18, theorem 19 after the proof of the description. In mathematics, we often use empathy to prove, not to prove. Therefore, after the proof of theorem 17, after the proof of B and C in theorem 18, after the proof of theorem 19, the answers to the above nine questions will not be repeated.
After you have reviewed the proof of theorem 17, the proof of A,B, and C in theorem 18, and the proof of theorem 19, if you still have questions, please look at the answers to the above 9 questions.
(5.4) Suppose that 2n-1 is an odd composite number.
B If n is an odd prime number, there must be
num(n
≤
h
≤
2n-3)=num(k
≤
h
≤
2k-3)-1.
Note: On the right side of the formula, -1, is to subtract an odd composite number (2n-1) = (2k-3).
Under these conditions, the conjecture must be true.
The proof is as follows.
From num(n≤h≤2n-3)=num(k≤h≤2k-3)-1, we can get:
num(k≤h≤2k-3)-1
=num(n≤h≤2n-3)
=num(2n=ht+hu)+num(2n=ps+hr) (4.0.4)
=num(2n+2=ht+2+hu)+num(2n+2=ps+2+hr)
=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)). (5.13.2)
that's it
num(k≤h≤2k-3)-1=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.2)
The derivation process of each step above has mathematical axioms, theorems, definitions, and bases, so (5.13.2) must be true!
Our aim is to prove that there must be (2+hr) that is odd prime numbers. 2k=ps+(2+hr), 2k is the sum of the odd prime ps and the odd prime (2+hr).
The proof is as follows:
Theorem 16C states that at least one of (2+hu) and (2+hr) is an odd
prime number.
The following proof by contradiction shows that at least one (2+hr)
is an odd prime number.
Suppose each (2+hr) is an odd composite number.
Theorem 16C states that at least one of (2+hu) is an odd prime number.
Now analyze (5.13.2) as follows.
On the left side of the equal sign, num(k≤h≤2k-3) is the number of
all the odd composite number h in the interval [k, 2k-3].
Therefore, num(k≤h≤2k-3)-1 is the number of partial odd composite
number h in the interval [k, 2k-3].
(5.13.2), is a mathematical equation, on the right of the equals sign,
num(2k=ht+(2+hu))+num(2k=ps+(2+hr)),
it must also be the number of partial odd composite numbers h in the interval [k, 2k-3]. This is a necessary condition for the establishment of (5.13.2).
It can be seen that num(2k=ht+(2+hu)), num(2k=ps+(2+hr)), must be the number of partial odd composite numbers h in the interval [k, 2k-3]. Otherwise, it contradicts the mathematical principle that only numbers with the same name can be equal, and only numbers with the same name can be added or subtracted.
num(2k=ht+(2+hu)) represents the number of (2k=ht+(2+hu)) according to the definition (1.2 Meaning of mathematical symbols in the paper).
Number of (2k=ht+(2+hu)) is the number of ht in the interval [3, k]; Of course is also the number of (2+hu)in the interval [k, 2k-3].
Since num(2k=ht+(2+hu)) must be the number of partial odd composite numbers h in the interval [k, 2k-3],therefore, every (2+hu) must be the odd composite number h in the interval [k,2k-3]; Otherwise, num(2k=ht+(2+hu)) cannot be the number of odd composite number h in the interval [k, 2k-3].
Each (2+hu) must be an odd composite number h in the interval [k, 2k-3]; Contradicted by the fact that at least one of (2+hu) is an odd prime.
Therefore, the assumption that every (2+hr) is an odd composite number is not true. So at least one of (2+hr) is an odd prime.
At least one of (2+hr) is an odd prime, which guarantees that at 2k, the conjecture must be true.
This paper is accompanied by 8 mathematical examples. In every instance, at least one of (2+hr) is an odd prime p.
Some readers will ask: If a certain (2+hr) is an odd prime number, it also contradicts that every (2+hr) must be an odd composite number. What do you do?
The answer is as follows: At this point, 2k=ps+(2+hr), 2k is already the sum of the odd prime ps and the odd prime (2+hr), 2k, the conjecture is established.
At this point, 2k=ps+(2+hr) should be removed from (5.13.2). After copying, transfer to position of(2k=pi++pj+) in (4.3.1), (4.3.3). Make it part of num(2k=pi++pj+).
Regarding this issue, (4.3.1) answer 9 relevant questions with detailed answers. Please refer to.
B If n is an odd prime number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: On the right side of the formula, -1, is to subtract an odd
composite number (2n-1) = (2k-3).
Under these conditions, the conjecture must be true.
End of proof.
(5.5) Suppose that 2n-1 is an odd composite number.
C If n is even, there must be num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: On the right side of the formula ,-1, is to subtract an odd
composite number (2n-1) = (2k-3).
Under these conditions, the conjecture must be true.
The proof is as follows.
From num(n≤h≤2n-3)=num(k≤h≤2k-3)-1, there must be:
num(k≤h≤2k-3)-1
=num(n≤h≤2n-3)
=num(2n=ht+hu)+num(2n=ps+hr) (4.0.4)
=num(2n+2=ht+2+hu)+num(2n+2=ps+2+hr)
=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)). (5.13.3)
That Is:
num(k≤h≤2k-3)-1=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.3)
The derivation process of each step above has mathematical axioms, theorems, definitions, and bases, so (5.13.3) must be true!
Our aim is to show that at least one of (2+hr) is an odd prime number.
2k=ps+(2+hr), 2k is the sum of the odd prime ps and the odd prime (2+hr).
The proof is as follows:
Theorem 16C states that at least one of (2+hu) and (2+hr) is an odd
prime number.
The following proof by contradiction shows that at least one of (2+hr)
is an odd prime number.
Suppose each (2+hr) is an odd composite number.
Theorem 16C states that at least one of (2+hu) is an odd prime number.
Now analyze (5.13.3) as follows.
On the left side of the equal sign, num(k≤h≤2k-3) is the number of all the odd composite number h in the interval [k, 2k-3].
Therefore, num(k≤h≤2k-3)-1 is the number of partial odd composite number h in the interval [k, 2k-3].
num(k≤h≤2k-3)-1=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.3)
(5.13.3), is a mathematical equation,
num(2k=ht+(2+hu)+num(2k=ps+(2+hr))
It must also be the number of partial odd composite numbers h in the interval [k, 2k-3]. This is a necessary condition for the establishment of (5.13.3).
It can be seen that num(2k=ht+(2+hu)),num(2k=ps+(2+hr)), must be the
number of partial odd composite numbers h in the interval [k, 2k-3]. Otherwise, it contradicts the mathematical principle that only
numbers with the same name can be equal, and only numbers with the same
name can be added or subtracted.
num(2k=ht+(2+hu)) represents the number of (2k=ht+(2+hu)) according to the definition (1.2 Meaning of mathematical symbols in the paper).
number of (2k=ht+(2+hu)) is the number of ht in the interval [3, k]; Of course is also the number of (2+hu) in the interval [k, 2k-3].
Since num(2k=ht+(2+hu)) must be the number of partial odd composite numbers h in the interval [k,2k-3], therefore, every (2+hu) must be the odd composite number h in the interval [k,2k-3]; Otherwise, num(2k=ht+(2+hu)) cannot be the number of odd composite number h in the interval [k, 2k-3].
Each (2+hu) must be an odd composite number h in the interval
[k, 2k-3]; Contradicted by the fact that at least one (2+hu) is an odd prime.
Therefore, the assumption that every (2+hr) is an odd composite number is not valid.
So at least one of (2+hr) is an odd prime.
At least one of (2+hr) is an odd prime, which guarantees that at 2k, the conjecture must be true.
C If n is even, there must be num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: On the right side of the formula, -1, is to subtract an odd composite number (2n-1) = (2k-3).
Under these conditions, the conjecture must be true.
Theorem 18, end of proof
This paper is accompanied by 8 mathematical examples. In every instance, at least one of (2+hr) is an odd prime p.
Experts and readers are welcome to test theorem 18 with their own examples.
(5.5.1) : Answer questions that readers may have.
Question: 2k=ps+(2+hr), (2+hr) is an odd prime number, does it not
contradict that every (2+hu), every (2+hr), must be an odd composite number in the interval [k,2k-3]?
Answer the question: Contradictory. To resolve this contradiction, when (2+hr) is an odd prime, we need to move 2k=ps+(2+hr) out of (5.13.3); Move to the (2k=pi++pj+)location of (4.3.1), (4.3.3), making it part of (2k=pi++pj+).
Regarding this issue, (5.3.1) answer 9 relevant questions with detailed answers. Please refer to.
(5.6) : Appendix: The original text of proof by contradiction in a small dictionary of mathematics, for readers' reference.
The original text of the proof by contradiction in the mathematical dictionary is as follows:
proof by contradiction Proof by contradiction is the equivalent proposition of proving a proposition - the inverse negative proposition. Thus the original proposition is proved indirectly. The general steps of proof by contradiction are: (1) Assume that the opposite of the conclusion is true; ② Draw wrong conclusions based on reason;③ To assert the opposite of the conclusion is wrong; ④ To assert the positive side of the conclusion is correct.
If there is only one case on the opposite side of the conclusion, it is sufficient to conclude that this case is not true. This proof by contradiction is called reduction;
If there is more than one case on the opposite side of the conclusion, it is necessary to refute each case one by one in order to affirm the positive truth of the conclusion. This kind of proof by contradiction is called exhaustion.
Example 1 We know that in △ABC, ∠B≠∠C. Seek confirmation: AB≠AC.
Proof (Use the reductive method) : If AB≠AC is not true, then AB=AC. Then ∠B=∠C, and the known contradiction.So AB≠AC.
(So much for quoting the original dictionary of mathematics).
Any expert or reader who cannot find a specific error in the above proof should admit that we have proved the conjecture. Please recommend this proof to a famous magazine for publication and make your contribution to the proof of Goldbach's conjecture.
(5.7) : Summary of theorem 18 proof work:
5.13 This part is to prove theorem 18: If the conjecture is true for 2n and k (=n+1) is composite, then at least one of (hr+2) is an odd prime, which guarantees that the conjecture is true for 2k.
We want to complete the proof of theorem 18 using the formula (4.0.4).
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr) (4.0.4)
num(k≤h≤2k-3)-1=num(2k=ht+(2+hu))+num(2k=ps+(2+hr)) (5.13.3)
There are two basic reasons for choosing (4.0.4). The first, (4.0.4) exists 2n=ps+hr, from which we get 2k=ps+(2+hr). The second, hu and hr, are odd composite numbers. However, theorem 16C has proved that at least one of (2+hu),(2+hr), is an odd prime. Moreover, when (2+hu) is an odd prime, it is not satisfied (5.13.3). These basic conditions provide us with the most favorable basis for proving theorem 18.
In order to prove theorem 18, the relationship between
num(n≤h≤2n-3) and num(k≤h≤2k-3) must be studied. Otherwise, there's no proof to begin with.
In (5.1), we prove that if 2n-1 is an odd prime number, the conjecture must be true for 2k.
In (5.2), we first assume that 2n-1 is odd composite number. Then we assume that n is odd composite number, n is odd prime, and n is even. The following three relationships are obtained.
A If n is an odd composite number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3).
B If n is an odd prime number, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: On the right side of the formula, -1, is to subtract an odd composite number (2n-1) = (2k-3).
C If n is even, there must be
num(n≤h≤2n-3)=num(k≤h≤2k-3)-1.
Note: On the right side of the formula, -1, is to subtract an odd composite number (2n-1) = (2k-3).
For the above three relations, it is necessary to prove separately that (2+hr) is an odd prime number. The whole proof of 5.13 was carried out under the guidance of this mathematical thought.
For the above three relations, we have separately proved that (2+hr) exists as odd prime numbers. So there is at least one 2k=ps+(2+hr), 2k is the sum of the odd prime ps and the odd prime (2+hr), 2k, the conjecture is established.
So the proof of theorem 18 is complete.
Theorem 18, finished proving.
Theorem 18 proves that if the conjecture is true for 2n and k (=n+1) is composite number, then at least one of (hr+2) is an odd prime, which guarantees that the conjecture is true for 2k.
Theorem 18 proves that a source of 2k=pi++pj+ must exist. One source is: if one of (hr+2) is an odd prime, then 2k=ps+(2+hr) is a source of 2k=pi++pj+. Theorem 18 affirms that there must be a number (hr+2) that is an odd prime, which guarantees that the conjecture holds at 2k.
Similar to theorem 18, Theorem 19 will prove that another source of 2k=pi++pj+ must exist. Another source is: if one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+. Theorem 19 affirms that there must be a number (hk+2) that is an odd prime, which guarantees that the conjecture holds for 2k.
5.14 Theorem 19: If the conjecture is true for 2n and k (=n+1) is a composite number h, then at least one of (hk+2) is an odd prime p, which guarantees that the conjecture is true for 2k.
Proof: (1) Quote theorem 11 as follows:
Theorem 11: If the 2n conjecture is true, then 2k=pi++pj+ has the
following four sources, as long as one of the sources exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
If the Goldbach conjecture holds for 2n, 2k=pi++pj+ has four sources, so long as one source exists, the conjecture will continue to hold for 2k. So, to prove the conjecture, you just need to prove the existence of a source.
Conversely, if the Goldbach conjecture is true at 2n, and if it is not true at 2k, then none of the four sources of 2k=pi++pj+ can exist.
As a proof by contradiction, if the conjecture is not valid when 2k is assumed, it is assumed that: 2k=pi++pj+ 4 sources, none of which exist; As long as the contradiction can be found according to rigorous mathematical reasoning, it is proved that 2k=pi++pj+ 4 sources, at least one of the existence. That proves the 2k conjecture. That's the mathematical idea behind proving theorem 19.
(2) The inference of Hou Shaosheng's theorem is as follows:
About the formula:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
There is always:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Among them, the convention is: 3≤q≤n,q is odd.
pi, pj, ps, pd, are all odd prime numbers; ht, hu, hr, hk are all odd composite numbers.
And pi≤pj, ht≤hu, ps≤hr, hk≤pd.
pi, ht, ps, hk, ∈[3,n]; pj, hu, hr, pd∈[n,2n-3].
Under our theoretical system, if the conjecture is true for 2n, it is accepted that the above (4.0.1), (4.0.2), (4.0.3), (4.0.4) are also true. Therefore, the simultaneous holding of (4.0.1), (4.0.2), (4.0.3), and (4.0.4) is also the theoretical basis for our proof of theorem 19, in particular the admission that 1≤num(2n=hk+pd).
(3)It is only necessary to prove that when k is a composite number h, Goldbach's conjecture holds for 2k.
Since 2k=k+k, if k is an odd prime p,2k is already the sum of two odd prime numbers, and the conjecture naturally holds.
Therefore, as long as it can be shown that k is a composite number h, the 2k conjecture must hold, then Goldbach's conjecture must hold.
k is a composite number h, and in general, it is necessary to distinguish between k being an odd composite number h and k being an even composite number h.
(4) At least one of (ht+2) and (hk+2) is an odd prime p.
Quote theorem 16C as follows:
In both (ht+2) and (hk+2), at least one is an odd prime p.
In both (hu+2) and (hr+2), at least one is an odd prime p.
(5)If k is a composite number h, then at least oneof(hk+2) is an odd prime p, ensuring that the conjecture holds for 2k.
In the following proof, it is necessary to distinguish between k being an even composite number h and k being an odd composite number h, given separately prove it.
(5.1) If k is aoddcomposite number h, then at least oneof(hk+2) is an odd prime p, ensuring that the conjecture holds for 2k.
Specific proof is as follows:
Quote (4.0.2) below.
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd). (4.0.2)
By assuming that the conjecture is true for 2n, we admit that (4.0.2)
is true.
(4.0.2) is the object of our next study.
Since k (=n+1) is an odd composite number, n is an even number. It can
be obtained from (4.0.2) :
num(3≤h≤k)
=num(3≤h≤n)+1
=num(2n=ht+hu)+num(2n=hk+pd)+1
=num(2n+2=ht+2+hu)+num(2n+2=hk+2+pd)+1
=num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd)+1 (5.14.1)
That is:
num(3≤h≤k)=num(2k=(ht+2)+hu)+ num(2k=(hk+2)+pd)+1 (5.14.1)
Note: To the right of the above formula ,+1, is to add an odd composite number k (=n+1), K of course in the interval [3, k].
Each of these steps is based on a mathematical definition, or a mathematical axiom, or a mathematical theorem. So (5.14.1) must be true.
Now analyze (5.14.1) as follows:
num(3≤h≤k) is the number of odd composite number h in the interval [3, k].
Because (5.14.1) is true, according to our convention, (ht+2)≤hu, (hk+2)≤pd, so in (5.14.1), (ht+2), (hk+2), are within the interval [3, k].
hu, pd, all in the range [k, 2k-3].
hu, is odd composite number; pd is an odd prime number.
ht, hk, are both odd composite numbers, both in the interval [3, n].
(ht+2), (hk+2), whether the odd prime number p or the odd composite number h, is what needs to be discussed below.
The following, through the way of debating questions and answers, proves that 2k, Goldbach conjecture must be true.
Problem 1: If there is a (hk+2 ) is an odd prime p, Goldbach's conjecture is established.
Theorem 16C states that at least one of (ht+2) and (hk+2) is an odd prime p.
If at least one of (hk+2) is an odd prime p, then the Goldbach conjecture holds for 2k.
This is because, in (2k=(hk+2)+pd), there is at least one 2k, which is already the sum of the odd prime (hk+2) and the odd prime pd. So at 2k, Goldbach's conjecture is established.
If there is not at least one of (hk+2) that is an odd prime p, then every (hk+2) is an odd composite number h.
Now, under the assumption that each (hk+2) is an odd composite number h, we will continue our argumentative question and answer.
Problem 2: Under the assumption that every (hk+2) is an odd composite number h, at least one of (ht+2) is an odd prime number p. Theorem 16C states that at least one of (ht+2) and (hk+2) is an odd prime p.
If every (hk+2) is an odd composite number h, then at least one of (ht+2) is an odd prime number p.
Next, we continue our argumentative question and answer under the assumption that every (hk+2) is an odd composite number h and at least one of (ht+2) is an odd prime number p.
Problem3: If every (hk+2) is an odd composite number h and at least oneof(ht+2) is an odd prime number p, does (5.14.1) still hold?
num(3≤h≤k)=num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd)+1 (5.14.1)
(5.14.1), is a mathematical equation, both sides of the equal sign, should have the same quantity, the same name of things.
On the left side of the equal sign, num(3≤h≤k) is the number of odd composite number h in the interval [3, k].
To the right of the equal sign, num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd)+1 must also be the number of odd composite numbers h in the interval
[3, k].
It follows that num(2k=(ht+2)+hu), num(2k=(hk+2)+pd), 1, must all be the number of odd composite numbers h in the interval [3, k].
Conversely, if num(2k=(ht+2)+hu), num(2k=(hk+2)+pd), 1, one is not
the number of odd composite number h in the interval [3, k], their sum, cannot be the number of odd composite numbers h in the interval [3, k].
Since it is assumed that every (hk+2) is an odd composite number h in the interval [3, k], according to the definition (1.2 Meaning of mathematical notation in the paper), num (2k=(hk+2)+pd) represents the number of odd composite numbers (hk+2) in the interval [3, k].
Now note: to the right of the above formula, +1, is to add an odd composite number k (=n+1), K of course in the interval [3, k].
It can be seen from the note that 1 refers to an odd composite number k (=n+1), and K is of course in the interval [3, k]. Thus, 1 is the number of odd composite number h in the interval [3, k].
Since num (2k=(ht+2)+hu) must be the number of odd composite numbers h in the interval [3, k],therefore, every (ht+2) must be the odd composite number h in the interval [3, k].
Conversely,If there is a (ht+2) that is not an odd composite number h in the interval [3, k], According to the definition (1.2 Meaning of mathematical notation in the paper), num (2k=(ht+2)+hu) is not the number of odd composite numbers h in the interval [3, k].
Note that every (ht+2) must be an odd composite number h in the interval [3, k], contradicting the assumption that at least oneof(ht+2) is an odd prime number p.
Above, we derive the contradiction under the assumption that every (hk+2) is an odd composite number h and at least oneof(ht+2) is an odd prime number p.
This proves that the assumption that every (hk+2) is an odd composite number h, and at least oneof(ht+2) is an odd prime number p, is not valid! Since at least one of (hk+2), (ht+2) is an odd prime p,thereforeat least one of (hk+2) is an odd prime p. So at 2k, Goldbach's conjecture works.
The main logical procedure for proving our conjecture is to assume that every (hk+2) is an odd composite number h, and at least oneof(ht+2) is an odd prime number p, and then derive the contradiction, so the hypothesis cannot be established. And since at least one of (hk+2), (ht+2) is an odd prime p,therefore,at least one of (hk+2) is an odd prime p. So at 2k, Goldbach's conjecture works.
Above, we have proved that Goldbach's conjecture holds when k is a odd composite number h.
It is shown below that if k is an even composite number h, Goldbach's conjecture holds.
(5.2) If k is an even composite number h, then at least oneof(hk+2) is an odd prime p, in 2k, the conjecture must hold.
Specific proof is as follows:
Quote (4.0.2) below.
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
By assuming that the conjecture is true for 2n, we admit that (4.0.2) is true.
(4.0.2) is the object of our next study.
Since k(=n+1) is an even composite number, from (4.0.2):
num(3≤h≤k)
=num(3≤h≤n)
=num(2n=ht+hu)+num(2n=hk+pd)
=num(2n+2=ht+2+hu)+num(2n+2=hk+2+pd)
=num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd) (5.14.2)
that's it
num(3≤h≤k)=num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd) (5.14.2)
Each of these steps is based on a mathematical definition, or a mathematical axiom, or a mathematical theorem. So (5.14.2) must be true.
Now analyze (5.14.2) as follows:
num(3≤h≤k) is the number of odd composite numbers h in the interval
[3, k].
Because (5.14.2) is true, and because we agree that (ht+2)≤hu; (hk+2)
≤pd; So in (5.14.2), (ht+2), (hk+2), are all in the interval [3, k].
hu, pd, all in the interval[k, 2k-3].
hu, is odd composite number; pd is an odd prime number.
ht, hk, are both odd composite numbers, both in the interval [3, n].
(ht+2), (hk+2), whether it is odd prime number or odd composite number, is what needs to be discussed below.
The following, through the way of debating questions and answers, proves that 2k, Goldbach conjecture must be true.
Problem 1: If there is oneof(hk+2)is an odd prime number, Goldbach's conjecture is established.
If at least one of(hk+2) is an odd prime, then the Goldbach conjecture holds for 2k.
This is because 2k=(hk+2)+pd, with at least one 2k, is already the sum of the odd prime number (hk+2) and the odd prime number pd. So at 2k, Goldbach's conjecture is established.
If there is not at least one of (hk+2) that is an odd prime, then every (hk+2) is an odd composite number.
Now, under the assumption that each (hk+2) is an odd composite number, we will continue our argumentative question and answer.
Problem 2: Under the assumption that every (hk+2) is an odd composite number, at least one of (ht+2) is an odd prime number.
Theorem 16C states that at least one of (ht+2) and (hk+2) is an odd prime p.
Since every (hk+2) is assumed to be an odd composite number h,therefore,at least one of (ht+2) is an odd prime number p.
Next, we continue our argumentative question and answer under the assumption that every (hk+2) is an odd composite number h and at least one of (ht+2) is an odd prime number p.
problem3: If every (hk+2) is an odd composite number h and at least oneof(ht+2) is an odd prime number p, does (5.14.2) still hold?
num(3≤h≤k)=num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd) (5.14.2)
(5.14.2), is a mathematical equation, both sides of the equal sign, should have the same quantity, the same name of things.
On the left side of the equal sign, num(3≤h≤k) is the number of odd composite numbers h in the interval [3, k].
To the right of the equal sign, num(2k=(ht+2)+hu)+num(2k=(hk+2)+pd) must also be the number of odd composite numbers h in the interval
[3, k].
It follows that num(2k=(ht+2)+hu),num(2k=(hk+2)+pd), must be the number of odd composite numbers h in the interval [3, k].
Conversely, if num(2k=(ht+2)+hu), num(2k=(hk+2)+pd), one of them is not the number of odd composite numbers h in the interval [3, k], their sum, cannot be the number of odd composite numbers h in the interval
[3, k].
Since it is assumed that every (hk+2) is an odd composite number h in the interval [3, k],therefore, num (2k=(hk+2)+pd) is the number of odd composite numbers (hk+2) in the interval [3, k], according to the definition (meaning of mathematical notation in 1.2 paper).
Since num (2k=(ht+2)+hu) must be the number of odd composite numbers h in the interval [3, k], therefore,every (ht+2) must be the odd composite number h in the interval [3, k].
Conversely, if there is a (ht+2) that is not an odd composite number h in the interval [3, k], num (2k=(ht+2)+hu) is not the number of odd composite numbers h in the interval [3, k] according to the definition (meaning of mathematical notation in 1.2 paper).
Note that every (ht+2) must be an odd composite number h in the interval [3, k], contradicting the assumption that at least oneof(ht+2) is an odd prime number p.
Above, we derive the contradiction under the assumption that every (hk+2) is an odd composite number h and at least oneof(ht+2) is an odd prime number p.
This proves that the assumption that every (hk+2) is an odd composite numbersh, and at least oneof(ht+2) is an odd prime number p, is not valid! Since at least one of (hk+2), (ht+2) is an odd prime p,therefore ,at least one of (hk+2) is an odd prime p. So at 2k, Goldbach's conjecture works.
The main logical procedure for proving our conjecture is to assume that every (hk+2) is an odd composite numbersh, and at least oneof(ht+2) is an odd prime number p, and then derive the contradiction, so the hypothesis cannot be established. And since at least one of (hk+2), (ht+2) is an odd prime p, therefore,at least one of (hk+2) is an odd prime p. So at 2k, Goldbach's conjecture works.
This proves that if k is an even composite number, Goldbach's conjecture holds.
(6) At 2k, Goldbach's conjecture is valid
In (5.1) and (5.2) above, we have respectively proved that Goldbach's conjecture is valid when k is an odd composite number h and when k is an even composite number h. This proves that as long as k is a composite number h, at 2k Goldbach's conjecture holds.
Also because Goldbach's conjecture holds when k is an odd prime p,therefore, 2k is any positive integer Goldbach's conjecture is all true.
(7) The 8 mathematical examples in the paper are completely consistent with theorem 19
In the paper, there are 8 mathematical examples. We examined each of these examples. In every example, at least one of (hk+2) is an odd prime p. Such that 2k=(hk+2)+pd, at least one of 2k is the sum of the odd prime number (hk+2) and the odd prime number pd, guaranteeing the Goldbach conjecture at 2k.
Theorem 19, end of proof.
Please note to the reader:
Theorem 17 is an independent proof of Goldbach's conjecture.
Theorem 18 is an independent proof of Goldbach's conjecture.
Theorem 19 is another independent proof of Goldbach's conjecture.
Three proofs, if only one of them is correct, Goldbach's conjecture is true.
We, the prover, are, of course, sure that all three proofs are correct. Otherwise I wouldn't ask you to review it. At the same time, you are welcome to put forward valuable comments, we will refer to your comments, modify the paper better. You are welcome to participate and contribute to the proof of Goldbach's conjecture.
5.15 Summary of proof of conjecture:
From numerous concrete mathematical examples, the abstract mathematical theory (proposition) is raised, and then the abstract mathematical theory is proved as a mathematical theorem; The mathematical theorems we have obtained must be tested by mathematical examples. This is the mathematical idea and method followed by this proof of Goldbach's conjecture.
If the Goldbach conjecture holds for 2n, and proves that it holds for 2k, from n to k(=n+1), it appears that the difference is only one integer. However, it caused great changes.
If 2n=pi+pj is true, then pi and pj are symmetric with respect to n.
If 2k=pi++pj+ is true, then pi+, pj+ is symmetric with respect to k.
It should be noted that in the interval [3, n] and [3, k], the odd primes are exactly the same if k is composite number. That is, where the number of odd primes is equal, and the same odd prime is in the interval [3, n] and [3, k].
In the interval [n, 2n-3] and [k, 2k-3], where k is composite number, the odd primes are exactly the same except for n and 2k-3.
In the figure below, k=n+1, 2k-3=2n-1.

At 2n, the inference of Hou Shaosheng's theorem has the following four formulas:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-3)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-3)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Of these 4 formulas, there are only 4 basic formulas, as follows:
2n=pi+pj, 2n=ps+hr, 2n=ht+hu, 2n=hk+pd.
and that 2n+2=pi+2+pj, 2n+2=ps+2+hr, 2n+2=ht+2+hu, 2n+2=hk+2+pd.
Further there are:
2k=pi+2+pj, 2k=ps+2+hr, 2k=ht+2+hu, 2k=hk+2+pd.
2n+2=2k, that's what every schoolboy knows.
Hou Shao-sheng's theorem is the theoretical basis for proving Goldbach's conjecture. The proof of Hou's theorem was the first hurdle. Before proving Hou Shaosheng's theorem, no one knows the content of Hou Shaosheng's theorem, which is the biggest difficulty encountered in proving it. After obtaining Hou Shao-sheng's theorem, how to obtain the inference of Hou Shao-sheng's theorem is the second difficulty.
After proving Hou's theorem and its inferences, the difficulty of proving the conjecture is how to prove that at least one of the odd prime numbers is in (pi+2), or in (2+pj), or in (2+hr), or in (hk+2).
This is what all the above proof is about.
2n=pi+pj. pi, pj are symmetric about n;
2n=ps+hr. ps, hr are symmetric about n;
2n=ht+hu. ht, hu are symmetric about n;
2n=hk+pd. hk, pd are symmetric about n.
From 2n=pi+pj, 2n=ps+hr, 2n=ht+hu, 2n=hk+pd,
to 2k=pi++pj+, 2k=ps++hr+, 2k=ht++hu+, 2k=hk++pd+,
it is a process from quantitative change to qualitative change .
We have proved with new theories and methods that:If the conjecture is true at 2n, then the conjecture must be true at 2k.
The above proof shows that the theoretical system we have established is absolutely rigorous and correct. It can prove Goldbach's conjecture under various harsh conditions.
The above proof answers the core question:Do the four sources of 2k=pi++pj+not exist,at the same time? Now you can beabsolutely certain: 4 sourcesof2k=pi++pj+, at the same time do not exist, completely impossible. If only one of these four sources exists, the 2k conjecture is guaranteed. This is the decisive factor and the fundamental guarantee of the continuity of the conjecture.
The four sources of2k=pi++pj+are the fundamental guarantee that Goldbach's conjecture must be true.
The four sources of2k=pi++pj+are the genetic code that Goldbach's conjecture must hold.
The four sources of2k=pi++pj+are the mysteries that Goldbach's conjecture must be true
5.12 Theorem 17, we proved: if the conjecture is true for 2n and k (=n+1) is composite number, then at least one of (pi+2), (hr+2) is an odd prime number, which guarantees that the conjecture must be true for 2k.
Theorem 17, proved two sources of 2k=pi++pj+: 2k=(pi+2)+pj , 2k=ps+(hr+2), at least one of them exists.
5.13 Theorem 18, we proved: if the conjecture is true for 2n and k (=n+1) is composite number, then at least one of (hr+2) is an odd prime number, which guarantees that the conjecture must be true for 2k.
Theorem 18, proved one source of 2k=pi++pj+:2k=ps+(hr+2) must exist.
5.14 Theorem 19, we proved: if the conjecture is true for 2n and k (=n+1) is composite number, then at least one of (hk+2) is an odd prime number, which guarantees that the conjecture must be true for 2k.
Theorem 19, proved one source of 2k=pi++pj+:2k=(hk+2)+pd must exist.
Theorem 17, theorem 18, and theorem 19 are theorems that exist independently of each other. Any one of them would guarantee Goldbach's conjecture. If you want to disprove our proof of Goldbach's conjecture, you must find specific mathematical errors in each of the above three proofs!
So much for the proof of Goldbach's conjecture.
5.16 A Note
The above proof of Goldbach's conjecture is a proof of conjecture under the second theoretical system.
In 1967, Hou learned about Chen Jingrun and Goldbach's conjecture. In 1977, Hou Shaosheng proved the sufficient and necessary condition theorem of conjectures. In 2000, after 23 years of preparation, he gave the first proof of Goldbach's conjecture. In 2006, in the Guangming Daily Press, published the monograph "Proof of Goldbach's conjecture·Proof of Fermat's last conjecture ". 1,000 volumes of Chinese books have been circulated so far, and no one has found fundamental mathematical errors.
The proof of Goldbach's conjecture in the book is the first proof of Goldbach's conjecture given by Hou Shaosheng, that is, the proof of Goldbach's conjecture under the first theoretical system. The proof under the first theoretical system has three core mathematical ideas.
The first core mathematical idea is that proved that theorem of the sufficient and necessary condition that the conjecture is hold,and insists that a proof satisfies the theorem of sufficient and necessary conditions is a correct proof, and a proof does not satisfy the theorems of sufficient and necessary conditions must be wrong.
The second core mathematical idea is: It is proved that the single digits is all the odd composite numbers of 1,3,7,9, and are only the function values of 10 odd composite number formulas.Thus, all positive integers can be divided into 17 classes: the single digits is all the odd composite numbers of 1,3,7,9, divided into 10 classes; The single digit is all the odd composite numbers of 5, divided into 1 class;the single digits is all even numbers of 2,4,6,8,0, divided into 5 classes; All prime numbers, as 1 class.
The third core mathematical idea is that if n is a prime number, 2n, the conjecture naturally holds, without the need for proof. For the remaining 16 classes of composite numbers, classification proof. When proving a certain class of composite numbers, the other composite numbers are excluded, the interference is eliminated to the maximum extent, and the conditions for proving are created.
In fact, Goldbach's conjecture is divided into 17 small conjectures and proved separately. It's like a piece of cake that you can't eat all at once, so we divide it into 17 pieces and then eat each piece one by one.
The core mathematical idea of the second theoretical system is to uncover the genetic code between the 2n conjecture and the 2 (n+1) conjecture.
The second theoretical system is based on Hou Shao-sheng's identity 2n=q+(2n-q). This identity has three most valuable properties: first, it is an identity;Second, this identity contains all the possibilities of factorization of 2n into the sum of two integers; The third one, q, takes an odd number, 2n is just the sum of two odd numbers.In this way, all forms of decomposition that are irrelevant to the conjecture are deleted, and all elements that may be relevant to the conjecture are retained. We obtained all the elements that to prove conjecture.
The proof of Hou Shaosheng's theorem is a great achievement under the second theoretical system, and also a major breakthrough in the history of Goldbach's conjecture. The mathematical expression of Hou Shaosheng's theorem contains all the elements needed to prove the conjecture, which provides a solid theoretical basis for proving the conjecture.
The four inferential formulas of Hou Shaosheng's theorem provide specific and highly targeted mathematical formulas for the proof of conjectures.
The four major theoretical questions put forward by the author reveal the mystery of Goldbach's conjecture that there are only four genetic codes, and as long as there is a genetic code, the conjecture is guaranteed to continue to be true!
Under the second theoretical system, Hou Shaosheng proved theorem 17, theorem 18 and theorem 19. These three theorems exist independently of each other.Any one of these theorems is sufficient to ensure that the proof of the conjecture is completely completed.
Let real mathematicians review the proofs under the second theoretical system, and spare no efforts to teach and comment. Hou Shaosheng looks forward to your guidance and cooperation, and looks forward to your joint publication in the world's most famous magazine.
On November 15, 2007, Professor Ma Linjun and Professor Li Baitian of Sun Yat-sen University, after reviewing the Chinese book for half a year, wrote in the written Proof Materials: Wang Yuan, Chen Jingrun and other famous mathematicians said that it would be difficult to find a new mathematical idea to prove Goldbach's conjecturing in 1,000 years, and now Hou Shaosheng has solved the problem. We have studied "1+1" for decades, and Hou's research results are of the highest level within the scope of our knowledge, which has the basis for holding expert meetings and deserves the help and support of the government.
After Chen Jingrun proved 1+2, mathematicians all over the world almost recognized that the theory and method of 1+2 can not provide any help for proving 1+1! To prove 1+1, one has to look for new mathematical ideas.
In 1992, Wang Yuan, Chen Jingrun, Pan Chengdong, Yang Le, four academicians of the Chinese Academy of Sciences held a press conference, openly said: in another 1,000 years will be difficult to find a new mathematical idea to prove the conjecture, let alone prove 1+1!
In 2006, Wang Yuan said on CCTV4: I am firmly against the Chinese trying to prove 1+1.Because it's impossible!
Since Chen Jingrun proved 1+2, Wang Yuan, and Pan Chengdong proved 1+4, the leading position in the world and the absolute authority in China, their remarks made the proof of Goldbach's conjecture enter a frozen period: No magazine in the world is willing to review the "proof of Goldbach's conjecture", and in China there is a major tragedy that the review did not find the problem and did not dare to publish it!
In October 2013, Beijing Today Keyuan magazine published Hou Shaosheng's "New Mathematical Thought to Prove Goldbach Conjecture" and "Defect of Eratosthenes screening method ,and Ideal final screening method". Two papers, Hou Shaosheng invited Beijing Today Keyuan magazine editor Chen Jiazhong personally sent to Wang Yuan. Wang Yuan promised to give an explanation. Until Wang Yuan died, he did not give an explanation. Wang Yuan claimed to be proficient in screening; And said that it would be difficult to find new mathematical ideas to prove the conjecture for another 1,000 years. However, in the face of two papers, he was dumb!!
I sincerely hope that some competent mathematician will cooperate with me and publish a proof of Goldbach's conjecture! Let mathematicians around the world get out of the quagmire of proving Goldbach's conjecture, and end the sad history of the Chinese nation without mathematical theorems!
For references, see the end.
Section 6,Summary of the proof process of Goldbach conjecture
6.1 Study the three historical stages of Goldbach's conjecture
In 1742, Goldbach and Euler proposed the Goldbach conjecture. For 282 years, countless mathematicians have tried to prove it.
The period from 1742 to 1920 was a period of constant testing of Goldbach's conjecture. At this stage, by expanding the scope of the test, humans are convinced that the conjecture is highly likely to be correct.This is the first historical stage in the study of conjecture. The most important thing at this stage was that Goldbach made the conjecture, and then Euler published the conjecture and hoped that future mathematicians would prove it.
From 1920 to 1977, humans used electronic computers to verify Goldbach's conjecture on a larger scale. At this stage, the 9+9 proof method was created, and the 1+2 proof method was developed by Chen Jingrun. But mathematicians, including Chen, believed that to prove the conjecture, new mathematical ideas had to be sought. According to the Academy of Mathematics of the Chinese Academy of Sciences, it will be difficult to find new mathematical ideas to prove conjectures in another 1,000 years.
From 1977 to 2020, this is the third historical phase hypothesized by human research. The outstanding feature of this stage is that in 1977, Hou Shaosheng proved the sufficient and necessary condition theorem of Goldbach's conjecture in an unpublished manuscript. And the conclusion is drawn: if the proof satisfies the theorem of sufficient and necessary conditions, the proof is correct; If the proof does not satisfy the sufficient and necessary conditions theorem, the proof must be wrong. This new mathematical idea ended the history of groping in the dark and pointed the way to proving Goldbach's conjecture.
What is more valuable is that under the guidance of the theorem of sufficient and necessary conditions, after 23 years of hard and meticulous work, a series of problems satisfying the theorem of sufficient and necessary conditions have been creatively solved. And a proof of Goldbach's conjecture is written (Method 1).In 2006, he published the Proof of Goldbach's Conjecture and the Proof of Fermar's Last Conjecture. So far no one has been able to deny any a of the proofs.
6.2 Hou Shaosheng's theorem is a historic breakthrough in the study of conjecture.
The proof of Goldbach's conjecture (Method 1), while correct, does not, however, have the ability to answer: what is the genetic code between the conjecture at 2n and the conjecture at 2 (n+1)?
In order to uncover the secret that the conjecture holds for 2n and also for 2 (n+1), we study Hou Shao-sheng's identity. We have shown that 2n=q+(2n-q), where q≤(2n-q), contains the full possibility of factorising the even 2n into the sum of two odd numbers.
The mathematical representation of Goldbach's conjecture, 2n=pi+pj, is only a part of 2n=q+(2n-q). In order to fully grasp 2n=pi+pj, we must study the basic law of 2n=q+(2n-q). The basic law of 2n=q+(2n-q) is Hou Shaosheng's theorem.
The proof of Hou Shaosheng's theorem is the first difficulty. Before proving Hou Shaosheng's theorem, no one knew what the mathematical formula of Hou Shaosheng's theorem looked like. Therefore, proving Hou Shaosheng's theorem was the first major breakthrough.
Proving the sufficient and necessary condition theorem of the conjecture, Hou Shaosheng only used about 25 minutes, it should be said that the hand can be obtained. However, it took thousands of hours to prove Hou Shaosheng's theorem, and this was after the publication of the Proof of Goldbach's conjecture and the Proof of Fermar's Last Conjecture, and about 10 years of preparation.
The mathematical formula of Hou Shaosheng's theorem contains all the law of change of 2n=q+(2n-q), which lays a theoretical foundation for us to study Goldbach's conjecture comprehensively.
Hou Shaosheng theorem, no answer:
How do all odd prime numbers p in the interval [3,n] combine with odd numbers in the interval [n,2n-3]?
How do all odd composite numbers h in the interval [3,n] combine with odd numbers in the interval [n,2n-3]?
How do all odd prime numbers p in the interval [n,2n-3] combine with odd numbers in the interval [3,n]?
How do all odd composite numbers h in the interval [n,2n-3] combine with odd numbers in the interval [3,n]?
In order to answer the above questions, it is necessary to deduce the inference of Hou Shaosheng's theorem. Proving the inference of Hou Shaosheng's theorem is much easier than proving Hou Shaosheng's theorem. However, it still requires a certain amount of wisdom and a lot of work. Until it is proved, no one knows what the mathematical formula of inference looks like. The Angle from which it should be inferred still needs to be thought over. Therefore, the inference of Hou Shaosheng's theorem is the second major breakthrough.
The essence of the inference of Hou Shaosheng's theorem is to divide the mathematical formula of Hou Shaosheng's theorem, 1 into 4. Each of the four mathematical formulas represents only a part of the mathematical formulas of Hou Shaosheng's theorem.
The four mathematical formulas in the inference describe a certain aspect of Hou Shaosheng's theorem very deeply and carefully, and play an important role in our in-depth understanding of the basic law of 2n=q+(2n-q). Played a key role in proving Goldbach's conjecture.
6.3 Four major theoretical problems are the core theoretical problems in the proof of conjectures
The four major theoretical problems proposed by the author are the core theoretical problems in the proof of Goldbach's conjecture.
Goldbach's conjecture holds, essentially, for 2n it holds, and for 2 (n+1) it still holds.
The proof of Goldbach's conjecture must answer: If the conjecture is true for 2n, why is it still true for 2 (n+1)? What is the close relationship between the establishment of 2n and the establishment of 2k? This is the most critical, the core essence of the conjecture proof, but also the most difficult problem.
The four major theoretical questions theoretically fully answer:the close relationship between the establishment of 2n and the establishment of 2 (n+1).
Theorem 11 in Section 5 proves that: if Goldbach's conjecture 2n=pi+pj is true for 2n, then Goldbach's conjecture 2k=pi++pj+ for 2k has four sources; And as long as one source exists, Goldbach's conjecture will continue to hold at 2k. This is the most important discovery and theory in the proof of Goldbach's conjecture.
2k=pi++pj+ has four sources, which is the fundamental reason and guarantee for the continued establishment of Goldbach conjecture; Is the fundamental mystery of the Goldbach conjecture.
Theorem 12 in Section 5 proves that if Goldbach's conjecture 2n=pi+pj is true for 2n, then 2k=ps++hr+ for 2k has four sources.
Theorem 13 in Section 5 proves that if Goldbach's conjecture 2n=pi+pj is true for 2n, then 2k=ht++hu+ has four sources for 2k.
Theorem 14 in Section 5 proves that if Goldbach's conjecture 2n=pi+pj is true for 2n, then 2k=hk++pd+ for 2k has four sources.
Four major theoretical questions not only theoretically answer the close relationship between 2n establishment and 2k establishment, but also accept the strict test of 8 examples.
The test shows that 2k=pi++pj+ has four sources, which are perfectly consistent with complex mathematical examples.
2k=ps++hr+ has 4 sources; 2k=ht++hu+ has 4 sources; 2k=hk++pd+ has 4 sources; These theories are in perfect agreement with complex mathematical examples.
The test shows that Hou Shaosheng's theorem is correct and the inference of Hou Shaosheng's theorem is correct. Our reasoning process is correct. Readers are welcome to test with other examples.
It has already been pointed out that as long as one of the four sources of 2k=pi++pj+ exists, the 2k time conjecture is guaranteed. Could it be that none of the four sources exist?
This is a central question that must be answered.
5.12 Theorem 17: If the conjecture is true at 2n and k (=n+1) is composite number, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 17, proves two sources of 2k=pi++pj+: 2k=(pi+2)+pj; 2k=ps+(hr+2), at least one of them exists.
The clever thing about this theorem proving procedure is that it captures two of the four sources of 2k=pi++pj+ : 2k=(pi+2)+pj, 2k=ps+(hr+2), and at least one of them exists. In a short process, it is proved that conjecture must be true at 2k.
5.13 Theorem 18: If theconjecture is true at 2n and k (=n+1) is compositenumber, then at least one of (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 18, proves that2k=pi++pj+1 source :2k=ps+(hr+2), must exist.
5.14 Theorem 19: Ifthe conjecture is true at 2n and k (=n+1) is compositenumber, then at least one of (hk+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 19, proves that2k=pi++pj+1 source : 2k=(hk+2)+pd, must exist.
In the above proof, the four inferential formulas of Hou Shaosheng's theorem play a decisive role.
We also prove that the formula for calculating the number of odd primes in the interval [n,2n], there must be odd primes in the interval [n,2n].
Now, we have two different theories that can prove Goldbach's conjecture. One theoretical system is based on the sufficient and necessary condition theorem of Goldbach conjecture. The other one is based on Hou Shao-sheng's theorem and centers on the four inferential formulas of Hou Shao-sheng's theorem and the four sources of 2k=pi++pj+. Both proofs satisfy the necessary and sufficient theorems that the conjecture is true!
6.4Our main logical process for proving Goldbach's conjecture is as follows:
6.4.1: Object of study: Goldbach conjecture 2n=pi+pj.
Ask the question: What are the necessary and sufficient conditions for the conjecture to be true?
Answer the question: 2n=pi+pj, the sufficient and necessary condition theorem: (n-△), (n+△), are odd prime numbers.
Ask the question: n+△, in the interval [n,2n]. It must be proved that there are odd primes in the interval [n,2n].
Answer the question: Derive the formula for calculating the number of odd primes in the interval [n,2n].
It is proved that there are odd prime numbers in the interval [n,2n], which satisfies one of the necessary conditions for the conjecture to be established.
The above is a branch of the logical process of proving the conjecture.
6.4.2: The main logical process for proving Goldbach's conjecture is as follows:
Research object: 2n=pi+pj, 2n=q+(2n-q), where q≤(2n-q),q is odd.
The conjecture expression: 2n=pi+pj, is only a part of 2n=q+(2n-q).
Asking questions: Hou Shao-sheng's expansion of identity

Answer the question: Prove Hou Shaosheng's theorem:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
Where: 2n=pi+pj, pi is an odd prime number in the interval [3,n]; pj is an odd prime number in the interval [n,2n-3].
Ask questions:
How do all odd prime numbers p in the interval [3,n] combine with odd
numbers in the interval [n,2n-3]?
How do all odd composite numbers h in the interval [3,n] combine with odd numbers in the interval [n,2n-3]?
How do all odd prime numbers p in the interval [n,2n-3] combine with odd numbers in the interval [3,n]?
How do all odd composite numbers h in the interval [n,2n-3] combine with odd numbers in the interval [3,n]?
Answer the question: Inference of Hou Shaosheng's theorem:
num(2n=q+(2n-q))=num(2n=pi+pj)+num(2n=ht+hu)
+ num(2n=ps+hr)+num(2n=hk+pd). (3.3.0)
There are always:
num(3≤p≤n)=num(2n=pi+pj)+num(2n=ps+hr); (4.0.1)
num(3≤h≤n)=num(2n=ht+hu)+num(2n=hk+pd); (4.0.2)
num(n≤p≤2n-2)=num(2n=pi+pj)+num(2n=hk+pd); (4.0.3)
num(n≤h≤2n-2)=num(2n=ht+hu)+num(2n=ps+hr). (4.0.4)
Ask questions: From the expression 2n=pi+pj:
2n=pi+pj;2n+2=pi+2+pj;2(n+1)=(pi+2)+pj;
2n=pi+pj;2n+2=pi+2+pj;2(n+1)=pi+(2+pj);
2n=ps+hr;2n+2=ps+2+hr;2(n+1)=ps+(2+hr);
2n=hk+pd;2n+2=hk+2+pd;2(n+1)=(hk+2)+pd;
These questions suggest that 2(n+1)=2k=pi++pj+ has four sources.
Ask the question: It must be proved that 2(n+1)=2k=pi++pj+ has 4 sources.
Answer the question:Section 5 Theorem 11: If the 2n conjecture is
true, then 2k=pi++pj+ has the following four sources, as long as one source exists, it will ensure that the conjecture continues to be true at 2k.
The 4 sources of 2k=pi++pj+ are as follows:
If one of (pi+2) is an odd prime, then 2k=(pi+2)+pj becomes one of 2k=pi++pj+;
If one of (pj+2) is an odd prime, then 2k=pi+(pj+2) becomes one of 2k=pi++pj+;
If one of (hr+2) is an odd prime, then 2k=ps+(hr+2) becomes one of 2k=pi++pj+;
If one of (hk+2) is an odd prime, then 2k=(hk+2)+pd becomes one of 2k=pi++pj+.
Ask the question: As long as one of the four sources exists, Goldbach's conjecture continues to hold true.
Finally, the only question: Is it possible that none of the four sources of 2k=pi++pj+ exist?
Answer the question:
5.12 Theorem 17: If the conjecture is true at 2n and k (=n+1) is
composite number, then at least one of (pi+2), (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 17, proves two sources of 2k=pi++pj+: 2k=(pi+2)+pj; 2k=ps+(hr+2), at least one of them exists.
5.13 Theorem 18: If the conjecture is true at 2n and k (=n+1) is composite number, then at least one of (hr+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 18, proves that 2k=pi++pj+ 1 source :2k=ps+(hr+2), must exist.
5.14 Theorem 19: If the conjecture is true at 2n and k (=n+1) is composite number, then at least one of (hk+2) is an odd prime, which guarantees that the conjecture is true at 2k.
Theorem 19, proves that 2k=pi++pj+ 1 source: 2k=(hk+2)+pd, must exist.
Looking back on the process of proving Goldbach's conjecture, the rule follows:
First, the sufficient and necessary condition theorems of conjectures are proved. The theorem of sufficient and necessary conditions points out the general direction for proving conjectures. In this proof, the proof of Hou Shaosheng's theorem is very important and very difficult. Because that's the theoretical basis for proving the conjecture. On this basis, what other problems can we solve and what progress can we make through our efforts? Every step of the way is to reach the final goal. After we have obtained the four sources of 2k=pi++pj+, our ultimate goal is to prove that at least one of the four sources of 2k=pi++pj+ exists. It is under such logical thinking that the proof of Goldbach's conjecture is finally completed.
So much for the proof of Goldbach's conjecture.
6.5Your help is welcome
Welcome any experts to contact us, welcome any experts to ask questions, welcome any experts to cooperate with us! Welcome anyone who can help us! Please make your valuable contribution to the proof of Goldbach conjecture!
Hou Shao-Sheng Email: ayhss@163.com.
References
- Mathematical dictionary. Beijing: Surveying and Mapping Publishing House, 1982.
- Chen Jingrun, Elementary Number Theory (Ⅰ, Ⅱ)[M], Beijing: Science Press. Ⅰ, 1978, Ⅱ, 1980.
- Introduction to number theory. Hua Luogeng.
- Wang Yuan on Goldbach Conjecture [M]. Jinan: Shandong Education Press, 1999.
- Concise Number Theory, Pan Chengdong, Pan Chengbiao, Beijing University Press, 1998.
- Unsolved Problems in number Theory (2nd Ed.). [Gar] By R.K. Gay. Science Press. 2003.
- https://en.wikipedia.org/wiki/Goldbach’s_conjecture.
- Hou Shao-Sheng1, Wang Shun-qing2, Decomposition formula of odd composite numbers, prime distribution and screening method. Journal of Northwest Institute for Nationalities [Natural Science Edition], 2002, 23(2) : 1-6.
- Hou Shao-sheng1, Wang Shun-qing2, The Relationship between prime numbers and composite number, the condition that positive integers are prime numbers. Journal of Northwest Institute for Nationalities [Natural Science Edition], 2002, 23(4) : 1-7.
- Hou Shao-sheng, Proof of Goldbach's Conjecture. Guangming Daily Press, 2006.
- Hou Shao-sheng, A Note on the equation xn+yn=zn. Mathematics Quarterly, 1993.
- By Hou Shao-sheng, Translated by QIN Jianmin, PROOF OF GOLDBACH CONJECTURE; TSINGHUA UNIVERSITY PRESS. 2009.
- Hou Shao-Sheng1, MA Lin-Jun2, LI Bai-tian3, QIN Jian-min4, ZHANG Kai-Dao5, Defects and Ideal Final screening Method of Eratosthenes Screening. Beijing Jinri Science Garden, 2013/13. No. 279.
- Hou Shao-Sheng1, MA Lin-Jun2, LI Bai-tian3, QIN Jian-min4, ZHANG Kai-Dao5, A New idea to prove Goldbach's conjecture. Beijing Jinri Science Garden, 2013/13. No.279.
- Pan Chengdong, Pan Chengbiao, Goldbach Conjecture [M]. Beijing: Science Press, 1981.
- P, RibenboiM, The New Book of Prime Number Records[M],Springer,1996.
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Downloads
136
Views
352
Comments
0
Subscription
Notify me about updates to this article or when a peer-reviewed version is published.
MDPI Initiatives
Important Links
© 2025 MDPI (Basel, Switzerland) unless otherwise stated




