Prerpints.org logo
Preprint
Article

Non-Markovian Gravitational Decoherence and the Black Hole Information Paradox

Altmetrics

Downloads

97

Views

52

Comments

0

A peer-reviewed article of this preprint also exists.

This version is not peer-reviewed

Submitted:

07 October 2024

Posted:

08 October 2024

Read the latest preprint version here

Alerts
Abstract
An isolated system always evolves according to unitary evolution and maintain coherence. However the system inevitably interacts with the environment, information about the relative phases between the quantum states leaks into the environment and becomes delocalized, known as environment-induced decoherence. Here we consider the gravitational decoherence of a quantum system near the event horizon of a Schwarzschild black hole. We show that the gravitational decoherence is non-Markovian, which is consistent with information conservation and the Pagecurve-like entanglement dynamics. Moreover, taking the point of view that information on the collapsed matter is stored as the quantum fluctuation of the horizon, the horizon can be regarded as an “uncertain” quantum object instead of random fluctuations of graviton noise. Now the Hawking radiation bath plays the role of the environment, leading to the decoherence of the horizon. Therefore, information about the location of the horizon, or equivalently information about the collapsed matter, is carried away by the Hawking radiation. The memory time of the non-Markovian process and the Page time are estimated.
Keywords: 
Subject: Physical Sciences  -   Theoretical Physics

1. Introduction

In quantum mechanics, systems can exist in a superposition of states, described by a wave function that represents the probability amplitude of finding the system in each possible state. As long as there exists a definite phase relation between the components of the superposition, the system is said to be coherent and exhibits interference effects. An isolated system always evolves according to unitary evolution and maintain coherence. But as soon as a system becomes entangled with its surroundings, the information about the relative phases between the quantum states leaks into the environment, known as environment-induced decoherence proposed by Zeh [1] (for a review see Ref. [2,3,4]). In such circumstances, a description of the quantum system under consideration in terms of a reduced density matrix obtained by tracing out the large number of degrees of freedom in the environment is employed instead. Environment-induced decoherence is a fundamental process that plays a crucial role in the transition from quantum to classical behavior.
On the other hand, to explain the quantum-to-classical transition, many objective collapse theories, including the Ghirardi–Rimini–Weber (GRW) model [5] and the continuous spontaneous localization (CSL) model [6], have been proposed. The wave function collapse can be caused by various forms of noise in the environment. Instead of introducing some vague concept of the “unobservable” environmental degrees of freedom, Penrose (and Diósi, independently) suggested that the wave function collapse is induced by the interaction of the system with its gravitational field, the so-called DP model [7,8,9,10]. The wave function describing the state of a quantum system progressively loses its validity when the mass of the system is large enough. Although the DP model is the most influential model of gravitational decoherence, it appears to have been ruled out in recent experiments [11]. To resolve the contradiction between quantum theory and general relativity, Jonathan Oppenheim suggested that the spacetime with random noise is classical, which provides a picture of how the gravitational field responds to the superposition of mass [12]. However, the most radical consequence of this theory is that it allows for the destruction of quantum information, which conflicts with the fundamental principle of information conservation.
In this paper, we focus on the gravitational decoherence, which refers to the loss of coherence in matter due to gravity. Most gravitational decoherence models are established in the non-relativistic limit. There are also some other versions of the gravitational decoherence models. But these models make strong assumptions about the basic spacetime structure and the behavior of spacetime fluctuations [13,14,15,16]. A particular promising candidate is the decoherence model of Anastopoulos and Hu [17,18] and of Blencowe [19], which is the most conservative model in that it assumes maximal validity of quantum field theory and general relativity. It is worth mentioning that the Hamiltonian of this model is formally similar to a quantum Brownian motion (QBM) model [20], with the transverse traceless degrees of freedom playing the role of the bath oscillators. Furthermore, by using the Feynman-Vernon influence functional method, Hu and Matacz investigate the QBM in a bath of parametric oscillators [21]. An important result of this model is the derivation of the influence functional and thus the noise and dissipation kernels in terms of the Bogolubov coefficients. This enables one to trace the source of statistical processes like decoherence and dissipation to vacuum fluctuations and particle creation, and in turn impart a statistical mechanical interpretation of quantum field processes.
In this paper we follow the method of Ref. [21] and show that the decoherence of quantum states near the event horizon is Non-Markovian, just like most QBM models. Thus, one might expect that decoherence could be reversed to ensure information conservation. Some studies have shown that non-Markovian dynamics and the resulting memory effects can result in a backflow of information from the environment to the system in a manner that impedes the creation of robust, classical, redundant environmental records [22,23,24]. In the spirit of this, we will argue that for a non-Markovian open quantum system, the presence of memory effects allows information to flow back from the environment to the system, leading to a Page-curve-like entanglement dynamics. In this line of thought, if the dynamics of gravitational decoherence are non-Markovian, information will flow back from the black hole to the outsider world. As expected, we show that information on the collapsed matter can be carried away by the Hawking radiation. The proposal presented here may allow us to resolve the black hole information paradox.
This paper is organized as follow. In Sec.2 we make a brief review of the system-field interactions model. In Sec.3 we investigate the decoherence of quantum systems near the event horizon. Sec.4 is dedicated to the decoherence of the event horizon and information backflow. Finally, in Sec.5 we summarize the main results obtained.
For convenience, we use units with c=1 in Sec.2 and Sec.3. The signature of the metric is ( − , + , + , + ) .

2. System-Field Interactions Model

Let us first review the model for system-field interactions [21]. In order to study the noise properties of the environment (bath), we introduce an interaction between the system, which can be a particle detector, and the environment (bath). Here the particle detector is modeled by a Brownian particle with mass m ( s ) , cross term B ( s ) and bare frequency Ω ( s ) . The environment is modeled by an infinite collection of parametric oscillators with mass μ n ( s ) , cross term b n ( s ) and bare frequency ω n ( s ) . The system is coupled to the bath through an arbitrary function F ( x ) of the system variable and linear in the bath variables q n with coupling strength c n ( s ) in each oscillator. The action of the particle detector interacting with the bath is given by
S [ x , q ] = S [ x ] + S E [ q ] + S int [ x , q ] = ∫ 0 t d s 1 2 m ( s ) x ˙ 2 + B ( s ) x x ˙ − Ω 2 ( s ) x 2 + ∑ n 1 2 μ n ( s ) q ˙ n 2 + b n ( s ) q n q ˙ n − ω n 2 ( s ) q n 2 + ∑ n − c n ( s ) F ( x ) q n ,
where x and q n are the coordinates of the particle and the oscillators, respectively. The bare frequency Ω is different from the physical frequency Ω p due to its interaction with the bath, which depends on the cutoff frequency.
By using the Feynman-Vernon influence functional method, we can derive the evolution operator J for the system reduced density matrix ρ ^ , which is defined by
ρ ^ ( t ) = J t , t i ρ ^ t i
We assume that at a given time t = t i the system and the environment are uncorrelated
ρ ^ t = t i = ρ ^ s t i × ρ ^ b t i .
Then the evolution operator does not depend on the initial state of the system. In the position basis, it can be written as
J x f , x f ′ , t ∣ x i , x i ′ , t i = ∫ x i x f D x ∫ x i ′ x f ′ D x ′ exp i ℏ S [ x ] − S x ′ F x , x ′ ,
where F x , x ′ is the influence functional. In the case of a squeezed thermal initial state, it has the form
F x , x ′ = exp { − 1 ℏ ∫ t i d s ∫ t i d s ′ F ( x ( s ) ) − F x ′ ( s ) ν s , s ′ F x s ′ − F x ′ s ′ − i ℏ ∫ t i t d s ∫ t i s d s ′ F ( x ( s ) ) + F x ′ ( s ) η s , s ′ F x s ′ + F x ′ s ′ ,
where the functions ν s , s ′ and η s , s ′ are known respectively as the noise and dissipation kernels. In the semiclassical limit, the noise and dissipation kernels are given by
ν s , s ′ = 1 2 ∫ 0 ∞ d ω J ω , s , s ′ coth ℏ ω t i 2 k B T × cosh 2 r ( ω ) α ω ( s ) + β ω ( s ) * α ω s ′ + β ω s ′ + cosh 2 r ( ω ) α ω ( s ) + β ω ( s ) α ω s ′ + β ω s ′ * − sinh 2 r ( ω ) e − 2 i ϕ ( ω ) α ω ( s ) + β ω ( s ) * α ω s ′ + β ω s ′ * − sinh 2 r ( ω ) e 2 i ϕ ( ω ) α ω ( s ) + β ω ( s ) α ω s ′ + β ω s ′
and
η s , s ′ = i 2 ∫ 0 ∞ d ω J ω , s , s ′ α ω ( s ) + β ω ( s ) * α ω s ′ + β ω s ′ − α ω ( s ) + β ω ( s ) α ω s ′ + β ω s ′ * ,
where the complex numbers α and β are the Bogolubov coefficients, J ω , s , s ′ is the spectral density and we consider the initial state of the bath as a squeezed thermal state.
We now can derive the master equation from the evolution operator and it has a generic form
i ℏ ∂ ∂ t ρ ^ ( t ) = H ^ R , ρ ^ + Γ ( t ) [ x ^ , { p ^ , ρ ^ } ] + i D p p ( t ) [ x ^ , [ x ^ , ρ ^ ] ] + i D x x ( t ) [ p ^ , [ p ^ , ρ ^ ] ] + i D x p ( t ) [ x ^ , [ p ^ , ρ ^ ] ] + i D p x ( t ) [ p ^ , [ x ^ , ρ ^ ] ]
where H ^ R is the renormalized Hamiltonian and all the coefficients depend on the dissipation and noise kernels. The first term on the right-hand side of Eq. (8) represents the usual unitary dynamics. The second term describes dissipation at a rate Γ ( t ) . The third and fourth diffusion terms have the Lindblad double-commutator form and describe spatial and momentum decoherence at the rates D p p ( t ) and D x x ( t ) , respectively. The last two terms describe mixed spatial-momentum coherence.
Note that the time dependence of the decoherence rate is rather complicated, but, given a particular form of the spectral density and the initial state of the environment, it can be explicitly calculated. The spectral density J ( ω ) encode physical properties of the environment. It measures the number of environmental modes with a given frequency and the strength of the interaction. For the usual decoherence process, the decoherence rate D ( t ) is always negative, leading to a dynamical semigroup of completely positive and trace preserving (CPT) maps, known as quantum Markovian processes. But this model (1) depicts non-Markovian processes due to the the indefinite sign of the decoherence rate.

3. Gravitional Decoherence Near the Black Hole Horizon

In the classical limit where the number of gravitons per mode is extremely large, most contributions to gravitational fluctuations come from classical gravitational waves and correlation functions are completely characterized by the number of gravitons per mode. Here we are interested in the gravitational decoherence inside a black hole. However, since an external observer is limited in observing beyond the interior of a black hole, for simplicity we will investigate the gravitational decoherence near the horizon of a Schwarzschild black hole. We are arguing that when the particle is one Compton wavelength from the horizon, it is considered to be part of the black hole. We assume that the gravitons are in a squeezed thermal state, which is the appropriate state for quantum particle creation processes, i.e. the Hawking radiation. We consider time independent coupling constants. In this case, the noise and dissipation kernels given in Eqs. (6) and (7) becomes
ν t , t ′ = ∫ 0 ∞ d ω coth ℏ ω 2 k B T J ( ω ) cosh 2 r ( ω ) cos ω t − t ′ − sinh 2 r ( ω ) cos 2 ϕ ( ω ) − ω t + t ′
and
η t , t ′ = − ∫ 0 ∞ d ω J ( ω ) sin ω t − t ′ ,
where r and θ denote squeeze parameters. Without loss of generality, let us consider the metric of a two-dimensional Schwarzschild black hole with mass M
d s 2 = − 1 − 2 G M r d t 2 + 1 − 2 G M r − 1 d r 2 .
In the Kruskal coordinates (T, R), we get a conformal metric
d s 2 = 2 G M r exp − r 2 G M − d T 2 + d R 2
with
T = 4 G M exp r * 4 G M sinh t 4 G M
and
R = 4 G M exp r * 4 G M cosh t 4 G M ,
where the tortoise coordinate r * is defined by
r * = r + 2 G M ln r 2 G M − 1 .
The spectral density is the same as the case of the accelerating observer. That is
J k , t , t ′ = J ( k ) cos k R ( t ) − R t ′
with
J ( k ) = ϵ 2 2 π ω ,
where ϵ is the coupling strength and ω = | k | . Thus for a massless scalar field in a two-dimensional black hole spacetime, we have
ζ t , t ′ ≡ ν t , t ′ + i η t , t ′ = 1 2 ∫ 0 ∞ d k J ( k ) e − i k R ( t ) − R t ′ + T ( t ) − T t ′ + 1 2 ∫ 0 ∞ d k J ( k ) e − i k R t ′ − R ( t ) + T ( t ) − T t ′ .
By using Eqs. (13) and (14), it can be written as
ζ t , t ′ = 1 2 ∫ 0 ∞ d k ′ J k ′ exp − 8 i G M k ′ e ( t + t ′ ) / 8 G M sinh [ ( t − t ′ ) / 4 G M ] + exp − 8 i G M k ′ e − ( t + t ′ ) / 8 G M sinh [ ( t − t ′ ) / 4 G M ] .
Upon using the gamma functions and the four-dimensional ohmic spectral density J 4 ( k ) = ϵ 2 m ω ( 2 π ) 2 , the dissipation and noise kernels become
ν t , t ′ = ∫ 0 ∞ d k J 4 ( k ) exp − r * 2 M coth 4 π G M k exp r * 4 M cos k ( t − t ′ ) exp r * 4 M
and
η t , t ′ = − ∫ 0 ∞ d k J 4 ( k ) exp − r * 2 M sin k ( t − t ′ ) exp r * 4 M .
The explicit expressions for these coefficients of the master equation are rather huge and we do not write it here, but it is remarkable that this model depicts non-Markovian processes and all the non-Markovian behavior is embodied in the complicated time dependence of the coefficients.
As r → 2 M and exp ( r * / 4 M ) → 0 , we consider that the oscillatory terms in the integral expression remain constant and obtain the evolution of the reduced density matrix that is formally similar to the Caldeira–Leggett master equation [25]. Therefore, in this high temperature limit, one arrives at
D ( t ) ∝ − m M exp − 3 r * 4 M → − ∞ .
This means a decoherence at an extremely fast rate near the horizon.

4. Non-Markovianity and Information Backflow

In this section, we will study the non-Markovian gravitational decoherence processes. As an example, suppose that the initial state of the combined system at time t = 0 is a tensor product state. As the system becomes entangled with its environment, it experiences a decoherence under a Markovian evolution with D ( t ) < 0 , and information flows from the system to the environment, leading to an increase in the entanglement entropy. This is a general feature of typical decoherence processes, implying that a pair of states generally become indistinguishable as time increases. However, due to specific forms of the spectral density and the oscillatory terms in the integral, the value of D ( t ) could become positive for certain times. It is this type of processes which we define as non-Markovian. Physically, we interpret this as a flow of information from the environment back to the system which enhances the possibility of distinguishing the given states. When the information flowing out of the system completely returns at time t = t f , the entanglement entropy decrease back to zero, which reproduces a Page-curve-like entanglement dynamics. Similar to the Page curve in the black hole dynamics, we assume that the curve of the entanglement entropy for the reduced system has only one peak, and then the Page time t c is the solution of this equation
D ( t c ) = 0 .
Markovian quantum processes are defined by the master equation during the time interval ( 0 , t c ) and non-Markovian quantum processes are defined by the master equation during the time interval ( t c , t f ) .
As mentioned above, the evolution of the system near the event horizon contains a nonlocal integral expression and the sign of the decoherence rate is indefinite. It turns out that the value of D ( t ) is positive for certain times. Therefore, the gravitational decoherence of a quantum state near the horizon is a non-Markovian process. This means that after the decoherence of the infalling particles, information flows to the spacetime and subsequently leaks out in some way, e.g. the Hawking radiation. Since the quantum coherence is not lost but rather mixed with many more degrees of freedom in the environment and hence information becomes delocalized, we make the assumption that information is conserved and localized on the horizon. Then the horizon can be regarded as an “uncertain” quantum object instead of random fluctuations of graviton noise. In this framework information about the in-falling particles is encoded in the horizon dynamics. Physically, the quantum properties of the horizon can be demonstrated by considering a black hole in a superposition of two position states with separation Δ x . However, we suggest that the quantum superposition is translated into the horizon fluctuations rather than the uncertainty in the position of the black hole center because the parameter describing the evolution of a black hole is the radius and horizon, and the horizon cannot be described as a classical geometric boundary as the Schwarzschild radius approaches the Planck length, although the two proposals above give the same result. Consider the case of a black hole immersed in a thermal bath of the Hawking radiation particles. As the horizon becomes entangled with the thermal bath, it experiences a decoherence and information about the location of the horizon is carried away by the Hawking radiation. This reversible non-Markovian process describes information flowing back from the black hole to the outside world (see Figure 1). Consider a Schwarzschild black hole in thermal equilibrium with a radiation bath. The decoherence of the horizon is caused both by the quanta emitted by the black hole and by the quanta in the external heat bath that are scattered by it. The resulting decoherence rate of the horizon D h ( t ) is the same as the case of a non-local superposition of a Schwarzschild black hole in two distinct locations separated by Δ x . In the geometrical optics approximation, it is given by a simple expression [26]
1 D h ( t ) = τ ( Δ x ) = d − 1 R S Δ x 2 R S c ≃ 17.37 R S Δ x 2 R S c ,
where τ is the decoherence time, d ≃ 0.0576 is a numerical factor and R S is the Schwarzschild radius. It turns out that the decoherence rate of the horizon increases with 1 / M 3 .
According to Bekenstein’s idea for the derivation of the area law, suppose that we form a black hole of size R S by injecting a quanta with energy ℏ / R S many times. Since the s-wave enters the black hole with a probability of 1/2 and is bounced back with the same probability, we can model the formation as a stochastic process according to binomial distribution. Then, the average number of trialsis N is given by
N ∼ R S 2 G × 1 ℏ / R S ∼ R S 2 l p 2 ,
where l p is the Planck length. Thus the statistical fluctuation of mass M is [27]
Δ M ∼ M N ∼ m p ,
where m p is the Planck mass. This means that the horizon fluctuates is of order l p . Indeed, it is well known that the quantum fluctuation of the horizon is suppressed by the black hole mass [28]. As the black hole shrinks due to the Hawking radiation, the quantum fluctuation of the horizon increases. When the Schwarzschild radius approaches the Planck length, it is no more characterized by a classical geometric boundary. But our assumption is that the horizon fluctuates have a constant magnitude l p . This is reasonable because the duration of the final stage of Hawking radiation is very short in comparison to the evaporation time of the black hole, and it has little impact on our time scale estimation. By using Eq. (24), the decoherence time of the horizon is given by
τ ( l p ) ≃ 139 G 2 M 3 ℏ c 4
The non-Markovian properties of the horizon are completely determined by the spectral density. There are two factors enter in the spectral density function J ( ω , Λ ) . For the decoherence of a particle near the horizon, the horizon play the role of the environment. A generic form of the ohmic spectral density of the horizon in the frequency domain ω is
J ( ω , Λ ) = G ℏ c 5 ω P Λ ( ω ) .
The cutoff scale Λ is introduced since we expect the spectral density to fall off to zero when ω > Λ . We also require that P Λ ( ω ) → 1 as Λ → ∞ will give the spectral density of a Markovian environment in that limit [29]. The evolution of the system contains a nonlocal integral expression if the cutoff scale in the spectral density is finite and the inverse of the cutoff scale gives the memory time. From Eq. (27), we immediately see that the cutoff scale is of order
Λ ∼ 1 τ ( l p ) ≃ ℏ c 4 139 G 2 M 3
and τ ( l p ) is the memory time, which measures the time scale of the system evolution depending on its past history. On the other hand, the total lifetime of a Schwarzschild black hole due to evaporation is
t BH = 5120 π G 2 M 3 ℏ c 4
Therefore, the Page time is given by
t c = t BH − τ ( l p ) ≃ 15938 G 2 M 3 ℏ c 4 .
In the vicinity of the horizon of the black hole, the cutoff scale of the horizon is much smaller than the usual frequency scale of the system. The black hole with a very long memory time has non-Markovian properties and hence information is conserved. Note that in the final stage of Hawking radiation, the renormalized gravitational constant G R becomes large and the horizon dynamics should be described by a quantum gravity theory.

5. Discussion

In this paper, we investigate the decoherence of quantum states near the event horizon. The indefinite sign of decoherence rate implies that the gravitational decoherence occurs in the vicinity of the horizon is a non-Markovian process. Here the particle is considered to be part of the black hole when it is one Compton wavelength from the horizon. The result is based on the model of QBM in a bath of parametric oscillators. Note that the Hawking radiation effect has been included because we can reproduce the Hawking radiation formula by using Eq. (9). The interior spacetime of the black hole, also called “T-sphere” which is globally hyperbolic, gains the status of a cosmological model [30]. Clearly, an isolated black hole can be treated as a closed system regardless of weak Hawking radiation. Thus it is reasonable to believe that the decoherence inside the event horizon is reversible. In Ref. [31], it has been shown that the evolution of the entanglement entropy of a harmonic oscillator linearly coupled to a continuum of harmonic oscillators with a ohmic spectral density follows the Page curve when the impurity is initialized in a pure state far from equilibrium. All these results implied the non-Markovianity of the black hole, which is consistent with information conservation and the Page-curve-like entanglement dynamics. It demonstrates the differences between gravitational decoherence in the usual curved spacetime and that inside the event horizon. This phenomenon may be interpreted as due to the fact that information is localized on the horizon and the black hole has a finite number of degrees of freedom measured by its horizon area. However for the typical environment, quantum decoherence is generally irreversible except for those pointer states because it has an infinite number of degrees of freedom and we cannot manipulate the environment. Another important point concerning our results is that the decoherence measured in the frame of a geodesic observer falling through the horizon. According to the equivalence principle, the freely-falling observers see nothing special happen when crossing the horizon until they arrive at the singularity. In spacetime, the effect of a black hole singularity is to absorb and destroy all the matter that impinges upon it. More importantly for our present purposes, it destroys information. To resolve the contradiction, one might expect that there exists an information creation processes to compensate exactly for information loss due to the singularity. This process may be an objective state-vector reduction process in quantum mechanics as proposed by Penrose. However, in the absence of a quantum gravity theory, this guess needs a further exploration. If we recall the fundamental lesson from the special relativity and general relativity, we conclude that some paradoxes arise from the observation results being reference frame dependent. But objective physical quantities are something that all observers agree on and hence the observation results should be measured by an invariant variable derived from a new theory. In the special relativity and general relativity, the corresponding invariant variables are proper time and curvature tensor, respectively. The coordinate time and gravity are non physical.
We then further investigate the quantum properties of the event horizon. We examine a possibility that, when a black hole is formed, the information on the collapsed matter is stored as the quantum fluctuation of the horizon. In this case, the horizon becomes an “uncertain” quantum object. The Hawking radiation bath now plays the role of the environment, leading to the decoherence of the horizon. Therefore, information about the location of the horizon, or equivalently information on the collapsed matter, is carried away by the Hawking radiation. We are just providing intuitive and heuristic solutions to the black hole information paradox based on plausible physics. Since the quantum fluctuations of the horizon are suppressed by the mass of the black hole, we have a exact Bekenstein–Hawking entropy for massive black holes. When the black hole is small, the quantum fluctuations of the horizon increase, leading to an increase in decoherence rate of the horizon and a decrease in memory time. It would be interesting to investigate the decoherence behavior and the deviation from the area law due to quantum corrections in the final stage of the Hawking radiation. However, at the Planck scale the quantum fluctuations of the horizon become comparable to the Schwarzschild radius and therefore the horizon loses its classical geometrical meaning. In this regime a theory of quantum gravity is required.

References

  1. Zeh, H.D. On the interpretation of measurement in quantum theory. Foundations of Physics 1970, 1, 69–76. [CrossRef]
  2. Schlosshauer, M. Quantum decoherence. Physics Reports 2019, 831, 1–57. [CrossRef]
  3. Zurek, W.H. Decoherence, einselection, and the quantum origins of the classical. Reviews of modern physics 2003, 75, 715. [CrossRef]
  4. Schlosshauer, M. Decoherence, the measurement problem, and interpretations of quantum mechanics. Reviews of Modern physics 2004, 76, 1267–1305. [CrossRef]
  5. Ghirardi, G.C.; Rimini, A.; Weber, T. Unified dynamics for microscopic and macroscopic systems. Physical review D 1986, 34, 470. [CrossRef]
  6. Pearle, P. Combining stochastic dynamical state-vector reduction with spontaneous localization. Physical Review A 1989, 39, 2277. [CrossRef]
  7. Diósi, L. Models for universal reduction of macroscopic quantum fluctuations. Physical Review A 1989, 40, 1165. [CrossRef]
  8. Penrose, R. On gravity’s role in quantum state reduction. General relativity and gravitation 1996, 28, 581–600. [CrossRef]
  9. Penrose, R. Wavefunction collapse as a real gravitational effect. In Mathematical physics 2000; World Scientific, 2000; pp. 266–282.
  10. Penrose, R. On the gravitization of quantum mechanics 1: Quantum state reduction. Foundations of Physics 2014, 44, 557–575. [CrossRef]
  11. Donadi, S.; Piscicchia, K.; Curceanu, C.; Diósi, L.; Laubenstein, M.; Bassi, A. Underground test of gravity-related wave function collapse. Nature Physics 2021, 17, 74–78. [CrossRef]
  12. Oppenheim, J. A postquantum theory of classical gravity? Physical Review X 2023, 13, 041040. [CrossRef]
  13. Gambini, R.; Porto, R.A.; Pullin, J. Realistic clocks, universal decoherence, and the black hole information paradox. Physical review letters 2004, 93, 240401. [CrossRef]
  14. Milburn, G. Lorentz invariant intrinsic decoherence. New Journal of Physics 2006, 8, 96. [CrossRef]
  15. Bonifacio, R. Time as a statistical variable and intrinsic decoherence. AIP Conference Proceedings. American Institute of Physics, 1999, Vol. 461, pp. 122–134.
  16. Anastopoulos, C.; Hu, B. Intrinsic and fundamental decoherence: issues and problems. Classical and Quantum Gravity 2008, 25, 154003. [CrossRef]
  17. Anastopoulos, C.; Hu, B. A master equation for gravitational decoherence: probing the textures of spacetime. Classical and Quantum Gravity 2013, 30, 165007. [CrossRef]
  18. Hsiang, J.T.; Cho, H.T.; Hu, B.L. Graviton physics: Quantum field theory of gravitons, graviton noise and gravitational decoherence–a concise tutorial. arXiv preprint arXiv:2405.11790 2024. arXiv:2405.11790 2024.
  19. Blencowe, M. Effective field theory approach to gravitationally induced decoherence. Physical review letters 2013, 111, 021302. [CrossRef]
  20. Hu, B.L.; Paz, J.P.; Zhang, Y. Quantum Brownian motion in a general environment: Exact master equation with nonlocal dissipation and colored noise. Physical Review D 1992, 45, 2843. [CrossRef]
  21. Hu, B.; Matacz, A. Quantum Brownian motion in a bath of parametric oscillators: A model for system-field interactions. Physical Review D 1994, 49, 6612. [CrossRef]
  22. Galve, F.; Zambrini, R.; Maniscalco, S. Non-markovianity hinders quantum darwinism. Scientific reports 2016, 6, 19607. [CrossRef]
  23. Pleasance, G.; Garraway, B.M. Application of quantum Darwinism to a structured environment. Physical Review A 2017, 96, 062105. [CrossRef]
  24. Ciampini, M.A.; Pinna, G.; Mataloni, P.; Paternostro, M. Experimental signature of quantum Darwinism in photonic cluster states. Physical Review A 2018, 98, 020101. [CrossRef]
  25. Caldeira, A.O.; Leggett, A.J. Path integral approach to quantum Brownian motion. Physica A: Statistical mechanics and its Applications 1983, 121, 587–616. [CrossRef]
  26. Arrasmith, A.; Albrecht, A.; Zurek, W.H. Decoherence of black hole superpositions by Hawking radiation. Nature communications 2019, 10, 1024. [CrossRef]
  27. Landau, L.; Lifshitz, E.; Reichl, L. Statistical Physics, Part 1, Butterworth, 1984.
  28. Ford, L.; Svaiter, N. Cosmological and black hole horizon fluctuations. Physical Review D 1997, 56, 2226. [CrossRef]
  29. Hsiang, J.T.; Arısoy, O.; Hu, B.L. Entanglement dynamics of coupled quantum oscillators in independent nonMarkovian baths. Entropy 2022, 24, 1814. [CrossRef]
  30. Ruban, V. Spherically symmetric T-models in the general theory of relativity. General Relativity and Gravitation 2001, 33, 375–394. [CrossRef]
  31. Glatthard, J. Page-curve-like entanglement dynamics in open quantum systems. Physical Review D 2024, 109, L081901. [CrossRef]
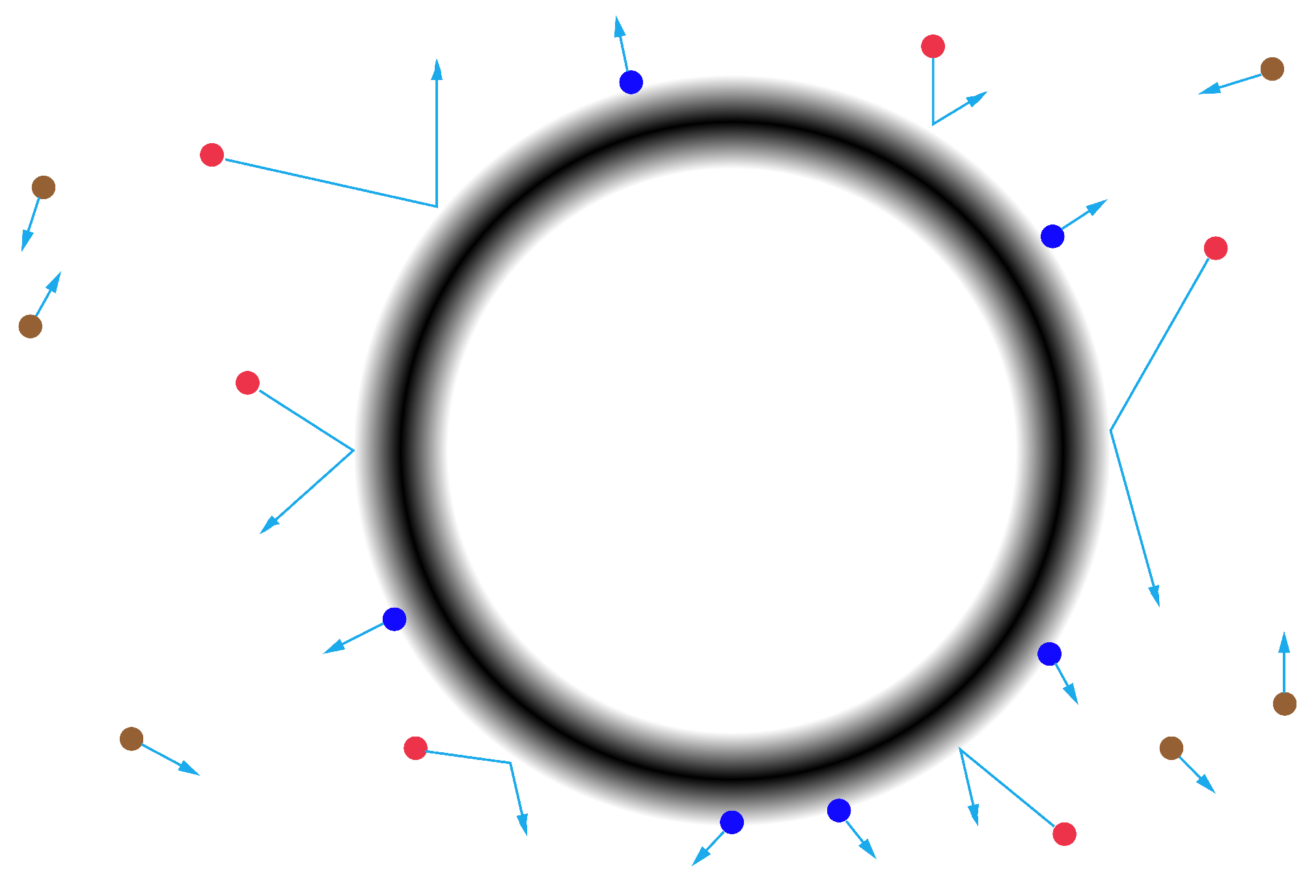
Preprints 120508 g001
Figure 1. A Schwarzschild black hole is immersed in a thermal bath of Hawking radiation particles. Similar to the probability cloud of hydrogen atoms in quantum mechanics, the quantum event horizon (grey ring) is smeared out around the classical event horizon (black circle). The “uncertain” event horizon encodes information about the collapsed matter. The particles from the thermal bath are shown in brown. Information on the location of the event horizon, or equivalently information on the collapsed matter, is carried away by the scattered (red dot) and emitted (blue dot) particles, leading to a decoherence of the event horizon.
Figure 1. A Schwarzschild black hole is immersed in a thermal bath of Hawking radiation particles. Similar to the probability cloud of hydrogen atoms in quantum mechanics, the quantum event horizon (grey ring) is smeared out around the classical event horizon (black circle). The “uncertain” event horizon encodes information about the collapsed matter. The particles from the thermal bath are shown in brown. Information on the location of the event horizon, or equivalently information on the collapsed matter, is carried away by the scattered (red dot) and emitted (blue dot) particles, leading to a decoherence of the event horizon.
Preprints 120508 g001
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.
Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2024 MDPI (Basel, Switzerland) unless otherwise stated