Validity of Centrifuge-based Method for Determining Water Retention Curve of Growing Media

This study presented a centrifuge-based method for determining hydraulic properties in growing media, offering insights into water dynamics in growth substrates. The objectives of this study were to test the practical applicability of the centrifuge method as a quick test for a wide range of growing media, ii) to compare a simple substitution method to describe the bulk density of growing media with results of the standard method, and iii) to give a recommendation under which conditions the centrifuge quick test could serve as a replacement for the standard method. Methods involved centrifugation and correlation analyses. Results showed good agreement between the centrifuge and DIN methods, with notable compression effects at lower pF stages. The method's rapidity (2 days vs. up to 8.5 days) and correlation with standard methods highlight its potential for practical applications. Limitations, such as compression effects, were noted, emphasizing the need for careful interpretation. Overall, the centrifuge method provided a valuable tool for research and substrate development. This study contributes to refining measurement techniques in growth substrates, encouraging ongoing research in water dynamics in growing media.

Keywords:

Subject:

Biology and Life Sciences - Agricultural Science and Agronomy

1. Introduction

In recent years, soilless plant cultivation systems have drawn more attention due to the pressing need for a reliable food supply to satisfy a growing global population, particularly in lesser developed arid and semiarid regions of the world and the growing demand for high-quality, out-of-season vegetables, fruits, and ornamentals in the industrial world. Organic or mineral substrates with low buffer capabilities, good water permeability, high water storage capacity, and appropriate aeration are frequently used in soilless growth systems. For best results, these porous media should be inert to avoid chemical and biological reactions [1]. The rising interest in cultivating fruit crops, greens, herbs, and ornamentals in soilless container systems has further accelerated the growth in global production of crops in controlled environmental systems. They are also utilized to boost metabolites in aromatic and medicinal plants as well as to introduce new crops [2]. As a result, the necessity for innovative research to solve challenges and continue opening doors for this sector is developing, as has the demand for soilless cultivation systems and growing media on a global scale [3]. Physical and chemical properties of various soilless substrate materials may be conveniently altered to create optimal plant growth conditions. Soilless growing media were initially introduced for potted crop production to improve drainage by preserving a relatively high percentage of air-filled porosity (AFP) as compared to mineral soils [4] (p 5).

The hydraulic characteristics of growing media are critical because they have a major influence on air and water access to plant roots and cannot be modified by growers. Furthermore, the volume of growth material per plant is limited, emphasizing the significance of hydraulic parameters. The analytical methods used to assess physical characteristics vary, and various definitions or terminology are frequently utilized [5]. The range of values for physical attributes in organic media differs significantly from those in mineral soils [6]. Determining a substrate's ability to retain and release water is crucial for enhancing greenhouse crop water efficiency. Bunt [7] first described the water retention curve (WRC) of soilless materials, which is determined similar to mineral soils. Because growing media mixtures are more porous and often have bigger diameter pores, they allow water to drain at lower tensions. The analytical suction range is typically performed at lower tensions (0 to -30 kPa) than in mineral soils, while most greenhouse crops uptake the water with matric potentials ranging from -1 kPa to -10 kPa. The reason for extending the tension up to -30 kPa is to develop the water retention curve near converging levels for the curve-fitting purposes.

Soil water is a vital resource in agricultural and horticultural production, and as such, it is the subject of much investigation in soil physics. A better knowledge of water movement and redistribution would optimize soil water retention and agriculture water use efficiency [8,9]. The water retention curve (WRC) describes the relationship between soil water potential and water content under equilibrium conditions. WRC is one of the main physical properties of porous materials that is necessary for modelling water flow and solute transport within soils and growing media [10,11,12]. WRC is also applied to determine the characteristics of numerous hydraulic processes, such as drainage, infiltration, and plant water availability [13]. WRC can be determined either by dehydration or by a water absorbing process, although the dehydration process is commonly used in laboratory [14]. The functional relationship between suction and water content is usually described with several mathematical functions [15,16,17,18]. The WRC can be measured in the laboratory with different methods, such as sandbox, pressure plate, tensiometer, dew point potentiometer, filter paper, suction-controlled triaxial, and centrifuge [14,19,20]. Applying these methods to growing media is made more difficult by the fact that substrates under production conditions are used with different bulk densities and therefore the WRC varies in each case. Standard method to determine the WRC of growing media is a modified sandbox method [21]. This method determines the WRC at a method-specific bulk density and is widely accepted. However, it is a tedious and very time-consuming method. The use of a centrifuge to induce an unsaturated condition inside a soil sample is not new. Soil water release tests utilizing centrifugal flow have long been acknowledged as a valid and effective technique for determining the WRC of mineral soils [11,22,23,24,25]. However, to our best knowledge, very rare, if any studies have investigated the validity of the centrifuge method for determining the WRC of growing media in the laboratory.

Therefore, the objective of this study was i) to compare a simple substitution method to describe the bulk density of growing media with results of the standard method, ii) to test the practical applicability of the centrifuge method as a quick test for a wide range of growing media, and iii) to give a recommendation under which conditions the centrifuge quick test could serve as a replacement for the standard method.

2. Materials and Methods

2.1. The Studied Growing Media

In this study, 16 types of growing media were selected to cover a broad range of particle size, organic matter content and raw materials, including peat free or peat reduced mixtures. The mixtures included commercial potted media for end users (garden market), growing media for commercial producers (wholesale companies), and own mixtures, particularly with wood fiber (Table 1). Basic physical and chemical properties of the growing media were initially characterised (Table 2). pH and EC (electrical conductivity) of the substrates were determined according to [26]. The pH ranged from 3.2 (peat) to 6.5 (bio mixture), EC from 0.05 dS m^-1 (peat) to 1.23 dS m^-1 (Black potting soil), depending on the materials and the fertilizers added. Organic matter percentages were determined according to [27] ranging from 29.35 %mas (green compost) to 99.72 %mas (wood fibres). Based on [28], the mean weighted diameter ranged from very fine (0.97 mm; composted coco fibres) to coarse (4.93 mm; PHP15 substrate) (Table 2). The particle density was estimated from ignition loss [27]. Pore volume, container capacity, air capacity and easily available water were determined according to DIN EN 13041 [21].

2.2. Reference DIN Method (DIN EN 13041, 2011)

The standard method to determine the water retention properties of growing media is the reference DIN method [21]. The procedure is as follows: for each material, two polyethylene vinyl chloride (PVC) pipes (16 cm height, 16 cm diameter) of almost two litres were filled with the substrate without compaction. The filled PVC pipes were placed in a water bath. Water was incrementally added to the water bath from the bottom of the PVC pipes up to one centimetre below the top of the pipe over 30 minutes. The saturation was initiated from the bottom of the pipes to avoid air entrapping within substrate pores. The samples were saturated for 24 hours. After saturation, the samples were subjected to -50 hPa on a sandbox suction table for 48 hours to equilibrate. Each substrate variant was then poured onto a tray and mixed thoroughly. Four double rings were filled with substrate for each variant. The double rings comprised of lower and upper rings. The internal dimensions of each ring were 5 x 10 cm in height and diameter, respectively. The bottom of the lower ring was secured with a non-biodegradable synthetic gauze. All the sample rings were transferred to the sand suction table and pressure heads of -5, -10, and -50 hPa corresponding to pF 0.7, 1.0 and 1.7) were applied. The pressure heads were measured from the centre of the lower ring, for details see [21]. After each individual pressure head and equilibration, the samples were weighed. After the last suction step (pF 1.7), the upper ring was removed, the excess material was carefully removed, and the gravimetric water content and the dry matter of the lower ring material was determined after drying at 105°C for 48 hours. The dry bulk density (BD) was calculated on the basis of the dried sample volume.

Additional pressure heads of -100, -316, -1000, and -3162 hPa corresponding to pF 2.0, 2.5, 3.0, and 3.5 were applied using small rings (1 cm height, 5 cm diameter) in a pressure plate apparatus [29]. The water content/suction results were used to construct the whole water retention curve. The easily available water (EAW) was calculated as the difference of the water content at container capacity (CC, pF 1.0) and at pF 1.7 [21].

2.3. Centrifuge Method

2.3.1. Theoretical Background

A centrifuge bucket is forced to spin in a circular pattern, accelerating towards the sample. As a result, an object within the bucket feels pushed to the bottom of the bucket. This force on the object within the bucket is known as a centrifugal force because it is directed away from the centre of rotation. The centrifugal force develops linearly with distance from the centre and the square of rotational speed [23]. In the centrifuge method, water is removed from the sample under the influence of centrifugal force. [30] was the first investigator who proposed the formula for measuring the soil water retention curve over a wide range of moisture content. But the most used formula for calculating matric potential Ψ or the equivalent water pressure (P) in a porous medium has been recently updated by [25,31] as follows:

P = \frac{ρ_{l} {\cdot ω}^{2}}{2} \cdot (R_{2}^{2} - R_{1}^{2})

(1)

where

ρ_{l}

is the density of the liquid, ω is the angular velocity of the rotation, R₁ is the distance from the rotation axis to the centre of the sample, and R₂ is the distance from the rotation axis to the free surface of the insert tube at the bottom of the sample.

Equation 1 is derived as follows: the water pressure in a sample subjected to centrifugation is equal to the ratio of the force (F) exerted on the water to the cross-section of the sample area (S): P = F/S. It is taken into account that the force F is the product of the liquid mass (

m_{l}

) in the sample by the centrifugal acceleration (a) and that the water area in a cylindrical shape of the sample holder is equal to the quotient of its volume (

V_{l}

) by the height (h), and we have

{P = m}_{l} \cdot

\cdot

h /

V_{l}

. As a = ω² R, where R is the rotation radius from the axis to the centre of the sample mass, and

m_{l}

V_{l}

ρ_{l}

, we obtain:

{P = ρ}_{l} \cdot ω^{2} \cdot R \cdot h

(2)

At different positions in the sample there will be variations in the rotation radius. To overcome such variations, the pressure function can be integrated within the R₁ and R_2, and we obtain (Smagin et al.,1998):

P = \int_{R 1}^{R 2} P d h = \int_{R 1}^{R 2} ρ_{l} ω^{2} R d h = \frac{ρ_{l} ω^{2}}{2} (R_{2}^{2} - R_{1}^{2})

(3)

The centrifuge construction often requires the sample to be positioned at an incline concerning the rotation axis. Consequently, equation (1) should incorporate a correction factor cos(α), where α represents the angle between the horizontal and the central symmetry axis of the sample. In the case of a horizontal rotor spider, α equals 0°, leading to cos(α) being 1. At lower centrifuge speeds, it is essential to consider the gravity component of the pressure (Р_g = - g·h·sin(α)), where h is the sample's height, and g is the acceleration of gravity. The angular velocity (ω) is determined by the equation ω = π·N/30, where ω is in rad·s^–1 and N the centrifuge’s revolutions per min (rpm).

With the density of water as 1000 kg m^-3 and

\frac{ρ_{l \cdot ω^{2}}}{2} = \frac{1000}{2} \cdot {(\frac{π \cdot N}{30})}^{2} = 5.483 \cdot N [P a]

(4)

the ultimate expression for calculating the matrix potential Ψ_m is as follows:

P [h P a] = Ψ_{m} [h P a] = 0.0548 \cdot N^{2} \cdot (R_{2}^{2} - R_{1}^{2}) \cdot \cos α + g \cdot h \cdot \sin α

(5)

where N is the revolutions per minute (rpm), R is in m, and h is in m.

In case of a horizontal centrifuge eq. 5 simplifies to

P [h P a] = Ψ_{m} [h P a] = 0.0548 \cdot N^{2} \cdot (R_{2}^{2} - R_{1}^{2})

(6)

2.3.2. Description of the Centrifuge Apparatus

A simple laboratory centrifuge (Sigma, Germany) was used with control options for speed, time, and temperature. The centrifuge with an operating rotor of 15.3 cm in radius was used in the experiment. The rotor assembly of the centrifuge consisted of four swinging type buckets capable of carrying eight sample tubes in one test run. Each pocket contained an adaptor designed to accommodate two sample tubes (Figure 1).

Each sample tube comprised of a sleeve (external tube) and an insert (internal tube). Although the insert tubes were perforated from bottom, each insert tube was secured with a very fine mesh cloth at the bottom. The fine mesh cloth supports the material and induces drainage at the bottom boundary of the insert tube in order that all water flow coming from the substrate sample is fully transmitted to the collection reservoir located below the sleeve tube. In addition, the upper side of the insert tube was screwed up to the sleeve during the centrifugation. The buckets in the centrifuge can be subjected to different velocities varying from 100 to 5000 rpm. The minimum and maximum suctions that can be applied to the samples range from approximately 3 to 5700 hPa (corresponding to pF 0.5 to pF 3.8). When the centrifuge is spinning, the swinging buckets of the centrifuge acquire horizontal positions. The centrifuge buckets are cautiously weighed to avoid any imbalances. After reaching equilibrium, the sample is weighed. Higher suctions can be produced in the same substrate specimen by increasing the rotational speed and centrifuging the specimens until new equilibrium conditions are reached. At the end of the measurement, the samples are dried at 105°C and the sample dry weight and the gravimetric water content is determined. With the sample dry weight, the gravimetric water content at the previous steps is calculated. The soil suction in the specimen is calculated using eq. [1].

2.3.3. The Measurement Procedure

Prior to centrifugation, the density of the material must be defined to determine the amount which is inserted into the centrifuge tubes. A simple tapping method VDLUFA A 13.2.1 was used [26] . The procedure involves standardized shaking of approximately 250 ml of moist substances in a graduated measuring cylinder, with the substances brought to an optimal water content for potting. The filled graduated cylinder is successively dropped down ten times from a height of 10 cm to adequately settle the substrate inside the cylinder.

The wet density is calculated from mass and volume of the sample. For our measurements, however, we measured the substrate density with 10,15, and 20 taps to compare with the reference method to see which tapping number is the most similar to the density measured with the reference method.

Based on the wet density and the volume of the insert tube (21.69 cm³), the fresh weight of the samples was calculated. The insert tubes were filled with substrates and saturated. For the saturation process, the samples were placed in a tray and water was incrementally added from bottom to avoid air entrap among the substrate pores. The samples were left saturated over 12 hours. Next, the insert tubes were put in the sleeve tubes and the sleeve tubes were screwed up with caps. The samples were submitted to the centrifuge buckets. After each centrifugation, the samples were taken out and weighed with a precision of 0.001 g. The samples were then placed back to the centrifuge and the RPM of the centrifuge was incrementally increased. The volumetric water content of the samples was calculated by multiplying the gravimetric water content by the corresponding dry density of the substrates.

The volumetric water contents of the substrates measured with centrifuge method were compared to the reference method. Moreover, the water contents measured with the two methods were fitted to the van Genuchten model [32] to allow a pairwise comparison of the resulting soil water retention curves, further proving the methods' similarity. Given that the volumetric water content and the suctions are two measured variables, these two variables were assigned in the van Genuchten model to estimate the parameters. The model was expressed as follows (van Genuchten, 1980) [32]

{θ (h) = ϴ}_{r} + \frac{ϴ_{s} - ϴ_{r}}{{[1 + {| α h |}^{n}]}^{m}} for < 7

(7)

where θ_s and θ_r are the fitted saturated and residual water content respectively, α and n are the fitting parameters. α is the inverse of the air entry value, n is the medium pore-size distribution index, and m is usually taken as m=1-1/n. The parameters were estimated using the Microsoft Excel solver procedure.

3. Results and Discussion

3.1. Bulk Density of the Substrates

The bulk densities obtained through different tapping frequencies (10, 15, and 20 times) and the reference DIN method were compared. For an optimum agreement, R² and slope of the regression line should be close to 1 and the bias, root mean square error (RMSE) and bias% should be close to zero. Table 3 indicates a consistently high correlation (R² values approaching 1) and a slope close to 1 between all tapping frequencies and the DIN method.

However, with the highest R² and lowest error, these results collectively emphasize the accuracy and reliability of the 10 times tapping frequency in comparison to the other investigated tapping frequencies.

The 6.15% higher bulk density values obtained through the 10 times tapping method indicated a minor but consistent overestimation compared to the DIN method. While this discrepancy was relatively small, it necessitated consideration in practical applications, where the acceptable margin of error might play a critical role.

To evaluate this discrepancy, the results of interlaboratory trials of the DIN method were used. The reproducibility limit R is the value below or equal to which the magnitude of the difference between two laboratories can be expected with a probability of 95 %. The reproducibility of the bulk density determination of the DIN method was determined as 0.007 to 0.049 g cm-3 with an average of 0.029 g cm-3 for six growing media with very different physical properties [21].

Figure 2 shows the comparison between bulk densities measured with the DIN method and the 10 times tapping method. The green dashed lines denote the average reproducibility interval of the DIN method. Despite the bias of 6.15 % of the tapping method, all values (except 1) were within the reproducibility interval of the standard method. This indicates that the quality of the determination of the bulk density with the quick tapping method is similar to that of the DIN method.

3.2. Bulk Density of the Substrates

To measure each pressure potential level, the running duration for the centrifuge may range from 0.25 to 72 hours, as documented by various studies for mineral soils [22,24,25,31,33,34]. Notably, it was observed that no specific literature sources addressed centrifugation durations for growing media. Considering the unique characteristics of growing media, particularly the presence of more coarse pores compared to mineral soils, the study anticipated a more rapid dewatering process. This expectation served as a foundational hypothesis for the subsequent experimentation. Figure 3 represents the results derived from the examination of a representative mixture comprising 80% peat and 20% wood fibre. Following a mere 10 minutes of centrifugation, a significant portion of the water expected at the given pF stage had already been expelled from the material. This rapid initial dewatering can be attributed to the coarse pores present in the growing media, aligning with the hypothesis formulated based on the unique characteristics of this material. After about 40 minutes, the water content in the growing media reached a state of constancy under practical conditions. This implies that, beyond this duration, further centrifugation did not yield substantial changes in water content. This stabilization of water content after 50 minutes serves as a crucial benchmark, suggesting a sufficient duration of 60 mins for practical measurements of growing media with the centrifugation method.

3.3. Comparison of the Centrifuge Method to the Reference DIN Method

Table 4 provides a detailed breakdown of statistical measures, including R², slope, bias, and RMSE, with respect to differences between both methods in volumetric water content at different pF values, air capacity (AC) and easily available water (EAW). Examining the R² values, it is evident that they predominantly exceed 0.96, indicating a high degree of correlation between the centrifuge and DIN results. This robust correlation reaffirms the reliability of the centrifuge method in assessing volumetric water content in growing media. Notably, exceptions were observed in the case of EAW, where the R² value was marginally lower at 0.9265. This suggested a slightly weaker correlation in this specific scenario, urging further investigation into the factors influencing the results. The slope values, consistently close to unity, signified a proportional relationship between the volumetric water contents obtained from the centrifuge and DIN methods. This alignment reinforced the overall congruence between the two techniques. However, it is crucial to acknowledge variations in bias across different moisture levels. The bias values, generally below +/- 3.5%vol, indicate that centrifuge values tended to be slightly higher than DIN values, with easily available water (EAW) being an exception where the bias reaches 6.5%vol. This discrepancy prompts a closer examination of the specific conditions influencing the accuracy of the methods, particularly in the context of EAW.

As before, some of the discrepancies (pF1, pF 1.7 and AC) could be compared against the reproducibility limits of the results of interlaboratory trials of the DIN method (Table 4) [21], They are generally much higher than the bias and RMSE of the differences between centrifuge and DIN method.

Figure 4 shows the comparison between water content at pF 1.0, pF 1.7 and AC measured with centrifuge and DIN method. The green dashed lines denote the average reproducibility interval of the DIN method. All values (except 2 outliers) were within the reproducibility interval of the standard method. This indicates that the quality of the determination of container capacity (CC) at pF 1.0, water content at pF 1.7 and AC is similar to that of the DIN method.

Examples of water retention curves (WRC) are given in Fig. 5. The results highlight instances where centrifuge WRC exhibited a close resemblance to DIN WRC, such as in the case of pure wood fibre and its mixture (Figure 5 c, d). Conversely, variations emerged in scenarios like potgrond and seedling, where deviations were observed in higher pF ranges (Figure 5 a, b), or bio mixture and peat where the whole WRC of the centrifuge method lies slightly above or below the DIN method (Figure 5 e, f). This divergence emphasises the importance of considering specific growing media characteristics that may influence the performance of the methods differently. The overall similarity between the centrifuge WRC and DIN values is acknowledged, affirming the reliability of the centrifuge method in capturing water retention characteristics.

The results show generally a good reliability of the centrifuge method giving similar result as the standard method. However, the identified exceptions and variations in specific scenarios highlighted the importance of a precise interpretation, taking into account the unique characteristics of different growing media. A possible reason for some deviations could be the material’s compression which takes place during the centrifugation process and may modify the pore system.

3.4. Compression during Centrifugation

Compared to sand table or pressure plate systems, the centrifugation forces do not act only on the water, but also on the material of the growing media itself resulting in an increasing compression of the material with increasing rotation speed and increasing pF values. Compression will lead to increasing bulk density in the course of the measurement. This may have an effect on the WRC as the pore system is modified. However, experiences with mineral soils show that the effect of bulk density plays an important role at lower tensions but is insignificant at higher tensions because water adsorption principle changes gradually from capillary storage (depending on pore system) to adsorption at particles (pore system independent) [14]. Figure 6 illustrates the impact of centrifugation on substrate compression across different pF levels. At the lower end (< pF 2.0), the compression was less pronounced, ranging from less than 5% for materials like Coco fibre, Wood fibre, and Typha, to a maximum of 15% for seedling and clay substrate at pF 2.0. At pF 1.0, corresponding to CC and AC, the compaction values were much smaller. However, a more distinct compression was observed between pF 2.0 and pF 3.5, reaching up to nearly 50% for clay substrate and 35% for Wood fibre.

In the subsequent analysis (Table 5), the correlation between bias (centrifuge results deviating systematically from DIN results) and compression at various pF stages was explored based on all materials. At the lower pF stages, namely pF 1.0, pF 1.7, and pF 2.5, a significant correlation between compression (as a percentage of the original volume) and bias was identified. The correlation coefficient (R²) values for these stages were small (0.2541, 0.1342, and 0.0775, respectively) and indicating a decreasing strength of correlation towards higher pF stages. However, at higher pF stages (pF 3.0 and pF 3.5), no significant correlation between compression and bias was observed, despite the relative compression being higher at these stages. There is also no correlation between compression and EAW.

The water retention curves (WRC, Fig. 5) for all materials depicted that the major water loss occurred between saturation (pF 0) and pF 2.5. Beyond pF 2.5, the WRC exhibited a near-vertical trend, signifying minimal water loss. The water remaining between pF 2.5 and pF 4.2 is strongly bound to particles, making the compression of pores emptied during centrifugation inconsequential to the water content of the samples. As a result, the pronounced compression during centrifugation, particularly at higher pF stages, could be disregarded.

The analysis underscored the importance of considering compression effects, primarily at lower pF stages (pF 1.0, pF 1.7, and pF 2.5), when interpreting results obtained through the centrifuge method. The diminishing strength of correlation at higher pF stages suggested that the relative compression, despite being more substantial, did not significantly impact the bias between centrifuge and DIN methods.

Although compression modifies the pore system and may have some effect on the water storage properties, the good correspondence between the centrifuge and the reference method also at lower pF stages shows that this is insignificant for practical purposes.

4. Conclusions

The investigation into the hydraulic properties of growing media, specifically focusing on bulk density, centrifugation duration, and the comparison of the centrifuge method with the reference DIN method, provides valuable insights with practical implications for researchers and practitioners in growing media.

With respect to the objectives of the study it can be concluded:

i) The analysis of bulk density revealed a strong correlation between the quick tapping and the DIN method. Notably, the 10 times tapping frequency stood out with optimal slope, minimal bias, and low RMSE, indicating its superior performance in capturing variations in bulk density. The results, supported by statistical indicators and visual representations, position the 10 times tapping method as the most accurate and reliable among the investigated frequencies.

ii) The centrifugation method can be used as a quick test for a wide range of growing media. The study on centrifugation duration in growing media showcased a rapid initial dewatering process, with water content stabilizing after 50 minutes. This finding suggests an optimal duration for efficient dewatering of about one hour for practical applications.

The comparison of the centrifuge method with the DIN method demonstrated a high correlation, generally close slopes, and low biases, affirming the effectiveness of the centrifuge method in assessing volumetric water content and water retention curves in growing media. The findings show that centrifuge and DIN methods are fundamentally correlated, with variations ranging from 3 to 6%vol for low pF levels to <1.6%vol for higher pF values. The vast majority of the results were within the reproducibility interval of the standard method indicating that the quality of the centrifuge method is similar to that of the reference method. However, exceptional materials, such as coarse pine bark, were not part of this investigation and may need further investigation with respect to reliability of the method and necessary size of the centrifuge tubes.

Compression during centrifugation emerged as a factor influencing outcomes, particularly at lower pF stages. The study highlighted a significant correlation between compressed volume and bias at lower pF stages, suggesting the need to consider compression effects in result interpretation. The diminishing correlation at higher pF stages indicated a lesser impact of compression on bias. However, good agreement between centrifuge and standard method shows that the effect of compaction seems to be not important for practical applications.

iii) Recommendation for the practical use of the centrifuge method: The centrifuge method takes only around 2 days to determine pF 1.0 (container capacity CC), air capacity (AC), pF 1.7 (easily available water) and more pF values (pF 2.5, 3.0, and 3.5) to describe the whole WRC. This is a major improvement over the up to 8.5 days required by the usual DIN approach (only for CC, AC and EAW). Only a speed-regulated laboratory centrifuge and suitable centrifuge inserts are necessary for the process.

In this study, only growing media with mean weighted diameters up to about 5 cm were tested with thin and flexible fibres in standard centrifuge tubes with 2.35 cm diameter and 5 cm height. Coarse and rigid materials, such as pine bark, may react differently, which needs to be tested. Also, coarse and larger materials might need larger centrifuge tubes which can be accomplished without much effort.

Because there is currently no DIN or recognized lab regulation, the centrifuge method cannot be used in place of the DIN process for legally compliant measurements. However, the centrifuge method turns out to be a very helpful and time-saving tool in fields like research and substrate development.

Author Contributions

Hadi Hama Aziz Muhammed: Writing - original draft; visualization. Lisa Schumm: methodology; Investigation. Ruediger Anlauf: Supervision; conceptualization; Formal analysis. Tobias Reineke: data curation; validation. Diemo Daum: Funding acquisition; project administration.

Funding

The study was partly financed by the German Federal Ministry of Food and Agriculture (BMEL), Project promotor FNR, Project NaKuHo, FKZ 2220MT001A.

Data Availability Statement

Data supporting this work will be made available upon request.

Conflicts of Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

References

Chamindu Deepagoda, T.; Chen Lopez, J.C.; Møldrup, P.; Jonge, L.W. de; Tuller, M. Integral parameters for characterizing water, energy, and aeration properties of soilless plant growth media. Journal of Hydrology 2013, 502, 120–127. [Google Scholar] [CrossRef]
Gruda, N.S. Advances in Soilless Culture and Growing Media in Today’s Horticulture—An Editorial. Agronomy 2022, 12, 2773. [Google Scholar] [CrossRef]
Gruda, N.S.; Fernández, J.A. Optimising Soilless Culture Systems and Alternative Growing Media to Current Used Materials. Horticulturae 2022, 8, 292. [Google Scholar] [CrossRef]
Soilless culture: Theory and practice; Raviv, M. ; Lieth, J.H.; Bar-Tal, A., Ed.; 2nd ed.; Academic Press an imprint of Elsevier: Amsterdam, 2019. [Google Scholar]
Barrett, G.E.; Alexander, P.D.; Robinson, J.S.; Bragg, N.C. Achieving environmentally sustainable growing media for soilless plant cultivation systems – A review. Scientia Horticulturae 2016, 212, 220–234. [Google Scholar] [CrossRef]
Caron, J.; Price, J.S.; Rochefort, L. Physical Properties of Organic Soil: Adapting Mineral Soil Concepts to Horticultural Growing Media and Histosol Characterization. Vadose Zone Journal 2015, 14, vzj2014–10. [Google Scholar] [CrossRef]
Bunt, A.C. Some physical properties of pot-plant composts and their effect on plant growth: I. Bulky physical conditioners. Plant and soil 1960, 13, 322–332. [Google Scholar] [CrossRef]
Lai, Y.; Garg, A.; Chang, K.; Xing, X.; Fan, J.; Liang, J.; Yu, M. A novel method to inverse the water retention curves with consideration of volume change during centrifuge testing. Soil Sci. Soc. Am. j. 2021, 85, 207–216. [Google Scholar] [CrossRef]
Liang, J.; Xing, X.; Gao, Y. A modified physical-based water-retention model for continuous soil moisture estimation during infiltration: experiments on saline and non-saline soils. Archives of Agronomy and Soil Science 2020, 66, 1344–1357. [Google Scholar] [CrossRef]
Bayat, H.; Mazaheri, B.; Mohanty, B.P. Estimating soil water characteristic curve using landscape features and soil thermal properties. Soil and Tillage Research 2019, 189, 1–14. [Google Scholar] [CrossRef]
Khanzode, R.M.; Vanapalli, S.K.; Fredlund, D.G. Measurement of soil-water characteristic curves for fine-grained soils using a small-scale centrifuge. Can. Geotech. J. 2002, 39, 1209–1217. [Google Scholar] [CrossRef]
Rezanezhad, F.; Price, J.S.; Quinton, W.L.; Lennartz, B.; Milojevic, T.; van Cappellen, P. Structure of peat soils and implications for water storage, flow and solute transport: A review update for geochemists. Chemical Geology 2016, 429, 75–84. [Google Scholar] [CrossRef]
Blanco, C.M.G.; Gomez, V.M.B.; Crespo, P.; Ließ, M. Spatial prediction of soil water retention in a Páramo landscape: Methodological insight into machine learning using random forest. Geoderma 2018, 316, 100–114. [Google Scholar] [CrossRef]
Methods of soil analysis, part 4, physical methods; Dane, J. H. and Topp, G.C., Ed.; Soil Science Society of America, Inc., Madison, USA: Madison, USA, 2002. [Google Scholar]
Brooks, R.H.; Corey, A.T. Hydraulic properties of porous media. Hydrology Paper No. 3. Civil Engineering Department, Colorado State University, Fort Collins, CO, 1964. [Google Scholar]
van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. j. 1980, 44, 892–898. [Google Scholar] [CrossRef]
Fredlund, D.G.; Xing, A. Equations for the soil-water characteristic curve. Can. Geotech. J. 1994, 31, 521–532. [Google Scholar] [CrossRef]
Kosugi, K. Three-parameter lognormal distribution model for soil water retention. Water Resour. Res. 1994, 30, 891–901. [Google Scholar] [CrossRef]
Khaksar Najafi, E.; Arabani, M.; Chenari, R.J. Investigation of Water-Retention Characteristics of Alkali-Activated Clay-Fly Ash Using Small Geotechnical Centrifuge. J. Mater. Civ. Eng. 2022, 34. [Google Scholar] [CrossRef]
Zhang, X.; Mavroulidou, M.; Gunn, M.J. A study of the water retention curve of lime-treated London Clay. Acta Geotech. 2017, 12, 23–45. [Google Scholar] [CrossRef]
DIN EN 13041. Soil Improvers and Growing Media-Determination of Physical Properties-Dry Bulk Density, Air Volume, Water Volume, Shrinkage Value and Total Pore Space; Brussels., 2011 ((13041).
Amonette, J.E.; Flury, M.; Zhang, J. A Rapid Test for Plant-Available Water-Holding Capacity in Soil-Biochar Mixtures. A report for The Waste to Fuels Technology Partnership 2017–2019 Biennium: Advancing Organics Management in; Washington State. P, 2019.
Nimmo, J.R.; Perkins, K.; Lewis, A.M. Steady-state centrifuge [simultaneous determination of water transmission and retention properties--direct methods--laboratory]. In Methods of soil analysis, part 4, physical methods; Dane, J.H. and Topp, G.C., Ed.; Soil Science Society of America, Inc., Madison, USA: Madison, USA, 2002; pp. 903–916. [Google Scholar]
Reatto, A.; Da Silva, E.M.; Bruand, A.; Martins, E.S.; Lima, Jorge Enoch Furquim Werneck. Validity of the centrifuge method for determining the water retention properties of tropical soils. Soil science society of America journal 2008, 72, 1547–1553. [Google Scholar] [CrossRef]
Smagin, A.V. Column-centrifugation method for determining water retention curves of soils and disperse sediments. Eurasian Soil Sc. 2012, 45, 416–422. [Google Scholar] [CrossRef]
VDLUFA, 1991. VDLUFA (Association of German Agricultural Analytic and Research Institutes) (1991) Methods Book I "Soil Analysis" (1st-6th supplement delivery), 4th edn. VDLUFA-Verlag, Darmstadt.
DIN EN 13039. Soil Improvers and growing media – Determination of organic content and ash. German Version.; Beuth, Berlin, 2009 ((13039).
Kemper, W.D.; Rosenau, R.C. Aggregate stability and size distribution. Methods of soil analysis: Part 1 Physical and mineralogical methods 1986, 5, 425–442. [Google Scholar]
DIN EN ISO 11274. Soil quality – Determination of the water-retention characteristic – Laboratory methods., 2019 ((11274).
Gardner, R. A method of measuring the capillary tension of soil moisture over a wide moisture range. Soil Science 1937, 43, 277–284. [Google Scholar] [CrossRef]
Smagin, A.V.; Sadovnikova, N.B.; Ali, M.M.B. The determination of the primary hydrophysical function of soil by the centrifuge method. Eurasian Soil Sc. 1998, 31, 1237–1244. [Google Scholar]
van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil science society of America journal 1980, 44, 892–898. [Google Scholar] [CrossRef]
Nimmo, J.R. Experimental testing of transient unsaturated flow theory at low water content in a centrifugal field. Water Resources Research 1990, 26, 1951–1960. [Google Scholar] [CrossRef]
Bowers, W.H.; Mercer, J.J.; Pleasants, M.S.; Williams, D.G. A combination of soil water extraction methods quantifies the isotopic mixing of waters held at separate tensions in soil. Hydrol. Earth Syst. Sci. 2020, 24, 4045–4060. [Google Scholar] [CrossRef]

Figure 1. A schematic view of the centrifuge and the sleeve and insert tubes.

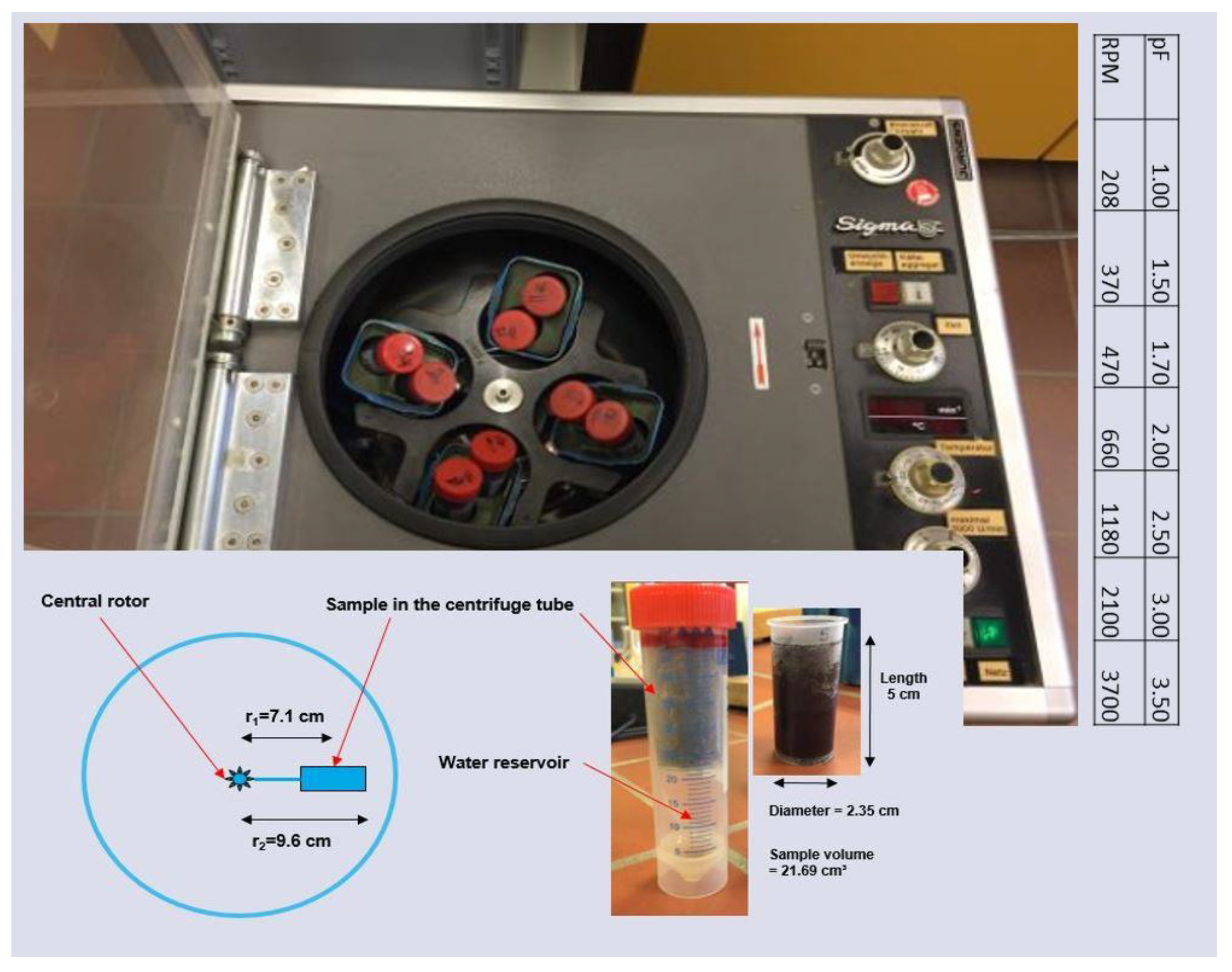
Figure 2. Comparison of the bulk densities measured with DIN method and tapping method. The green dashed lines denote the average reproducibility interval of the DIN method.

Figure 3. Volumetric water content of the representative mixture against centrifugation duration.

Figure 4. Comparison of the volumetric water content at pF 1.0 and pF 1.7 and AC measured with DIN method and centrifuge method for the 16 different growing media. The green dashed lines denote the average reproducibility interval of the DIN method.

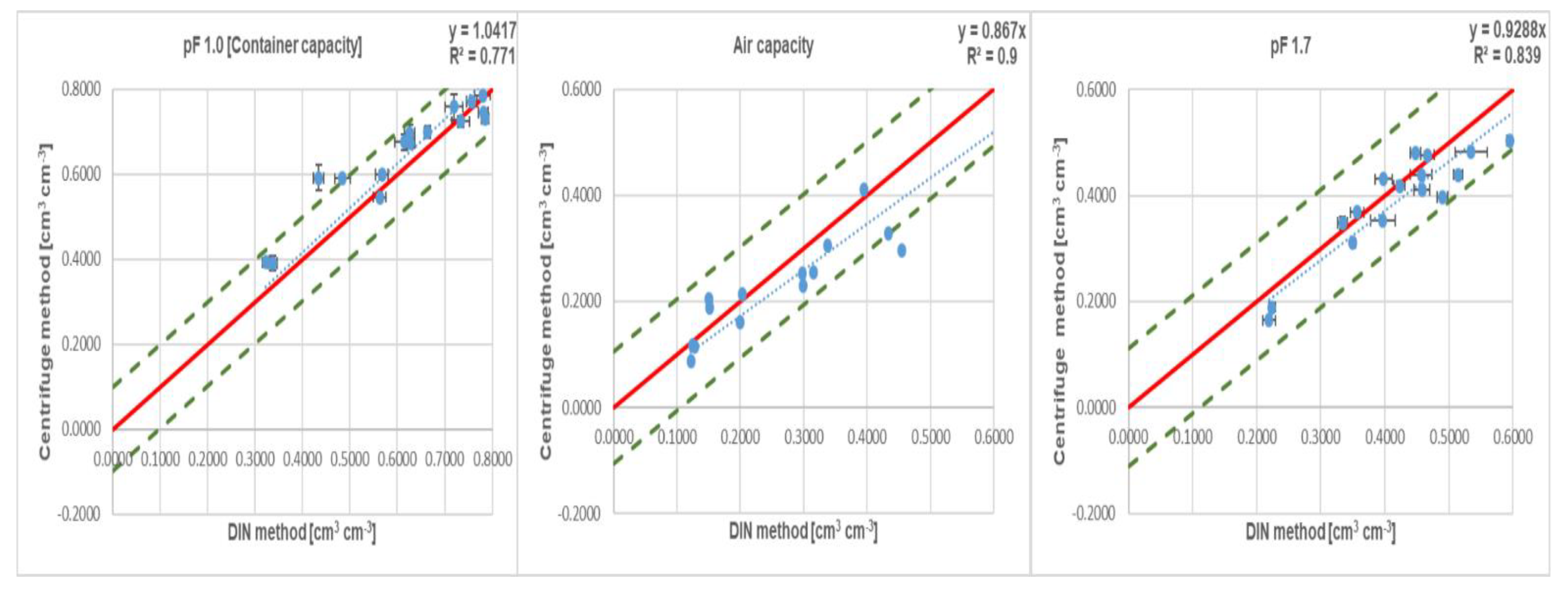
Figure 5. Fitted and measured WRC data for selected growing media.

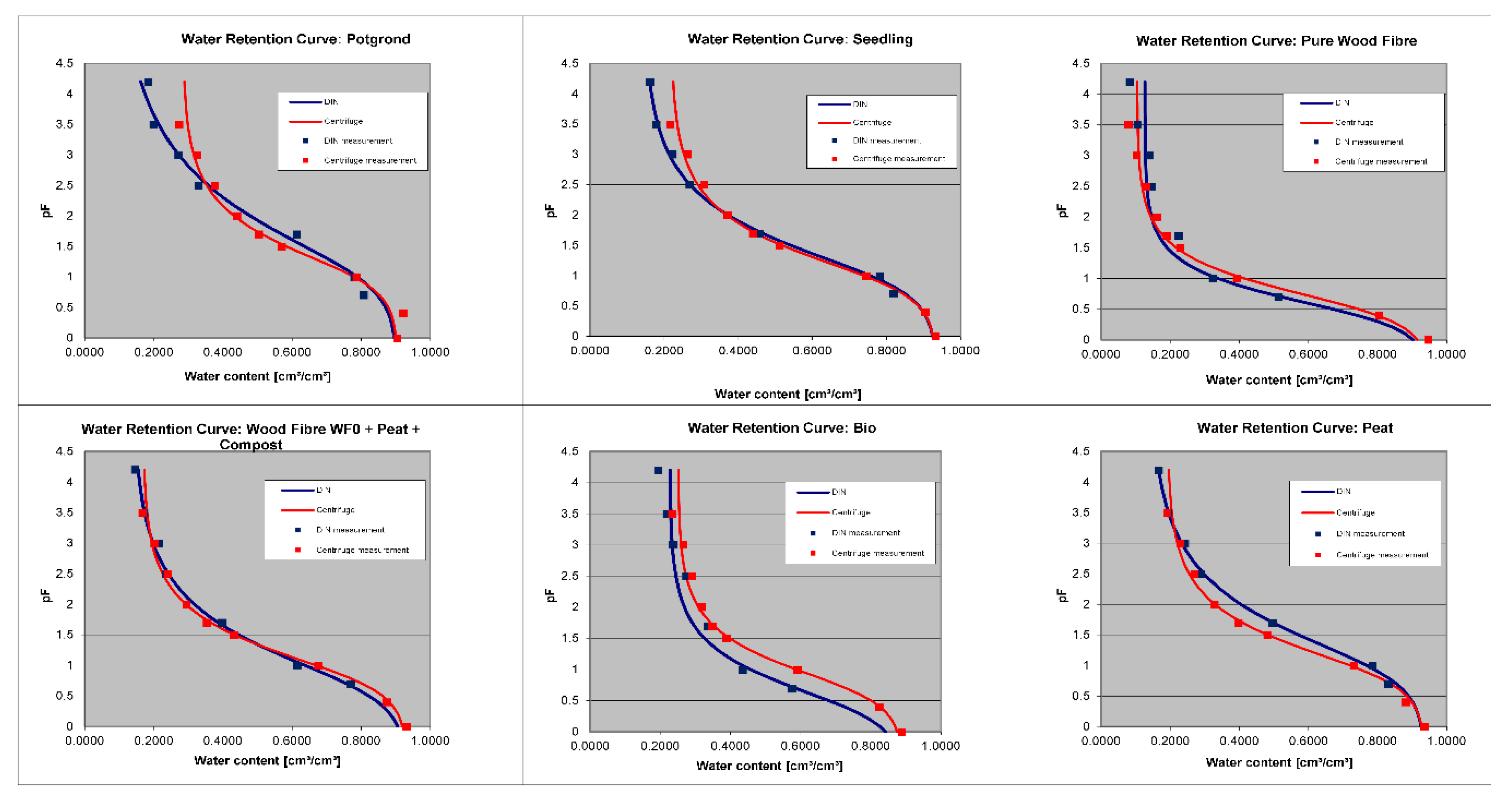
Figure 6. Impact of the centrifugation on the substrate’s compression.

Table 1. Type and composition of the growing media.

Substrate	Composition	Type	Source
Peat	100 %vol black peat	Wholesale (comm. farms)	Floragard
WF0	100 %vol untreated wood fibre	own mixture	---
WF0_mixt.	47.5 %vol untreated wood fibre, 47.5% black peat, 5 %vol green compost	own mixture	---
WF4	100 %vol thermally treated wood fibre	own mixture	---
WF4_mixt.	47.5 %vol thermally treated wood fibre, 47.5% black peat, 5 %vol green compost	own mixture	---
Black Potting Soil	98 % black peat, decorative material for covering grave areas	Garden market (end user)	Dehner Gartencenter
Potgrond	100 % black and white peat, used mainly for press pots	Wholesale (comm. farms)	Klasmann-Deilmann
Clay substrate	80%vol white and black peat, 20 %vol clay granules	Wholesale (comm. farms)	Klasmann-Deilmann
RHP15: special mixture	Black and white sphagnum peat, perlite, GreenFibre, TerrAktiv, and Argile	Wholesale (comm. farms)	Klasmann-Deilmann
Seedling	80 % black and white peat, 20%vol coco fibre, mainly used for vegetable seedlings	Wholesale (comm. farms)	Klasmann-Deilmann
Bio mixture	green compost, coco fibre, composted pine bark, wood fibre, perlite, clay; percentages not given	Garden market (end user)	Dehner Gartencenter
Coco fibre	100 %vol composted coco fibre	Garden market (end user)	Pokon Naturado, NL
Compost	100 %vol green compost	Wholesale (comm. farms)	Klasmann-Deilmann
Typha_mixt.	30%vol shredded Typha, 30 %vol composted coco fibre, 10%vol compost, 30 %vol composted pine bark	own mixture	---
MiscTyPt	15%vol Miscanthus compost, 15 %vol shredded Typha, 70% black peat	own mixture	---
Misc_mixt.	30%vol Miscanthus compost, 30 %vol composted coco fibre, 10%vol compost, 30 %vol composted pine bark	own mixture	---

Table 2. Basic physical and chemical properties of the growing media.

Substrate	pH	EC	Organic Matter	Particle density	MWD	Pore volume	Container capacity	Air capacity	Easily available water
	---	[dS m^-1]	[%mas]	[g cm^-3]	[mm]	[cm³.cm^-3]	[cm³.cm^-3]	[cm³.cm^-3]	[cm³.cm^-3]
Peat	3.2	0.05	96.47	1.57	1.18	0.935	0.785	0.151	0.295
WF0	4.1	0.07	99.72	1.55	2.87	0.946	0.323	0.623	0.099
WF0_mixt.	3.8	0.11	81.43	1.68	1.71	0.930	0.615	0.316	0.218
WF4	4.3	0.16	99.47	1.55	1.30	0.942	0.337	0.605	0.118
WF4_mixt.	3.9	0.13	83.56	1.66	1.19	0.925	0.627	0.298	0.277
Black Potting Soil	4.8	1.23	77.34	1.71	2.10	0.885	0.757	0.128	0.223
Potgrond	5.6	0.57	91.22	1.61	1.66	0.904	0.780	0.124	0.184
Clay substrate	5.8	0.57	68.83	1.78	3.62	0.918	0.720	0.199	0.253
RHP15	5.9	0.29	65.09	1.81	4.93	0.924	0.626	0.298	0.203
Seedling	5.7	0.28	89.91	1.62	2.08	0.933	0.781	0.151	0.325
Bio mixture	6.5	0.80	51.67	1.93	2.94	0.888	0.434	0.454	0.100
Coco fiber	4.9	0.21	88.76	1.63	0.97	0.958	0.563	0.395	0.206
Compost	7.4	0.53	29.35	2.19	1.05	0.785	0.664	0.122	0.149
MiscTyPt	3.4	0.12	95.90	1.58	1.52	0.936	0.734	0.203	0.276
Typha_mixt.	6.4	0.21	65.35	1.81	2.52	0.918	0.484	0.433	0.086
Misc_mixt.	6.3	0.15	69.63	1.77	2.80	0.905	0.568	0.337	0.120

Table 3. The statistical indicators for the comparison between the tapping method and DIN method with respect to bulk density.

Tapping frequency	R²	slope	bias Din vs. Tap	RMSE	bias%
tap 10	0.9908	1.0534	-0.0094	0.0131	-6.15
tap 15	0.9882	1.0883	-0.0157	0.0188	-10.34
tap 20	0.9865	1.1159	-0.0202	0.0235	-13.26

Table 4. The statistical measures for comparison between DIN method and centrifuge method with respect to volumetric water content.

pF value	R²	slope	bias centr. vs. DIN	RMSE	Reproducibility range of the DIN method	Average reproducibility limit of the DIN method
			%vol	%vol	%vol	%vol
pF1.0	0.9926	1.0417	3.5	6.3	9.13 - 10.68	9.80
pF1.7	0.9908	0.9288	-2.8	4.9	8.89 - 15.46	11.09
pF2.5	0.9946	1.0658	1.6	2.9	---	---
pF3.0	0.9877	1.054	1.0	3.2	---	---
pF3.5	0.9684	1.0188	0.6	4.0	---	---
EAW	0.9265	1.0743	6.5	8.1	---	---
AC	0.9795	0.8670	-3.5	6.3	9.31 - 11.94	10.62

Table 5. Correlation of bias (centrifuge method – DIN method) with compression.

pF value	R²	sign. at 95%
pF 1.0	0.2541	*
pF 1.7	0.1342	*
pF 2.5	0.0775	*
pF 3.0	0.0142	-
pF 3.5	0.0162	-
EAW	0.0009	-
AC	0.1832	*

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

Alerts

MDPI Initiatives

Important Links

Choose an area of interest and we will send you notifications of new preprints at your preferred frequency.

Disclaimer