Prerpints.org logo
Preprint
Article

A Model and Quantitative Framework for Evaluating Iterative Steganography

This version is not peer-reviewed.

Submitted:

18 November 2024

Posted:

19 November 2024

You are already at the latest version

A peer-reviewed article of this preprint also exists.

Abstract
This study presents a comprehensive characterization of iterative steganography, a distinct class of information-hiding techniques, and proposes a formal mathematical model for their description. We introduce a novel quantitative measure, the Incremental Information Function (IIF), designed to evaluate information gain in iterative steganographic methods. The IIF provides a comprehensive framework for analyzing the step-by-step process of embedding information into a cover medium, focusing on the cumulative effects of each iteration in the encoding and decoding cycles. The practical application and efficacy of the proposed method are demonstrated through detailed case studies in video steganography. These examples illustrate the utility of the IIF in delineating the properties and characteristics of iterative steganographic techniques. Our analysis reveals that the IIF effectively captures the incremental nature of information embedding and serves as a valuable tool for assessing the robustness and capacity of steganographic systems. This research offers significant insights into the field of information hiding, particularly in the development and evaluation of advanced steganographic methods. The IIF emerges as a promising analytical tool for researchers, providing a quantitative approach to understanding and optimizing iterative steganographic techniques.
Keywords: 
steganography
;  
information quantification
;  
iterative steganography
;  
Incremental Information Function (IIF)
;  
steganographic modeling
;  
information hiding
Subject: 
Computer Science and Mathematics  -   Computer Science

1. Introduction

Steganography constitutes a well-established field of study [1] within the broader framework of information theory [2]. The primary focus of this discipline lies in the development and analysis of methods and techniques for concealing information within overt covers in such a manner that third parties remain oblivious to the existence of the hidden data [3].

1.1. Steganography

A diverse array of steganographic techniques has been extensively documented in the literature [4]. The fundamental objective of these methods is to encode information within a digital cover medium, thereby generating a steganogram that exhibits a high degree of similarity to the original cover. This similarity is designed to render the detection of embedded messages extremely challenging or practically infeasible [5]. The selection of an appropriate steganographic technique is contingent upon multiple factors [6]. These factors include the nature of the cover medium, which may encompass text files, digital images, audio streams, video sequences, or network transmissions. Additionally, the choice is influenced by specific requirements pertaining to the desired properties of the steganographic method, such as capacity, robustness, and undetectability [7].
We employ three fundamental metrics to characterize the core properties of various steganographic techniques. These metrics are often interrelated and subject to trade-offs in steganographic system design. The optimal balance among these properties depends on the specific requirements of the application and the anticipated threats to the steganographic communication channel:
  • undetectability: this metric quantifies a steganographic technique's capacity to conceal information within a steganogram such that its presence remains imperceptible to both human perception and statistical detection methods [8]. High undetectability indicates that the alterations introduced into the cover medium by the steganographic process are minimally discernible and resistant to detection [9],
  • capacity: this metric measures the volume of information that can be embedded within a digital cover medium without inducing perceptible degradation in its quality. The capacity is contingent upon both the properties of the cover medium and the specific steganographic technique employed. While high capacity allows for greater information concealment, it may inversely affect undetectability by increasing the risk of detection [10],
  • robustness: this metric assesses a steganographic technique's ability to preserve the embedded information within a steganogram when subjected to various disruptions or transformations, including but not limited to compression, format conversion, filtering, rotation, or scaling. Enhanced robustness implies that the embedded message is more likely to remain intact and undistorted after such modifications, or that a larger proportion of the original information will be retained [11].
A typical steganographic method is defined by two core functions: an encoding function, which embeds the message within the cover medium, and a decoding function, which retrieves the embedded message from the steganogram:
  • encoding function - maps a given cover object and a message to a steganogram. The encoding process embeds the message within the cover medium while minimizing perceptible alterations,
  • decoding function - extracts the embedded message from a given steganogram. The decoding function is designed to accurately retrieve the message without requiring access to the original cover object. It is noteworthy that the decoding function can also be conceptualized as an integral component of the steganalysis process, serving as a means to verify the presence and content of hidden information [12].
The efficacy of these functions is evaluated based on their ability to maintain the integrity of the cover medium, maximize the capacity for hidden information, and resist detection by steganalysis techniques. The design of these functions often involves complex trade-offs between undetectability, capacity, and robustness.

1.2. Iterative Steganography

Iterative steganography represents a distinct approach to information hiding, differing from traditional techniques that encode data within a single, complete unit, such as individual images [13] or text documents [14]. This method is applicable to covers with an inherent iterative structure, comprising multiple repeated elements over time. In iterative steganography, information is encoded incrementally, with the cover divided into homogeneous segments, each containing partial encoded information. Complete retrieval of the hidden message is only achievable after multiple iterations.
Prominent examples of iterative steganography include video steganography [15], where individual video frames serve as natural iterations for encoding information or network steganography [16] utilizing repeated network packet calls as iterations in the steganographic process.
A fundamental aspect of iterative steganography is the temporal dimension, which organizes consecutive iterations. Any repetitive phenomenon with regular information transmission intervals can potentially serve as an effective cover for iterative steganography.
In steganographic methods based on iterative algorithms, each iteration involves two opposing processes:
  • information increment: resulting from decoding the data encoded in the steganogram,
  • information degradation: due to transformations or interference with the steganogram.
The success of hidden information retrieval is contingent upon the rate of information gain exceeding the rate of degradation, and the decoded information level reaching a critical threshold for complete message recovery.
This study aims to develop a formal method for quantifying the differential between information gain and loss in each iteration of iterative steganographic techniques, with the goal of determining whether the incremental accumulation of information across successive decoding iterations can provide meaningful insights into their characteristics and performance. By introducing the Incremental Information Function (IIF), this research establishes a comprehensive framework for analyzing information increments in iterative steganographic processes. Unlike traditional steganography, which focuses on single instance encoding, iterative methods require a distinct approach to understanding how information is progressively embedded and extracted over multiple iterations. Practical examples are presented to demonstrate the applicability of the proposed method, offering a robust tool for evaluating and optimizing the efficiency, robustness, and capacity of iterative steganographic systems.

2. A Model for Iterative Steganography

We assume that, in the context of steganography, individual iterations in iterative covers are homogeneous - that is, they are identical in terms of structure, properties, and characteristics relevant to the encoding and decoding algorithms.

2.1. Iterative Cover

We define the iterative cover  c i as an i -element vector (see Equation 1).
c i = c 1 ,   c 2 , . . . , c i
where: c i – the i -th iteration of the cover c i corresponds to the i -th encoding step.
We can regard an iterative cover as a vector of iteration, formed by sequentially appending each iteration from the first to the last (see Equation 2).
⋀ i ∈ 1 . . . Z c i = c 1   , i = 1 c i − 1 ⨁ c i   , i ∈ 2 . . . Z
⋀ i ∈ 1 . . . Z c i ∈ I ,   I = 0,1 * ⋀ i ∈ 1 . . . Z c i ∈ C , C = I * Z ∈ N
where: Z – number of iterations of the cover c i = number of encoding steps,
I – the set of all iterations,
C – the set of all iterative covers.

2.2. Message

We define the message  m as a vector of L bits (see Equation 3).
m = m 1 ,   m 2 ,   … ,   m k , . . . , m L
⋀ k ∈ 1 . . . Z m k ∈ 0,1 L ∈ N ,   m ∈ M , M = 0,1 *
where: L – number of bits in the message m (message length),
m k – the k -th bit of message m ,
M – the set of all messages.
Let define m 1 as a number of one-bites in message m (see Equation 4).
m 1 = ∑ k = 1 L 1 m k = 1
where: L – number of bits in the message m (message length),
m k – the k -th bit of message m .
Let define m 0 as a number of zero-bites in message m (see Equation 5).
m 0 = ∑ k = 1 L 1 m k = 0
m 0 + m 1 = L
where: L – number of bits in the message m (message length),
m k – the k -th bit of message m .

2.3. Iterative Steganogram

We define the iterative steganogram  s i analogously as iterative cover c i as an i -element vector (see Equation 6).
s i = s 1 ,   s 2 , . . . , s i
where: s i – the i -th iteration of the s i corresponds to the i -th decoding step.
We can regard an iterative steganogram as a vector of iteration, formed by sequentially appending each iteration from the first to the last. (see Equation 7).
⋀ i ∈ 1 . . . Z s i = s 1   , i = 1 s i − 1 ⨁ s i   , i ∈ 2 . . . Z
⋀ i ∈ 1 . . . Z s i ∈ I ⋀ i ∈ 1 . . . Z s i ∈ S , S = I * Z ∈ N
where: Z – number of iterations of the s i = number of decoding steps,
I – the set of all iterations,
S – the set of all iterative steganograms.

2.4. Coding Indices

From the set of all possible indices of the iterative cover and simultaneously the iterative steganogram, let us select a subset, which we will refer to as the coding indices (see Equation 8).
Ι ⊆ 2 . . . Z
Ι ∈ 2 2 . . . Z
where: Ι – the set of coding indices.

2.5. Encoding and Decoding Iterations

From the set of all iterations in cover and the set of all iterations in steganogram, let us select subsets using coding indices, which we will refer to as the encoding and decoding iterations. (see Equation 9 and Equation 10).
c I = c i : i ∈ I
s I = s i : i ∈ I
where: c I – encoding iterations represented as a subset of all cover iterations, selected using the coding indices I ,
s I – decoding iterations represented as a subset of all steganogram iterations, selected using the coding indices I .

2.6. Encoding Function

We assume that in each iteration, the entire message m is encoded. In general, it is also possible to consider cases where the message m is divided into disjoint parts, and each part is treated as a separate message subjected to independent encoding and decoding.
First, let us define the encoding function  f ' , which encodes the message m in the i -th iteration of the cover (see Equation 11).
f ' : I × M → I
⋀ i ∈ 1 . . . Z s i : = f ' c i , m
where: c i – the i -th iteration of the cover corresponds to the i -th encoding step,
s i – the i -th iteration of the steganogram corresponds to the i -th decoding step,
m – the message to encode in i -th iteration,
Z – number of encoding steps.
We define the iterative encoding function  f as the function, which encodes the message m in the cover (see Equation 12).
f : C × M → S
⋀ i ∈ 1 . . . Z s i : = f c i , m
where: c i – the i -th iteration of the cover corresponds to the i -th encoding step,
s i – the steganogram created by function f by encoding message m in cover c i ,
m – the message to be encoded by function f in cover c i , Z – number of encoding steps.
We can regard an iterative steganogram as a vector of formed by sequentially appending values of f ' calculated for consecutive iterations (see Equation 13).
⋀ i ∈ 1 . . . Z f c i , m = c 1   , i = 1 f c i − 1 , m ⨁ c i ,   , i ∉ I f c i − 1 , m ⨁ f ' c i , m   , i ∈ I
where: c i – the i -th iteration of the cover corresponds to the i -th encoding step,
s i – the steganogram created by function f by encoding message m in cover c i ,
m – the message to be encoded by function f in cover c i , Ι – the set of coding indices,
Z – number of encoding steps.

2.7. Decoding Function

The iterative decoding function  f − 1 is defined as the function that assigns a decoded message m − 1 to a given steganogram s i (see Equation 14).
As a result of the operation of the decoding function, we obtain the decoded message m − 1 , which, like the original message m , is a bit vector from the set {0,1}.
The purpose of the decoding function is to recover the information encoded in the original message m . If the decoded message m − 1 is identical to the original message m , this goal is fully achieved. Otherwise, if m ≠ m − 1 , the ability to correctly read the information from the decoded message primarily depends on two factors: (1) the amount of preserved information in the message m − 1 , which can be determined, for instance, by the distance measure between the decoded and the original message; and (2) the properties of the steganographic technique used, including the degree of redundancy applied during the encoding of information in the message.
f − 1 : S → M
m − 1 : = f − 1 s i m − 1 ∈ M
where: s i – the steganogram created by encoding message m in cover c i ,
m − 1 – the message decoded by function f − 1 from steganogram s i .

3. The Proposed Method

3.1. IIF (Incremental Information Function)

The definition of the Incremental Information Function (IIF) is inspired by the concept of the Bit Error Rate (BER), which measures the ratio of incorrectly encoded bits to the total number of encoded bits in a message [17]. A lower BER indicates less information loss in the encoded message and higher robustness. In contrast, for the IIF, a higher function value signifies a greater amount of information decoded from the steganogram.
Let us first define the auxiliary function I I F o r i g , d e c (see Equation 15), which assigns to a given steganogram s i and message m the relative number of occurrences of decoded bits with the value d e c that had the value o r i g in the original message m .
I I F o r i g , d e c : S × M → R +
⋀ o r i g ∈ 0,1 d e c ∈ 0,1 ⋀ i ∈ 1 . . . Z I I F o r i g , d e c s i ,   m = ∑ k = 1 L 1 m k = o r i g ∧ f − 1 s i k = d e c / m o r i g ⋀ i ∈ 1 . . . Z ⋀ o r i g ∈ 0,1 ∑ d e c ∈ 0,1 I I F o r i g , d e c s i ,   m = 1
where: L – number of bits in the message m (message length),
o r i g – the value of bit in original message m ,
d e c – the value of bit in decoded message m − 1 ,
s i – the steganogram created by function f by encoding message m in cover c i ,
m k – the k -th bit of message m ,
m ( o r i g ) – number of o r i g -bites in message m ( m ( 0 ) or m ( 1 ) ) ,
f − 1 – the decoding function,
m – the message to be encoded by function f in cover c i , Z – number of decoding steps.
Let us define the I I F function (see Equation 16) as a weighted sum of the functions I I F 0,0 and I I F 1,1 , where the weights are proportional to the number of bits with values 0 and 1 in the original message m . This corresponds to the proportion of all correctly decoded bits in the message m − 1 .
I I F : S × M → 0,1
⋀ i ∈ 1 . . . Z I I F s i ,   m = I I F 0,0 s i ,   m ∙ m 0 L + I I F 1,1 s i ,   m ∙ m ( 1 ) L = ∑ k = 1 L 1 m k = f − 1 s i k / L
where: L – number of bits in the message m (message length),
Z – number of encoding steps,
f − 1 – the decoding function,
m – the message to be decoded by function f − 1 in steganogram s i ,
m ( 0 ) – number of zero-bites in message m ,
m ( 1 ) – number of one-bites in message m ,
m k – the k -th bit of message m ,
s i – the steganogram created by function f by encoding message m in cover c i .
In Equation 16, which defines the IIF function, it is also possible to incorporate different weights, particularly in scenarios where the steganographic method differentiates between zero and one bits in its processing. In a hypothetical case where zero bits are much less important than one bits, the weight for the function I I F 0,0 may not be proportional to m 0 but could instead be, for example, 1/10 of the weight for the function I I F 1,1 . In other specific steganographic techniques, it is also feasible to include the values of the functions I I F 0,1 and I I F 1,0 in the definition of the I I F function, serving as "penalties" for errors made in training the decoding algorithm (keeping in mind that I I F 0,1 = 1 − I I F 0,0 and I I F 1,0 = 1 − I I F 1,1 ) .

3.2. Properties of the IIF function

The behavior of the Incremental Information Function (IIF) depends on the specific implementation of the encoding and decoding functions. From the conducted experiments, it appears that this behavior depends more significantly on the form of the decoding function, which makes important assumptions from the initial iterations - for example, concerning the initial bit values of the decoded message. Subsequent iterations provide additional portions of information that incrementally build up the overall information, ultimately leading (or not) to the decoding of the entire message.
Based on the adopted definition and the nature of the IIF, the following key properties can be highlighted:
  • Value range: The values of the IIF function lie within the closed interval from 0 to 1, with values in the range of approximately 0.5 to 1 being particularly significant. The interpretation of these values is analogous to the Bit Error Rate (BER) indicator; however, in the case of the IIF function, lower values indicate a higher presence of noise, while higher values reflect a greater amount of encoded information.
  • Monotonicity: In the case of a properly functioning steganographic technique, including a well-defined encoding and decoding algorithm, the IIF function should not be decreasing, except for possible singular, isolated instances where the iterative steganogram might exhibit non-uniformity, leading to periodic disturbances in monotonicity. Successive iterations of the RAI decoding algorithm should lead to an increase in the amount of information contained in the decoded message, or at worst, not lead to its decrease.
  • Asymptotic behavior: The IIF function exhibits an asymptotic nature, tending to reach the maximum possible value of information that can be decoded from a given steganogram under specified noise conditions and given parameter values of the basic RAI encoding algorithm. In most cases, this value will not be equal to 1, which would correspond to the decoding of the full information encoded in the original steganogram. Nevertheless, it should constitute a sufficiently significant portion of the information to enable the recipient to correctly decode the message content.

3.3. Characteristic Values

We define the characteristic value c h I I F s as the smallest value of the I I F function for which the amount of information read from the steganogram s is sufficient to decode the message m . Depending on the steganographic technique used, particularly on the implementation of the decoding algorithm, the characteristic value c h I I F s ranges between 0.7 and 1.0. The level of c h I I F s is a feature that strongly characterizes a specific steganographic technique.
Conversely, we define the characteristic value c h I t e r a t i o n s as the first iteration for which the I I F function reaches the characteristic value c h I I F s (see Eq. 17).
c h I I F s = I I F ( s c h I t e r a t i o n s ,   m )
where: s – the steganogram created by encoding message m ,
m – the message to be encoded in the steganogram s ,
c h I I F s – minimal value of I I F enough to decode message from steganogram s ,
c h I t e r a t i o n s – the first iteration when I I F reach value of c h I I F s .
The characteristic value c h I I F s can be used as an indicator of the robustness of a given steganographic technique and the steganogram s itself. The lower the value of c h I I F s , the less decoded information is needed to uncover the hidden message, which means that greater distortions or transformations of the steganogram can be tolerated.
On the other hand, the characteristic value c h I t e r a t i o n s can be used as an indicator of the steganogram's capacity. The lower the value of c h I t e r a t i o n s , the faster the hidden message is decoded, requiring fewer iterations. In the case of iterative steganography, this implies higher capacity, meaning the ability to encode and decode more bits of information in a shorter time.

3.4. IIF Value Matrix

For each iteration of the decoding algorithm, a matrix can be constructed (see Table 1) that presents the results of the function I I F o r i g , d e c corresponding to that iteration.

4. Results

4.1. Research Experiments

As an example of iterative steganography, we have selected a video steganography method that encodes a message in successive frames of a video file, representing it as a black-and-white two-dimensional image. The details of this specific steganographic method, named RAI (Robust Adaptive Incremental), will be published in the author's subsequent works. For the purposes of this study, the following general assumptions of this steganographic technique are important:
  • The covers are video files treated as sequences of consecutive frames, each being an image.
  • The successive iterations in which the message is encoded occur every third frame of the video files.
  • The message is encoded using a steganographic method that operates in the spatial domain, altering the color values of the pixels in the video frames.
  • The decoding function assumes that from the first iteration, all bits of the message are initialized to zero. Subsequent iterations incrementally build information by setting one bits and clearing zero bits of the message.
  • The message is encoded in the form of a version 1 QR code with error correction level H. This allows encoding up to 72 bits of the message in a 21×21 module matrix with error correction up to 30% [18] enabling the use of automatic tools for reading the decoded message.

4.4. Example 1

For the study, we selected video steganogram No. 1 encoded using the RAI video steganography method, whose assumptions are presented above. As a result of the decoding function's operation, we calculated successive values of the functions I I F 0,0 , I I F 0,1 , I I F 1,0 , I I F 1,1 and I I F . The plots of these functions are shown in Figure 1.
Figure 1 highlights in blue vertical line the characteristic values c h I I F s and c h I t e r a t i o n s indicating the minimum threshold at which the information gain becomes sufficient to decode the hidden message accurately.

4.3. Example 2

For the next experiment, another video steganogram, No. 2, was selected. This steganogram was encoded using five different encoding levels corresponding to varying degrees of alteration to the original cover. The higher the encoding level, the greater the modification of the cover, resulting in higher information capacity - the amount of information that can be encoded in the steganogram - but also reduced imperceptibility, meaning a greater difference between the steganogram and the original cover.
Table 2 presents an example of the process of incremental information increase in the decoded message for the first ten iterations for video steganogram No. 2. For each decoding iteration, as the amount of information decoded from the steganogram increases, the QR code representing the message becomes clearer. This is associated with the increase in the value of the I I F function, which, after a certain number of decoding iterations, reaches the characteristic value c h I I F s , enabling the message to be decoded.
Figure 2, corresponding to Table 2, presents the plots of the I I F function for each encoding level of video steganogram No. 2.

4.2. Discussion

Figure 1 presents the plots of the functions I I F 0,0 , I I F 0,1 , I I F 1,0 , I I F 1,1 , along with the plot of the overall I I F function for video steganogram No. 1. Analyzing the plots, the following observations can be made:
  • for the example of video steganogram No. 1, the message was successfully decoded at the 18th iteration ( c h I t e r a t i o n s = 18) with an I I F value of c h I I F s =0.828,
  • the functions I I F 0,0 , I I F 1,1 and the overall I I F function asymptotically converge to a maximum value close to 1.0,
  • the values of I I F 0,1 and I I F 1,0 in this example do not carry any significant information, as they are complementary to the values of I I F 0,0 and I I F 1,1 ,
  • the initial value of I I F 0,0 is very high, which is due to a specific property of the RAI decoding function, which assumes that all information bits are zeroed at the start of the algorithm,
  • in general, the behavior of the I I F functions align with the theoretical assumptions of the model.
Table 2 illustrates examples of the incremental information increase process in decoded messages for the first ten iterations of example video steganogram No. 2, encoded at five different encoding levels. Each cell in the table contains a black-and-white image of a QR code representing the decoded message and the I I F value for the corresponding iteration of the decoded steganogram. It is clearly visible that the increase in the I I F value is positively correlated with the amount of information decoded from the steganogram - the higher the I I F value, the more information can be read from the steganogram. Cells highlighted in yellow indicate where the amount of decoded information allowed the automatic recognition of the QR code content, thus enabling the retrieval of the encoded message. The first yellow-highlighted cell for each encoding level determines the characteristic value c h I I F s .
Figure 2, corresponding to the results shown in Table 2, presents the plots of the I I F function across the full range of decoded iterations for video steganogram No. 2, encoded at five different encoding levels. The plots of the I I F functions display points where, for each steganogram, the I I F functions reach the characteristic value c h I I F s , along with an indication of the characteristic value c h I t e r a t i o n s at which c h I I F s is achieved. The experimental results show that, within the range of studied iterations, the information was successfully decoded for the three highest encoding levels of video steganogram No. 2, while for the two lowest encoding levels, the I I F values remained at noise levels.
Based on the experiments conducted, two characteristic regions of I I F values can be identified: the noise region and the decoding region. The noise region corresponds to I I F values in the range of 0.5-0.7, while the decoding region corresponds to I I F values in the range of 0.7-1.0.
As previously described properties of the I I F function suggest, the plots clearly show that the I I F function (in cases where hidden information can be decoded from the steganogram) is increasing and asymptotically approaches the maximum value characteristic of the specific steganogram. The rate of increase in function values in our examples is directly correlated with the encoding level, as higher encoding levels result in a greater information increment per iteration. If the information gain in subsequent iterations does not exceed the information loss associated with transitioning between iterations (for example, due to inter-frame compression mechanisms used by video codecs), the I I F function will not increase, and its values will remain in the noise region.

5. Conclusions

This study provides a detailed characterization and modeling framework for a class of iterative steganographic techniques. A novel method is introduced for quantifying and evaluating the efficacy of these techniques, leveraging the Incremental Information Function (IIF). Through a case study utilizing a selected video steganography method, the research demonstrates the application of the IIF in analyzing the properties of iterative steganographic techniques, including the quantification of robustness and capacity by determining characteristic IIF values. This approach offers a fresh perspective on the information dynamics within iterative steganographic systems, with the potential to advance both the implementation of steganography and the effectiveness of steganalysis.
The study successfully accomplishes its primary objective by developing a formal method for quantifying and analyzing information increments in iterative steganographic systems. It introduces an effective framework for assessing the efficiency and robustness of these techniques, supported by practical examples that illustrate its application to elucidate the properties of the studied steganographic methods. This advancement lays the foundation for further research and development in the field of steganography, offering a systematic and robust analytical tool to evaluate and enhance the performance of iterative techniques.

5.1. Theoretical Contributions

In this work, we have proposed:
  • a formal mathematical model for characterizing a class of iterative steganographic methods,
  • a novel quantitative method for evaluating the efficacy of iterative steganographic techniques, based on the proposed Incremental Information Function (IIF),
  • the application of characteristic IIF values to quantify robustness and capacity metrics in iterative steganographic systems.
These contributions provide an effective framework for analyzing and optimizing iterative steganographic techniques, enhancing the theoretical foundations of this field and facilitating more precise evaluation of steganographic performance.

5.2. Practical Implications

The findings presented in this study demonstrate that the proposed method, based on the Incremental Information Function (IIF) and the analysis of its characteristic values, constitutes an effective analytical tool for investigating the properties of iterative steganographic techniques. This approach enables quantitative assessment of key performance metrics, including the robustness and capacity of iterative steganograms. The IIF-based method provides a systematic framework for evaluating and comparing different iterative steganographic algorithms, potentially facilitating the optimization of steganographic systems and enhancing the efficacy of steganalysis techniques.

5.3. Future Research

We propose the following avenues for further investigation:
  • extension of IIF application: explore the utilization of the Incremental Information Function (IIF) in diverse iterative steganography methods beyond video steganography, such as network steganography protocols,
  • universal IIF characteristics: examine the potential existence of universal characteristic values (chIIF) of the IIF that may describe and differentiate various iterative steganography techniques, including multiple video steganography methods,
  • IIF in steganalysis: investigate the potential applications of IIF properties in steganalysis processes for the detection and analysis of steganographic content, potentially enhancing the efficacy of current steganalysis techniques.
These proposed research directions aim to expand the applicability and deepen the understanding of the IIF methodology in the broader context of steganography and steganalysis.

Author Contributions

The results of the work were obtained mainly by the first author under the scientific supervision of the second one.

Funding

The work was partially financed by the Military University of Technology in Warsaw, Poland as part of the project No. UGB 22-701.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Kahn, D. The Codebreakers: The Story of Secret Writing. NYC, USA : Macmillan, 1967, ISBN 978-0025604605.
  2. Shannon, C. A Mathematical Theory of Communication. NYC, USA : Bell System Technical Journal 1948, 27, 379–423, ISSN 0005. [Google Scholar]
  3. Johnson, N. and Jajodia, S. Exploring Steganography: Seeing the Unseen. Los Alamitos, USA : IEEE Computer 1998, 31, 26–34, ISSN 0018. [Google Scholar]
  4. Johnson, N. , Duric, Z. and Jajodia, S. Information Hiding: Steganography and Watermarking - Attacks and Countermeasures. Boston, USA : Springer, 2001, ISBN 978-0-7923-7204-2, DOI 10.1007/978-1-4615-4375-6, 2001.
  5. Petitcolas, F. , Anderson, R. and Kuhn, M. Information hiding - a survey. NYC, USA : Proceedings of the IEEE 1999, 87, 1062–1078, ISSN 0018. [Google Scholar]
  6. Fridrich, J. Steganography in Digital Media: Principles, Algorithms, and Applications. Cambridge, UK : Cambridge University Press, 2009, DOI 10.1017/CBO9781139192903.014.
  7. Sencar, H. , Ramkumar, M. and Akansu, A. Data Hiding Fundamentals and Applications. Burlington, USA : Elsevier Academic Press, 2004, ISBN 9780120471447, DOI 10.1016/B978-0-12-047144-7.X5000-5.
  8. Huynh-Thu, Q. and Ghanbari, M. Scope of validity of PSNR in image/video quality assessment. London, UK : Electronics Letters 2008, 44, 800–801, ISSN 0013. [Google Scholar]
  9. Wang, Z. , et al. Image Quality Assessment: From Error Visibility to Structural Similarity. NYC, USA : IEEE Transactions on Image Processing 2004, 13, 600–612, ISSN 1057. [Google Scholar]
  10. Cox, I. , Miller, M. and Kalker, T. Digital Watermarking and Steganography. Burlington, USA : Morgan Kaufmann Publishers, 2007, ISBN 0123725852.
  11. Anderson, R. and Petitcolas, F. On the limits of steganography. NYC, USA : IEEE Journal on Selected Areas in Communications 1998, 16, 474–481, ISSN 0733. [Google Scholar]
  12. Kadhim, I. , et al. Comprehensive survey of image steganography: Techniques, Evaluations, and trends in future research. Amsterdam, Netherlands : Neurocomputing 2019, 335, 299–326, ISSN 0925. [Google Scholar]
  13. Chan, C. and Cheng, L. Hiding data in images by simple LSB substitution. Amsterdam, Netherlands : Elsevier, Pattern Recognition 2004, 37, 469–474, ISSN 0031. [Google Scholar]
  14. Bennett, K. Linguistic Steganography: Survey, Analysis, and Robustness Concerns for Hiding Information in Text. West Lafayette, USA : CERIAS Tech Report 2004-13, Purdue University, Available online:. Available online: https://www.cerias.purdue.edu/assets/pdf/bibtex_archive/2004-13.pdf (accessed on 16 November 2024).
  15. Kunhoth, J. , et al. Video steganography: recent advances and challenges. Berlin, Germany : Springer - Multimedia Tools and Applications 2023, 82, 11817–11868, ISSN 1380. [Google Scholar]
  16. Lubacz, J. , Mazurczyk, W. and Szczypiorski, K. Principles and Overview of Network Steganography. NYC, USA : IEEE Communications Magazine. 2012; 52. [Google Scholar] [CrossRef]
  17. Katzenbeisser, S. and Petitcolas, F. Information Hiding Techniques for Steganography and Digital Watermarking. Norwood, USA : Artech House, 2000, ISBN 978-1-58053-035-4.
  18. DensoWave. QR code. Denso Wave, Available online:. Available online: https://www.qrcode.com (accessed on 16 November 2024).
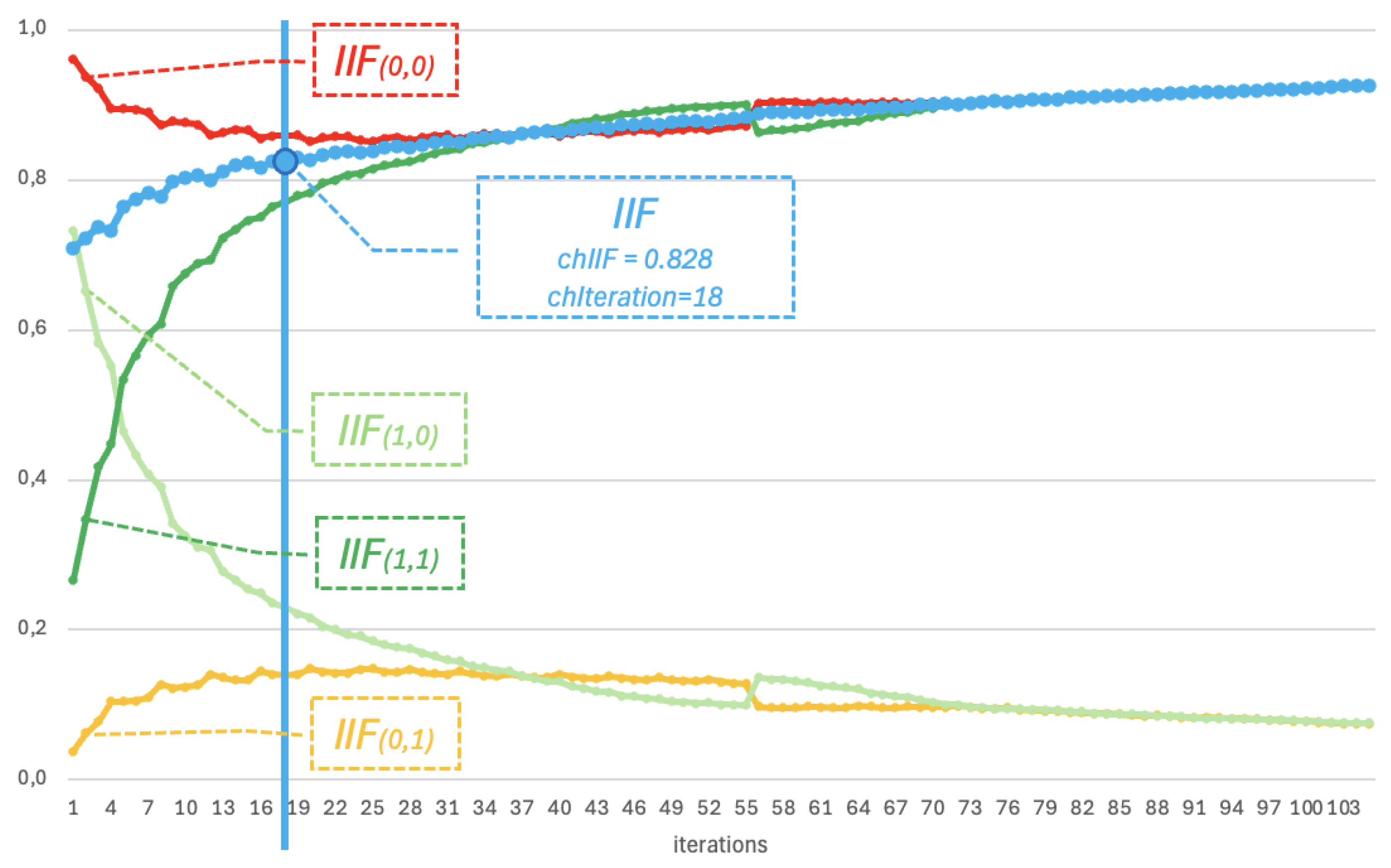
Preprints 140000 g001
Figure 1. Plots of the functions IIF(0,0), IIF(1,1), IIF(0,1), IIF(1,0) and IIF for video steganogram No. 1.
Figure 1. Plots of the functions IIF(0,0), IIF(1,1), IIF(0,1), IIF(1,0) and IIF for video steganogram No. 1.
Preprints 140000 g001
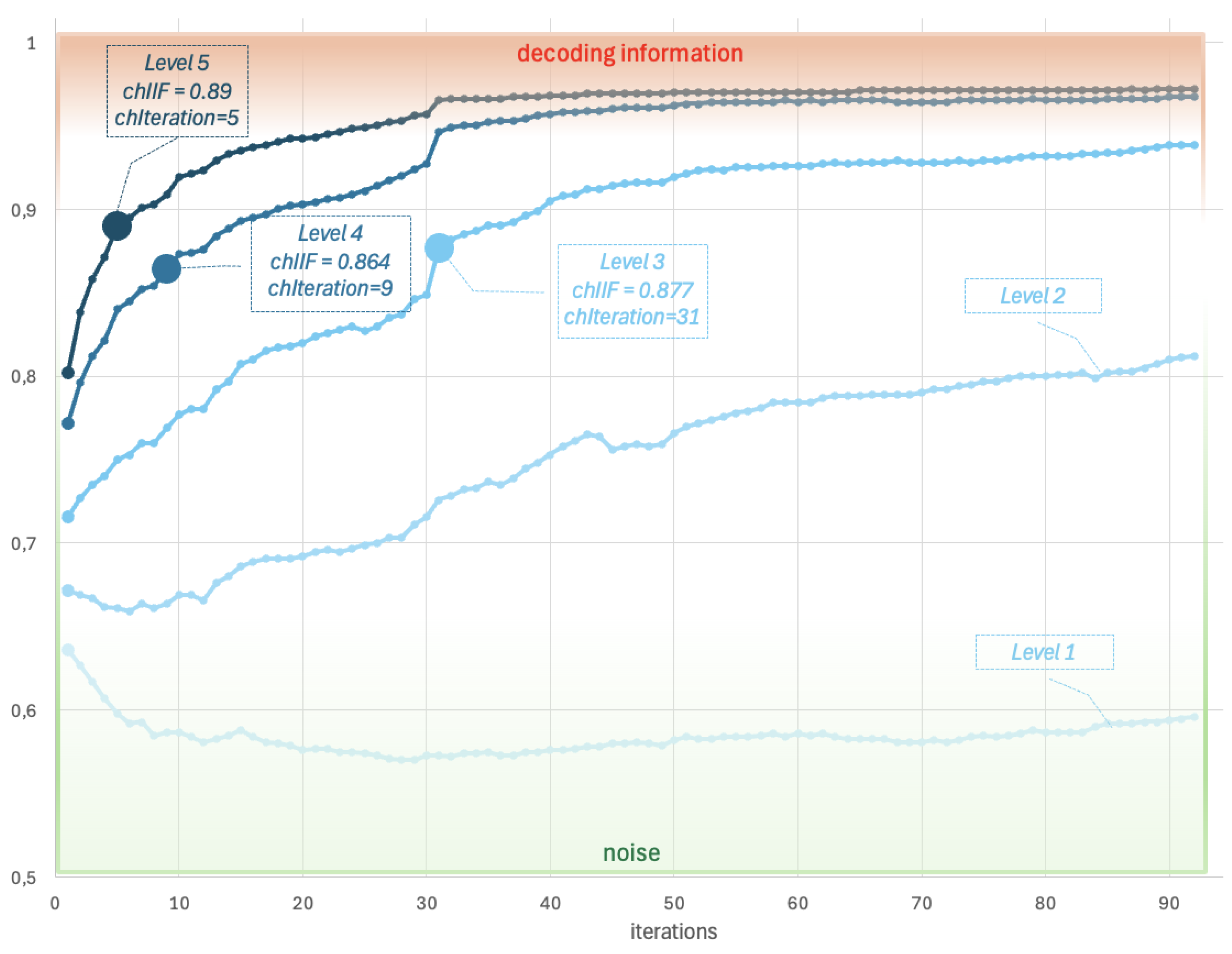
Preprints 140000 g002
Figure 2. Plots of the IIF function for video steganogram no. 2 encoded with 5 levels of encoding.
Figure 2. Plots of the IIF function for video steganogram no. 2 encoded with 5 levels of encoding.
Preprints 140000 g002
Table 1. Values of I I F o r i g , d e c for i -th iteration of decoding algorithm.
Table 1. Values of I I F o r i g , d e c for i -th iteration of decoding algorithm.
original bit values in m
0 1
decoded bit values in m − 1 0 I I F 0,0 I I F 1,0
1 I I F 0,1 I I F 1,1
Table 2. Incremental increase of information in the initial iterations for video steganogram No. 2.
Table 2. Incremental increase of information in the initial iterations for video steganogram No. 2.
iteration Level 1 Level 2 Level 3 Level 4 Level 5
1 Preprints 140000 i001 Preprints 140000 i002 Preprints 140000 i003 Preprints 140000 i004 Preprints 140000 i005
IIF=0.636 IIF=0.672 IIF=0.716 IIF=0.772 IIF=0.802
2 Preprints 140000 i006 Preprints 140000 i007 Preprints 140000 i008 Preprints 140000 i009 Preprints 140000 i010
IIF=0.627 IIF=0.669 IIF=0.727 IIF=0.796 IIF=0.838
3 Preprints 140000 i011 Preprints 140000 i012 Preprints 140000 i013 Preprints 140000 i014 Preprints 140000 i015
IIF=0.617 IIF=0.667 IIF=0.735 IIF=0.812 IIF=0.858
4 Preprints 140000 i016 Preprints 140000 i017 Preprints 140000 i018 Preprints 140000 i019 Preprints 140000 i020
IIF=0.607 IIF=0.662 IIF=0.740 IIF=0.821 IIF=0.871
5 Preprints 140000 i021 Preprints 140000 i022 Preprints 140000 i023 Preprints 140000 i024 Preprints 140000 i025
IIF=0.598 IIF=0.661 IIF=0.750 IIF=0.840 IIF=0.890
6 Preprints 140000 i026 Preprints 140000 i027 Preprints 140000 i028 Preprints 140000 i029 Preprints 140000 i030
IIF=0.592 IIF=0.659 IIF=0.753 IIF=0.845 IIF=0.895
7 Preprints 140000 i031 Preprints 140000 i032 Preprints 140000 i033 Preprints 140000 i034 Preprints 140000 i035
IIF=0.593 IIF=0.664 IIF=0.760 IIF=0.852 IIF=0.901
8 Preprints 140000 i036 Preprints 140000 i037 Preprints 140000 i038 Preprints 140000 i039 Preprints 140000 i040
IIF=0.585 IIF=0.661 IIF=0.760 IIF=0.854 IIF=0.903
9 Preprints 140000 i041 Preprints 140000 i042 Preprints 140000 i043 Preprints 140000 i044 Preprints 140000 i045
IIF=0.587 IIF=0.664 IIF=0.769 IIF=0.864 IIF=0.909
10 Preprints 140000 i046 Preprints 140000 i047 Preprints 140000 i048 Preprints 140000 i049 Preprints 140000 i050
IIF=0.587 IIF=0.669 IIF=0.777 IIF=0.873 IIF=0.919
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.
Copyright: This open access article is published under a Creative Commons CC BY 4.0 license, which permit the free download, distribution, and reuse, provided that the author and preprint are cited in any reuse.

Downloads

29

Views

50

Comments

0

Subscription

Notify me about updates to this article or when a peer-reviewed version is published.

Email

Prerpints.org logo

Preprints.org is a free preprint server supported by MDPI in Basel, Switzerland.

facebook logotwitter logolinkedin logo
MDPI Initiatives
Important Links
Subscribe

Disclaimer

Terms of Use

Privacy Policy

© 2025 MDPI (Basel, Switzerland) unless otherwise stated